Analysis of Field Oriented Control of Permanent Magnet Synchronous Motor for a Valveless Pump-Controlled Actuator †
Abstract
:1. Introduction
2. System Description
2.1. Pump-Controlled Actuator
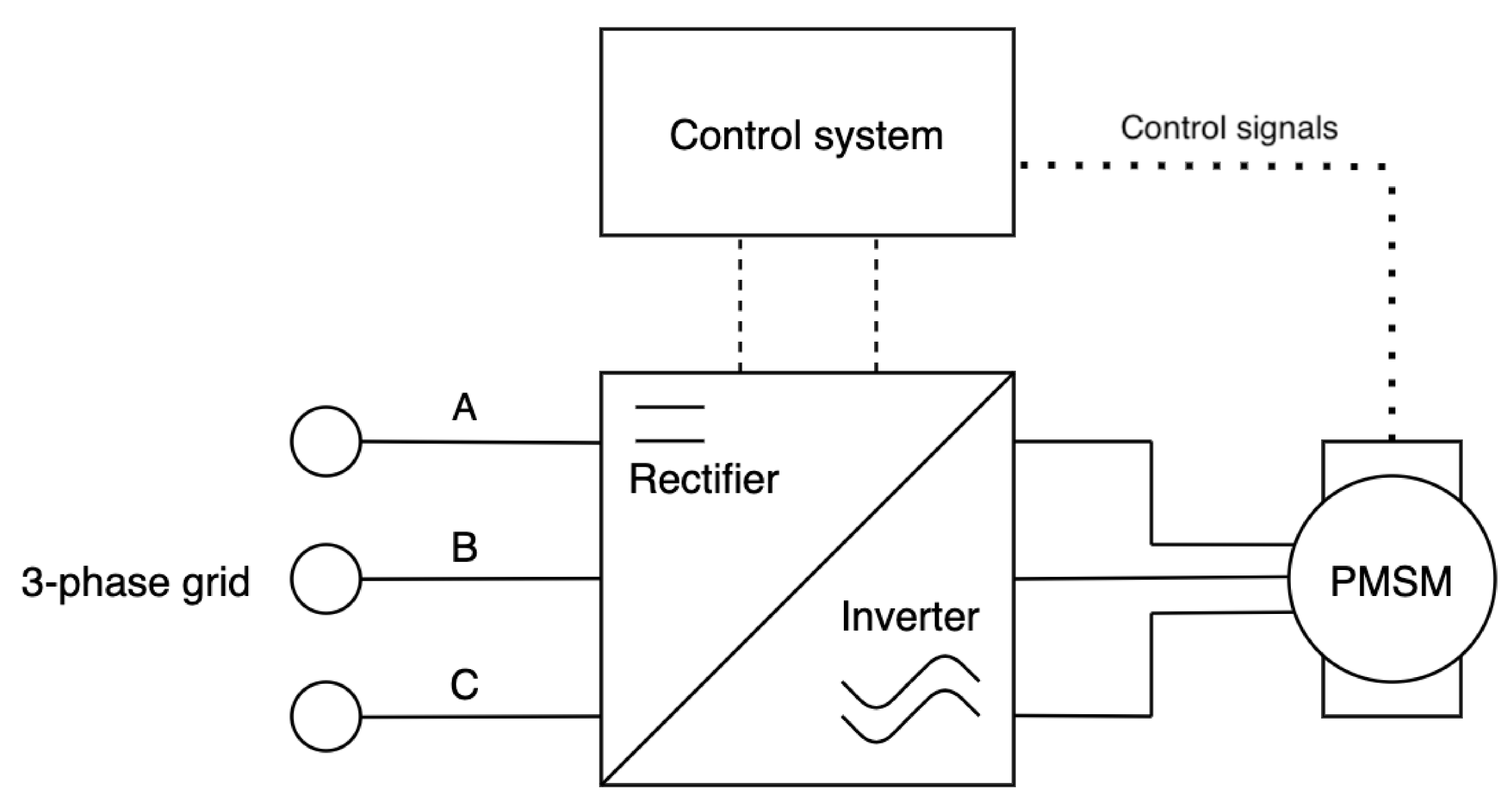
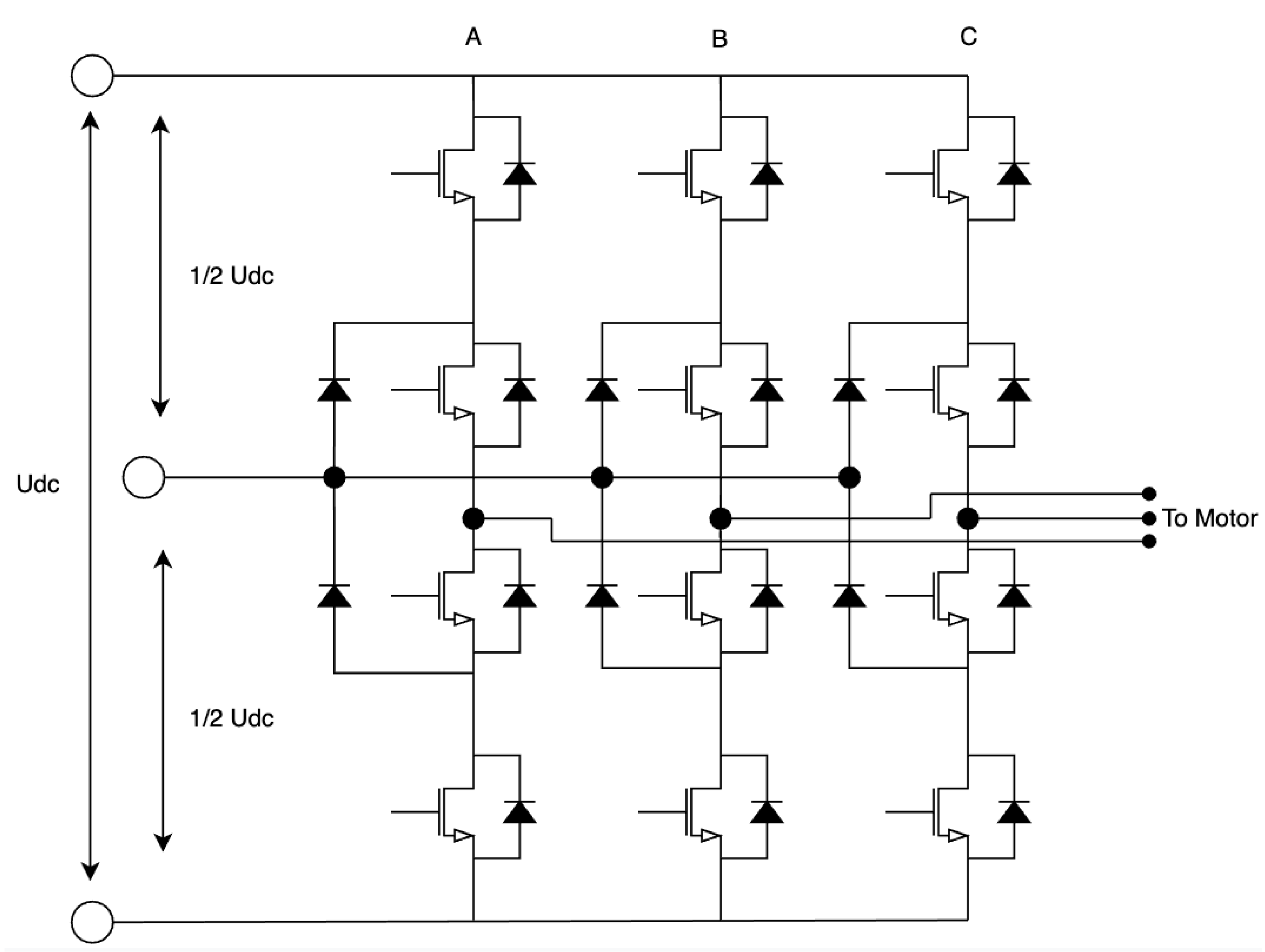
2.2. Electric Drive
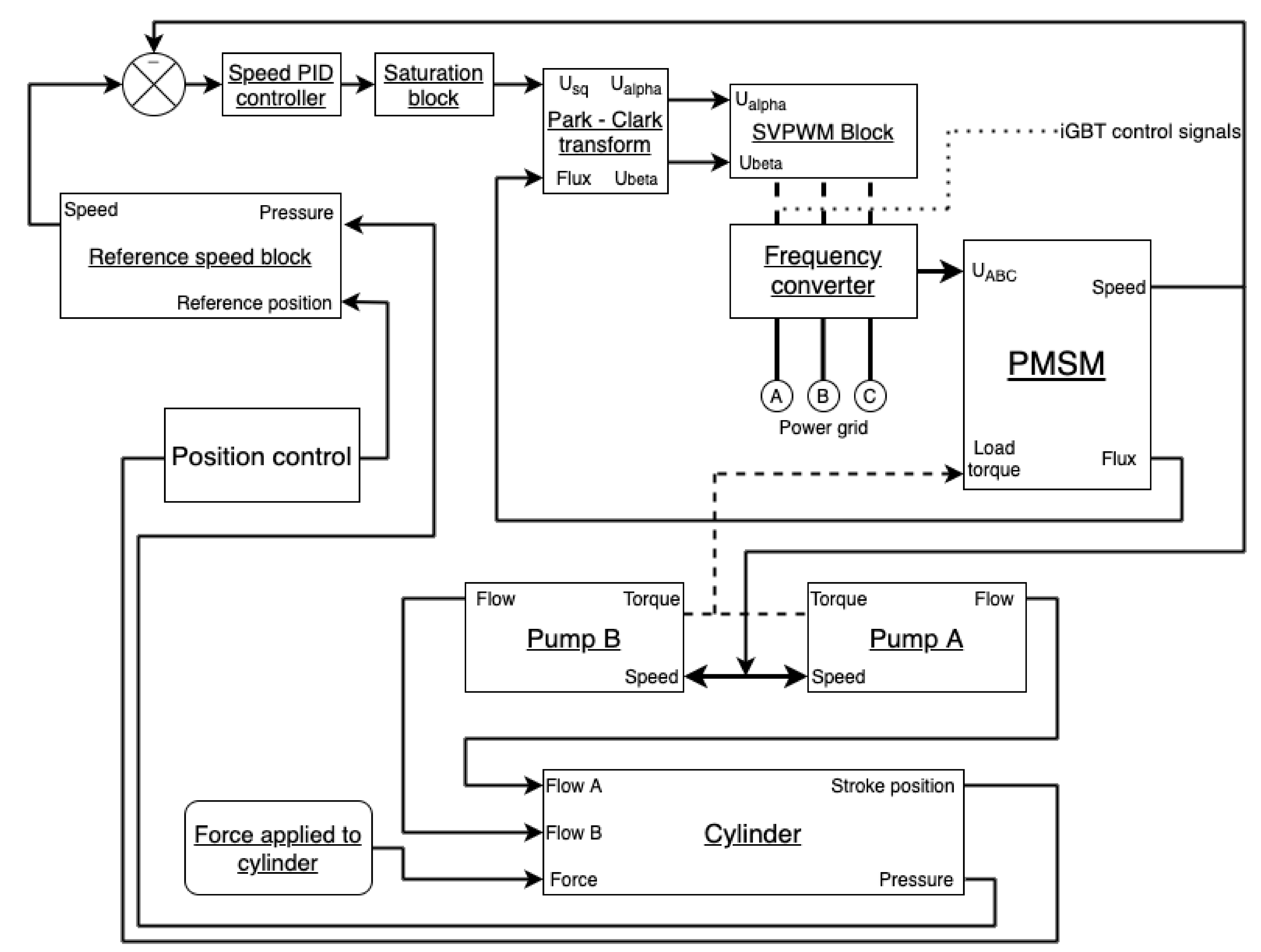
2.3. Control System Description
3. Modelling
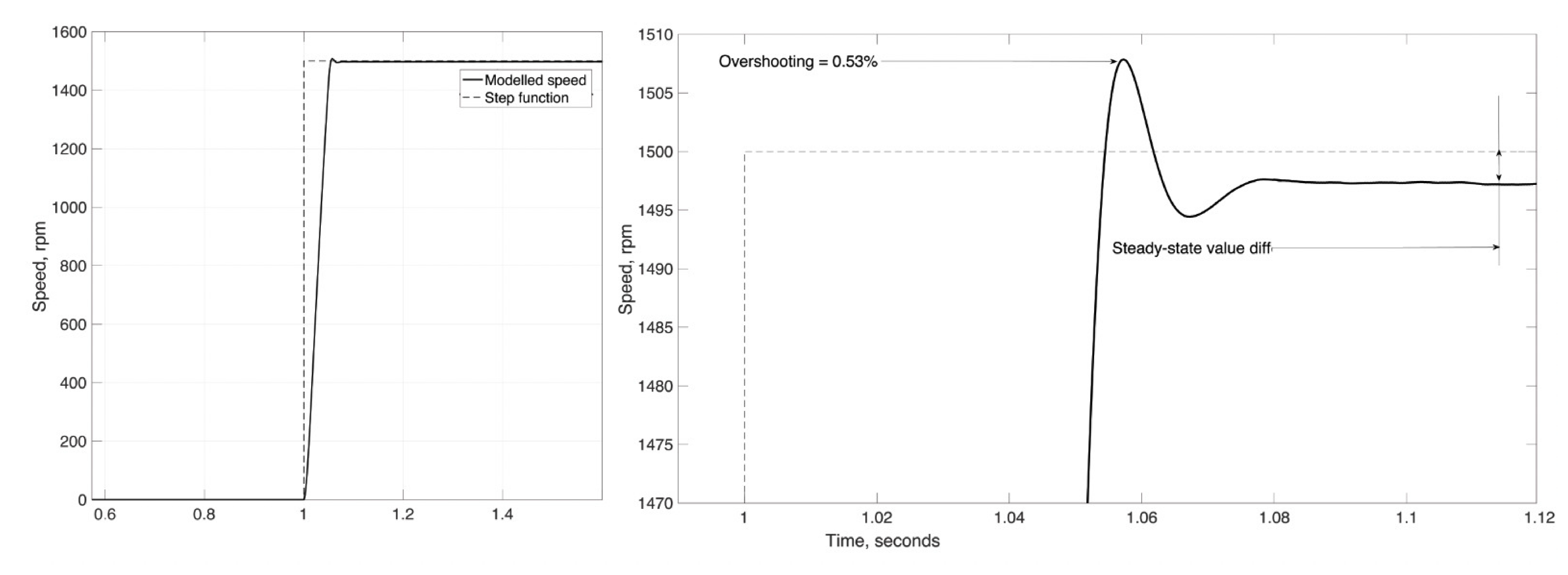
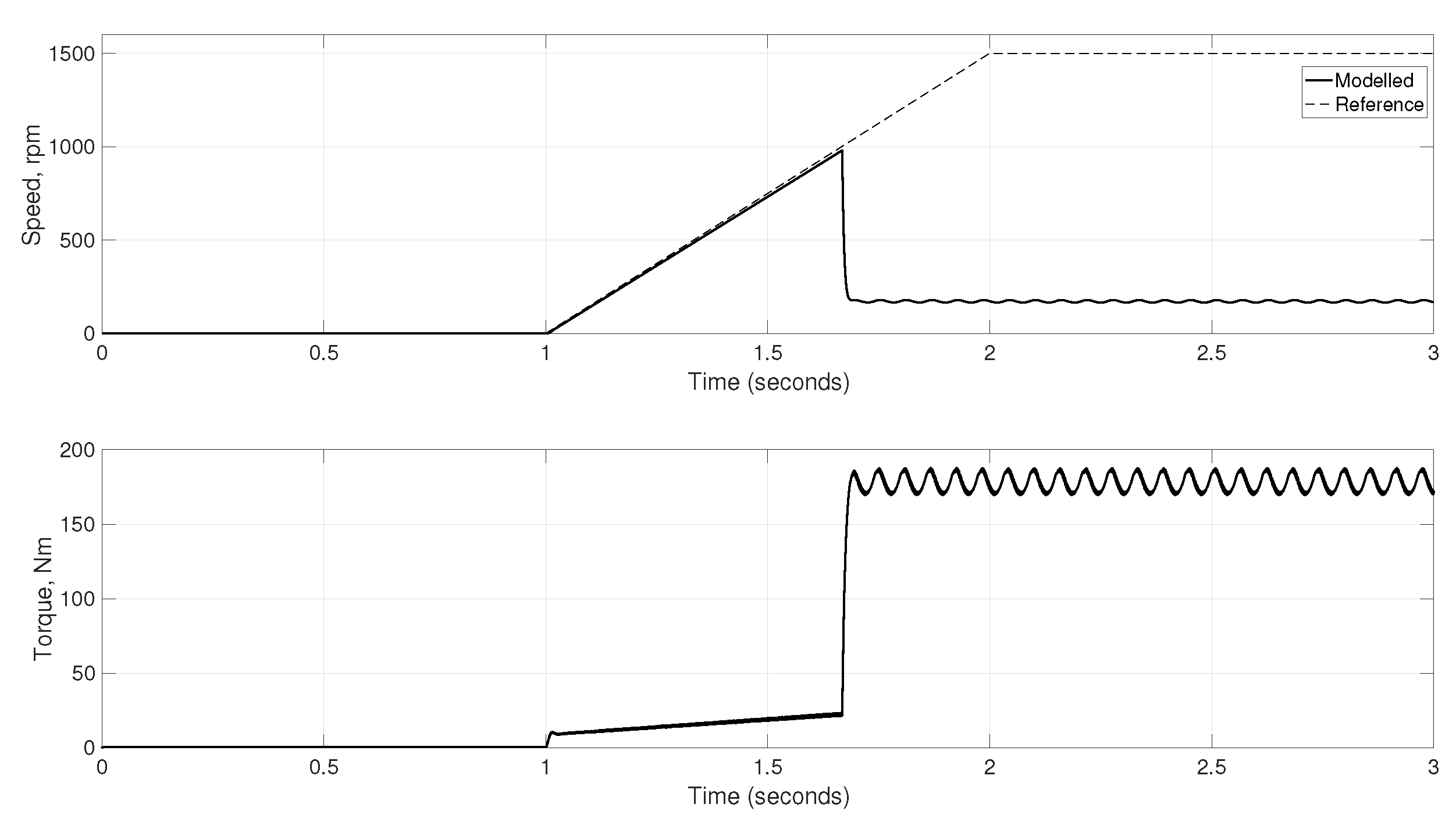
3.1. Step Function Response
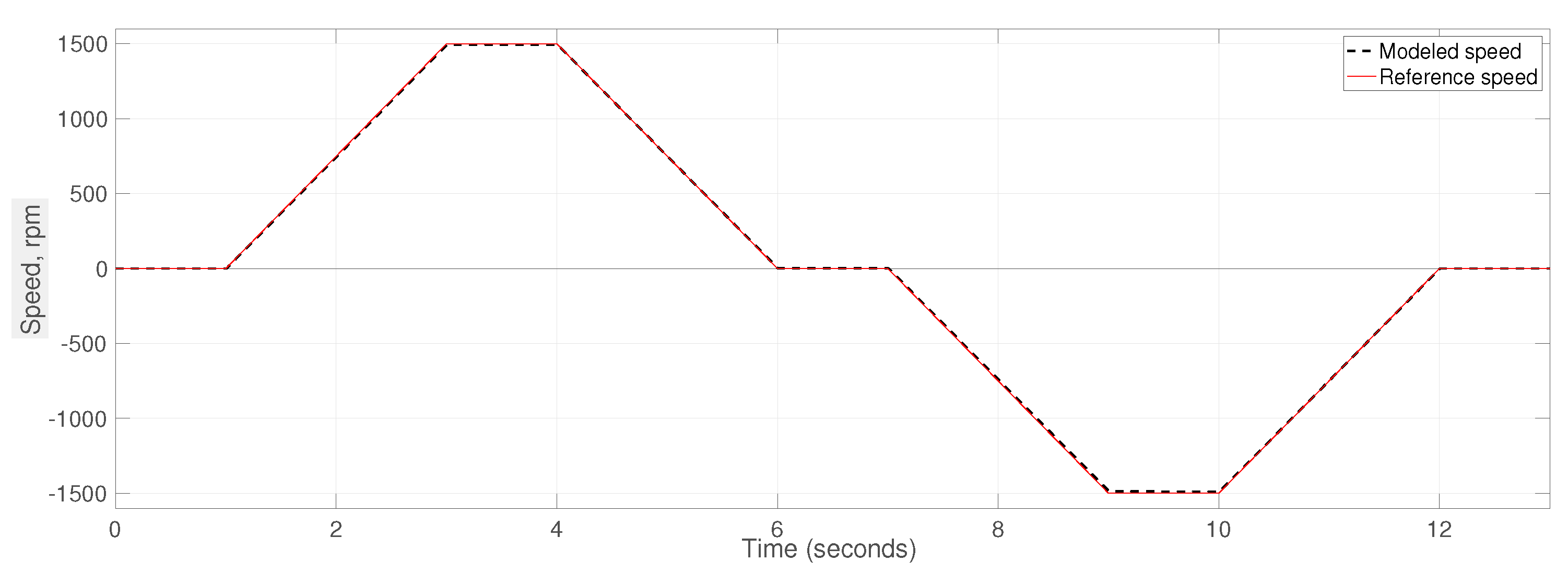
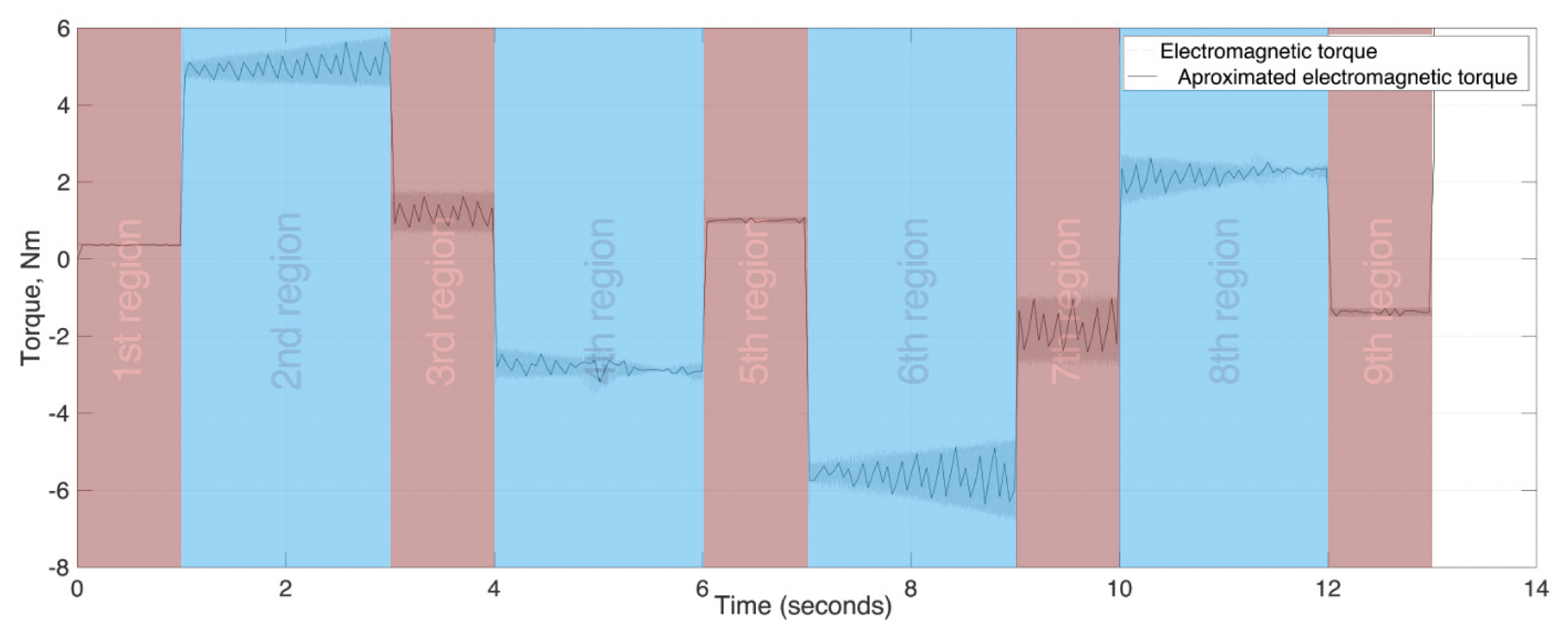
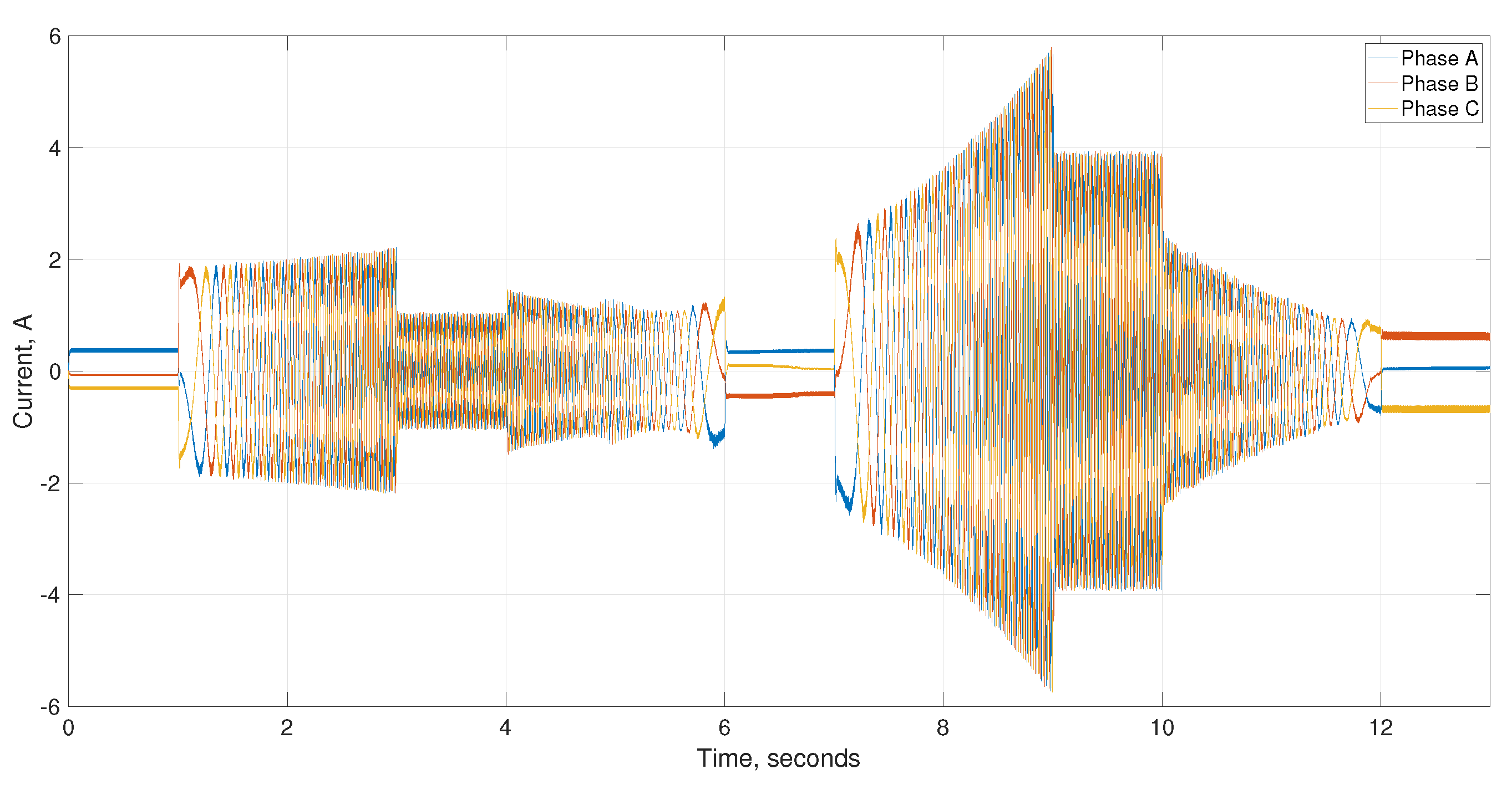
3.2. Operation in the Normal Conditions
- In the 1st region (from 0 s to 1 s) torque is around zero because EM has no load and zero reference speed, but currents and voltages are applied to EM to maintain the required position.
- 2nd region is from 1 s to 3 s. Increasing reference speed launches electromagnetic processes in the motor and rotation of the shaft gets an additional load from the hydraulic part. Maximal positive torque is achieved.
- 3rd region is from 3 s to 4 s. At this moment acceleration is equal to zero and the motor has a constant speed, however, the hydraulic load is still applied.
- 4th region is from 4 s to 6 s. This period has deceleration of the motor and the same hydraulic load. Torques from hydraulic and electric parts have different signs and the resulting electromagnetic torque is the smallest non-zero one.
- The 5th and 9th regions are identical to the 1st.
- 6th, 7th, and 8th correspond to 2nd, 3rd, and 4th regions but with a negative sign. As a result, the level of torque fluctuations is about 10% in the positive part (motoring mode) and 13.5% in the negative part (generating mode). The negative part demonstrates a higher level of fluctuations, because of applied to the cylinder mass, which creates an additional lowering force disturbing the system.
3.3. Operation in Failure Mode
4. Discussion
5. Conclusions
Author Contributions
Funding
Abbreviations
| EM | Electric Motor |
| PMSM | Permanent Magnet Synchronous Machine |
| FOC | Field Oriented Control |
| PWM | Pulse Width Modulation |
| DDH | Direct-Driven Hydraulics |
| AC | Alternative Current |
| DC | Direct Current |
| SRM | Switched-Reluctance Motor |
| THD | Total Harmonic Distortion |
| MCU | Microcontroller Unit |
References
- Manring, N.D.; Fales, R.C. Hydraulic Control Systems; John Wiley & Sons: Hoboken, NJ, USA, 2019. [Google Scholar]
- Love, L.J. Estimating the Impact (Energy, Emissions and Economics) of the US Fluid Power Industry; ech. Rep. ORNL/TM-2011/14,1061537; Oak Ridge National Laboratory (ORNL): Oak Ridge, TN, USA, 2012. [Google Scholar]
- Minav, T.; Sainio, P.; Pietola, M. Direct-driven hydraulic drive without conventional oil tank. In Proceedings of the ASME/BATH 2014 Symposium on Fluid Power & Motion Control, Bath, UK, 10–12 September 2014. [Google Scholar]
- Minav, T.; Laurila, L.; Pyrhönen, J. Analysis of electro-hydraulic lifting system’s energy efficiency with direct electric drive pump control. Autom. Constr. 2013, 30, 144–150. [Google Scholar] [CrossRef]
- Guo, Q.; Zhang, C.M.; Li, L.; Zhang, J.; Wang, M. Maximum Efficiency Control of Permanent-Magnet Synchronous Machines for Electric Vehicles. Energy Procedia 2017, 105, 2267–2272. [Google Scholar] [CrossRef]
- Chandekar, V.S.; Chaudhari, M.A.; Chakravarthi, V.K. A Low cost three phase induction motor drive operated on single phase input source. In Proceedings of the International Conference on Smart Electric Drives & Power System, Nagpur, India, 12–13 June 2018. [Google Scholar]
- Jezernik, K.; Horvat, R. High performance control of PMSM. In Proceedings of the First Symposium on Sensorless Control for Electrical Drives, Padova, Italy, 9–10 July 2010. [Google Scholar]
- Goldenstein, C. Advanced Combustion Engines; Stanford University: Stanford, CA, USA, 2011. [Google Scholar]
- Chunmei, Z.; Heping, L.; Shujin, C.; Fangjun, W. Application of neural networks for permanent magnet synchronous motor direct torque control. J. Syst. Eng. Electron. 2008, 19, 555–561. [Google Scholar] [CrossRef]
- Holmes, D.G.; Lipo, T.A. Pulse Width Modulation for Power Converters. Principles and Practice; John Wiley & Sons: Hoboken, NJ, USA, 2003. [Google Scholar]
- Sokolovskiy, G.G. AC Drives with Frequency Regulation; Moscow Academia: Moscow, Russia, 2006. [Google Scholar]
- Filatov, D.; Minav, T.; Heikkinen, J. Adaptive control for Direct-Driven Hydraulic Drive. In Proceedings of the 11th International Fluid Power Conference, Aachen, Germany, 19–21 March 2018. [Google Scholar]
- Zakharov, V.; Minav, T. Analysis of Frequency Adjustable Control of Permanent Magnet Synchronous Motor for Pump-Controlled Actuators. In Proceedings of the 2020 IEEE Global Fluid Power Society PhD Symposium, online, 19–21 October 2020. [Google Scholar]
- Koitto, T.; Kauranne, H.; Calonius, O.; Minav, T.; Pietola, M. Experimental Investigation of a Directly Driven Hydraulic Unit in an Industrial Stationary Application. In Proceedings of the 11th International Fluid Power Conference, 19–21 March 2018. [Google Scholar]
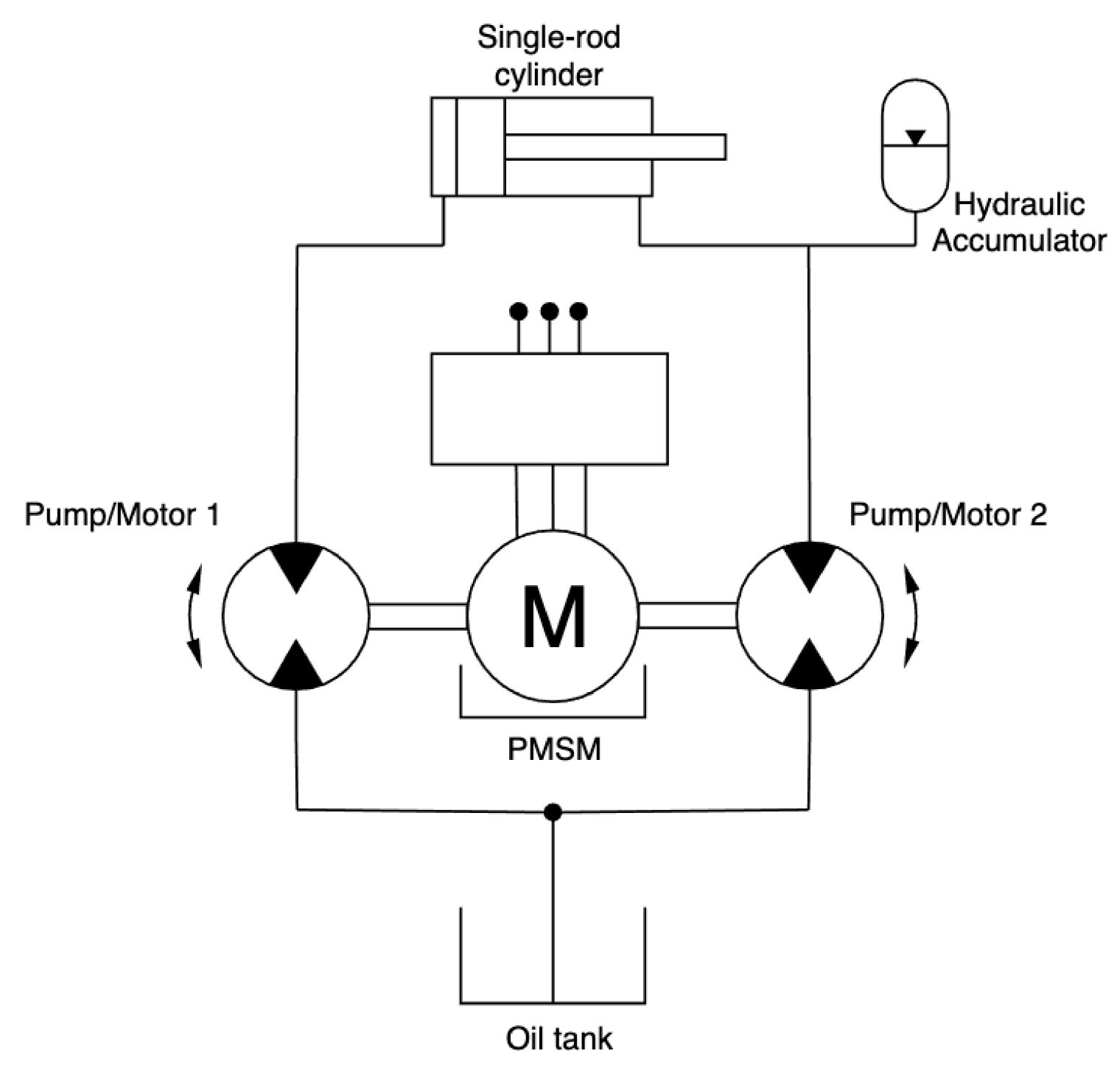





| Hydraulic Cylinder | Hydraulic Pump/Motor 1 | Hydraulic Pump/Motor 2 | Hydraulic Accumulator |
|---|---|---|---|
| MIRO C-10-60/30x400 | XV-2M/14 | XV-2M/22 | HAD0.7-250-1X/80G04A |
| Max pressure 190 bar | Max pressure 250 bar | Max pressure 200 bar | Max pressure 350 bar |
| Area A 0.0028 mm | Displacement 14.4 cm/rev | Displacement 22.8 cm/rev | Precharge pressure 10 bar |
| Area B 0.0021 mm | Vol. efficiency 97.22 % | Vol. efficiency 96.49 % | Volume 0.7 l |
| Stroke length 0.4 m | Max. rot. speed 3500 rpm | Max. rot. speed 3000 rpm | Type: Hydro-pneumatic |
| Rated/Peak Torque, [Nm] | Inertia, [kgcm] | Rated/Max Speed, [rpm] | Rated Current, [A] | Voltage, [V AC] |
|---|---|---|---|---|
| 9.4/37.6 | 9.0 | 3000/4800 | 5.9 | 380/480 |
| Rising Time, [s] | Transient Process Time, [s] | Overshooting, [%] | Steady-State Value Difference, [%] |
|---|---|---|---|
| 0.051 | 0.13 | 0.53 | 0.16 |
| Sim. Speed Difference, [%] | Torque Fluct., [%] | Flow Ampl. Pump A/Pump B, [m/s] | Current Ampl., [A] |
|---|---|---|---|
| 0.33 | 11.75 average | 5.26/3.75 10 | 5.8 |
| Current Amplitude, [A] | Rising Time, [s] | Steady-State Torque, [Nm] | Steady-State Speed, [rpm] |
|---|---|---|---|
| 75.52 | 0.097 | 180.1 | 210 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zakharov, V.; Minav, T. Analysis of Field Oriented Control of Permanent Magnet Synchronous Motor for a Valveless Pump-Controlled Actuator. Proceedings 2020, 64, 19. https://doi.org/10.3390/IeCAT2020-08491
Zakharov V, Minav T. Analysis of Field Oriented Control of Permanent Magnet Synchronous Motor for a Valveless Pump-Controlled Actuator. Proceedings. 2020; 64(1):19. https://doi.org/10.3390/IeCAT2020-08491
Chicago/Turabian StyleZakharov, Viacheslav, and Tatiana Minav. 2020. "Analysis of Field Oriented Control of Permanent Magnet Synchronous Motor for a Valveless Pump-Controlled Actuator" Proceedings 64, no. 1: 19. https://doi.org/10.3390/IeCAT2020-08491
APA StyleZakharov, V., & Minav, T. (2020). Analysis of Field Oriented Control of Permanent Magnet Synchronous Motor for a Valveless Pump-Controlled Actuator. Proceedings, 64(1), 19. https://doi.org/10.3390/IeCAT2020-08491






