Exploration of Center of Gravity, Moment of Inertia, and Launch Direction for Putters with Ball Speed Normalizing Face Properties †
Abstract
1. Introduction
2. Materials and Methods
2.1. Impact Model
2.2. Discretionary Mass Placement
2.3. Physical Testing
3. Results
4. Discussion
5. Conclusions
Funding
Conflicts of Interest
References
- Pelz, D.; Mastroni, N. Putt Like the Pros: Dave Pelz’s Scientific Way to Improving Your Stroke, Reading Greens, and Lowering Your Score; Harper & Row: New York, NY, USA, 1989. [Google Scholar]
- Maltby, R. The Complete Book of Golf Club Fitting & Performance; The GolfWorks: Newark, OH, USA, 2011. [Google Scholar]
- Cochran, A.; Stobbs, J. The Search for the Perfect Swing; Lippincott: Philadelphia, PA, USA, 1968. [Google Scholar]
- Daish, C.B. The Physics of Ball Games; The English Universities Press Ltd.: London, UK, 1972. [Google Scholar]
- Lindsay, N. Topspin in putters—A study of vertical gear-effect and its dependence on shaft coupling. Sports Eng. 2003, 6, 81–93. [Google Scholar] [CrossRef]
- Brouillette, M. Putter features that influence the rolling motion of a golf ball. Procedia Eng. 2010, 2, 3223–3229. [Google Scholar] [CrossRef][Green Version]
- Emerson, N.J.; Morris, T.; Potts, J.R. A Novel Putter Design to Minimise Range Variability in Golf Putts. Proceedings 2018, 2, 242. [Google Scholar]
- Lambeth, J.; Brekke, D.; Brunski, J. Variable Face Milling to Normalize Putter Ball Speed and Maximize Forgiveness. Proceedings 2018, 2, 248. [Google Scholar]
- Werner, F.D.; Greig, R.C. How Golf Clubs Really Work and How to Optimize Their Designs; Origin Inc: Jackson, MI, USA, 2000. [Google Scholar]
- Penner, A. The physics of golf: The convex face of a driver. Am. J. Phys. 2001, 69, 1073–1081. [Google Scholar] [CrossRef]
- Tanaka, K.; Oodaira, H.; Teranishi, Y.; Sato, F.; Ujihashi, S.; Estivalet, M. Finite-Element Analysis of the Collision and Bounce between a Golf Ball and Simplified Clubs. In The Engineering of Sport 7; Springer: Paris, France, 2009; pp. 653–662. [Google Scholar]
- McNally, W.; McPhee, J.; Henrikson, E. The Golf Shaft’s Influence on Clubhead-Ball Impact Dynamics. Proceedings 2018, 2, 245. [Google Scholar]
- Broadie, M. Assessing Golfer Performance on the PGA Tour. Interfaces 2011, 42, 146–165. [Google Scholar] [CrossRef]
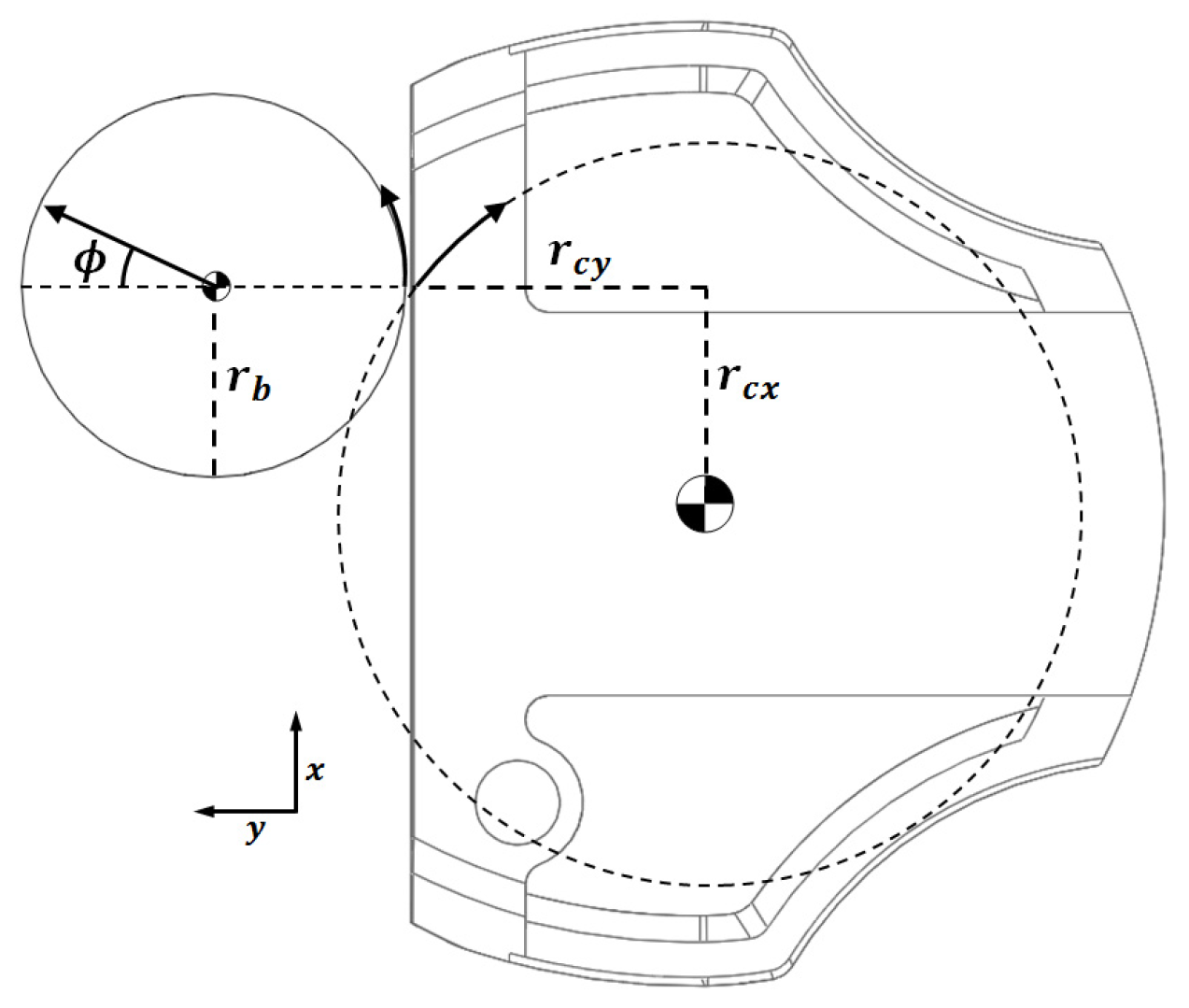
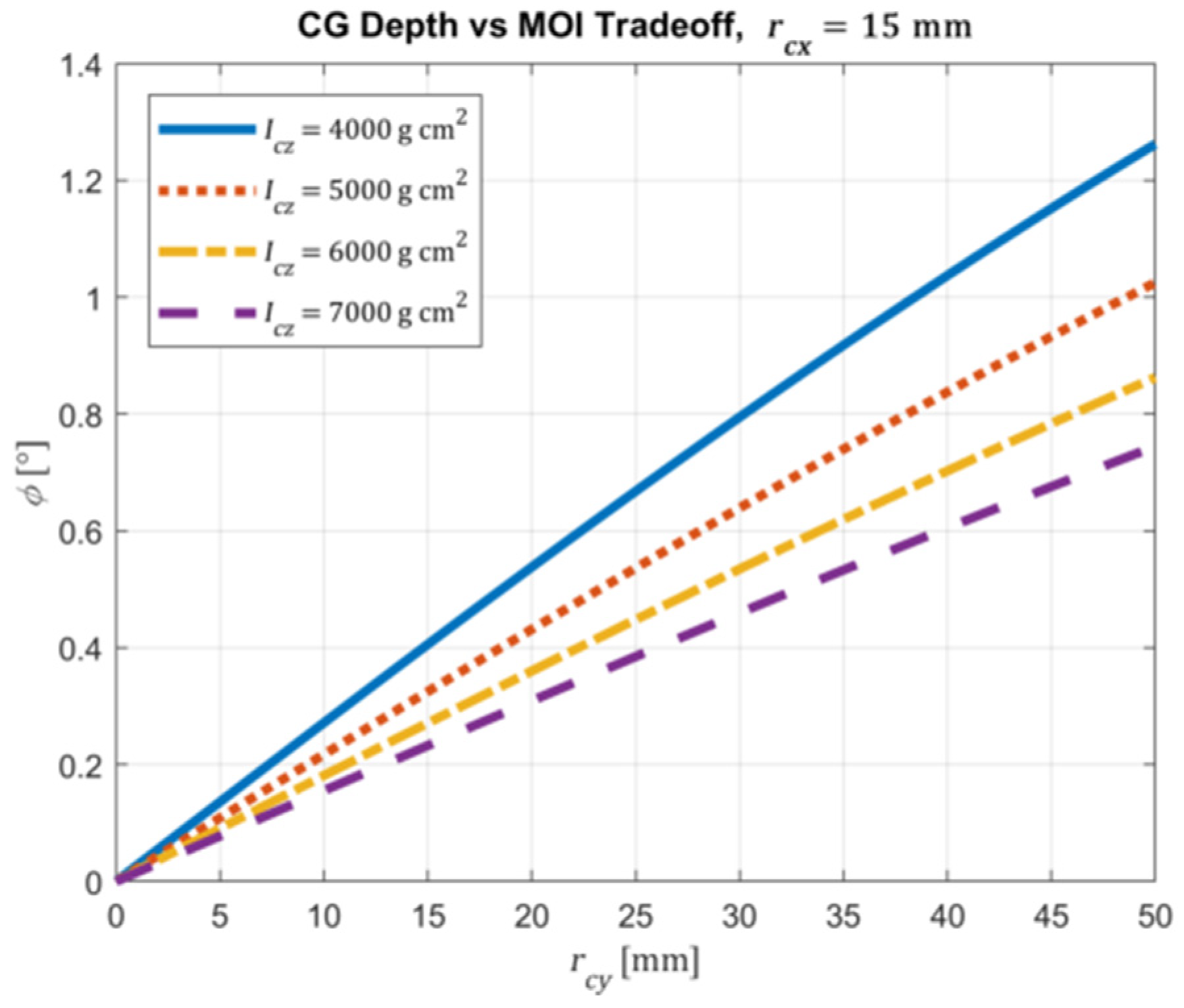
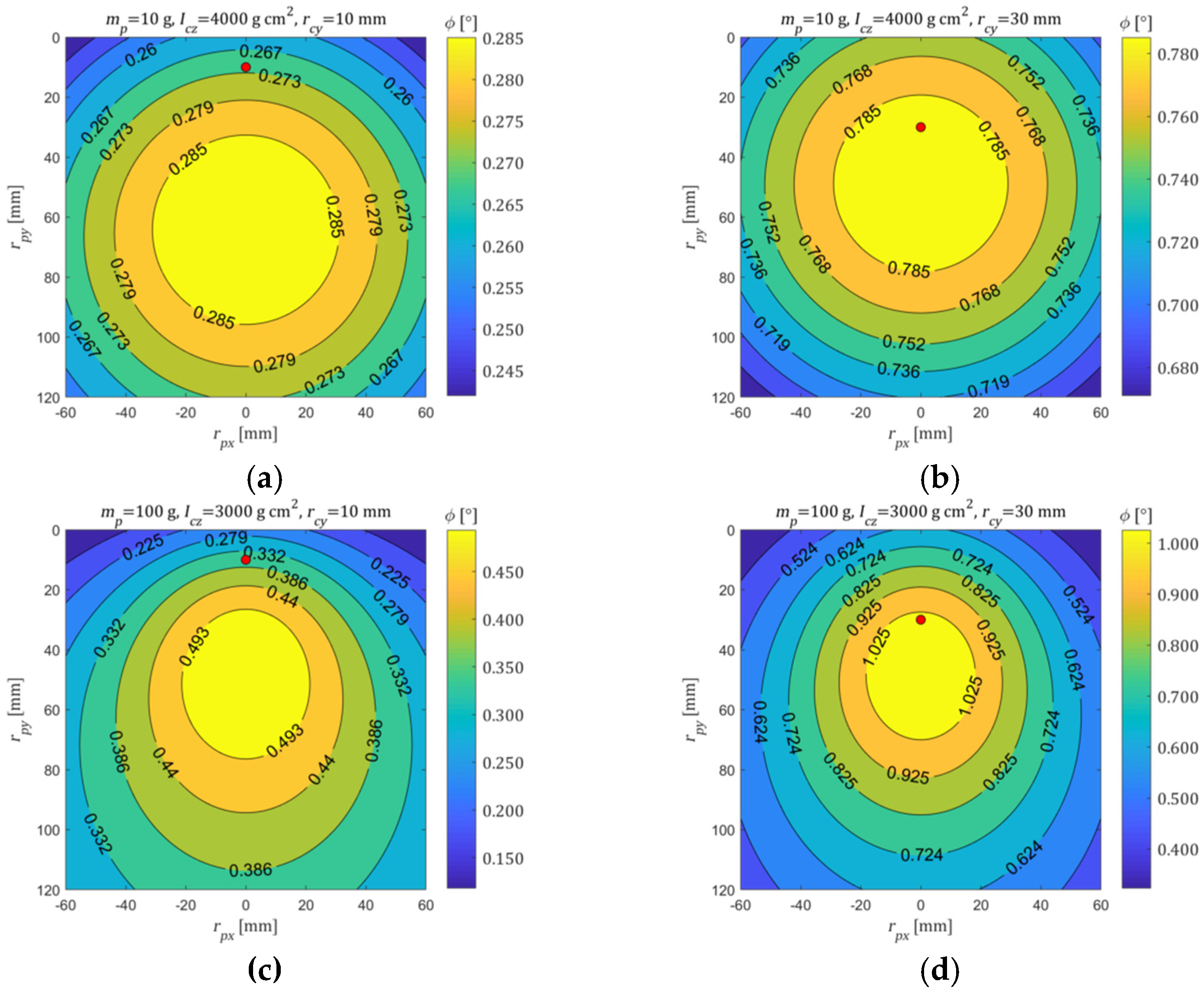

| Putter | ± 95% CI | Difference | ||
|---|---|---|---|---|
| Configuration A | −15 mm (Heel) | −0.58° ± 0.13° | −0.30° | −0.28° |
| 0 mm (Center) | 0.00° ± 0.10° | 0.00° | 0.00° | |
| 15 mm (Toe) | 0.23° ± 0.18° | 0.30° | −0.07° | |
| Configuration B | −15 mm (Heel) | −0.72° ± 0.18° | −0.56° | −0.16° |
| 0 mm (Center) | 0.00° ± 0.05° | 0.00° | 0.00° | |
| 15 mm (Toe) | 0.59° ± 0.10° | 0.56° | 0.03° |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lambeth, J.; Brekke, D.; Brunski, J. Exploration of Center of Gravity, Moment of Inertia, and Launch Direction for Putters with Ball Speed Normalizing Face Properties. Proceedings 2020, 49, 2. https://doi.org/10.3390/proceedings2020049002
Lambeth J, Brekke D, Brunski J. Exploration of Center of Gravity, Moment of Inertia, and Launch Direction for Putters with Ball Speed Normalizing Face Properties. Proceedings. 2020; 49(1):2. https://doi.org/10.3390/proceedings2020049002
Chicago/Turabian StyleLambeth, Jacob, Dustin Brekke, and Jeff Brunski. 2020. "Exploration of Center of Gravity, Moment of Inertia, and Launch Direction for Putters with Ball Speed Normalizing Face Properties" Proceedings 49, no. 1: 2. https://doi.org/10.3390/proceedings2020049002
APA StyleLambeth, J., Brekke, D., & Brunski, J. (2020). Exploration of Center of Gravity, Moment of Inertia, and Launch Direction for Putters with Ball Speed Normalizing Face Properties. Proceedings, 49(1), 2. https://doi.org/10.3390/proceedings2020049002




