Modelling and Simulation of Microplasma Discharge Device for Sterilization Applications †
Abstract
1. Introduction
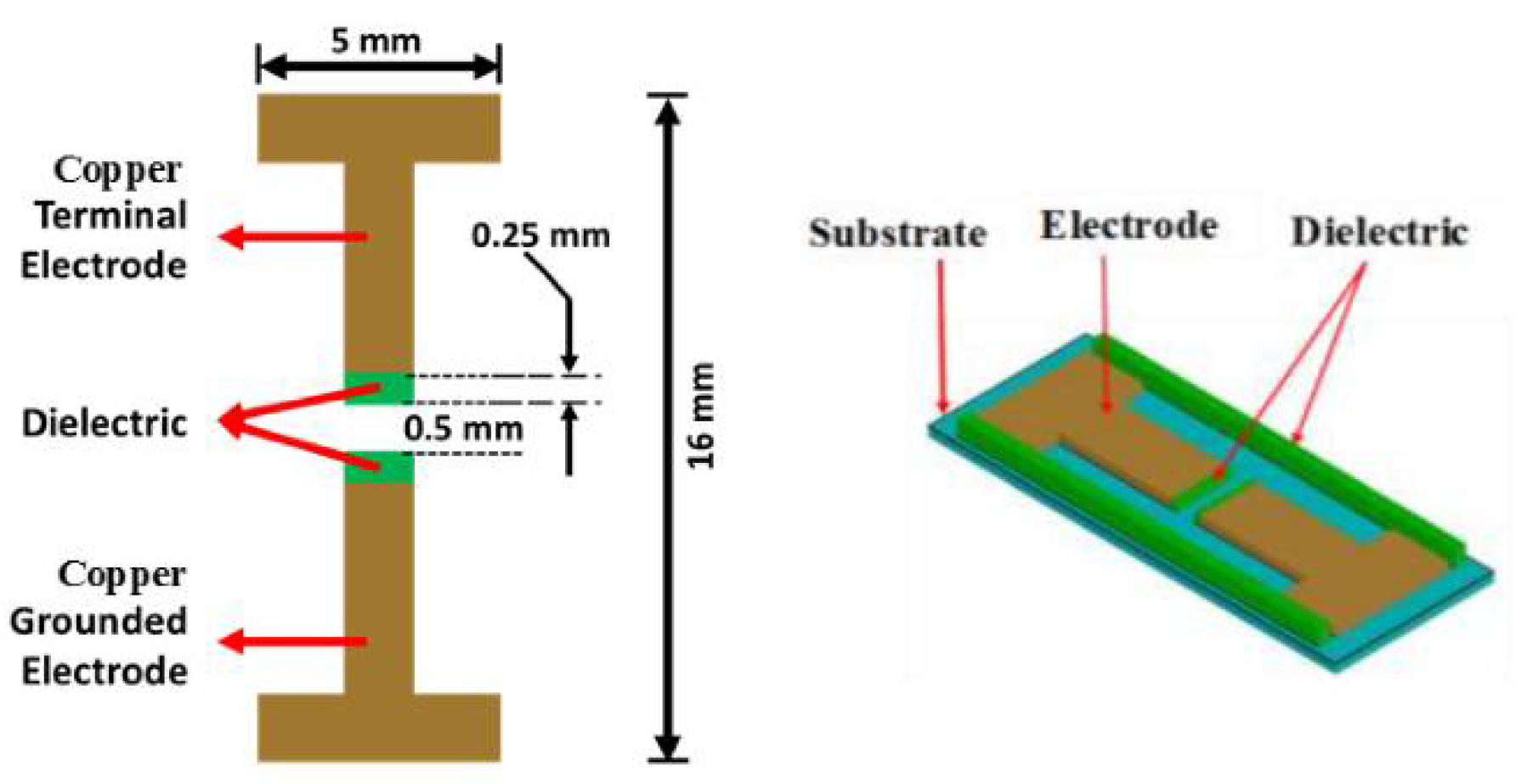
2. Design and Theory
3. Numerical Simulation
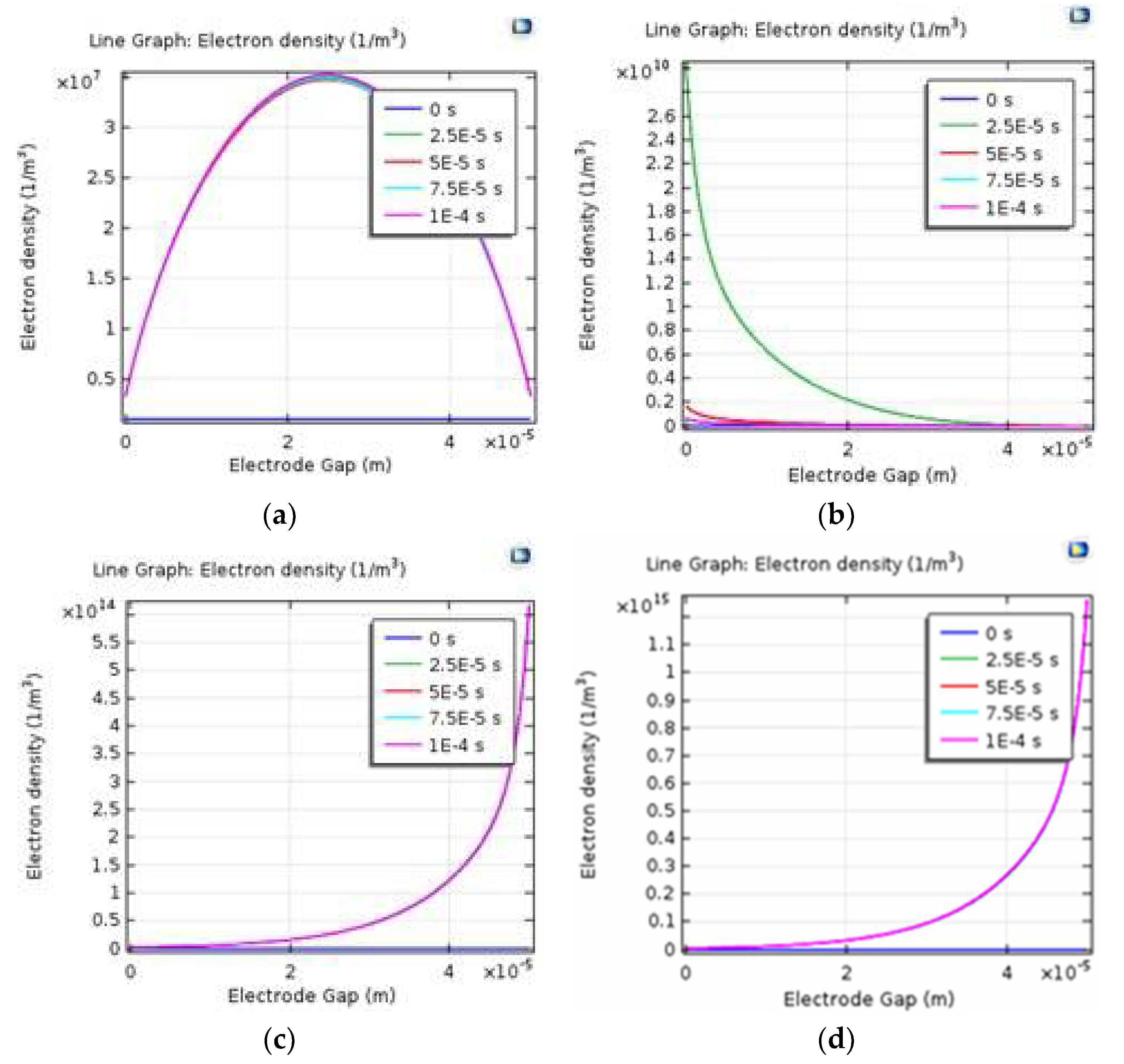
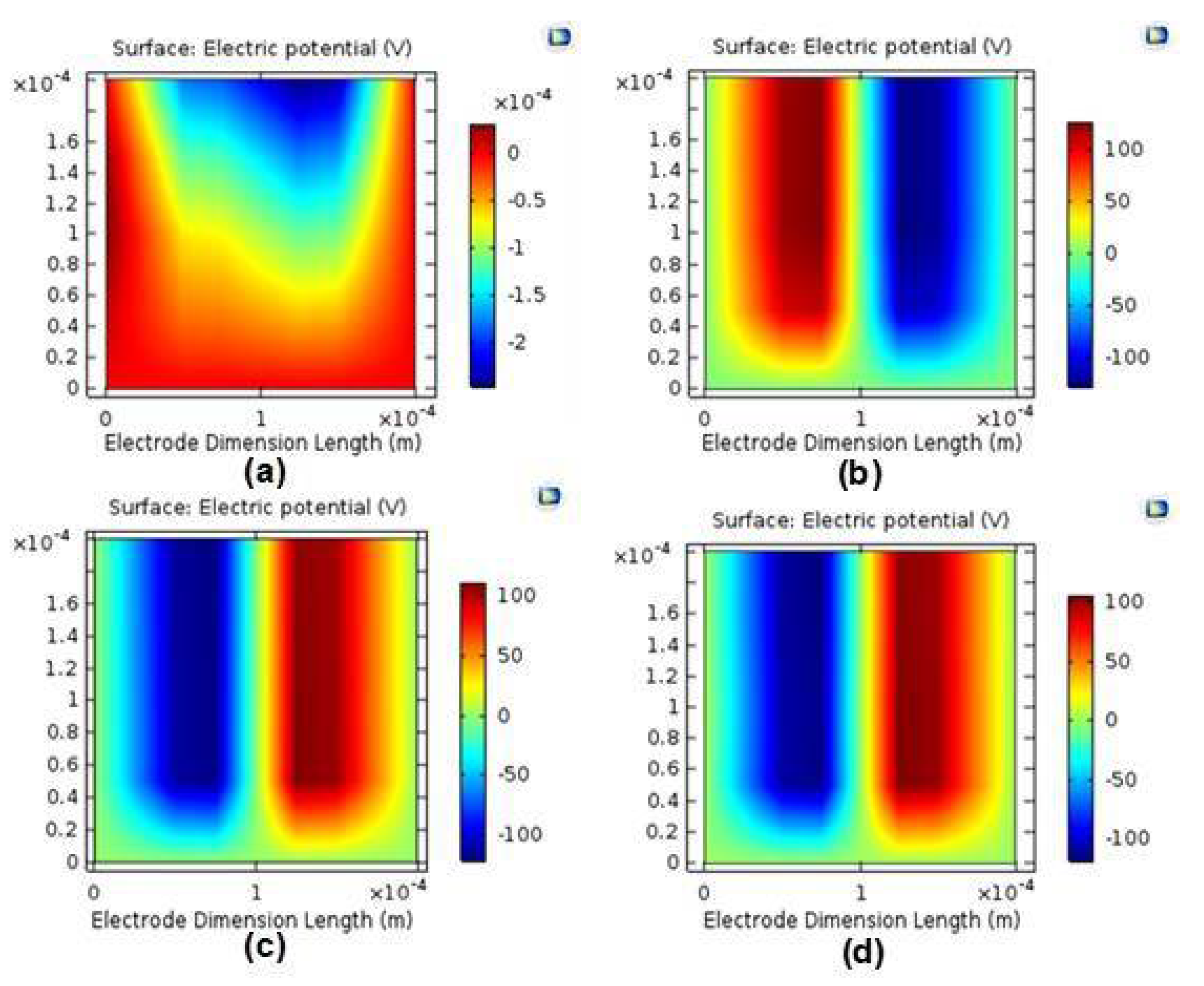
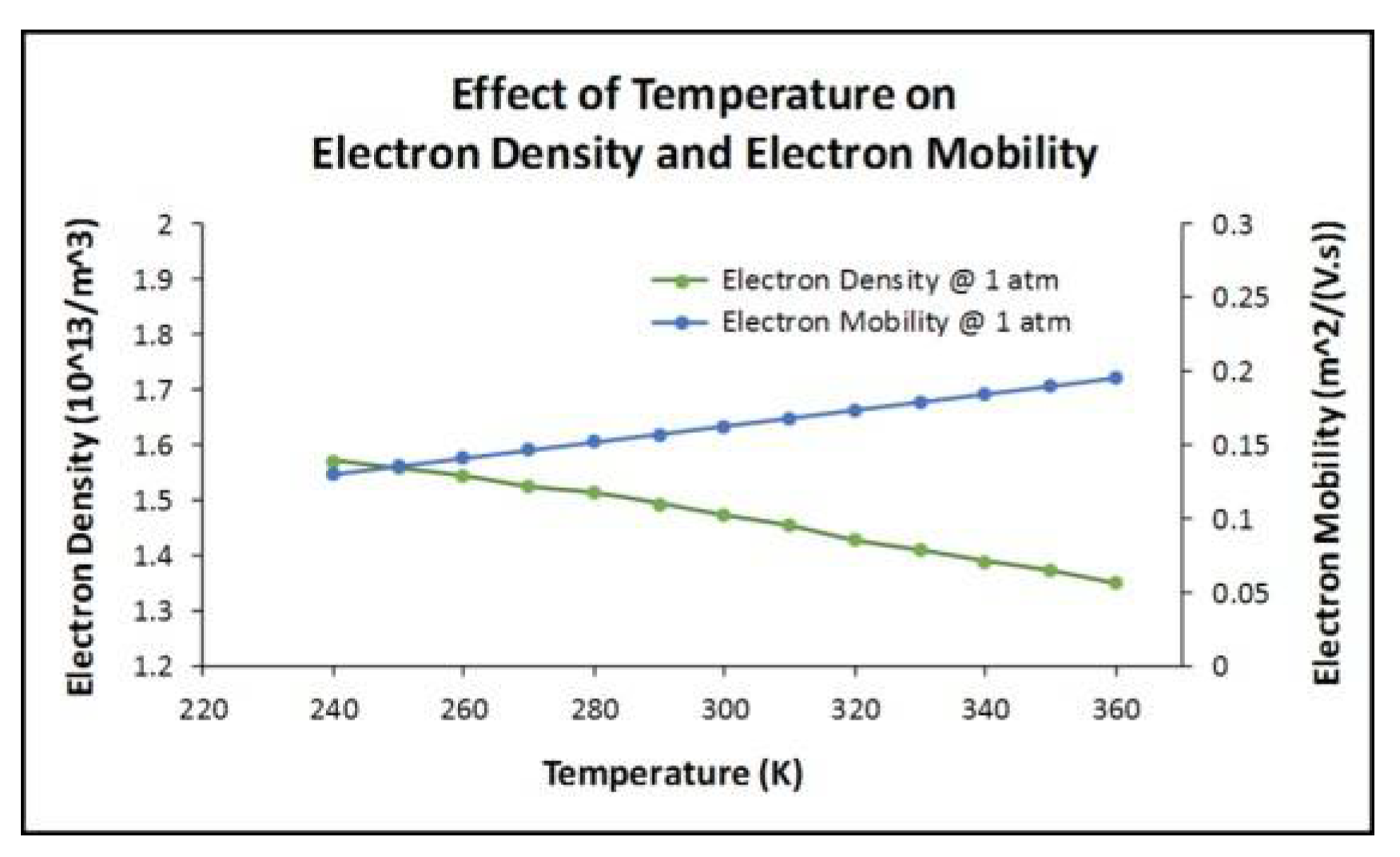
4. Results
5. Conclusions
References
- Becker, K.H.; Schoenbach, K.H.; Eden, J.G. Microplasmas and applications. J. Phys. D Appl. Phys. 2006, 39, 55–70. [Google Scholar] [CrossRef]
- Becker, K. Microplasmas, a platform technology for a plethora of plasma applications. Eur. Phys. J. Spec. Top. 2017, 226, 2853–2858. [Google Scholar] [CrossRef][Green Version]
- Bose, A.K.; Maddipatla, D.; Narakathu, B.B.; Turkani, V.S.; Bazuin, B.J.; Atashbar, M.Z. Flexible microplasma discharge device for the detection of biochemical. In Proceedings of the 17th International Meeting on Chemical Sensors—IMCS 2018, Vienna, Austria, 15–19 July 2018; pp. 419–420. [Google Scholar] [CrossRef]
- Stauss, S.; Muneoka, H.; Terashima, K. Review on plasmas in extraordinary media: Plasmas in cryogenic conditions and plasmas in supercritical fluids. Plasma Sources Sci. Technol. 2018, 27. [Google Scholar] [CrossRef]
- Berzak, L.F.; Dorfman, S.E.; Smith, S.P. Paschen’s Law in Air and Noble Gases; Lawrence Berkeley National Laboratory: Berkeley, CA, USA, 2006. [Google Scholar]
- COMSOL: Application Gallery. Available online: https://www.comsol.com/model/dielectric-barrier-discharge (accessed on 7 April 2018).
- Borghesani, A.F.; Lamp, P. Electron mobility in dense argon gas at several temperatures. Trans. Dielectr. Electr. Insul. 2003, 10, 977–984. [Google Scholar] [CrossRef]
- Kautz, C.H.; Heron, P.R.; Loverude, M.E.; McDermott, L.C. Student understanding of the ideal gas law, Part I: A macroscopic perspective. Am. J. Phys. 2005, 73, 1055–1063. [Google Scholar] [CrossRef]
- Rossi, F.; Kylian, O.; Rauscher, H.; Hasiwa, M.; Gilliland, D. Low pressure plasma discharges for the sterilization and decontamination of surfaces. New J. Phys. 2009, 11, 115017. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bose, A.; Narakathu, B.; Bazuin, B.; Atashbar, M. Modelling and Simulation of Microplasma Discharge Device for Sterilization Applications. Proceedings 2018, 2, 948. https://doi.org/10.3390/proceedings2130948
Bose A, Narakathu B, Bazuin B, Atashbar M. Modelling and Simulation of Microplasma Discharge Device for Sterilization Applications. Proceedings. 2018; 2(13):948. https://doi.org/10.3390/proceedings2130948
Chicago/Turabian StyleBose, Arnesh, Binu Narakathu, Bradley Bazuin, and Massood Atashbar. 2018. "Modelling and Simulation of Microplasma Discharge Device for Sterilization Applications" Proceedings 2, no. 13: 948. https://doi.org/10.3390/proceedings2130948
APA StyleBose, A., Narakathu, B., Bazuin, B., & Atashbar, M. (2018). Modelling and Simulation of Microplasma Discharge Device for Sterilization Applications. Proceedings, 2(13), 948. https://doi.org/10.3390/proceedings2130948





