Design and Numerical Evaluation of a Highly Selective CMOS-Compatible Mid-IR Thermal Emitter/Detector Structure Using Optical Tamm-States †
Abstract
:1. Introduction
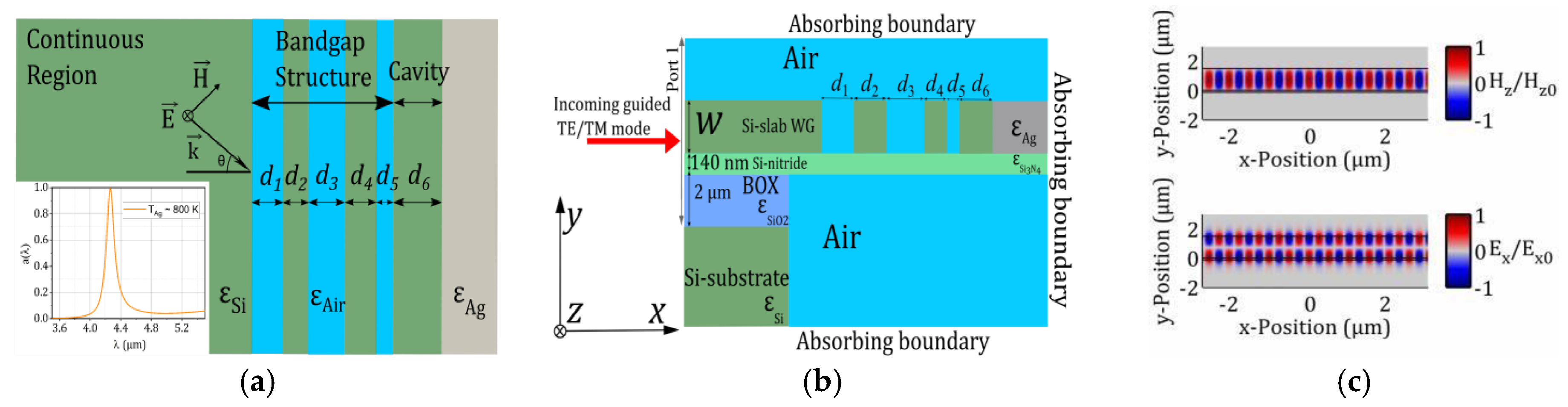
2. Design and Methods
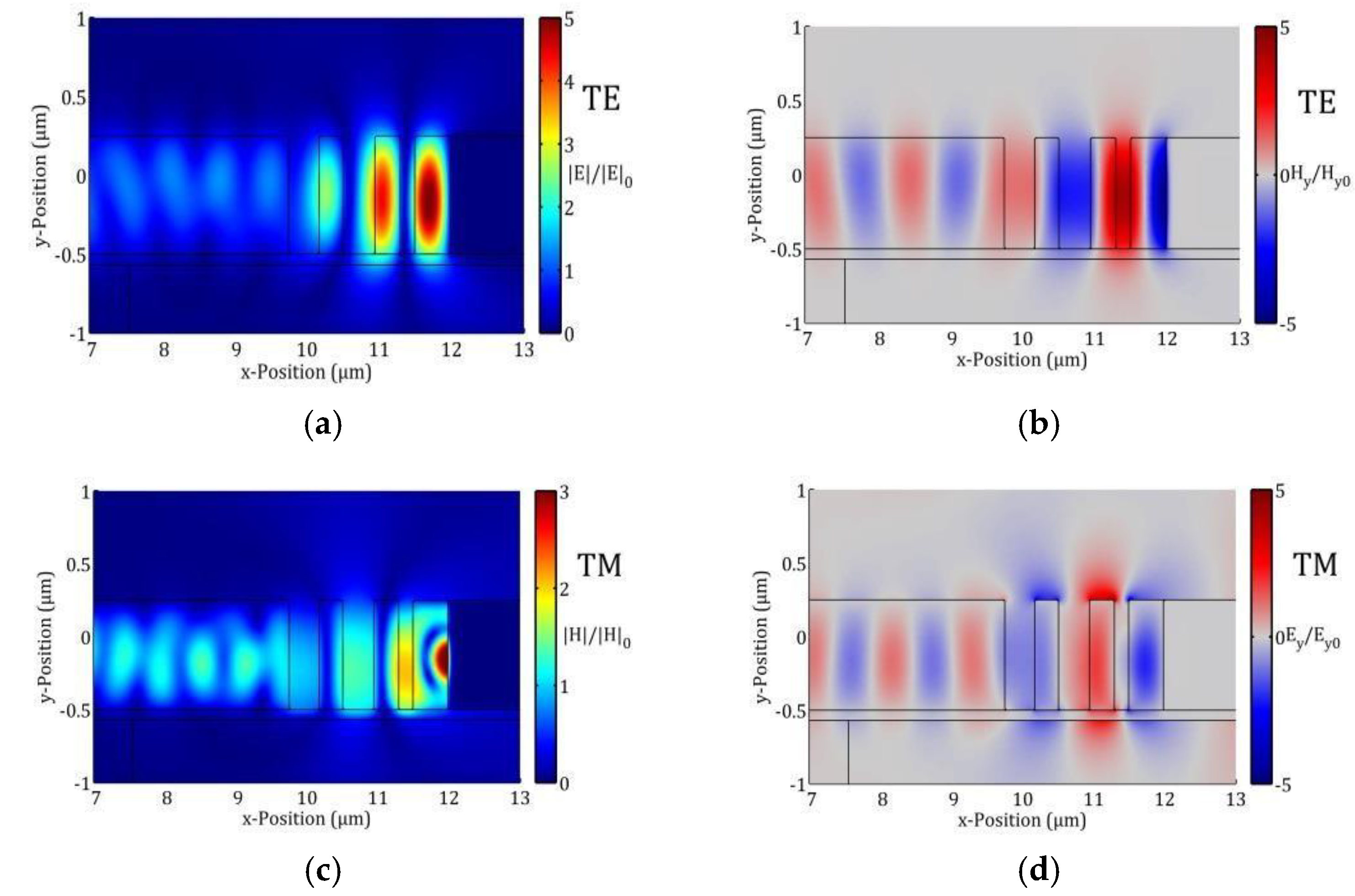
3. Properties of TPP Structures and Design Considerations
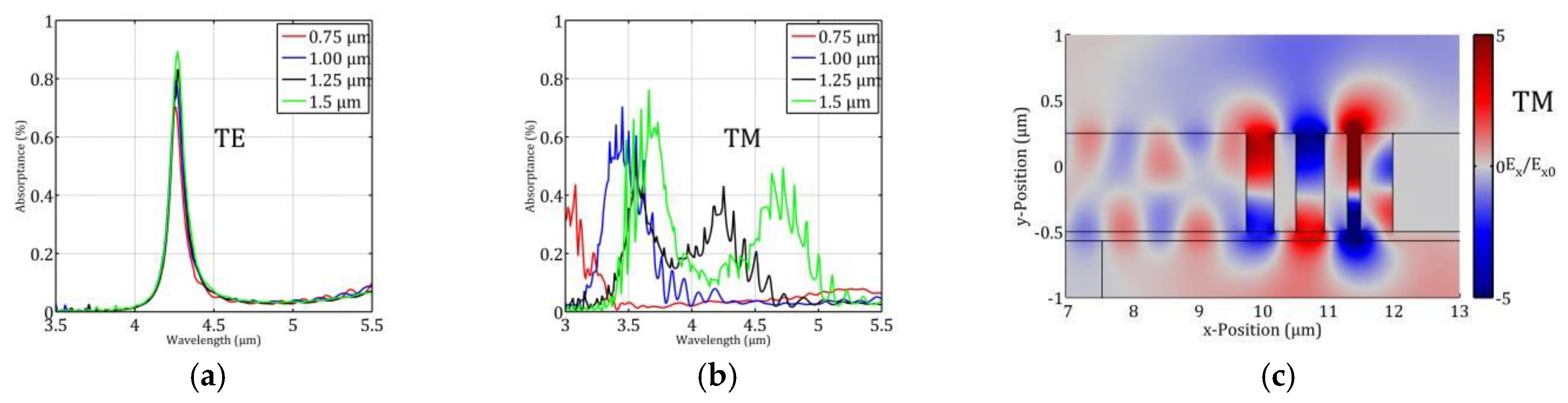
4. Evaluation and Results
Acknowledgments
Conflicts of Interest
References
- Yang, Z.Y.; Ishii, S.; Yokoyama, T.; Dao, T.D.; Sun, M.G.; Pankin, P.S.; Timofeev, I.V.; Nagao, T.; Chen, K.P. Narrowband wavelength selective thermal emitters by confined tamm plasmon polaritons. ACS Photonics 2017, 4, 2212–2219. [Google Scholar] [CrossRef]
- Pühringer, G.; Jakoby, B. Taming parasitic thermal emission by Tamm plasmon polaritons for the mid-infrared. J. Opt. Soc. Am. B 2018, 35, 1490–1503. [Google Scholar] [CrossRef]
- Celanovic, I.; Perreault, D.; Kassakian, J. Resonant-cavity enhanced thermal emission. Phys. Rev. B 2005, 72, 2–7. [Google Scholar] [CrossRef]
- Joannopoulos, J.J.D.; Johnson, S.; Winn, J.N.J.; Meade, R.R.D. Photonic Crystals: Molding the Flow of Light; Prinston University Press: Princeton, NJ, USA, 2008. [Google Scholar]
- Villeneuve, P.R.; Fan, S.; Johnson, S.G.; Joannopoulos, J.D. Three-dimensional photon confimement in photonic crystals of low-dimensional periodicity. IEE Proc. 1998, 145, 384–390. [Google Scholar]
- Lipson, M.; Pollock, C. Integrated Photonics; Kluwer Academic Publishers: Norwell, MA, USA, 2003. [Google Scholar]
- Pühringer, G.; Jakoby, B. Efficient Vertical-Cavity Mid-IR Thermal Radiation to Silicon-Slab Waveguide Coupling Using a Shallow Blazed Grating. Proceedings 2017, 1, 286. [Google Scholar] [CrossRef]
- Granier, C.H.; Afzal, F.O.; Min, C.; Dowling, J.P.; Veronis, G. Optimized aperiodic highly directional narrowband infrared emitters. J. Opt. Soc. Am. B 2014, 31, 1316–1321. [Google Scholar] [CrossRef]
| 𝒘 (µm) | 𝒅𝟏 | 𝒅𝟐 | 𝒅𝟑 | 𝒅𝟒 | 𝒅𝟓 | 𝒅𝟔 | |
|---|---|---|---|---|---|---|---|
| ∞ 1 | 0.40 | 0.33 | 0.41 | 0.35 | 0.15 | 0.48 | 100% |
| 1.5 | 0.42 | 0.33 | 0.44 | 0.35 | 0.2 | 0.49 | 90% |
| 1.25 | 0.44 | 0.34 | 0.45 | 0.36 | 0.2 | 0.50 | 84% |
| 1 | 0.42 | 0.35 | 0.44 | 0.37 | 0.2 | 0.52 | 80% |
| 0.75 | 0.45 | 0.37 | 0.46 | 0.39 | 0.2 | 0.54 | 70% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pühringer, G.; Jakoby, B. Design and Numerical Evaluation of a Highly Selective CMOS-Compatible Mid-IR Thermal Emitter/Detector Structure Using Optical Tamm-States. Proceedings 2018, 2, 1032. https://doi.org/10.3390/proceedings2131032
Pühringer G, Jakoby B. Design and Numerical Evaluation of a Highly Selective CMOS-Compatible Mid-IR Thermal Emitter/Detector Structure Using Optical Tamm-States. Proceedings. 2018; 2(13):1032. https://doi.org/10.3390/proceedings2131032
Chicago/Turabian StylePühringer, Gerald, and Bernhard Jakoby. 2018. "Design and Numerical Evaluation of a Highly Selective CMOS-Compatible Mid-IR Thermal Emitter/Detector Structure Using Optical Tamm-States" Proceedings 2, no. 13: 1032. https://doi.org/10.3390/proceedings2131032
APA StylePühringer, G., & Jakoby, B. (2018). Design and Numerical Evaluation of a Highly Selective CMOS-Compatible Mid-IR Thermal Emitter/Detector Structure Using Optical Tamm-States. Proceedings, 2(13), 1032. https://doi.org/10.3390/proceedings2131032




