Management of Water Distribution Systems in PDA Condition with Isolation Valves ^†

Abstract

The correct management of Water Distribution Networks (WDNs) allows to obtain a reliable system. When a pipe failure occurs in a network and it is necessary to isolate a zone, it is possible that some nodes do not guarantee service for the users due to inadequate heads. In these conditions a Pressure Driven Analysis (PDA) is the correct approach to evaluate network behavior. This analysis is more appropriate than the Demand Driven Analysis (DDA) because it is known that the effective delivered flow at each node is influenced by the pressure value. In this case, it is important to identify a subset of isolation valves to limit disrupting services in the network. For a real network, additional valves must be added to existing ones. In this paper a new methodological analysis is proposed: it defines an objective function (OF) to provide a measure of the system correct functioning. The network analysis using the OF helps to choose the optimal number of additional valves to obtain an adequate system control. In emergency conditions, the OF takes into account the new network topology obtained excluding the zone where the broken pipe is located. OF values depend on the demand deficit caused by the head decrement in the network nodes for each pipe burst considered. The results obtained for a case study confirm the efficiency of the methodology.

1. Introduction

The correct management of a WDN requires the use of valves along the pipes to control the pressure or to isolate a zone when it is necessary to operate on the network. It is important to define and to limit the number of active valves in order to contain costs, to avoid management problems and to guarantee good performances of the system during each operating condition.

The shut-off valves are used when a failure occurs. In this case, the area of the network (segment) where the broken pipe is located must be isolated for the repair, removal or substitution of the pipe. It is possible to isolate each pipe by using different subsets of valves and, consequently, it is important to define their position in the network. Different subsets correspond to different network topologies.

To choose the position and the number of the valves for planned or unplanned interruptions and to limit disruptions in the network, some authors [1,2], proposed an algorithm based on topological matrix. Alvisi et al. [3] suggested a method to identify where to locate isolation valves for the network segmentation based on a pseudo-inverse matrix. In an additional work Giustolisi and Ridolfi [4] presented a multi-objective strategy for optimal network segmentation using a modularity index to identify groups of nodes with strong interconnection. Perelman and Ostfeld [5] proposed a new methodology for the partition of WDN based on a clustering algorithm and on topological and hydraulics connectivity properties.

Giustolisiet al. [6] proposed an optimization procedure to evaluate valve system configuration in the network when the hydraulic parameters change and become inadequate to satisfy water requests. To obtain a correct network segmentation, the authors proposed a new approach based on minimization of costs and fixing constraints on nodal heads.

The aim of this paper is to test a new Objective Function (OF) to define a measure of the operation of the system in real conditions when shut-off valves are active. If the OF decreases, the system performances increase. Starting from an initial condition, characterized by a set of existing valves, it is possible to determine the minimum number of additional isolation valves to limit disruptions in the network when there is a failure in one or more pipes [7].

The new approach is based on a PDA model related to the minimum head H_min [8] to satisfy the requested demand at each node of the network.

2. Materials and Methods

When in a WDN a failure in a pipe occurs, it is necessary to isolate this pipe closing any shut-off valves in the network for needed repair works.

Failures in one or more pipes modify the network functioning and can determine the inefficiency of the system: the analysis with the new topology, taking into account the pipe exclusion, leads to different results. The head in some nodes (here named critical nodes) could be inadequate to deliver the requested nodal demand and to guarantee service for the users.

The minimum head H_min at each node is related to the ground level (z) and to the height of each supplied building (H) and it is defined with the relationship:

$$H_{\min }=z+H+P_{ms}+P_p+P_D$$

(1)

where:

• P_ms is the minimum pressure necessary for the most disadvantaged user;
• P_p are the head losses along the riser column;
• P_D are the head losses starting from the network node and ending at the base of each building.

When the head is lower than H_min the system works in PDA conditions and the delivered demand depends on the real head value.

The aim of this work is to define an Objective Function (OF) to characterize the network behavior when a defined subset of the shut-off valves is activated. An improvement of the head in the network nodes can be obtained by using additional valves to limit the number of nodes of the isolated area and the undelivered demand to the users: the values assumed by OF help to localize the position of the valves and to define their number. The methodology is based on the identification of different network configurations to exclude the segment where the burst pipe is located. Each scenario has been obtained with the activation of one or more shut-off valves, some already existing and other additional valves. The goal is to achieve the minimum of the objective function OF.

$$\mathrm{OF}={\displaystyle \sum _{i=1}^{n_{seg}}\left(\frac{nc{v}_i}{nv}+\frac{Q_i}{Q_{PD{A}_i}}+\frac{Q_{PD{A}_i}-Q_{R{D}_i}}{Q_{PD{A}_i}}\right)}{w}_i$$

(2)

where:

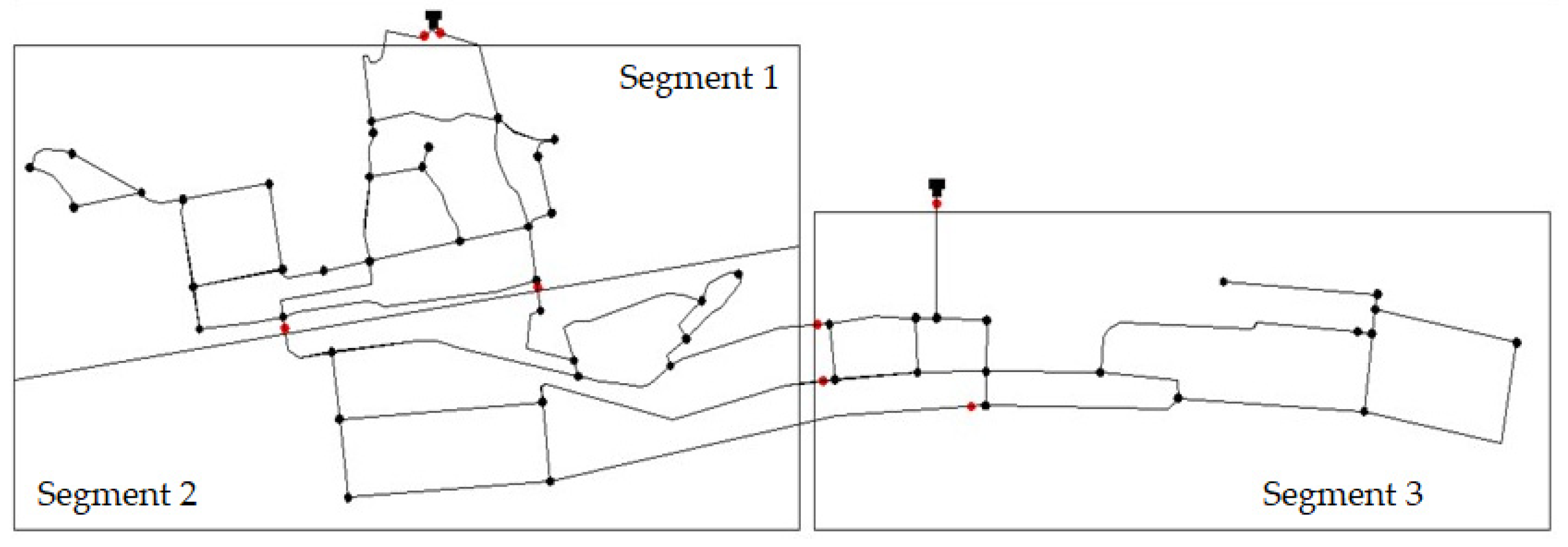
• n_seg is the number of segments of the network;
• n_CVi is the number of operating shut-off valves isolating the segment;
• n_V is the total number of shut-off valves in the network;
• Q_PDAi is the deliverable demand at each segment before the closure of the valves;
• Q_i is the undeliverable demand at each segment after the closure of the valves;
• Q_RDi is the deliverable demand at each segment after the closure of the valves;
• w_i is the weight for each segment calculated as the ratio between the segment base demand in DDA condition and the total base demand in the network.

For each failure scenario, i.e., for each pipe burst, different OF values can be calculated varying the subset of activated shut-off valves. The minimum value of OF defines the best subset of valves to isolate the area where the broken pipe is located.

3. Results

The methodology was applied to a real case, which is the network of Praia a Mare (Cosenza, Italy), a city in the Northern zone of Calabria. The network, consisting of 73 pipes, 53 nodes and 2 source/tanks of water with a fixed head [9], is shown in Figure 1. The total base demand, in summer conditions, is 47.25 L/s. The minimum head H_min varies from 29 to 68 m.

In the real network there are 8 shut-off valves. By closing these shut-off valves it is possible to have 3 segments as shown in Figure 2.

Different configurations with a defined number of shut-off valves was considered for the network. In particular, twelve configurations were assumed. By increasing the number of valves in the network a higher number of segments can be obtained as indicated in

Table 1. Different scenarios obtained with different numbers of shut-off valve.

Scenarios	Segments	N° Valves
1	1	3
2	2	6
3	3	8
4	4	9
5	5	12
6	6	14
7	7	16
8	7	17
9	9	22
10	12	26
11	14	30
12	21	38

. See, for example, scenario n°6 in which there are 14 valves and 6 segments as shown in Figure 3.

For each scenario, corresponding to different pipe bursts, the isolated area has been defined and the network analysis with closed valves has been performed. To close off a broken pipe a specific subset of valves has been chosen.

For scenario n°6 the subset is indicated as example in

Table 2. Characteristics of the scenario n°6.

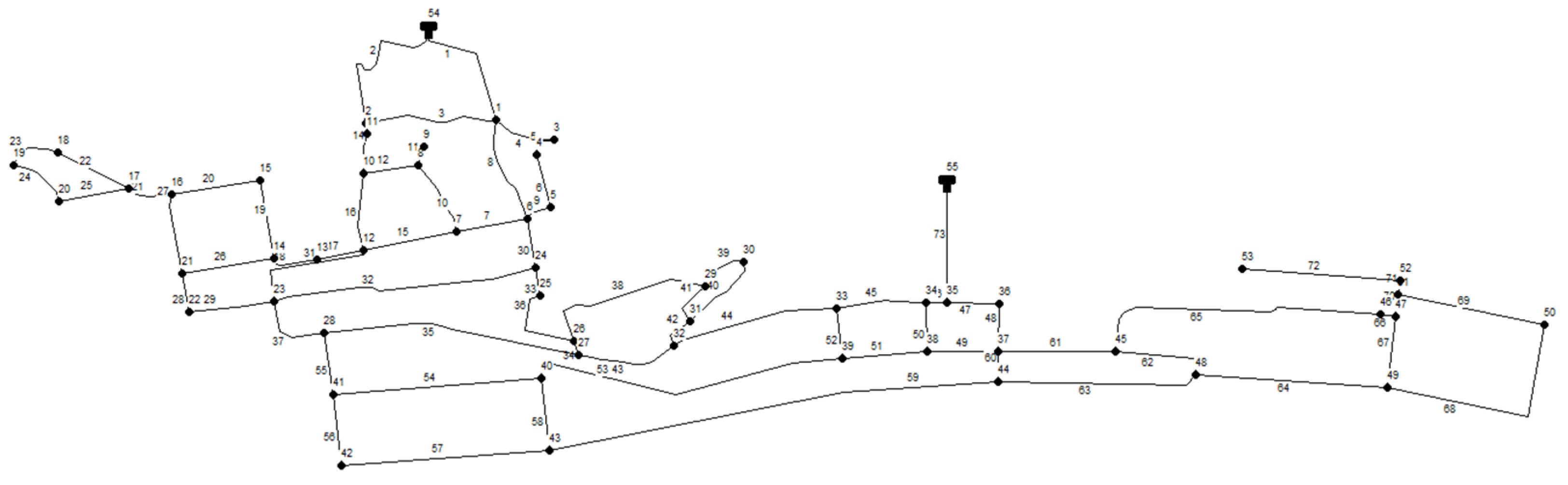
Scenario N°6	Segment	Broken Pipes	Subset of Valves	Operating Valves ncv
nv = 14 valves	1	3-4-5-6-7-8-9-10-11-12-13-14-15-16-17-30-31-32	1-2-33-37	4
	2	19-20-26-27-28	18-29	2
	3	22-23-24-25	21	1
	4	34-35-36-38-39-40-41-42-43-54-55-56-57-58	37-33-44-53-59	5
	5	5-46-47-48-49-50-51-52-60-61-62-63-64	73-44-53-59	4
	6	66-69-70-71-72	68-67	2

.

The analysis in PDA conditions provides the parameters necessary to calculate the terms of the OF and its value. Referring to the example case, see

Table 3. Terms to calculate OF for the scenario n°6.

Segment	ncvi/nv	Qi/QPDA	(QPDAi-QRDi)/QPDA	wi	OFi
1	0.43	0.37	0.38	0.26	0.307
2	0.21	0.11	0.11	0.07	0.031
3	0.07	0.04	0.04	0.04	0.007
4	0.36	0.27	0.27	0.27	0.248
5	0.43	0.35	0.41	0.26	0.311
6	0.21	0.09	0.09	0.09	0.036
				OF	0.940

, these terms are:

Q_i is equal to the difference between Q_PDAi and Q_RDi when, closing each segment, the network works in DDA conditions. Closing segments 1 and 5, the network works in PDA conditions and Q_RDi is lesser than Q_RDi in DDA conditions.

For the other cases the OF value decreases when the number of total valves n_v increases as shown in

Table 4. OF Values for the different scenarios.

Scenario	N° Valves	OF
1	3	3.000
2	6	1.885
3	8	1.234
4	9	1.167
5	12	1.025
6	14	0.940
7	16	0.598
8	17	0.643
9	22	0.528
10	26	0.425
11	30	0.342
12	38	0.249

and in Figure 4.

The OF function decreases when the number of valves increases; it then levels off when the number of valves exceeds a specific value. The solution does not improve significantly when the number of valves reaches a particular number. Therefore, good results can also be achieved for a limited number of valves.

In other words, the improvement becomes insignificant, for technical purposes, by increasing the number of valves in the network beyond a defined threshold. So, the idea is to verify how the solution and the OF values change when the additional valves disposition is different.

In the case study scenario n°6 with 14 total valves (6 valves in addition to the existing 8) is the one beyond which the OF levelled off. By changing the position of the 6 additional valves the OF value was evaluated to verify how the solution changes. With differently located shut-off valves a different topology of the network must be analyzed; different results characterize each solution.

The OF value for each different position, chosen using a heuristic approach, of the 6 additional valves was calculated and the change in the OF value has been analyzed.

The results are shown in

Table 5. OF Value with different position of the 6 additional valves.

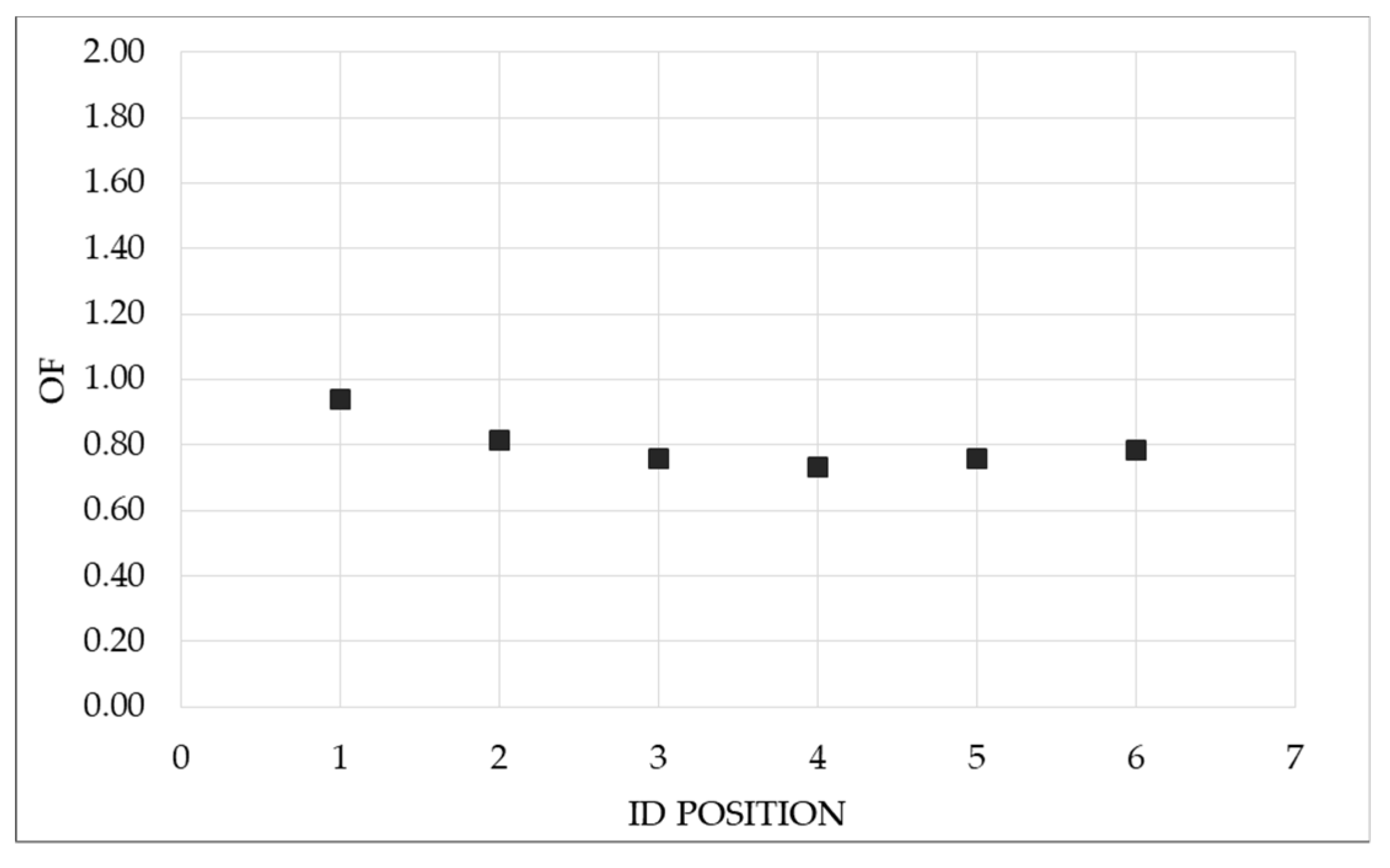
ID Position	OF
1	0.940
2	0.817
3	0.760
4	0.733
5	0.759
6	0.785

and in Figure 5.

For different position of the additional valves, the OF value does not significantly change and the solution seems to be independent from the disposition of additional valves.

The analysis of OF values makes it possible to determine the number of additional valves, beyond which the improvement of OF for management purpose becomes negligible. The position of additional valves, in this case, does not influence the solution probably because the position of exiting valves does not change.

The results have been confirmed by applying the methodology to other real networks and other tests are currently being carried out [10] to confirm the hypothesis.

4. Conclusions

The management of WDN is related with the use of valves that are activated in failure conditions. When there is a pipe failure, it is not possible to guarantee an adequate service to all the users because, for the repair or substitution the broken pipe, it is necessary to isolate not only the pipe but also a wider area.

The extension of the area depends both on the position and on the number of shut-off valves. Furthermore, when a valve is active a different network topology must be considered and the head at each node is influenced by the new topology.

In these conditions, the delivered demand at each node must be determined using a PDA approach and the effective nodal demand depends on the real heads.

In this paper, a new methodology has been proposed: the aim is to define the number of valves that allows a useful management of the network in failure conditions.

An Objective Function was defined and when the OF value decreases, the network behavior improves for management purposes.

When the number of valves n_v increases, the OF decreases and it is possible to determine a value of n_v that represents a technical threshold beyond which improvements become negligible, for technical purposes.

The methodology has also applied to others real networks and the results have been confirmed. Other tests are being conducted to confirm the hypothesis that the position of additional valves does not influence the OF value.