Experimental Investigation of Quantum Decay via Integrated Photonics †
Abstract
:1. Introduction
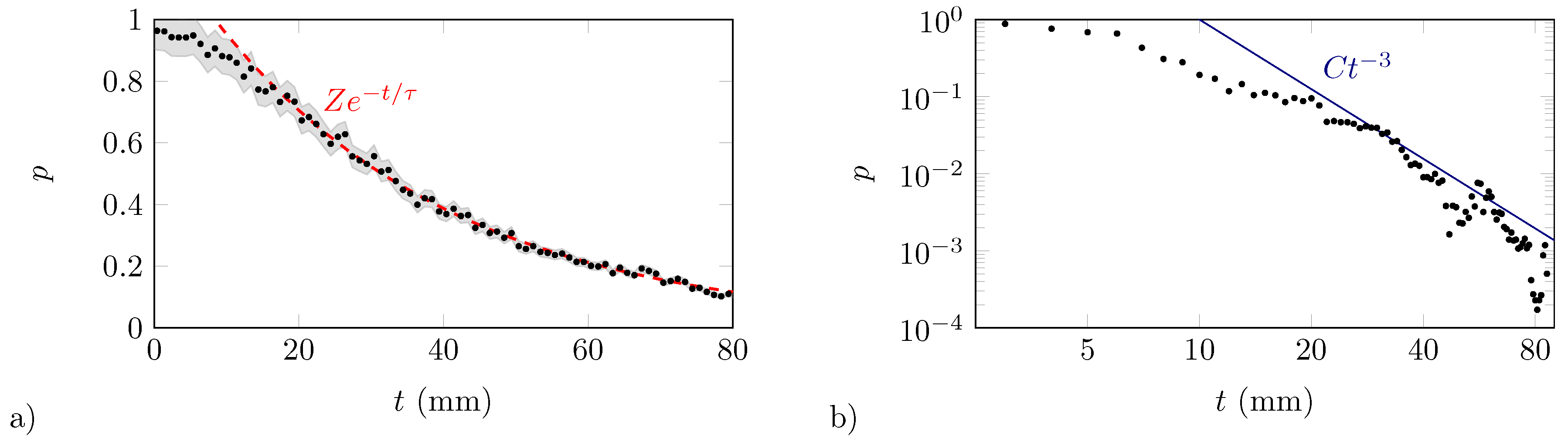
2. Results
3. Discussion
4. Materials and Methods
Waveguide Fabrication
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Nakazato, H.; Namiki, M.; Pascazio, S. Temporal behavior of quantum mechanical systems. Int. J. Mod. Phys. B 1996, 10, 247. [Google Scholar] [CrossRef]
- Wilkinson, S.R.; Bharucha, C.F.; Fischer, M.C.; Madison, K.W.; Morrow, P.R.; Niu, Q.; Sundaram, B.; Raizen, M.G. Experimental evidence for non-exponential decay in quantum tunnelling. Nature 1997, 387, 575–577. [Google Scholar] [CrossRef]
- Biagioni, P.; Della Valle, G.; Ornigotti, M.; Finazzi, M.; Duo, L.; Laporta, P.; Longhi, S. Experimental demonstration of the optical Zeno effect by scanning tunneling optical microscopy. Opt. Express 2008, 16, 3762–3767. [Google Scholar] [CrossRef] [PubMed]
- Longhi, S. Quantum-optical analogies using photonic structures. Laser Photonics Rev. 2009, 3, 243–261. [Google Scholar] [CrossRef]
- Szameit, A.; Nolte, S. Discrete optics in femtosecond-laser-written photonic structures. J. Phys. B 2010, 43, 163001. [Google Scholar] [CrossRef]
- Chiodo, N.; Della Valle, G.; Osellame, R.; Longhi, S.; Cerullo, G.; Ramponi, R.; Laporta, P.; Morgner, U. Imaging of Bloch oscillations in erbium-doped curved waveguide arrays. Opt. Lett. 2006, 31, 1651–1653. [Google Scholar] [CrossRef] [PubMed]
- Corrielli, G.; Crespi, A.; Della Valle, G.; Longhi, S.; Osellame, R. Fractional Bloch oscillations in photonic lattices. Nat. Commun. 2014, 4, 1555. [Google Scholar] [CrossRef] [PubMed]
- Martin, L.; Di Giuseppe, G.; Perez-Leija, A.; Keil, R.; Dreisow, F.; Heinrich, M.; Nolte, S.; Szameit, A.; Abouraddy, A.F.; Christodoulides, D.N.; Saleh, B.E. Anderson localization in optical waveguide arrays with off-diagonal coupling disorder. Opt. Express 2011, 19, 13636–13646. [Google Scholar] [CrossRef]
- Osellame, R.; Cerullo, G.; Ramponi, R. (Eds.) Femtosecond Laser Micromachining: Photonic and Microfluidic Devices in Transparent Materials; Springer: Berlin/Heidelberg, Germany, 2012; ISBN 978-3-642-23365-4. [Google Scholar]
- Crespi, A.; Longhi, S.; Osellame, R. Photonic realization of the quantum Rabi model. Phys. Rev. Lett. 2012, 108, 163601. [Google Scholar] [CrossRef]
- Crespi, A.; Pepe, F.V.; Facchi, P.; Sciarrino, F.; Mataloni, P.; Nakazato, H.; Pascazio, S.; Osellame, R. Experimental investigation of quantum decay at short, intermediate and long times via integrated photonics. Phys. Rev. Lett. 2019, 122, 130401. [Google Scholar] [CrossRef] [PubMed]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Crespi, A.; Pepe, F.V.; Facchi, P.; Sciarrino, F.; Mataloni, P.; Nakazato, H.; Pascazio, S.; Osellame, R. Experimental Investigation of Quantum Decay via Integrated Photonics. Proceedings 2019, 12, 9. https://doi.org/10.3390/proceedings2019012009
Crespi A, Pepe FV, Facchi P, Sciarrino F, Mataloni P, Nakazato H, Pascazio S, Osellame R. Experimental Investigation of Quantum Decay via Integrated Photonics. Proceedings. 2019; 12(1):9. https://doi.org/10.3390/proceedings2019012009
Chicago/Turabian StyleCrespi, Andrea, Francesco V. Pepe, Paolo Facchi, Fabio Sciarrino, Paolo Mataloni, Hiromichi Nakazato, Saveri Pascazio, and Roberto Osellame. 2019. "Experimental Investigation of Quantum Decay via Integrated Photonics" Proceedings 12, no. 1: 9. https://doi.org/10.3390/proceedings2019012009
APA StyleCrespi, A., Pepe, F. V., Facchi, P., Sciarrino, F., Mataloni, P., Nakazato, H., Pascazio, S., & Osellame, R. (2019). Experimental Investigation of Quantum Decay via Integrated Photonics. Proceedings, 12(1), 9. https://doi.org/10.3390/proceedings2019012009








