Generation of Photon Pairs in the Light-Matter Ultrastrong Coupling Regime: From Casimir Radiation to Stimulated Raman Adiabatic Passage †
Abstract
:1. Introduction
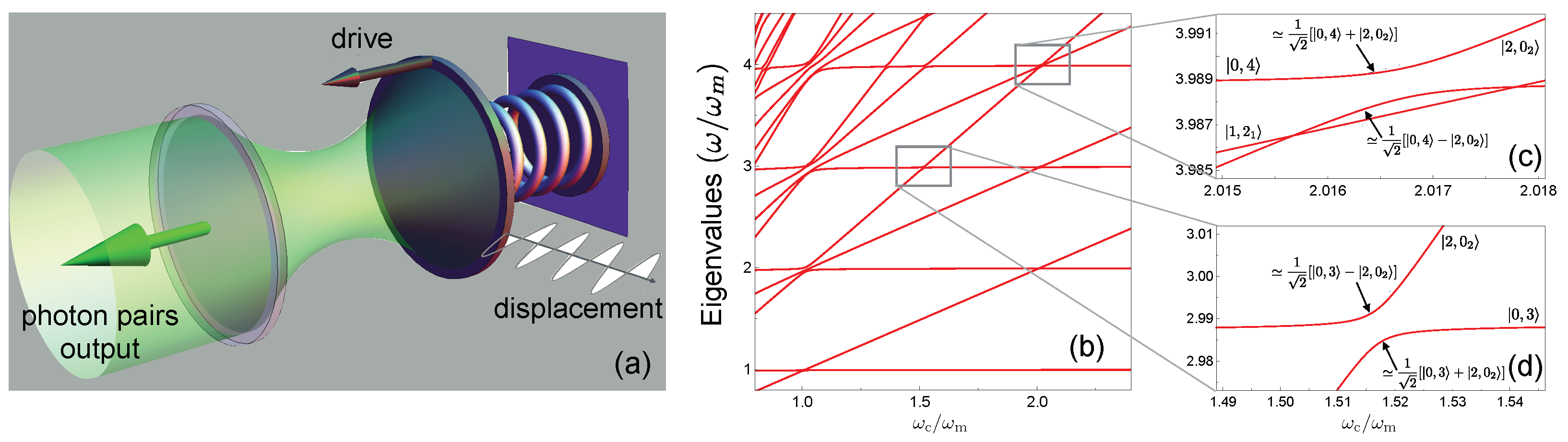
2. Casimir Radiation from Optomechanical Systems
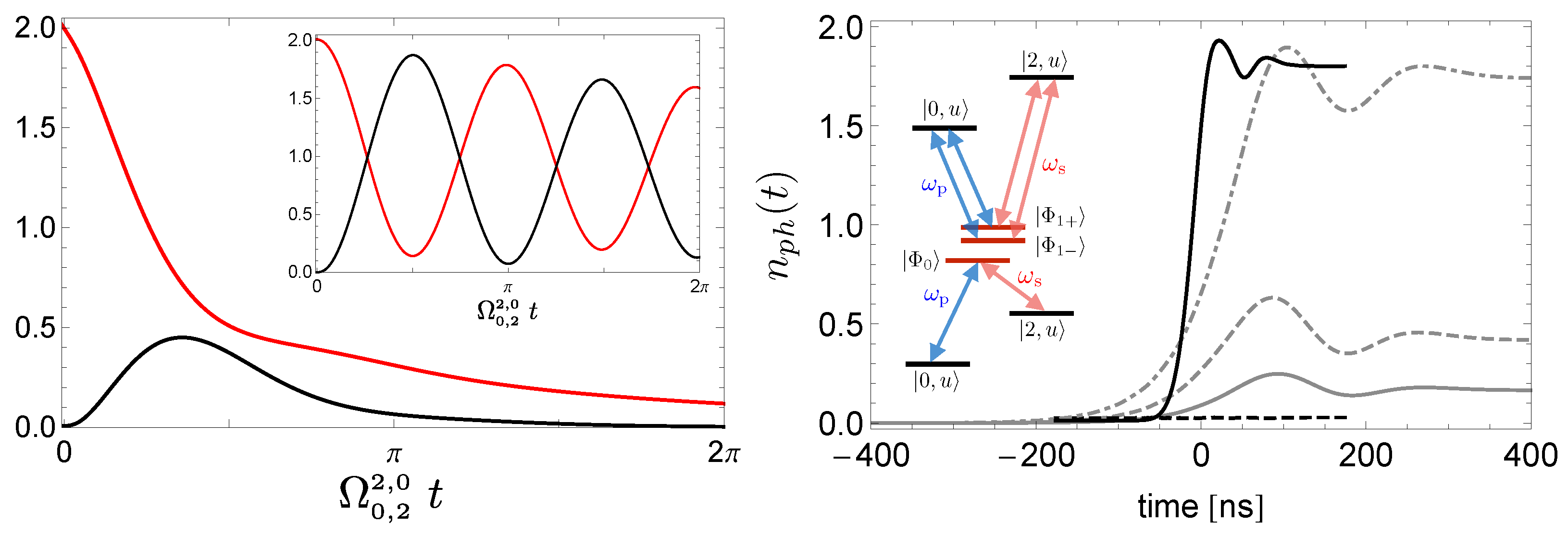
3. Generation of Two-Photon Pairs by STIRAP
4. Conclusions
Conflicts of Interest
References
- Haroche, S.; Raimond, J.M. Exploring the Quantum; Oxford University Press: Oxford, UK, 2008. [Google Scholar]
- Mohseni, M.; Read, P.; Neven, H.; Boixo, S.; Denchev, V.; Babbush, R.; Fowler, A.; Smelyanskiy, V.; Martinis, J. Commercialize quantum technologies in five years. Nature 2017, 543, 171. [Google Scholar] [CrossRef] [PubMed]
- Schoelkopf, R.J.; Girvin, S.M. Wiring up quantum systems. Nature 2008, 451, 664. [Google Scholar] [CrossRef] [PubMed]
- Wallraff, A.; Schuster, D.I.; Blais, A.; Frunzio, L.; Huang, R.S.; Majer, J.; Kumar, S.; Girvin, S.; Schoelkopf, R. Strong coupling of a single photon to a superconducting qubit using circuit quantum electrodynamics. Nature 2004, 421, 162–167. [Google Scholar] [CrossRef] [PubMed]
- Di Stefano, P.G.; Alonso, J.J.; Lutz, E.; Falci, G.; Paternostro, M. Nonequilibrium thermodynamics of continuously measured quantum systems: A circuit QED implementation. Phys. Rev. B 2018, 98, 144514. [Google Scholar] [CrossRef]
- Benenti, G.; D’Arrigo, A.; Falci, G. Enhancement of Transmission Rates in Quantum Memory Channels with Damping. Phys. Rev. Lett. 2009, 103, 020502. [Google Scholar] [CrossRef]
- Kockum, A.F.; Miranowicz, A.; De Liberato, S.; Savasta, S.; Nori, F. Ultrastrong coupling between light and matter. Nat. Rev. Phys. 2019, 1, 19–40. [Google Scholar] [CrossRef]
- Forn-Diaz, P.; Lamata, L.; Rico, E.; Kono, J.; Solano, E. Ultrastrong coupling regime of light-matter interaction. arXiv 2018, arXiv:1804.09275. [Google Scholar] [CrossRef]
- Niemczyk, T.; Deppe, F.; Huebl, H.; Menzel, E.P.; Hocke, F.; Schwarz, M.J.; Garcia-Ripoll, J.J.; Zueco, D.; Hümmer, T.; Solano, E.; et al. Circuit quantum electrodynamics in the ultrastrong-coupling regime. Nat. Phys. 2010, 6, 772. [Google Scholar] [CrossRef]
- Ridolfo, A.; Leib, M.; Savasta, S.; Hartmann, M.J. Photon Blockade in the Ultrastrong Coupling Regime. Phys. Rev. Lett. 2012, 109, 193602. [Google Scholar] [CrossRef]
- Ridolfo, A.; Savasta, S.; Hartmann, M.J. Nonclassical Radiation from Thermal Cavities in the Ultrastrong Coupling Regime. Phys. Rev. Lett. 2013, 110, 163601. [Google Scholar] [CrossRef]
- Stassi, R.; Ridolfo, A.; Di Stefano, O.; Hartmann, M.J.; Savasta, S. Spontaneous Conversion from Virtual to Real Photons in the Ultrastrong-Coupling Regime. Phys. Rev. Lett. 2013, 110, 243601. [Google Scholar] [CrossRef] [PubMed]
- Garziano, L.; Ridolfo, A.; Stassi, R.; Di Stefano, O.; Savasta, S. Switching on and off of ultrastrong light-matter interaction: Photon statistics of quantum vacuum radiation. Phys. Rev. A 2013, 88, 063829. [Google Scholar] [CrossRef]
- Di Stefano, O.; Kockum, A.F.; Ridolfo, A.; Savasta, S.; Nori, F. Photodetection probability in quantum systems with arbitrarily strong light-matter interaction. Sci. Rep. 2018, 8, 17825. [Google Scholar] [CrossRef]
- Macrì, V.; Ridolfo, A.; Di Stefano, O.; Kockum, A.F.; Nori, F.; Savasta, S. Nonperturbative Dynamical Casimir Effect in Optomechanical Systems: Vacuum Casimir-Rabi Splittings. Phys. Rev. X 2018, 8, 011031. [Google Scholar] [CrossRef]
- Falci, G.; Ridolfo, A.; Di Stefano, P.G.; Paladino, E. Ultrastrong coupling probed by Coherent Population Transfer. arXiv 2017, arXiv:1708.00906. [Google Scholar] [CrossRef] [PubMed]
- Moore, G.T. Quantum Theory of the Electromagnetic Field in a Variable-Length One-Dimensional Cavity. J. Math. Phys. (N. Y.) 1970, 11, 2679. [Google Scholar] [CrossRef]
- Fulling, S.A.; Davies, P.C.W. Radiation from a moving mirror in two dimensional space-time: conformal anomaly. Proc. R. Soc. A 1976, 348, 393. [Google Scholar]
- Sassaroli, E.; Srivastava, Y.N.; Widom, A. Photon production by the dynamical Casimir effect. Phys. Rev. A 1994, 50, 1027. [Google Scholar] [CrossRef]
- Johansson, J.R.; Johansson, G.; Wilson, C.M.; Nori, F. Dynamical Casimir Effect in a Superconducting Coplanar Waveguide. Phys. Rev. Lett. 2009, 103, 147003. [Google Scholar] [CrossRef]
- Wilson, C.M.; Johansson, G.; Pourkabirian, A.; Simoen, M.; Johansson, J.R.; Duty, T.; Nori, F.; Delsing, P. Observation of the dynamical Casimir effect in a superconducting circuit. Nature 2011, 479, 376. [Google Scholar] [CrossRef]
- Lambrecht, A.; Jaekel, M.T.; Reynaud, S. Motion Induced Radiation from a Vibrating Cavity. Phys. Rev. Lett. 1996, 77, 615. [Google Scholar] [CrossRef] [PubMed]
- Di Stefano, O.; Settineri, A.; Macrì, V.; Ridolfo, A.; Stassi, R.; Kockum, A.F.; Savasta, S.; Nori, F. Interaction of Mechanical Oscillators Mediated by the Exchange of Virtual Photon Pairs. Phys. Rev. Lett. 2019, 122, 030402. [Google Scholar] [CrossRef] [PubMed]
- Falci, G.; Di Stefano, P.; Ridolfo, A.; D’Arrigo, A.; Paraoanu, G.; Paladino, E. Advances in quantum control of three-level superconducting circuit architectures. Fortschr. Phys. 2017, 65, 1600077. [Google Scholar] [CrossRef]
- Ridolfo, A.; Falci, G.; Pellegrino, F.M.D.; Maccarrone, G.D.; Paladino, E. Photon pair production by STIRAP in ultrastrongly coupled matter-radiation systems. Eur. Phys. J. Spec. Top. 2019, 227, 2183–2188. [Google Scholar] [CrossRef]
- Vitanov, N.V.; Rangelov, A.A.; Shore, B.W.; Bergmann, K. Stimulated Raman adiabatic passage in physics, chemistry, and beyond. Rev. Mod. Phys. 2017, 89, 015006. [Google Scholar] [CrossRef]
- Siewert, J.; Brandes, T.; Falci, G. Advanced control with a Cooper-pair box: Stimulated Raman adiabatic passage and Fock-state generation in a nanomechanical resonator. Phys. Rev. B 2009, 79, 024504. [Google Scholar] [CrossRef]
- Di Stefano, P.G.; Paladino, E.; Pope, T.J.; Falci, G. Coherent manipulation of noise-protected superconducting artificial atoms in the Lambda scheme. Phys. Rev. A 2016, 93, 051801. [Google Scholar] [CrossRef]
- Di Stefano, P.G.; Paladino, E.; D’Arrigo, A.; Falci, G. Population transfer in a Lambda system induced by detunings. Phys. Rev. B 2015, 91, 224506. [Google Scholar] [CrossRef]
- Kumar, K.; Vepsäläinen, A.; Danilin, S.; Paraoanu, G. Stimulated Raman adiabatic passage in a three-level superconducting circuit. Nat. Commun. 2016, 7, 10628. [Google Scholar] [CrossRef]
- Xu, H.K.; Song, C.; Liu, W.Y.; Xue, G.M.; Su, F.F.; Deng, H.; Tian, Y.; Zheng, D.N.; Han, S.; Zhong, Y.P.; et al. Coherent population transfer between uncoupled or weakly coupled states in ladder-type superconducting qutrits. Nat. Commun. 2016, 7, 11018. [Google Scholar] [CrossRef]
- Vepsäläinen, A.; Danilin, S.; Paladino, E.; Falci, G.; Paraoanu, G.S. Quantum Control in Qutrit Systems Using Hybrid Rabi-STIRAP Pulses. Photonics 2016, 3, 62. [Google Scholar] [CrossRef]
- Paladino, E.; Galperin, Y.; Falci, G.; Altshuler, B. 1/f noise: Implications for solid-state quantum information. Rev. Mod. Phys. 2014, 86, 361–418. [Google Scholar] [CrossRef]
- D’Arrigo, A.; Mastellone, A.; Paladino, E.; Falci, G. Effects of low-frequency noise cross-correlations in coupled superconducting qubits. New J. Phys. 2008, 10, 115006. [Google Scholar] [CrossRef]
- Paladino, E.; Mastellone, A.; D’Arrigo, A.; Falci, G. Optimal tuning of solid-state quantum gates: A universal two-qubit gate. Phys. Rev. B 2010, 81, 052502. [Google Scholar] [CrossRef]
- Chiarello, F.; Paladino, E.; Castellano, M. G.; Cosmelli, C.; D’Arrigo, A.; Torrioli, G.; Falci, G. Superconducting qubit manipulated by fast pulses: experimental observation of distinct decoherence regimes. New J. Phys. 2012, 14, 023031. [Google Scholar] [CrossRef]
- Falci, G.; La Cognata, A.; Berritta, M.; D’Arrigo, A.; Paladino, E.; Spagnolo, B. Design of a Lambda system for population transfer in superconducting nanocircuits. Phys. Rev. B 2013, 87, 214515-1–214515-13. [Google Scholar] [CrossRef]
- Paladino, E.; D’Arrigo, A.; Mastellone, A.; Falci, G. Decoherence times of universal two-qubit gates in the presence of broad-band noise. New J. Phys. 2011, 13. [Google Scholar] [CrossRef]
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ridolfo, A. Generation of Photon Pairs in the Light-Matter Ultrastrong Coupling Regime: From Casimir Radiation to Stimulated Raman Adiabatic Passage. Proceedings 2019, 12, 37. https://doi.org/10.3390/proceedings2019012037
Ridolfo A. Generation of Photon Pairs in the Light-Matter Ultrastrong Coupling Regime: From Casimir Radiation to Stimulated Raman Adiabatic Passage. Proceedings. 2019; 12(1):37. https://doi.org/10.3390/proceedings2019012037
Chicago/Turabian StyleRidolfo, Alessandro. 2019. "Generation of Photon Pairs in the Light-Matter Ultrastrong Coupling Regime: From Casimir Radiation to Stimulated Raman Adiabatic Passage" Proceedings 12, no. 1: 37. https://doi.org/10.3390/proceedings2019012037
APA StyleRidolfo, A. (2019). Generation of Photon Pairs in the Light-Matter Ultrastrong Coupling Regime: From Casimir Radiation to Stimulated Raman Adiabatic Passage. Proceedings, 12(1), 37. https://doi.org/10.3390/proceedings2019012037




