Quantum Enhanced Optical Measurements: From Ultra-High Sensitivity in Absorption Measurements to Ghost Microscopy †
Abstract
:1. Introduction
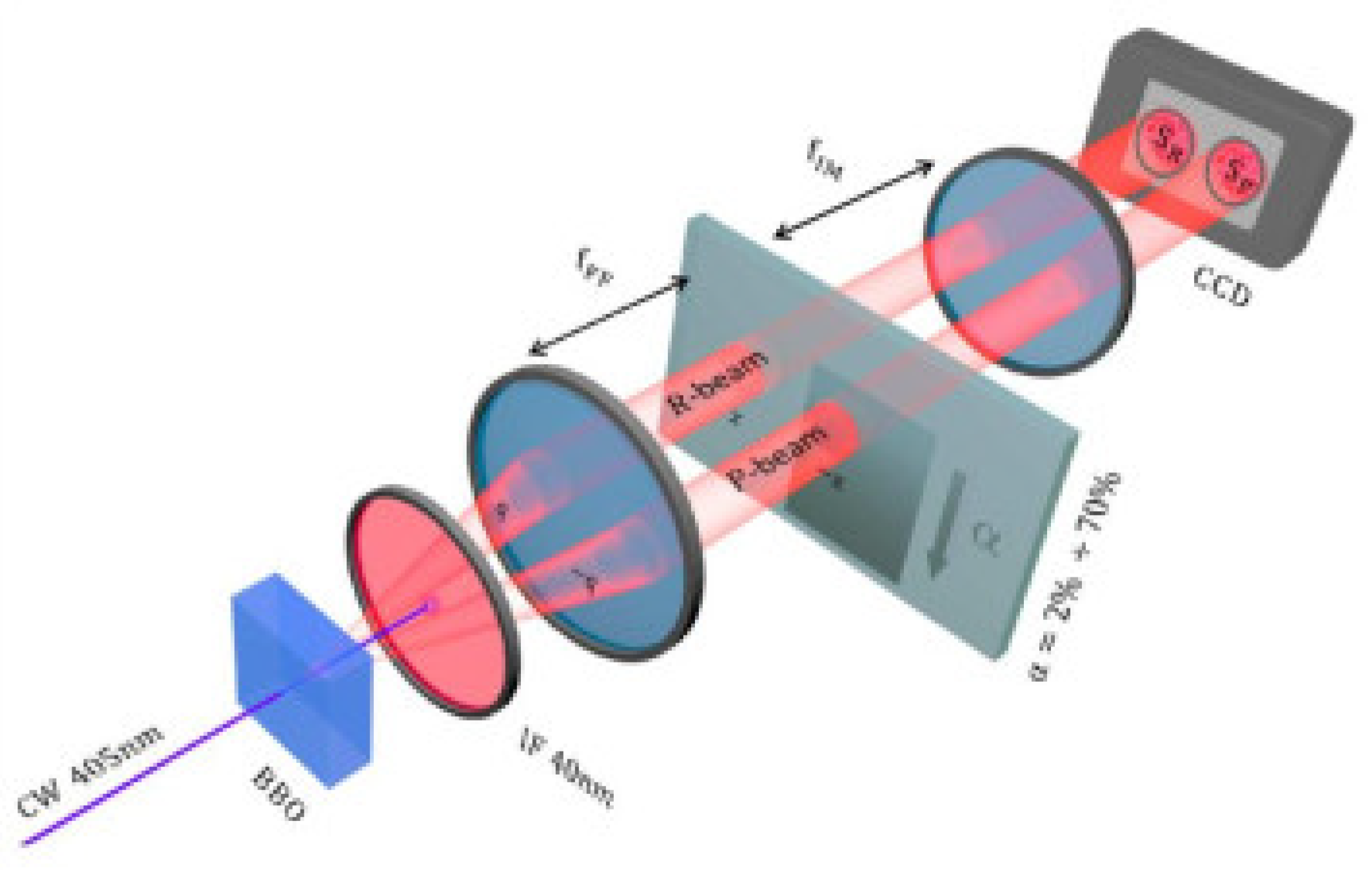
2. Absorption Measurement
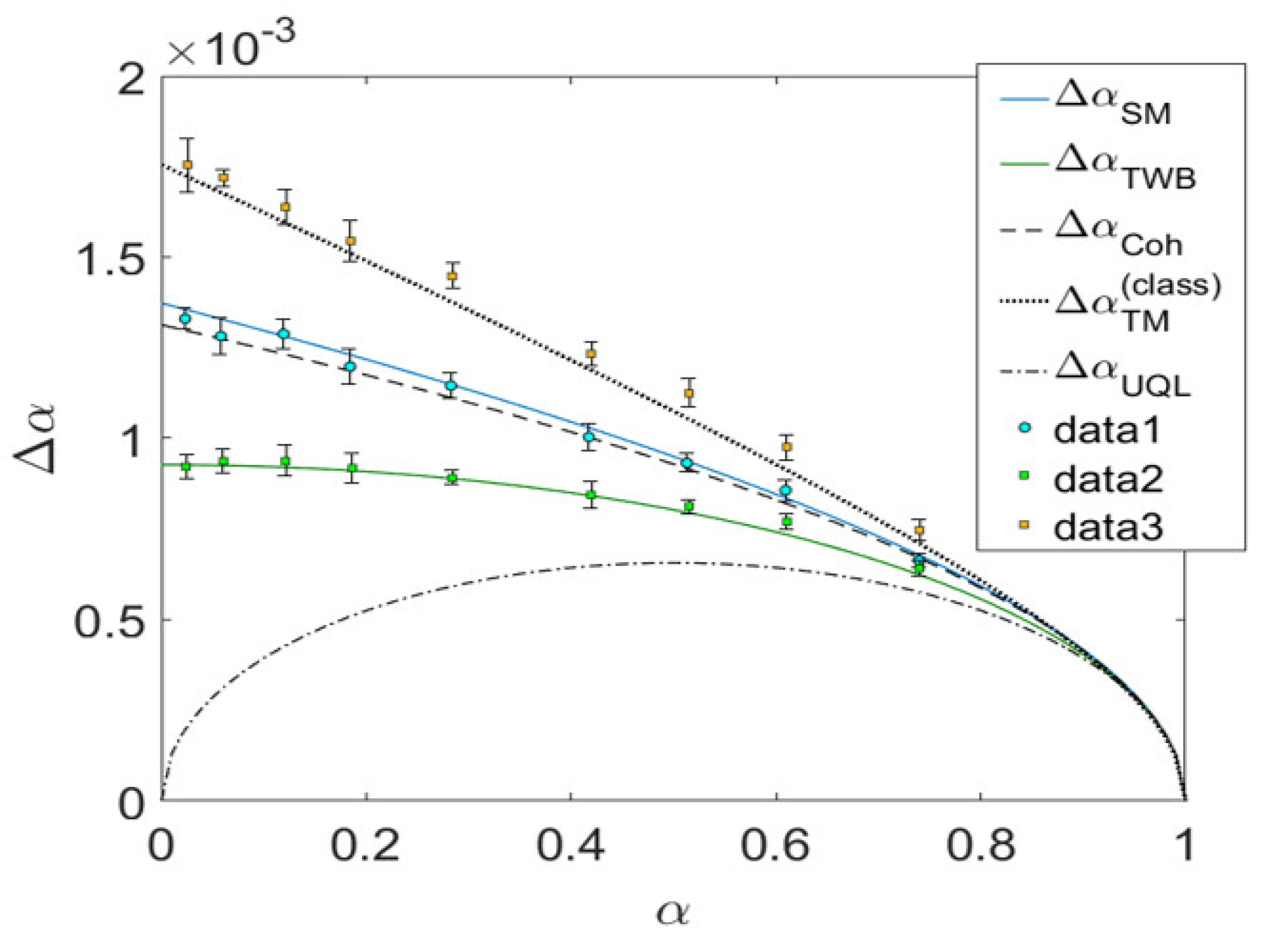
- . and are the number of photons on in two different moments, when the sample is present and when it has been removed respectively. Since the single beam is well approximated by a coherent beam (being a thermal beam with very low number of photons per mode), the uncertainty on is close to the best classical case (). Note that this strategy does not take into account possible drifts of the source, therefore two mode strategy should be preferred in real situations for having unbiased estimation.
- . and are the number of photons on and respectively and is the unbalance between the two beams, obtained from a preliminary acquisition without the sample inserted. Exploiting the correlation between the two beams it is possible to go below the classical limit, reaching the ultimate quantum limit in the ideal case of unitary efficiencies. The presence of losses leads to a detachment from the ultimate quantum limit .
- . In this case and have been chosen in order to be only classically correlated.
3. Optimized Ghost Imaging
Author Contributions
Conflicts of Interest
References
- Meda, A.; Losero, E.; Samantaray, N.; Scafirimuto, F.; Pradyumna, S.; Avella1, A.; Ruo-Berchera1, I.; Genovese, M. Photon-number correlation for quantum enhanced imaging and sensing. J. Opt. 2017, 19, 094002. [Google Scholar] [CrossRef]
- Losero, E.; Ruo-Berchera, I.; Meda, A.; Avella, A.; Genovese, M. Unbiased estimation of an optical loss at the ultimate quantum limit with twin-beams. Sci. Rep. 2018, 8, 7431. [Google Scholar] [CrossRef] [PubMed]
- Adesso, G.; Dell’Anno, F.; De Siena, S.; Illuminati, F.; Souza, L.A.M. Optimal estimation of losses at the ultimate quantum limit with non-Gaussian states. Phys. Rev. A 2009, 79, 040305. [Google Scholar] [CrossRef]
- Ferri, F.; Magatti, D.; Lugiato, L.A.; Gatti, A. Differential ghost imaging. Phys. Rev. Lett. 2010, 104, 253603. [Google Scholar] [CrossRef] [PubMed]
- Pittman, T.B.; Shih, Y.H.; Strekalov, D.V.; Sergienko, A.V. Optical imaging by means of two-photon quantum entanglement. Phys. Rev. A 1995, 52, R3429–R3432. [Google Scholar] [CrossRef] [PubMed]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Losero, E.; Ruo-Berchera, I.; Avella, A.; Meda, A.; Genovese, M. Quantum Enhanced Optical Measurements: From Ultra-High Sensitivity in Absorption Measurements to Ghost Microscopy. Proceedings 2019, 12, 14. https://doi.org/10.3390/proceedings2019012014
Losero E, Ruo-Berchera I, Avella A, Meda A, Genovese M. Quantum Enhanced Optical Measurements: From Ultra-High Sensitivity in Absorption Measurements to Ghost Microscopy. Proceedings. 2019; 12(1):14. https://doi.org/10.3390/proceedings2019012014
Chicago/Turabian StyleLosero, Elena, Ivano Ruo-Berchera, Alessio Avella, Alice Meda, and Marco Genovese. 2019. "Quantum Enhanced Optical Measurements: From Ultra-High Sensitivity in Absorption Measurements to Ghost Microscopy" Proceedings 12, no. 1: 14. https://doi.org/10.3390/proceedings2019012014
APA StyleLosero, E., Ruo-Berchera, I., Avella, A., Meda, A., & Genovese, M. (2019). Quantum Enhanced Optical Measurements: From Ultra-High Sensitivity in Absorption Measurements to Ghost Microscopy. Proceedings, 12(1), 14. https://doi.org/10.3390/proceedings2019012014





