Bulk Observables within a Hybrid Approach for Heavy Ion Collisions with SMASH Afterburner †
Abstract
:1. Introduction
2. Materials and Methods
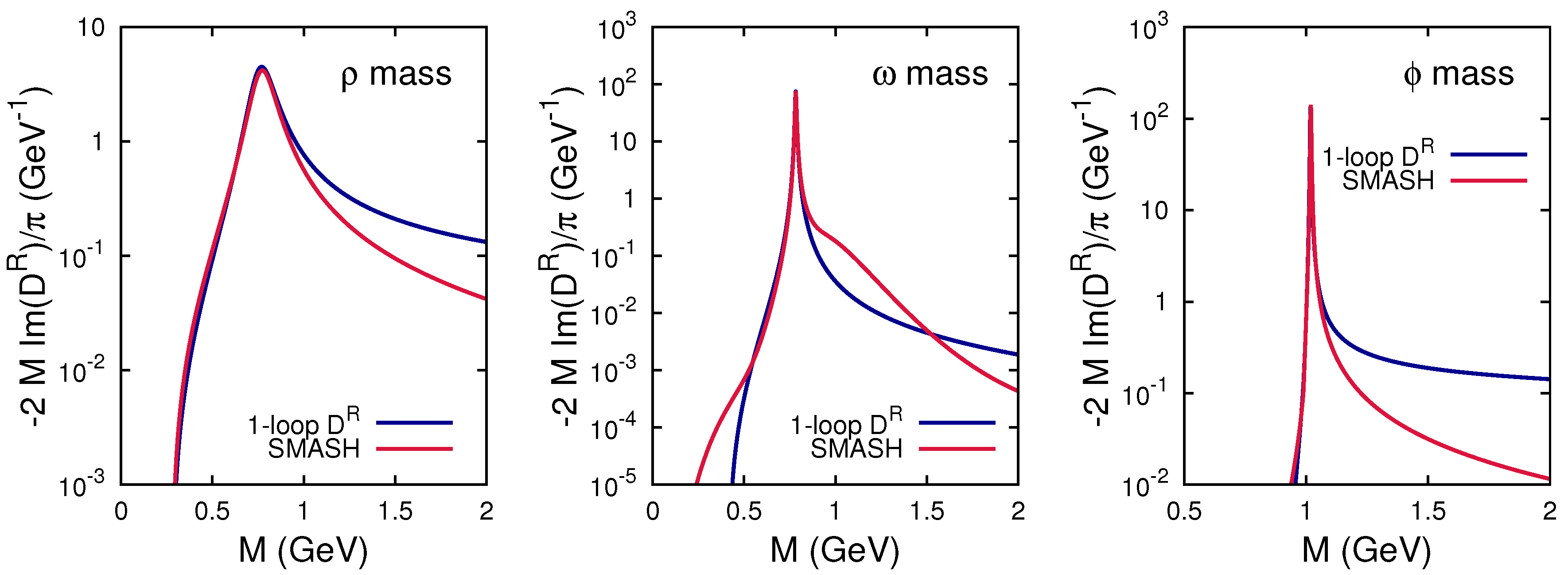
3. Results
4. Discussion
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Arsene, I.; Bearden, I.G.; Beavis, D.; Besliu, C.; Budick, B.; Bøggild, H.; Chasman, C.; Christensen, C.H.; Christiansen, P.; Cibor, J.; et al. Quark gluon plasma and color glass condensate at RHIC? The Perspective from the BRAHMS experiment. Nucl. Phys. A 2005, 757, 1–27. [Google Scholar] [CrossRef]
- Back, B.B.; Baker, M.D.; Ballintijn, M.; Barton, D.S.; Becker, B.; Betts, R.R.; Bickley, A.A.; Bindel, R.; Budzanowski, A.; Busza, W.; et al. The PHOBOS perspective on discoveries at RHIC. Nucl. Phys. A 2005, 757, 28–101. [Google Scholar] [CrossRef]
- Adams, J.; Aggarwal, M.M.; Ahammed, Z.; Amonett, J.; Anderson, B.D.; Arkhipkin, D.; Averichev, G.S.; Badyal, S.K.; Bai, Y.; Balewski, J.; et al. Experimental and theoretical challenges in the search for the quark gluon plasma: The STAR Collaboration’s critical assessment of the evidence from RHIC collisions. Nucl. Phys. A 2005, 757, 102–183. [Google Scholar] [CrossRef]
- Adcox, K.; Adler, S.S.; Afanasiev, S.; Aidala, C.; Ajitanand, N.N.; Akiba, Y.; Al-Jamel, A.; Alexander, J.; Amirikas, R.; Aoki, K.; et al. Formation of dense partonic matter in relativistic nucleus-nucleus collisions at RHIC: Experimental evaluation by the PHENIX collaboration. Nucl. Phys. A 2005, 757, 184–283. [Google Scholar] [CrossRef]
- Gyulassy, M.; McLerran, L. New forms of QCD matter discovered at RHIC. Nucl. Phys. A 2005, 750, 30–63. [Google Scholar] [CrossRef]
- Gale, C.; Jeon, S.; Schenke, B. Hydrodynamic Modeling of Heavy-Ion Collisions. Int. J. Mod. Phys. A 2013, 28, 1340011. [Google Scholar] [CrossRef]
- De Souza, R.D.; Koide, T.; Kodama, T. Hydrodynamic Approaches in Relativistic Heavy Ion Reactions. Prog. Part. Nucl. Phys. 2016, 86, 35–85. [Google Scholar] [CrossRef]
- Petersen, H.; Steinheimer, J.; Burau, G.; Bleicher, M.; Stocker, H. A Fully Integrated Transport Approach to Heavy Ion Reactions with an Intermediate Hydrodynamic Stage. Phys. Rev. C 2008, 78, 044901. [Google Scholar] [CrossRef]
- Ryu, S.; Paquet, J.-F.; Shen, C.; Denicol, G.S.; Schenke, B.; Jeon, S.; Gale, C. Importance of the Bulk Viscosity of QCD in Ultrarelativistic Heavy-Ion Collisions. Phys. Rev. Lett. 2015, 115, 132301. [Google Scholar] [CrossRef]
- Ryu, S.; Paquet, J.F.; Shen, C.; Denicol, G.; Schenke, B.; Jeon, S.; Gale, C. Effects of bulk viscosity and hadronic rescattering in heavy ion collisions at energies available at the BNL Relativistic Heavy Ion Collider and at the CERN Large Hadron Collider. Phys. Rev. C 2018, 97, 034910. [Google Scholar] [CrossRef]
- Schenke, B.; Tribedy, P.; Venugopalan, R. Fluctuating Glasma initial conditions and flow in heavy ion collisions. Phys. Rev. Lett. 2012, 108, 252301. [Google Scholar] [CrossRef]
- Schenke, B.; Jeon, S.; Gale, C. (3+1)D hydrodynamic simulation of relativistic heavy-ion collisions. Phys. Rev. C 2010, 82, 014903. [Google Scholar] [CrossRef]
- Schenke, B.; Jeon, S.; Gale, C. Elliptic and triangular flow in event-by-event (3+1)D viscous hydrodynamics. Phys. Rev. Lett. 2011, 106, 042301. [Google Scholar] [CrossRef]
- Cooper, F.; Frye, G. Comment on the Single Particle Distribution in the Hydrodynamic and Statistical Thermodynamic Models of Multiparticle Production. Phys. Rev. D 1974, 10, 186. [Google Scholar] [CrossRef]
- Weil, J.; Steinberg, V.; Staudenmaier, J.; Pang, L.G.; Oliinychenko, D.; Mohs, J.; Kretz, M.; Kehrenberg, T.; Goldschmidt, A.; Bäuchle, B.; et al. Particle production and equilibrium properties within a new hadron transport approach for heavy-ion collisions. Phys. Rev. C 2016, 94, 054905. [Google Scholar] [CrossRef]
- Denicol, G.S.; Jeon, S.; Gale, C. Transport Coefficients of Bulk Viscous Pressure in the 14-moment approximation. Phys. Rev. C 2014, 90, 024912. [Google Scholar] [CrossRef]
- Denicol, G.S.; Niemi, H.; Molnar, E.; Rischke, D.H. Derivation of transient relativistic fluid dynamics from the Boltzmann equation. Phys. Rev. D 2012, 85, 114047, Erratum: [Phys. Rev. D 2015, 91, 039902, doi: 10.1103/PhysRevD.91.039902.]. [Google Scholar] [CrossRef]
- Huovinen, P.; Petreczky, P. QCD Equation of State and Hadron Resonance Gas. Nucl. Phys. A 2010, 827, 26–53. [Google Scholar] [CrossRef]
- Dusling, K.; Moore, G.D.; Teaney, D. Radiative energy loss and v(2) spectra for viscous hydrodynamics. Phys. Rev. C 2010, 81, 034907. [Google Scholar] [CrossRef]
- Bozek, P. Bulk and shear viscosities of matter created in relativistic heavy-ion collisions. Phys. Rev. C 2010, 81, 034909. [Google Scholar] [CrossRef]
- Vujanovic, G.; Young, C.; Schenke, B.; Rapp, R.; Jeon, S.; Gale, C. Dilepton emission in high-energy heavy-ion collisions with viscous hydrodynamics. Phys. Rev. C 2014, 89, 034904. [Google Scholar] [CrossRef]
- Manley, D.M.; Saleski, E.M. Multichannel resonance parametrization of pi N scattering amplitudes. Phys. Rev. D 1992, 45, 4002. [Google Scholar] [CrossRef]
- Adler, S.S.; Afanasiev, S.; Aidala, C.; Ajitanand, N.N.; Akiba, Y.; Alexander, J.; Amirikas, R.; Aphecetche, L.; Aronson, S.H.; Averbeck, R.; et al. Identified charged particle spectra and yields in Au+Au collisions at S(NN)**1/2 = 200-GeV. Phys. Rev. C 2004, 69, 034909. [Google Scholar] [CrossRef]
- Adare, A.; Afanasiev, S.; Aidala, C.; Ajitanand, N.N.; Akiba, Y.; Al-Bataineh, H.; Alexander, J.; Aoki, K.; Aramaki, Y.; Atomssa, E.T.; et al. Measurement of the higher-order anisotropic flow coefficients for identified hadrons in Au+Au collisions at = 200 GeV. Phys. Rev. C 2016, 93, 051902. [Google Scholar] [CrossRef]
- Adams, J.; Aggarwal, M.M.; Ahammed, Z.; Amonett, J.; Anderson, B.D.; Arkhipkin, D.; Averichev, G.S.; Badyal, S.K.; Bai, Y.; Balewski, J.; et al. Azimuthal anisotropy in Au+Au collisions at s(NN)**(1/2) = 200-GeV. Phys. Rev. C 2005, 72, 014904. [Google Scholar] [CrossRef]
- Bass, S.A.; Belkacem, M.; Bleicher, M.; Brandstetter, M.; Bravina, L.; Ernst, C.; Gerland, L.; Hofmann, M.; Hofmann, S.; Konopka, J.; et al. Microscopic models for ultrarelativistic heavy ion collisions. Prog. Part. Nucl. Phys. 1998, 41, 255. [Google Scholar] [CrossRef]
- Bleicher, M.; Zabrodin, E.; Spieles, C.; Bass, S.A.; Ernst, C.; Soff, S.; Bravina, L.; Belkacem, M.; Weber, H.; Stöcker, H.; et al. Relativistic hadron hadron collisions in the ultrarelativistic quantum molecular dynamics model. J. Phys. G 1999, 25, 1859. [Google Scholar] [CrossRef]
- Pan, Y.; Pratt, S. Baryon annihilation and regeneration in heavy ion collisions. Phys. Rev. C 2014, 89, 044911. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ryu, S.; Staudenmaier, J.; Elfner, H. Bulk Observables within a Hybrid Approach for Heavy Ion Collisions with SMASH Afterburner. Proceedings 2019, 10, 44. https://doi.org/10.3390/proceedings2019010044
Ryu S, Staudenmaier J, Elfner H. Bulk Observables within a Hybrid Approach for Heavy Ion Collisions with SMASH Afterburner. Proceedings. 2019; 10(1):44. https://doi.org/10.3390/proceedings2019010044
Chicago/Turabian StyleRyu, Sangwook, Jan Staudenmaier, and Hannah Elfner. 2019. "Bulk Observables within a Hybrid Approach for Heavy Ion Collisions with SMASH Afterburner" Proceedings 10, no. 1: 44. https://doi.org/10.3390/proceedings2019010044
APA StyleRyu, S., Staudenmaier, J., & Elfner, H. (2019). Bulk Observables within a Hybrid Approach for Heavy Ion Collisions with SMASH Afterburner. Proceedings, 10(1), 44. https://doi.org/10.3390/proceedings2019010044




