Mechanical Characterization of (La,Sr)MnO3 Microbridges for Thermometric Applications †
Abstract
:1. Introduction
2. Material and Methods
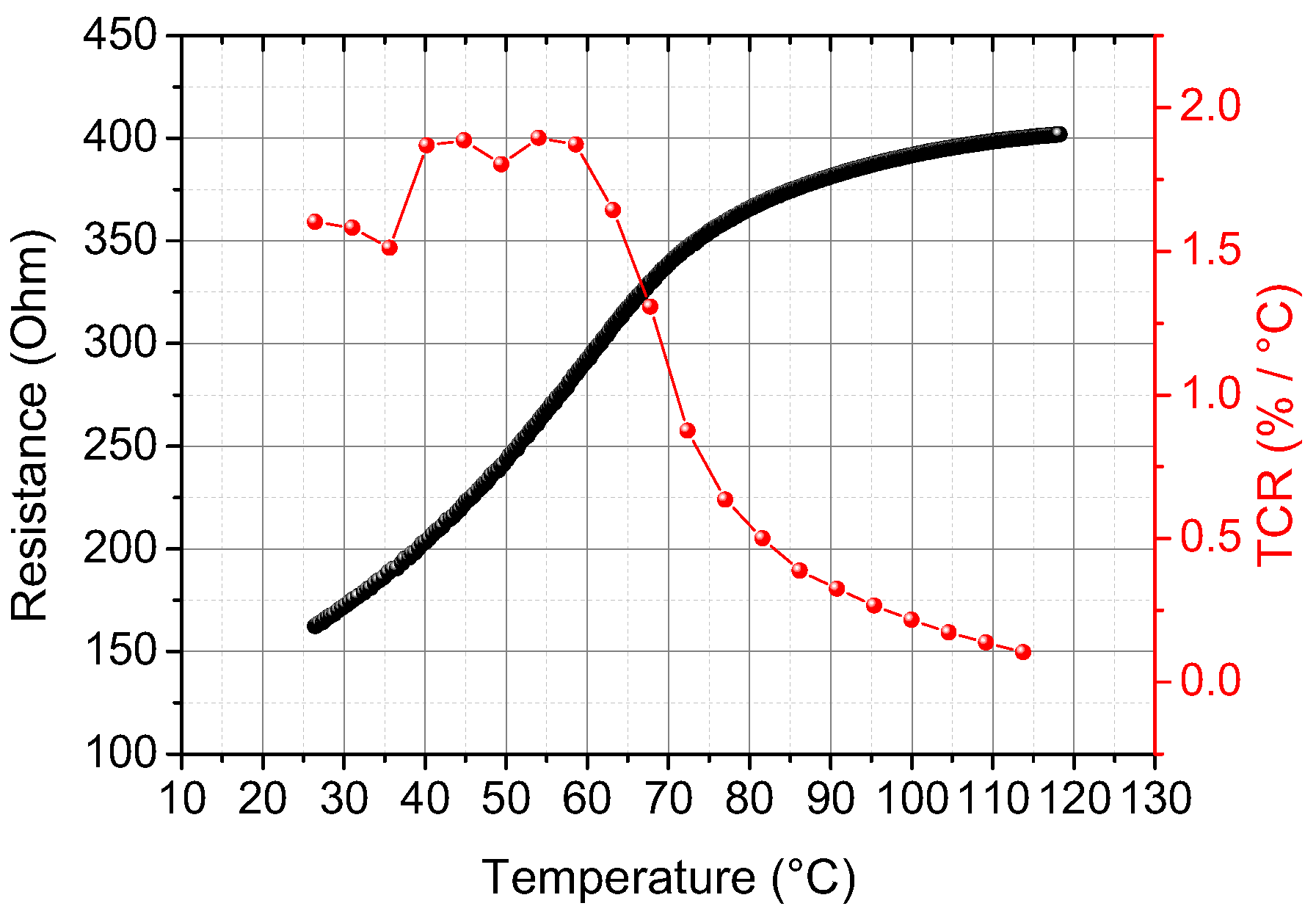
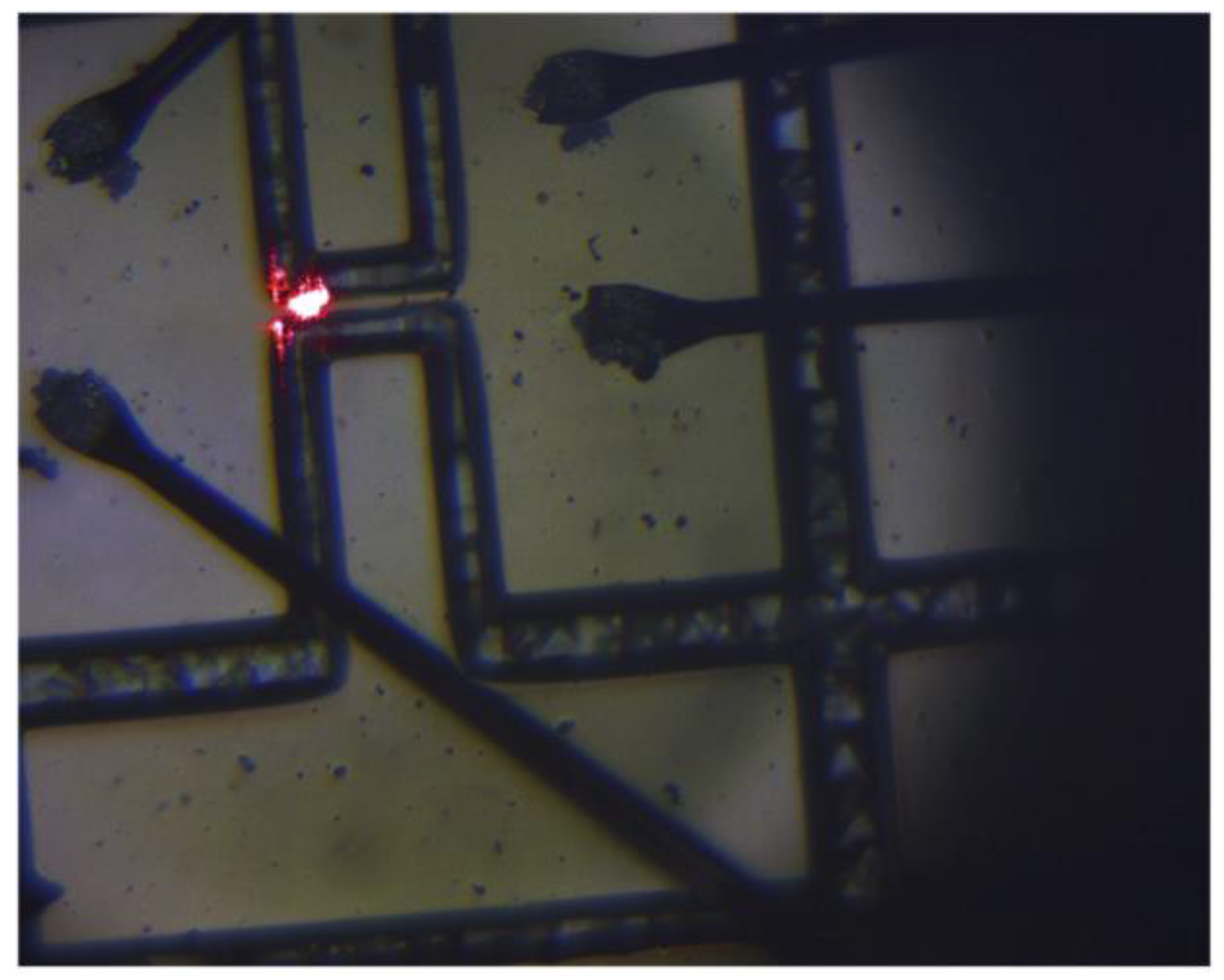
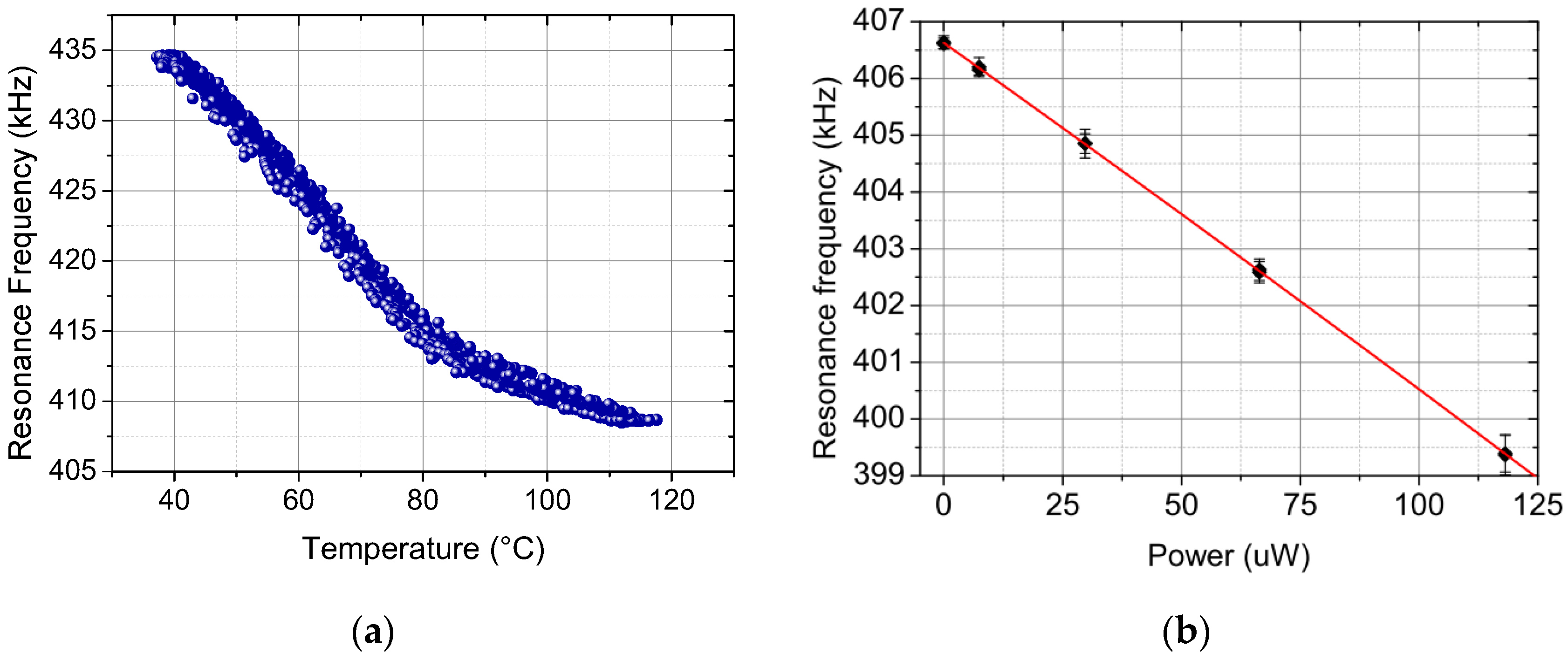
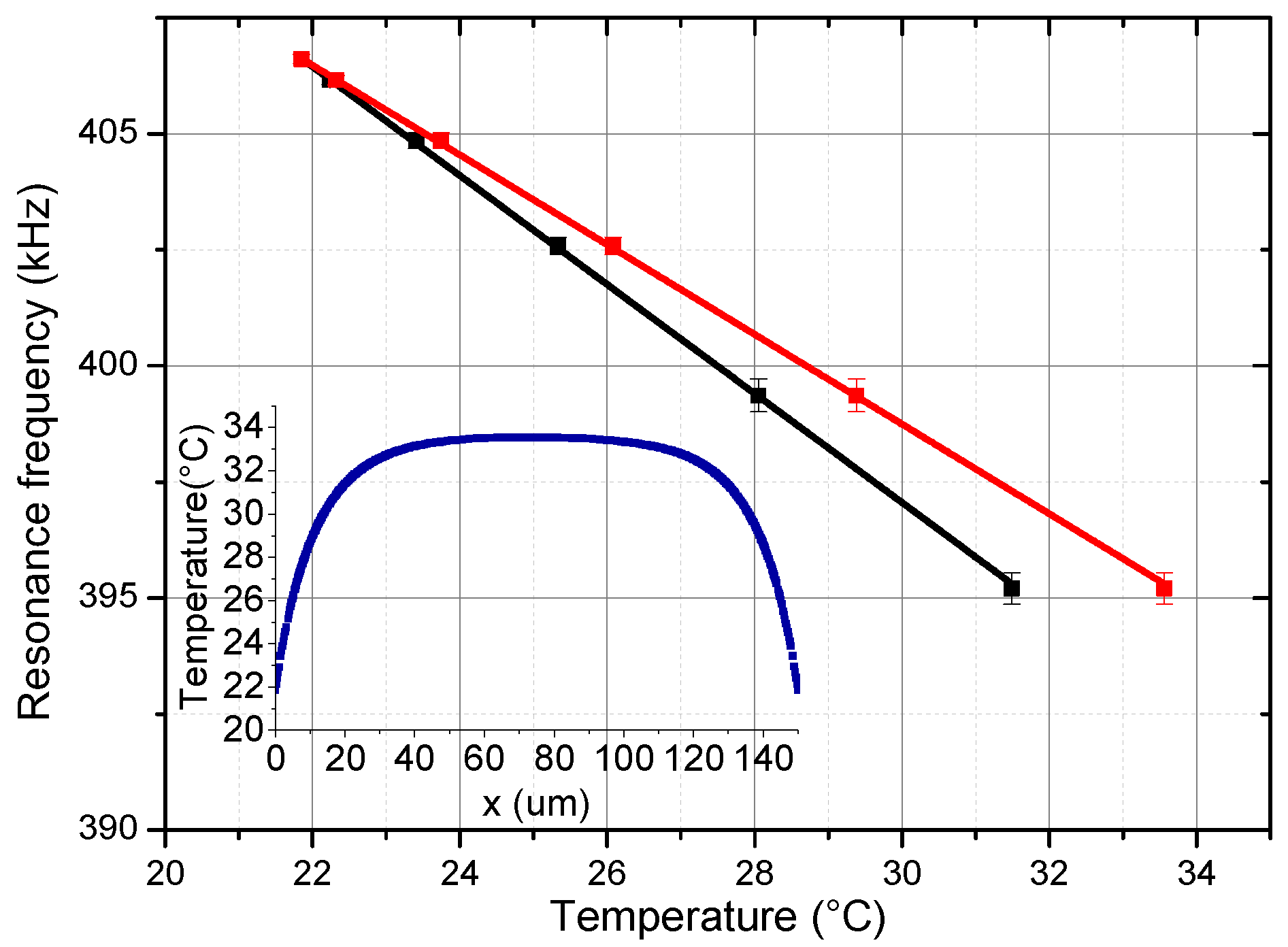
3. Results
4. Discussion
Acknowledgments
Conflicts of Interest
References
- Pellegrino, L.; Biasotti, M.; Bellingeri, E.; Bernini, C.; Siri, A.S.; Marré, D. All-oxide crystalline microelectromechanical systems: Bending the functionalities of transition-metal oxide thin films. Adv. Mater. 2009, 21, 2377–2381. [Google Scholar] [CrossRef]
- Manca, N.; Pellegrino, L.; Kanki, T.; Yamasaki, S.; Tanaka, H.; Siri, A.S.; Marré, D. Programmable mechanical resonances in MEMS by localized Joule heating of phase change materials. Adv. Mater. 2013, 25, 6430–6435. [Google Scholar] [CrossRef] [PubMed]
- Haghiri-Gosnet, A.-M.; Renard, J.-P. CMR Manganites: Physics, thin films and devices. J. Phys. D Appl. Phys. 2003, 36, R127–R150. [Google Scholar] [CrossRef]
- Liu, S.; Guillet, B.; Aryan, A.; Adamo, C.; Fur, C.; Routoure, J.-M.; Lemarié, F.; Schlom, D.G.; Méchin, L. La0.7Sr0.3MnO3 suspended microbridges for uncooled bolometers made using reactive ion etching of the silicon substrates. Microelectron. Eng. 2013, 111, 101–104. [Google Scholar] [CrossRef]
- Le Bourdais, D.; Agnus, G.; Maroutian, T.; Pillard, V.; Aubert, P.; Bachelet, R.; Saint-Girons, G.; Vilquin, B.; Lefeuvre, E.; Lecoeur, P. Epitaxial manganite freestanding bridges for low power pressure sensors. J. Appl. Phys. 2015, 118, 124509. [Google Scholar] [CrossRef]
- Ceriale, V.; Pellegrino, L.; Manca, N.; Marré, D. Electro-thermal bistability in (La0.7Sr0.3)MnO3 suspended microbridges: Thermal characterization and transient analysis. J. Appl. Phys. 2014, 115, 054511. [Google Scholar] [CrossRef]
- Manca, N.; Pellegrino, L.; Marré, D. Reversible oxygen vacancies doping in (La0.7Sr0.3)MnO3 microbridges by combined self-heating and electromigration. Appl. Phys. Lett. 2015, 106, 203502. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Remaggi, F.; Pellegrino, L.; Manca, N.; Bernini, C.; Marré, D. Mechanical Characterization of (La,Sr)MnO3 Microbridges for Thermometric Applications. Proceedings 2017, 1, 365. https://doi.org/10.3390/proceedings1040365
Remaggi F, Pellegrino L, Manca N, Bernini C, Marré D. Mechanical Characterization of (La,Sr)MnO3 Microbridges for Thermometric Applications. Proceedings. 2017; 1(4):365. https://doi.org/10.3390/proceedings1040365
Chicago/Turabian StyleRemaggi, Federico, Luca Pellegrino, Nicola Manca, Cristina Bernini, and Daniele Marré. 2017. "Mechanical Characterization of (La,Sr)MnO3 Microbridges for Thermometric Applications" Proceedings 1, no. 4: 365. https://doi.org/10.3390/proceedings1040365
APA StyleRemaggi, F., Pellegrino, L., Manca, N., Bernini, C., & Marré, D. (2017). Mechanical Characterization of (La,Sr)MnO3 Microbridges for Thermometric Applications. Proceedings, 1(4), 365. https://doi.org/10.3390/proceedings1040365




