Analysis on Chattering Phenomena by the Tilt of the Proof Mass in MEMS Switch †
Abstract
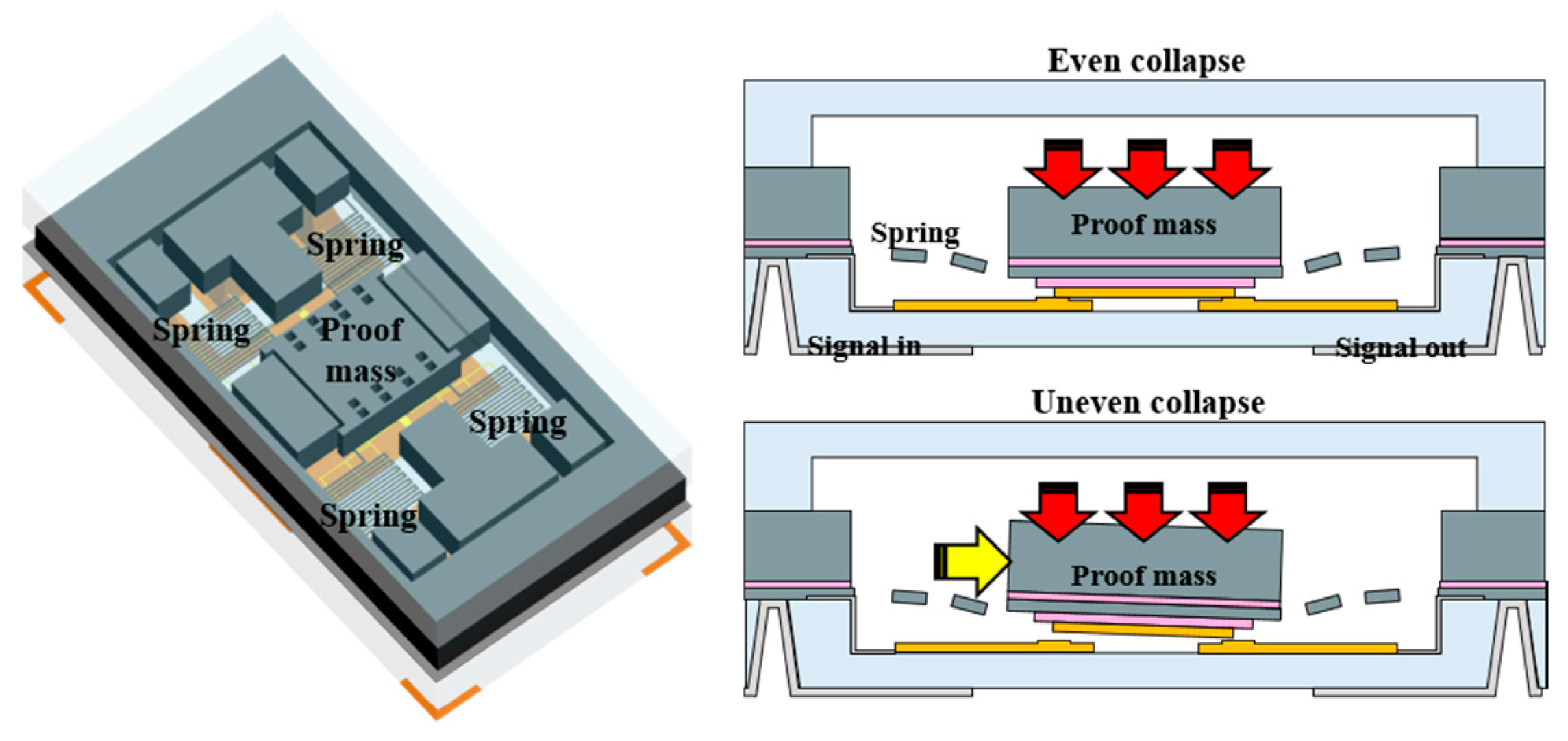
:1. Introduction
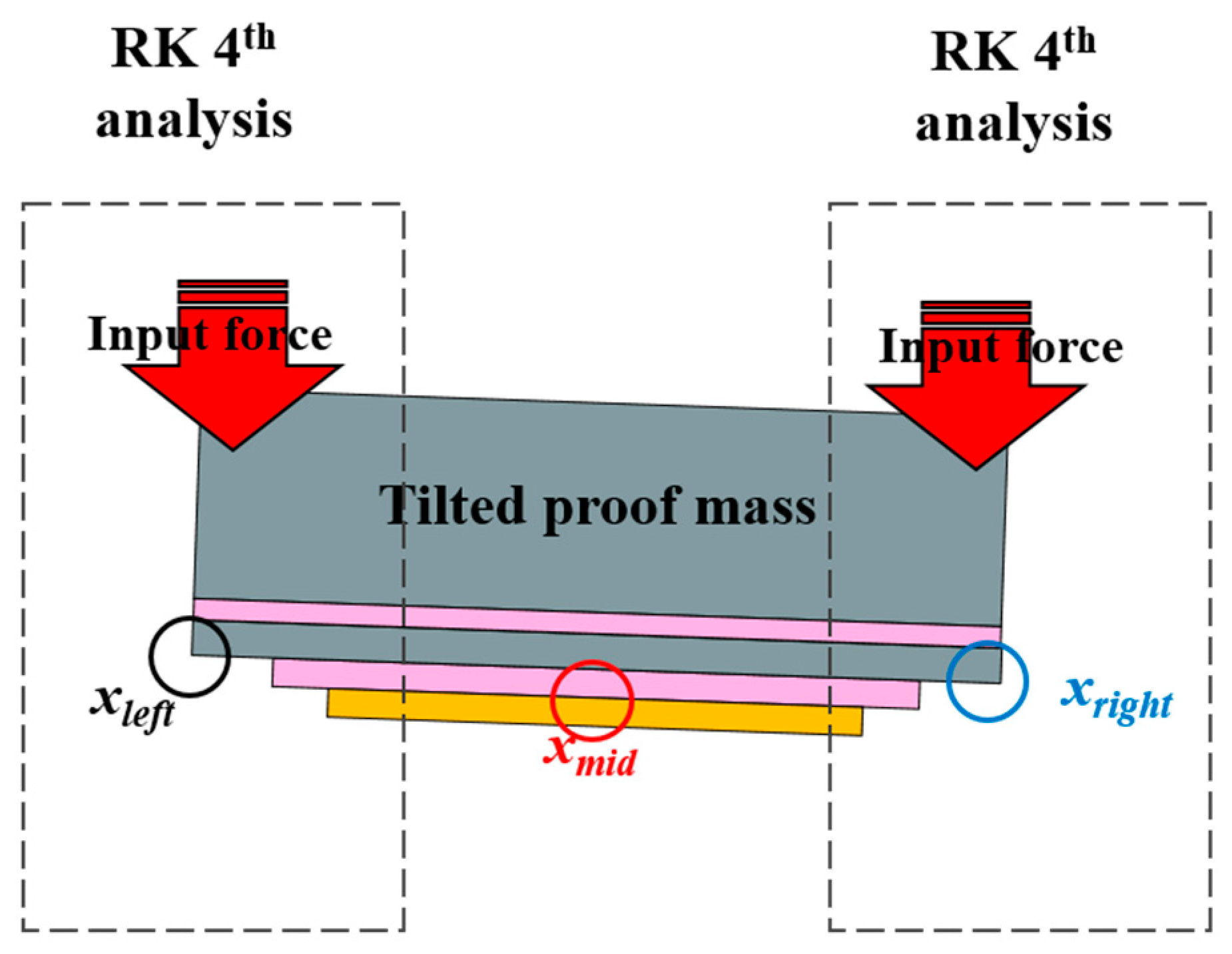
2. Analysis Methods
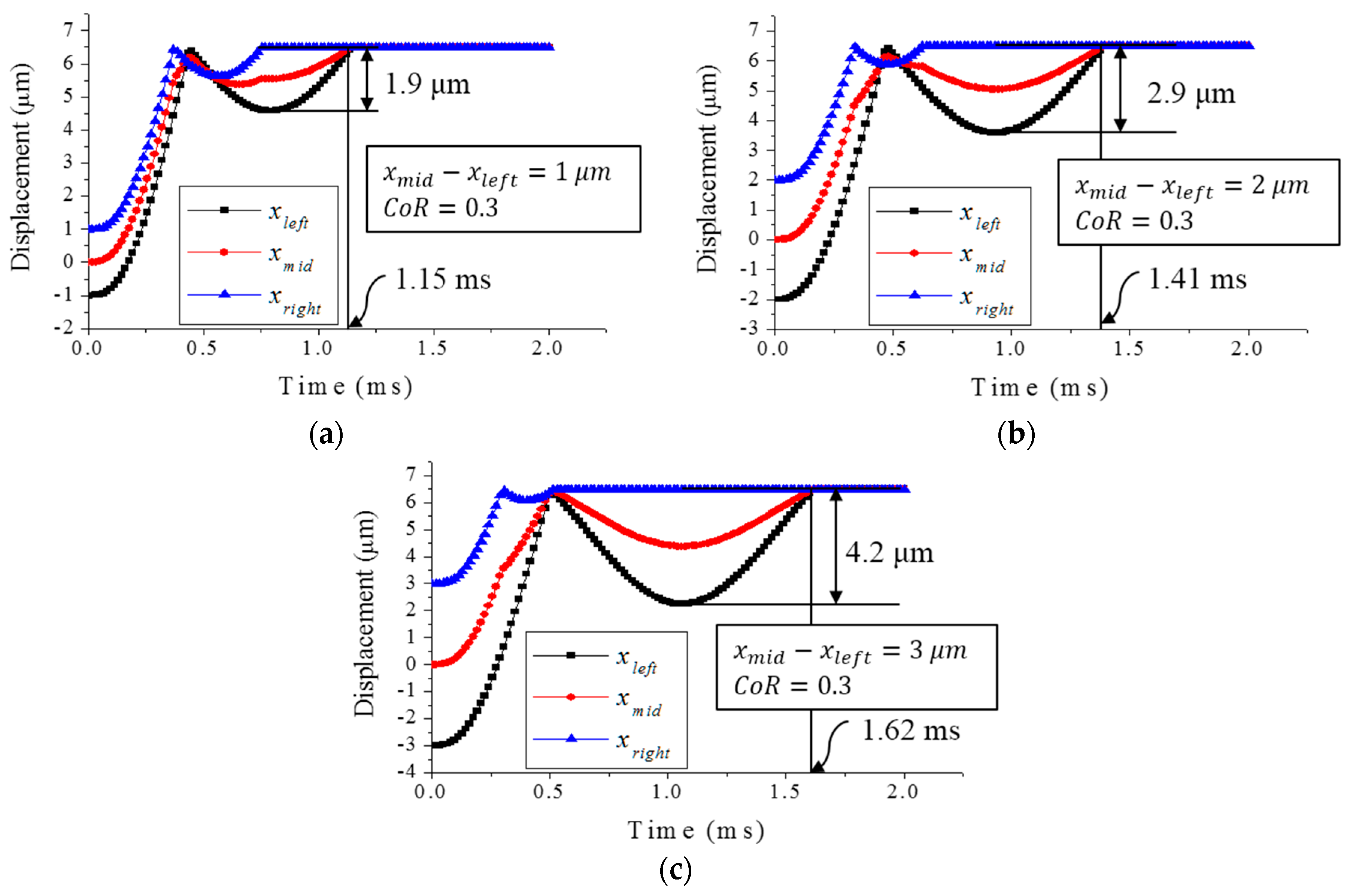
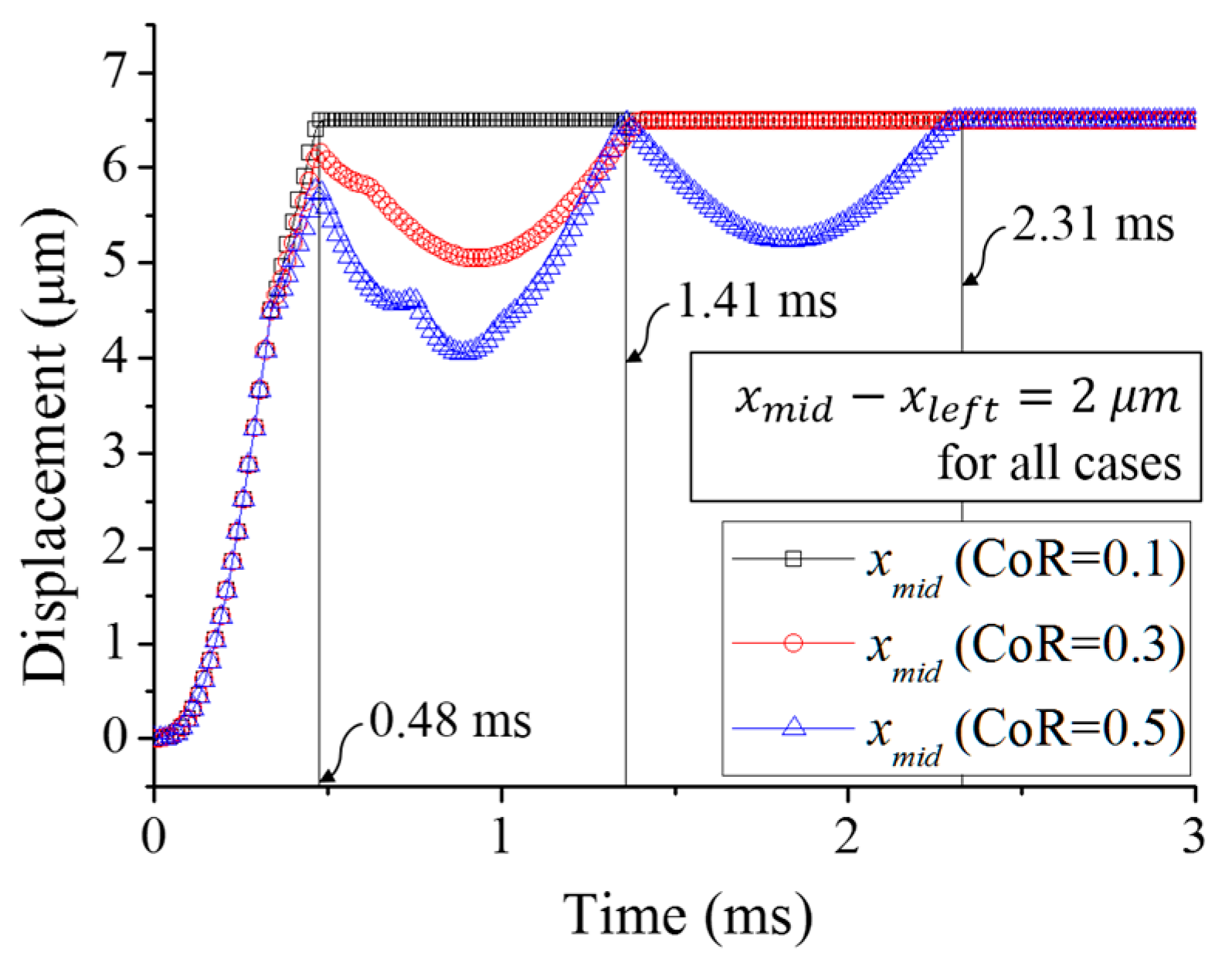
3. Results
4. Discussion
5. Conclusions
Conflicts of Interest
References
- Barbour, N.M. Inertial Navigation Sensors DTIC Document. Available online: http://oai.dtic.mil/oai/oai?verb=getRecord&metadataPrefix=html&identifier=ADA422180 (accessed on 7 August 2017).
- Froyum, K.; Goepfert, S.; Henrickson, J.; Thorland, J. Honeywell micro electro mechanical systems (MEMS) inertial measurement unit (IMU). In Proceedings of the 2012 IEEE/ION Position Location and Navigation Symposium (PLANS), Myrtle Beach, SC, USA, 23–26 April 2012; pp. 831–836. [Google Scholar]
- Nie, W.-R.; Xi, Z.-W.; Xue, W.-Q.; Zhou, Z.-J. Study on inertial response performance of a micro electrical switch for fuze. Def. Technol. 2013, 9, 187–192. [Google Scholar] [CrossRef]
- Robinson, C.H.; Wood, R.H.; Gelak, M.R.; Hoang, T.Q.; Smith, G.L. Ultra-miniature Electro-Mechanical Safety and Arming Device. Google Patents US8276515 B1, 28 May 2013. [Google Scholar]
- Hwang, J.; Ryu, D.; Park, C.; Jang, S.-G.; Lee, C.-I.; Kim, Y.-K. Design and Fabrication of Silicon-based MEMS Acceleration Switch Working Lower than 10 g. J. Micromech. Microeng. 2017, 27, 065009. [Google Scholar] [CrossRef]
- William, H.; Saul, A.T.; William, T.V.; Brian, P.F. Numerical Recipes: The Art of Scientific Computing; Cambridge University Press: Cambridge, UK, 1987; pp. 120–122. [Google Scholar]
| Proof mass [kg] (w × l × t) [μm3] | 3.07 × 10−7 (910 × 910 × 200) |
| Spring constant [N/m] | 4.5 |
| Initial gap [μm] | 6.5 |
| Input force [N] | 3.61× 10-5 |
| Coefficient of Restitution | 0.1/0.3/0.5 |
| Vertical height between center and end of the proof mass ( − ) [μm] | 1/2/3 |
| Tilted angle [°] | 1.14/2.29/3.43 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hwang, J.-M.; Hwang, J.; Ryu, D.; Jang, S.-G.; Kim, Y.-K. Analysis on Chattering Phenomena by the Tilt of the Proof Mass in MEMS Switch. Proceedings 2017, 1, 349. https://doi.org/10.3390/proceedings1040349
Hwang J-M, Hwang J, Ryu D, Jang S-G, Kim Y-K. Analysis on Chattering Phenomena by the Tilt of the Proof Mass in MEMS Switch. Proceedings. 2017; 1(4):349. https://doi.org/10.3390/proceedings1040349
Chicago/Turabian StyleHwang, Jung-Min, Jeongki Hwang, Daeho Ryu, Seung-Gyo Jang, and Yong-Kweon Kim. 2017. "Analysis on Chattering Phenomena by the Tilt of the Proof Mass in MEMS Switch" Proceedings 1, no. 4: 349. https://doi.org/10.3390/proceedings1040349
APA StyleHwang, J.-M., Hwang, J., Ryu, D., Jang, S.-G., & Kim, Y.-K. (2017). Analysis on Chattering Phenomena by the Tilt of the Proof Mass in MEMS Switch. Proceedings, 1(4), 349. https://doi.org/10.3390/proceedings1040349





