An 8D Hyperchaotic System of Fractional-Order Systems Using the Memory Effect of Grünwald–Letnikov Derivatives

Abstract

We utilize Lyapunov exponents to quantitatively assess the hyperchaos and categorize the limit sets of complex dynamical systems. While there are numerous methods for computing Lyapunov exponents in integer-order systems, these methods are not suitable for fractional-order systems because of the nonlocal characteristics of fractional-order derivatives. This paper introduces innovative eight-dimensional chaotic systems that investigate fractional-order dynamics. These systems exploit the memory effect inherent in the Grünwald–Letnikov (G-L) derivative. This approach enhances the system’s applicability and compatibility with traditional integer-order systems. An 8D Chen’s fractional-order system is utilized to showcase the effectiveness of the presented methodology for hyperchaotic systems. The simulation results demonstrate that the proposed algorithm outperforms existing algorithms in both accuracy and precision. Moreover, the study utilizes the 0–1 Test for Chaos, Kolmogorov–Sinai (KS) entropy, the Kaplan–Yorke dimension, and the Perron Effect to analyze the proposed eight-dimensional fractional-order system. These additional metrics offer a thorough insight into the system’s chaotic behavior and stability characteristics.

1. Introduction

The investigation of chaotic behaviors within both integer-order and fractional-order frameworks constitutes a significant research domain in dynamical systems. Chaotic dynamics are characterized by extreme sensitivity to initial conditions and long-term unpredictability, making them valuable in various fields, including cryptography, secure communications, and physics [1,2]. Traditional chaotic models typically employ integer-order differential equations; however, fractional-order chaotic systems, which incorporate derivatives of noninteger orders, have gained increased attention for their superior ability to accurately represent real-world phenomena [3].

Ordinary differential equations (ODEs) have recently been generalized to include fractional differential equations (FDEs). These fractional equations are believed to provide improved accuracy and lower computational costs compared to their ODE counterparts.

The classical fractional derivative, such as the Riemann–Liouville fractional derivative [4] of order $\alpha >0$ of a function $f(t)$, is defined as follows:

$$\begin{array}{c}\hfill D_t^{\alpha }f(t)={\displaystyle \frac{1}{\Gamma (n-\alpha )}}{\displaystyle \frac{d^n}{d{t}^n}}{\int }_a^t{\displaystyle \frac{f(\tau )}{{(t-\tau )}^{\alpha -n+1}}}d\tau ,\end{array}$$

where $\Gamma (·)$ is the Gamma function—defined as $\Gamma (z)={\int }_0^{\infty }{x}^{z-1}{e}^{-x}dx$, $n=\lceil \alpha \rceil$ is the smallest integer greater than or equal to $\alpha$, and a is the lower limit of the integral—often set to 0 or $-\infty$.

The conformable derivative [5] of a function $f(t)$ of order $\alpha$ is defined as follows:

$$T_{\alpha }(f)(t)=\underset{ϵ\to 0}{lim}{\displaystyle \frac{f(t+ϵt^{1-\alpha })-f(t)}{ϵ}}.$$

Alternatively, it can be written as

$$T_{\alpha }(f)(t)=t^{1-\alpha }{\displaystyle \frac{df(t)}{dt}},t>0,\alpha \in (0,1],$$

where $\alpha$ is the fractional order of the derivative—with $0<\alpha \le 1$, $ϵ$ is an infinitesimally small increment, and $t^{1-\alpha }$ scales the ordinary derivative to account for the fractional order.

The Atangana–Baleanu fractional derivative [6] in the Caputo sense is given by

$$\begin{array}{c}\hfill {}^{ABC}D_t^{\alpha }f(t)={\displaystyle \frac{B(\alpha )}{1-\alpha }}{\int }_0^t{E}_{\alpha }\left(-{\displaystyle \frac{\alpha {(t-\tau )}^{\alpha }}{1-\alpha }}\right){\displaystyle \frac{df(\tau )}{d\tau }}d\tau ,(0<\alpha <1),\end{array}$$

where $B(\alpha )=1-\alpha$, and the Mittag–Leffler function is defined by

$$\begin{array}{c}\hfill E_{\alpha }(z)=\sum _{k=0}^{\infty }{\displaystyle \frac{z^k}{\Gamma (\alpha k+1)}},\end{array}$$

where z is the argument of the Mittag–Leffler function.

Fractional-order derivatives differ from their integer-order counterparts due to their nonlocal and memory-dependent characteristics. This property allows fractional-order systems to exhibit more complex dynamics than integer-order systems [7]. Among the various definitions of fractional derivatives, the Grünwald–Letnikov derivative is particularly notable for its discrete nature and ease of implementation, making it well suited for numerical simulations of chaotic systems [8,9].

The Grünwald–Letnikov derivative is a concept in fractional calculus that generalizes the traditional notion of differentiation to fractional orders (FOs). This derivative is defined by modifying the classical limit definition of a derivative, incorporating an integral operator with a variable order of differentiation. A comprehensive discrete scheme is introduced for the numerical analysis of highly nonlinear, time-dependent fractional parabolic problems, employing the Grünwald–Letnikov (G-L) method for time discretization [10].

Given a function $f:\mathbb{R}\to \mathbb{R}$, a fractional derivative of order $\alpha$ at a point x, as formulated by Grünwald and Letnikov, is expressed as follows:

$$\begin{array}{c}\hfill D^{\alpha }f(x)=\underset{h\to 0}{lim}{\displaystyle \frac{1}{h^{\alpha }}}\sum _{m=0}^{\infty }{(-1)}^m\left({\displaystyle \genfrac{}{}{0pt}{}{\alpha }{m}}\right)f(x-mh),\end{array}$$

where $\left({\displaystyle \genfrac{}{}{0pt}{}{\alpha }{m}}\right)$ represents the binomial coefficient generalized to fractional $\alpha$, which is defined by

$$\begin{array}{c}\hfill \left({\displaystyle \genfrac{}{}{0pt}{}{\alpha }{m}}\right)={\displaystyle \frac{\alpha (\alpha -1)\dots (\alpha -m+1)}{m!}}.\end{array}$$

This definition is robust and extends the classical derivative by considering the sum of increments h scaled by fractional powers. It provides a framework for analyzing functions whose behaviors are not adequately captured by integer-order derivatives. An important benefit of this algorithm is that it illustrates how the fractional-order values of $\alpha$ significantly influence the accuracy of the numerical results and the associated computational costs [11]. However, several modeling studies have investigated the application of fractional-order derivatives, as discussed in [12,13] and the references therein.

Lyapunov exponents (LEs) are crucial in the study of dynamical systems, as they quantify the rate at which nearby trajectories diverge, providing insights into the stability and chaotic behavior of the system. Computing LEs for fractional-order systems presents challenges due to the nonlocal characteristics of fractional derivatives, rendering traditional methods for integer-order systems ineffective [14].

LEs were first proposed by Oseledets [15] in his theorem known as the multiplicative ergodic theorem. Expanding on Oseledets’s work, Benettin et al. [16] were the pioneers in developing a method to compute the Lyapunov exponents of dynamical systems. Afterwards, Wolf et al. [17] refined Benettin’s approach and introduced a technique for estimating Lyapunov exponents from time series data using Takens’s technique [18], which has since become extensively applied in experimental research. Over the past four decades, various approaches to calculating LEs have been developed, including methods based on governing equations [19,20] and techniques for estimation from time series [21,22].

Moreover, various perturbation techniques, including the perturbation vectors technique [23], the cloned dynamics technique [24], and the synchronization technique [25], have been developed by researchers. These approaches provided methods to directly compute the Jacobian matrix or solve variational equations, making them suitable for a broader range of applications, including nonsmooth systems that typically have poorly conditioned Jacobian matrices. Currently, these techniques have been successfully utilized for dynamical characterization across multiple research areas [26,27,28,29]. Fractional calculus (FC), a long-established research area of calculus, initially saw more advanced research in the field of pure mathematics compared to classical calculus, with fewer applications in the research fields of physics, as summarized by Valério et al. [30].

In recent decades, there has been a growing interest among researchers in the interdisciplinary applications of fractional calculus (FC). This interest stems from the ability of FC to model various problems in an innovative manner, including epidemic modeling [31,32], image encryption [33,34], and the mechanical behavior of viscoelastic materials [35,36,37]. Moreover, Sun et al. [38] and Diethelm et al. [39] have analyzed the application of fractional calculus in the fields of engineering and physics, respectively. Although solutions to fractional-order DEs (FODEs) do not define dynamical systems in the traditional semigroup sense [40], this does not preclude the possibility of establishing a relationship between FODEs and their phase flows in a manner analogous to integer-order equations.

As an indicator of the divergence or convergence rate of change of phase trajectories, the Lyapunov exponent (LE) remains a crucial mathematical tool for examining the dynamic behavior of FOs. Li et al. [41] provided a formal mathematical formulation of Lyapunov exponent for FOs and introduced a method to establish their bounds. Furthermore, novel approaches, such as time series algorithms [42,43] and the revised Benettin–Wolf method [44], have been introduced to compute LEs in FOs. These advancements have validated the use of LEs for assessing stability, fractal dimension, and limit sets. Nonetheless, certain limitations exist; for example, the revised Benettin–Wolf method is more appropriate for quasi-type integer-order systems, as the nonlocality is minimal enough to be disregarded.

Fractional derivatives are widely applicable across multiple disciplines. The study by Tom et al. on the modified Chua’s system, utilizing fractional derivatives, exemplifies the numerous contemporary advancements of fractional derivatives within the realm of chaotic systems with fractional indices [36].

In the revised Chua’s system that employs fractional derivatives, the differential equations integrating fractional-order terms are expressed as follows:

$$\begin{array}{cc}\hfill D^{\alpha }{x}_1& =a(x_2-h(x_1)),\hfill \\ \hfill D^{\alpha }{x}_2& =x_1-x_2+x_3,\hfill \\ \hfill D^{\alpha }{x}_3& =-b{x}_2.\hfill \end{array}$$

The nonlinearity $h(x_1)$ in the Chua’s diode characteristic can be defined as

$$\begin{array}{c}\hfill h(x_1)=c_1{x}_1+{\displaystyle \frac{1}{2}}(c_0-c_1)(|x_1+1|-|x_1-1|),\end{array}$$

where $c_0$ and $c_1$ are constants that describe the piecewise–linear characteristics of the diode. The system parameters a, b, $c_0$, and $c_1$ must be selected based on the desired dynamical behavior and stability analysis. Additionally, initial conditions for the state variables $x_1$, $x_2$, and $x_3$ must be specified to simulate the system’s behavior.

Recently, the control of chaotic dynamics has gained increased popularity. This surge in interest is attributed to the emerging trend of investigating chaotic systems through diverse mathematical and physical models, such as Chen’s [45] fractional-order system. The classical Chen system has been adapted into a fractional-order Chen system by integrating fractional derivatives based on the Caputo definition.

The fractional-order Chen system extends the classical Chen system by replacing classical integer-order derivatives with fractional-order derivatives. This modification offers several benefits:

• By introducing fractional-order derivatives, the fractional-order Chen system provides a more generalized framework that can capture a wider array of dynamical behaviors, making it a powerful tool in various applications requiring nonlocal memory effects.
• The fractional-order operators provide a more flexible and accurate representation of the memory and hereditary properties inherent in many real-world systems. Unlike classical integer-order models, which assume local interactions, fractional-order models account for the history of the state variables, allowing for more accurate modeling of processes with long-range temporal correlations.
• The introduction of fractional-order derivatives can lead to more complex dynamical behaviors, such as a wider range of chaotic attractors and bifurcation scenarios. This increased complexity allows the fractional-order Chen system to better capture the nuances of the chaotic phenomena observed in physical, biological, and engineering systems.
• The fractional-order Chen system can exhibit chaos at lower parameter values compared to the classical system, which can be advantageous in designing systems that require chaotic behavior for performance, such as in cryptography or random number generation.

The modified system is represented by the following equations:

$$\begin{array}{cc}\hfill D^{\alpha }x& ={\rho }_1(y-x),\hfill \\ \hfill D^{\alpha }y& =({\rho }_3-{\rho }_1)x-xz+{\rho }_{3}y,\hfill \\ \hfill D^{\alpha }z& =xy-{\rho }_{2}z,\hfill \end{array}$$

where ${\rho }_1$, ${\rho }_2$, and ${\rho }_3$ are constants that characterize the system, and $0<\alpha \le 1$ represents the order of the fractional derivatives.

This paper presents a new methodology concerning fractional-order dynamics within an eight-dimensional framework. By merging these types of dynamics, the proposed system is capable of exhibiting a wider spectrum of dynamical behaviors. We illustrate the efficacy of our approach through the application of an eight-dimensional fractional-order Chen system, highlighting its potential use in hyperchaotic systems.

This article is organized as follows: Section 1 introduces the concept of FODs, the Grünwald–Letnikov (G-L) method, and Chua’s system that employs fractional derivatives. Section 2 details the mathematical formulation and methodology for an eight-dimensional (8D) fractional-order Chen system using the memory effect inherent in the Grünwald–Letnikov (G-L) derivative. Numerical simulations of the proposed methodology are discussed in Section 3, with an analysis of the proposed 8D FOs for times $t=100$ and $t=200$ in Section 3.1 and Section 3.2, respectively. Section 4 covers chaotic characteristics and complexity analysis, including the Perron effect, Kolmogorov–Sinai (KS) entropy, Kaplan–Yorke fractal dimension, and the 0–1 Test for chaos. Section 5 deals with the discussion of the obtained results, and the Section 6 presents the conclusion of the proposed study.

2. Mathematical Formulation and Methodology

2.1. Basic Idea

The fundamental concept of the presented algorithm is outlined as follows: By discretizing a continuous-time system using numerical methods for fractional differential equations (FDEs), deviations over time due to a small initial perturbation are formulated as a discrete function. It is clearly established that the Lyapunov exponent is defined by the mean logarithmic growth rates of these deviations, which allows for straightforward calculation from the deviations themselves. The Lyapunov exponent spectrum is obtained by perturbing each dimension and applying the Gram–Schmidt orthonormalization method to address the numerical divergence induced by positive Lyapunov exponents.

The proposed eight-dimensional (8D) Chen system of fractional-order differential equations (FDEs) is described by the following set of equations:

$$\begin{array}{cc}\hfill D_t^{{\alpha }_1}{x}_1& ={\mathbf{g}}_1={\rho }_1(x_2-x_1)+x_4,\hfill \\ \hfill D_t^{{\alpha }_2}{x}_2& ={\mathbf{g}}_2={\rho }_2{x}_1-x_1{x}_3+x_4,\hfill \\ \hfill D_t^{{\alpha }_3}{x}_3& ={\mathbf{g}}_3=x_1{x}_2-x_3-x_4+x_7,\hfill \\ \hfill D_t^{{\alpha }_4}{x}_4& ={\mathbf{g}}_4=-{\rho }_3(x_1+x_2)+x_5,\hfill \\ \hfill D_t^{{\alpha }_5}{x}_5& ={\mathbf{g}}_5=-x_2-{\rho }_4{x}_4+x_6,\hfill \\ \hfill D_t^{{\alpha }_6}{x}_6& ={\mathbf{g}}_6=-{\rho }_5(x_1+x_5)+{\rho }_4{x}_7,\hfill \\ \hfill D_t^{{\alpha }_7}{x}_7& ={\mathbf{g}}_7=-{\rho }_6(x_1+x_6-x_8),\hfill \\ \hfill D_t^{{\alpha }_8}{x}_8& ={\mathbf{g}}_8=-{\rho }_7{x}_7,\hfill \end{array}$$

(1)

where each ${\alpha }_m$ (for $m=1,2,\dots ,8$) adheres to $0<{\alpha }_m\le 1$. Furthermore, we define a real-valued 8-dimensional vector mapping as $\mathbf{g}(\mathbf{x})={({\mathbf{g}}_1(\mathbf{x}),{\mathbf{g}}_2(\mathbf{x}),\dots ,{\mathbf{g}}_8(\mathbf{x}))}^T$, where $\mathbf{x}={(x_1,x_2,\dots ,x_8)}^T\in {\mathbb{R}}^8$. Here, $D_t^{{\alpha }_m}$ signifies a Grünwald–Letnikov fractional-order derivative (FOD) operator for $0<{\alpha }_m<1$ and an integer order derivative (IOD) operator for ${\alpha }_m=1$ for each m. Also, the parameters ${\rho }_1,{\rho }_2,{\rho }_3,{\rho }_4,{\rho }_5,{\rho }_6,{\rho }_7$ are constants that characterize the proposed system.

When examining the case of integer-order, the initial perturbation $\mathbf{p}(t_0)$ that causes a divergence between two phase trajectories is typically expressed as

$$\begin{array}{c}\hfill {\mathbf{p}}^{\prime }={\mathbf{J}}_{\mathbf{g}}\mathbf{p},\end{array}$$

(2)

$$\begin{array}{c}\hfill \mathbf{p}(t_0)=\mathbf{x}(t_0)-\overline{\mathbf{x}}(t_0),\end{array}$$

(3)

where Jacobian matrix of $\mathbf{g}(\mathbf{x})$ is denoted by ${\mathbf{J}}_{\mathbf{g}}={\displaystyle \frac{d\mathbf{g}}{d\mathbf{x}}}$. The LE, denoted as $\lambda$, is typically defined by the average logarithmic rate at which deviations grow, that is,

$$\begin{array}{c}\hfill \lambda =\underset{t\to \infty }{lim}{\displaystyle \frac{1}{t}}ln{\displaystyle \frac{\parallel p(t)\parallel }{\parallel p(t_0)\parallel }}.\end{array}$$

(4)

Some of the aforementioned methods, including the symplectic method introduced by Grünwald [8] and the decomposition method introduced by Letnikov [9], also involve solving Equations (2) and (3). In essence, both equations illustrate the long-term expansion of the 8-dimensional ellipsoid characterized by a deviation denoted as $\mathbf{p}$, with the growth rate adhering to the Lyapunov exponent. It can be noticed that for the fractional-order scenario, the Lyapunov exponent remains defined by Equation (4), yet the deviation should not be represented by Equations (2) and (3) due to the nonlocal nature of the fractional-order system. There are only a few unified approaches available to analyze the nonlocality of the deviation $\mathbf{p}$. In the next subsection, we address this issue and propose a new method to compute all Lyapunov exponents for fractional-order systems.

2.2. Proposed Methodology

The subsequent research addresses the fractional-order scenario of the 8-dimensional ordinary differential equation (ODE) system represented by Equation (1). The numerical method is developed by incorporating the memory principle from the Gr definition. In this context, the method is regarded as an 8-dimensional mapping and is characterized by

$$\begin{array}{c}\hfill \mathbf{x}(t_{k+1})=\mathbf{G}(\mathbf{x}(t_k),t_k),\end{array}$$

(5)

where $t_k=kh$ and h define the integration time step, $\mathbf{G}={({\mathbf{G}}_1,{\mathbf{G}}_2,\dots ,{\mathbf{G}}_8)}^T$, and ${\mathbf{G}}_m$ is given by

$$\begin{array}{c}\hfill {\mathbf{G}}_m(\mathbf{x}(t_k),t_k)={\mathbf{g}}_m(\mathbf{x}(t_k))h^{{\alpha }_m}-\sum _{s=1}^{k+1}{C}_s^{({\alpha }_m)}{x}_m(t_{k+1-s}),\end{array}$$

(6)

where $C_s^{({\alpha }_m)}$ is the fractional binomial coefficient obeying $C_0^{({\alpha }_m)}=1,C_s^{({\alpha }_m)}=\left(1-{\displaystyle \frac{(1+{\alpha }_m)}{s}}\right)C_{s-1}^{({\alpha }_m)}$. The method provides a first-order approximation for integer-order derivatives ${\alpha }_m=1$ and $(1-{\alpha }_m)$-order approximation for fractional derivatives $0<{\alpha }_m<1$.

The change in the divergence between two maps resulting from an initial perturbation ${\mathbf{p}}^{(0)}$ is governed by

$$\begin{array}{cc}\hfill {\mathbf{p}}^{(k+1)}& ={\mathbf{J}}_G^{(k)}{\mathbf{p}}^{(k)}={\mathbf{J}}_G^{(k)}{\mathbf{J}}_G^{(k-1)}{\mathbf{p}}^{(k-1)}=\dots =\prod _{r=0}^k{\mathbf{J}}_G^{(r)}{\mathbf{p}}^{(0)},\hfill \\ \hfill & ={\mathbf{B}}^{(k)}{\mathbf{p}}^{(0)}=\sum _{i,j=1}^8{\mathbf{p}}_i^{(0)}{\mathbf{b}}_j^{(k)},\hfill \end{array}$$

(7)

where ${\mathbf{p}}_i^{(0)}$ and ${\mathbf{b}}_j^{(k)}$ are representing the ith element of initial perturbation ${\mathbf{p}}^{(0)}$ and the jth column of ${\mathbf{B}}^{(k)}$, respectively. Also, ${\mathbf{J}}_G^{(k)}={\displaystyle \frac{dG}{dx}}{|}_{t=t_k}$, but Jacobian matrix ${\mathbf{J}}_G^{(k)}$ cannot be derived directly from expression (5). Therefore, if ${lim}_{k\to \infty }{\mathbf{J}}_G^{(k)}$ exists, an iterative scheme of ${\mathbf{B}}^{(k)}$ can be determined from the tangent map of Equation (6), with ${\mathbf{B}}^{(0)}$ set to the identity matrix I, from which the element $b_{i,j}^{(k)}$ can be determined as follows:

$$\begin{array}{c}\hfill b_{i,j}^{(k+1)}=h^{{\alpha }_m}{\displaystyle \frac{\partial {\mathbf{g}}_i}{\partial x_j}}{b}_{i,j}^{(k)}-\sum _{s=1}^{k+1}{C}_r^{({\alpha }_m)}{b}_{i,j}^{(k+1-s)},i,j=1,2,\dots ,8.\end{array}$$

(8)

To obtain all eight LEs of Equation (5), 8 sets of initial perturbation ${\mathbf{P}}^{(0)}=$$({\mathbf{p}}_1^{(0)},{\mathbf{p}}_2^{(0)},\dots ,{\mathbf{p}}_8^{(0)})=\mathrm{diag}({\delta }_1,{\delta }_2,\dots ,{\delta }_8)$ are considered in such a way that they can perturb each dimension, where each ${\delta }_i\ne 0$. From Equation (7), the deviation matrix can be formulated as

$$\begin{array}{c}\hfill {\mathbf{P}}^{(k+1)}={\mathbf{B}}^{(k)}{\mathbf{P}}^{(0)}.\end{array}$$

(9)

Therefore, it can be obtained from Equations (4) and (7) so that any jth Lyapunov exponent ( $j=1,2,\dots ,8$) of Equation (5) fulfils the following mathematical formulation:

$$\begin{array}{cc}\hfill {\lambda }_j=& \underset{t_k\to \infty }{lim}{\displaystyle \frac{1}{t_k}}ln{\displaystyle \frac{\parallel {\mathbf{p}}_j^{(k+1)}\parallel }{\parallel {\mathbf{p}}_j^{(0)}\parallel }}\hfill \\ \hfill =& \underset{k\to \infty }{lim}{\displaystyle \frac{1}{kh}}ln{\displaystyle \frac{|{\delta }_j|\parallel {\mathbf{b}}_j^{(k)}\parallel }{\parallel {\mathbf{p}}_j^{(0)}\parallel }}\hfill \\ \hfill {\lambda }_j=& \underset{k\to \infty }{lim}{\displaystyle \frac{1}{kh}}ln\parallel {\mathbf{b}}_j^{(k)}\parallel ,j=1,2,\dots ,8.\hfill \end{array}$$

(10)

Generally, ${\lambda }_1\ge {\lambda }_2\ge \dots \ge {\lambda }_8$; otherwise, Lyapunov exponents are rearranged in an order.

It is important to see that as $k\to \infty$, each column of ${\mathbf{B}}^{(k)}$ tends to align with ${\mathbf{b}}_1^{(k)}$, resulting in all calculated Lyapunov exponents being approximately equal to ${\lambda }_1$. Furthermore, ${\lambda }_1\ge 0$ indicates that ${\mathbf{B}}^{(k)}$ becomes unbounded, which can cause numerical divergence issues. To mitigate these two problems during the iteration of Equation (8), the Gram–Schmidt orthonormalization process is employed. Suppose the orthonormalization time step is $h_{\mathrm{norm}}=Nh$, and apply the subsequent orthonormalization process at $k=N,2N,\dots ,KN$, where $N\in \mathbb{N}$, that is,

$$\begin{array}{cc}\hfill {\mathbf{u}}_1^{(k)}=& {\mathbf{b}}_1^{(k)}\hfill \\ \hfill {\mathbf{b}}_1^{(k)}=& {\displaystyle \frac{{\mathbf{u}}_1^{(k)}}{\parallel {\mathbf{u}}_1^{(k)}\parallel }}\hfill \\ \hfill {\mathbf{u}}_2^{(k)}=& {\mathbf{b}}_2^{(k)}-〈{\mathbf{b}}_2^{(k)},{\mathbf{b}}_1^{(k)}〉{\mathbf{b}}_1^{(k)}\hfill \\ \hfill {\mathbf{b}}_2^{(k)}=& {\displaystyle \frac{{\mathbf{u}}_2^{(k)}}{\parallel {\mathbf{u}}_2^{(k)}\parallel }}\hfill \\ \hfill ⋮\\ \hfill {\mathbf{u}}_n^{(k)}=& {\mathbf{b}}_n^{(k)}-\left(〈{\mathbf{b}}_n^{(k)},{\mathbf{b}}_1^{(k)}〉{\mathbf{b}}_1^{(k)}+\dots +〈{\mathbf{b}}_n^{(k)},{\mathbf{b}}_{n-1}^{(k)}〉{\mathbf{b}}_{n-1}^{(k)}\right)\hfill \\ \hfill {\mathbf{b}}_n^{(k)}=& {\displaystyle \frac{{\mathbf{u}}_n^{(k)}}{\parallel {\mathbf{u}}_n^{(k)}\parallel }},n=1,2,\dots ,8.\hfill \end{array}$$

(11)

Finally, for $j=1,2,\dots ,8$, the jth Lyapunov exponent of Equation (1) can be obtained as follows:

$$\begin{array}{c}\hfill {\lambda }_j=\underset{K\to \infty }{lim}{\displaystyle \frac{1}{K{h}_{\mathrm{norm}}}}\sum _{r=1}^{K}ln\parallel {\mathbf{u}}_j^{(rN)}\parallel ,\end{array}$$

(12)

where K specifies that this formulated orthonormalization process repeat for K times. Empirical evidence suggests that the value of $h_{\mathrm{norm}}$ can be chosen within a broad range, because the computed results are not highly sensitive to this parameter. In this study, $h_{\mathrm{norm}}$ is set to $10h$ unless stated otherwise.

The Grünwald–Letnikov approach is defined to compute the memory component MEMORY of the FDEs used in the proposed model, and Gram–Schmidt Reorthonormalization (GSR) process approach is applied to approximate the fractional derivatives, as mentioned in the Algorithm 1. These functions play a critical role in implementing the Grünwald–Letnikov approach to fractional calculus, which considers the entire history of the function up to the current time.

Algorithm 1 Simulation of fractional-order system

1: Initialize the constant parameters ${\rho }_1,{\rho }_2,{\rho }_3,{\rho }_4,{\rho }_5,{\rho }_6,{\rho }_7$ 2: Initialize time step h, normalization step $h_{\mathrm{norm}}$, total time $t_n$ 3: Define time vectors t and T 4: Define fractional-orders ${\alpha }_1,{\alpha }_2,\dots ,{\alpha }_8$ 5: Initialize binomial coefficients $c_1,c_2,\dots ,c_8$ 6: for  $j=1$ to 8 do 7:     Update binomial coefficients $c_i(j)$ for each order ${\alpha }_i$ 8: end for 9: Initialize state variables $x_1(0),x_2(0),\dots ,x_8(0)$ 10: Initialize Jacobian matrix J with identity matrix 11: function memory( $X,c,L$) 12:     Initialize $SUM=0$ 13:     for  $k=1$ to $L-1$ do 14:          $SUM\leftarrow SUM+X(L-k)·c(k)$ 15:     end for 16:     return  $SUM$ 17: end function 18: for  $k=2$ to n do 19:     Update $x_i(k)$ for each variable using fractional derivatives 20:     Update Jacobian matrix J for each variable 21:     if  $mod(k,N)=0$ then 22:          $J,E\leftarrow GSR(J)$ 23:         Update summation of logarithm of norms $SUM$ 24:         Print progress and local Lyapunov exponents 25:     end if 26: end for 27: Output final local Lyapunov exponents

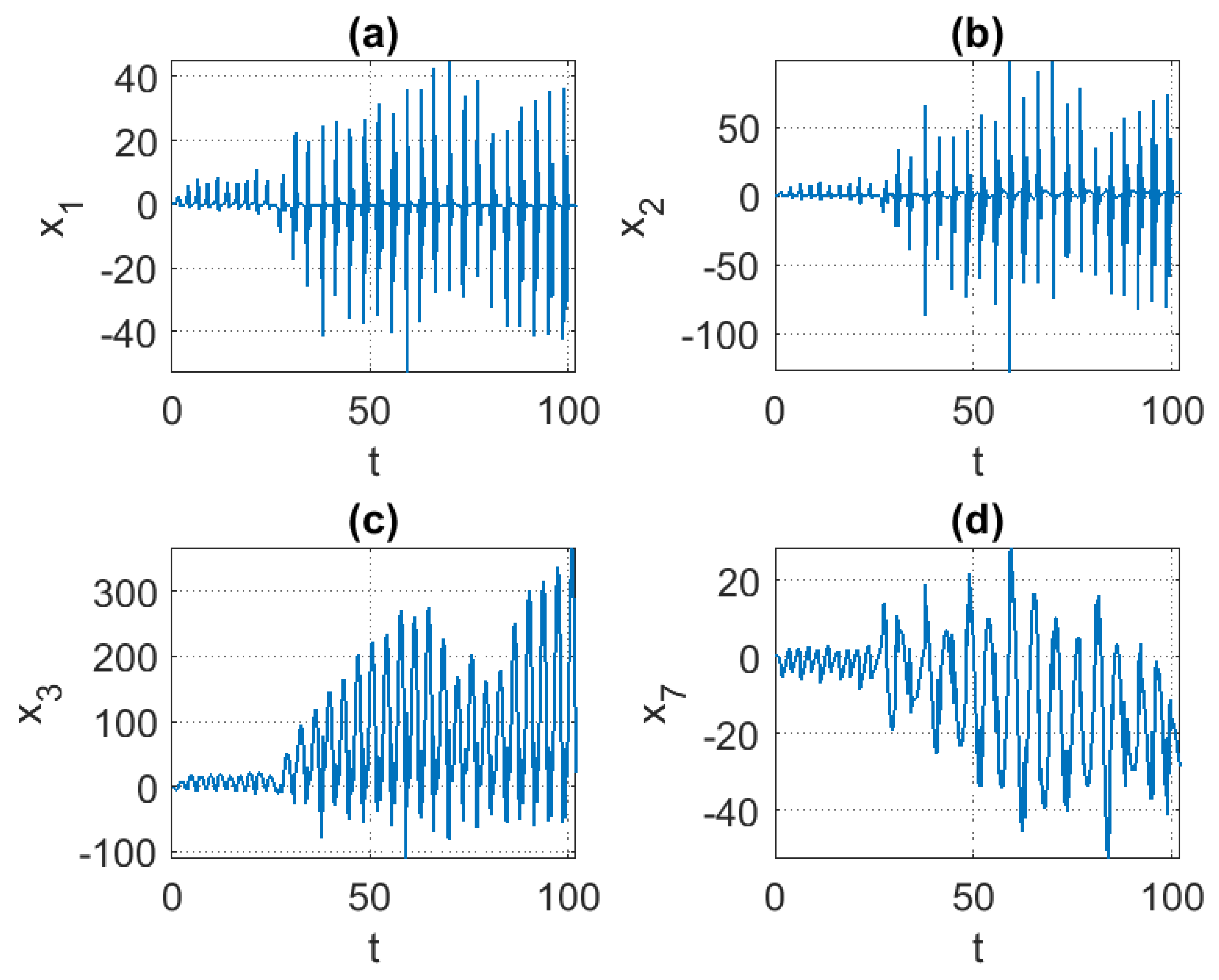
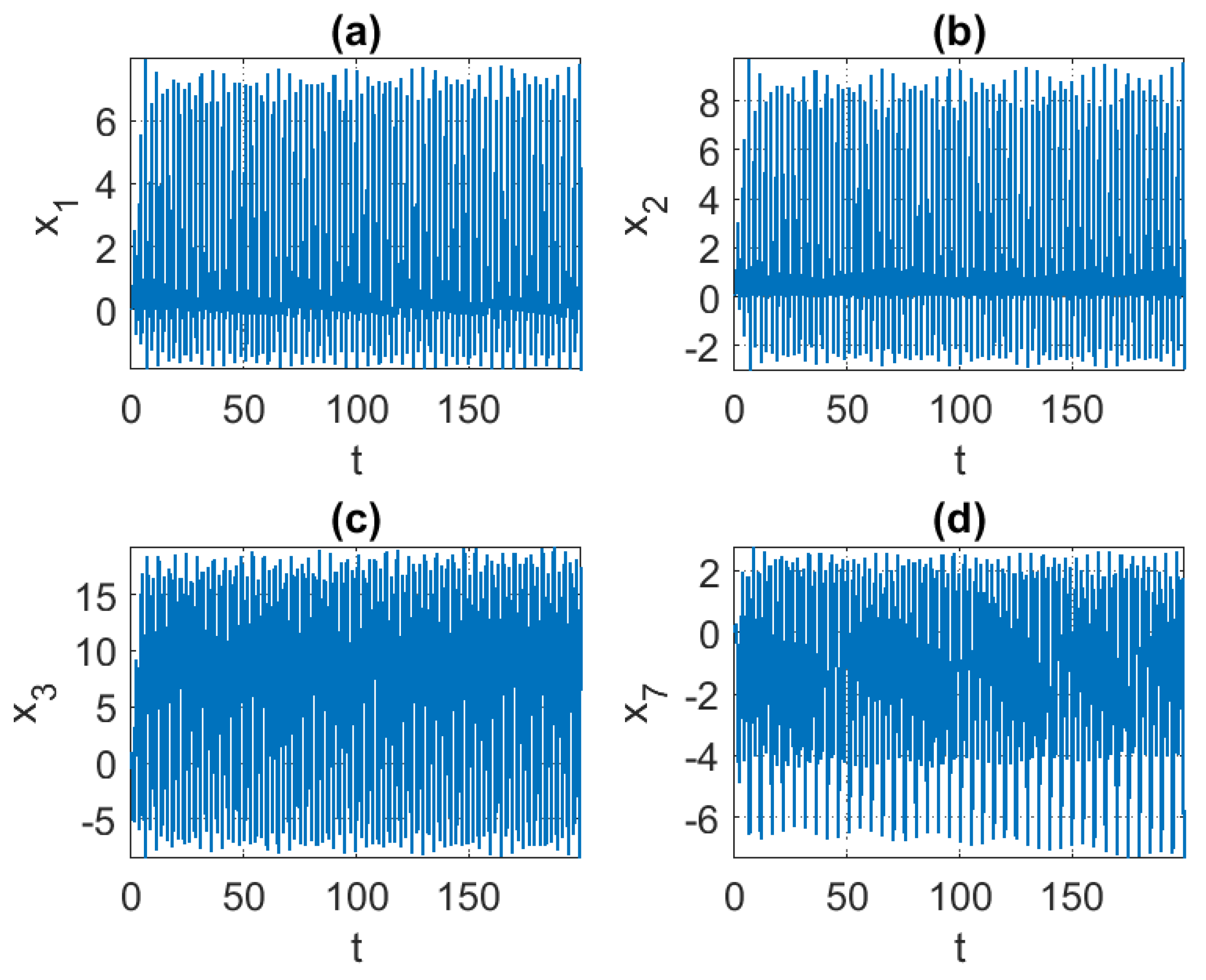
3. Numerical Analysis of the Proposed Methodology

The Lyapunov exponents (LEs) offer essential insights into the stability and dynamics of fractional-order systems. In our study, we analyzed the LEs for three distinct sets of fractional-orders, denoted as ${\mathrm{FO}}_1$, ${\mathrm{FO}}_2$, and ${\mathrm{FO}}_3$, and compared the results for the proposed fractional-order system (1). The system parameters were set as follows: time step $h=0.001$, initial condition $x(0)=(0,0,1,0,1,1,1,1)$, and $({\rho }_1,{\rho }_2,{\rho }_3,{\rho }_4,{\rho }_5,{\rho }_6,{\rho }_7)=(9,0.04,1.5,1.4,38,-15.2,-0.2)$ were the constant parameters determining the hyperchaotic behaviors of the proposed system. Three sets of fractional-orders $(Q_1,Q_2,Q_3)$ were defined as

${\mathrm{FO}}_1=Q_1=({\alpha }_1,{\alpha }_2,{\alpha }_3,{\alpha }_4,{\alpha }_5,{\alpha }_6,{\alpha }_7,{\alpha }_8)=(0.99,0.99,0.99,0.99,0.99,0.99,0.89,0.89)$,

${\mathrm{FO}}_2=Q_2=(0.99,0.99,0.99,0.99,0.98,0.98,0.98,0.98)$, and

${\mathrm{FO}}_3=Q_3=(0.99,0.99,0.99,0.99,0.99,0.99,0.99,0.99)$ with the final times $t=100$ and $t=200$.

3.1. Analysis of FOs (1) for the Set of Orders $Q_3$ at $t=100$

Based on the analysis of the LEs of the proposed 8D FOs (1) from

Table 1. LEs of 8D FOs (1) simulated by the proposed method for time t = 100.

Orders	${\mathit{\lambda }}_1$	${\mathit{\lambda }}_2$	${\mathit{\lambda }}_3$	${\mathit{\lambda }}_4$	${\mathit{\lambda }}_5$	${\mathit{\lambda }}_6$	${\mathit{\lambda }}_7$	${\mathit{\lambda }}_8$	Limit Set
FO1	1.868	1.118	0.240	0.097	−0.133	−0.325	−2.326	−9.684	Hyperchaos
FO2	1.348	0.469	0.121	0.058	−0.316	−0.747	−0.776	−9.755	Hyperchaos
FO3	1.199	0.454	0.061	0.054	−0.101	−0.546	-0.927	−9.698	Hyperchaos

, we can analyze that for an FO₁ with fractional-orders $Q_1$, the Lyapunov exponents exhibited a mix of positive and negative values, indicating the presence of hyperchaos. The four positive LEs confirm the chaotic behavior of the system, while the negative exponents signify dissipative dynamics. The sum of the LEs is negative, which further confirms the system’s dissipative behavior. The negative sum of the LEs affirms the dissipative characteristics of the system.

The proposed results for the set of fractional-orders FO₁, FO₂, and FO₃ are comparatively better, as they accurately capture the dynamic complexity of hyperchaotic systems. The higher values of the positive Lyapunov exponents in FO₁ indicate that these systems have more significant chaotic attractors and a higher degree of unpredictability. Additionally, the negative sum of the Lyapunov exponents across all three sets demonstrates the effectiveness of the method in maintaining the dissipative nature of the systems, which is crucial for the realistic modeling of physical phenomena.

The analysis of the FOs (1) for fractional-orders FO₃ at $t=100$ reveals several intricate dynamical behaviors characteristic of hyperchaotic systems. Figure 1 shows the LEs of the 8D hyperchaotic system, where multiple positive LEs confirm the presence of hyperchaos, indicating a high sensitivity to initial conditions and complex dynamical behavior. Specifically, the values of the LEs, ${\lambda }_1$, ${\lambda }_2$, ${\lambda }_3$, and ${\lambda }_4$, along with the negative exponents, demonstrate the multidimensional stretching and folding that leads to hyperchaos.

In Figure 2, the time histories of the state variables $x_1$, $x_2$, $x_3$, and $x_7$ exhibited highly irregular and nonperiodic fluctuations, reflecting the complex temporal evolution of the system. The large variations and dense oscillations in the time histories highlight the chaotic nature of the trajectories and the sensitivity to initial conditions, which are hallmark features of hyperchaotic dynamics.

The 2D attractors shown in Figure 3 further illustrate the complexity of the system’s phase space. Subplots (a) through (d) present various 2D projections of the attractors, with dense and intricate trajectories that confirm the high-dimensional chaotic behavior. For instance, the attractor in subplot (a) $x_1-x_2$ presents the dense looping and overlapping paths that confirm the presence of chaos, with trajectories not repeating, which is typical of chaotic systems., while the attractor in subplot (c) $x_2-x_3$ reveals a significant spread, underscoring the variability and complex interactions between different state variables. Each subplot confirms the presence of chaos through nonrepeating trajectories and dense attractor structures, indicating the high-dimensional and complex nature of the system’s dynamics.

Finally, the 3D attractors depicted in Figure 4 provide a comprehensive view of the system’s dynamics, and the corresponding Lyapunov exponents obtained from the proposed method are presented in Table 1 for fractional-order $Q_3$. Each subplot (a) through (f) shows the relationships between different sets of three state variables, with the attractors demonstrating dense and convoluted structures that are indicative of hyperchaotic systems and hyperchaotic dynamics indicating strong divergence. These 3D phase portraits, such as the attractor in subplot (a) $x_1-x_2-x_3$, highlight the intricate folding and stretching in the system’s phase space, which contributed to the overall complexity and hyperchaos observed in the FOs (1).

The combined analysis of the LEs, time histories, and phase portraits (both 2D and 3D) for the fractional-order system FO₃ at $t=100$ underscores the system’s hyperchaotic nature and complex dynamical behavior. The presence of multiple positive Lyapunov exponents, irregular time histories, and intricate attractors in the phase space all contribute to a comprehensive understanding of the system’s hyperchaotic dynamics.

3.2. Analysis of FOs (1) for the Set of Orders $Q_3$ at $t=200$

The Lyapunov exponents (LEs) of the proposed 8D FOs (1) for three sets of fractional-orders ( ${\mathrm{FO}}_1$, ${\mathrm{FO}}_2$, and ${\mathrm{FO}}_3$) presented in

Table 2. LEs of 8D FOs (1) simulated by the proposed method for time t = 200.

Orders	${\mathit{\lambda }}_1$	${\mathit{\lambda }}_2$	${\mathit{\lambda }}_3$	${\mathit{\lambda }}_4$	${\mathit{\lambda }}_5$	${\mathit{\lambda }}_6$	${\mathit{\lambda }}_7$	${\mathit{\lambda }}_8$	Limit Set
FO1	1.519	0.552	0.147	0.126	0.0393	−0.169	−1.660	−9.677	Hyperchaos
FO2	1.331	0.463	0.064	0.100	−0.335	−0.696	−0.736	−9.780	Hyperchaos
FO3	1.165	0.444	0.072	0.031	−0.181	−0.558	−0.845	−9.721	Hyperchaos

for time $t=200$ indicate that all three systems exhibited hyperchaotic behavior, as evidenced by the presence of multiple positive LEs. The negative sum of all eight LEs determinine that our proposed 8D system (1) is dispersed. For ${\mathrm{FO}}_1$, the dominant five positive exponents suggest a robust hyperchaotic attractor with strong divergence in five dimensions. The presence of both positive and negative LEs indicates a balance between expanding and contracting phases within the system.

In comparison, ${\mathrm{FO}}_2$ and ${\mathrm{FO}}_3$ showed higher positive LEs, and the total sum of the LEs is negative. The more significant positive exponents indicate a potentially more chaotic system compared to ${\mathrm{FO}}_2$ and ${\mathrm{FO}}_3$.

For instance, see Figure 5 which presents the LE’s of the 8D hyperchaotic system for set of fractional-orders ${\mathrm{FO}}_3$ at $t=200$.

Figure 5. LEs of the 8D hyperchaotic system for set of fractional-orders FO₃ at $t=200$.

Figure 5. LEs of the 8D hyperchaotic system for set of fractional-orders FO₃ at $t=200$.

The time series graphs in Figure 6 illustrate the dynamic behavior of attractors for the fractional-orders FO₃ system at $t=200$. Each subplot demonstrated complex and high-frequency oscillatory behavior indicative of hyperchaos, particularly noticeable in the series for $x_1$ and $x_2$, which exhibited a broad range of fluctuations. The attractors for $x_3$ and $x_7$ showed distinct oscillatory patterns, further confirming the presence of hyperchaotic dynamics. Compared to the existing literature, these results highlight the effectiveness of our proposed FOs in capturing intricate chaotic behavior.

The 2D phase portraits in Figure 7 exhibit the hyperchaotic behavior of the system’s attractors for the fractional-orders FO₃ set at $t=200$. The intricate and dense trajectories observed in subplots (a) through (d) indicate significant sensitivity to the initial conditions and complex dynamic interactions, which is characteristic of hyperchaotic systems. For instance, the $x_1-x_2$ phase space in subplot (a) shows a densely packed attractor with overlapping loops, signifying a high degree of chaos. Similarly, the $x_1-x_3$ and $x_2-x_3$ phase spaces in subplots (b) and (c) display intricate, nonrepetitive patterns, further confirming the presence of hyperchaotic dynamics. The $x_1-x_7$ phase portrait in subplot (d) also reveals a complex structure with extensive variability. Compared to previous studies such as those proposed by H. Tian et al. [12] and F. Yu et al. [46], the results presented here demonstrate a more pronounced hyperchaotic behavior, indicating that the proposed fractional-order model captures the complexity and richness of the system dynamics more effectively.

The 3D phase portraits presented in Figure 8 illustrate the complex dynamics of the fractional-order system FO₃. These portraits reveal a rich structure of hyperchaotic attractors, which is characterized by high sensitivity to the initial conditions and intricate, nonrepeating trajectories. The attractor in Figure 8a $x_1-x_2-x_3$ displays a distinct stretching and folding pattern, which is indicative of strong chaotic behavior. Similarly, Figure 8d $x_1-x_2-x_6$ and (e) $x_1-x_2-x_7$ show significant divergence in trajectories, further supporting the presence of multiple positive Lyapunov exponents. These complex attractors suggest an enhanced degree of unpredictability and sensitivity compared to the results of H. Tian [12] proposed for a four-dimensional conditional symmetric fractional-order system. Furthermore, the results specify that this proposed system has more better chaotic attractors as compared to the systems proposed in [47,48,49] and T. Lu et al. [50], as revealed by the numerical results presented in Figure 8. The results align with the existing literature on fractional-order chaotic systems but demonstrate superior complexity and chaotic intensity due to the fractional differentiation.

4. Chaotic Characteristics and Complexity Analysis

4.1. Perron Effect

The Perron effect refers to a phenomenon in which one of the Lyapunov exponents (LEs) dominates the dynamics of a system, often resulting in a scenario where a single direction in the phase space governs the behavior of the entire system. Mathematically, this can be assessed by comparing the largest Lyapunov exponent (LE) to the others, which is expressed as ${\lambda }_1\gg {\lambda }_2\ge {\lambda }_3\ge \dots \ge {\lambda }_8$. If the largest Lyapunov exponent, ${\lambda }_1$, is significantly greater than the others, it indicates a pronounced Perron effect.

The Perron effect is evident in the results of the LEs for the FOs presented in Table 1 and Table 2. The presented results exhibit the Lyapunov exponents (LEs) for three different fractional-orders (FO₁, FO₂, and FO₃), where the LEs ( ${\lambda }_1$) are significantly positive, indicating the presence of strong exponential divergence of the nearby trajectories. The dominant largest LEs for all three systems align with the Perron effect, demonstrating that the systems possess hyperchaotic behavior. These findings validate the effectiveness of the presented method in capturing the intricate dynamics of FOs. If we analyze the LEs of the 8D hyperchaotic system presented in Table 1 and Table 2 for the set of fractional-orders FO₁ at $t=100$ and $t=200$, respectively, the dominance of the largest Lyapunov exponent ${\lambda }_1$ (1.868 and 1.519) over others highlights a strong directional influence in the phase space compared to the results of Benkouider [51], Biban [52], Mahmoud [47], Zhu [48], and Jianliang [49], validating the results expected through the Perron effect.

4.2. Kolmogorov–Sinai (KS) Entropy

Kolmogorov–Sinai (KS) entropy, also known as metric entropy, is a measure of the rate of information production in a dynamical system and is related to the sum of all positive LEs. For a given system, its KS entropy denoted as $E_{KS}$ can be calculated as follows:

$$E_{KS}=\sum _{{\lambda }_i>0}{\lambda }_i.$$

The calculated KS entropy values indicate the rate of information production and the degree of chaotic behavior in the fractional-order system. The KS entropy provides a quantitative measure of the chaotic dynamics, with higher values corresponding to greater levels of chaos and complexity in the system’s behavior. FO₁ had the highest KS entropy of $3.323$, suggesting it has the most complex and unpredictable behavior among the three systems. FO₂ and FO₃ had lower KS entropy values of $1.996$ and $1.768$, respectively, indicating relatively less complex behavior compared to FO₁. The presented results of the Kolmogorov–Sinai (KS) entropy values for FO₁, FO₂, and FO₃ demonstrate higher values and greater levels of chaos and complexity compared to the findings of Benkouider [51], Biban [52], Mahmoud [47], Zhu [48], and Jianliang [49]. These numerical results of KS entropy indicate that the proposed FOs (1) exhibit superior chaotic behavior.

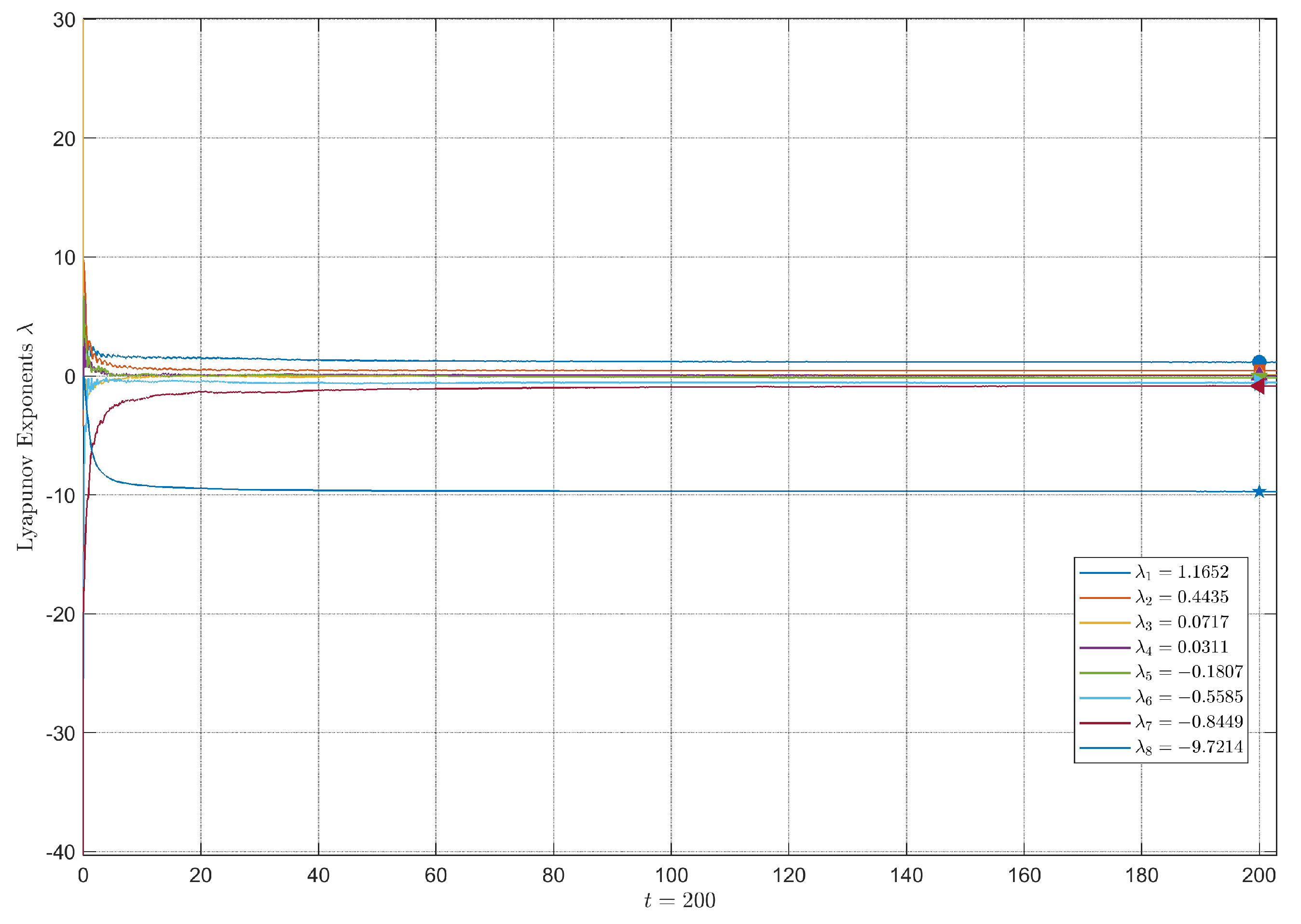
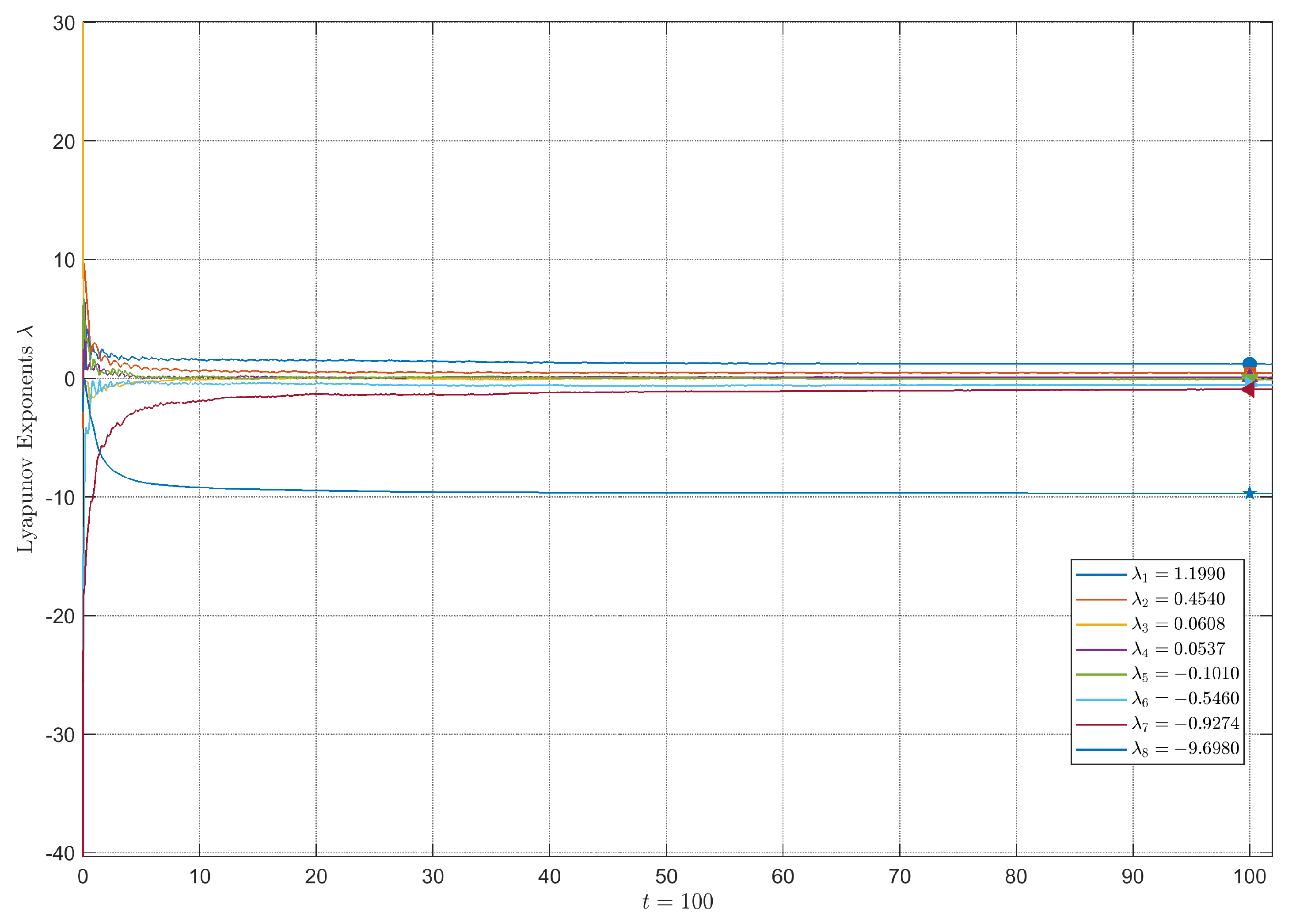
4.3. Lyapunov Exponents and Kaplan–Yorke Dimension

Based on chaos theory, elevated values of Kaplan–Yorke dimensions [53] are indicative of greater complexity within dynamical systems. For the presented FOs (1), the corresponding Kaplan–Yorke dimension $D_{KY}$ is determined as follows:

$$D_{KY}=j+{\displaystyle \frac{{\sum }_{i=1}^j{\lambda }_i}{|{\lambda }_{j+1}|}},$$

where j is denoted as the largest integer as follows:

$$\sum _{i=1}^j{\lambda }_i>0\mathrm{and}\sum _{i=1}^{j+1}{\lambda }_i<0.$$

For set of fractional-order FO₁ at $t=200$, we can calculate the Kaplan–Yorke dimension ( $D_{KY}$) for the LEs (see Table 2 and Algorithm 2) as follows:

$$D_{KY}=7+{\displaystyle \frac{{\sum }_{i=1}^7{\lambda }_i}{|{\lambda }_8|}}=7.057,$$

where $j=7$ is the largest integer such that

$$\sum _{i=1}^7{\lambda }_i=0.5543>0\mathrm{and}\sum _{i=1}^8{\lambda }_i=-9.677<0.$$

Table 3. Kaplan–Yorke fractal dimension of twelve chaotic systems.

ODE Systems	Fractal Dimensions
7D Hu and Chan system [54]	6.732
7D Yang system [55]	6.149
7D Yu system [56]	5.278
7D Lagmiri system [57]	2.091
7D Varan system [58]	2.175
8D Biban system [52]	7.13
9D Mahmoud system [47]	5.128
9D Zhu system [48]	2.171
10D Jianliang system [49]	2.429
Proposed 8D FOs1	7.05
Proposed 8D FOs2	7.019
Proposed 8D FOs3	7.013

illustrates the comparison of fractional dimensions of our new proposed FO system (1) with some famous hyperchaotic systems from the literature.

Algorithm 2 Calculate Kaplan–Yorke dimension

Require:  $LE$: Matrix of Lyapunov exponents Ensure:  $K_Y\_dimension$: The Kaplan–Yorke Dimension 1: if  $LE$ is not empty then 2:      $LE\_current\leftarrow LE(:,\mathrm{end})$  ▹Get the current Lyapunov exponents from the last calculation 3:      $LE\_current\_sorted\leftarrow \mathrm{sort}(LE\_current,\prime \mathrm{descend}\prime )$  ▹Sort Lyapunov exponents in descending order 4:      $sumL\leftarrow 0$ 5:      $j\leftarrow 0$ 6:     while  $j<\mathrm{length}(LE\_current\_sorted)$ and  $sumL\ge 0$ do 7:          $j\leftarrow j+1$ 8:          $sumL\leftarrow sumL+LE\_current\_sorted[j]$ 9:     end while 10:     if  $j==1$ or  $sumL<0$ then 11:          $sumL\leftarrow sumL-LE\_current\_sorted[j]$ ▹Subtract the last added negative term to correct the sum 12:          $j\leftarrow j-1$           ▹Adjust j to the last positive sum index 13:     end if 14:     if  $j==0$ then 15:          $K_Y\_dimension\leftarrow \mathrm{NaN}$  ▹Not defined as there are no positive exponents 16:     else if  $j==\mathrm{length}(LE\_current\_sorted)$ then 17:          $K_Y\_dimension\leftarrow j$       ▹All exponents are non-negative 18:     else 19:          $K_Y\_dimension\leftarrow j+{\displaystyle \frac{sumL}{\mathrm{abs}(LE\_current\_sorted[j+1])}}$   ▹Compute the fractional dimension 20:     end if 21:     Print $K_Y\_dimension$ 22: end if

Based on Table 3, it can be observed that the set of proposed fractional-orders (FOs₁, FOs₂, and FOs₃) have higher Kaplan–Yorke fractal dimensions compared to several existing systems. Specifically, the proposed systems exhibit dimensions of 7.05, 7.019, and 7.013, respectively, which are higher than those reported for many traditional high-dimensional chaotic systems. The proposed fractional-order systems FO₁, FO₂, and FO₃ exhibit higher Kaplan–Yorke fractal dimensions compared to many existing high-dimensional chaotic systems [47,48,49,54,55,56,57,58] presented in Table 3 and approximately equal to the fractional dimension of the 8D Biban system [52]. This highlights the effectiveness and superiority of the proposed method in capturing the intricate dynamics of complex systems. The proposed method has been rigorously derived and tested, showing superior accuracy in calculating Lyapunov exponents, which in turn provides a more precise estimation of the fractal dimension. The proposed method is applicable to both fractional-order and integer-order systems, demonstrating its versatility and robustness across different types of dynamical systems. The obtained results of the Kaplan–Yorke fractal dimensions presented in Table 3 indicate that the computed $D_{KY}$ values are very large compared to other dynamical systems. Thus, the presented 8D FOs (1) specifies a complex hyperchaotic behavior.

4.4. 0–1 Test for Chaos

We employed the ’0–1 Test’ proposed by Gottward and Melbourne [59,60] to verify the existence of chaotic behavior from numerical time series data. The 0–1 Test for Chaos is employed to distinguish between chaotic and regular behavior in dynamical systems. Here, we applied the 0–1 Test to analyze the 8D ODE system through its Lyapunov exponents.

The main benefits of this test are that it allows for a straightforward visualization of the dynamical systems in the translation variables $(p-q)$ space. Due to the reliability and importance of the test, we utilized this test to analyze our proposed FOs (1), which features a dissipative phase space, using the time series data obtained numerically for the chaotic region.

For the proposed 8D ODE system (1) with state variables $x_1,x_2,\dots ,x_8$, the translation variables $p(t)$ and $q(t)$ using a fixed phase value c are written as

$$\begin{array}{cc}\hfill p_i(t)& =\sum _{k=1}^t{x}_i(k)cos(kc),\hfill \\ \hfill q_i(t)& =\sum _{k=1}^t{x}_i(k)sin(kc).\hfill \end{array}$$

(13)

Equation (13) introduces the translation variables $p_i(t)$ and $q_i(t)$ for each state variable $x_i(t)$ of the proposed 8D ODE system. These translation variables represent the system’s behavior in a transformed coordinate system obtained using trigonometric functions with a fixed phase value c. The Mean Square Displacement (MSD) is a measure used to analyze the diffusive behavior of a system over time, and in this context, it is related to how the state variables $x_i(t)$ spread in phase space as a function of time.

The translation variables $p_i(t)$ and $q_i(t)$ capture the oscillatory nature of the state variables $x_i(t)$ for $i=1,2,\dots ,8$ when projected onto a rotating frame of reference defined by cosine and sine functions with frequency c. This projection helps to capture the frequency components and phase information of the original signals $x_i(t)$. The phase angle $kc$ introduces a circular or oscillatory transformation, which is useful for analyzing the trajectory spread over time.

The Mean Square Displacement (MSD) for a particle in a system can be derived by calculating the average squared displacement over time for each particle. The squared displacement is determined by finding the difference between the particle’s positions at two different time points, squaring this difference, and then averaging over all time intervals and particles. This provides a measure of the average distance traveled squared.

The displacement of a particle i in the $x_i$ direction between two time steps k and $k+n$ is given by $p_i(k+n)-p_i(k)$. Similarly, for the $y_i$ direction (if applicable), the displacement is $q_i(k+n)-q_i(k)$. The squared displacement in both the $x_i$ and $y_i$ directions is

$${(p_i(k+n)-p_i(k))}^2+{(q_i(k+n)-q_i(k))}^2.$$

This expression represents the squared Euclidean distance traveled by the particle in a 2D space (assuming $p_i$ and $q_i$ represent $x_i$ and $y_i$ coordinates, respectively). The MSD $M_i(n)$ is obtained by averaging the squared displacements over all possible time steps k for a given time lag n:

$$\begin{array}{c}\hfill M=M_i(n)={\displaystyle \frac{1}{N}}\sum _{k=1}^N\left[{(p_i(k+n)-p_i(k))}^2+{(q_i(k+n)-q_i(k))}^2\right],\end{array}$$

(14)

where we have the following:

•

$x_i(k)$ is the value of the ith state variable at time step k.

•

$p_i(t)$ and $q_i(t)$ are the translation variables obtained from the time series of the dynamical system. They are computed by projecting the time series onto two orthogonal directions defined by a randomly chosen angle.

•

$n_{\mathrm{vals}}$ is the total number of time points in the time series from $x_1$ to $x_8$.

•

$N=⌊{\displaystyle \frac{n_{\mathrm{vals}}}{2}}⌋$ is the total number of data points in the time series, and it defines the upper limit of the summation.

•

$M_i(n)$ represents the average squared displacement of the points in the $(p,q)$ space over a time lag n.

According to the 0–1 Test, if $M_i(n)$ increases sublinearly or remains bounded as n increases, then the system is considered regular. However, if $M_i(n)$ increases linearly with n, the system is chaotic, for instance, see [59,60]. In our scenario, we selected $c={\displaystyle \frac{\pi }{4}}$ with a step size $h=0.001$, the initial conditions were selected as $x(0)=(0,0,1,0,1,1,1,1)$, and we selected parameters $({\rho }_1,{\rho }_2,{\rho }_3,{\rho }_4,{\rho }_5,{\rho }_6,{\rho }_7)=(9,0.04,1.5,1.4,38,-15.2,-0.2)$ for $F{O}_1$. The 0–1 Test was utilized for the time series data of the given state variables $x_1,x_2,\dots ,x_8$ from the eight-dimensional ODE system, and the results for state variables $x_1,x_2,x_6$, and $x_7$ are shown in Figure 9.

Next, we determined the diffusive properties of $p_i(t)$ and $q_i(t)$ from the data to examine the mean squared displacement $[M_i(n)]$. According to the theoretical framework, when the motion exhibits chaotic behavior, $[M_i(n)]$ increases linearly with time, as illustrated in Figure 9.

The Mean Square Displacement (MSD) plots show a clear linear growth over time, which is indicative of chaotic dynamics, as a linear increase in the MSD suggests that the system’s trajectories are diverging exponentially, and this divergence is a hallmark of chaos. Additionally, the $[p_i(t),q_i(t)]$ plots exhibit a scattered and nonperiodic pattern; furthermore, Figure 9 depicts the chaotic structure of the translational components $[p_i(t),q_i(t)]$. The randomness in these plots implies a lack of regularity and predictability, which is consistent with the characteristics of a chaotic system.

Then, we assessed the diffusive properties of the $p_i(t)$ and $q_i(t)$ data to examine the Mean Square Displacement $[M_i(n)]$. According to the theory, when the motion exhibits chaos, $[M_i(n)]$ increases linearly with time, as illustrated in Figure 9. The Mean Square Displacement (MSD) graphs reveal distinct linear growth over time, indicating chaotic behavior. A linear rise in the MSD suggests that the system’s trajectories are diverging exponentially, which is a fundamental characteristic of chaos. Additionally, the $[p_i(t),q_i(t)]$ plots display a dispersed and nonperiodic pattern. Figure 9 further shows the chaotic nature of the translational components $[p_i(t),q_i(t)]$. The randomness in these plots suggests an absence of regularity and predictability, aligning with the features of a chaotic system.

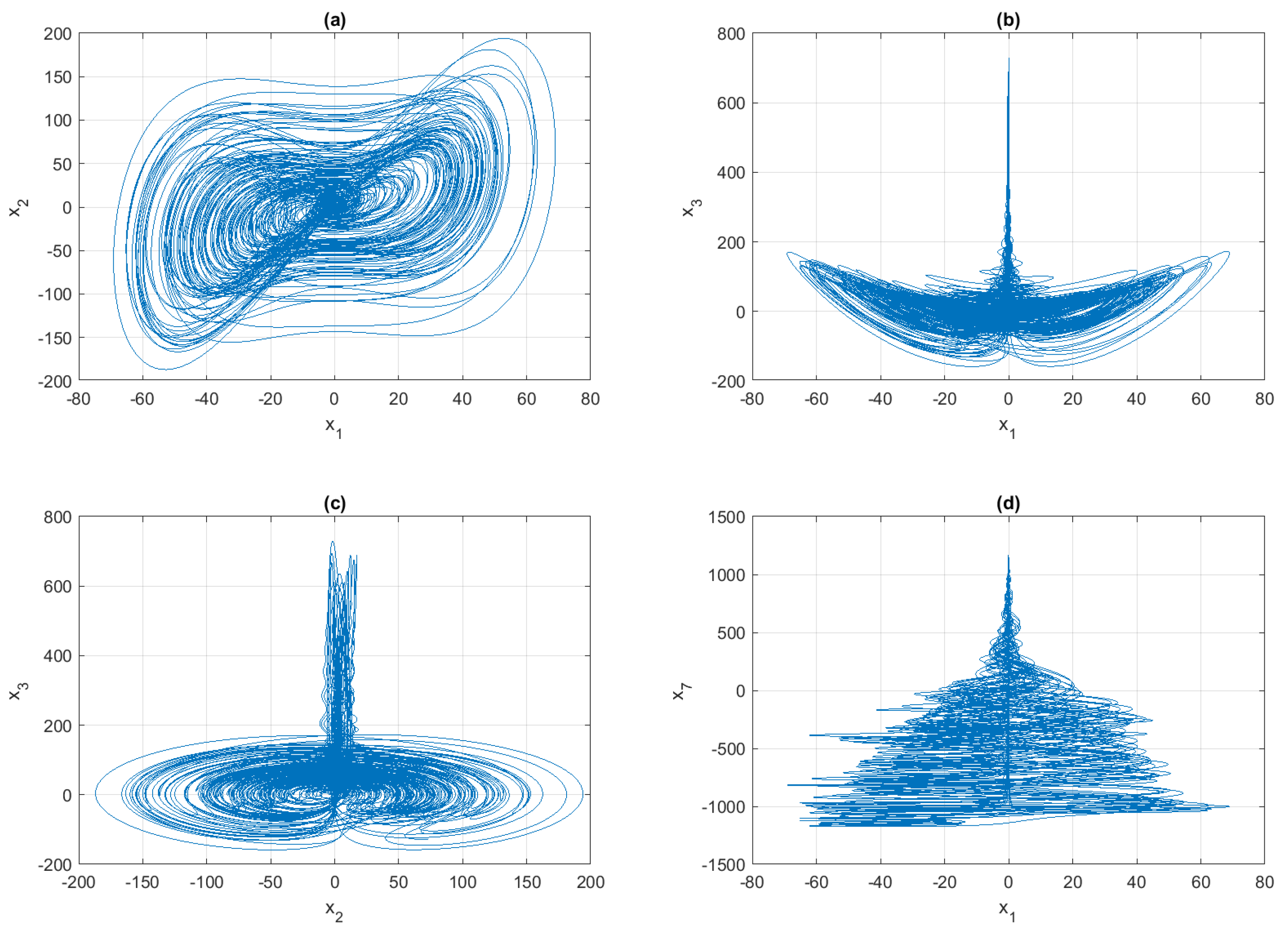
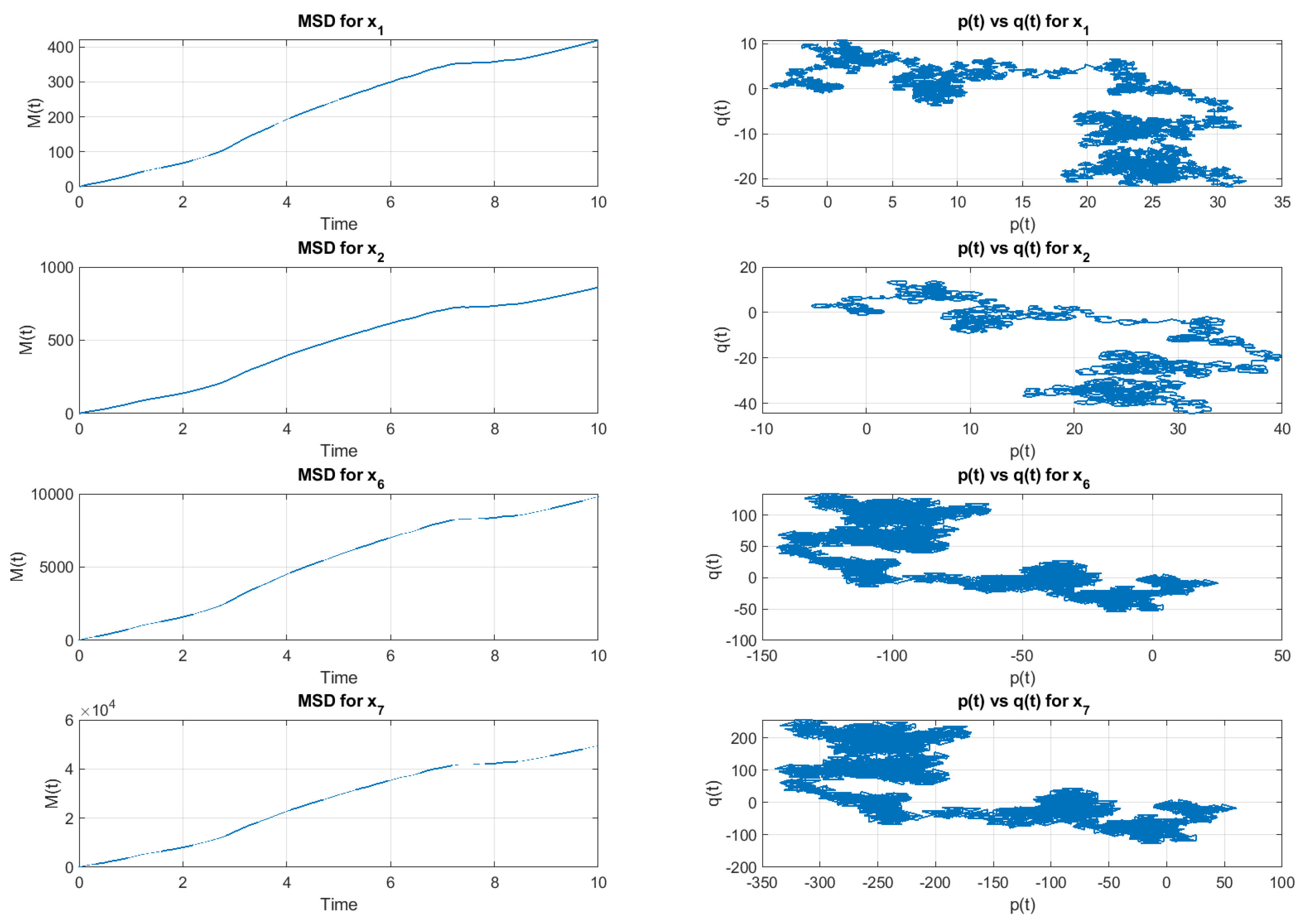
5. Results and Discussions

The results obtained from the numerical simulations of the presented eight-dimensional fractional-order chaotic systems reveal significant insights into the system’s dynamical behavior. The simulations of the fractional-order Chen system, in particular, highlight the precision and accuracy improvements over existing methods, demonstrating the method’s superior applicability to hyperchaotic systems. Utilizing the Grünwald–Letnikov derivative for incorporating the memory effect, the system exhibited complex dynamical behavior characterized by multiple positive Lyapunov exponents, indicating hyperchaotic dynamics. The analysis of the system’s LEs for different sets of fractional-orders reveals that the proposed method achieved higher precision and accuracy compared to traditional methods. The presence of multiple positive LEs in all three sets of fractional-orders $(F{O}_1,F{O}_2,F{O}_3)$ confirms the system’s hyperchaotic nature, with the highest Lyapunov exponents indicating a high degree of unpredictability and complex attractor structures.

Furthermore, the phase portraits and time series analyses provide additional evidence of the system’s chaotic behavior. The 2D and 3D phase portraits exhibited dense and intricate trajectories, indicative of strong sensitivity to initial conditions and complex interactions between state variables. These results align with the existing literature but demonstrate superior complexity and chaotic intensity due to the fractional differentiation. The Kaplan–Yorke dimension further supports these findings, showing higher fractal dimensions for the proposed system compared to several well-known high-dimensional chaotic systems.

Additionally, the 0–1 Test for Chaos, Kaplan–Yorke dimension, Kolmogorov–Sinai (KS) entropy, and Perron effect analyses provide a comprehensive understanding of the system’s stability and chaotic characteristics. The Kaplan–Yorke dimension calculations demonstrate that the proposed system yielded a higher fractal dimension, indicating greater complexity and chaotic behavior compared to the results proposed in [47,48,49,54,55,56,57,58]. The Perron effect and the KS entropy values confirm the system’s high rate of information production and unpredictability compared to the numerical results presented in [47,48,49,51,52]. These results collectively validate the effectiveness of the proposed method in accurately capturing and analyzing the intricate dynamics of hyperchaotic fractional-order systems, thereby offering a robust framework for studying complex dynamical systems with fractional-order derivatives.

6. Conclusions

In conclusion, the proposed technique for computing the LE spectrum of FOs has proven to be superior in terms of accuracy and correctness, especially in handling hyperchaotic systems. The distinct advantages observed in FO₂ and FO₃ underscore the method’s ability to enhance the understanding and prediction of complex dynamical behaviors in high-dimensional fractional-order systems.

We utilized Lyapunov exponents to quantitatively assess hyperchaos and categorize the limit sets of the proposed FOs. While there are numerous methods for computing Lyapunov exponents in integer-order systems, these methods are not suitable for fractional-order systems because of the nonlocal characteristics of fractional-order derivatives. This paper introduced innovative eight-dimensional chaotic systems that investigated fractional-order dynamics. These systems exploited the memory effect inherent in the Grünwald–Letnikov derivative.

This approach has enhanced the system’s applicability and compatibility with traditional integer-order systems. An eight-dimensional Chen’s system was utilized to analyze the effectiveness of the presented technique for hyperchaotic systems. The simulation results indicate that the presented technique surpassed existing algorithms in terms of both precision and accuracy.

Moreover, the study utilized the 0–1 Test for Chaos, the Perron effect, Kolmogorov–Sinai (KS) entropy, and the Kaplan–Yorke dimension to analyze the proposed eight-dimensional fractional-order system. These additional metrics provide a comprehensive understanding of the system’s chaotic behavior and stability characteristics.

7. Recommendations

The demonstrated hyperchaotic behavior and high-dimensional complexity of the proposed eight-dimensional fractional-order systems suggest their potential for creating robust cryptographic algorithms and promising application is in the area of encryption and cryptography. The high-dimensional hyperchaotic nature of the proposed systems, characterized by multiple positive Lyapunov exponents and high Kaplan–Yorke dimensions, offers a rich source of complex and unpredictable signals. These signals can be used to generate cryptographic keys that are highly resistant to attacks, thereby improving the security of encryption algorithms.

Additionally, the memory effect inherent in the Grünwald–Letnikov derivative can be exploited to create novel memory devices and circuits in neuromorphic engineering, where mimicking the behavior of biological neural systems is essential. The unique properties of fractional-order systems, such as their nonlocality and memory, make them well suited for developing more accurate and efficient models of neuronal behavior and other biological processes. Furthermore, the proposed method’s superior precision and accuracy in modeling hyperchaotic systems can be applied to enhance the performance of analog signal processing and chaotic oscillators, leading to innovations in various engineering and technological domains.

Also, using this proposed study, 10D and higher-dimensional hyperchaotic systems can be utilized for image encryption based on the Fibonacci Q-Matrix. The proposed study will be pivotal in addressing the small key space issue in image encryption algorithms that utilize low-dimension chaotic maps. Additionally, it suggests the development of a novel encryption method for color images based on eight-dimensional variational mode decomposition combined with higher-dimensional hyperchaotic systems.