Robust Control Design of Under-Actuated Nonlinear Systems: Quadcopter Unmanned Aerial Vehicles with Integral Backstepping Integral Terminal Fractional-Order Sliding Mode
Abstract
1. Introduction
2. Problem Formulation
3. Control Law Design
3.1. Case-I
3.2. Case-II
4. Benchmark Example of Cart–Pendulum System
4.1. System Description
4.2. Controller Design
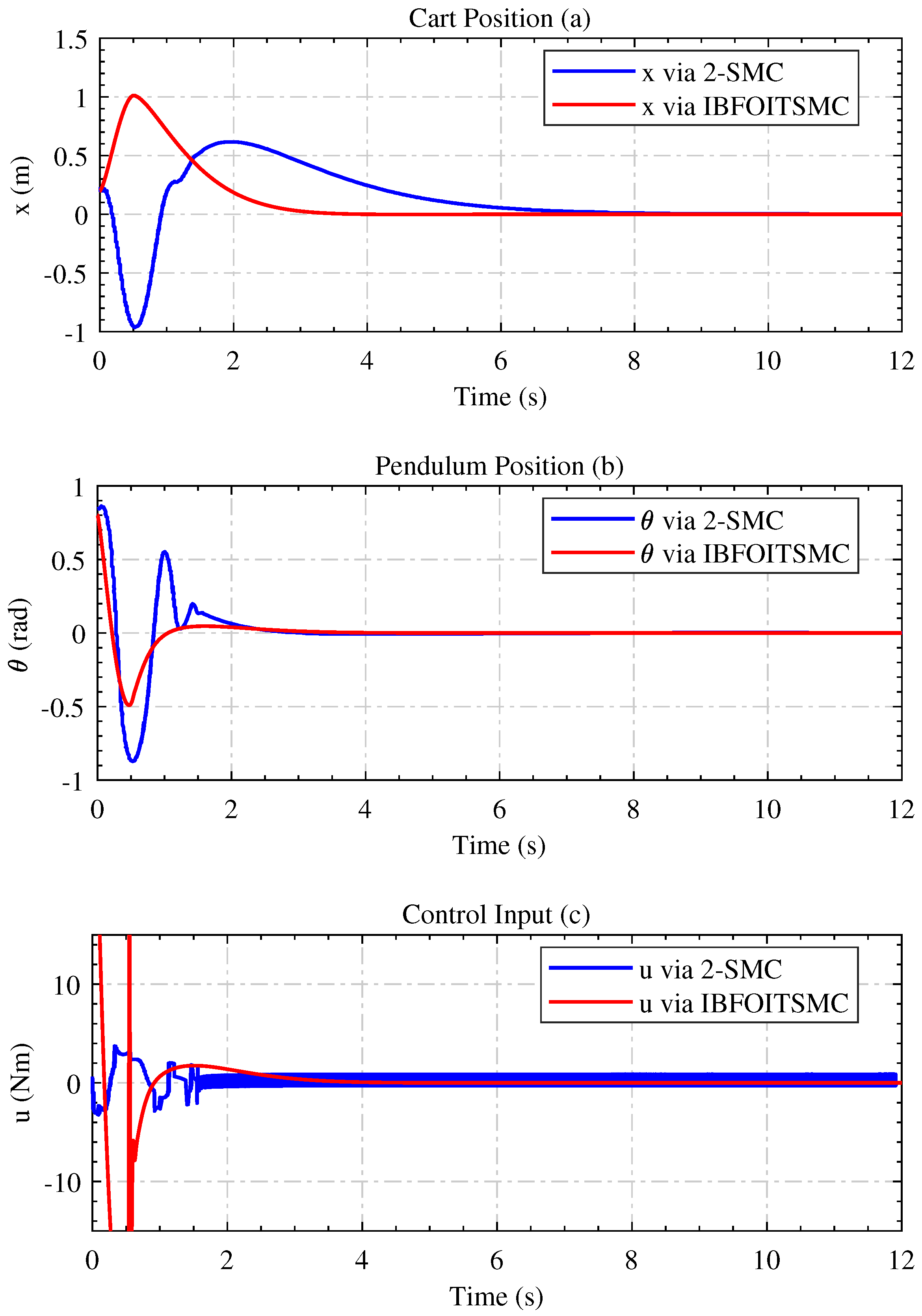
4.3. Results Discussion
5. Benchmark Example of Quadcopter System
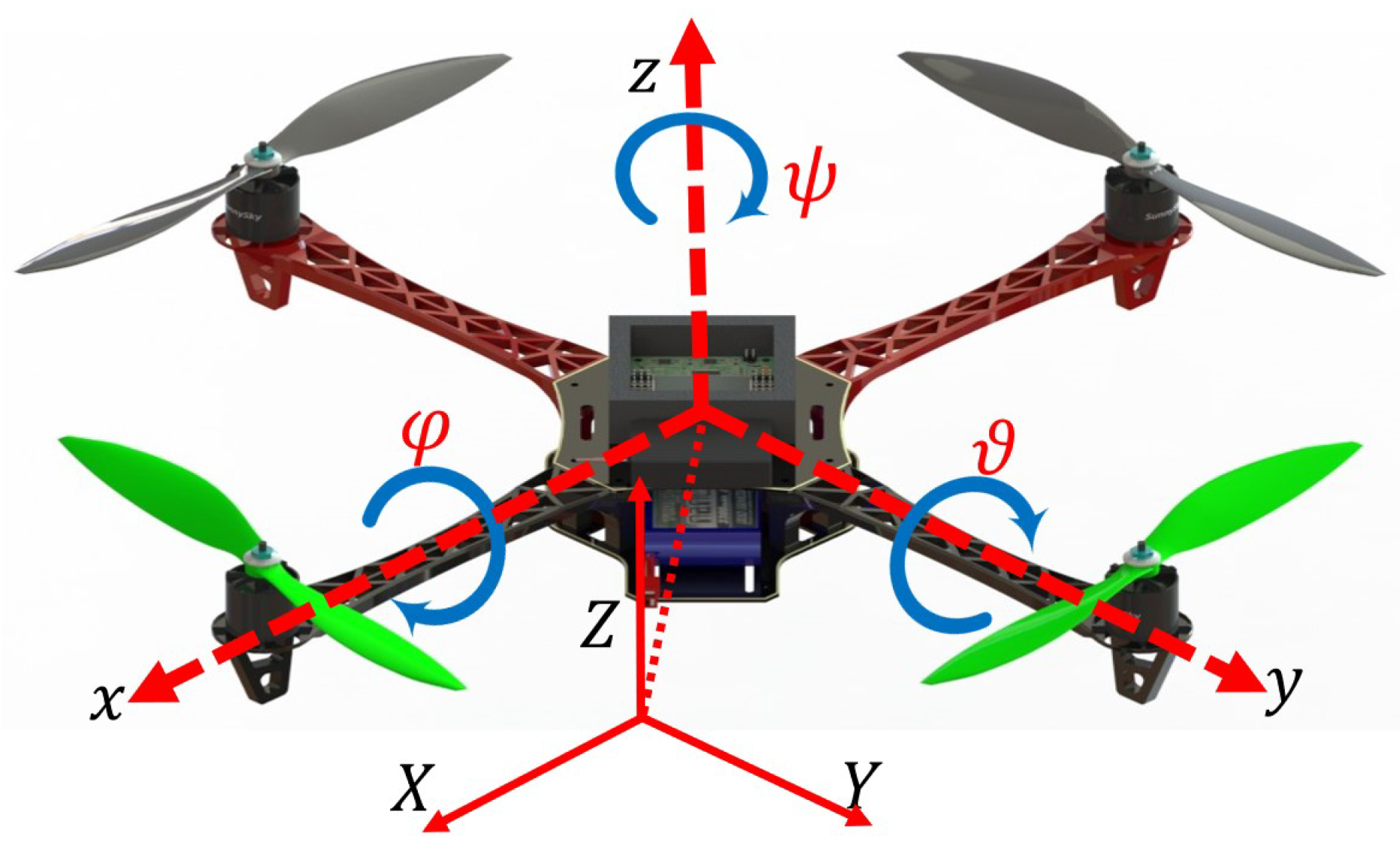
5.1. System Description
5.2. Control Law Design
5.2.1. Fully Actuated Subsystem
5.2.2. Under-Actuated Subsystem
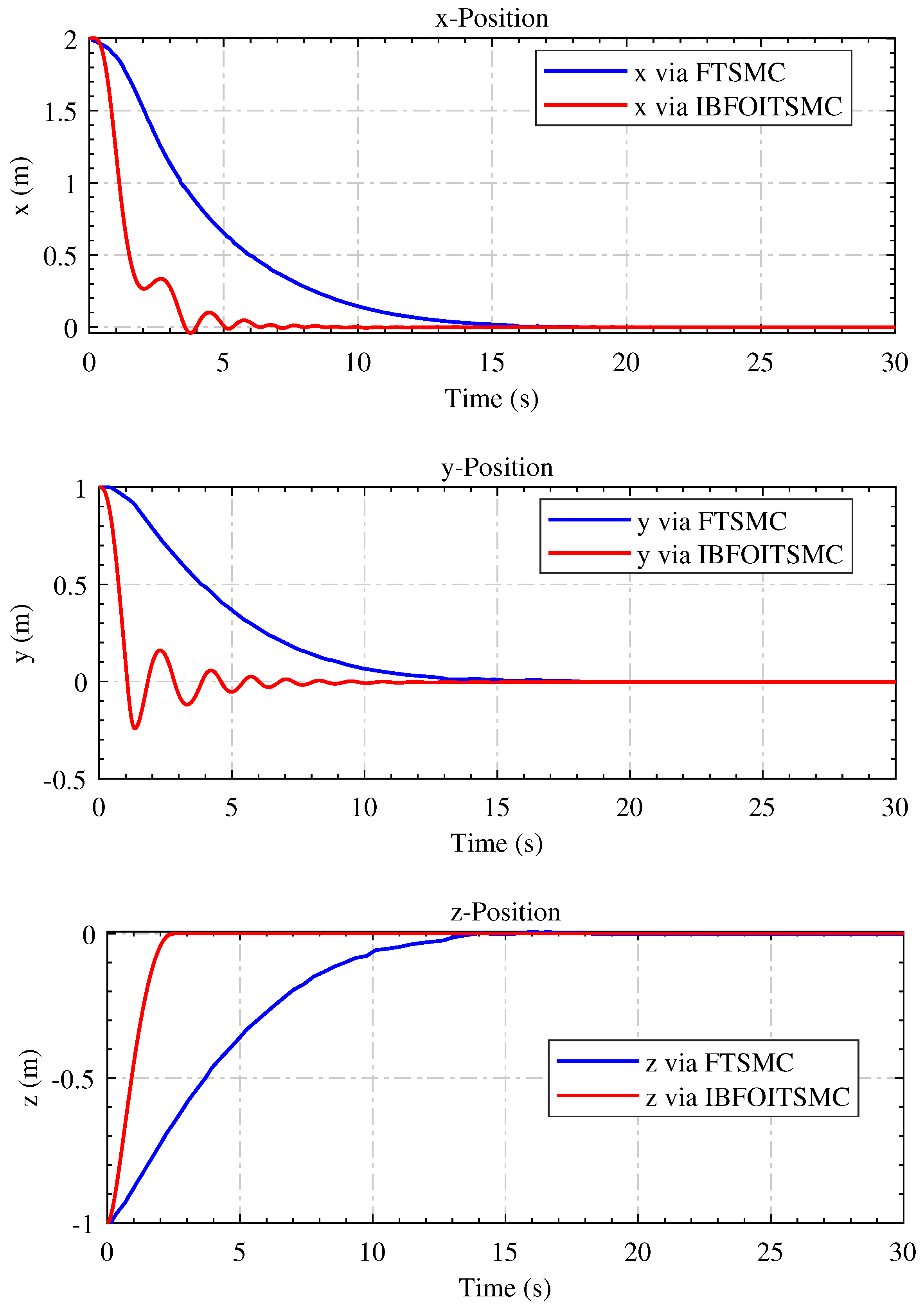
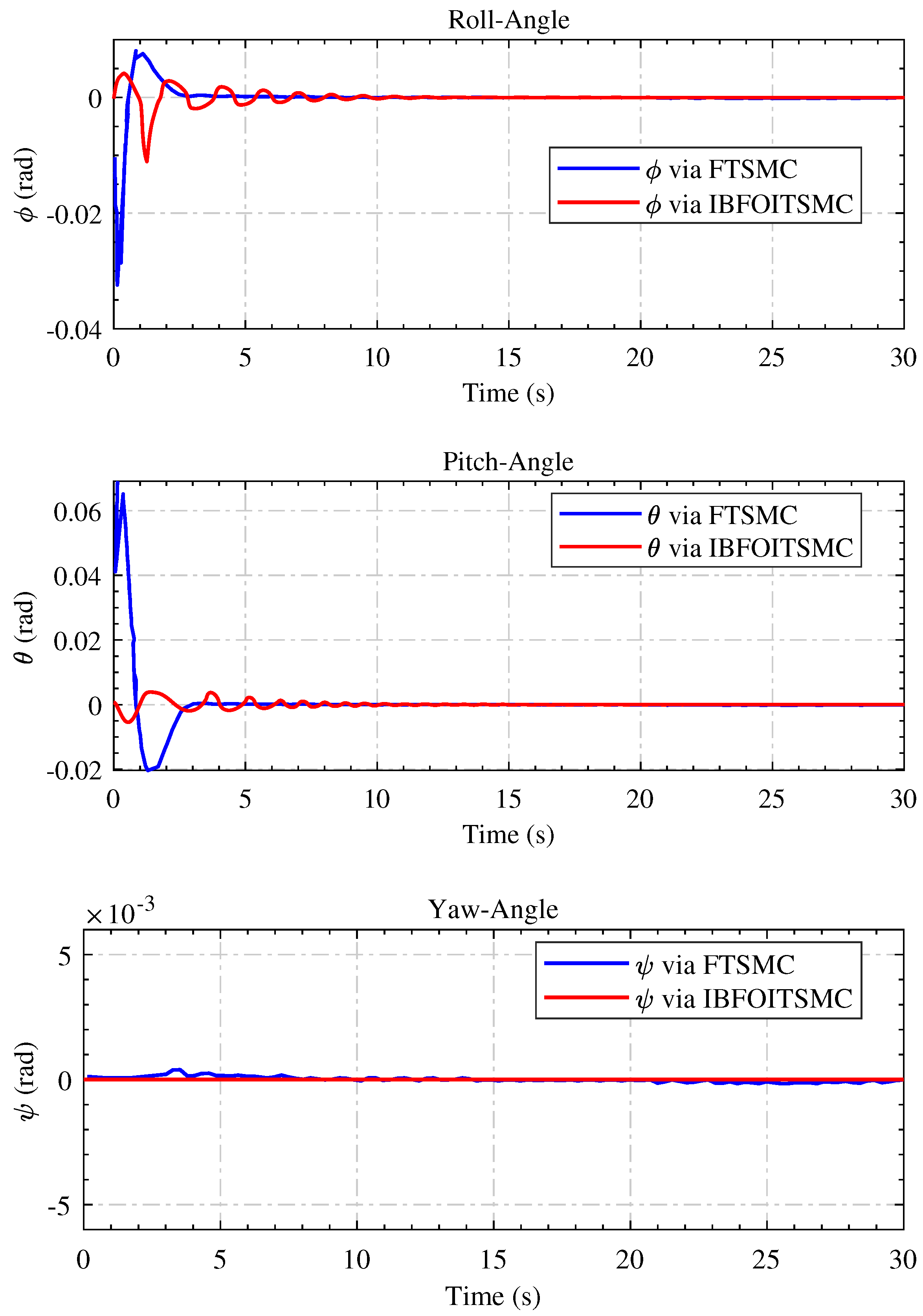
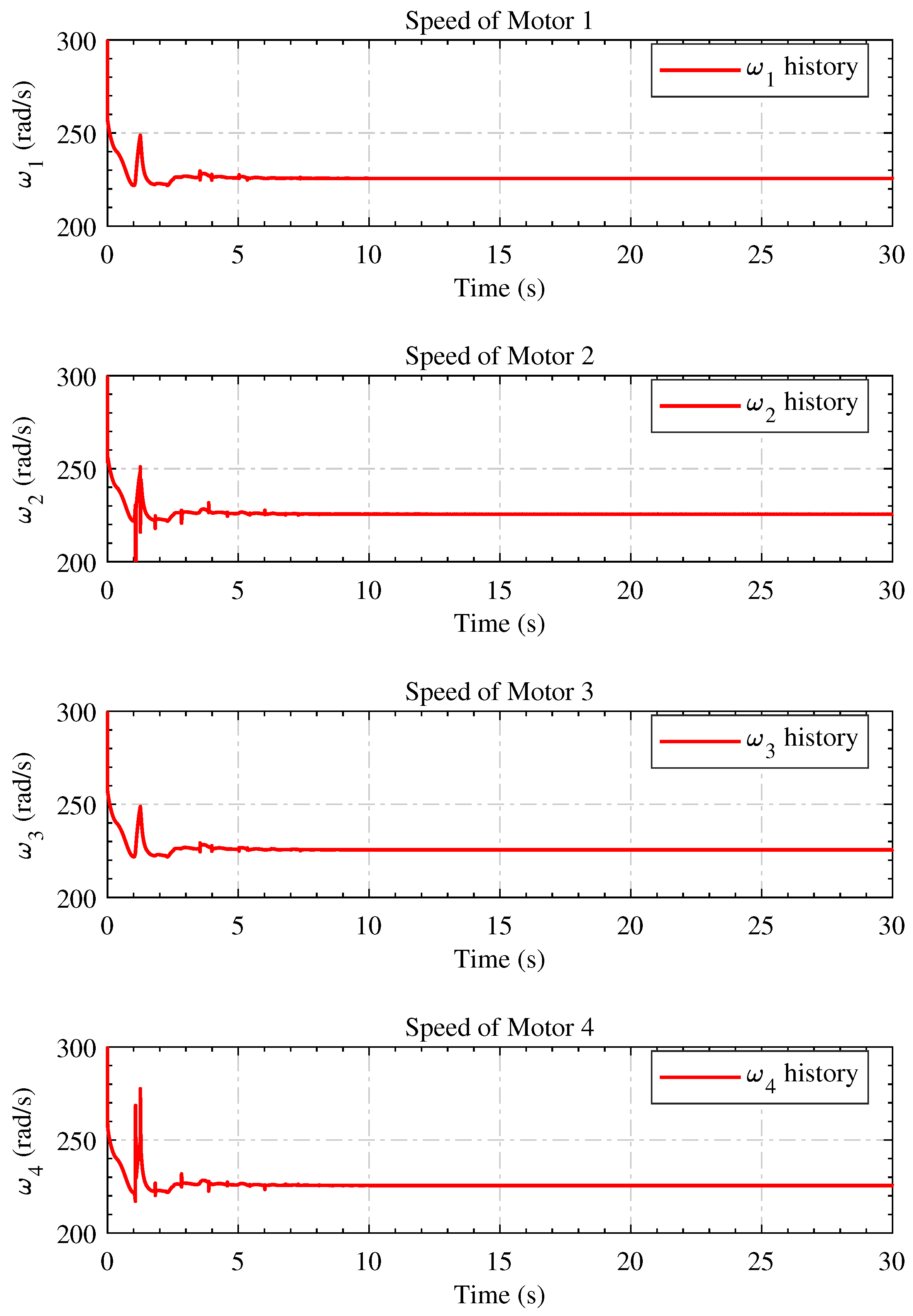
5.3. Simulation Results
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Huang, Z.; Wang, W.; Zeng, B.; Yu, C.; Zhou, Y. Comprehensive Stable Control Strategy for a Typical Underactuated Manipulator Considering Several Uncertainties. Appl. Sci. 2024, 14, 3663. [Google Scholar] [CrossRef]
- Li, N.; Liu, X.; Liu, C.; He, W.; Wang, H. Adaptive Stabilization Control for a Class of Non-Strict Feedback Underactuated Nonlinear Systems by Backstepping. IEEE Trans. Autom. Sci. Eng. 2024. [Google Scholar] [CrossRef]
- Alsaade, F.W.; Jahanshahi, H.; Yao, Q.; Al-zahrani, M.S.; Alzahrani, A.S. On the Development of a Data-Driven-Based Fractional-Order Controller for Unmanned Aerial Vehicles. Fractal Fract. 2023, 7, 236. [Google Scholar] [CrossRef]
- Shojaei, K.; Chatraei, A. Robust platoon control of underactuated autonomous underwater vehicles subjected to nonlinearities, uncertainties and range and angle constraints. Appl. Ocean Res. 2021, 110, 102594. [Google Scholar] [CrossRef]
- Reis, J.; Yu, G.; Silvestre, C. Kalman-based velocity-free trajectory tracking control of an underactuated aerial vehicle with unknown system dynamics. Automatica 2023, 155, 111148. [Google Scholar] [CrossRef]
- Mija, S. Multiswitching Surface Based Sliding Mode Controller for a Class of Underactuated Nonlinear Systems: With Application to Ball and Plate System. IEEE Trans. Ind. Electron. 2024. [Google Scholar] [CrossRef]
- Ullah, S.; Mehmood, A.; Khan, Q.; Rehman, S.; Iqbal, J. Robust Integral Sliding Mode Control Design for Stability Enhancement of Under-actuated Quadcopter. Int. J. Control. Autom. Syst. 2020, 18, 1671–1678. [Google Scholar] [CrossRef]
- Riachy, S.; Orlov, Y.; Floquet, T.; Santiesteban, R.; Richard, J.P. Second-order sliding mode control of underactuated mechanical systems I: Local stabilization with application to an inverted pendulum. Int. J. Robust Nonlinear Control. IFAC-Affil. J. 2008, 18, 529–543. [Google Scholar] [CrossRef]
- de Groot, O.; Valk, L.; Keviczky, T. Cooperative Passivity-Based Control of Nonlinear Mechanical Systems. Robotics 2023, 12, 142. [Google Scholar] [CrossRef]
- Li, Z.; Zeng, J.; Chen, S.; Sreenath, K. Autonomous navigation of underactuated bipedal robots in height-constrained environments. Int. J. Robot. Res. 2023, 42, 565–585. [Google Scholar] [CrossRef]
- Wu, J.; Zhang, P.; Meng, Q.; Wang, Y. Control of Underactuated Manipulators: Design and Optimization; Springer Nature: Berlin/Heidelberg, Germany, 2023. [Google Scholar]
- Ullah, S.; Khan, Q.; Mehmood, A.; Bhatti, A.I. Robust backstepping sliding mode control design for a class of underactuated electro–mechanical nonlinear systems. J. Electr. Eng. Technol. 2020, 15, 1821–1828. [Google Scholar] [CrossRef]
- Xuan-Mung, N.; Golestani, M.; Nguyen, H.T.; Nguyen, N.A.; Fekih, A. Output feedback control for spacecraft attitude system with practical predefined-time stability based on anti-windup compensator. Mathematics 2023, 11, 2149. [Google Scholar] [CrossRef]
- Yu, K.; Li, Y.; Lv, M.; Tong, S. Adaptive Backstepping Control for Underactuated 6-DoF USV with Uncertain Dynamics. IEEE Trans. Intell. Veh. 2024. [Google Scholar] [CrossRef]
- Yang, N.; Shen, C.; Song, Z.; Johnson-Roberson, M.; Sun, J. Robust Energy-Optimal Control for 3-D Path-Following of Autonomous Underwater Vehicles Under Ocean Currents. IEEE Trans. Control. Syst. Technol. 2023, 32, 680–687. [Google Scholar] [CrossRef]
- Mason, P.; Broucke, M.; Piccoli, B. Time optimal swing-up of the planar pendulum. IEEE Trans. Autom. Control 2008, 53, 1876–1886. [Google Scholar] [CrossRef]
- Kristiansen, B.A.; Gravdahl, J.T.; Johansen, T.A. Energy optimal attitude control for a solar-powered spacecraft. Eur. J. Control 2021, 62, 192–197. [Google Scholar] [CrossRef]
- Cui, Z.; Liu, L.; Zhu, B.; Zhang, L.; Yu, Y.; Zhao, Z.; Li, S.; Liu, M. Spiral Dive Control of Underactuated AUV Based on a Single-Input Fractional-Order Fuzzy Logic Controller. Fractal Fract. 2022, 6, 519. [Google Scholar] [CrossRef]
- Ichida, K.; Watanabe, K.; Izumi, K.; Uchida, N. Fuzzy switching control of underactuated manipulators with approximated switching regions. In Proceedings of the 2006 IEEE/RSJ International Conference on Intelligent Robots and Systems, Beijing, China, 9–15 October 2006; IEEE: New York, NY, USA, 2006; pp. 586–591. [Google Scholar]
- Raguraman, S.; Tamilselvi, D.; Shivakumar, N. Mobile robot navigation using fuzzy logic controller. In Proceedings of the 2009 International Conference on Control, Automation, Communication and Energy Conservation, Perundurai, India, 4–6 June 2009; IEEE: New York, NY, USA, 2009; pp. 1–5. [Google Scholar]
- Aslam, J.; Qin, S.Y.; Alvi, M.A. Fuzzy sliding mode control algorithm for a four-wheel skid steer vehicle. J. Mech. Sci. Technol. 2014, 28, 3301–3310. [Google Scholar] [CrossRef]
- Nguyen, N.P.; Oh, H.; Kim, Y.; Moon, J.; Yang, J.; Chen, W.H. Fuzzy-based super-twisting sliding mode stabilization control for under-actuated rotary inverted pendulum systems. IEEE Access 2020, 8, 185079–185092. [Google Scholar] [CrossRef]
- Mousavi, A.; Markazi, A.H. A predictive approach to adaptive fuzzy sliding-mode control of under-actuated nonlinear systems with input saturation. Int. J. Syst. Sci. 2021, 52, 1599–1617. [Google Scholar] [CrossRef]
- Wang, X.; Duan, G. High-order fully actuated system approaches: Model predictive control with applications to under-actuated systems. J. Frankl. Inst. 2023, 360, 6953–6975. [Google Scholar] [CrossRef]
- Yao, X.Y.; Park, J.H.; Ding, H.F.; Ge, M.F. Event-triggered consensus control for networked underactuated robotic systems. IEEE Trans. Cybern. 2020, 52, 2896–2906. [Google Scholar] [CrossRef] [PubMed]
- Dai, S.L.; He, S.; Cai, H.; Yang, C. Adaptive leader–follower formation control of underactuated surface vehicles with guaranteed performance. IEEE Trans. Syst. Man, Cybern. Syst. 2020, 52, 1997–2008. [Google Scholar] [CrossRef]
- Zheng, W.; Tang, T.L.; Wang, H.B. Distributed leaderless consensus for a class of multiple underactuated systems with unknown nonlinearities and external disturbances. Int. J. Control. Autom. Syst. 2022, 20, 1449–1460. [Google Scholar] [CrossRef]
- Ullah, S.; Khan, Q.; Mehmood, A.; Akmeliawati, R. Integral backstepping based robust integral sliding mode control of underactuated nonlinear electromechanical systems. J. Control Eng. Appl. Inf. 2019, 21, 42–50. [Google Scholar]
- Ullah, S.; Khan, Q.; Zaidi, M.M.; Hua, L.G. Neuro-adaptive non-singular terminal sliding mode control for distributed fixed-time synchronization of higher-order uncertain multi-agent nonlinear systems. Inf. Sci. 2024, 659, 120087. [Google Scholar] [CrossRef]
- Ullah, S.; Khan, Q.; Mehmood, A. Neuro-adaptive fixed-time non-singular fast terminal sliding mode control design for a class of under-actuated nonlinear systems. Int. J. Control 2023, 96, 1529–1542. [Google Scholar] [CrossRef]
- Yang, T.; Chen, H.; Sun, N.; Fang, Y. Adaptive neural network output feedback control of uncertain underactuated systems with actuated and unactuated state constraints. IEEE Trans. Syst. Man, Cybern. Syst. 2021, 52, 7027–7043. [Google Scholar] [CrossRef]
- Efe, M.Ö. Integral sliding mode control of a quadrotor with fractional order reaching dynamics. Trans. Inst. Meas. Control 2011, 33, 985–1003. [Google Scholar] [CrossRef]
- Shi, X.; Cheng, Y.; Yin, C.; Dadras, S.; Huang, X. Design of fractional-order backstepping sliding mode control for quadrotor UAV. Asian J. Control 2019, 21, 156–171. [Google Scholar] [CrossRef]
- Qi, W.; Zong, G.; Karim, H.R. Observer-based adaptive SMC for nonlinear uncertain singular semi-Markov jump systems with applications to DC motor. IEEE Trans. Circuits Syst. I Regul. Pap. 2018, 65, 2951–2960. [Google Scholar] [CrossRef]
- Bartolini, G.; Ferrara, A.; Usai, E. Chattering avoidance by second-order sliding mode control. IEEE Trans. Autom. Control 1998, 43, 241–246. [Google Scholar] [CrossRef]
- Levant, A. Quasi-continuous high-order sliding-mode controllers. In Proceedings of the 42nd IEEE Conference Proceedings on Decision and Control, Maui, HI, USA, 9–12 December 2003; IEEE: New York, NY, USA, 2003; Volume 5, pp. 4605–4610. [Google Scholar]
- Utkin, V.; Guldner, J.; Shi, J. Sliding Mode Control in Electro-Mechanical Systems; CRC Press: Boca Raton, FL, USA, 2009. [Google Scholar]
- Khan, Q.; Akmeliawati, R.; Bhatti, A.I.; Khan, M.A. Robust stabilization of underactuated nonlinear systems: A fast terminal sliding mode approach. ISA Trans. 2017, 66, 241–248. [Google Scholar] [CrossRef] [PubMed]
- Olfati-Saber, R. Nonlinear Control of Underactuated Mechanical Systems with Application to Robotics and Aerospace Vehicles. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 2001. [Google Scholar]
- Bouabdallah, S. Design and Control of Quadrotors with Application to Autonomous Flying; Technical Report; Epfl: Lausanne, Switzerland, 2007. [Google Scholar]
- Ullah, S.; Khan, Q.; Mehmood, A.; Kirmani, S.A.M.; Mechali, O. Neuro-adaptive fast integral terminal sliding mode control design with variable gain robust exact differentiator for under-actuated quadcopter UAV. ISA Trans. 2022, 120, 293–304. [Google Scholar] [CrossRef] [PubMed]
- Yun, H.; Zongyu, Z.; Zhiguang, S. Fixed-time terminal sliding mode trajectory tracking control of quadrotor helicopter. In Proceedings of the 2015 34th Chinese Control Conference (CCC), Hangzhou, China, 28–30 July 2015; IEEE: New York, NY, USA, 2015; pp. 4361–4366. [Google Scholar]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ullah, S.; Alghamdi, H.; Algethami, A.A.; Alghamdi, B.; Hafeez, G. Robust Control Design of Under-Actuated Nonlinear Systems: Quadcopter Unmanned Aerial Vehicles with Integral Backstepping Integral Terminal Fractional-Order Sliding Mode. Fractal Fract. 2024, 8, 412. https://doi.org/10.3390/fractalfract8070412
Ullah S, Alghamdi H, Algethami AA, Alghamdi B, Hafeez G. Robust Control Design of Under-Actuated Nonlinear Systems: Quadcopter Unmanned Aerial Vehicles with Integral Backstepping Integral Terminal Fractional-Order Sliding Mode. Fractal and Fractional. 2024; 8(7):412. https://doi.org/10.3390/fractalfract8070412
Chicago/Turabian StyleUllah, Safeer, Hisham Alghamdi, Abdullah A. Algethami, Baheej Alghamdi, and Ghulam Hafeez. 2024. "Robust Control Design of Under-Actuated Nonlinear Systems: Quadcopter Unmanned Aerial Vehicles with Integral Backstepping Integral Terminal Fractional-Order Sliding Mode" Fractal and Fractional 8, no. 7: 412. https://doi.org/10.3390/fractalfract8070412
APA StyleUllah, S., Alghamdi, H., Algethami, A. A., Alghamdi, B., & Hafeez, G. (2024). Robust Control Design of Under-Actuated Nonlinear Systems: Quadcopter Unmanned Aerial Vehicles with Integral Backstepping Integral Terminal Fractional-Order Sliding Mode. Fractal and Fractional, 8(7), 412. https://doi.org/10.3390/fractalfract8070412







