Properties and Applications of Complex Fractal–Fractional Operators in the Open Unit Disk
Abstract
1. Introduction
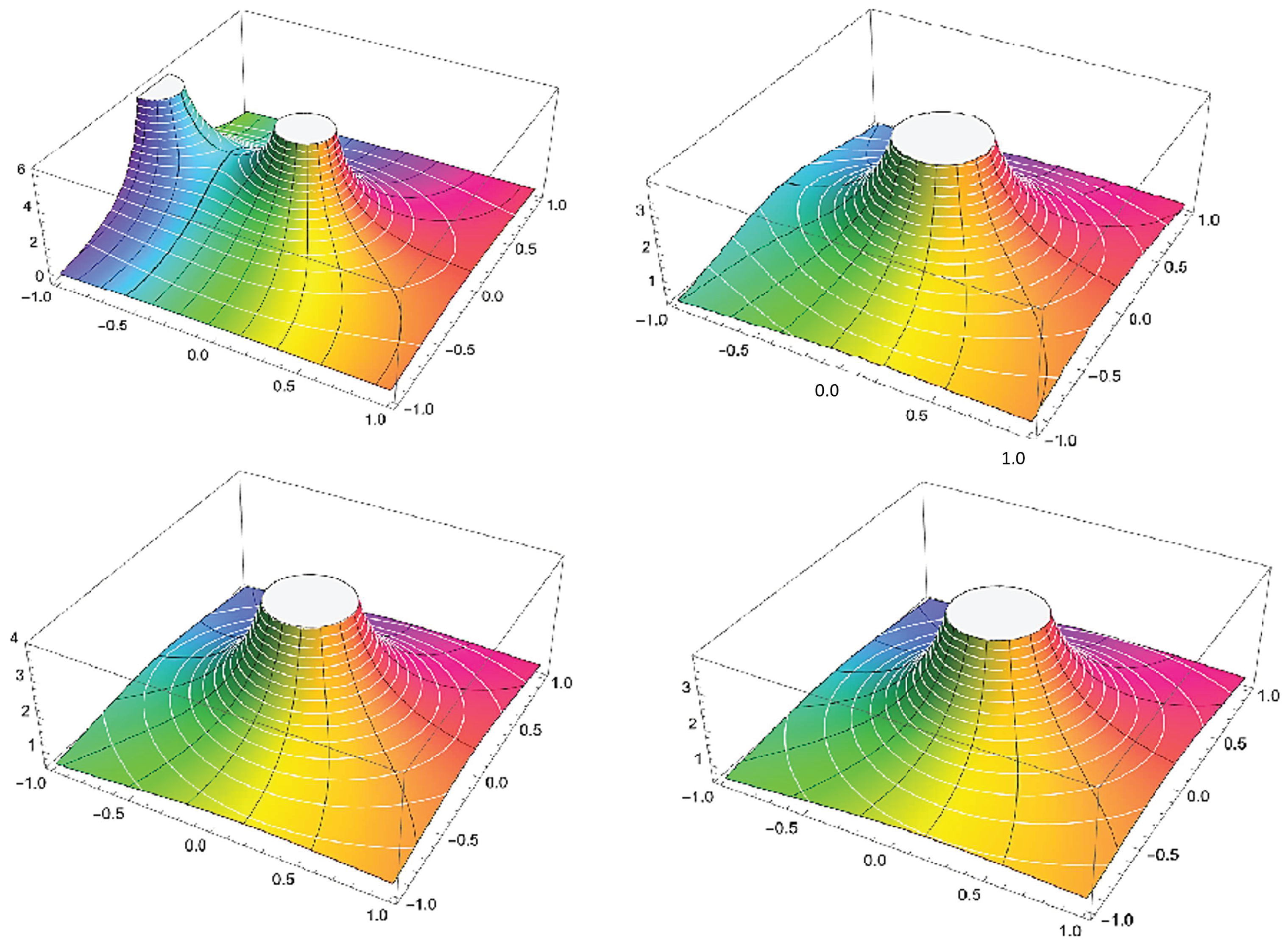
2. Illustrations of Fractal–Fractional Operator
3. Complex Fractal–Fractional Operators
- with
- whenever
- whenever
- The fractal–fractional operators can be regarded as members of the class of normalized analytic functions in the unit disk, as demonstrated by Theorem 1. In other words, they are converted from the normalized analytic function into a function that belongs to the same class. We may analyze the fractal–fractional operators in the unit disk geometrically thanks to this feature.
- Furthermore, Theorem 1 suggests that the normalized Fox–Wright functions in the unit disk may be seen as entangled operators with fractal–fractional operators. However, this unique function possesses a geometrical characteristic in the open unit disk given specific argument circumstances (see [11]).
- Three complex analytic functions are used to formulate the operator : the fundamental function σ, the extreme function of starlikeness, and the normalized Fox-Wright function. But, the operator is structured by double analytic functions. In the same way, for the integral operator As a conclusion of this point, the normalized fractal–fractional operators can be checked geometrically based on the properties of (see Theorem 2).
4. Main Results
- (a)
- or
- (b)
- together with the inequalitythen has a convex conduct.
5. Boundedness of ℓ-Fractal–Fractional Operator
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Atangana, A. Fractal-fractional differentiation and integration: Connecting fractal calculus and fractional calculus to predict complex system. Chaos Solitons Fractals 2017, 102, 396–406. [Google Scholar] [CrossRef]
- Murtaza, S.; Kumam, P.; Kaewkhao, A.; Khan, N.; Ahmad, Z. Fractal fractional analysis of non linear electro osmotic flow with cadmium telluride nanoparticles. Sci. Rep. 2022, 12, 20226. [Google Scholar] [CrossRef] [PubMed]
- Khan, H.; Alam, K.; Gulzar, H.; Etemad, S.; Rezapour, S. A case study of fractal-fractional tuberculosis model in China: Existence and stability theories along with numerical simulations. Math. Comput. Simul. 2022, 198, 455–473. [Google Scholar] [CrossRef]
- Avci, I.; Hussain, A.; Kanwal, T. Investigating the impact of memory effects on computer virus population dynamics: A fractal-fractional approach with numerical analysis. Chaos Solitons Fractals 2023, 174, 113845. [Google Scholar] [CrossRef]
- Sabbar, Y.; Din, A.; Kiouach, D. Influence of fractal-fractional differentiation and independent quadratic Levy jumps on the dynamics of a general epidemic model with vaccination strategy. Chaos Solitons Fractals 2023, 171, 113434. [Google Scholar] [CrossRef]
- Diaz, R.; Pariguan, E. On Hypergeometric Functions and Pochhammer K-symbol. Divulg. Mat. 2007, 15, 179–192. [Google Scholar]
- Yildiz, C.; Cotirla, L.I. Examining the Hermite-Hadamard Inequalities for k-Fractional Operators Using the Green Function. Fractal Fract. 2023, 7, 161. [Google Scholar] [CrossRef]
- Ibrahim, R.W. K-symbol fractional order discrete-time models of Lozi system. J. Differ. Eqs. Appl. 2022, 29, 1045–1064. [Google Scholar] [CrossRef]
- Hadid, S.B.; Ibrahim, R.W. Geometric Study of 2D-Wave Equations in View of K-Symbol Airy Functions. Axioms 2022, 11, 590. [Google Scholar] [CrossRef]
- Ibrahim, R.W. Studies in fractal-fractional operators with examples. Ex. Counterexamples 2024, 6, 100148. [Google Scholar] [CrossRef]
- Kumar, A.; Mondal, S.R.; Das, S. Certain Geometric Properties of the Fox-Wright Functions. Axioms 2022, 11, 629. [Google Scholar] [CrossRef]
- Guney, H.O.; Acu, M.; Breaz, D.; Owa, S. Applications of fractional derivatives for Alexander integral operator. Afr. Mat. 2021, 32, 673–683. [Google Scholar] [CrossRef]
- MacGregor, T.H. A class of univalent functions. Proc. Am. Math. Soc. 1964, 15, 311–317. [Google Scholar] [CrossRef]
- Mocanu, P. Some starlikeness conditions for analytic functions. Rev. Roum. Math. Pures Appl. 1988, 33, 117–124. [Google Scholar]
- Stankiewicz, J.; Stankiewicz, Z. Some applications of the Hadamard convolution in the theory of functions. Ann. Univ. Mariae Curie-Sklodowska Sect. A 1986, 40, 251–265. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Attiya, A.A.; Salahshour, S.; Ibrahim, R.W.; Yassen, M.F. Properties and Applications of Complex Fractal–Fractional Operators in the Open Unit Disk. Fractal Fract. 2024, 8, 584. https://doi.org/10.3390/fractalfract8100584
Attiya AA, Salahshour S, Ibrahim RW, Yassen MF. Properties and Applications of Complex Fractal–Fractional Operators in the Open Unit Disk. Fractal and Fractional. 2024; 8(10):584. https://doi.org/10.3390/fractalfract8100584
Chicago/Turabian StyleAttiya, Adel A., Soheil Salahshour, Rabha W. Ibrahim, and Mansour F. Yassen. 2024. "Properties and Applications of Complex Fractal–Fractional Operators in the Open Unit Disk" Fractal and Fractional 8, no. 10: 584. https://doi.org/10.3390/fractalfract8100584
APA StyleAttiya, A. A., Salahshour, S., Ibrahim, R. W., & Yassen, M. F. (2024). Properties and Applications of Complex Fractal–Fractional Operators in the Open Unit Disk. Fractal and Fractional, 8(10), 584. https://doi.org/10.3390/fractalfract8100584





