The Multiscale Principle in Nature (Principium luxuriæ): Linking Multiscale Thermodynamics to Living and Non-Living Complex Systems
Abstract
1. Introduction
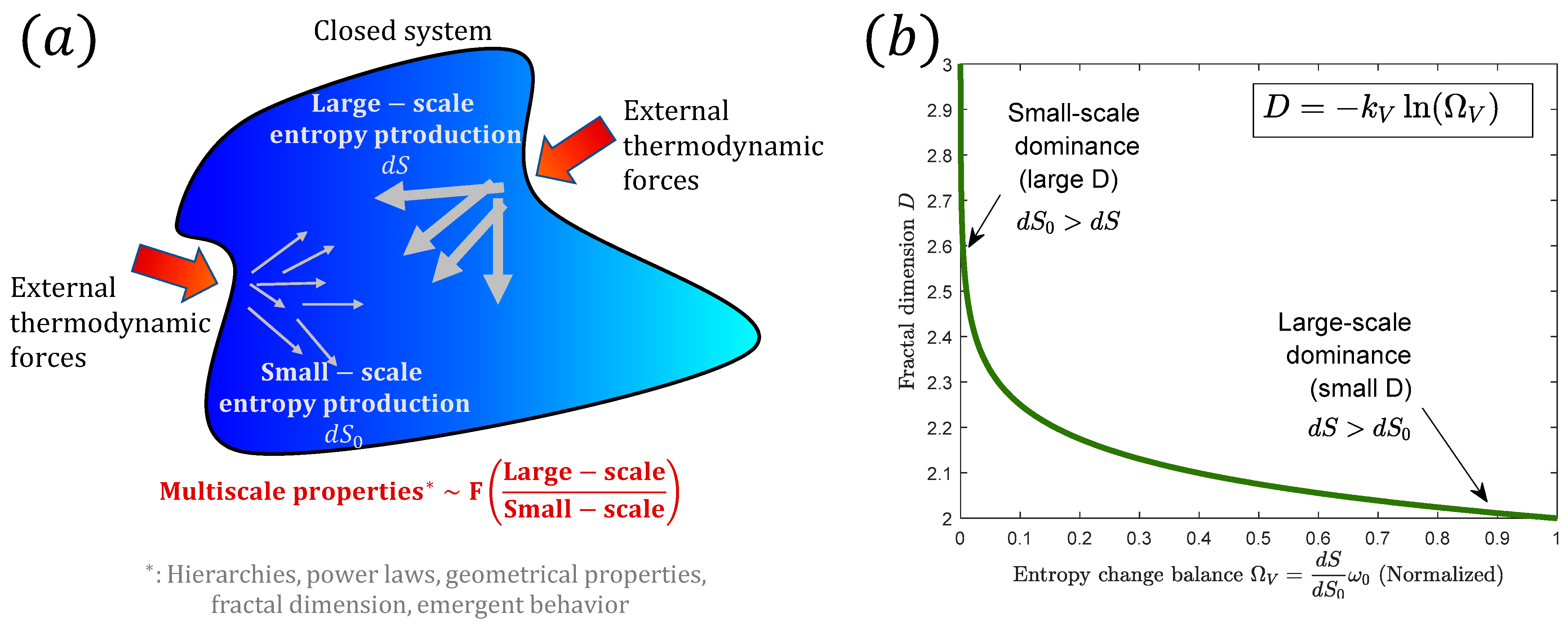
2. The Multiscale Entropy
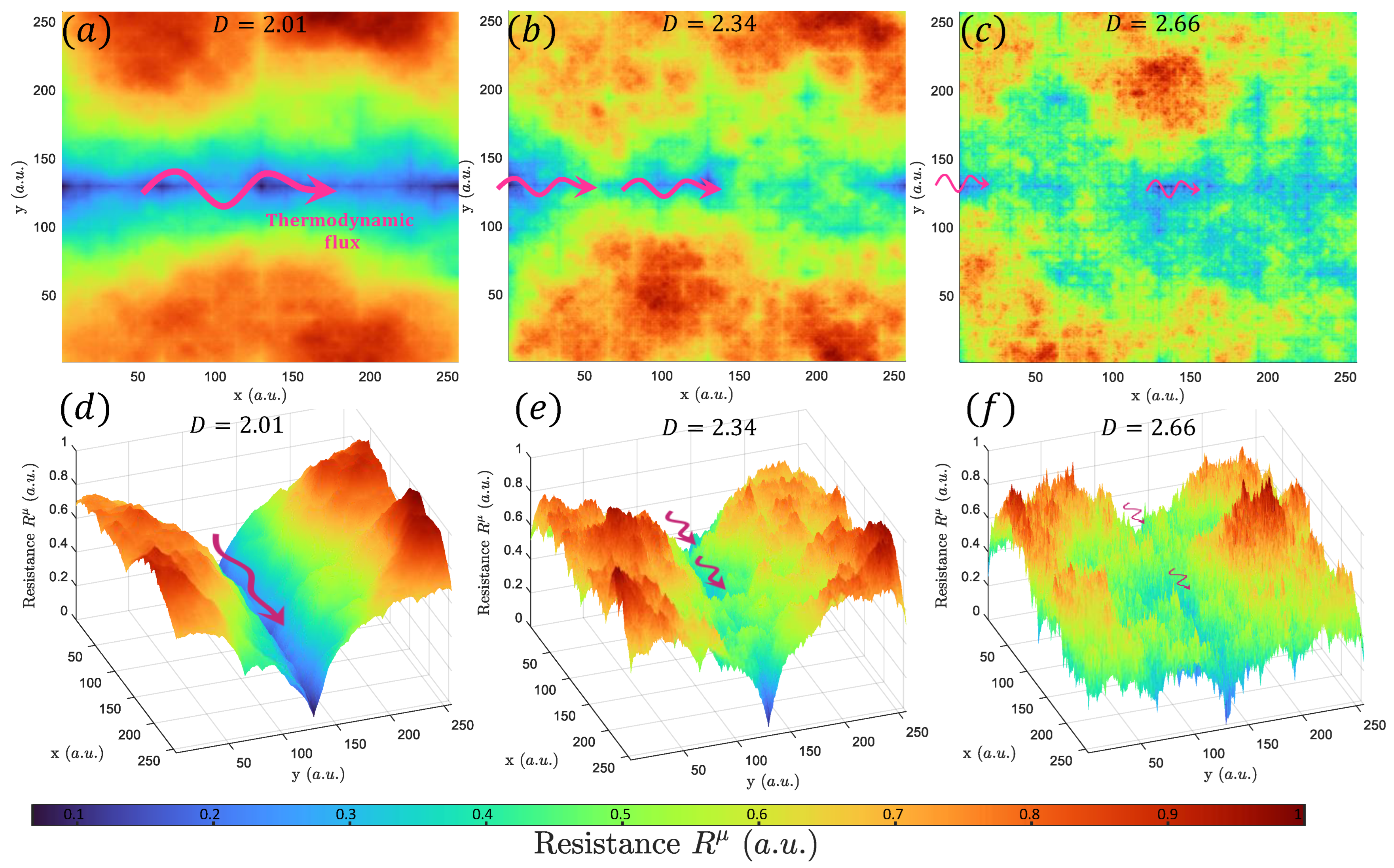
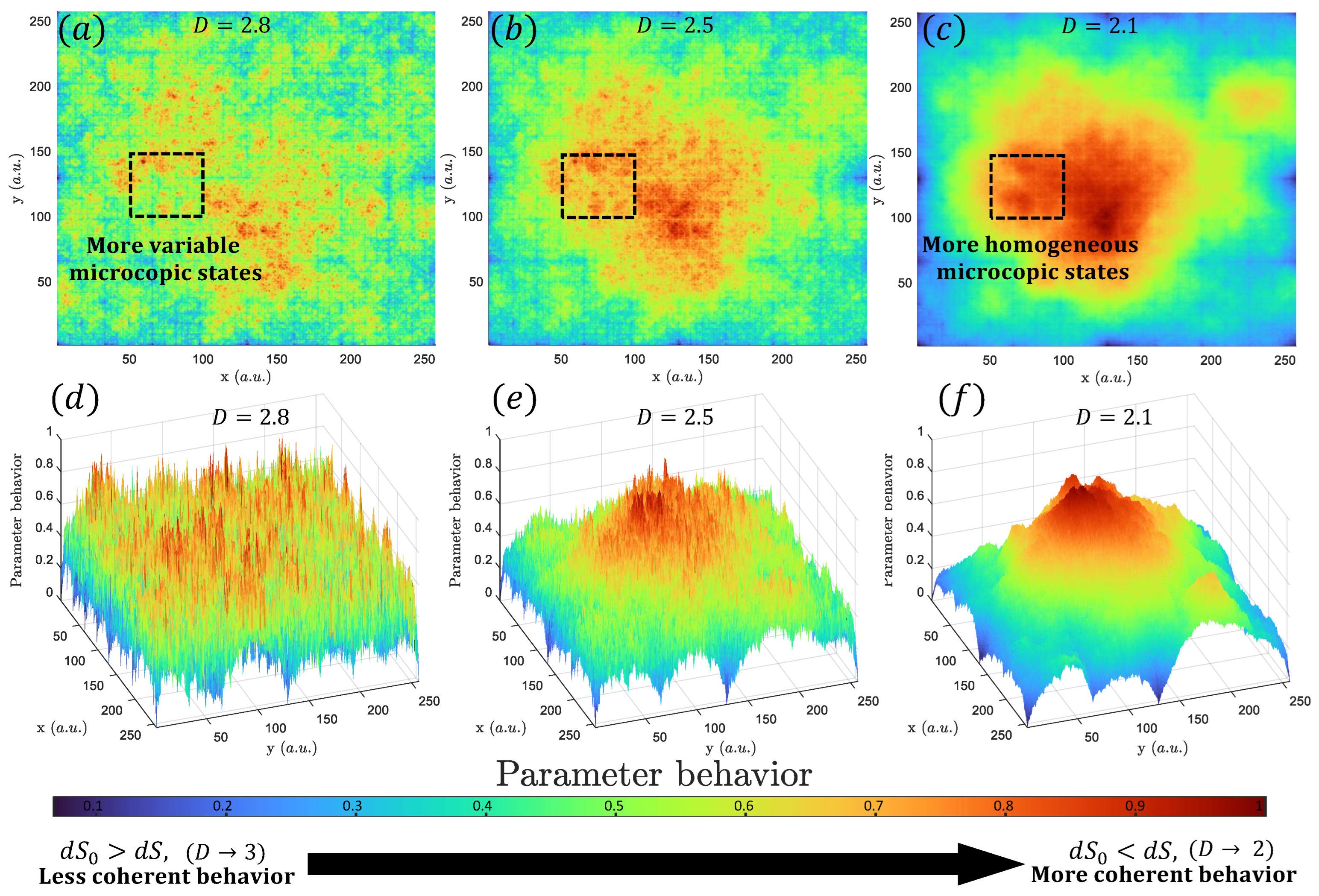
3. On the Emergent Behavior
4. The Nature of Fluctuations and Dissipation in Multiscale Systems
4.1. Temperature and Critical Phenomena in Biological Systems
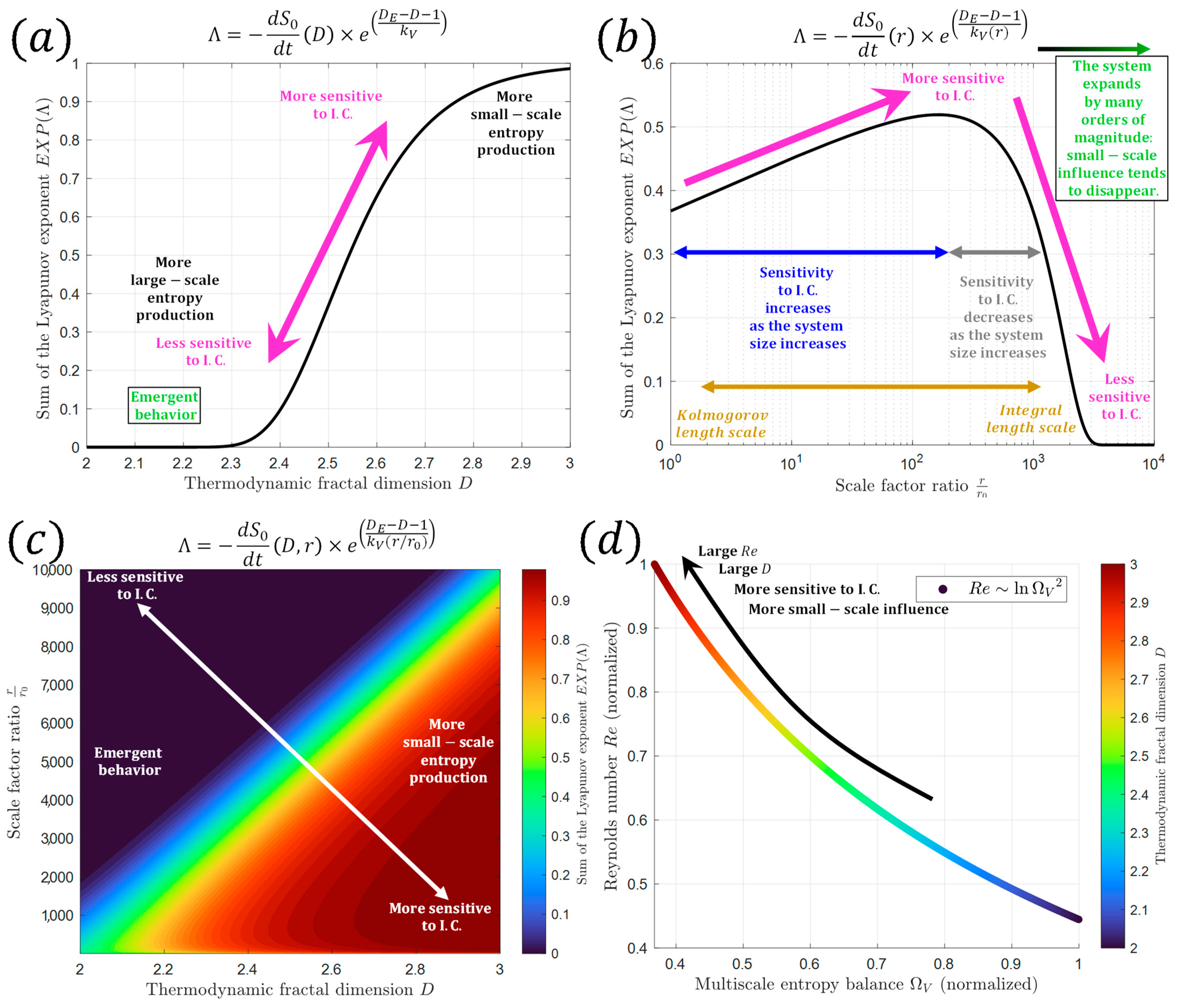
5. Chaos Theory
6. Turbulence
7. Other Examples
7.1. Complexity and Cosmic Evolution
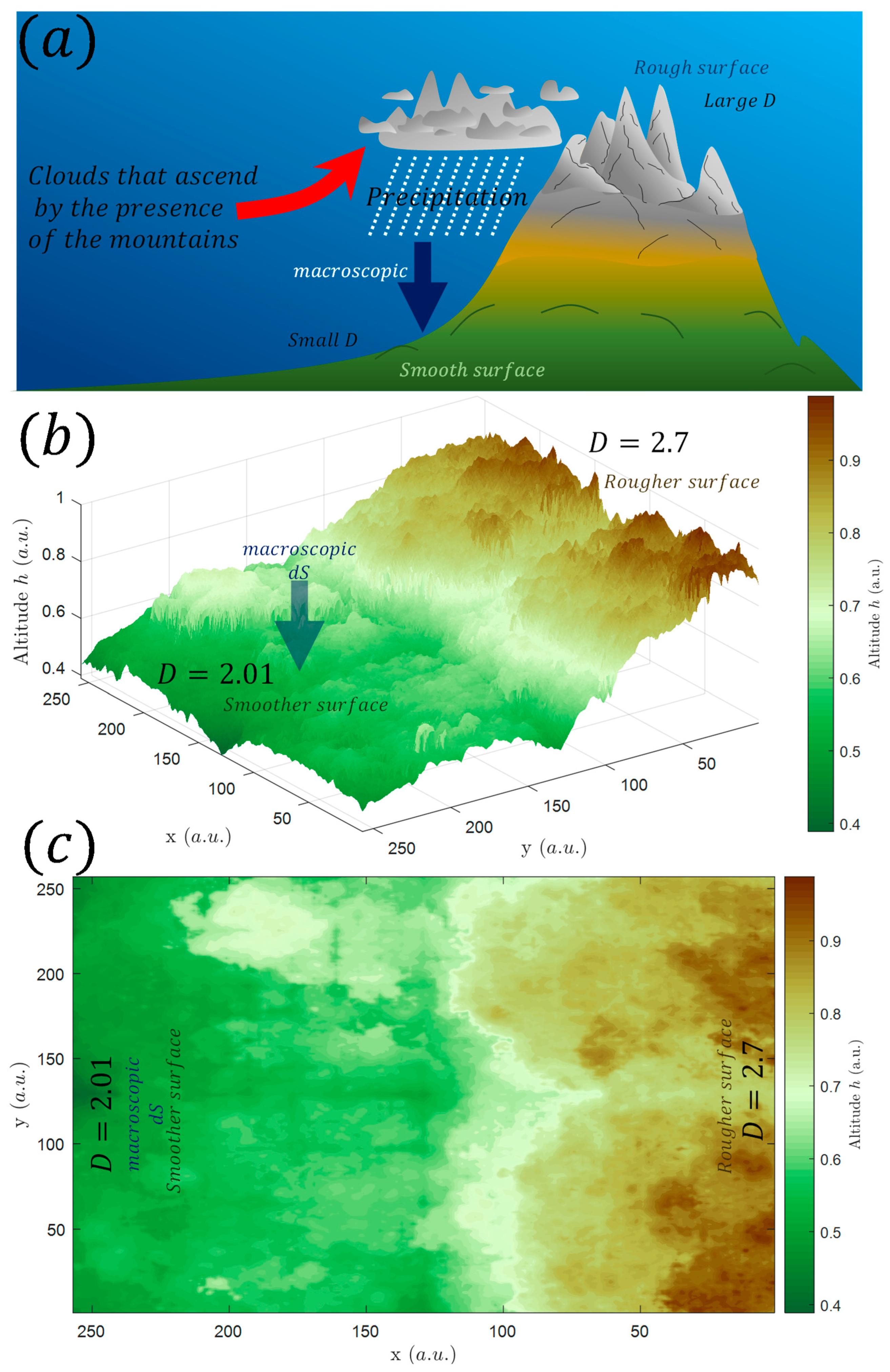
7.2. Mountain Range Geomorphology
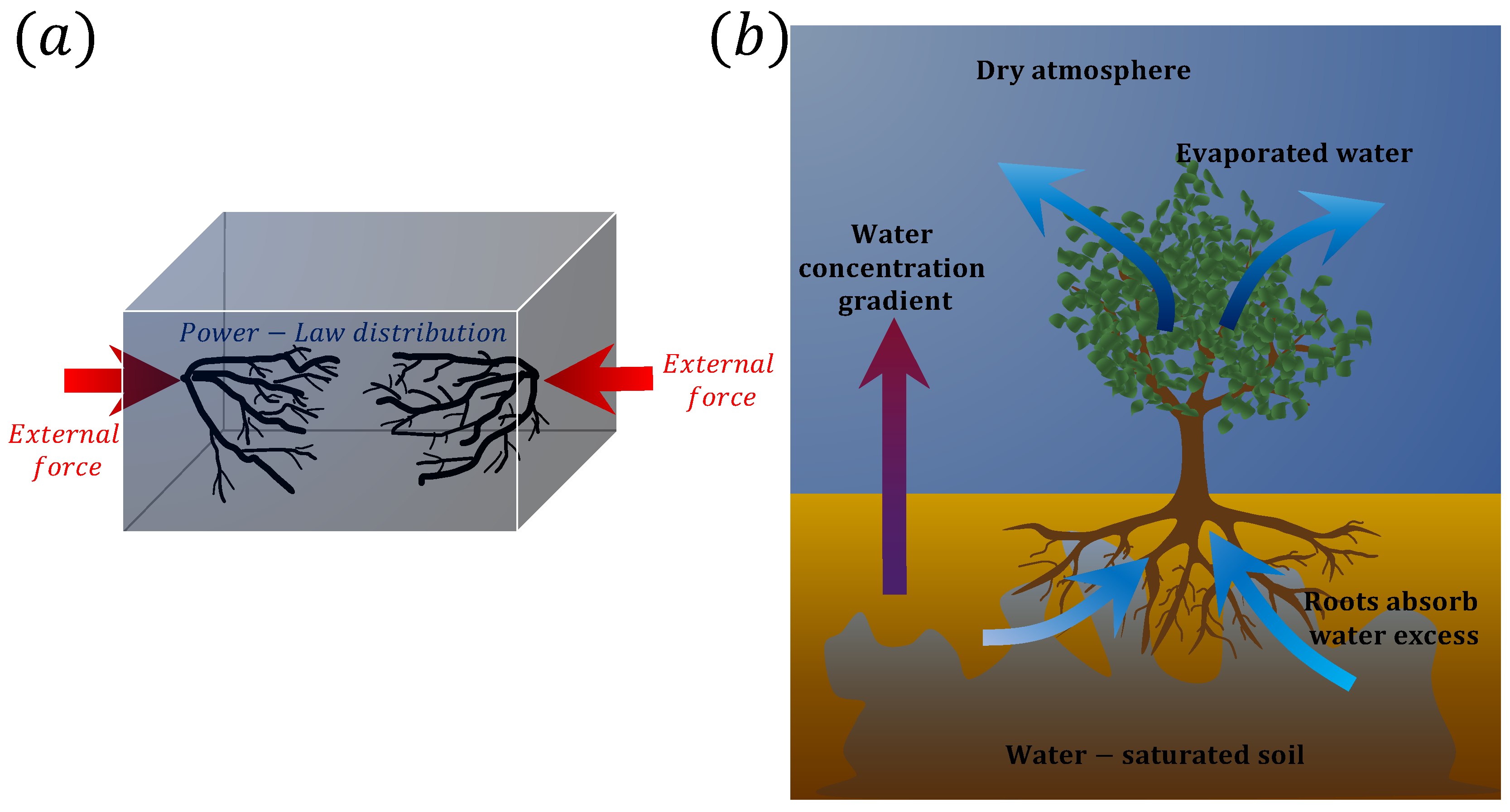
7.3. Tree’s Transpiration Process and Life System Governed by Power Laws
7.4. Geometry of Human Organs and Aging
7.5. Human Brain and Cognition
7.6. Evolutionary Moral
7.7. Updating the Perspective of Natural Selection and Biological Death
8. Summary and Discussion
9. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. The Nonadditive Multiscale Thermodynamics
Appendix B. Homeostasis
References
- Bar-Yam, Y. Dynamics of Complex Systems; Westview Press: Boulder, CO, USA, 2003. [Google Scholar]
- Boccara, N. Modeling Complex Systems; Springer: New York, NY, USA, 2010. [Google Scholar]
- West, G.B. Scale: The Universal Laws of Growth, Innovation, Sustainability, and the Pace of Life in Organisms, Cities, Economies, and Companies; Penguin Press: London, UK, 2017. [Google Scholar]
- Ladyman, J.; Wiesner, K. What is a Complex System? Yale University Press: New Haven, CT, USA, 2020. [Google Scholar] [CrossRef]
- Vitiello, G. Fractals, coherent states and self-similarity induced noncommutative geometry. Phys. Lett. A 2012, 376, 2527–2532. [Google Scholar] [CrossRef]
- Nigmatullin, R.R.; Chen, Y. Self-Similarity Principle and the General Theory of Fractal Elements: How to Fit a Random Curve with a Clearly Expressed Trend? Mathematics 2023, 11, 2781. [Google Scholar] [CrossRef]
- Pinto, C.M.; Lopes, A.M.; Machado, J.T. Double power laws, fractals and self-similarity. Appl. Math. Model. 2014, 38, 4019–4026. [Google Scholar] [CrossRef]
- Ziepke, A.; Maryshev, I.; Aranson, I.S.; Frey, E. Multi-scale organization in communicating active matter. Nat. Commun. 2022, 13, 6727. [Google Scholar] [CrossRef] [PubMed]
- Yao, Z.; Lundqvist, E.; Kuang, Y.; Ardoña, H.A.M. Engineering Multi-Scale Organization for Biotic and Organic Abiotic Electroactive Systems. Adv. Sci. 2023, 10, 2205381. [Google Scholar] [CrossRef] [PubMed]
- An, G. Introduction of an agent-based multi-scale modular architecture for dynamic knowledge representation of acute inflammation. Theor. Biol. Med. Model. 2008, 5, 11. [Google Scholar] [CrossRef] [PubMed]
- Buizer, M.; Arts, B.; Kok, K. Governance, Scale and the Environment: The Importance of Recognizing Knowledge Claims in Transdisciplinary Arenas. Ecol. Soc. 2011, 16, 21. [Google Scholar] [CrossRef]
- del Castillo, L.F.; Vera-Cruz, P. Thermodynamic Formulation of Living Systems and Their Evolution. J. Mod. Phys. 2011, 02, 379–391. [Google Scholar] [CrossRef]
- Gatti, R.C. The fractal nature of the latitudinal biodiversity gradient. Biologia 2016, 71, 669–672. [Google Scholar] [CrossRef]
- Yu, J.S.; Bagheri, N. Multi-class and multi-scale models of complex biological phenomena. Curr. Opin. Biotechnol. 2016, 39, 167–173. [Google Scholar] [CrossRef]
- Schaffer, L.V.; Ideker, T. Mapping the multiscale structure of biological systems. Cell Syst. 2021, 12, 622–635. [Google Scholar] [CrossRef] [PubMed]
- Einstein, A. Bemerkung zu der Franz Seletyschen Arbeit “Beiträge zum kosmologischen System”. Ann. Phys. 1922, 374, 436–438. [Google Scholar] [CrossRef]
- Bremer, K.; Wanntorp, H.-E. Hierarchy and Reticulation in Systematics. Syst. Zool. 1979, 28, 624. [Google Scholar] [CrossRef]
- Cilliers, P. Boundaries, Hierarchies and Networks in Complex Systems. Int. J. Innov. Manag. 2001, 5, 135–147. [Google Scholar] [CrossRef]
- Chen, Y.; Zhou, Y. The Rank-Size Rule and Fractal Hierarchies of Cities: Mathematical Models and Empirical Analyses. Environ. Plan. B Plan. Des. 2003, 30, 799–818. [Google Scholar] [CrossRef]
- Pavé, A. Biological and Ecological Systems Hierarchical Organisation. In Hierarchy in Natural and Social Sciences Methodos Series; Pumain, D., Ed.; Springer: Dordrecht, The Netherlands, 2006. [Google Scholar] [CrossRef]
- Beck, F.; Burch, M.; Munz, T.; Di Silvestro, L.; Weiskopf, D. Generalized Pythagoras Trees: A Fractal Approach to Hierarchy Visualization. In Computer Vision, Imaging and Computer Graphics—Theory and Applications; VISIGRAPP Communications in Computer and Information Science; Battiato, S., Coquillart, S., Pettré, J., Laramee, R., Kerren, A., Braz, J., Eds.; Springer: Cham, Switzerland, 2015. [Google Scholar] [CrossRef]
- Komvopoulos, K. A Multiscale Theoretical Analysis of the Mechanical, Thermal, and Electrical Characteristics of Rough Contact Interfaces Demonstrating Fractal Behavior. Front. Mech. Eng. 2020, 6, 36. [Google Scholar] [CrossRef]
- Dos Anjos, P.H.R.; Gomes-Filho, M.S.; Alves, W.S.; Azevedo, D.L.; Oliveira, F.A. The Fractal Geometry of Growth: Fluctuation–Dissipation Theorem and Hidden Symmetry. Front. Phys. 2021, 9, 741590. [Google Scholar] [CrossRef]
- Dublanchet, P. Shear Stress and b-value Fluctuations in a Hierarchical Rate-and-State Asperity Model. Pure Appl. Geophys. 2022, 179, 2423–2435. [Google Scholar] [CrossRef]
- Kovchegov, Y.; Zaliapin, I.; Foufoula-Georgiou, E. Random Self-Similar Trees: Emergence of Scaling Laws. Surv. Geophys. 2022, 43, 353–421. [Google Scholar] [CrossRef]
- Mohanty, V.; Greenbury, S.F.; Sarkany, T.; Narayanan, S.; Dingle, K.; Ahnert, S.E.; Louis, A.A. Maximum mutational robustness in genotype–phenotype maps follows a self-similar blancmange-like curve. J. R. Soc. Interface 2023, 20, 20230169. [Google Scholar] [CrossRef]
- Brown, J.H.; Gupta, V.K.; Li, B.-L.; Milne, B.T.; Restrepo, C.; West, G.B. The fractal nature of nature: Power laws, ecological complexity and biodiversity. Philos. Trans. R. Soc. B Biol. Sci. 2002, 357, 619–626. [Google Scholar] [CrossRef] [PubMed]
- West, G.B. Size, Scale and the Boat Race; Conceptions, Connections and Misconceptions. In Hierarchy in Natural and Social Sciences; Methodos Series; Pumain, D., Ed.; Springer: Dordrecht, The Netherlands, 2006. [Google Scholar] [CrossRef]
- Sales-Pardo, M.; Guimerà, R.; Moreira, A.A.; Amaral, L.A.N. Extracting the hierarchical organization of complex systems. Proc. Natl. Acad. Sci. USA 2007, 104, 15224–15229. [Google Scholar] [CrossRef] [PubMed]
- Corominas-Murtra, B.; Goñi, J.; Solé, R.V.; Rodríguez-Caso, C. On the origins of hierarchy in complex networks. Proc. Natl. Acad. Sci. USA 2013, 110, 13316–13321. [Google Scholar] [CrossRef] [PubMed]
- Johnson, C.W. What are emergent properties and how do they affect the engineering of complex systems? Reliab. Eng. Syst. Saf. 2006, 91, 1475–1481. [Google Scholar] [CrossRef]
- Luo, J.; Magee, C.L. Detecting evolving patterns of self-organizing networks by flow hierarchy measurement. Complexity 2011, 16, 53–61. [Google Scholar] [CrossRef]
- San Martin, D. Clarity: Un Modelo Unificador de las Ciencias…y un Relato del Todo; Planeta Editor: Barcelona, Spain, 2021. (In Spanish) [Google Scholar]
- Marković, D.; Gros, C. Power laws and self-organized criticality in theory and nature. Phys. Rep. 2014, 536, 41–74. [Google Scholar] [CrossRef]
- Bejan, A.; Lorente, S. Constructal law of design and evolution: Physics, biology, technology, and society. J. Appl. Phys. 2013, 113, 151301. [Google Scholar] [CrossRef]
- Lopes, A.M.; Machado, J.A.T. Power Law Behaviour in Complex Systems. Entropy 2018, 20, 671. [Google Scholar] [CrossRef]
- Ortman, S.G.; Lobo, J.; Smith, M.E. Cities: Complexity, theory and history. PLoS ONE 2020, 15, e0243621. [Google Scholar] [CrossRef]
- Bashkirov, A.; Vityazev, A. Information entropy and power-law distributions for chaotic systems. Phys. A Stat. Mech. Its Appl. 1998, 277, 136–145. [Google Scholar] [CrossRef]
- Chen, Y. The rank-size scaling law and entropy-maximizing principle. Phys. A Stat. Mech. Its Appl. 2012, 391, 767–778. [Google Scholar] [CrossRef]
- Curado, E.M.F.; Nobre, F.D.; Plastino, A. Associating an Entropy with Power-Law Frequency of Events. Entropy 2018, 20, 940. [Google Scholar] [CrossRef] [PubMed]
- Jin, C.; Song, C.; Bjelland, J.; Canright, G.; Wang, D. Emergence of scaling in complex substitutive systems. Nat. Hum. Behav. 2019, 3, 837–846. [Google Scholar] [CrossRef]
- Nosonovsky, M.; Roy, P. Scaling in Colloidal and Biological Networks. Entropy 2020, 22, 622. [Google Scholar] [CrossRef] [PubMed]
- Jahanmiri, F.; Parker, D.C. An Overview of Fractal Geometry Applied to Urban Planning. Land 2022, 11, 475. [Google Scholar] [CrossRef]
- Wawrzaszek, A.; Modzelewska, R.; Krasińska, A.; Gil, A.; Glavan, V. Fractal Dimension Analysis of Earth Magnetic Field during 26 August 2018 Geomagnetic Storm. Entropy 2022, 24, 699. [Google Scholar] [CrossRef]
- Newman, M.E.J. Power laws, Pareto distributions and Zipf’s law. Contemp. Phys. 2005, 46, 323–351. [Google Scholar] [CrossRef]
- Abe, S.; Suzuki, N. Scale-free network of earthquakes. EPL 2004, 65, 581–586. [Google Scholar] [CrossRef]
- Song, C.; Havlin, S.; Makse, H.A. Self-similarity of complex networks. Nature 2005, 433, 392–395. [Google Scholar] [CrossRef]
- Wang, B.; Zhu, J.; Wei, D. The self-similarity of complex networks: From the view of degree–degree distance. Mod. Phys. Lett. B 2021, 35, 2150331. [Google Scholar] [CrossRef]
- Pastor-Satorras, R.; Wagensberg, J. The maximum entropy principle and the nature of fractals. Phys. A Stat. Mech. Its Appl. 1998, 251, 291–302. [Google Scholar] [CrossRef]
- Tate, N.J. Maximum entropy spectral analysis for the estimation of fractals in topography. Earth Surf. Process. Landf. 1999, 23, 1197–1217. [Google Scholar] [CrossRef]
- Dover, Y. A short account of a connection of power laws to the information entropy. Phys. A Stat. Mech. Its Appl. 2004, 334, 591–599. [Google Scholar] [CrossRef]
- Grmela, M. Multiscale Thermodynamics. Entropy 2021, 23, 165. [Google Scholar] [CrossRef] [PubMed]
- Venegas-Aravena, P.; Cordaro, E.G.; Laroze, D. Natural Fractals as Irreversible Disorder: Entropy Approach from Cracks in the Semi Brittle-Ductile Lithosphere and Generalization. Entropy 2022, 24, 1337. [Google Scholar] [CrossRef]
- Gokcen, N.A.; Reddy, R.G. Entropy and Related Functions. In Thermodynamics; Springer: Boston, MA, USA, 1996. [Google Scholar] [CrossRef]
- Lebowitz, J.L. Emergent phenomena. Phys. J. 2007, 6, 41. [Google Scholar]
- Atmanspacher, H. On Macrostates in Complex Multi-Scale Systems. Entropy 2016, 18, 426. [Google Scholar] [CrossRef]
- Adams, A.M. A graph-theoretic approach to understanding emergent behavior in physical systems. In Proceedings of the ALIFE 2021: The 2021 Conference on Artificial Life, Online, 18–22 July 2021; ASME: New York, NY, USA, 2021; p. 63. [Google Scholar] [CrossRef]
- Redondo, J.M.; Garcia, J.S.; Bustamante-Zamudio, C.; Pereira, M.F.; Trujillo, H.F. Heterogeneity: Method and applications for complex systems analysis. J. Physics Conf. Ser. 2022, 2159, 012013. [Google Scholar] [CrossRef]
- Venegas-Aravena, P.; Cordaro, E.; Laroze, D. Fractal Clustering as Spatial Variability of Magnetic Anomalies Measurements for Impending Earthquakes and the Thermodynamic Fractal Dimension. Fractal Fract. 2022, 6, 624. [Google Scholar] [CrossRef]
- Mandelbrot, B.B. The Fractal Geometry of Nature; W. H. Freeman and Company: New York, NY, USA, 1982. [Google Scholar]
- Newton, I. Philosophiae Naturalis Principia Mathematica; Smithsonian Institution: Washington, DC, USA, 1687. [Google Scholar]
- Onsager, L. Reciprocal Relations in Irreversible Processes. I. Phys. Rev. 1931, 37, 405–426. [Google Scholar] [CrossRef]
- Onsager, L. Reciprocal Relations in Irreversible Processes. II. Phys. Rev. B 1931, 38, 2265–2279. [Google Scholar] [CrossRef]
- Boltzmann, L. “Über die Mechanische Bedeutung des Zweiten Hauptsatzes der Wärmetheorie” (german). Wien. Berichte 1866, 53, 195–220. [Google Scholar]
- Callen, H.B. Thermodynamics and an Introduction to Thermostatistics; Wiley: New York, NY, USA, 1960. [Google Scholar]
- De Groot, S.R.; Mazur, P. Nonequilibrium Thermodynamics; North-Holland Pub. Co.: Amsterdam, The Netherlands, 1962. [Google Scholar]
- Frisch, D.H. The Microscopic Interpretation of Entropy. Am. J. Phys. 1966, 34, 1171–1173. [Google Scholar] [CrossRef]
- Riek, R. A Derivation of a Microscopic Entropy and Time Irreversibility from the Discreteness of Time. Entropy 2014, 16, 3149–3172. [Google Scholar] [CrossRef]
- Sahimi, M.; Arbabi, S. Scaling Laws for Fracture of Heterogeneous Materials and Rock. Phys. Rev. Lett. 1996, 77, 3689–3692. [Google Scholar] [CrossRef]
- Vallianatos, F.; Tzanis, A. On the nature, scaling and spectral properties of pre-seismic ULF signals. Nat. Hazards Earth Syst. Sci. 2003, 3, 237–242. [Google Scholar] [CrossRef]
- Anastasiadis, C.; Triantis, D.; Stavrakas, I.; Vallianatos, F. Pressure Stimulated Currents (PSC) in marble samples. Ann. Geophys. 2009, 47, 21–28. [Google Scholar] [CrossRef]
- Kuksenko, V.; Tomilin, N.; Chmel, A. The role of driving rate in scaling characteristics of rock fracture. J. Stat. Mech. Theory Exp. 2005, 2005, P06012. [Google Scholar] [CrossRef]
- Malakhovsky, I.; Michels, M.A.J. Scaling and localization in fracture of disordered central-force spring lattices: Comparison with random damage percolation. Phys. Rev. B 2006, 74, 014206. [Google Scholar] [CrossRef]
- Triantis, D.; Vallianatos, F.; Stavrakas, I.; Hloupis, G. Relaxation phenomena of electrical signal emissions from rock following application of abrupt mechanical stress. Ann. Geophys. 2012, 55, 207–212. [Google Scholar] [CrossRef]
- Venegas-Aravena, P.; Cordaro, E.G.; Laroze, D. A review and upgrade of the lithospheric dynamics in context of the seismo-electromagnetic theory. Nat. Hazards Earth Syst. Sci. 2019, 19, 1639–1651. [Google Scholar] [CrossRef]
- Potirakis, S.M.; Contoyiannis, Y.; Eftaxias, K.; Melis, N.S.; Nomicos, C. Post-spontaneous-symmetry-breaking power-laws after a very strong earthquake: Indication for the preparation of a new strong earthquake or not? Phys. A Stat. Mech. Its Appl. 2021, 589, 126607. [Google Scholar] [CrossRef]
- Loukidis, A.; Tzagkarakis, D.; Kyriazopoulos, A.; Stavrakas, I.; Triantis, D. Correlation of Acoustic Emissions with Electrical Signals in the Vicinity of Fracture in Cement Mortars Subjected to Uniaxial Compressive Loading. Appl. Sci. 2022, 13, 365. [Google Scholar] [CrossRef]
- Yang, C.; Wu, Z.; Wang, W.; Qu, H.; Ren, N.; Li, H. Study on the influence of natural cracks on the mechanical properties and fracture mode for shale at the microscale: An example from the Lower Cambrian Niutitang Formation in northern Guizhou. Front. Earth Sci. 2023, 10, 1032817. [Google Scholar] [CrossRef]
- Mandelbrot, B.B. Negative fractal dimensions and multifractals. Phys. A Stat. Mech. Its Appl. 1990, 163, 306–315. [Google Scholar] [CrossRef]
- Dvoretskaya, O.A.; Kondratenko, P.S. Anomalous transport in fractal media with randomly inhomogeneous diffusion barrier. Cond-Mat. Soft 2013, 103, 325–339. [Google Scholar] [CrossRef]
- Chen, Y.; Yang, H. Numerical simulation and pattern characterization of nonlinear spatiotemporal dynamics on fractal surfaces for the whole-heart modeling applications. Eur. Phys. J. B 2016, 89, 181. [Google Scholar] [CrossRef]
- Yang, H. The Generator of Fractal Surfaces or Images, MATLAB Central File Exchange. Available online: https://www.mathworks.com/matlabcentral/fileexchange/78179-the-generator-of-fractal-surfaces-or-images (accessed on 30 August 2023).
- Greiner, W.; Neise, L.; Stöcker, H. Number of Microstates Ω and Entropy S. In Thermodynamics and Statistical Mechanics. Classical Theoretical Physics; Springer: New York, NY, USA, 1995. [Google Scholar] [CrossRef]
- Nicolis, G.; Prigogine, I. Self-Organization in Nonequilibrium Systems: From Dissipative Structures to Order through Fluctuations, 1st ed.; Wiley: Hoboken, NJ, USA, 1977. [Google Scholar]
- Heylighen, F. The science of self-organization and adaptivity. Encycl. Life Support Syst. 2001, 5, 253–280. [Google Scholar]
- De Wolf, T.; Holvoet, T. Emergence Versus Self-Organisation: Different Concepts but Promising When Combined. In Engineering Self-Organising Systems. ESOA Lecture Notes in Computer Science; Brueckner, S.A., Di Marzo Serugendo, G., Karageorgos, A., Nagpal, R., Eds.; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar] [CrossRef]
- Addiscott, T. Entropy, non-linearity and hierarchy in ecosystems. Geoderma 2010, 160, 57–63. [Google Scholar] [CrossRef]
- Cavagna, A.; Cimarelli, A.; Giardina, I.; Parisi, G.; Santagati, R.; Stefanini, F.; Viale, M. Scale-free correlations in starling flocks. Proc. Natl. Acad. Sci. USA 2010, 107, 11865–11870. [Google Scholar] [CrossRef]
- Gershenson, C.; Polani, D.; Martius, G. Editorial: Complexity and Self-Organization. Front. Robot. AI 2021, 8, 2021. [Google Scholar] [CrossRef]
- Navarrete, Y.; Davis, S. Quantum Mutual Information, Fragile Systems and Emergence. Entropy 2022, 24, 1676. [Google Scholar] [CrossRef] [PubMed]
- Cottam, R.; Vounckx, R. Entropy is better related to unification than to order. Biosystems 2023, 223, 104815. [Google Scholar] [CrossRef] [PubMed]
- Newman, D.V. Emergence and Strange Attractors. Philos. Sci. 1996, 63, 245–261. [Google Scholar] [CrossRef]
- Bedau, M.A. Weak emergence. In Philosophical Perspectives: Mind, Causation, and World; Tomberlin, J., Ed.; Blackwell: Malden, MA, USA, 1997; Volume 31, pp. 375–399. [Google Scholar] [CrossRef]
- Nolte, D.D. The tangled tale of phase space. Phys. Today 2010, 63, 33–38. [Google Scholar] [CrossRef]
- Oprisan, S.A.; Lynn, P.E.; Tompa, T.; Lavin, A. Low-dimensional attractor for neural activity from local field potentials in optogenetic mice. Front. Comput. Neurosci. 2015, 9, 125. [Google Scholar] [CrossRef] [PubMed]
- Nabika, H.; Itatani, M.; Lagzi, I. Pattern Formation in Precipitation Reactions: The Liesegang Phenomenon. Langmuir 2019, 36, 481–497. [Google Scholar] [CrossRef]
- Medina, M.; Huffaker, R.; Muñoz-Carpena, R.; Kiker, G. An empirical nonlinear dynamics approach to analyzing emergent behavior of agent-based models. AIP Adv. 2021, 11, 035133. [Google Scholar] [CrossRef]
- Jirsa, V.; Sheheitli, H. Entropy, free energy, symmetry and dynamics in the brain. J. Phys. Complex. 2022, 3, 015007. [Google Scholar] [CrossRef]
- Callen, H.B.; Welton, T.A. Irreversibility and Generalized Noise. Phys. Rev. B 1951, 83, 34–40. [Google Scholar] [CrossRef]
- Kubo, R. The fluctuation-dissipation theorem. Rep. Prog. Phys. 1966, 29, 255–284. [Google Scholar] [CrossRef]
- Prost, J.; Joanny, J.-F.; Parrondo, J.M.R. Generalized Fluctuation-Dissipation Theorem for Steady-State Systems. Phys. Rev. Lett. 2009, 103, 090601. [Google Scholar] [CrossRef] [PubMed]
- Turing, A.M. The chemical basis of morphogenesis. Philos. Trans. Roy Soc. Lond Ser. B 1952, 237, 37–72. [Google Scholar] [CrossRef]
- Miller, D.G. Thermodynamics of Irreversible Processes. The Experimental Verification of the Onsager Reciprocal Relations. Chem. Rev. 1960, 60, 15–37. [Google Scholar] [CrossRef]
- De Corato, M.; Pagonabarraga, I. Onsager reciprocal relations and chemo-mechanical coupling for chemically active colloids. J. Chem. Phys. 2022, 157, 084901. [Google Scholar] [CrossRef] [PubMed]
- O’Shaughnessy, B.; Procaccia, I. Analytical Solutions for Diffusion on Fractal Objects. Phys. Rev. Lett. 1985, 54, 455–458. [Google Scholar] [CrossRef] [PubMed]
- Qian, L.H.; Chen, M.W. Ultrafine nanoporous gold by low-temperature dealloying and kinetics of nanopore formation. Appl. Phys. Lett. 2007, 91, 083105. [Google Scholar] [CrossRef]
- Erlebacher, J. Mechanism of Coarsening and Bubble Formation in High-Genus Nanoporous Metals. Phys. Rev. Lett. 2011, 106, 225504. [Google Scholar] [CrossRef]
- Wada, T.; Kato, H. Three-dimensional open-cell macroporous iron, chromium and ferritic stainless steel. Scr. Mater. 2013, 68, 723–726. [Google Scholar] [CrossRef]
- Geslin, P.-A.; McCue, I.; Gaskey, B.; Erlebacher, J.; Karma, A. Topology-generating interfacial pattern formation during liquid metal dealloying. Nat. Commun. 2015, 6, 8887. [Google Scholar] [CrossRef]
- Olsen, K.S.; Campbell, J.M. Diffusion Entropy and the Path Dimension of Frictional Finger Patterns. Front. Phys. 2020, 8, 83. [Google Scholar] [CrossRef]
- Heller, P. Experimental investigations of critical phenomena. Rep. Prog. Phys. 1967, 30, 731. [Google Scholar] [CrossRef]
- Hohenberg, P.C.; Halperin, B.I. Theory of dynamic critical phenomena. Rev. Mod. Phys. 1977, 49, 435–479. [Google Scholar] [CrossRef]
- Dorogovtsev, S.N.; Goltsev, A.V.; Mendes, J.F.F. Critical phenomena in complex networks. Rev. Mod. Phys. 2008, 80, 1275–1335. [Google Scholar] [CrossRef]
- Dorogovtsev, S.N.; Mendes, J.F.F. The Nature of Complex Networks; Oxford University Press (OUP): Oxford, UK, 2022; ISBN 0199695113. [Google Scholar]
- Potirakis, S.M.; Papadopoulos, P.; Matiadou, N.-L.; Hanias, M.P.; Stavrinides, S.G.; Balasis, G.; Contoyiannis, Y. Spontaneous Symmetry Breaking in Systems Obeying the Dynamics of On–Off Intermittency and Presenting Bimodal Amplitude Distributions. Symmetry 2023, 15, 1448. [Google Scholar] [CrossRef]
- Nishimori, H.; Ortiz, G. Elements of Phase Transitions and Critical Phenomena; Oxford University Press (OUP): Oxford, UK, 2010; ISBN 9780199577224. [Google Scholar]
- Halperin, B.I. Theory of dynamic critical phenomena. Phys. Today 2019, 72, 42–43. [Google Scholar] [CrossRef]
- Fisher, M.E. Critical Phenomena: Proceedings, Stellenbosch, South Africa, 1982; Hahne, F.J.W., Ed.; Springer: Berlin/Heidelberg, Germany, 1983. [Google Scholar]
- Chen, H.; Yue, Z.; Ren, D.; Zeng, H.; Wei, T.; Zhao, K.; Yang, R.; Qiu, P.; Chen, L.; Shi, X. Thermal Conductivity during Phase Transitions. Adv. Mater. 2018, 31, e1806518. [Google Scholar] [CrossRef]
- Pietruszka, M.; Olszewska, M. Extracellular ionic fluxes suggest the basis for cellular life at the 1/f ridge of extended criticality. Eur. Biophys. J. 2020, 49, 239–252. [Google Scholar] [CrossRef]
- Pietruszka, M.A. Non-equilibrium phase transition at a critical point of human blood. Sci. Rep. 2021, 11, 1–8. [Google Scholar] [CrossRef]
- Schroeder, M. Fractals, Chaos, Power Laws: Minutes from an Infinite Paradise; W.H. Freeman and Company: New York, NY, USA, 1991. [Google Scholar]
- Moore, B.J. Chaos Theory: Unpredictable Order in Chaos. In Shaking the Invisible Hand; Palgrave Macmillan: London, UK, 2006. [Google Scholar] [CrossRef]
- Eppel-Meichlinger, J.; Kobleder, A.; Mayer, H. Developing a theoretical definition of self-organization: A principle-based concept analysis in the context of uncertainty in chronic illness. Nurs. Forum 2022, 57, 954–962. [Google Scholar] [CrossRef]
- Ottino, J.M.; Muzzio, F.J.; Tjahjadi, M.; Franjione, J.G.; Jana, S.C.; Kusch, H.A. Chaos, Symmetry, and Self-Similarity: Exploiting Order and Disorder in Mixing Processes. Science 1992, 257, 754–760. [Google Scholar] [CrossRef]
- Lorenz, E. The Essence of Chaos; University of Washington Press: Seattle, DA, USA, 1993; pp. 181–206. [Google Scholar]
- Ruelle, D. Chaotic Evolution and Strange Attractors; Cambridge University: New York, NY, USA, 1983. [Google Scholar]
- Lichtenberg, A.J.; Lieberman, M.A. Regular and Stochastic Motion; Springer: New York, NY, USA, 1983; ISBN 9780387097237. [Google Scholar]
- Tabor, M. Chaos and Integrability in Nonlinear Dynamics; Wiley: New York, NY, USA, 1989. [Google Scholar]
- Hoover, W.G.; Posch, H.A. Second-law irreversibility and phase-space dimensionality loss from time-reversible nonequilibrium steady-state Lyapunov spectra. Phys. Rev. E 1994, 49, 1913–1920. [Google Scholar] [CrossRef]
- Gaspard, P. Time Asymmetry in Nonequilibrium Statistical Mechanics. Spec. Vol. Mem. Ilya Prigogine Adv. Chem. Phys. 2007, 135, 83–133. [Google Scholar] [CrossRef]
- Betancourt-Mar, J.A.; Rodríguez-Ricard, M.; Mansilla, R.; Cocho, G.; Nieto-Villar, J.M. Entropy production: Evolution criteria, robustness and fractal dimension. Rev. Mex. Fis. 2016, 62, 164–167. [Google Scholar]
- Benzi, R.; Paladin, P.; Parisi, G.; Vulpiani, A. On the multifractal nature of fully developed turbulence and chaotic systems. J. Phys. A Math. Gen. 1984, 17, 3521. [Google Scholar] [CrossRef]
- Menon, E.S. Chapter Five—Fluid Flow in Pipes. In Transmission Pipeline Calculations and Simulations Manual 2015; Gulf Professional Publishing: Woburn, MA, USA, 2015; pp. 149–234. [Google Scholar] [CrossRef]
- Nicolleau, F. Numerical determination of turbulent fractal dimensions. Phys. Fluids 1996, 8, 2661–2670. [Google Scholar] [CrossRef]
- Makris, C.V.; Memos, C.D.; Krestenitis, Y.N. Numerical modeling of surf zone dynamics under weakly plunging breakers with SPH method. Ocean Model. 2016, 98, 12–35. [Google Scholar] [CrossRef]
- Landahl, M.T.; Mollo-Christensen, E. Turbulence and Random Processes in Fluid Mechanics, 2nd ed.; Cambridge University Press, (CUP): Cambridge, UK, 1992; ISBN 978-0521422130. [Google Scholar]
- Kraichnan, R.H. Inertial Ranges in Two-Dimensional Turbulence. Phys. Fluids 1967, 10, 1417–1423. [Google Scholar] [CrossRef]
- Lorenz, E.N. The predictability of a flow which possesses many scales of motion. Tellus 1969, 21, 289–307. [Google Scholar] [CrossRef]
- Siggia, E.D.; Aref, H. Point-vortex simulation of the inverse energy cascade in two-dimensional turbulence. Phys. Fluids 1981, 24, 171–173. [Google Scholar] [CrossRef]
- Frisch, U.; Sulem, P.-L. Numerical simulation of the inverse cascade in two-dimensional turbulence. Phys. Fluids 1984, 27, 1921–1923. [Google Scholar] [CrossRef]
- Chaisson, E.J. Cosmic Evolution: The Rise of Complexity in Nature; Harvard University Press: Cambridge, MA, USA, 2001; ISBN 9780674003422. [Google Scholar]
- Coleman, P.H.; Pietronero, L. The fractal structure of the universe. Phys. Rep. 1992, 213, 311–389. [Google Scholar] [CrossRef]
- Evans, N.W. The power-law galaxies. Mon. Not. R. Astron. Soc. 1994, 267, 333–360. [Google Scholar] [CrossRef][Green Version]
- Joyce, M.; Anderson, P.W.; Montuori, M.; Pietronero, L.; Labini, F.S. Fractal cosmology in an open universe. Lett. J. Explor. Front. Phys. 2000, 50, 416–422. [Google Scholar] [CrossRef]
- Boutloukos, S.G.; Lamers, H.J.G.L.M. Star cluster formation and disruption time-scales—I. An empirical determination of the disruption time of star clusters in four galaxies. Mon. Not. R. Astron. Soc. 2003, 338, 717–732. [Google Scholar] [CrossRef][Green Version]
- Iovane, G.; Laserra, E.; Tortoriello, F. Stochastic self-similar and fractal universe. Chaos Solitons Fractals 2004, 20, 415–426. [Google Scholar] [CrossRef]
- Labini, F.S.; Vasilyev, N.L.; Baryshev, Y.V. Power law correlations in galaxy distribution and finite volume effects from the Sloan Digital Sky Survey Data Release Four. Astron. Astrophys. 2007, 465, 23–33. [Google Scholar] [CrossRef]
- Watson, D.F.; Berlind, A.A.; Zentner, A.R. A cosmic coincidence: The power-law galaxy correlation function. Astrophys. J. 2011, 738, 22. [Google Scholar] [CrossRef]
- Hogan, M.T.; McNamara, B.R.; Pulido, F.; Nulsen, P.E.J.; Russell, H.R.; Vantyghem, A.N.; Edge, A.C.; Main, R.A. Mass Distribution in Galaxy Cluster Cores. Astrophys. J. 2017, 837, 51. [Google Scholar] [CrossRef]
- Gaite, J. The Fractal Geometry of the Cosmic Web and Its Formation. Adv. Astron. 2019, 2019, 6587138. [Google Scholar] [CrossRef]
- Tarakanov, P.A.; Yezhkov, M.Y.; Kostina, M.V. Fractal Dimension of the Cosmic Microwave Background as a Test of “Planck” Spacecraft Data. Astrophysics 2020, 63, 288–295. [Google Scholar] [CrossRef]
- Gaite, J. Scaling Laws in the Stellar Mass Distribution and the Transition to Homogeneity. Adv. Astron. 2021, 2021, 6680938. [Google Scholar] [CrossRef]
- Kirillov, A.A.; Savelova, E.P.; Vladykina, P.O. Exact fractal model of the universe and possible machine learning methods for the verification of the fractality. In Proceedings of the Sixteenth Marcel Grossmann Meeting 2023, Virtual, 5–10 July 2023; pp. 352–367. [Google Scholar] [CrossRef]
- McShea, D.W. Unnecessary Complexity. Science 2013, 342, 1319–1320. [Google Scholar] [CrossRef]
- Chaisson, E.J. Energy Flows in Low-Entropy Complex Systems. Entropy 2015, 17, 8007–8018. [Google Scholar] [CrossRef]
- Bejan, A. Discipline in Thermodynamics. Energies 2020, 13, 2487. [Google Scholar] [CrossRef]
- Barsky, E. Entropy of Complex Processes and Systems; Elsevier: Amsterdam, The Netherlands, 2020; ISBN 9780128216620. [Google Scholar]
- Kobayashi, N.; Yamazaki, Y.; Kuninaka, H.; Katori, M.; Matsushita, M.; Matsushita, S.; Chiang, L.-Y. Fractal Structure of Isothermal Lines and Loops on the Cosmic Microwave Background. J. Phys. Soc. Jpn. 2011, 80, 074003. [Google Scholar] [CrossRef]
- Mylläri, A.A.; Raikov, A.A.; Orlov, V.V.; Tarakanov, P.A.; Yershov, V.N.; Yezhkov, M.Y. Fractality of Isotherms of the Cosmic Microwave Background Based on Data from the Planck Spacecraft. Astrophysics 2016, 59, 31–37. [Google Scholar] [CrossRef]
- Teles, S.; Lopes, A.R.; Ribeiro, M.B. Galaxy distributions as fractal systems. Eur. Phys. J. C 2022, 82, 896. [Google Scholar] [CrossRef]
- Rovelli, C. Where Was Past Low-Entropy? Entropy 2019, 21, 466. [Google Scholar] [CrossRef]
- Maturana, H.R. Autopoiesis: Reproduction, heredity and evolution. In Autopoiesis, Dissipative Structures and Spontaneous Social Orders, AAAS Selected Symposium 55 (AAAS National Annual Meeting, Houston, TX, USA, 3–8 January 1979); Zeleny, M., Ed.; Westview Press: Boulder, CO, USA, 1980; pp. 45–79. Available online: https://cepa.info/552 (accessed on 27 December 2023).
- Kryazhimskii, F.V. Homeostasis and self-similarity of biological system dynamics. Dokl. Biol. Sci. 2007, 413, 156–158. [Google Scholar] [CrossRef]
- Rivera, A.L.; Toledo-Roy, J.C.; Frank, A. Symmetry and Signs of Self-Organized Criticality in Living Organisms. J. Physics Conf. Ser. 2020, 1612, 012024. [Google Scholar] [CrossRef]
- Rubin, S.; Veloz, T.; Maldonado, P. Beyond planetary-scale feedback self-regulation: Gaia as an autopoietic system. Biosystems 2021, 199, 104314. [Google Scholar] [CrossRef] [PubMed]
- Penrose, R. Cycles of Time: An Extraordinary New View of the Universe; The Bodley Head: London, UK, 2010. [Google Scholar]
- Rovelli, C.; Vidotto, F. Pre-Big-Bang Black-Hole Remnants and Past Low Entropy. Universe 2018, 4, 129. [Google Scholar] [CrossRef]
- An, D.; A Meissner, K.; Nurowski, P.; Penrose, R. Apparent evidence for Hawking points in the CMB Sky. Mon. Not. R. Astron. Soc. 2020, 495, 3403–3408. [Google Scholar] [CrossRef]
- Eckstein, M. Conformal Cyclic Cosmology, gravitational entropy and quantum information. Gen. Relativ. Gravit. 2023, 55, 26. [Google Scholar] [CrossRef]
- Richardson, L. The problem of contiguity: An appendix of statistics of deadly quarrels. Gen. Syst. 1961, 6, 139–187. [Google Scholar]
- Mandelbrot, B. How Long Is the Coast of Britain? Statistical Self-Similarity and Fractional Dimension. Science 1967, 156, 636–638. [Google Scholar] [CrossRef]
- Barnsley, M.F. Fractals Everywhere; Elsevier Inc.: Amsterdam, The Netherlands; Academic Press: Cambridge, MA, USA, 1993. [Google Scholar]
- Turcotte, D.L. Fractals and Chaos in Geology and Geophysics, 2nd ed.; Cambridge University Press: Cambridge, UK, 1997; pp. 231–244. [Google Scholar]
- Husain, A.; Reddy, J.; Bisht, D.; Sajid, M. Fractal dimension of coastline of Australia. Sci. Rep. 2021, 11, 6304. [Google Scholar] [CrossRef]
- Houze, R.A., Jr. Chapter 12—Clouds and Precipitation Associated with Hills and Mountains. Int. Geophys. 2014, 104, 369. [Google Scholar] [CrossRef]
- Chase, C.G. Fluvial landsculpting and the fractal dimension of topography. Geomorphology 1992, 5, 39–57. [Google Scholar] [CrossRef]
- Ferraro, P.; Godin, C.; Prusinkiewicz, P. Toward a quantification of self-similarity in plants. Fractals 2005, 13, 91–109. [Google Scholar] [CrossRef]
- Hellström, L.; Carlsson, L.; Falster, D.S.; Westoby, M.; Brännström, Å. Branch Thinning and the Large-Scale, Self-Similar Structure of Trees. Am. Nat. 2018, 192, E37–E47. [Google Scholar] [CrossRef] [PubMed]
- Bejan, A.; Zane, J.P. Design in Nature: How the Constructal Law Governs Evolution in Biology, Physics, Technology, and Social Organizations; Anchor Publisher: New York, NY, USA, 2013. [Google Scholar]
- Granier, A.; Biron, P.; Bréda, N.; Pontailler, J.; Saugier, B. Transpiration of trees and forest stands: Short and long-term monitoring using sapflow methods. Glob. Chang. Biol. 1996, 2, 265–274. [Google Scholar] [CrossRef]
- Tyree, M.T. Hydraulic limits on tree performance: Transpiration, carbon gain and growth of trees. Trees 2003, 17, 95–100. [Google Scholar] [CrossRef]
- Bejan, A.; Lorente, S.; Lee, J. Unifying constructal theory of tree roots, canopies and forests. J. Theor. Biol. 2008, 254, 529–540. [Google Scholar] [CrossRef]
- Shingleton, A. Allometry: The Study of Biological Scaling. Nat. Educ. Knowl. 2010, 3, 2. [Google Scholar]
- Rowland, M.; Dedrick, R.L. Chapter 32—Preclinical Prediction of Human Pharmacokinetics. In Principles of Clinical Pharmacology, 3rd ed.; Academic Press: Cambridge, MA, USA, 2012; pp. 531–540. [Google Scholar] [CrossRef]
- Pélabon, C.; Firmat, C.; Bolstad, G.H.; Voje, K.L.; Houle, D.; Cassara, J.; Le Rouzic, A.; Hansen, T.F. Evolution of morphological allometry. Ann. N. Y. Acad. Sci. 2014, 1320, 58–75. [Google Scholar] [CrossRef] [PubMed]
- Hallgrimsson, B.; Percival, C.J.; Green, R.; Young, N.M.; Mio, W.; Marcucio, R. Chapter Twenty—Morphometrics, 3D Imaging, and Craniofacial Development. Curr. Top. Dev. Biol. 2015, 115, 561–597. [Google Scholar] [CrossRef]
- Escala, A. The principle of similitude in biology. Theor. Ecol. 2019, 12, 415–425. [Google Scholar] [CrossRef]
- Cloyed, C.S.; Grady, J.M.; Savage, V.M.; Uyeda, J.C.; Dell, A.I. The allometry of locomotion. Ecology 2021, 102, e03369. [Google Scholar] [CrossRef]
- Escala, A. Universal ontogenetic growth without fitted parameters: Implications for life history invariants and population growth. Theor. Ecol. 2023, 16, 315–325. [Google Scholar] [CrossRef]
- Kleiber, M. Body size and metabolic rate. Physiol. Rev. 1947, 27, 511–541. [Google Scholar] [CrossRef] [PubMed]
- Fenchel, T. Intrinsic rate of natural increase: The relationship with body size. Oecologia 1974, 14, 317–326. [Google Scholar] [CrossRef] [PubMed]
- West, G.B.; Savage, V.M.; Gillooly, J.; Enquist, B.J.; Woodruff, W.H.; Brown, J.H. Why does metabolic rate scale with body size? Nature 2003, 421, 713. [Google Scholar] [CrossRef]
- Woodward, G.; Ebenman, B.; Emmerson, M.; Montoya, J.M.; Olesen, J.M.; Valido, A.; Warren, P.H.; Woodward, G.; Ebenman, B.; Emmerson, M.; et al. Body size in ecological networks. Trends Ecol. Evol. 2005, 20, 402–409. [Google Scholar] [CrossRef]
- Guidolin, D.; Crivellato, E.; Ribatti, D. The “self-similarity logic” applied to the development of the vascular system. Dev. Biol. 2011, 351, 156–162. [Google Scholar] [CrossRef]
- Chen, Y. Fractals and Fractal Dimension of Systems of Blood Vessels: An Analogy between Artery Trees, River Networks, and Urban Hierarchies. Fractal Geom. Nonlinear Anal. Med. Biol. 2015, 1, 8. [Google Scholar] [CrossRef]
- Schimpf, K. The Human Body as an Energy System. AJN Am. J. Nurs. 1971, 71, 117. [Google Scholar] [CrossRef]
- Bullmore, E.; Brammer, M.; Harvey, I.; Persaud, R.; Murray, R.; Ron, M. Fractal analysis of the boundary between white matter and cerebral cortex in magnetic resonance images: A controlled study of schizophrenic and manic-depressive patients. Psychol. Med. 1994, 24, 771–781. [Google Scholar] [CrossRef]
- Kenkel, N.C.; Walker, D.J. Fractals in the biological sciences. Coenoses 1996, 11, 77–100. [Google Scholar]
- Masters, B.R. Fractal Analysis of the Vascular Tree in the Human Retina. Annu. Rev. Biomed. Eng. 2004, 6, 427–452. [Google Scholar] [CrossRef] [PubMed]
- Gabryś, E.; Rybaczuk, M.; Kędzia, A. Blood flow simulation through fractal models of circulatory system. Chaos Solitons Fractals 2006, 27, 1–7. [Google Scholar] [CrossRef]
- Tanabe, N.; Sato, S.; Suki, B.; Hirai, T. Fractal Analysis of Lung Structure in Chronic Obstructive Pulmonary Disease. Front. Physiol. 2020, 11, 1661. [Google Scholar] [CrossRef]
- Honda, H.; Imayama, S.; Tanemura, M. A Fractal-Like Structure in the Skin. Fractals 1996, 4, 139–147. [Google Scholar] [CrossRef]
- Cattani, C. Fractals and Hidden Symmetries in DNA. Math. Probl. Eng. 2010, 2010, 507056. [Google Scholar] [CrossRef]
- Namazi, H.; Akrami, A.; Hussaini, J.; Silva, O.N.; Wong, A.; Kulish, V.V. The fractal based analysis of human face and DNA variations during aging. Biosci. Trends 2016, 10, 477–481. [Google Scholar] [CrossRef]
- Peng, C.-K.; Mietus, J.E.; Liu, Y.; Lee, C.; Hausdorff, J.M.; Stanley, H.E.; Goldberger, A.L.; Lipsitz, L.A. Quantifying Fractal Dynamics of Human Respiration: Age and Gender Effects. Ann. Biomed. Eng. 2002, 30, 683–692. [Google Scholar] [CrossRef]
- Hayano, K.; Yoshida, H.; Zhu, A.X.; Sahani, D.V. Fractal Analysis of Contrast-Enhanced CT Images to Predict Survival of Patients with Hepatocellular Carcinoma Treated with Sunitinib. Dig. Dis. Sci. 2014, 59, 1996–2003. [Google Scholar] [CrossRef]
- Lennon, F.E.; Cianci, G.C.; Cipriani, N.A.; Hensing, T.A.; Zhang, H.J.; Chen, C.-T.; Murgu, S.D.; Vokes, E.E.; Vannier, M.W.; Salgia, R. Lung cancer—A fractal viewpoint. Nat. Rev. Clin. Oncol. 2015, 12, 664–675. [Google Scholar] [CrossRef]
- Fernández, E.; Bolea, J.; Ortega, G.; Louis, E. Are neurons multifractals? J. Neurosci. Methods 1999, 89, 151–157. [Google Scholar] [CrossRef]
- Milošević, N.T.; Ristanović, D.; Stanković, J. Fractal analysis of the laminar organization of spinal cord neurons. J. Neurosci. Methods 2005, 146, 198–204. [Google Scholar] [CrossRef] [PubMed]
- Im, K.; Lee, J.; Yoon, U.; Shin, Y.; Hong, S.B.; Kim, I.Y.; Kwon, J.S.; Kim, S.I. Fractal dimension in human cortical surface: Multiple regression analysis with cortical thickness, sulcal depth, and folding area. Hum. Brain Mapp. 2006, 27, 994–1003. [Google Scholar] [CrossRef] [PubMed]
- Smith, J.H.; Rowland, C.; Harland, B.; Moslehi, S.; Montgomery, R.D.; Schobert, K.; Watterson, W.J.; Dalrymple-Alford, J.; Taylor, R.P. How neurons exploit fractal geometry to optimize their network connectivity. Sci. Rep. 2021, 11, 2332. [Google Scholar] [CrossRef] [PubMed]
- Grosu, G.F.; Hopp, A.V.; Moca, V.V.; Bârzan, H.; Ciuparu, A.; Ercsey-Ravasz, M.; Winkel, M.; Linde, H.; Mureșan, R.C. The fractal brain: Scale-invariance in structure and dynamics. Cereb. Cortex 2022, 33, 4574–4605. [Google Scholar] [CrossRef]
- Fernández, A.; Zuluaga, P.; Abásolo, D.; Gómez, C.; Serra, A.; Méndez, M.A.; Hornero, R. Brain oscillatory complexity across the life span. Clin. Neurophysiol. 2012, 123, 2154–2162. [Google Scholar] [CrossRef] [PubMed]
- Wu, K.; Taki, Y.; Sato, K.; Kinomura, S.; Goto, R.; Okada, K.; Kawashima, R.; He, Y.; Evans, A.C.; Fukuda, H. Age-related changes in topological organization of structural brain networks in healthy individuals. Hum. Brain Mapp. 2011, 33, 552–568. [Google Scholar] [CrossRef] [PubMed]
- Farahibozorg, S.; Hashemi-Golpayegani, S.M.; Ashburner, J. Age- and Sex-Related Variations in the Brain White Matter Fractal Dimension Throughout Adulthood: An MRI Study. Clin. Neuroradiol. 2014, 25, 19–32. [Google Scholar] [CrossRef]
- Alain, C.; Zendel, B.R.; Hutka, S.; Bidelman, G.M. Turning down the noise: The benefit of musical training on the aging auditory brain. Hear. Res. 2014, 308, 162–173. [Google Scholar] [CrossRef]
- Tulving, E. Episodic and semantic memory. In Organization of Memory; Tulving, E., Donaldson, W., Eds.; Academic: New York, NY, USA, 1972; pp. 381–403. [Google Scholar]
- Tulving, E. Episodic Memory: From Mind to Brain. Annu. Rev. Psychol. 2002, 53, 1–25. [Google Scholar] [CrossRef]
- Takahashi, T.; Murata, T.; Omori, M.; Kosaka, H.; Takahashi, K.; Yonekura, Y.; Wada, Y. Quantitative evaluation of age-related white matter microstructural changes on MRI by multifractal analysis. J. Neurol. Sci. 2004, 225, 33–37. [Google Scholar] [CrossRef]
- Zueva, M.V. Fractality of sensations and the brain health: The theory linking neurodegenerative disorder with distortion of spatial and temporal scale-invariance and fractal complexity of the visible world. Front. Aging Neurosci. 2015, 7, 135. [Google Scholar] [CrossRef]
- Mustafa, N.; Ahearn, T.S.; Waiter, G.D.; Murray, A.D.; Whalley, L.J.; Staff, R.T. Brain structural complexity and life course cognitive change. NeuroImage 2012, 61, 694–701. [Google Scholar] [CrossRef] [PubMed]
- Li, S.-C.; von Oertzen, T.; Lindenberger, U. A neurocomputational model of stochastic resonance and aging. Neurocomputing 2006, 69, 1553–1560. [Google Scholar] [CrossRef]
- Söderlund, G.; Sikström, S.; Smart, A. Listen to the noise: Noise is beneficial for cognitive performance in ADHD. J. Child Psychol. Psychiatry 2007, 48, 840–847. [Google Scholar] [CrossRef] [PubMed]
- Stadnitski, T. Tenets and Methods of Fractal Analysis (1/f Noise). In The Fractal Geometry of the Brain; Di Ieva, A., Ed.; Springer Series in Computational Neuroscience; Springer: New York, NY, USA, 2016. [Google Scholar] [CrossRef]
- Jonas, J.B. Global prevalence of age-related macular degeneration. Lancet Glob. Heal. 2014, 2, e65–e66. [Google Scholar] [CrossRef] [PubMed]
- MacLennan, B. Evolutionary Psychology, Complex Systems, and Social Theory. Sound. Interdiscip. J. 2007, 90, 169–189. [Google Scholar] [CrossRef]
- Lazer, D.; Pentland, A.; Adamic, L.; Aral, S.; Barabási, A.-L.; Brewer, D.; Christakis, N.; Contractor, N.; Fowler, J.; Gutmann, M.; et al. Computational social science. Science 2009, 323, 721–723. [Google Scholar] [CrossRef] [PubMed]
- Hudson, C.G. Complex Systems and Human Behavior, 1st ed.; Oxford University Press: Oxford, UK, 2010. [Google Scholar]
- Miguel, M.S. Frontiers in Complex Systems. Front. Complex Syst. 2023, 1, 1080801. [Google Scholar] [CrossRef]
- Tomasello, M. A Natural History of Human Morality; Harvard University Press: Cambridge, MA, USA, 2016. [Google Scholar]
- Suki, B.; Bates, J.H.T.; Frey, U. Complexity and emergent phenomena. Compr. Physiol. 2011, 1, 995–1029. [Google Scholar] [CrossRef]
- Darwin, C. The Origin of Species, by Means of Natural Selection, or the Preservation of Favoured Races in the Struggle for Life; Cambridge University Press (CUP): Cambridge, UK, 2009; ISBN 9780511694295. [Google Scholar]
- Spencer, H. The Principles of Biology; William and Norgate: London, UK, 1864; Volume 1. [Google Scholar]
- Gingerich, P.D. Rates of evolution on the time scale of the evolutionary process. Genetica 2001, 112, 127–144. [Google Scholar] [CrossRef]
- Bryson, B. A Short History of Nearly Everything; Broadway Books: New York, NY, USA, 2003. [Google Scholar]
- Dartnell, L. Origins: How Earth’s History Shaped Human History; Penguin Random House: New York, NY, USA, 2019. [Google Scholar]
- Andrews, G.; Fan, K.; Pratt, H.E.; Phalke, N.; Karlsson, E.K.; Lindblad-Toh, K.; Gazal, S.; Moore, J.E.; Weng, Z.; Armstrong, J.C.; et al. Mammalian evolution of human cis-regulatory elements and transcription factor binding sites. Science 2023, 380, eabn7930. [Google Scholar] [CrossRef] [PubMed]
- Fisher, R.A. The Genetical Theory of Natural Selection; Clarendon Press: Oxford, UK; Dover: New York, NY, USA, 1930. [Google Scholar]
- Price, G.R. Fisher’s ‘fundamental theorem’ made clear. Ann. Hum. Genet. 1972, 36, 129–140. [Google Scholar] [CrossRef] [PubMed]
- Basener, W.F.; Sanford, J.C. The fundamental theorem of natural selection with mutations. J. Math. Biol. 2017, 76, 1589–1622. [Google Scholar] [CrossRef] [PubMed]
- Basener, W.; Cordova, S.; Hössjer, O.; Sanford, J. Dynamical Systems and Fitness Maximization in Evolutionary Biology. In Handbook of the Mathematics of the Arts and Sciences; Sriraman, B., Ed.; Springer: Cham, Switzerland, 2022. [Google Scholar] [CrossRef]
- Elena, S.F.; de Visser, J.A.G. Environmental stress and the effects of mutation. J. Biol. 2003, 2, 12. [Google Scholar] [CrossRef]
- Raine, A. The Anatomy of Violence: The Biological Roots of Crime; Vintage Books: New York, NY, USA, 2014. [Google Scholar]
- Eagleman, D. The Brain: The Story of You; Penguin Random House: New York, NY, USA, 2015. [Google Scholar]
- Lewis, M. The Biology of Desire: Why Addiction Is Not a Disease; PublicAffairs: New York, NY, USA, 2015. [Google Scholar]
- Feldman Barret, L. How Emotions Are Made: The Secret Life of the Brain; Houghton Mifflin Harcourt: New York, NY, USA, 2017. [Google Scholar]
- Sapolsky, R.M. Behave: The Biology of Humans at Out Best and Worst; Penguin Press: New York, NY, USA, 2017. [Google Scholar]
- Bernard, C. Leçons sur les Phénomènes de la vie, Communs aux Animaux et aux Végétaux; Librairie, J.B., Ed.; Baillière et Fils: Paris, France, 1878. [Google Scholar]
- Cannon, W.B. Organization for Physiological Homeostasis. Physiol. Rev. 1929, 9, 399–431. [Google Scholar] [CrossRef]
- Billman, G.E. Homeostasis: The Underappreciated and Far Too Often Ignored Central Organizing Principle of Physiology. Front. Physiol. 2020, 11, 200. [Google Scholar] [CrossRef]
- Butke, P.; Sheridan, S.C. An Analysis of the Relationship between Weather and Aggressive Crime in Cleveland, Ohio. Weather. Clim. Soc. 2010, 2, 127–139. [Google Scholar] [CrossRef]
- Kotas, M.E.; Medzhitov, R. Homeostasis, Inflammation, and Disease Susceptibility. Cell 2015, 160, 816–827. [Google Scholar] [CrossRef]
- Zivin, J.G.; Hsiang, S.M.; Neidell, M. Temperature and Human Capital in the Short and Long Run. J. Assoc. Environ. Resour. Econ. 2018, 5, 77–105. [Google Scholar] [CrossRef]
- Damasio, A. The Strange Order of Things: Life, Feeling, and the Making of Cultures; Pantheon: New York, NY, USA, 2018; 336p. [Google Scholar]
- McEwen, B.S. Stress, Adaptation, and Disease: Allostasis and Allostatic Load. Ann. N. Y. Acad. Sci. 1998, 840, 33–44. [Google Scholar] [CrossRef]
- Torday, J.S. A Central Theory of Biology. Med. Hypotheses 2015, 85, 49–57. [Google Scholar] [CrossRef] [PubMed]
- Riggs, J.E. Aging, Increasing Genomic Entropy, and Neurodegenerative Disease. Neurol. Clin. 1998, 16, 757–770. [Google Scholar] [CrossRef] [PubMed]
- Houck, P.D. Should negative entropy be included in the fundamental laws of biology? OA Biol. 2014, 2, 7. [Google Scholar]
- Poudel, R.C. A unified science of matter, life and evolution. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2023, 381, 20220291. [Google Scholar] [CrossRef] [PubMed]
- Peto, R.; Roe, F.J.; Lee, P.N.; Levy, L.; Clack, J. Cancer and ageing in mice and men. Br. J. Cancer 1975, 32, 411–426. [Google Scholar] [CrossRef] [PubMed]
- Peto, R. Epidemiology, multistage models, and short-term mutagenicity tests. In The Origins of Human Cancer; Cold Spring Harbor Conferences on Cell Proliferation; Hiatt, H.H., Watson, J.D., Winsten, J.A., Eds.; Cold Spring Harbor Laboratory: New York, NY, USA, 1977; Volume 4, pp. 1403–1428. [Google Scholar]
- Trifunovic, A.; Wredenberg, A.; Falkenberg, M.; Spelbrink, J.N.; Rovio, A.T.; Bruder, C.E.; Bohlooly-Y, M.; Gidlöf, S.; Oldfors, A.; Wibom, R.; et al. Premature ageing in mice expressing defective mitochondrial DNA polymerase. Nature 2004, 429, 417–423. [Google Scholar] [CrossRef] [PubMed]
- Caulin, A.F.; Maley, C.C. Peto’s Paradox: Evolution’s prescription for cancer prevention. Trends Ecol. Evol. 2011, 26, 175–182. [Google Scholar] [CrossRef] [PubMed]
- Nunney, L. The real war on cancer: The evolutionary dynamics of cancer suppression. Evol. Appl. 2012, 6, 11–19. [Google Scholar] [CrossRef]
- Seluanov, A.; Gladyshev, V.N.; Vijg, J.; Gorbunova, V. Mechanisms of cancer resistance in long-lived mammals. Nat. Rev. Cancer 2018, 18, 433–441. [Google Scholar] [CrossRef]
- Boddy, A.M.; Abegglen, L.M.; Pessier, A.P.; Aktipis, A.; Schiffman, J.D.; Maley, C.C.; Witte, C. Lifetime cancer prevalence and life history traits in mammals. Evol. Med. Public Health 2020, 2020, 187–195. [Google Scholar] [CrossRef]
- Vincze, O.; Colchero, F.; Lemaître, J.-F.; Conde, D.A.; Pavard, S.; Bieuville, M.; Urrutia, A.O.; Ujvari, B.; Boddy, A.M.; Maley, C.C.; et al. Cancer risk across mammals. Nature 2021, 601, 263–267. [Google Scholar] [CrossRef] [PubMed]
- Cushman, S.A. Entropy, Ecology and Evolution: Toward a Unified Philosophy of Biology. Entropy 2023, 25, 405. [Google Scholar] [CrossRef] [PubMed]
- Scirè, A.; Annovazzi-Lodi, V. The emergence of dynamic networks from many coupled polar oscillators: A paradigm for artificial life. Theory Biosci. 2023, 142, 291–299. [Google Scholar] [CrossRef] [PubMed]
- Swenson, R.; Turvey, M. Thermodynamic Reasons for Perception-Action Cycles. Ecol. Psychol. 1991, 3, 317–348. [Google Scholar] [CrossRef]
- Swenson, R. Autocatakinetics, Evolution, and the Law of Maximum Entropy Production: A Principled Foundation Towards the Study of Human Ecology. Adv. Hum. Ecol. 1997, 6, 1–47. [Google Scholar]
- Aerts, D.; Argüelles, J.A.; Beltran, L.; Sozzo, S. Development of a thermodynamics of human cognition and human culture. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2023, 381, 20220378. [Google Scholar] [CrossRef] [PubMed]
- Athalye, V.; Haven, E. Causal viewpoint and ensemble interpretation: From physics to the social sciences. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2023, 381, 20220279. [Google Scholar] [CrossRef]
- Bejan, A. The principle underlying all evolution, biological, geophysical, social and technological. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2023, 381, 20220288. [Google Scholar] [CrossRef]
- De Bari, B.; Dixon, J.; Kondepudi, D.; Vaidya, A. Thermodynamics, organisms and behaviour. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2023, 381, 20220278. [Google Scholar] [CrossRef]
- Gay-Balmaz, F.; Yoshimura, H. Systems, variational principles and interconnections in non-equilibrium thermodynamics. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2023, 381, 20220280. [Google Scholar] [CrossRef]
- Hall, C.A.S.; McWhirter, T. Maximum power in evolution, ecology and economics. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2023, 381, 20220290. [Google Scholar] [CrossRef] [PubMed]
- Lanchares, M.; Haddad, W.M. Stochastic thermodynamics: Dissipativity, accumulativity, energy storage and entropy production. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2023, 381, 20220284. [Google Scholar] [CrossRef] [PubMed]
- Martin, J.L. Theories of disorder and order, energy and information, in sociological thought. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2023, 381, 20220292. [Google Scholar] [CrossRef] [PubMed]
- Swenson, R. A grand unified theory for the unification of physics, life, information and cognition (mind). Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2023, 381, 20220277. [Google Scholar] [CrossRef] [PubMed]
- Tsallis, C. Non-additive entropies and statistical mechanics at the edge of chaos: A bridge between natural and social sciences. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2023, 381, 20220293. [Google Scholar] [CrossRef] [PubMed]
- Ván, P. Toward a universal theory of stable evolution. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2023, 381, 20220276. [Google Scholar] [CrossRef]
- Sparavigna, A.C. Entropies and fractal dimensions. Philica 2016, 559, 01377975. [Google Scholar]
- Mageed, I.A.; Bhat, A.H. Generalized Z-Entropy (Gze) and Fractal Dimensions. Appl. Math. Inf. Sci. 2022, 16, 829–834. [Google Scholar] [CrossRef]
- Sasa, S.-I.; Yokokura, Y. Thermodynamic Entropy as a Noether Invariant. Phys. Rev. Lett. 2016, 116, 140601. [Google Scholar] [CrossRef]
- Hermann, S.; Schmidt, M. Why Noether’s theorem applies to statistical mechanics. J. Physics Condens. Matter 2022, 34, 213001. [Google Scholar] [CrossRef]
- Johari, G. Entropy, enthalpy and volume of perfect crystals at limiting high pressure and the third law of thermodynamics. Thermochim. Acta 2021, 698, 178891. [Google Scholar] [CrossRef]
- Hoffmann, H.-J. Energy and entropy of crystals, melts and glasses or what is wrong in Kauzmann’s paradox? (Energie und Entropie von Kristallen, Schmelzen und Gläsern oder wo steckt der Fehler in Kauzmanns Paradoxon?). Mater. Sci. Eng. Technol. 2012, 43, 528–533. [Google Scholar] [CrossRef]
- McFadden, J.; Al-Khalili, J. Life on the Edge: The Coming of Age of Quantum Biology; Transworld Publishers: London, UK; Penguin Random House: New York, NY, USA, 2015. [Google Scholar]
- Doudna, J.A.; Sternberg, S.H. A Crack in Creation: Gene Editing and the Unthinkable Power to Control Evolution; Houghton Mifflin Harcourt Publishing Company: New York, NY, USA, 2017. [Google Scholar]
- Meena, C.; Hens, C.; Acharyya, S.; Haber, S.; Boccaletti, S.; Barzel, B. Emergent stability in complex network dynamics. Nat. Phys. 2023, 19, 1033–1042. [Google Scholar] [CrossRef]
- Pavlos, G.; Iliopoulos, A.; Zastenker, G.; Zelenyi, L.; Karakatsanis, L.; Riazantseva, M.; Xenakis, M.; Pavlos, E. Tsallis non-extensive statistics and solar wind plasma complexity. Phys. A Stat. Mech. Its Appl. 2015, 422, 113–135. [Google Scholar] [CrossRef]
- Reis, A.H. Use and validity of principles of extremum of entropy production in the study of complex systems. Ann. Phys. 2014, 346, 22–27. [Google Scholar] [CrossRef]
- Ivanitskii, G.R. Self-organizing dynamic stability of far-from-equilibrium biological systems. Physics-Uspekhi 2017, 60, 705–730. [Google Scholar] [CrossRef]
- Benzi, R.; Ciliberto, S.; Tripiccione, R.; Baudet, C.; Massaioli, F.; Succi, S. Extended self-similarity in turbulent flows. Phys. Rev. E 1993, 48, R29–R32. [Google Scholar] [CrossRef]
- Sagan, H. Space-Filling Curves; Springer: Dordrecht, The Netherlands, 1994; ISBN 038720170X. [Google Scholar]
- Benitez, F.; Romero-Maltrana, D.; Razeto-Barry, P. (Re)interpreting E = mc2. Found. Phys. 2022, 52, 1–19. [Google Scholar] [CrossRef]
- Torday, J. The cell as the mechanistic basis for evolution. WIREs Syst. Biol. Med. 2015, 7, 275–284. [Google Scholar] [CrossRef]
- Torday, J.S. Life Is Simple—Biologic Complexity Is an Epiphenomenon. Biology 2016, 5, 17. [Google Scholar] [CrossRef]
- Torday, J.S.; Miller, W.B. Life is determined by its environment. Int. J. Astrobiol. 2016, 15, 345–350. [Google Scholar] [CrossRef]
- Venegas-Aravena, P.; Cordaro, E.G. Subduction as a Smoothing Machine: How Multiscale Dissipation Relates Precursor Signals to Fault Geometry. Geosciences 2023, 13, 243. [Google Scholar] [CrossRef]
- Contreras-Reyes, E.; Flueh, E.R.; Grevemeyer, I. Tectonic control on sediment accretion and subduction off south central Chile: Implications for coseismic rupture processes of the 1960 and 2010 megathrust earthquakes. Tectonics 2010, 29, 1–27. [Google Scholar] [CrossRef]
- Zielke, O.; Galis, M.; Mai, P.M. Fault roughness and strength heterogeneity control earthquake size and stress drop. Geophys. Res. Lett. 2017, 44, 777–783. [Google Scholar] [CrossRef]
- Schurr, B.; Moreno, M.; Tréhu, A.M.; Bedford, J.; Kummerow, J.; Li, S.; Oncken, O. Forming a Mogi Doughnut in the Years Prior to and Immediately Before the 2014 M8.1 Iquique, Northern Chile, Earthquake. Geophys. Res. Lett. 2020, 47, e2020GL088351. [Google Scholar] [CrossRef]
- Venegas-Aravena, P. Geological earthquake simulations generated by kinematic heterogeneous energy-based method: Self-arrested ruptures and asperity criterion. Open Geosci. 2023, 15, 20220522. [Google Scholar] [CrossRef]
- Venegas-Aravena, P.; Cordaro, E.G. Analytical Relation between b-Value and Electromagnetic Signals in Pre-Macroscopic Failure of Rocks: Insights into the Microdynamics’ Physics Prior to Earthquakes. Geosciences 2023, 13, 169. [Google Scholar] [CrossRef]
- Aoki, I. Entropy production in human life span: A thermodynamical measure for aging. AGE 1994, 17, 29–31. [Google Scholar] [CrossRef]
- Sacco, R.G.; Torday, J.S. Systems biology of human aging: A Fibonacci time series model. Prog. Biophys. Mol. Biol. 2023, 177, 24–33. [Google Scholar] [CrossRef]
- Sloman, S.; Fernbach, P. The Knowledge Illusion: Why We Never Think Alone; Penguin: New York, NY, USA, 2017. [Google Scholar]
- González-Gómez, P.; Razeto-Barry, P.; Araya-Salas, M.; Estades, C. Does Environmental Heterogeneity Promote Cognitive Abilities? Integr. and Comp. Bio. 2015, 55, 432–443. [Google Scholar] [CrossRef]
- Friston, K. The free-energy principle: A rough guide to the brain? Trends Cogn. Sci. 2009, 13, 293–301. [Google Scholar] [CrossRef]
- Andrews, M. The math is not the territory: Navigating the free energy principle. Biol. Philos. 2021, 36, 30. [Google Scholar] [CrossRef]
- Mazzaglia, P.; Verbelen, T.; Çatal, O.; Dhoedt, B. The Free Energy Principle for Perception and Action: A Deep Learning Perspective. Entropy 2022, 24, 301. [Google Scholar] [CrossRef] [PubMed]
- Friston, K.; Friston, K. The free-energy principle: A unified brain theory? Nat. Rev. Neurosci. 2010, 11, 127–138. [Google Scholar] [CrossRef] [PubMed]
- Dodel, S.; Tognoli, E.; Kelso, J.A.S. Degeneracy and Complexity in Neuro-Behavioral Correlates of Team Coordination. Front. Hum. Neurosci. 2020, 14, 328. [Google Scholar] [CrossRef] [PubMed]
- Carroll, S.B. The Serengeti Rules: The Quest to Discover How Life Works and Why It Matters; Princeton University: Princeton, NJ, USA, 2016. [Google Scholar]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Venegas-Aravena, P.; Cordaro, E.G. The Multiscale Principle in Nature (Principium luxuriæ): Linking Multiscale Thermodynamics to Living and Non-Living Complex Systems. Fractal Fract. 2024, 8, 35. https://doi.org/10.3390/fractalfract8010035
Venegas-Aravena P, Cordaro EG. The Multiscale Principle in Nature (Principium luxuriæ): Linking Multiscale Thermodynamics to Living and Non-Living Complex Systems. Fractal and Fractional. 2024; 8(1):35. https://doi.org/10.3390/fractalfract8010035
Chicago/Turabian StyleVenegas-Aravena, Patricio, and Enrique G. Cordaro. 2024. "The Multiscale Principle in Nature (Principium luxuriæ): Linking Multiscale Thermodynamics to Living and Non-Living Complex Systems" Fractal and Fractional 8, no. 1: 35. https://doi.org/10.3390/fractalfract8010035
APA StyleVenegas-Aravena, P., & Cordaro, E. G. (2024). The Multiscale Principle in Nature (Principium luxuriæ): Linking Multiscale Thermodynamics to Living and Non-Living Complex Systems. Fractal and Fractional, 8(1), 35. https://doi.org/10.3390/fractalfract8010035







