Several Quantum Hermite–Hadamard-Type Integral Inequalities for Convex Functions
Abstract
1. Introduction
2. Preliminary on -Calculus and Inequalities
3. Results
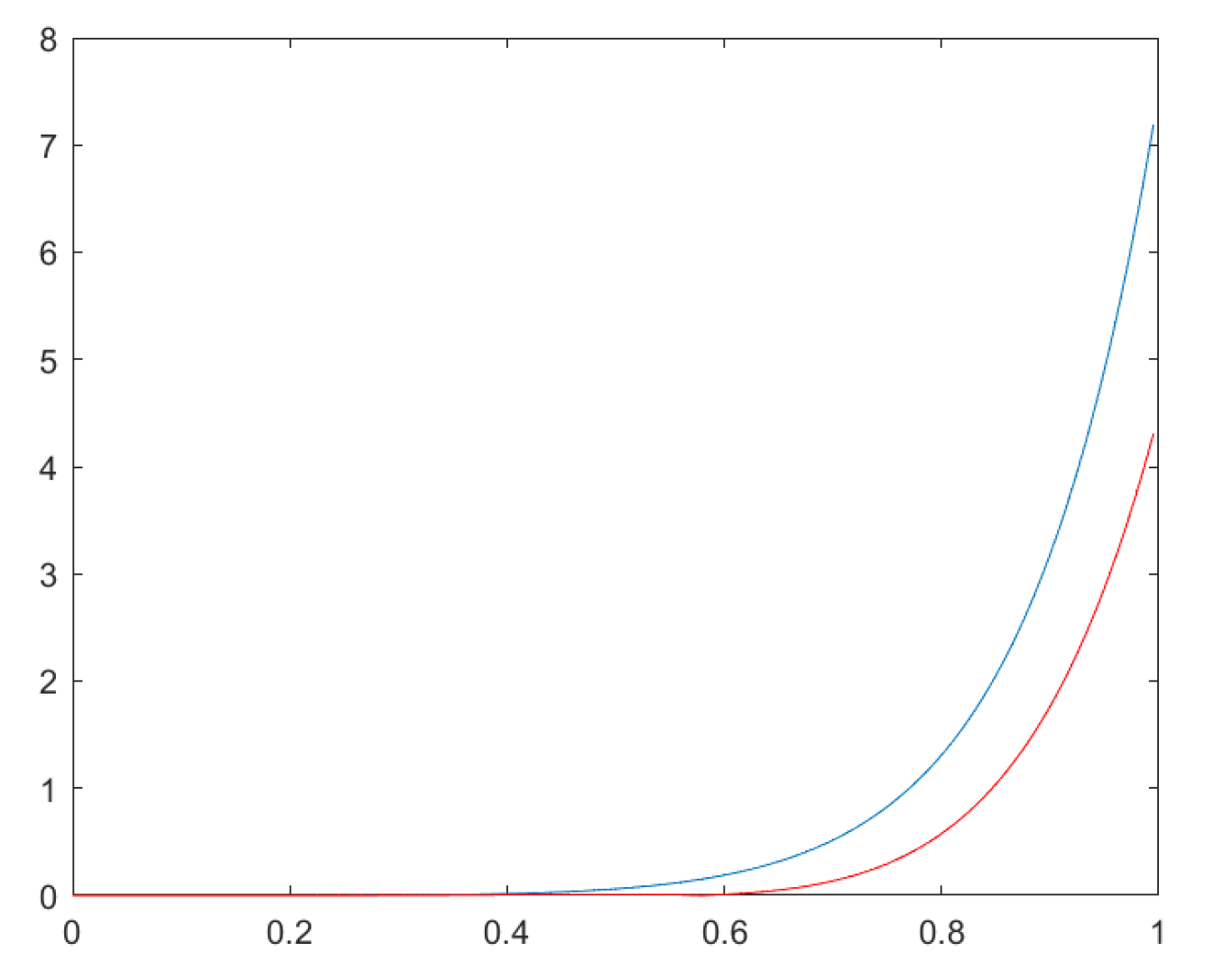
4. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Cloud, M.J.; Drachman, B.C.; Lebedev, L.P. Inequalities with Applications to Engineering; Springer: London, UK, 2014. [Google Scholar]
- Liao, J.G.; Berg, A. Sharpening Jensen’s inequality. Am. Stat. 2019, 73, 278–281. [Google Scholar]
- Khan, M.A.; Anwar, S.; Khalid, S.; Sayed, Z.M.M.M. Inequalities of the type Hermite-Hadamard-Jensen-Mercer for strong convexity. Math. Probl. Eng. 2021, 2021, 5386488. [Google Scholar]
- Fridli, S.; Schipp, F. Strong approximation via Sidon type inequalities. J. Approx. Theory 1998, 94, 263–284. [Google Scholar] [CrossRef]
- Milovanovic, G.V.; Rassias, M.T. Analytic Number Theory, Approximation Theory and Special Functions; Springer: Berlin/Heidelberg, Germany, 2014; p. 891. [Google Scholar]
- Dragomir, S.S. Inequalities with applications in numerical analysis. AIP Conf. Proc. 2007, 936, 681. [Google Scholar]
- Dragomir, S.S.; Rassias, M.T. Ostrowski Type Inequalities and Applications in Numerical Integration; Springer: Berlin/Heidelberg, Germany, 2013; p. 504. [Google Scholar]
- Kalsoom, H.; Vivas-Cortez, M. q1q2-Ostrowski-type integral inequalities involving property of generalized higher-order strongly n-polynomial preinvexity. Symmetry 2022, 14, 717. [Google Scholar] [CrossRef]
- Varosanec, S. On h-convexity. J. Math. Anal. Appl. 2007, 326, 303–311. [Google Scholar] [CrossRef]
- Bernstein, S.N. Sur les fonctions absolument monotones. Acta Math. 1929, 52, 1–66. [Google Scholar] [CrossRef]
- Avriel, M. r-Convex functions. Math. Program. 1972, 2, 309–323. [Google Scholar] [CrossRef]
- Dragomir, S.S. On Hadamard’s inequality for convex functions on the co-ordinates in a rectangle from the plane. Taiwan J. Math. 2001, 5, 775–788. [Google Scholar]
- Alomari, M.; Latif, M.A. On Hadamard-type inequalities for h-convex functions on the co-ordinates. Int. J. Math. Anal. 2009, 3, 1645–1656. [Google Scholar]
- Rashid, S.; Noor, M.A.; Noor, K.I. Some new estimates for exponentially (h,m)-convex functions via extended generalized fractional integral operators. Korean J. Math. 2019, 27, 843–860. [Google Scholar]
- Iqbal, W.; Rehman, A.U.; Farid, G.; Rathour, L.; Sharma, M.K.; Mishra, N. Further on Petrovic’s types inequalities. J. Appl. Math. Inform. 2022, 40, 1021–1034. [Google Scholar]
- Pal, S.; Wong, T.K. Exponentially concave functions and a new information geometry. Ann. Probab. 2018, 46, 1070–1113. [Google Scholar] [CrossRef]
- Hanson, M.A. On sufficiency of the Kuhn-Tucker conditions. J. Math. Anal. Appl. 1981, 80, 545–550. [Google Scholar] [CrossRef]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; North-Holland Mathematical Studies; Elsevier (North-Holland) Science Publishers: Amsterdam, The Netherlands; London, UK; New York, NY, USA, 2006; Volume 204. [Google Scholar]
- Srivastava, H.M.; Karlsson, P.W. Gaussian Hypergeometric Series; Halsted Press (Ellis Horwood Limited, Chichester): Chichester, UK; John Wiley and Sons: New York, NY, USA; Chichester, UK; Brisbane, Australia; Toronto, ON, Canada, 1985. [Google Scholar]
- Barnett, N.S.; Dragomir, S.S. Some elementary inequalities for the expectation and variance of a random variable whose pdf is defined on a finite interval. RGMIA Res. Rep. Coll. 1999, 2, 1–7. [Google Scholar]
- El-Deeb, A.A.; Awrejcewicz, J. Ostrowski-trapezoidal-Gruss-type on (q,ω)-Hahn difference operator. Symmetry 2022, 14, 1776. [Google Scholar] [CrossRef]
- Dasgupta, A.; Fajardo-Montenegro, J. Aspects of Quantum Gravity Phenomenology and Astrophysics. Universe 2023, 9, 128. [Google Scholar] [CrossRef]
- Jackson, F.H. On q-difference equations. Am. J. Math. 1910, 32, 305–314. [Google Scholar] [CrossRef]
- Siegel, W. Introduction to String Field Theory. Advanced Series in Mathematical Physics; World Scientific: Teaneck, NJ, USA, 1998; Volume 8. [Google Scholar]
- Agarwal, R. A propos d’une note de m. pierre humbert. Comptes Rendus De L’Academie Des Sci. 1953, 236, 2031–2032. [Google Scholar]
- Tariboon, J.; Ntouyas, S.K. Quantum calculus on finite intervals and aplications to impulsive difference equations. Adv. Differ. Equ. 2013, 2013, 282. [Google Scholar] [CrossRef]
- Noor, M.; Noor, K.; Awan, M. Quantum Ostrowski inequalities for q-differentiable convex functions. J. Math. Inequal. 2016, 10, 1013–1018. [Google Scholar] [CrossRef]
- Sahoo, S.K.; Kashuri, A.; Aljuaid, M.; Mishra, S.; Sen, M. On Ostrowski-Mercer’s type fractional inequalities for convex functions and applications. Fractal Fract. 2023, 7, 215. [Google Scholar] [CrossRef]
- Alp, N.; Sarikaya, M.Z.; Kunt, M.; Iscan, I. q2-Hermite-Hadamard inequalities and quantum estimates for midpoint type inequalities via convex and quasi-convex functions. J. King Saud Univ. Sci. 2018, 30, 193–203. [Google Scholar] [CrossRef]
- Deng, Y.; Awan, M.U.; Wu, S. Quantum Integral Inequalities of Simpson-Type for Strongly Preinvex Functions. Mathematics 2019, 7, 751. [Google Scholar] [CrossRef]
- Deng, Y.; Kalsoom, H.; Wu, S. Some new quantum Hermite-Hadamard-Type Estimates Within a Class of Generalized (s,m)-Preinvex Functions. Symmetry 2019, 10, 1283. [Google Scholar] [CrossRef]
- Kara, H.; Budak, H.; Alp, N.; Kalsoom, H.; Sarikaya, M.Z. On new generalized quantum integrals and related Hermite-Hadamard inequalities. J. Inequalities Appl. 2021, 2021, 180. [Google Scholar] [CrossRef]
- Khan, A.; Chu, Y.M.; Khan, T.U.; Khan, J. Some new inequalities of Hermite-Hadamard type for s-convex functions with applications. Open Math. 2017, 15, 1414–1430. [Google Scholar] [CrossRef]
- Latif, M.A.; Dragomir, S. New inequalities of Hermite-Hadamard type for n-times differentiable convex and concave functions with applications. Filomat 2016, 30, 2609–2621. [Google Scholar] [CrossRef]
- Luangboon, W.; Nonlaopon, K.; Tariboon, J.; Ntouyas, S.K.; Budak, H. Post-Quantum Ostrowski type integral inequalities for twice (p,q)-differentiable functions. J. Math. Ineq. 2022, 16, 1129–1144. [Google Scholar] [CrossRef]
- Alp, N.; Budak, H.; Sarikaya, M.Z.; Ali, M.A. On new refinements and generalizations of q-Hermite-Hadamard inequalities for convex functions. Rocky Mountain J. Math. 2023. Available online: https://projecteuclid.org/journals/rmjm/rocky-mountain-journal-of-mathematics/DownloadAcceptedPapers/220708-Budak.pdf (accessed on 4 June 2023).
- Jhanthanam, S.; Tariboon, J.; Ntouyas, S.K.; Nonlaopon, K. On q-Hermite-Hadamard inequalities for differentiable convex functions. Mathematics 2019, 7, 632. [Google Scholar] [CrossRef]
- Zhao, D.; Ali, M.A.; Luangboon, W.; Budak, H.; Nonlaopon, K. Some Generalizations of Different Types of Quantum Integral Inequalities for Differentiable Convex Functions with Applications. Fractal Fract. 2022, 6, 129. [Google Scholar] [CrossRef]
- Ciurdariu, L.; Grecu, E. Post-quantum integral inequalities for three-times (p,q)-differential functions. Symmetry 2023, 246, 15. [Google Scholar]
- Hadamard, J. Etude sur les proprietes des fonctions entieres en particulier d’une fonction consideree par Riemann. J. Math. Pures. Appl. 1893, 58, 171–215. [Google Scholar]
- Tariboon, J.; Ntouyas, S.K. Quantum integral inequalities on finite intervals. J. Inequal. Appl. 2014, 13, 121. [Google Scholar] [CrossRef]
- Ernst, T. A Comprehensive Treatment of q-Calculus; Springer: Basel, Switerland, 2012. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ciurdariu, L.; Grecu, E. Several Quantum Hermite–Hadamard-Type Integral Inequalities for Convex Functions. Fractal Fract. 2023, 7, 463. https://doi.org/10.3390/fractalfract7060463
Ciurdariu L, Grecu E. Several Quantum Hermite–Hadamard-Type Integral Inequalities for Convex Functions. Fractal and Fractional. 2023; 7(6):463. https://doi.org/10.3390/fractalfract7060463
Chicago/Turabian StyleCiurdariu, Loredana, and Eugenia Grecu. 2023. "Several Quantum Hermite–Hadamard-Type Integral Inequalities for Convex Functions" Fractal and Fractional 7, no. 6: 463. https://doi.org/10.3390/fractalfract7060463
APA StyleCiurdariu, L., & Grecu, E. (2023). Several Quantum Hermite–Hadamard-Type Integral Inequalities for Convex Functions. Fractal and Fractional, 7(6), 463. https://doi.org/10.3390/fractalfract7060463




