Characteristics of New Stochastic Solitonic Solutions for the Chiral Type of Nonlinear Schrödinger Equation
Abstract
1. Introduction
2. Preliminaries
2.1. Description of the Method
2.2. Wiener Process
- (i)
- is a continuous functions of ,
- (ii)
- For is independent of increments,
- (iii)
- has a normal distribution through mean 0 and variance .
3. The Stochastic Solutions for Equation (1)
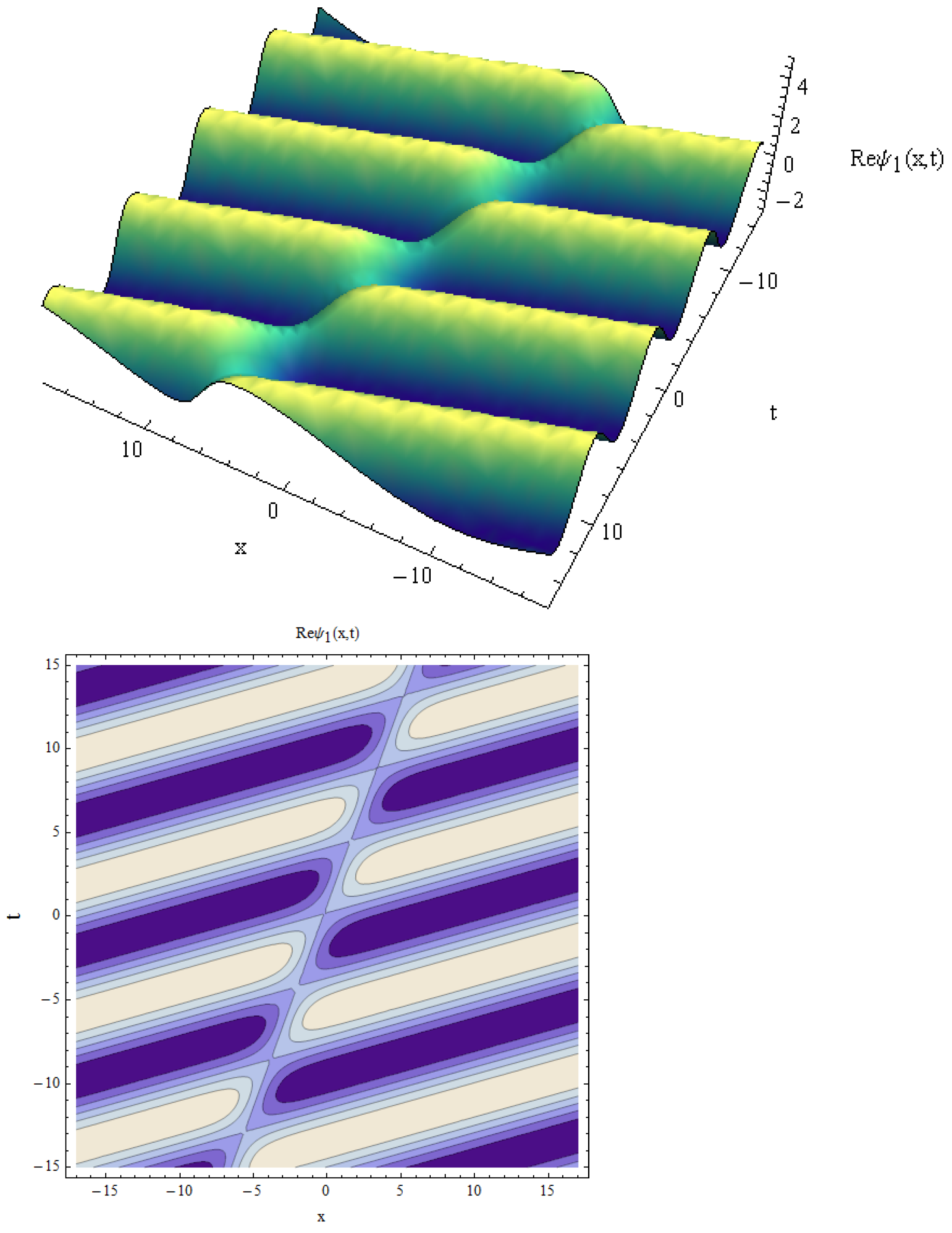
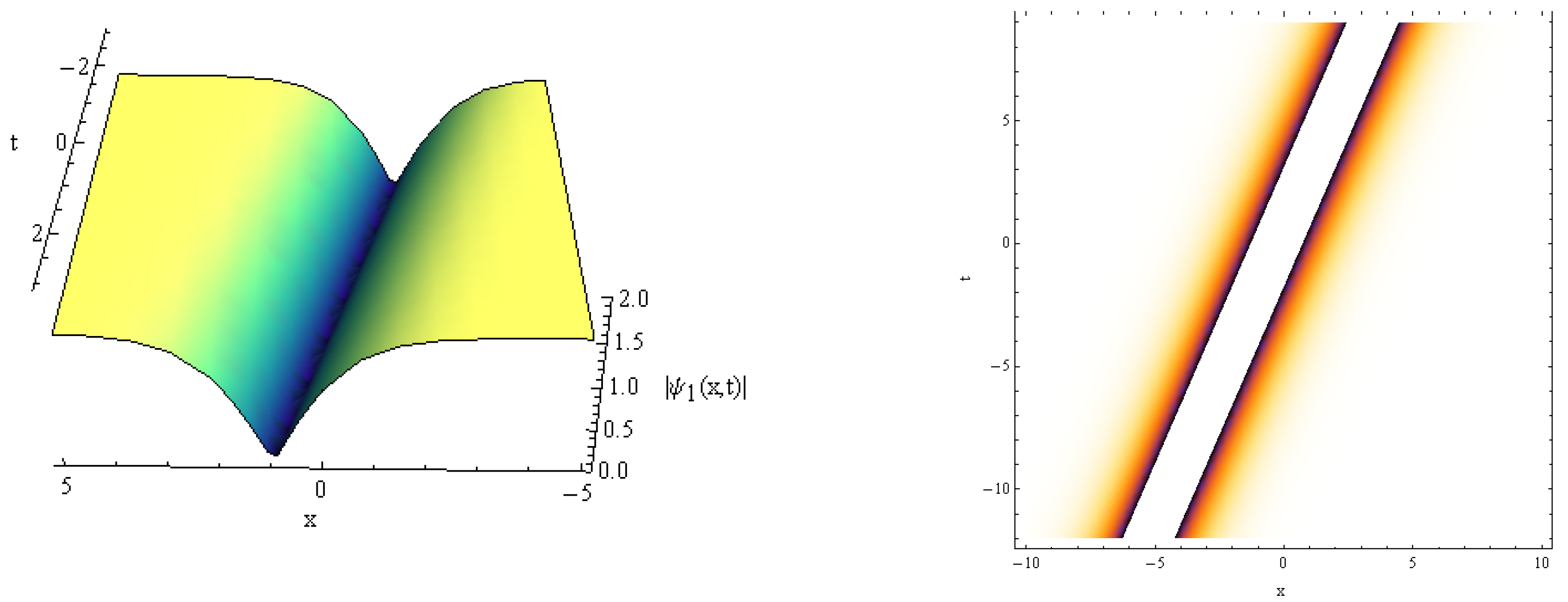
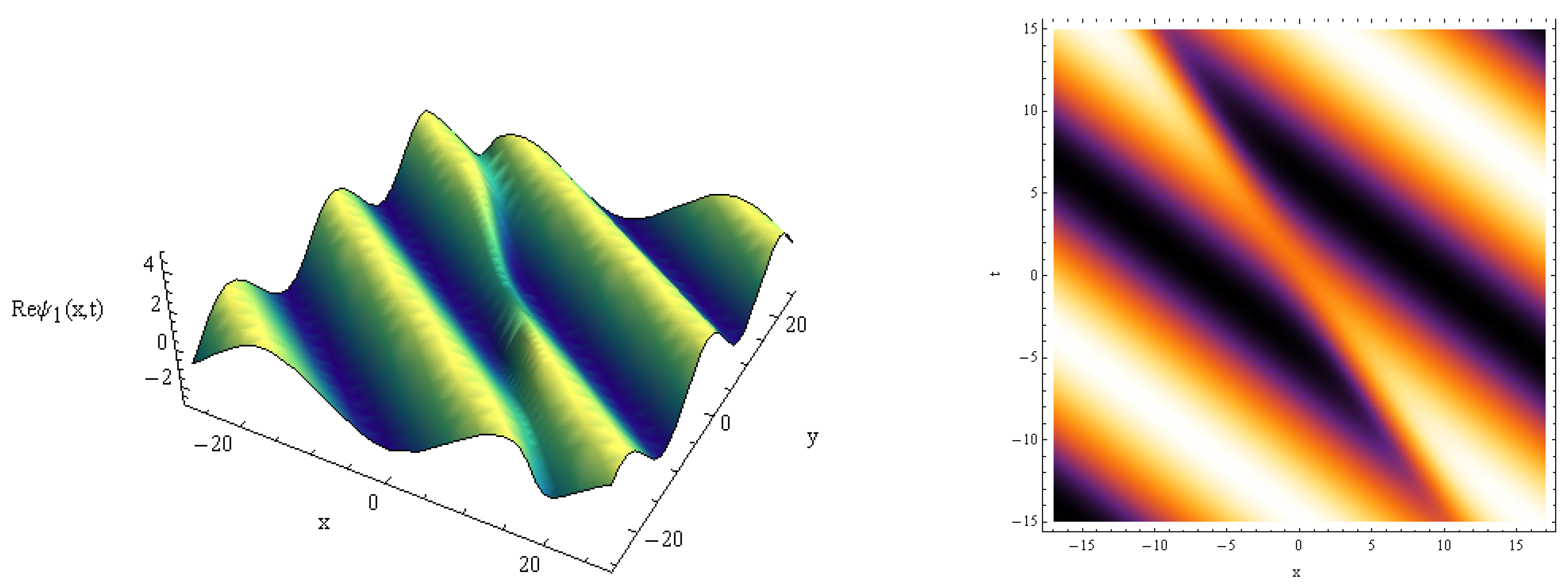
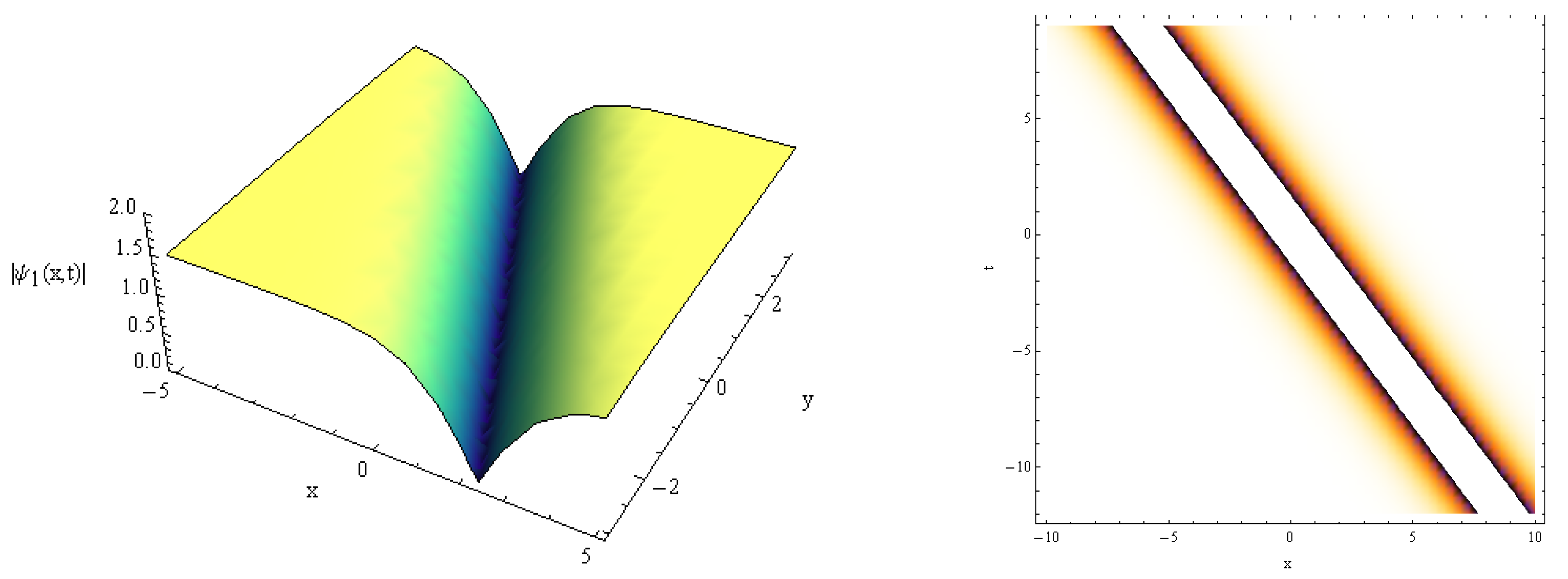
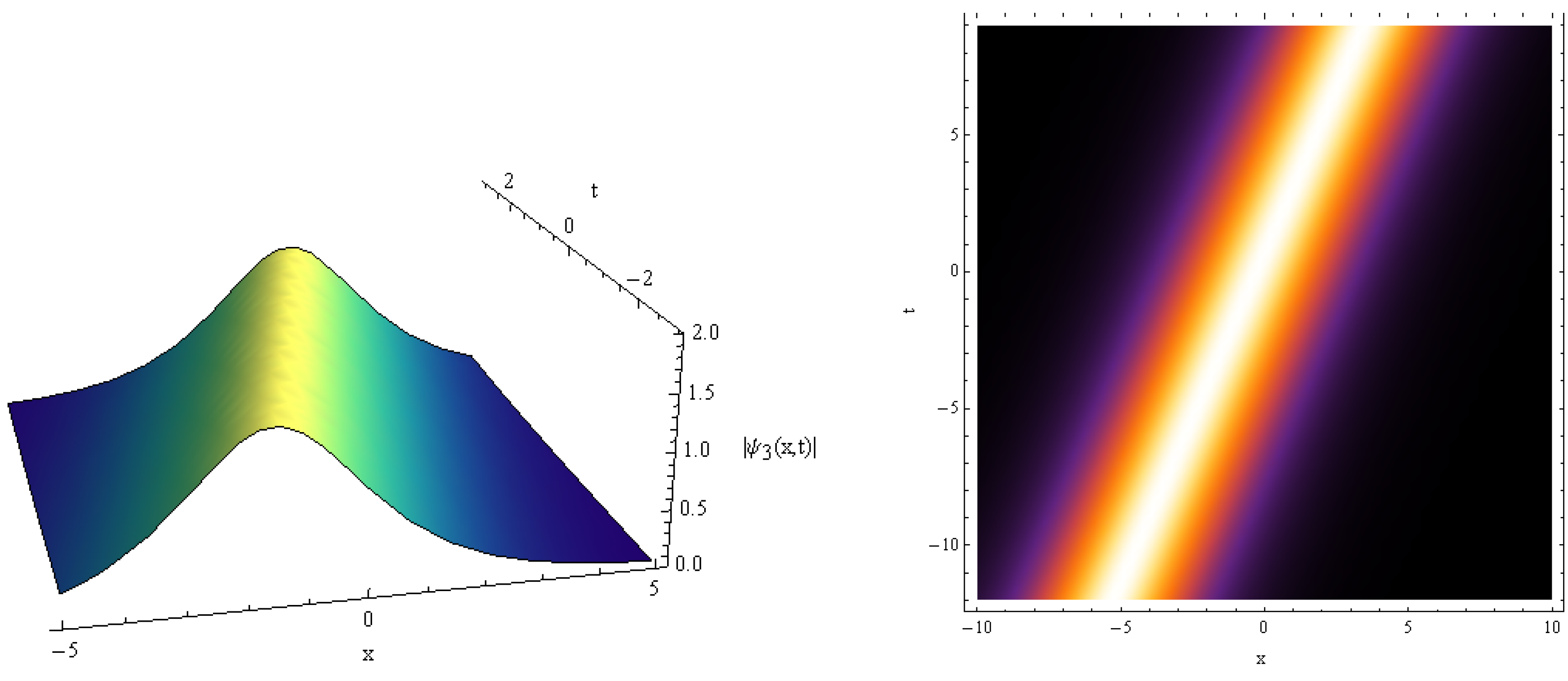
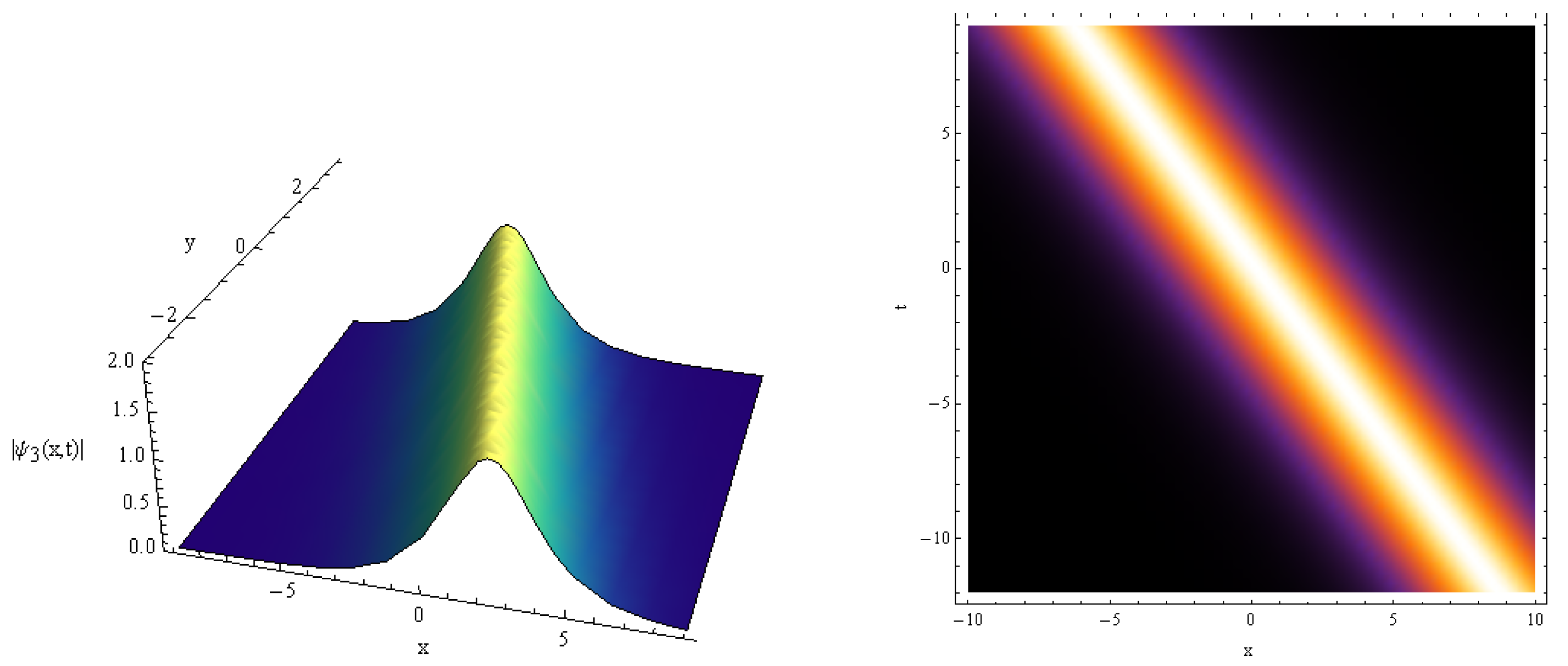
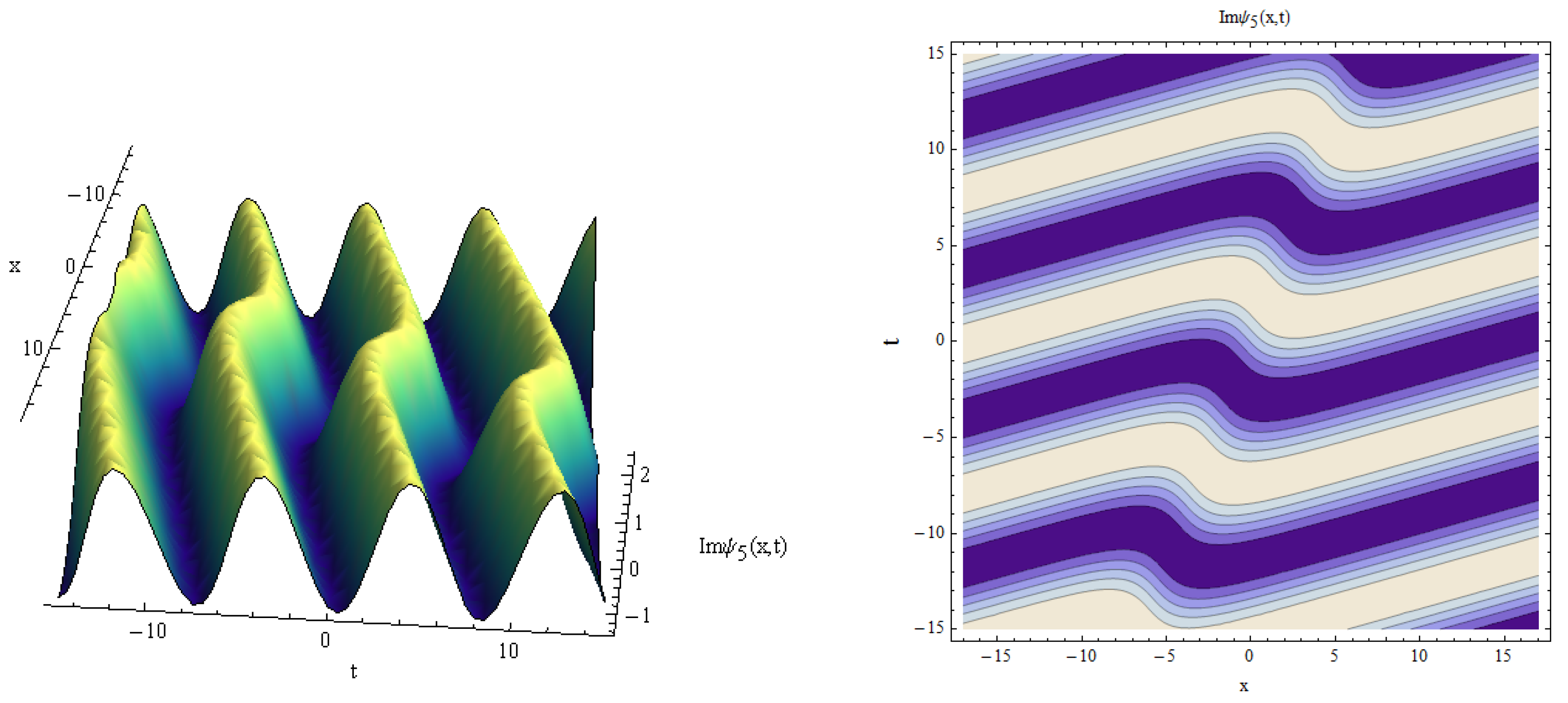
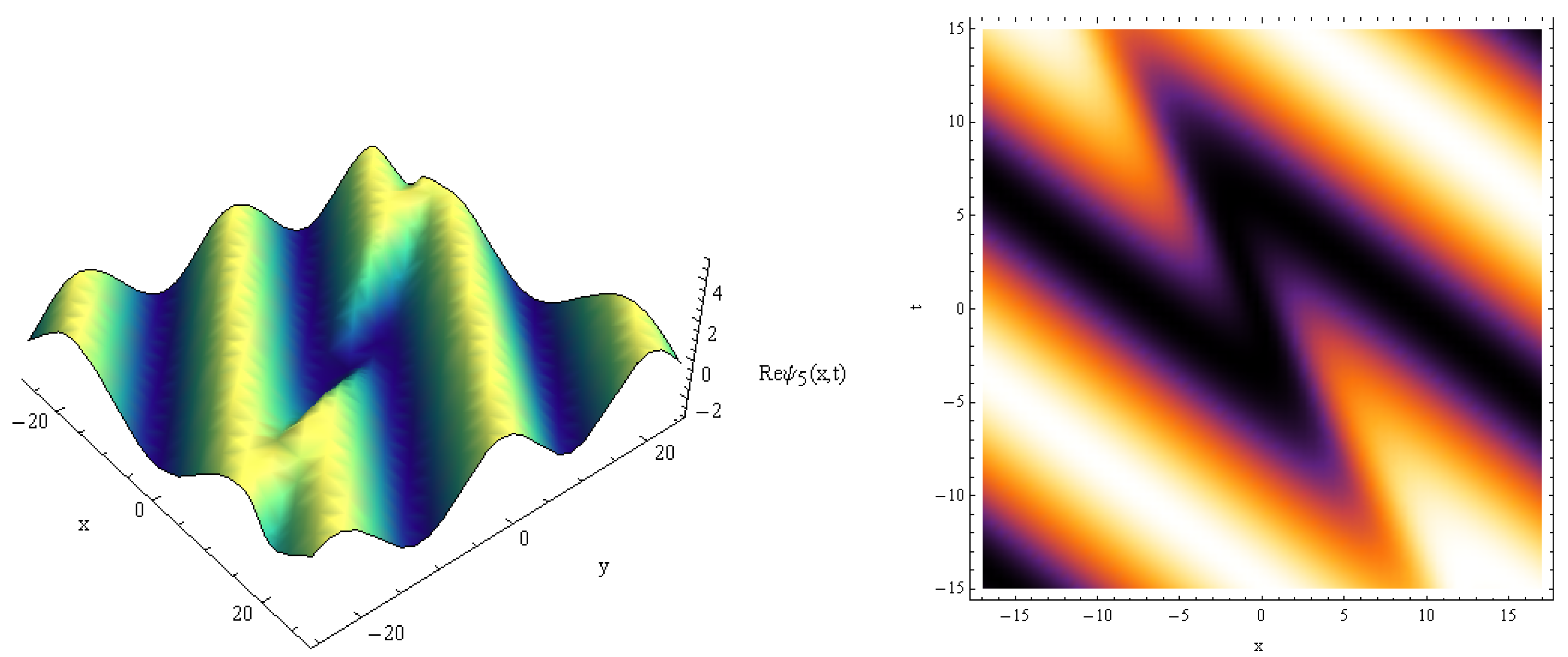
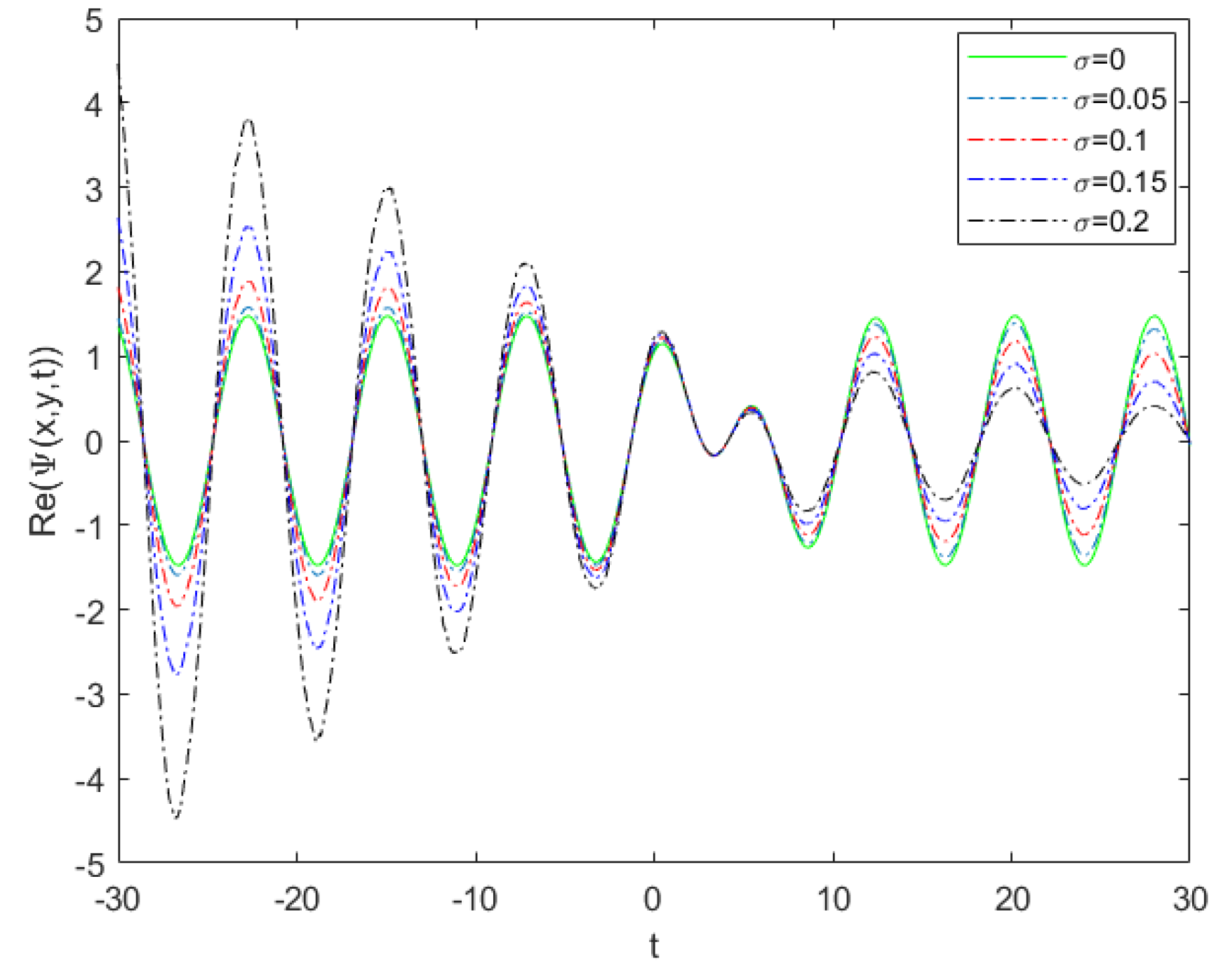
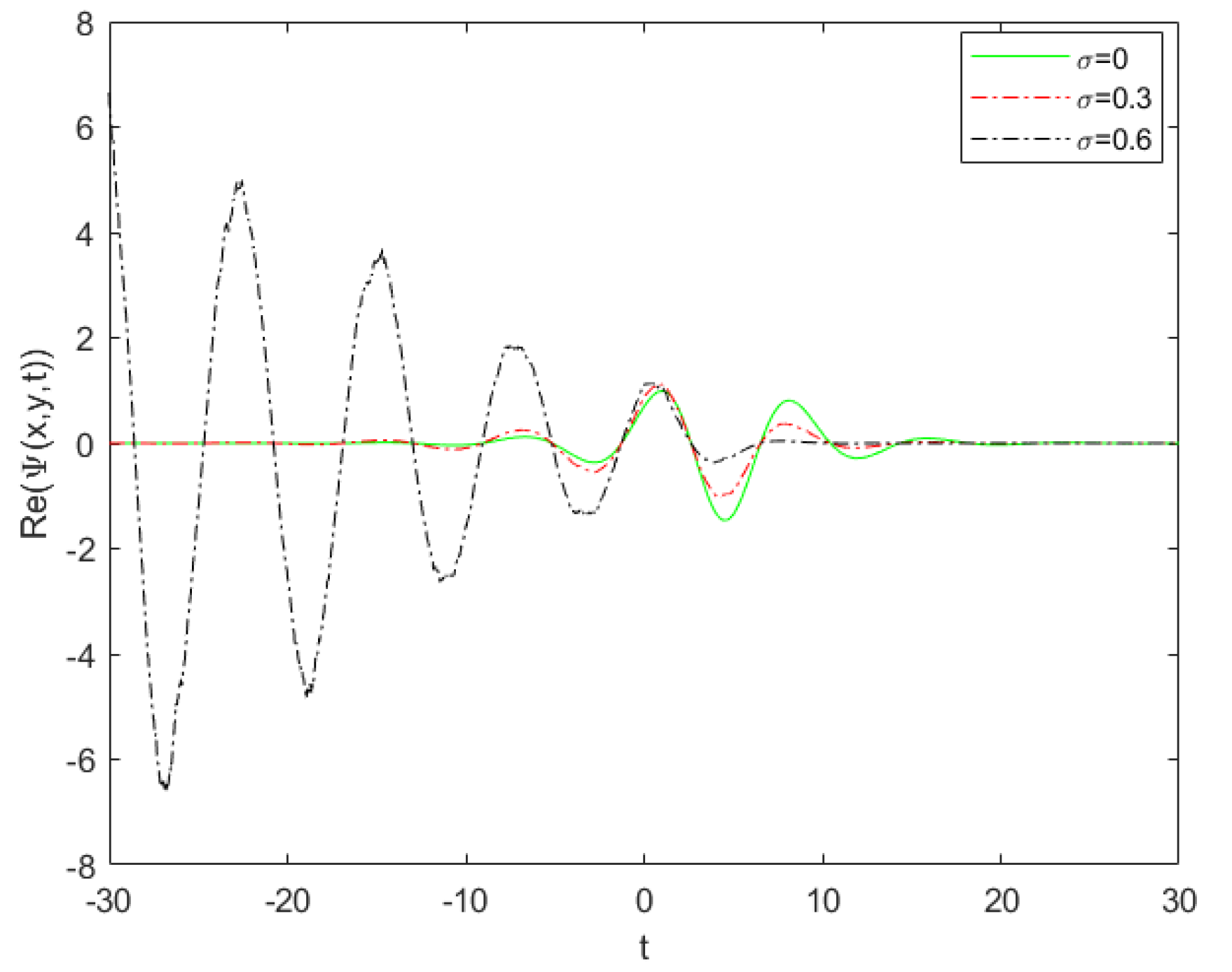
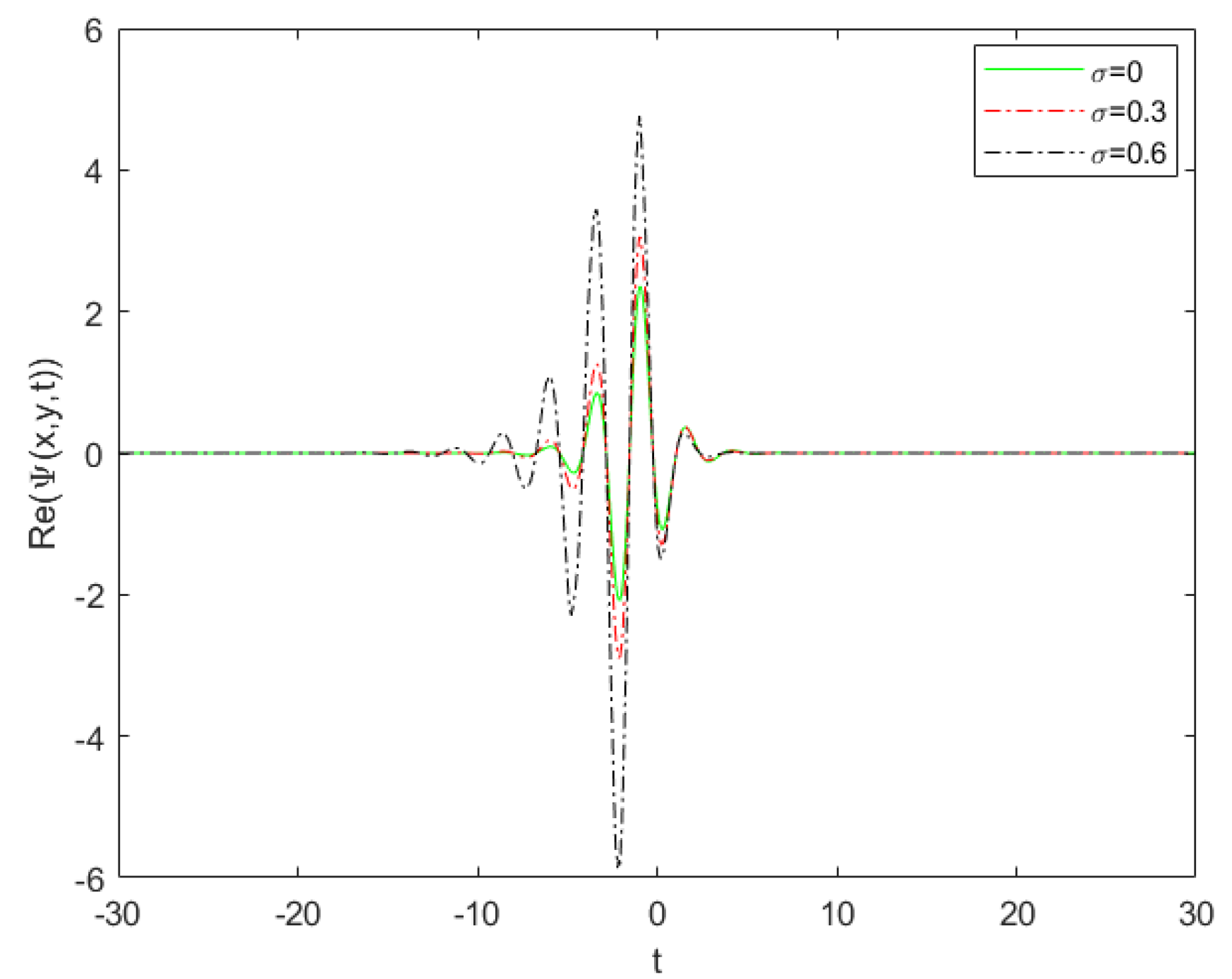
4. Results and Discussion
- The solver proposed in this study can be applied to large classes of nonlinear stochastic partial differential equations (NSPDEs).
- The suggested solver is simple to implement for solving stochastic fractional NPDEs.
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Younis, M.; Ali, S.; Mahmood, S.A. Solitons for compound KdV Burgers equation with variable coefficients and power law nonlinearity. Nonlinear Dyn. 2015, 81, 1191–1196. [Google Scholar] [CrossRef]
- Alharbi, A.; Almatrafi, M.B. Exact solitary wave and numerical solutions for geophysical KdV equation. J. King Saud Univ.-Sci. 2022, 34, 102087. [Google Scholar] [CrossRef]
- Alharbi, A.R.; Abdelrahman, M.A.E.; Almatrafi, M.B. Analytical and numerical investigation for the DMBBM equation. CMES-Comput. Model. Eng. Sci. 2020, 122, 743–756. [Google Scholar] [CrossRef]
- Abdelrahman, M.A.E.; Almatrafi, M.B.; Alharbi, A.R. Fundamental solutions for the coupled KdV system and its stability. Symmetry 2020, 12, 429. [Google Scholar] [CrossRef]
- Abdelrahman, M.A.E.; Abdo, N.F. On the nonlinear new wave solutions in unstable dispersive environments. Phys. Scr. 2020, 95, 045220. [Google Scholar] [CrossRef]
- Ullah, N.; Asjad, M.I.; Hussanan, A.; Akgül, A.; Alharbi, W.R.; Algarni, H.; Yahia, I.S. Novel waves structures for two nonlinear partial differential equations arising in the nonlinear optics via Sardar-subequation method. Alex. Eng. J. 2023, 71, 105–113. [Google Scholar] [CrossRef]
- Biswas, A.; Konar, S. Introduction to Non-Kerr Law Optical Solitons; Chapman and Hall, CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar]
- Mirzazadeh, Q.Z.Q.M.; Ekici, M.; Sonmezoglu, A. Analytical study of solitons in non-Kerr nonlinear negative-index materials. Nonlinear Dyn. 2016, 86, 623–638. [Google Scholar]
- Inc, M.; Aliyu, A.I.; Yusuf, A.; Bayram, M.; Baleanu, D. Optical solitons to the (n+1)-dimensional nonlinear Schrödinger’s equation with Kerr law and power law nonlinearities using two integration schemes. Mod. Lett. B 2019, 33, 1950223. [Google Scholar] [CrossRef]
- Kuo, C.K.; Ghanbari, B. Resonant multi-soliton solutions to new (3+1)-dimensional Jimbo-Miwa equations by applying the linear superposition principle. Nonlinear Dyn. 2019, 96, 459–464. [Google Scholar] [CrossRef]
- Almatrafi, M.B.; Alharbi, A.; Tunç, C. Constructions of the soliton solutions to the good Boussinesq equation. Adv. Differ. Equ. 2020, 2020, 629. [Google Scholar] [CrossRef]
- Shaikh, T.S.; Baber, M.Z.; Ahmed, N.; Shahid, N.; Akgül, A.; la Sen, M.D. On the soliton solutions for the stochastic Konno–Oono system in magnetic field with the presence of noise. Mathematics 2023, 11, 1472. [Google Scholar] [CrossRef]
- Younis, M.; Rizvi, S.T.R. Dispersive dark optical soliton in (2+1)-dimensions by -expansion with dual-power law nonlinearity. Optik 2015, 126, 5812–5814. [Google Scholar] [CrossRef]
- Khodadad, F.S.; Nazari, F.; Eslami, M.; Rezazadeh, H. Soliton solutions of the conformable fractional Zakharov-Kuznetsov equation with dual-power law nonlinearity. Opt. Quantum Electron. 2017, 49, 384. [Google Scholar] [CrossRef]
- Ashraf, R.; Ashraf, F.; Akgül, A.; Ashraf, S.; Alshahrani, B.; Mahmoud, M.; Weera, W. Some new soliton solutions to the (3+1)-dimensional generalized KdV-ZK equation via enhanced modified extended tanh-expansion approach. Alex. Eng. J. 2023, 69, 303–309. [Google Scholar] [CrossRef]
- Li, B.Q. Phase transitions of breather of a nonlinear Schrödinger equation in inhomogeneous optical fiber system. Optik 2020, 217, 164670. [Google Scholar] [CrossRef]
- Li, B.Q.; Ma, Y.L. Extended generalized Darboux transformation to hybrid rogue wave and breather solutions for a nonlinear Schrödinger equation. Appl. Math. Comput. 2020, 386, 125469. [Google Scholar] [CrossRef]
- Biswas, A. chiral solitons in 1+2 dimensions. Int. J. Theor. Phys 2009, 48, 3403–3409. [Google Scholar] [CrossRef]
- Alharbi, Y.F.; El-Shewy, E.K.; Abdelrahman, M.A.E. Effects of Brownian noise strength on new chiral solitary structures. J. Low Freq. Noise Vib. Act. Control. 2022, 42. [Google Scholar] [CrossRef]
- Javid, A.; Raza, N. Chiral solitons of the (1 + 2)-dimensional nonlinear Schrödinger’s equation. Mod. Phys. Lett. B 2019, 33, 1950401. [Google Scholar] [CrossRef]
- Eslami, M. Trial solution technique to chiral nonlinear Schrödinger’s equation in(1+2)-dimensions. Nonlinear Dyn. 2016, 85, 813–816. [Google Scholar] [CrossRef]
- Alomair, R.A.; Hassan, S.Z.; Abdelrahman, M.A.E. A new structure of solutions to the coupled nonlinear Maccari’s systems in plasma physics. AIMS Math. 2022, 7, 8588–8606. [Google Scholar] [CrossRef]
- Samadyar, N.; Ordokhani, Y.; Mirzaee, F. The couple of Hermite-based approach and Crank-Nicolson scheme to approximate the solution of two dimensional stochastic diffusion-wave equation of fractional order. Eng. Anal. Bound. Elem. 2020, 118, 285–294. [Google Scholar] [CrossRef]
- Almatrafi, M.B. Solitary wave solutions to a fractional model using the improved modified extended tanh-function method. Fractal Fract. 2023, 7, 252. [Google Scholar] [CrossRef]
- Mirzaee, F.; Samadyar, N. Numerical solution of time fractional stochastic Korteweg-de Vries equation via implicit meshless approach. Iran. J. Sci. Technol. Trans. A Sci. 2019, 43, 2905–2912. [Google Scholar] [CrossRef]
- Mirzaee, F.; Rezaei, S.; Samadyar, N. Numerical solution of two-dimensional stochastic time-fractional sine-Gordon equation on non-rectangular domains using finite difference and meshfree methods. Eng. Anal. Bound. Elem. 2021, 127, 53–63. [Google Scholar] [CrossRef]
- Almatrafi, M.B.; Alharbi, A. New soliton wave solutions to a nonlinear equation arising in plasma physics. Comput. Model. Eng. Sci. 2023, 137, 827–841. [Google Scholar] [CrossRef]
- Samadyar, N.; Ordokhani, Y.; Mirzaee, F. Hybrid Taylor and block-pulse functions operational matrix algorithm and its application to obtain the approximate solution of stochastic evolution equation driven by fractional Brownian motion. Commun. Nonlinear Sci. Numer. Simul. 2020, 90, 105346. [Google Scholar] [CrossRef]
- Dalfovo, F.; Giorgini, S.; Pitaevskii, L.P.; Stringari, S. Theory of Bose-Einstein condensation in trapped gases. Rev. Mod. Phys. 1999, 71, 463. [Google Scholar] [CrossRef]
- Nakkeeran, K. Bright and dark optical solitons in fiber media with higher-order effects. Chaos Solitons Fractals 2002, 13, 673–679. [Google Scholar] [CrossRef]
- Abdelrahman, M.A.E.; Sohaly, M.A.; Alharbi, Y.F. Fundamental stochastic solutions for the conformable fractional NLSE with spatiotemporal dispersion via exponential distribution. Phys. Scr. 2021, 96, 125223. [Google Scholar] [CrossRef]
- Mirzaee, F.; Sayevand, K.; Rezaei, S.; Samadyar, N. Finite difference and spline approximation for solving fractional stochastic advection-diffusion equation. Iran. J. Sci. Technol. A Sci. 2021, 45, 607–617. [Google Scholar] [CrossRef]
- Nishino, A.; Umeno, Y.; Wadati, M. Chiral nonlinear Schrödinger equation. Chaos Solitons Fractals 1998, 9, 1063–1069. [Google Scholar] [CrossRef]
- Tsitsas, N.L.; Lakhtakia, A.; Frantzeskakis, D.J. Vector solitons in nonlinear isotropic chiral metamaterials. J. Phys. A Math. Theor. 2011, 44, 435203. [Google Scholar] [CrossRef]
- Ismail, M.S.; Al-Basyouni, K.S.; Aydin, A. Conservative finite difference schemes for the chiral nonlinear Schrödinger equation. Bound. Value Probl. 2015, 89, 2015. [Google Scholar] [CrossRef]
- Younis, M.; Cheemaa, N.; Mahmood, S.A.; Rizvi, S.T.R. On optical solitons: The chiral nonlinear Schrödinger equation with perturbation and Bohm potential. Opt. Quant. Electron. 2016, 48, 542. [Google Scholar] [CrossRef]
- Yan, C. A simple transformation for nonlinear waves. Phys. Lett. A 1996, 224, 77–84. [Google Scholar] [CrossRef]
- Karatzas, I.; Shreve, S.E. Brownian Motion and Stochastic Calculus, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 1991. [Google Scholar]
- ∅ksendal, B. Stochastic Differential Equations: An Introduction with Applications, 6th ed.; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Griguolo, L.; Seminara, D. Chiral solitons from dimensional reduction of Chern-Simons gauged nonlinear Schrödinger equation: Classical and quantum aspects. Nucl. Phys. B 1998, 516, 467–498. [Google Scholar] [CrossRef]
- Lee, J.H.; Lin, C.K.; Pashev, O.K. Shock waves, chiral solitons and semi-classical limit of one-dimensional anyons. Chaos Solitons Fractals 2004, 19, 109–128. [Google Scholar] [CrossRef]
- Aglietti, U.; Griguolo, L.; Jackiw, R.; Pi, S.Y.; Semirara, D. Anyons and Chiral Solitons on a Line. Phys. Rev. Lett. 1996, 77, 44064409. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdelwahed, H.G.; Alsarhana, A.F.; El-Shewy, E.K.; Abdelrahman, M.A.E. Characteristics of New Stochastic Solitonic Solutions for the Chiral Type of Nonlinear Schrödinger Equation. Fractal Fract. 2023, 7, 461. https://doi.org/10.3390/fractalfract7060461
Abdelwahed HG, Alsarhana AF, El-Shewy EK, Abdelrahman MAE. Characteristics of New Stochastic Solitonic Solutions for the Chiral Type of Nonlinear Schrödinger Equation. Fractal and Fractional. 2023; 7(6):461. https://doi.org/10.3390/fractalfract7060461
Chicago/Turabian StyleAbdelwahed, H. G., A. F. Alsarhana, E. K. El-Shewy, and Mahmoud A. E. Abdelrahman. 2023. "Characteristics of New Stochastic Solitonic Solutions for the Chiral Type of Nonlinear Schrödinger Equation" Fractal and Fractional 7, no. 6: 461. https://doi.org/10.3390/fractalfract7060461
APA StyleAbdelwahed, H. G., Alsarhana, A. F., El-Shewy, E. K., & Abdelrahman, M. A. E. (2023). Characteristics of New Stochastic Solitonic Solutions for the Chiral Type of Nonlinear Schrödinger Equation. Fractal and Fractional, 7(6), 461. https://doi.org/10.3390/fractalfract7060461








