Applications of Fractional Differentiation Matrices in Solving Caputo Fractional Differential Equations
Abstract
1. Introduction
2. Jacobi Polynomials and Differentiation Matrix
3. Jacobi Fractional Differentiation Matrix
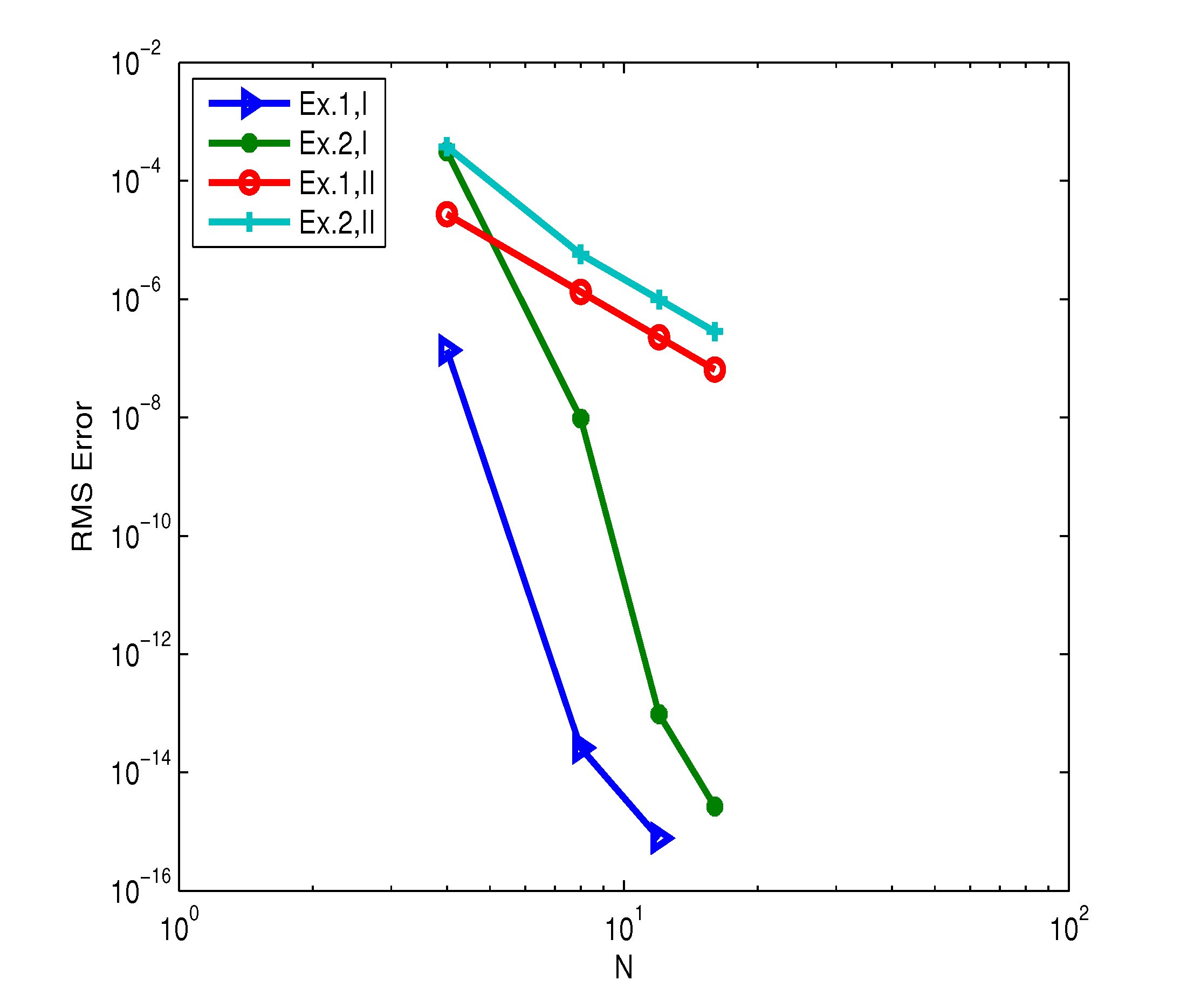
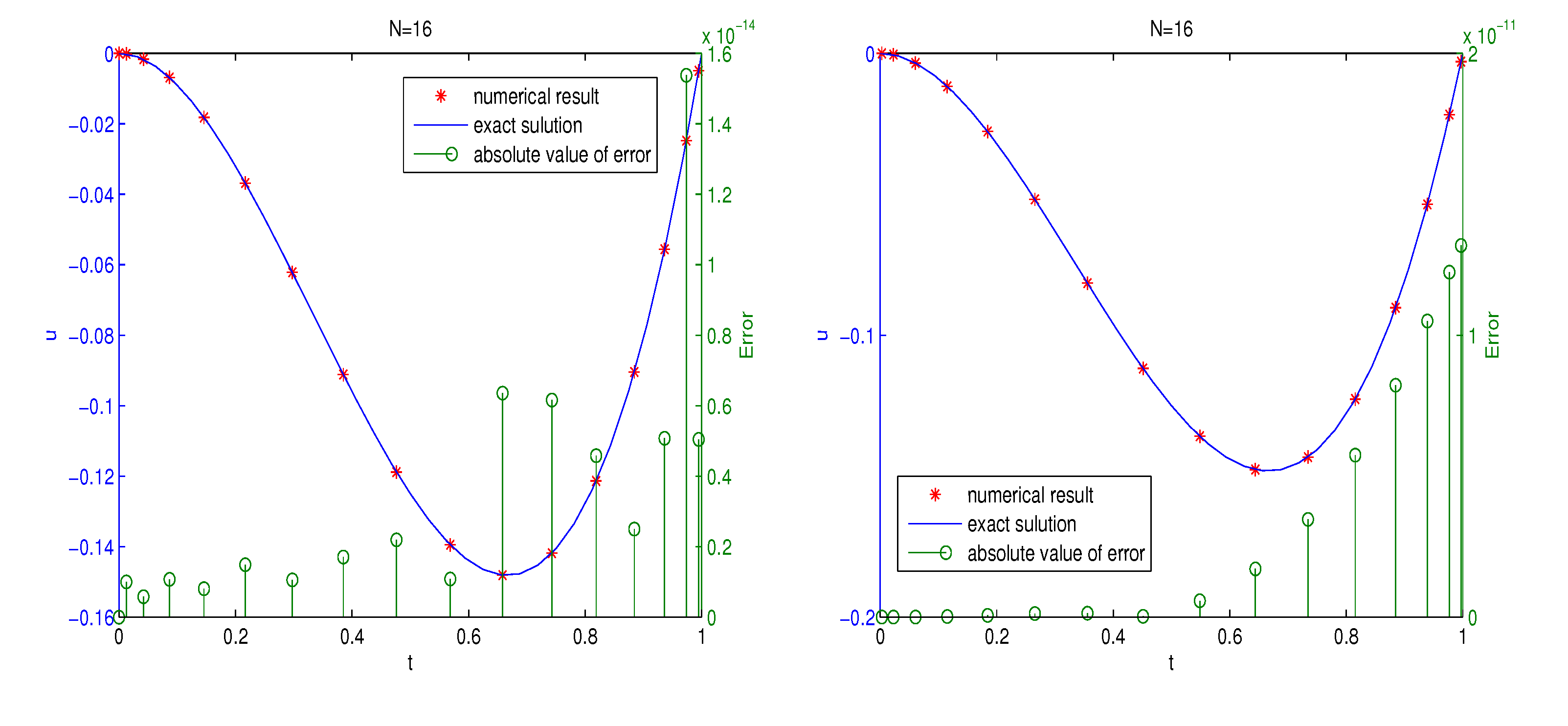
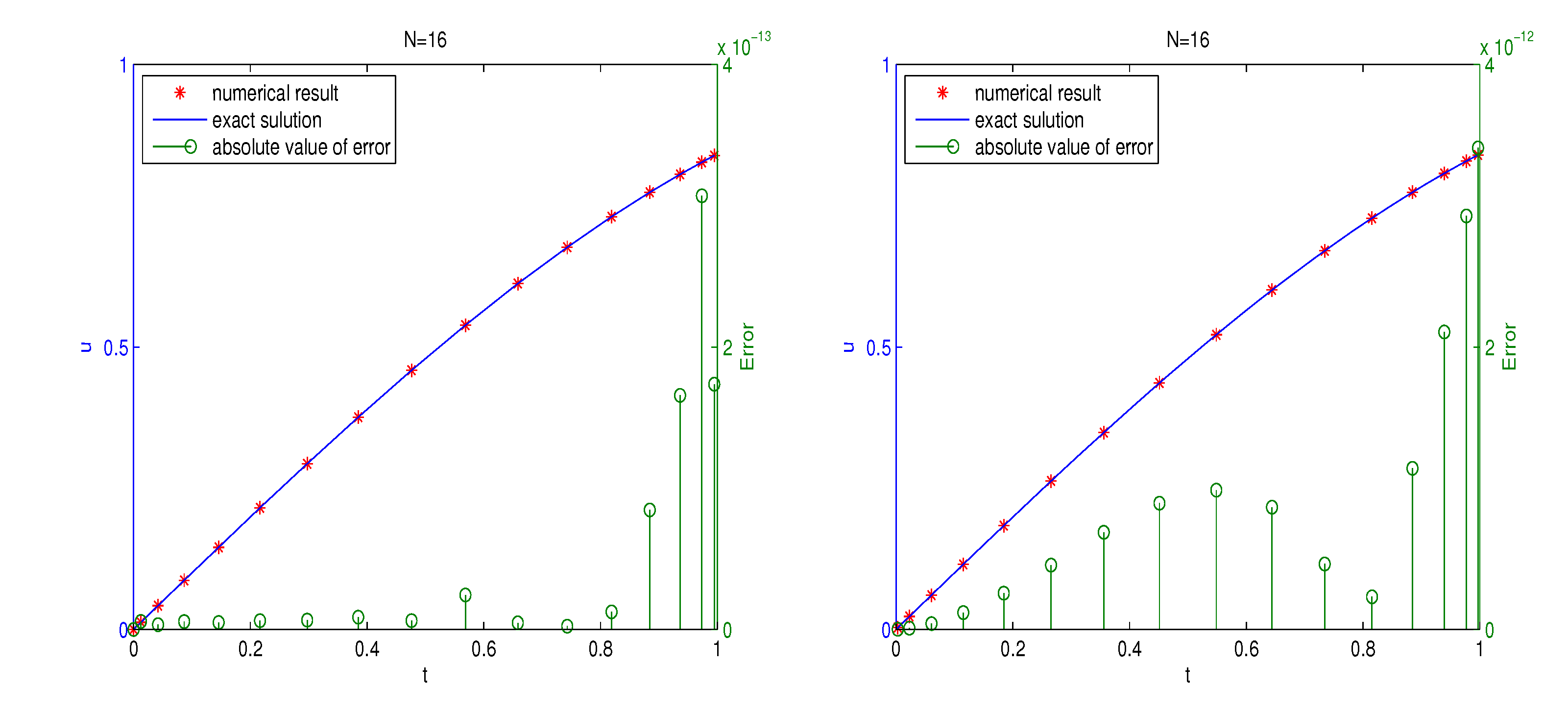
4. Algorithm and Numerical Examples for the Generalized Space–Fractional Burgers’ Equations
5. Algorithm and Numerical Examples for Initial Fractional Integro-Differential Equations
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| FDEs | fractional partial differential equations |
| JGL | Jacobi–Gauss–Lobatto |
| JGR | Jacobi–Gauss–Radau |
| CGL | Chebyshev–Gauss–Lobatto |
| CGR | Chebyshev–Gauss–Radau |
| LGL | Legendre–Gauss–Lobatto |
| LGR | Legendre–Gauss–Radau |
| RMS | root mean square |
| FIDE | fractional integro-differential equation |
References
- Podulubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Chester, W.N. Resonant ocillations in closed tubes. J. Fluid Mech. 1964, 18, 44–64. [Google Scholar] [CrossRef]
- Keller, J.J. Propagation of simple non-linear waves in gas filled tubes with friction. Z. Ang. Math. Phy. 1982, 32, 170–181. [Google Scholar] [CrossRef]
- Kakutanil, T.; Matsuuchi, K. Effect of viscosity on long gravity waves. J. Phys. Soc. Jpn. 1975, 39, 237–246. [Google Scholar] [CrossRef]
- Miksis, M.J.; Ting, L. Effective equations for multiphase flows-Waves in a bubbly liquid. Adv. Appl. Mech. 1991, 28, 141–260. [Google Scholar]
- Biler, P.; Funaki, T.; Woyczynski, W.A. Fractal Burgers equations. J. Diff. Equ. 1998, 148, 9–46. [Google Scholar] [CrossRef][Green Version]
- El-Shahed, M. Adomian decomposition method for solving Burgers equation with fractional derivative. J. Fract. Calc. 2003, 24, 23–28. [Google Scholar]
- Momani, S. Non-perturbative analytical solutions of the space- and time-fractional Burgers equations. Chaos Soliton. Fract. 2006, 28, 930–937. [Google Scholar] [CrossRef]
- Inc, M. The approximate and exact solutions of the space- and time-fractional Burgers equations with initial conditions by variational iteration method. J. Math. Anal. Appl. 2008, 345, 476–484. [Google Scholar] [CrossRef][Green Version]
- Esen, A.; Bulut, F.; Oruç, Ö. A unified approach for the numerical solution of time fractional Burgers’ type equations. Euro. Phys. J. Plus 2016, 131, 116. [Google Scholar] [CrossRef]
- Li, D.; Zhang, C.; Ran, M. A linear finite difference scheme for generalized time fractional Burgers equation. Appl. Math. Model. 2016, 40, 6069–6081. [Google Scholar] [CrossRef]
- Sugimoto, N. Burgers equation with a fractional derivative; hereditary effects on nonlinear acoustic waves. J. Fluid Mech. 1991, 225, 631–653. [Google Scholar] [CrossRef]
- Khatera, A.H.; Temsaha, R.S.; Hassanb, M.M. A Chebyshev spectral collocation method for solving Burgers’-type equations. J. Comput. Appl. Math. 2008, 222, 333–350. [Google Scholar] [CrossRef][Green Version]
- Hwang, C.; Shih, Y.-P. Laguerre operational matrices for fractional calculus and applications. Int. J. Control 1981, 34, 577–584. [Google Scholar] [CrossRef]
- Parand, K.; Dehghan, M.; Taghavi, A. Modified generalized Laguerre function Tau method for solving laminar viscous flow: The Blasius equation. Int. J. Numer. Method Heat 2010, 20, 728–743. [Google Scholar] [CrossRef]
- Saadatmandi, A.; Dehghan, M. A new operational matrix for solving fractional-order differential equations. Comput. Math. Appl. 2010, 59, 1326–1336. [Google Scholar] [CrossRef][Green Version]
- Maleknejad, K.; Hashemizadeh, E.; Basirat, B. Computational method based on Bernstein operational matrices for nonlinear Volterra–Fredholm–Hammerstein integral equations. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 52–61. [Google Scholar] [CrossRef]
- Bhrawy, A.H.; Alofi, A.S. The operational matrix of fractional integration for shifted Chebyshev polynomials. Appl. Math. Lett. 2013, 26, 25–31. [Google Scholar] [CrossRef][Green Version]
- Jafari, H.; Nemati, S.; Ganji, R.M. Operational matrices based on the shifted fifth-kind Chebyshev polynomials for solving nonlinear variable order integro-differential equations. Adv. Differ. Equ. 2021, 2021, 435. [Google Scholar] [CrossRef]
- Doha, E.H.; Bhrawy, A.H.; Ezz-Eldien, S.S. A new Jacobi operational matrix: An application for solving fractional differential equations. Appl. Math. Model. 2012, 36, 4931–4943. [Google Scholar] [CrossRef]
- Wu, Q.; Zeng, X. Jacobi Collocation Methods for Solving Generalized Space-Fractional Burgers’ Equations. Commun. Appl. Math. Comput. 2020, 36, 4931–4943. [Google Scholar] [CrossRef][Green Version]
- Sadeghi, S.; Jafari, H.; Nemati, S. Operational matrix for Atangana–Baleanu derivative based on Genocchi polynomials for solving FDEs. Chaos Solit. 2020, 135, 106973. [Google Scholar] [CrossRef]
- Zayernouri, M.; Karniadakis, G.E. Fractional spectral collocation method. SIAM J. Sci. Comput. 2014, 36, A40–A62. [Google Scholar] [CrossRef]
- Bhrawy, A.H.; Taha, M.T.; Machado, J.A.T. A review of operational matrices and spectral techniques for fractional calculus. Nonlinear Dyn. 2015, 81, 1023–1052. [Google Scholar] [CrossRef]
- Yang, Y.B.; Ma, H.P. The Legendre Galerkin-Chebyshev Collocation method for generalized space–fractional Burgers equations. J. Numer. Meth. Comp. Appl. 2017, 38, 236–244. [Google Scholar]
- Afsane, S.; Mahmoud, B. Application Jacobi spectral method for solving the time-fractional differential equation. J. Comput. Appl. Math. 2018, 399, 49–68. [Google Scholar]
- Guo, B.Y. Spectral Methods and Their Applications; World Scientific: Singapore, 1998. [Google Scholar]
- Guo, B.Y.; Wang, L.L. Jacobi interpolation approximations and their applications to singular differential equations. Adv. Comput. Math. 2001, 14, 227–276. [Google Scholar]
- Ma, H.P.; Sun, W.W. Optimal Error Estimates of the Legendre-Petrov-Galerkin Method for the Korteweg-de Vries Equation. SIAM J. Numer. Anal. 2001, 39, 1380–1394. [Google Scholar] [CrossRef]
- Wu, H.; Ma, H.P.; Li, H.Y. Optimal error estimates of the Chebyshev-Legendre method for solving the generalized Burgers equation. SIAM J. Numer. Anal. 2003, 41, 659–672. [Google Scholar] [CrossRef]
- Guo, B.Y.; Wang, L.L. Jacobi approximations in non-uniformly Jacobi-weighted Sobolev spaces. J. Approx. Theory 2004, 128, 1–41. [Google Scholar] [CrossRef][Green Version]
- Shen, J.; Tang, T. Spectral and High-Order Methods with Applications; Science Press: Beijing, China, 2006. [Google Scholar]
- Canuto, C.G.; Hussaini, M.Y.; Quarteroni, A.; Zang, T.A. Spectral Methods: Fundamentals in Single Domains; Springer: New York, NY, USA, 2010. [Google Scholar]
- Shen, J.; Tang, T.; Wang, L.L. Spectral Methods Algorithms, Analysis and Applications; Springer Series in Computational Mathematics: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Zhao, T.G.; Wu, Y.J.; Ma, H.P. Error Analysis of Chebyshev-Legendre Pseudo-spectral Method for a Class of Nonclassical Parabolic Equation. SIAM J. Sci. Comput. 2012, 52, 588–602. [Google Scholar] [CrossRef]
- Mockary, S.; Babolian, E.; Vahidi, A.R. A fast numerical method for fractional partial differential equations. Adv. Differ. Equ. 2019, 2019, 452. [Google Scholar] [CrossRef][Green Version]
- Wu, C.; Wang, Z. The spectral collocation method for solving a fractional integro-differential equation. AIMS Math. 2022, 7, 9577–9587. [Google Scholar] [CrossRef]
- Doha, E.H.; Abdelkawy, M.A.; Amin, A.Z.M.; Lopes, A.M. Shifted Jacobi–Gauss-collocation with convergence analysis for fractional integro-differential equations. Commun. Nonlinear Sci. Numer. Simul. 2019, 72, 342–359. [Google Scholar] [CrossRef]
- Wu, Q.; Wu, Z.; Zeng, X. A Jacobi Spectral Collocation Method for Solving Fractional Integro-Differential Equations. Commun. Appl. Math. Comput. 2021, 3, 509–526. [Google Scholar] [CrossRef]
| N | ||||
|---|---|---|---|---|
| 4 | 6.0362 × 10 | 5.9249 × 10 | 6.2110 × 10 | |
| 8 | 3.4641 × 10 | 3.4508 × 10 | 3.5460 × 10 | |
| 12 | 1.0282 × 10 | 9.6164 × 10 | 9.4303 × 10 | |
| 4 | 5.5072 × 10 | 1.3705 × 10 | 6.5986 × 10 | |
| 8 | 1.8781 × 10 | 2.6166 × 10 | 4.2505 × 10 | |
| 12 | 7.9866 × 10 | 7.7894 × 10 | 7.9360 × 10 | |
| 4 | 2.5969 × 10 | 3.8212 × 10 | 5.6797 × 10 | |
| 8 | 1.7825 × 10 | 2.2046 × 10 | 3.1671 × 10 | |
| 12 | 8.8606 × 10 | 1.1035 × 10 | 1.5604 × 10 |
| N | ||||
|---|---|---|---|---|
| 4 | 8.8219 × 10 | 8.9389 × 10 | 9.2472 × 10 | |
| 8 | 1.8921 × 10 | 1.8993 × 10 | 1.9393 × 10 | |
| 12 | 1.7631 × 10 | 1.7707 × 10 | 1.8036 × 10 | |
| 16 | 2.9591 × 10 | 3.6478 × 10 | 5.5501 × 10 | |
| 4 | 3.1279 × 10 | 3.1274 × 10 | 3.2005 × 10 | |
| 8 | 9.6568 × 10 | 9.6444 × 10 | 9.7385 × 10 | |
| 12 | 9.6773 × 10 | 9.6694 × 10 | 9.7314 × 10 | |
| 16 | 2.4675 × 10 | 2.6880 × 10 | 3.4963 × 10 | |
| 4 | 4.0505 × 10 | 4.9560 × 10 | 6.1909 × 10 | |
| 8 | 1.2005 × 10 | 1.3271 × 10 | 1.4142 × 10 | |
| 12 | 1.2032 × 10 | 1.2645 × 10 | 1.3179 × 10 | |
| 16 | 2.5721 × 10 | 4.3107 × 10 | 1.8257 × 10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, Z.; Zhang, X.; Wang, J.; Zeng, X. Applications of Fractional Differentiation Matrices in Solving Caputo Fractional Differential Equations. Fractal Fract. 2023, 7, 374. https://doi.org/10.3390/fractalfract7050374
Wu Z, Zhang X, Wang J, Zeng X. Applications of Fractional Differentiation Matrices in Solving Caputo Fractional Differential Equations. Fractal and Fractional. 2023; 7(5):374. https://doi.org/10.3390/fractalfract7050374
Chicago/Turabian StyleWu, Zhongshu, Xinxia Zhang, Jihan Wang, and Xiaoyan Zeng. 2023. "Applications of Fractional Differentiation Matrices in Solving Caputo Fractional Differential Equations" Fractal and Fractional 7, no. 5: 374. https://doi.org/10.3390/fractalfract7050374
APA StyleWu, Z., Zhang, X., Wang, J., & Zeng, X. (2023). Applications of Fractional Differentiation Matrices in Solving Caputo Fractional Differential Equations. Fractal and Fractional, 7(5), 374. https://doi.org/10.3390/fractalfract7050374






