Studying and Simulating the Fractional COVID-19 Model Using an Efficient Spectral Collocation Approach
Abstract
1. Introduction
- 1.
- Numerical programs in the suggested technique for managing the study’s model quickly produce Chebyshev coefficients for the solution;
- 2.
- The suggested approach using these polynomials is quicker than the alternatives. Moreover, these polynomials are widely employed and have a wide range of applications due to their favorable function-approximation characteristics;
- 3.
- The suggested approach using these polynomials is an easy-to-use numerical technique with finite and infinite domains for a variety of problems with excellent accuracy and exponential rates of convergence.
2. Preliminaries and Notations
2.1. Some Definitions of Fractional Derivatives
2.2. Shifted Chebyshev Polynomial Approximation
3. The Formulation and Qualitative Analysis of the Model
3.1. Region of Invariance
3.2. Disease-Free Equilibrium Point
3.3. Analysis of the Existence and Stability of an Endemic Equilibrium Point
4. Solution Procedure
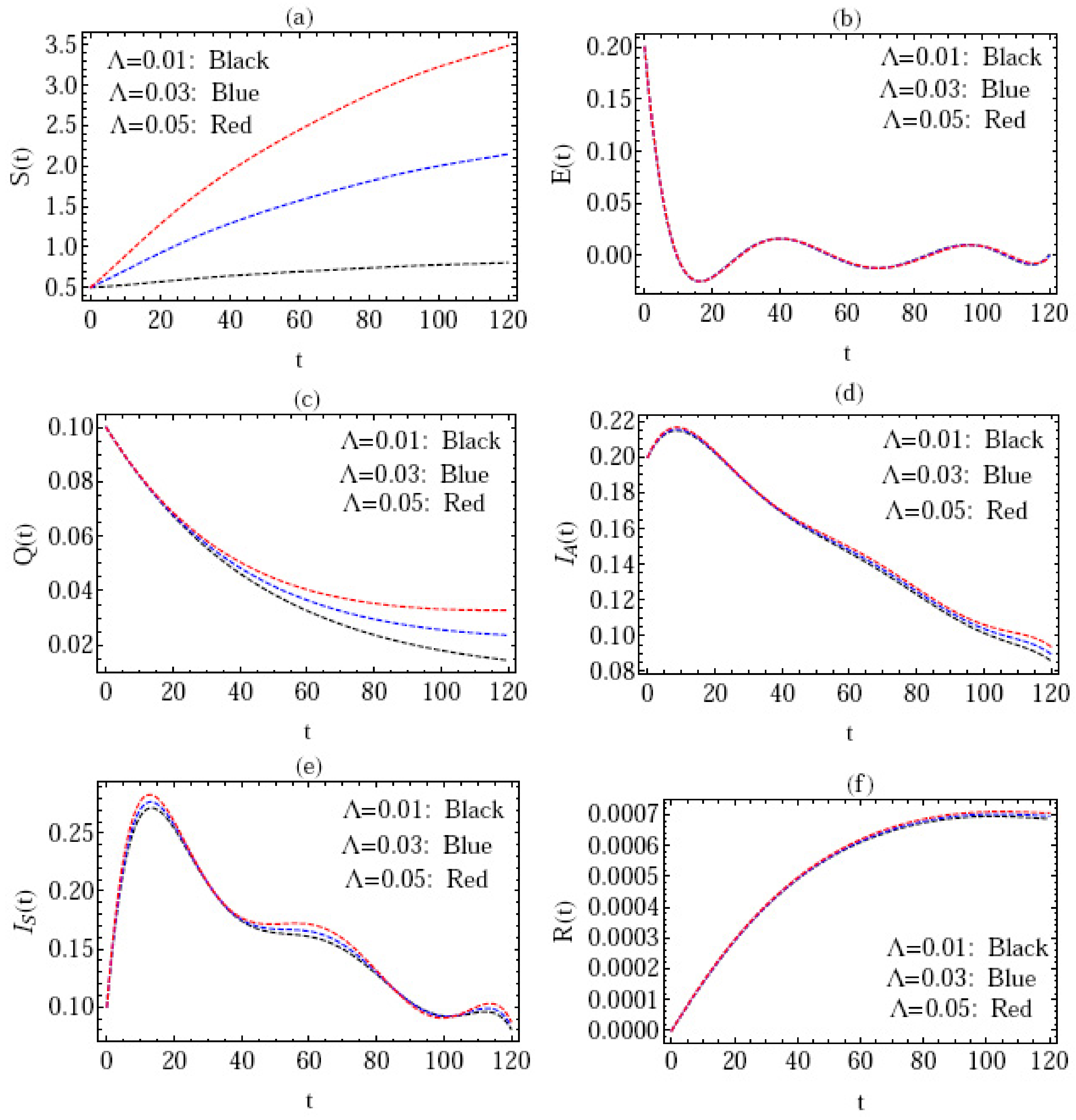
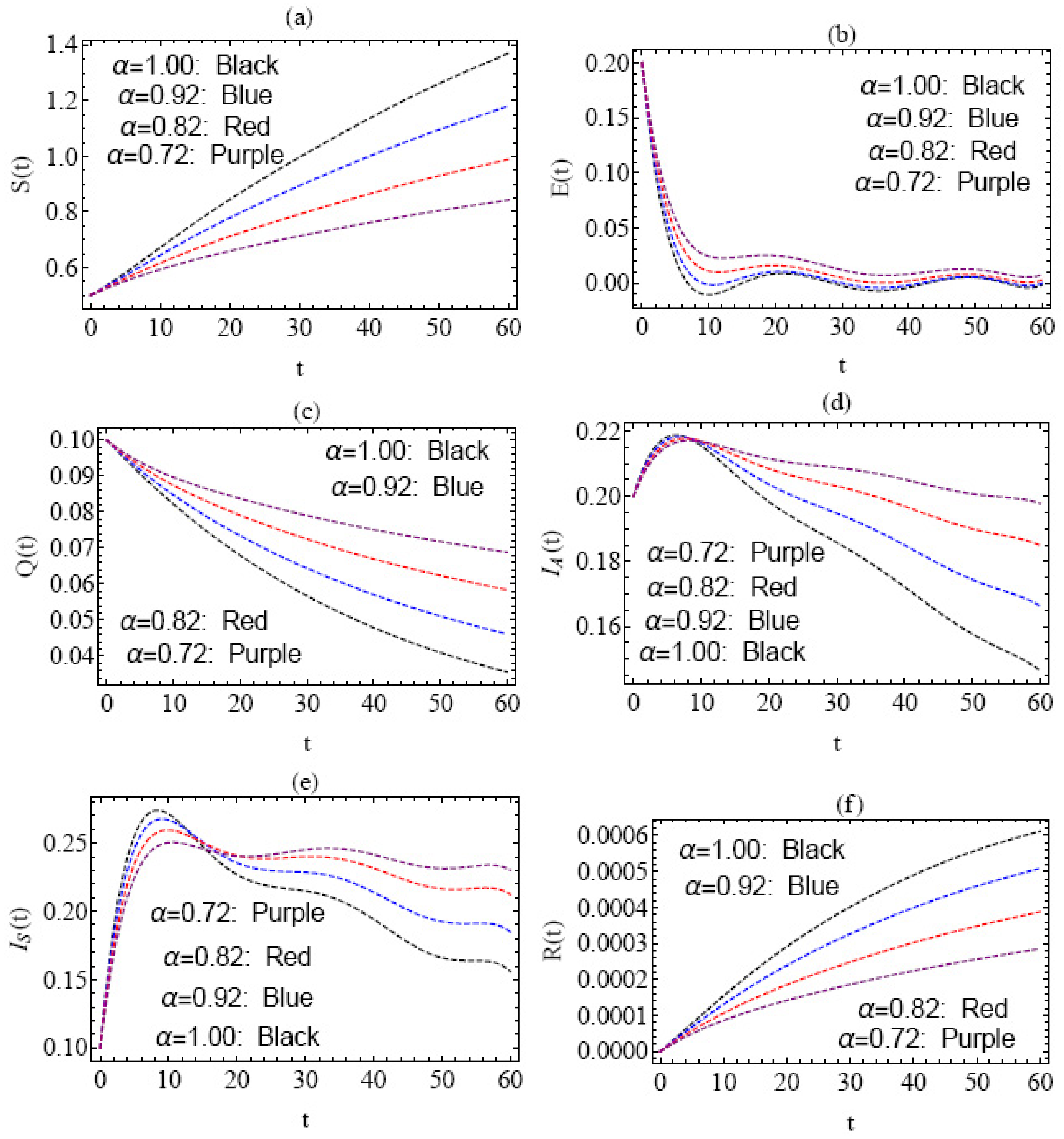
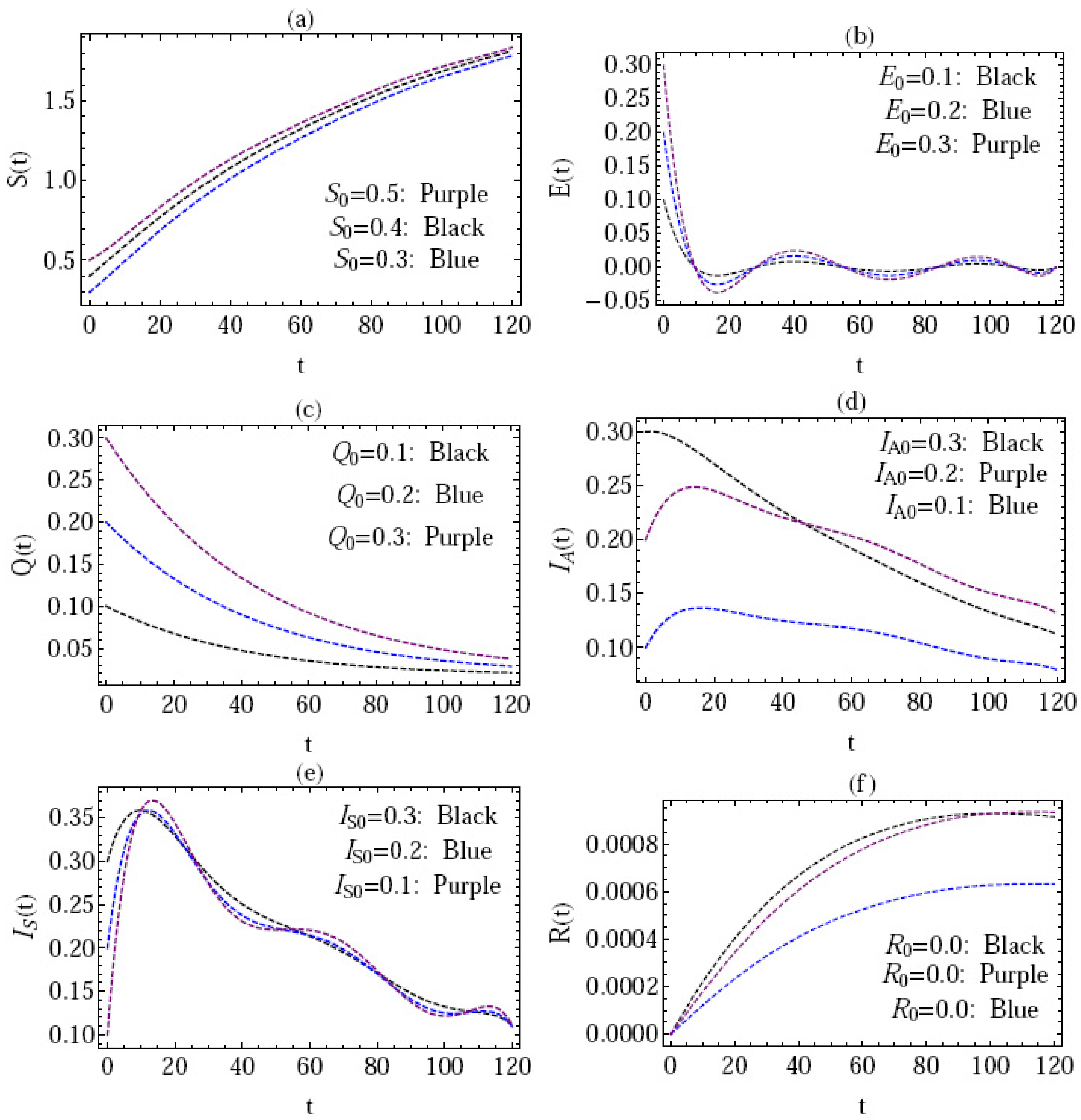
5. Numerical Simulation
- i.
- ;
- ii.
- ;
- iii.
- .
6. Conclusions and Remarks
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Anderson, R.M.; May, R.M. Helminth infections of humans: Mathematical models, population dynamics, and control. Adv. Parasitol. 1985, 24, 1–101. [Google Scholar]
- Edelstein, K.L. Mathematical Models in Biology; SIAM: Philadelphia, PA, USA, 2005. [Google Scholar]
- Khader, M.M.; Sweilam, N.H.; Mahdy, A.M.S.; Moniem, N.K.A. Numerical simulation for the fractional SIRC model and influenxa A. Appl. Math. Inf. Sci. 2014, 8, 1029–1036. [Google Scholar] [CrossRef]
- World Health Organization. Novel Coronavirus Diseases 2019. Available online: https://www.who.int/emergencies/diseases/novel-coronavirus-2019 (accessed on 14 October 2020).
- Ajisegiri, W.; Odusanya, O.; Joshi, R. COVID-19 outbreak situation in Nigeria and the need for effective engagement of community health workers for epidemic response. Glob. Biosecur. 2020, 1, 5–20. [Google Scholar] [CrossRef]
- Ahmad, S.; Ullah, A.; Al-Mdallal, Q.M.; Khan, H.; Shah, K.; Khan, A. Fractional order mathematical modeling of COVID-19 transmission. Chaos Solitons Fractals 2020, 139, 110256. [Google Scholar] [CrossRef]
- Baud, D.; Qi, X.; Nielsen-Saine, K.; Musso, D.; Pomar, L.; Favre, G. Real estimates of mortality following covid-19 infection. Lancet Infect. Dis. 2020, 11, 13–23. [Google Scholar] [CrossRef]
- Koo, J.R.; Cook, A.R.; Park, M.; Sun, Y.; Sun, H.; Lim, J.T.; Tam, C.; Dickens, B.L. Interventions to mitigate early spread of sarscov-2 in singapore: A modelling study. Lancet Infect. Dis. 2020, 15, 15–25. [Google Scholar]
- Adegboye, O.A.; Adekunle, A.I.; Gayawan, E. Early transmission dynamics of novel coronavirus (covid-19) in Nigeria. Int. J. Environ. Res. Public Health 2020, 17, 10–25. [Google Scholar] [CrossRef] [PubMed]
- Cao, Y.; Nikan, O.; Avazzadeh, Z. A localized meshless technique for solving 2D nonlinear integro-differential equation with multi-term kernels. Appl. Numer. Math. 2023, 183, 140–156. [Google Scholar] [CrossRef]
- Kalimbetov, B.; Abylkasymova, E.; Beissenova, G. On the asymptotic solutions of singulary perturbed differential systems of fractional order. J. Math. Comput. Sci. 2022, 24, 165–172. [Google Scholar] [CrossRef]
- Asjad, M.I.; Ullah, N.; Rehman, H.U.; Baleanu, D. Optical solitons for conformable space-time fractional nonlinear model. J. Math. Comput. Sci. 2022, 27, 28–41. [Google Scholar] [CrossRef]
- Xie, J.; Yan, X.; Ali, M.A.; Hammouch, Z. A linear decoupled physical-property-preserving difference method for fractional-order generalized Zakharov system. J. Comput. Appl. Math. 2023, 426, 115044. [Google Scholar] [CrossRef]
- Danane, J.; Allali, K.; Hammouch, Z. Mathematical analysis of a fractional differential model of HBV infection with antibody immune response. Chaos Solitons Fractals 2020, 136, 109787. [Google Scholar] [CrossRef]
- Hajji, M.A.; Al-Mdallal, Q. Numerical simulations of a delay model for immune system-tumor interaction. Sultan Qaboos Univ. J. Sci. 2018, 23, 19–31. [Google Scholar] [CrossRef]
- Abd-Elhameed, W.M. New Galerkin operational matrix of derivatives for solving Lane-Emden singular-type equations. Eur. Phys. J. Plus 2015, 130, 52. [Google Scholar] [CrossRef]
- Khader, M.M.; Adel, M. Modeling and numerical simulation for covering the fractional COVID-19 model using spectral collocation-optimization algorithms. Fractal Fract. 2022, 6, 363. [Google Scholar] [CrossRef]
- Sweilam, N.H.; Khader, M.M.; Adel, M. On the fundamental equations for modeling neuronal dynamics. J. Adv. Res. 2014, 5, 253–259. [Google Scholar] [CrossRef]
- Adel, M.; Srivastava, H.M.; Khader, M.M. Implementation of an accurate method for the analysis and simulation of electrical R-L circuits. Math. Methods Appl. Sci. 2022, 12, 8062. [Google Scholar] [CrossRef]
- Kolwankar, K.M.; Gangal, A.D. Fractional differentiability of nowhere differentiable functions and dimensions. Chaos Interdiscip. J. Nonlinear Sci. 1996, 6, 505–513. [Google Scholar] [CrossRef]
- Atta, A.G.; Youssri, Y.H. Advanced shifted first-kind Chebyshev collocation approach for solving the nonlinear time-fractional partial integro-differential equation with a weakly singular kernel. Comput. Appl. Math. 2022, 6, 381. [Google Scholar] [CrossRef]
- Abd-Elhameed, W.M. Novel expressions for the derivatives of sixth kind chebyshev polynomials: Spectral solution of the non-linear one-dimensional burgers’ equation. Fractal Fract. 2021, 5, 53. [Google Scholar] [CrossRef]
- Youssri, Y.H.; Atta, A.G. Spectral collocation approach via normalized shifted Jacobi polynomials for the nonlinear Lane-Emden equation with fractal-fractional derivative. Fractal Fract. 2023, 7, 133. [Google Scholar] [CrossRef]
- Khader, M.M.; Saad, K.M. A numerical study using Chebyshev collocation method for a problem of biological invasion: Fractional Fisher equation. Int. J. Biomath. 2018, 11, 1850099. [Google Scholar] [CrossRef]
- Atta, A.G.; Abd-Elhameed, W.M.; Moatimid, G.M.; Youssri, Y.H. Shifted fifth-kind Chebyshev Galerkin treatment for linear hyperbolic first-order partial differential equations. Appl. Numer. Math. 2021, 167, 237–256. [Google Scholar] [CrossRef]
- Saad, K.M.; Khader, M.M.; Gomez-Aguilar, J.F.; Baleanu, D. Numerical solutions of the fractional Fisher’s type equations with Atangana-Baleanu fractional derivative by using spectral collocation methods. Chaos 2019, 29, 023116. [Google Scholar] [CrossRef] [PubMed]
- Kumar, S.; Gomez-Aguilar, J.F.; Lavin-Delgado, J.E.; Baleanu, D. Derivation of operational matrix of Rabotnov fractional-exponential kernel and its application to fractional Lienard equation. Alex. Eng. J. 2020, 59, 991–2997. [Google Scholar] [CrossRef]
- Snyder, M.A. Chebyshev Methods in Numerical Approximation; Prentice-Hall, Inc.: Englewood Cliffs, NJ, USA, 1966. [Google Scholar]
- Mason, J.C.; Handscomb, D.C. Chebyshev Polynomials; Chapman and Hall: New York, NY, USA; CRC: Boca Raton, FL, USA, 2003. [Google Scholar]
- Handan, C.Y. Numerical solution of fractional Riccati differential equation via shifted Chebyshev polynomials of the third kind. J. Eng. Technol. Appl. Sci. 2017, 28, 1–11. [Google Scholar]
- Ahmed, I.; Modu, G.U.; Yusuf, A.; Kumam, P.; Yusuf, I. A mathematical model of coronavirus disease (COVID-19) containing asymptomatic and symptomatic classes. Results Phys. 2021, 21, 103776. [Google Scholar] [CrossRef]
- Diekmann, O.; Heesterbeek, J.A.P.; Metz, J.A. On the definition and the computation of the basic reproduction ratio R0 in models for infectious diseases in heterogeneous populations. J. Math. Biol. 1990, 28, 365–382. [Google Scholar] [CrossRef]
- El-Hawary, H.M.; Salim, M.S.; Hussien, H.S. Ultraspherical integral method for optimal control problems governed by ordinary differential equations. J. Glob. Optim. 2003, 25, 283–303. [Google Scholar] [CrossRef]
- Parand, K.; Delkhosh, M. Operational matrices to solve nonlinear Volterra-Fredholm integro-differential equations of multi-arbitrary order. Gazi Univ. J. Sci. 2016, 29, 895–907. [Google Scholar]
- Ibrahim, Y.F.; Khader, M.M.; Megahed, A.; Abd Elsalam, F.; Adel, M. An efficient numerical simulation for the fractional COVID-19 model by using the GRK4M together and the fractional FDM. Fractal Fract. 2022, 6, 304. [Google Scholar] [CrossRef]

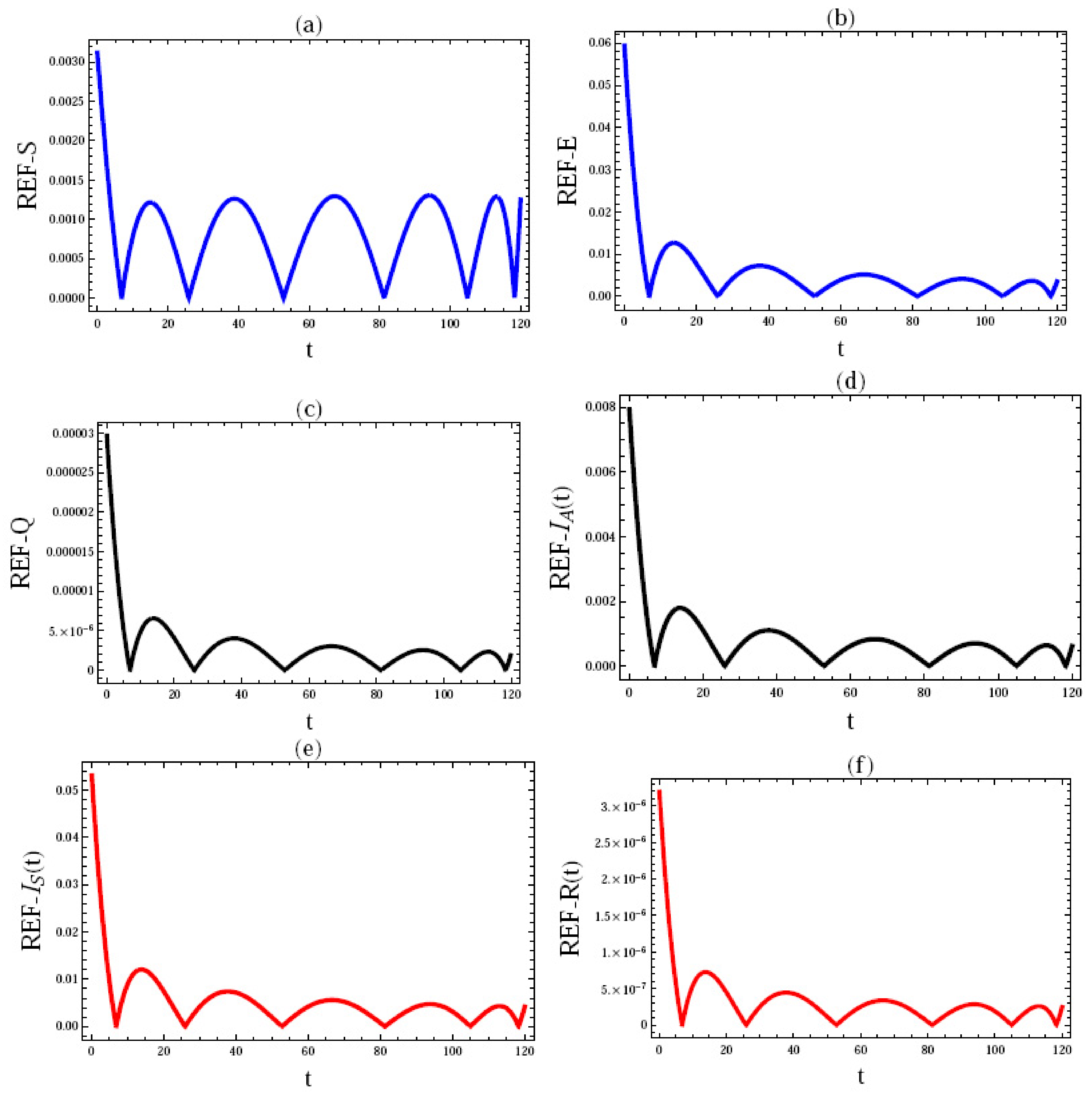

| N | h | Present Method | GRK4 Method | |
|---|---|---|---|---|
| 1.0 | 8 | 0.005 | 25 s | 24 s |
| 0.001 | 30 s | 28 s | ||
| 10 | 0.005 | 28 s | 26 s | |
| 0.001 | 35 s | 34 s | ||
| 0.95 | 8 | 0.005 | 27 s | 26 s |
| 0.001 | 32 s | 30 s | ||
| 10 | 0.005 | 30 s | 29 s | |
| 0.001 | 37 s | 35 s | ||
| 0.90 | 8 | 0.005 | 28 s | 27 s |
| 0.001 | 33 s | 31 s | ||
| 10 | 0.005 | 31 s | 30 s | |
| 0.001 | 38 s | 36 s |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ibrahim, Y.F.; Abd El-Bar, S.E.; Khader, M.M.; Adel, M. Studying and Simulating the Fractional COVID-19 Model Using an Efficient Spectral Collocation Approach. Fractal Fract. 2023, 7, 307. https://doi.org/10.3390/fractalfract7040307
Ibrahim YF, Abd El-Bar SE, Khader MM, Adel M. Studying and Simulating the Fractional COVID-19 Model Using an Efficient Spectral Collocation Approach. Fractal and Fractional. 2023; 7(4):307. https://doi.org/10.3390/fractalfract7040307
Chicago/Turabian StyleIbrahim, Yasser F., Sobhi E. Abd El-Bar, Mohamed M. Khader, and Mohamed Adel. 2023. "Studying and Simulating the Fractional COVID-19 Model Using an Efficient Spectral Collocation Approach" Fractal and Fractional 7, no. 4: 307. https://doi.org/10.3390/fractalfract7040307
APA StyleIbrahim, Y. F., Abd El-Bar, S. E., Khader, M. M., & Adel, M. (2023). Studying and Simulating the Fractional COVID-19 Model Using an Efficient Spectral Collocation Approach. Fractal and Fractional, 7(4), 307. https://doi.org/10.3390/fractalfract7040307







