Semi-Analytical Solutions for Some Types of Nonlinear Fractional-Order Differential Equations Based on Third-Kind Chebyshev Polynomials
Abstract
:1. Introduction
2. Important Preliminaries
2.1. Derivatives in Fractions
2.2. Third-Kind Chebyshev Polynomials: Definition and Properties
3. Operational Matrices of Derivatives for Third-Kind Chebyshev Polynomials
3.1. Third-Kind Chebyshev Polynomials: Operational Matrix of Integer-Order Derivatives IC3OM
3.2. Third-Kind Chebyshev Polynomials: Operational Matrix of Fractional-Order Derivatives FC3OM
4. Nonlinear Multi-Term Fractional-Order Differential Equations (NMFDEs)
4.1. Initial-Value Problem (INMFDEs)
4.2. Boundary Value Problem (BNMFDEs)
5. Error Bound
6. Numerical Applications
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; Elsevier: San Diego, CA, USA, 2006. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; Academic Press: New York, NY, USA, 1999. [Google Scholar]
- Baleanu, D.; Balas, V.E.; Agarwal, P. Fractional Order Systems and Applications in Engineering; Elsevier: San Diego, CA, USA; Academic Press: New York, NY, USA, 2023. [Google Scholar]
- Piotrowska, E.; Rogowski, K. Time-Domain analysis of fractional electrical circuit containing two ladder elements. Electronics 2021, 10, 475. [Google Scholar] [CrossRef]
- Khazayinejad, M.; Nourazar, S.S. Space-fractional heat transfer analysis of hybrid nanofluid along a permeable plate considering inclined magnetic field. Sci. Rep. 2022, 12, 5220. [Google Scholar] [CrossRef]
- Rabah, F.; Abukhaled, M.; Khuri, S.A. Solution of a complex nonlinear fractional biochemical reaction model. Math. Comput. Appl. 2022, 27, 45. [Google Scholar] [CrossRef]
- Ionescu, C.; Lopes, A.; Copot, D.; Machado, J.A.T.; Bates, J.H.T. The role of fractional calculus in modeling biological phenomena: A review. Commun. Nonlinear Sci. Numer. Simul. 2017, 51, 141–159. [Google Scholar] [CrossRef]
- Agarwal, P.; El-Sayed, A.A. Vieta-Lucas polynomials for solving a fractional-order mathematical physics model. Adv. Differ. Equ. 2020, 2020, 626. [Google Scholar] [CrossRef]
- Arora, S.; Mathur, T.; Agarwal, S.; Tiwari, K.; Gupta, P. Applications of fractional calculus in computer vision: A survey. Neurocomputing 2022, 489, 407–428. [Google Scholar] [CrossRef]
- Nagy, A.M.; El-Sayed, A.A. An accurate numerical technique for solving two-dimensional time fractional order diffusion equation. Int. J. Model Simul. 2019, 39, 214–221. [Google Scholar] [CrossRef]
- El-Sayed, A.A.; Agarwal, P. Spectral treatment for the fractional-order wave equation using shifted Chebyshev orthogonal polynomials. J. Comput. Appl. Math. 2023, 424, 114933. [Google Scholar] [CrossRef]
- Sweilam, N.H.; El-Sayed, A.A.; Boulaaras, S. Fractional-order advection-dispersion problem solution via the spectral collocation method and the non-standard finite difference technique. Chaos Solitons Fractals 2023, 144, 110736. [Google Scholar] [CrossRef]
- Mokhtary, P.; Ghoreishi, F.; Srivastavac, H.M. The Müntz-Legendre Tau method for fractional differential equations. Math. Comput. Simul. 2022, 194, 210–235. [Google Scholar] [CrossRef]
- Nedaiasl, K.; Dehbozorgi, R. Galerkin finite element method for nonlinear fractional differential equations. Numer. Algor. 2021, 88, 113–141. [Google Scholar] [CrossRef]
- Youssri, Y.H.; Abd-Elhameed, W.M.; Ahmed, H.M. New fractional derivative expression of the shifted third-kind Chebyshev polynomials: Application to a type of nonlinear fractional Pantograph differential equations. J. Funct. Spaces 2022, 2022, 3966135. [Google Scholar] [CrossRef]
- Arfan, M.; Khan, Z.A.; Zeb, A.; Shah, K. Study of numerical solution to some fractional order differential equation using Hermite polynomials. Int. J. Appl. Comput. Math. 2022, 8, 60. [Google Scholar] [CrossRef]
- El-Sayed, A.A.; Boulaaras, S.; Sweilam, N.H. Numerical solution of the fractional-order logistic equation via the first-kind Dickson polynomials and spectral tau method. Math. Methods Appl. Sci. 2023, 46, 8004–8017. [Google Scholar] [CrossRef]
- Agarwal, P.; El-Sayed, A.A.; Tariboong, J. Vieta-Fibonacci operational matrices for spectral solutions of variable-order fractional integro-differential equations. J. Comput. Appl. Math. 2021, 382, 113063. [Google Scholar] [CrossRef]
- Ghafoori, S.; Motevalli, M.; Nejad, M.G.; Shakeri, F.; Ganji, D.D.; Jalaal, M. Efficiency of differential transformation method for nonlinear oscillation: Comparison with HPM and VIM. Curr. Appl. Phys. 2011, 11, 1567–1739. [Google Scholar] [CrossRef]
- Baleanu, D.; Golmankhaneh, A.K.; Golmankhaneh, A.K.; Nigmatullin, R.R. Newtonian law with memory. Nonlinear Dyn. 2010, 60, 81–86. [Google Scholar] [CrossRef]
- Hilfer, R. Application of Fractional Calculus in Physics; World Scientific: Singapore, 2000. [Google Scholar]
- Hilfer, R. Mathematical and physical interpretations of fractional derivatives and integrals. In Handbook of Fractional Calculus with Applications, Volume1: Basic Theory; Kochubei, A., Luchko, Y., Eds.; De Gruyter: Berlin, Germany, 2019; pp. 47–85. [Google Scholar]
- Han, W.; Chen, Y.M.; Liu, D.Y.; Li, X.L.; Boutat, D. Numerical solution for a class of multi-order fractional differential equations with error correction and convergence analysis. Adv. Differ. Equ. 2018, 2018, 253. [Google Scholar] [CrossRef]
- Avci, I.; Mahmudov, N.I. Numerical solutions for multi-term fractional order differential equations with fractional Taylor operational matrix of fractional integration. Mathematics 2020, 8, 96. [Google Scholar] [CrossRef]
- Doha, E.H.; Bhrawy, A.H.; Ezz-Eldien, S.S. Efficient Chebyshev spectral methods for solving multi-term fractional orders differential equations. Appl. Math. Model. 2011, 35, 5662–5672. [Google Scholar] [CrossRef]
- Youssri, Y.H.; Abd-Elhameed, W.M. pectral solutions for multi-term fractional initial value problems using a new fibonacci operational matrix of fractional integration. Progr. Fract. Differ. Appl. 2016, 2, 141–151. [Google Scholar] [CrossRef]
- Abd-Elhameed, W.M.; Alsuyuti, M.M. Numerical treatment of multi-term fractional differential equations via new kind of generalized Chebyshev polynomials. Fractal Fract. 2023, 7, 74. [Google Scholar] [CrossRef]
- Nagy, A.M. Numerical solutions for nonlinear multi-term fractional differential equations via Dickson operational matrix. Int. J. Comput. Math. 2022, 99, 1505–1515. [Google Scholar] [CrossRef]
- Sahu, P.K.; Mallicki, B. Approximate solution of fractional order Lane-Emden type differential equation by orthonormal Bernoulli’s polynomials. Int. J. Appl. Comput. Math. 2019, 5, 89. [Google Scholar] [CrossRef]
- Babolian, E.; Javadi, S.; Moradi, E. RKM for solving Bratu-type differential equations of fractional order. Math. Methods Appl. Sci. 2016, 39, 1548–1557. [Google Scholar] [CrossRef]
- Ghomanjani, F.; Shateyi, S. Numerical solution for fractional Bratu’s initial value problem. Open Phys. 2017, 15, 1045–1048. [Google Scholar] [CrossRef]
- Hilfer, R. Threefold introduction to fractional derivatives. In Anomalous Transport: Foundations and Applications; Klages, R., Radons, G., Sokolov, I., Eds.; Wiley-VCH: Weinheim, Germany, 2008; pp. 17–74. [Google Scholar]
- Teodoro, G.S.; Machado, J.A.T. Capelas de Oliveira, E. A review of definitions of fractional derivatives and other operators. J. Comput. Phys. 2019, 388, 195–208. [Google Scholar] [CrossRef]
- Wang, Q.; Shi, X.; He, J.H.; Li, Z.B. Fractal calculus and its application to explanation of biomechanism of polar bear hairs. Fractals 2018, 26, 1850086. [Google Scholar] [CrossRef]
- Wang, K.J.; Xu, P. Generalized variational structure of the fractal modified KdV-Zakharov-Kuznetsov equation. Fractals 2023, 31, 2350084. [Google Scholar] [CrossRef]
- Wang, K.J.; Xu, P.; Shi, F. Nonlinear dynamic behaviors of the fractional (3+1)- dimensional modified Zakharov-Kuznetsov equation. Fractals 2023, 31, 2350088. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Adel, W.; Izadi, M.; El-Sayed, A.A. Solving Some Physics Problems Involving Fractional-Order Differential Equations with the Morgan-Voyce Polynomials. Fractal Fract. 2023, 7, 301. [Google Scholar] [CrossRef]
- Pakchin, S.I.; Lakestani, M.; Kheiri, H. Numerical approach for solving a class of nonlinear fractional differential equation. Bull. Iranian Math. Soc. 2016, 42, 1107–1126. [Google Scholar]
- Gharechahi, R.; Arabameri, M.; Bisheh-Niasar, M. Numerical solution of fractional Bratu’s initial value problem using compact finite difference scheme. Progr. Fract. Differ. Appl. 2021, 7, 103–115. [Google Scholar]
- Alsuyuti, M.M.; Doha, E.H.; Ezz-Eldien, S.S. Galerkin operational approach for multi-dimensions fractional differential equations. Commun. Nonlinear Sci. Numer. Simul. 2022, 114, 106608. [Google Scholar] [CrossRef]
- El-Sayed, A.A.; Agarwal, P. Numerical solution of multiterm variable-order fractional differential equations via shifted Legendre polynomials. Math. Meth. Appl. Sci. 2019, 41, 3978–3991. [Google Scholar] [CrossRef]
- Duan, J.S.; Zhang, J.Y.; Qiu, X. Exact solutions of fractional order oscillation equation with two fractional derivative terms. J. Nonlinear Math. Phys. 2022, 30, 531–552. [Google Scholar] [CrossRef]
- Heydari, M.H.; Avazzadeh, Z.; Haromi, M.F. A wavelet approach for solving multi-term variable-order time fractional diffusion wave equation. Appl. Math. Comput. 2019, 341, 215–228. [Google Scholar] [CrossRef]
- Mustahsan, M.; Younas, H.M.; Iqbal, S.; Rathore, S.; Nisar, K.S.; Singh, J. An efficient analytical technique for time-fractional parabolic partial differential equations. Front. Phys. 2020, 8, 1–8. [Google Scholar] [CrossRef]
- Kumar, S.; Gupta, V.; Gómez-Aguilar, J.F. An efficient operational matrix technique to solve the fractional order non-local boundary value problems. J. Math. Chem. 2022, 60, 1463–1479. [Google Scholar] [CrossRef]
- Abd-Elhameed, W.M.; Badah, B.M.; Amin, A.K.; Alsuyuti, M.M. Spectral solutions of even-order BVPs based on new operational matrix of derivatives of generalized Jacobi polynomials. Symmetry 2023, 15, 345. [Google Scholar] [CrossRef]
- Odibat, Z.M.; Shawagfeh, N.T. Generalized Taylor’s formula. Appl. Math. Comput. 2007, 186, 286–293. [Google Scholar] [CrossRef]
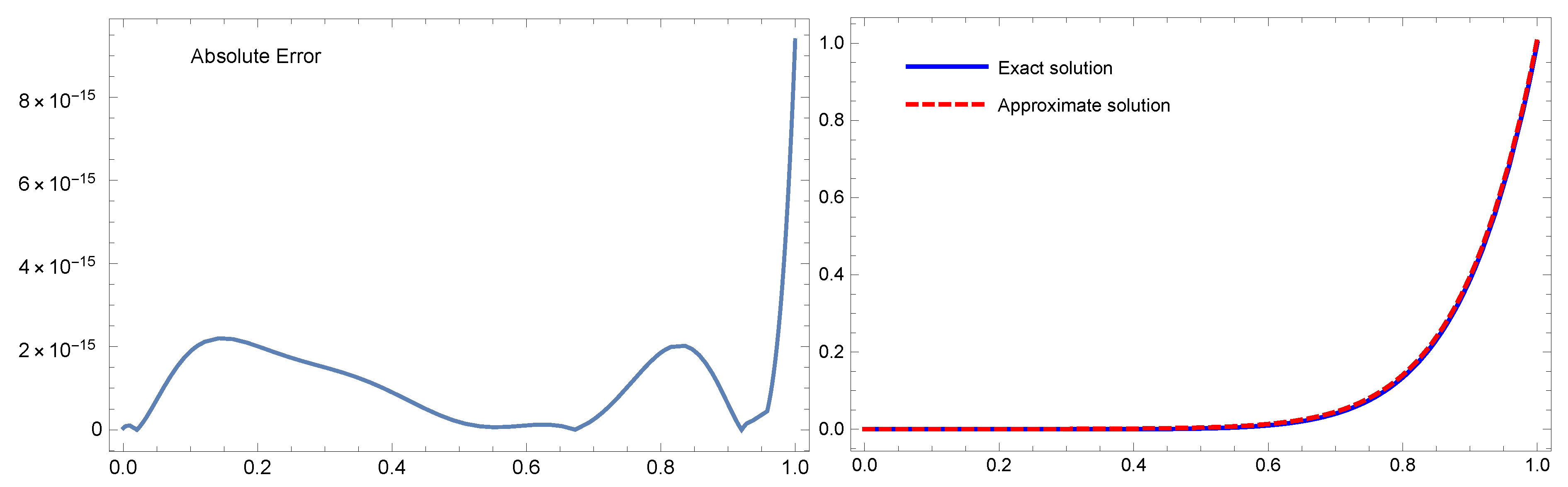
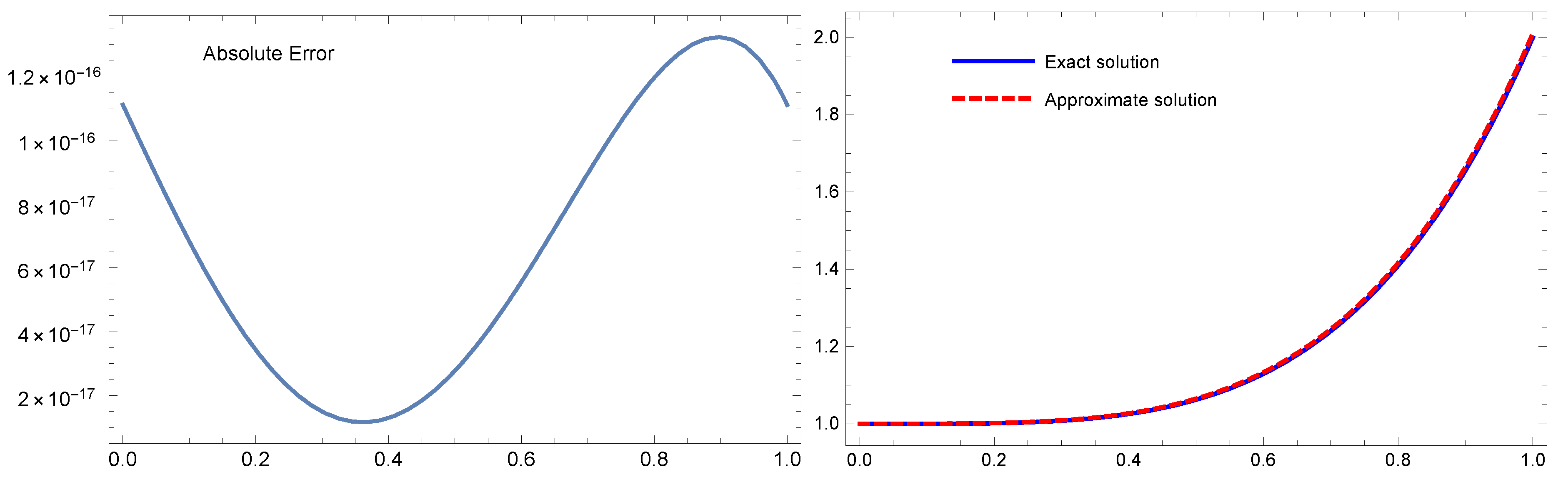

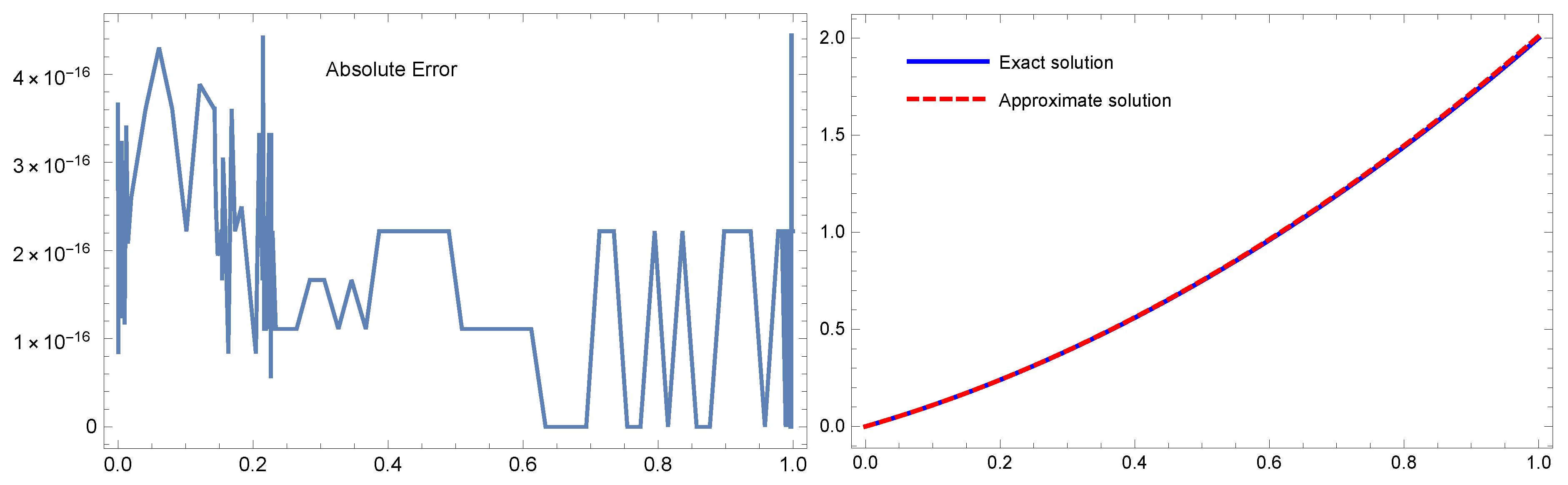

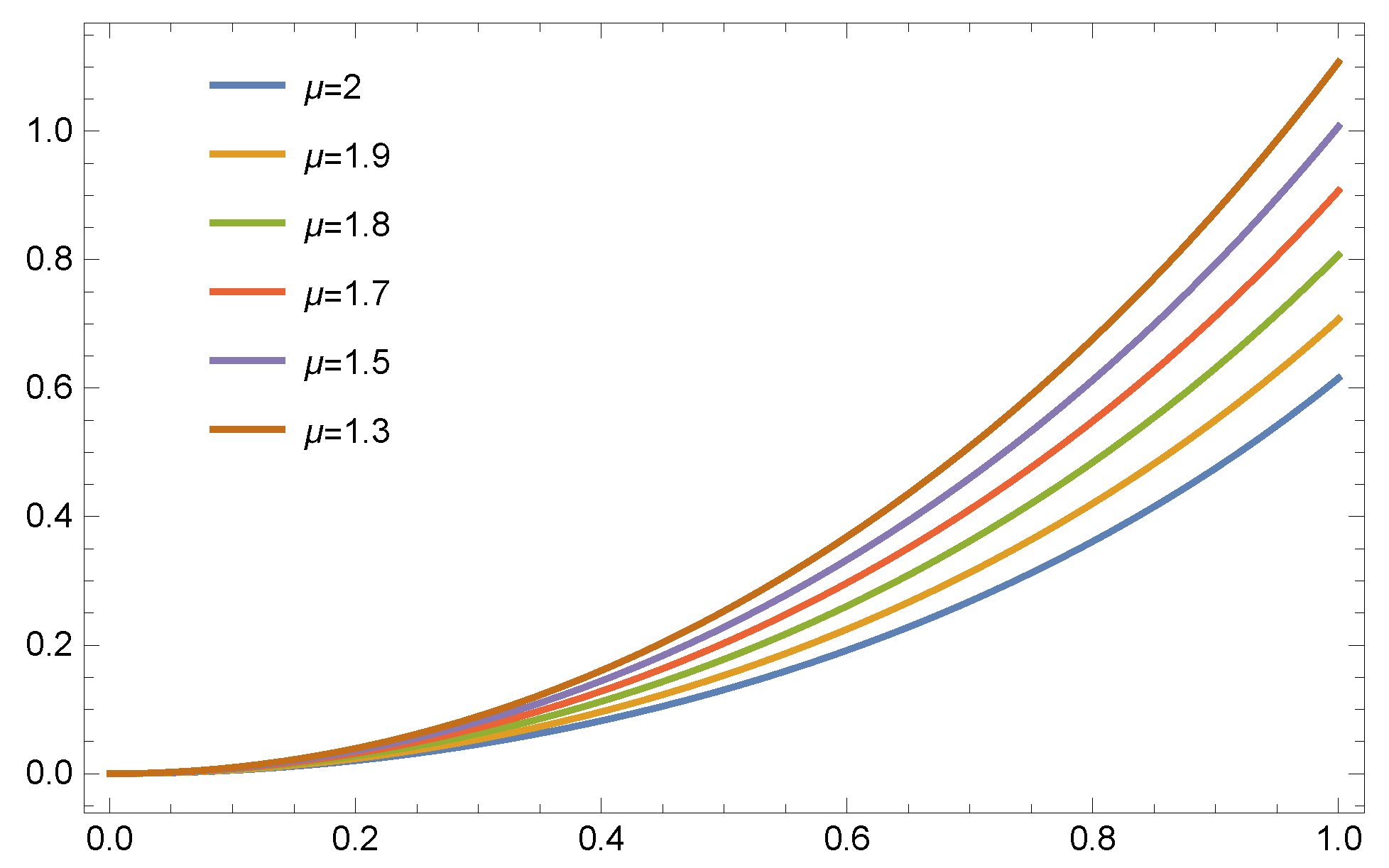
| m | -Error | -Error | ||||
|---|---|---|---|---|---|---|
| In [38] | In [28] | Our Method | In [38] | In [28] | Our Method | |
| 5 | - | - | ||||
| 7 | ||||||
| 8 | ||||||
| 9 | ||||||
| t | Example 1 | Example 2 | Example 3 |
|---|---|---|---|
| 0.1 | |||
| 0.2 | |||
| 0.3 | |||
| 0.4 | |||
| 0.5 | |||
| 0.6 | |||
| 0.7 | |||
| 0.8 | |||
| 0.9 |
| m | -Error | -Error | ||||
|---|---|---|---|---|---|---|
| In [38] | In [28] | Our Method | In [38] | In [28] | Our Method | |
| 2 | ||||||
| 4 | ||||||
| 8 | ||||||
| 10 | ||||||
| m | -Error | -Error | ||
|---|---|---|---|---|
| In [28] | Our Method | In [28] | Our Method | |
| 3 | ||||
| 5 | ||||
| 7 | ||||
| 9 | ||||
| t | In [29] | Proposed Method |
|---|---|---|
| 0.1 | ||
| 0.2 | ||
| 0.3 | ||
| 0.4 | ||
| 0.5 | ||
| 0.6 | ||
| 0.7 | 0.0 | |
| 0.8 | 0.0 | |
| 0.9 | 0.0 | |
| 1.0 | 0.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
El-Sayed, A.A.E.; Boulaaras, S.; AbaOud, M. Semi-Analytical Solutions for Some Types of Nonlinear Fractional-Order Differential Equations Based on Third-Kind Chebyshev Polynomials. Fractal Fract. 2023, 7, 784. https://doi.org/10.3390/fractalfract7110784
El-Sayed AAE, Boulaaras S, AbaOud M. Semi-Analytical Solutions for Some Types of Nonlinear Fractional-Order Differential Equations Based on Third-Kind Chebyshev Polynomials. Fractal and Fractional. 2023; 7(11):784. https://doi.org/10.3390/fractalfract7110784
Chicago/Turabian StyleEl-Sayed, Adel Abd Elaziz, Salah Boulaaras, and Mohammed AbaOud. 2023. "Semi-Analytical Solutions for Some Types of Nonlinear Fractional-Order Differential Equations Based on Third-Kind Chebyshev Polynomials" Fractal and Fractional 7, no. 11: 784. https://doi.org/10.3390/fractalfract7110784
APA StyleEl-Sayed, A. A. E., Boulaaras, S., & AbaOud, M. (2023). Semi-Analytical Solutions for Some Types of Nonlinear Fractional-Order Differential Equations Based on Third-Kind Chebyshev Polynomials. Fractal and Fractional, 7(11), 784. https://doi.org/10.3390/fractalfract7110784







