To the Theory of Decaying Turbulence
Abstract
:1. Introduction
1.1. Fluctuating Geometry in Gauge Theory and Turbulent Flow
1.2. Sparse Vorticity Picture
2. Loop Equation
2.1. Wilson Loop as Reduced Hopf Functional
- The Wilson loop is a dimensional reduction of the Hopf functional. Instead of a source mapping we have a source mapping a circle to physical space . This mapping makes the loop equation a one-dimensional problem with physical space becoming a target space, like in string theory. The functional differential equations in more than one dimension are never solved except for the linear (or linearizable) ones. One-dimensional problems, even nonlinear ones, are sometimes solvable, and the loop equation is no exception, as we shall see. Rather than being an abstract notion, the Hopf equation becomes an analytical tool after this projection to one dimension.
- We have lost most of the information about vector field by reducing the Hopf source to a one-dimensional subset of the physical space. This information would be irrecoverable if we only consider the set of smooth closed loops in space. However, with the loop functional defined for arbitrary non-smooth loop C, the information about velocity correlation functions and other statistical observables can be recovered [1] as follows. The small variation of the loop corresponding to attaching a closed loop at some point (which necessarily makes the loop non-smooth) brings down vorticity at this point. It allows to computation of the vorticity correlation functions. After that, velocity correlations can be recovered from incompressibility (the Biot-Savart integral in coordinate space or orthogonal projection in Fourier space).
- The imaginary factor in the source is necessary for the mathematical existence of this functional. As the DNS shows ([8,9,34]), and the Kelvinon theory supports [35], the PDF of circulation decays only exponentially. The Fourier transform of such PDF exists but in the case of real instead of imaginary factor , the integral over the circulation distribution would diverge either on the positive or the negative circulation, depending upon the sign of ; it should then be defined as an analytic continuation (Laplace transform).
2.2. Plane Wave in Loop Space
2.3. Random Global Vorticity
2.4. Reduced Loop Equation
2.5. Decay or Fixed Point
3. Fractal Curve in Complex Space
3.1. Random Walk
3.2. Constraints Imposed on a Random Step
3.3. Closure Condition
3.4. Mirror Pairs of Solutions
3.5. The Degenerate Fixed Point and Its Statistical Meaning
4. Exact Analytic Solution
4.1. Random Walk on a Circle
4.2. The Euler Ensemble
4.3. Grand Canonical Ensemble
5. Correlation Functions
5.1. General Formulas
5.2. Critical Phenomena in Statistical Limit
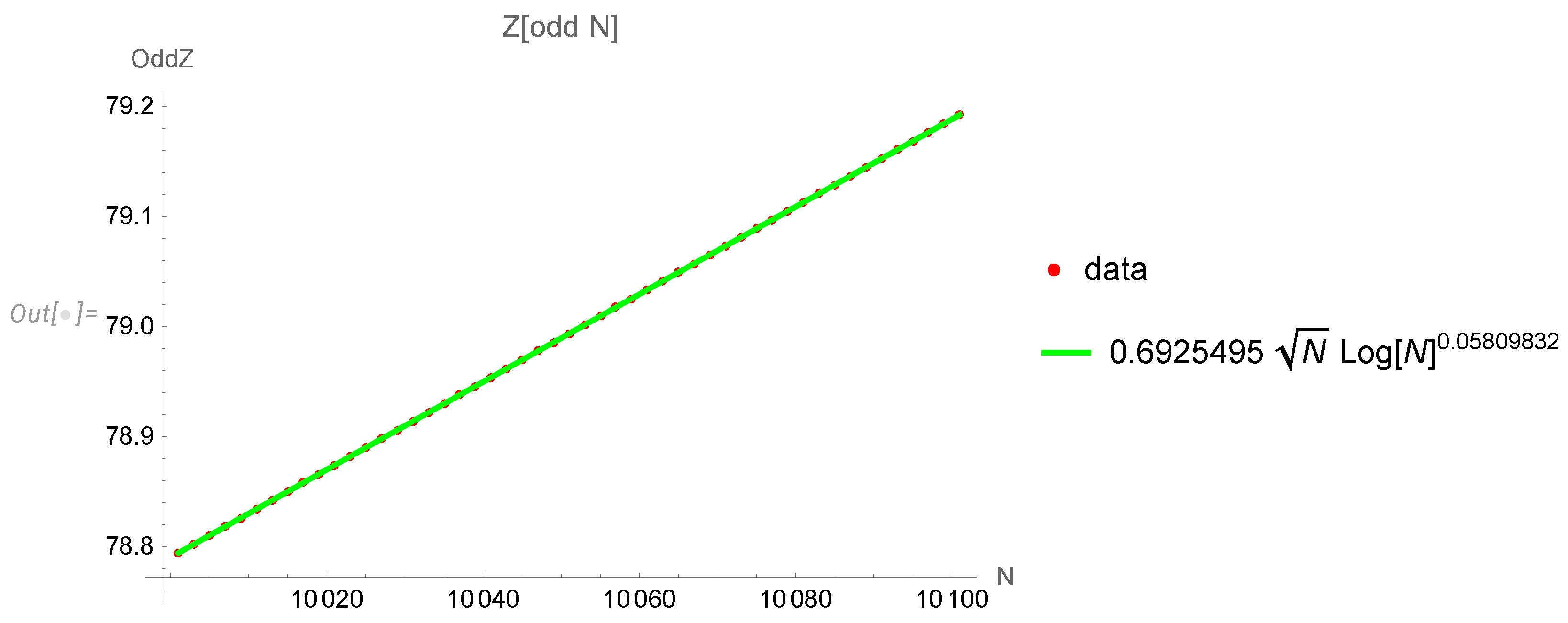
5.3. Analytic Solution for the Enstrophy
5.4. The Local Limit of the Energy Dissipation
5.5. The Higher Moments of the Enstrophy
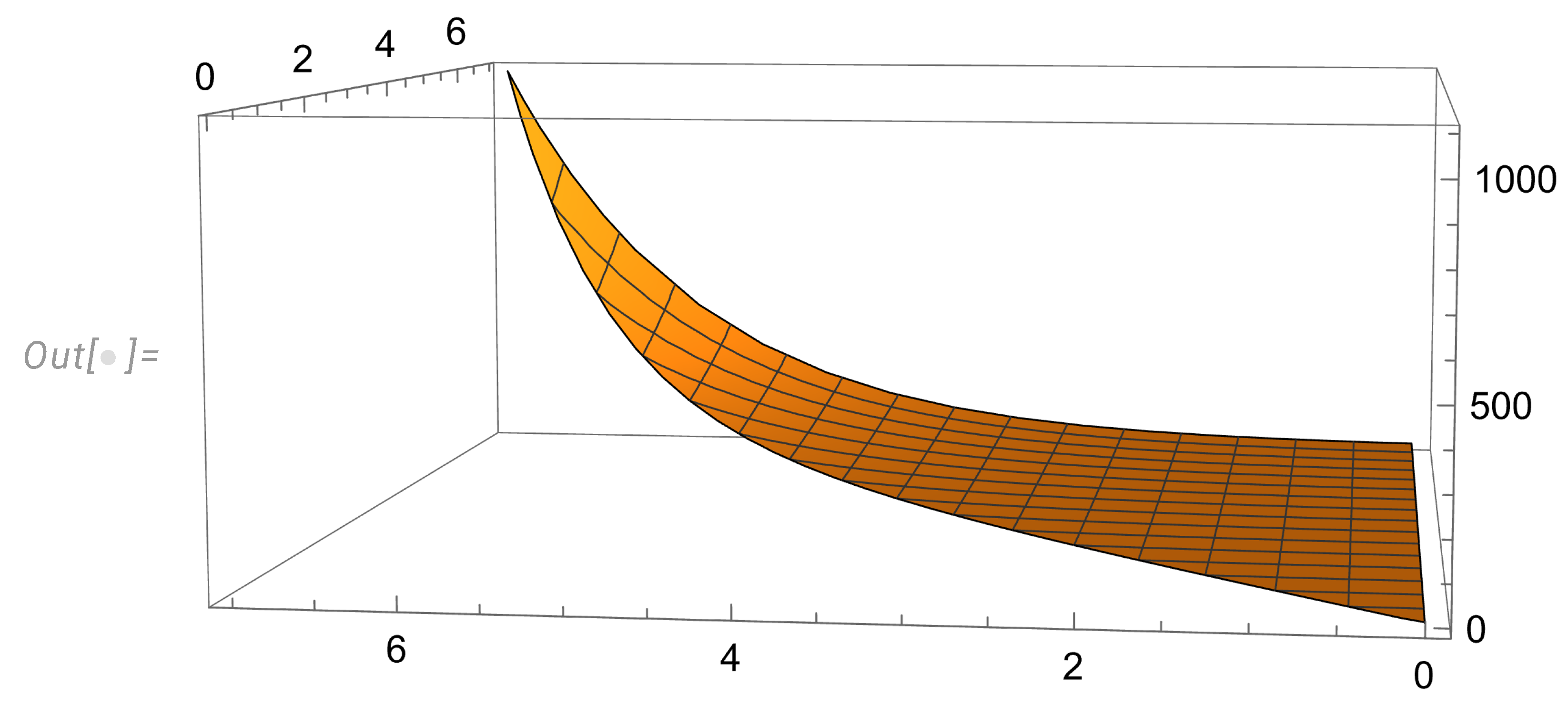
5.6. The Vorticity Distribution
6. The Decay Index Spectrum
6.1. Linearized Loop Equation
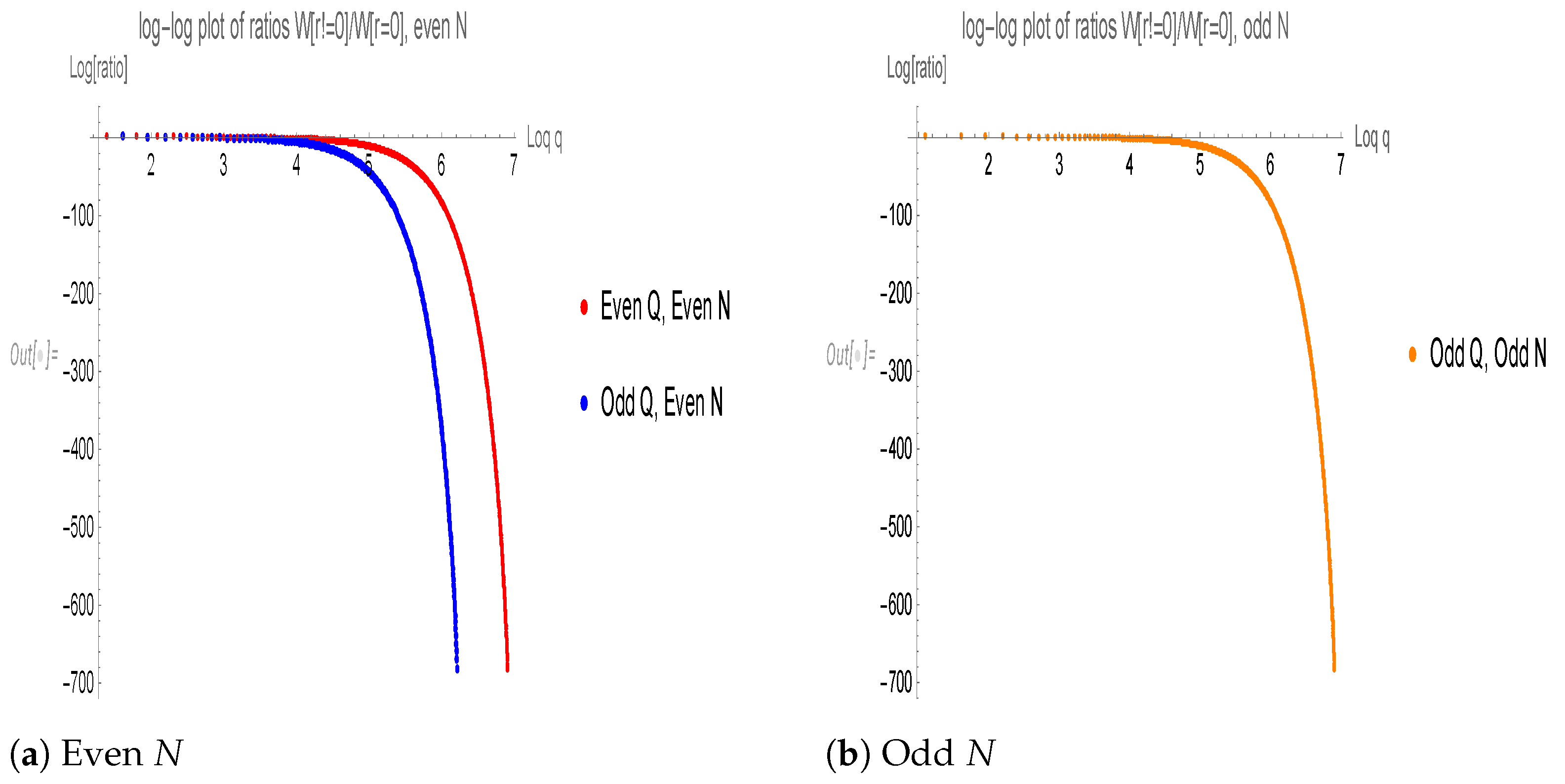
6.2. The Spectral Identity and Wilson Loop Asymptotics
7. Discussion
7.1. Singular Solutions of the Navier-Stokes Equation?
7.2. Stochastic Solution of the Navier-Stokes Equation and Ergodic Hypothesis
7.3. The Physical Meaning of the Loop Equation and Dimensional Reduction
7.4. Classical Flow and Quantum Geometry
7.5. Stokes-Type Functionals and Vorticity Correlations
7.6. Relation of Our Solution to the Weak Turbulence
7.7. Continuum Spectrum of Anomalous Dimensions and Multifractality
7.8. Conclusion
- The solution for in the loop equation for decaying turbulence, which we have found in this paper, exhibits nonperturbative features, particularly the quantization of parameters. These quantum effects follow from the exact equivalence of the Navier-Stokes statistics to the quantum mechanics in loop space [1]. The most striking nonperturbative feature is the singularity of vorticity distribution in the local limit for a finite viscosity.
- Compared to the other critical phenomena, this theory is amazingly simple: It is not a field theory but a quantum statistical system similar to the one-dimensional Ising ring in the presence of the imaginary quantized magnetic field. The moments of the distribution of vorticity are analytically calculable.
- Still, the solution is far from trivial and reveals unexpected relations between turbulence and number theory. The analytic computation of the decay spectrum from the general Equation (214) for the resolvent remains a challenge to the number theory.
- This work does not claim a full solution to the decaying turbulence problem. At best, this is the new path to the solution; following this path will require joint efforts of mathematical physicists, number theorists, and computer scientists.
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
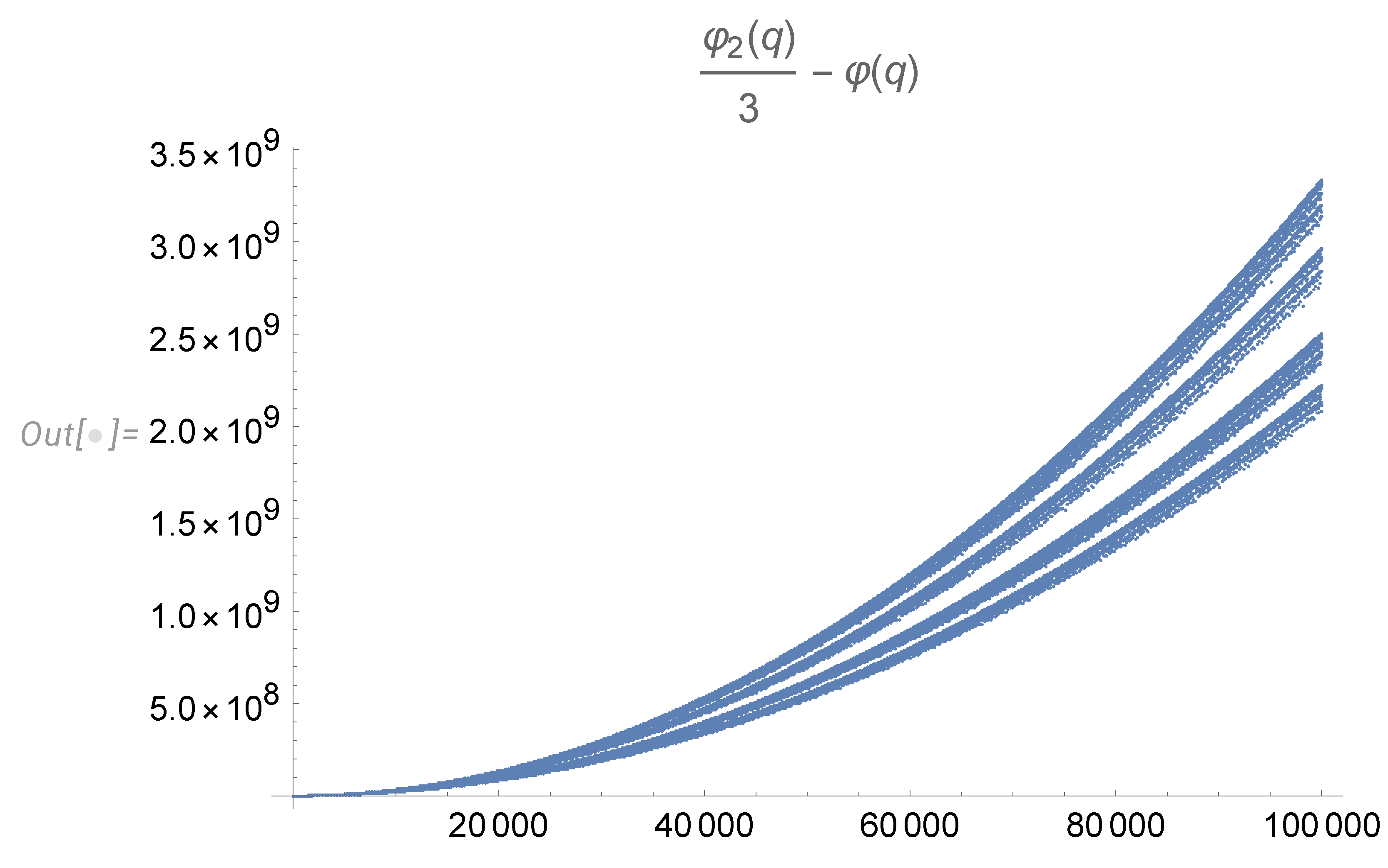
Appendix A. Euler Averages as Multitotient Functions
References
- Migdal, A. Statistical Equilibrium of Circulating Fluids. Phys. Rep. 2023, 1011C, 1–117. [Google Scholar] [CrossRef]
- Migdal, A. Momentum loop dynamics and random surfaces in QCD. Nucl. Phys. B 1986, 265, 594–614. [Google Scholar] [CrossRef]
- Migdal, A. Second quantization of the Wilson loop. Nucl. Phys. B Proc. Suppl. 1995, 41, 151–183. [Google Scholar] [CrossRef]
- Anderson, P.D.; Kruczenski, M. Loop equations and bootstrap methods in the lattice. Nucl. Phys. B 2017, 921, 702–726. [Google Scholar] [CrossRef]
- Kazakov, V.; Zheng, Z. Bootstrap for lattice Yang-Mills theory. Phys. Rev. D 2023, 107, L051501. [Google Scholar] [CrossRef]
- Ashtekar, A. New variables for classical and quantum gravity. Phys. Rev. Lett. 1986, 57, 2244–2247. [Google Scholar] [CrossRef]
- Rovelli, C.; Smolin, L. Knot Theory and Quantum Gravity. Phys. Rev. Lett. 1988, 61, 1155–1158. [Google Scholar] [CrossRef]
- Iyer, K.P.; Sreenivasan, K.R.; Yeung, P.K. Circulation in High Reynolds Number Isotropic Turbulence is a Bifractal. Phys. Rev. X 2019, 9, 041006. [Google Scholar] [CrossRef]
- Iyer, K.P.; Bharadwaj, S.S.; Sreenivasan, K.R. The area rule for circulation in three-dimensional turbulence. Proc. Natl. Acad. Sci. USA 2021, 118, e2114679118. [Google Scholar] [CrossRef]
- Apolinario, G.; Moriconi, L.; Pereira, R.; Valadão, V. Vortex Gas Modeling of Turbulent Circulation Statistics. Phys. Rev. E 2020, 102, 041102. [Google Scholar] [CrossRef]
- Müller, N.P.; Polanco, J.I.; Krstulovic, G. Intermittency of Velocity Circulation in Quantum Turbulence. Phys. Rev. X 2021, 11, 011053. [Google Scholar] [CrossRef]
- Parisi, G.; Frisch, U. On the singularity structure of full developed turbulence. In Turbulence and Predictability in Geophysical Fluid Dynamics and Climate Dynamics; Ghil, M., Benzi, R., Parisi, G., Eds.; North-Holland: Amsterdam, The Netherlands, 1985; p. 71. [Google Scholar]
- Ishihara, T.; Gotoh, T.; Kaneda, Y. Study of High–Reynolds Number Isotropic Turbulence by Direct Numerical Simulation. Annu. Rev. Fluid Mech. 2008, 41, 165–180. [Google Scholar] [CrossRef]
- Buaria, D.; Pumir, A.; Bodenschatz, E.; Yeung, P.K. Extreme velocity gradients in turbulent flows. New J. Phys. 2019, 21, 043004. [Google Scholar] [CrossRef]
- Sreenivasan, K.R. On the scaling of the turbulence energy dissipation rate. Phys. Fluids 1984, 27, 1048–1051. [Google Scholar] [CrossRef]
- Burgers, J. A Mathematical Model Illustrating the Theory of Turbulence. In Advances in Applied Mechanics; Von Mises, R., Von Kármán, T., Eds.; Elsevier: Amsterdam, The Netherlands, 1948; Volume 1, pp. 171–199. [Google Scholar] [CrossRef]
- Wikipedia. Burgers Vortex. 2022. Available online: https://en.wikipedia.org/wiki/Burgers_vortex (accessed on 27 April 2022).
- Townsend, A.A. On the fine-scale structure of turbulence. Proc. Roy. Soc. Lond. Ser. A 1951, 208, 534–542. [Google Scholar] [CrossRef]
- Baker, G.R.; Meiron, D.I.; Orszag, S.A. Boundary integral methods for axisymmetric and three-dimensional Rayleigh-Taylor instability problems. Phys. D Nonlinear Phenom. 1984, 12, 19–31. [Google Scholar] [CrossRef]
- Lamb, H. Hydrodynamics; Dover Publications: Mineola, NY, USA, 1945. [Google Scholar]
- Khalatnikov, I. The hydrodynamics of solutions of impurities in helium II. Zh. Eksp. Teor. Fiz. 1952, 23, 169. [Google Scholar]
- Kuznetsov, E.; Mikhailov, A. On the topological meaning of canonical Clebsch variables. Phys. Lett. A 1980, 77, 37–38. [Google Scholar] [CrossRef]
- Levich, E. The Hamiltonian formulation of the Euler equation and subsequent constraints on the properties of randomly stirred fluids. Phys. Lett. A 1981, 86, 165–168. [Google Scholar] [CrossRef]
- Marsden, J.; Weinstein, A. Coadjoint orbits, vortices, and Clebsch variables for incompressible fluids. Phys. D Nonlinear Phenom. 1983, 7, 305–323. [Google Scholar] [CrossRef]
- Yakhot, V.; Zakharov, V. Hidden conservation laws in hydrodynamics; energy and dissipation rate fluctuation spectra in strong turbulence. Phys. D Nonlinear Phenom. 1993, 64, 379–394. [Google Scholar] [CrossRef]
- Volovik, G. Linear momentum in ferromagnets. J. Phys. C 1987, 20, L83–L87. [Google Scholar] [CrossRef]
- Vollhardt, D.; Wölfle, P. The Superfluid Phases of Helium 3; Taylor & Francis: London, UK, 1990. [Google Scholar]
- Volovik, G.E. The Universe in a Helium Droplet; Clarendon Press: Oxford, UK, 2003. [Google Scholar]
- Blaha, S. Quantization rules for point singularities in superfluid 3He and liquid crystals. Phys. Rev. Lett. 1976, 36, 874. [Google Scholar] [CrossRef]
- Volovik, G.E.; Mineev, V.P. Vortices with free ends in superfluid 3He-A. JETP Lett. 1976, 24, 647–649. [Google Scholar]
- Volovik, G. Monopoles and fractional vortices in chiral superconductors. Proc. Natl. Acad. Sci. USA 2000, 97, 2431–2436. [Google Scholar] [CrossRef]
- Tao, T. Finite time blowup for an averaged three-dimensional Navier-Stokes equation. J. Am. Math. Soc. 2016, 29, 601–674. [Google Scholar] [CrossRef]
- Ohkitani, K. Study of the Hopf functional equation for turbulence: Duhamel principle and dynamical scaling. Phys. Rev. E 2019, 101, 013104. [Google Scholar] [CrossRef]
- Shariff, K.; Elsinga, G.E. Viscous vortex layers subject to more general strain and comparison to isotropic turbulence. Phys. Fluids 2021, 33, 033611. [Google Scholar] [CrossRef]
- Migdal, A. Topological Vortexes, Asymptotic Freedom, and Multifractals. Fractal Fract. 2023, 7, 351. [Google Scholar] [CrossRef]
- Makeenko, Y.; Migdal, A. Exact equation for the loop average in multicolor QCD. Phys. Lett. B 1979, 88, 135–137. [Google Scholar] [CrossRef]
- Migdal, A. Loop equations and expansion. Phys. Rep. 1983, 201, 199–290. [Google Scholar] [CrossRef]
- Migdal, A.A. Hidden symmetries of large N QCD. Prog. Theor. Phys. Suppl. 1998, 131, 269–307. [Google Scholar] [CrossRef]
- Migdal, A. Decaying Turbulence Computations. 2023. Available online: https://www.wolframcloud.com/obj/sasha.migdal/Published/DecayingTurbulenceComputations.nb (accessed on 4 October 2023).
- Hardy, G.H.; Wright, E.M. An Introduction to the Theory of Numbers, 6th ed.; Oxford University Press: Oxford, UK, 2008. [Google Scholar]
- Basak, D.; Department of Mathematics, University of Illinois at Urbana–Champaign, Illinois, USA; Zaharesku, A.; Department of Mathematics, University of Illinois at Urbana–Champaign, Illinois, USA. Euler ensemble for the odd case. Personal communication. to be published.
- Bulatov, M.; Tashkent University, Tashkent, Uzbekistan; Migdal, A.; New York University, Abu Dhabi, Abu Dhabi. Numerical Simulations of Fractal Curve in Decalying Turbulence Theory. Unpublished work.
- Lehmer, D.N. Asymptotic Evaluation of Certain Totient Sums. Am. J. Math. 1900, 22, 293–335. [Google Scholar] [CrossRef]
- Tao, T. Searching for singularities in the Navier–Stokes equations. Nat. Rev. Phys. 2019, 1, 418–419. [Google Scholar] [CrossRef]
- Feynman, R.P.; Leighton, R.B.; Sands, M. 3–7 How did it get that way? In The Feynman lectures on physics, Vol. I: Mainly Mechanics, Radiation, and Heat; Gottlieb, M.A., Pfeiffer, R., Eds.; Basic Books: New York, NY, USA, 2011; Volume 1. [Google Scholar]
- Migdal, A. Loop Equation and Area Law in Turbulence. In Quantum Field Theory and String Theory; Baulieu, L., Dotsenko, V., Kazakov, V., Windey, P., Eds.; Springer: New York, NY, USA, 1995; pp. 193–231. [Google Scholar] [CrossRef]
- Cioabă, S.M.; Murty, M.R. The Principle of Inclusion and Exclusion. In A First Course in Graph Theory and Combinatorics, 2nd ed.; Springer: Singapore, 2022; pp. 33–42. [Google Scholar] [CrossRef]
- Franke, J. Rational functions, cotangent sums and Eichler integrals. Res. Number Theory 2021, 7, 23. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Migdal, A. To the Theory of Decaying Turbulence. Fractal Fract. 2023, 7, 754. https://doi.org/10.3390/fractalfract7100754
Migdal A. To the Theory of Decaying Turbulence. Fractal and Fractional. 2023; 7(10):754. https://doi.org/10.3390/fractalfract7100754
Chicago/Turabian StyleMigdal, Alexander. 2023. "To the Theory of Decaying Turbulence" Fractal and Fractional 7, no. 10: 754. https://doi.org/10.3390/fractalfract7100754
APA StyleMigdal, A. (2023). To the Theory of Decaying Turbulence. Fractal and Fractional, 7(10), 754. https://doi.org/10.3390/fractalfract7100754





