A New Perspective on the Exact Solutions of the Local Fractional Modified Benjamin–Bona–Mahony Equation on Cantor Sets
Abstract
1. Introduction
2. Basic Theory
3. Construct of the NLFODEs
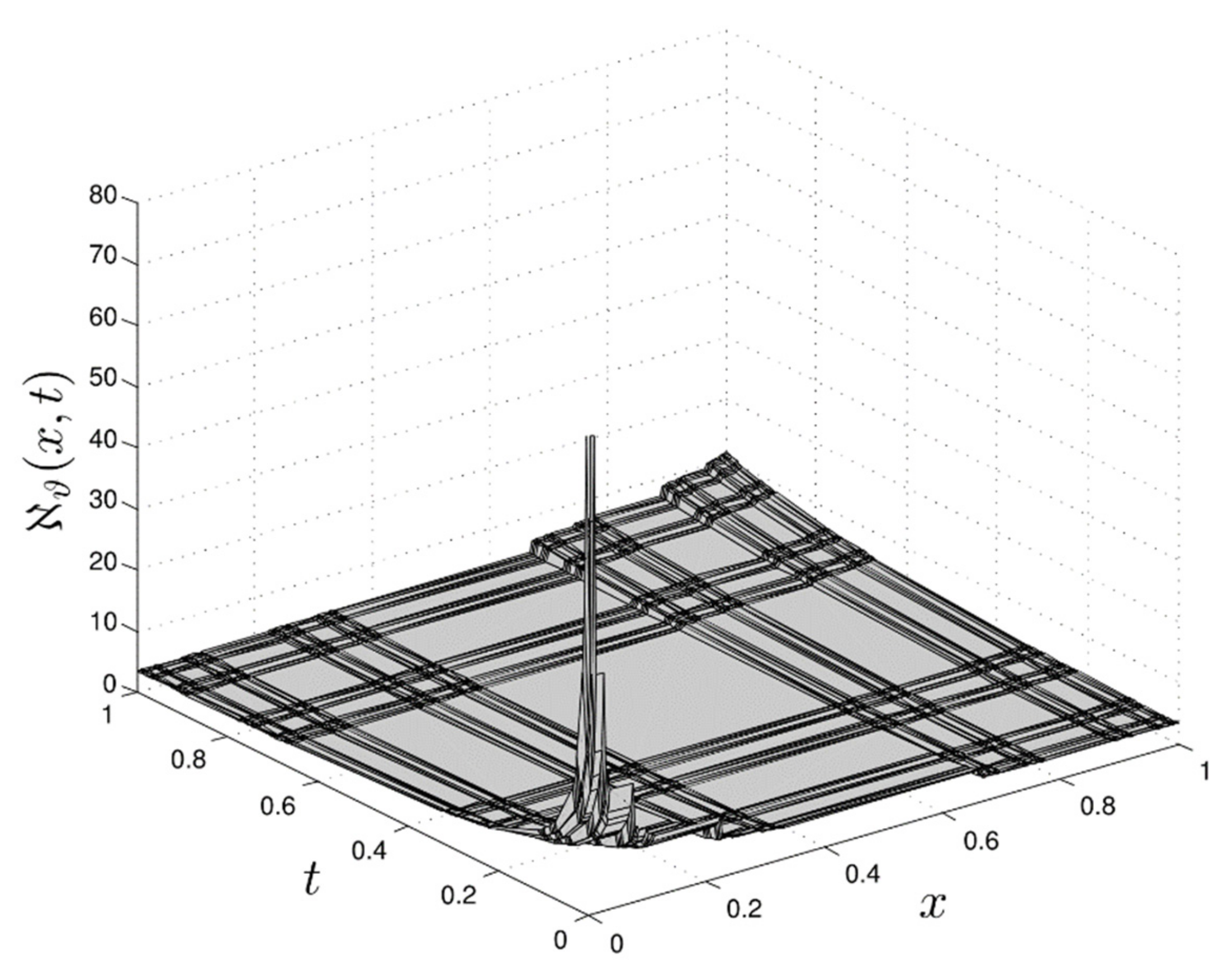
4. Yang’s Special Function Method
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Biswas, A.; Milovic, D.; Koh, R. Optical soliton perturbation in a log-law medium with full nonlinearity by He’s semi-inverse variational principle. Inverse Probl. Sci. Eng. 2021, 20, 227–232. [Google Scholar] [CrossRef]
- Wang, K.J.; Liu, J.-H. Diverse optical soliton solutions to the Kundu-Mukherjee-Naskar equation via two novel techniques. Optik 2023, 273, 170403. [Google Scholar] [CrossRef]
- Muniyappan, A.; Sahasraari, L.N.; Anitha, S.; Ilakiya, S.; Biswas, A.; Yıldırım, Y.; Triki, H.; Alshehri, H.M.; Belic, M.R. Family of optical solitons for perturbed Fokas–Lenells equation. Optik 2022, 249, 168224. [Google Scholar] [CrossRef]
- Wang, K.J. Diverse soliton solutions to the Fokas system via the Cole-Hopf transformation. Optik 2023, 272, 170250. [Google Scholar] [CrossRef]
- Lü, X.; Chen, S.-J. New general interaction solutions to the KPI equation via an optional decoupling condition approach. Commun. Nonlinear Sci. Numer. Simul. 2021, 103, 105939. [Google Scholar] [CrossRef]
- Qie, N.; Houa, W.F.; He, J.H. The fastest insight into the large amplitude vibration of a string. Rep. Mech. Eng. 2021, 2, 1–5. [Google Scholar] [CrossRef]
- He, C.H.; Tian, D.; Salman, H.F.; Zekry, M.H. Hybrid Rayleigh-Van der Pol-Duffing Oscillator (HRVD): Stability Analysis and Controller. J. Low Freq. Noise Vib. Act. Control. 2022, 41, 244–268. [Google Scholar] [CrossRef]
- Guo, A.; Ding, X.; Zhong, F.; Cheng, Q.; Huang, C. Predicting the future chinese population using shared socioeconomic pathways, the sixth national population census, and a PDE model. Sustainability 2019, 11, 3686. [Google Scholar] [CrossRef]
- Sohail, M.; Naz, R.; Shah, Z.; Kumam, P.; Thounthong, P. Exploration of temperature dependent thermophysical characteristics of yield exhibiting non-Newtonian fluid flow under gyrotactic microorganisms. AIP Adv. 2019, 9, 125016. [Google Scholar] [CrossRef]
- Abdelsalam, S.I.; Sohail, M. Numerical approach of variable thermophysical features of dissipated viscous nanofluid comprising gyrotactic micro-organisms. Pramana 2020, 94, 1–12. [Google Scholar] [CrossRef]
- Dashraath, P.; Wong, J.L.; Lim, M.X.; Lim, L.M.; Li, S.; Biswas, A.; Choolani, M.; Mattar, C.; Su, L.L. Coronavirus disease 2019 (COVID-19) pandemic and pregnancy. Am. J. Obstet. Gynecol. 2020, 222, 521–531. [Google Scholar] [CrossRef]
- Wang, K.J. Variational principle and diverse wave structures of the modified Benjamin-Bona-Mahony equation arising in the optical illusions field. Axioms 2022, 11, 445. [Google Scholar] [CrossRef]
- Singh, A.P.; Biswas, A.; Shukla, A.; Maiti, P. Targeted therapy in chronic diseases using nanomaterial-based drug delivery vehicles. Signal Transduct. Target. Ther. 2019, 4, 1–21. [Google Scholar] [CrossRef]
- Liu, J.-G.; Yang, X.-J.; Geng, L.-L.; Yu, X.-J. On fractional symmetry group scheme to the higher dimensional space and time fractional dissipative Burgers equation. Int. J. Geom. Methods Mod. Phys. 2022, 19, 2250173. [Google Scholar] [CrossRef]
- Wang, K.J. Bäcklund transformation and diverse exact explicit solutions of the fractal combined KdV-mKdV equation. Fractals 2022, 30, 2250189. [Google Scholar] [CrossRef]
- Muhammad, S.; Mohyud-Din, S.T. Reduced differential transform method for time-fractional heat equations. Int. J. Mark. Trade Policy 2012, 1, 13–22. [Google Scholar]
- Wang, K.L. New perspective to the fractal Konopelchenko-Dubrovsky equations with M-truncated fractional derivative. Int. J. Geom. Methods Mod. Phys. 2023, 2023, 2350072. [Google Scholar] [CrossRef]
- Xiao, B.; Huang, Q.; Chen, H.; Chen, X.; Long, G. A fractal model for capillary flow through a single tortuous capillary with roughened surfaces in fibrous porous media. Fractals 2021, 29, 2150017. [Google Scholar] [CrossRef]
- Baleanu, D.; Abadi, M.H.; Jajarmi, A.; Vahid, K.Z.; Nieto, J.J. A new comparative study on the general fractional model of COVID-19 with isolation and quarantine effects. Alex. Eng. J. 2022, 61, 4779–4791. [Google Scholar] [CrossRef]
- Zhang, Y.; Yu, X.; Sun, H.; Tick, G.R.; Wei, W.; Jin, B. Applicability of time fractional derivative models for simulating the dynamics and mitigation scenarios of COVID-19. Chaos Solitons Fractals 2020, 138, 109959. [Google Scholar] [CrossRef]
- Ghanbari, B.; Kumar, S.; Kumar, R. A study of behaviour for immune and tumor cells in immunogenetic tumour model with non-singular fractional derivative. Chaos Solitons Fractals 2020, 133, 109619. [Google Scholar] [CrossRef]
- He, J.H. A simple approach to one-dimensional convection-diffusion equation and its fractional modification for E reaction arising in rotating disk electrodes. J. Electroanal. Chem. 2019, 854, 113565. [Google Scholar] [CrossRef]
- Singh, H.; Srivastava, H.M. Numerical simulation for fractional-order Bloch equation arising in nuclear magnetic resonance by using the Jacobi polynomials. Appl. Sci. 2020, 10, 2850. [Google Scholar] [CrossRef]
- Kumar, S.; Kumar, A.; Momani, S.; Aldhaifallah, M.; Nisar, K.S. Numerical solutions of nonlinear fractional model arising in the appearance of the strip patterns in two-dimensional systems. Adv. Differ. Equ. 2019, 2019, 1–19. [Google Scholar] [CrossRef]
- Patnaik, S.; Sidhardh, S.; Semperlotti, F. Towards a unified approach to nonlocal elasticity via fractional-order mechanics. Int. J. Mech. Sci. 2021, 189, 105992. [Google Scholar] [CrossRef]
- Wang, G.; Liu, Y.; Wu, Y.; Su, X. Symmetry analysis for a seventh-order generalized KdV equation and its fractional version in fluid mechanics. Fractals 2020, 28, 2050044. [Google Scholar] [CrossRef]
- Raja, M.A.; Manzar, M.A.; Shah, S.M.; Chen, Y. Integrated intelligence of fractional neural networks and sequential quadratic programming for Bagley-Torvik systems arising in fluid mechanics. J. Comput. Nonlinear Dyn. 2020, 15, 051003. [Google Scholar] [CrossRef]
- Kiani-B, A.; Fallahi, K.; Pariz, N.; Leung, H. A chaotic secure communication scheme using fractional chaotic systems based on an extended fractional Kalman filter. Commun. Nonlinear Sci. Numer. Simul. 2009, 14, 863–879. [Google Scholar] [CrossRef]
- Tian, X.; Sun, X.; Yu, X.; Li, X. Modulation pattern recognition of communication signals based on fractional low-order Choi-Williams distribution and convolutional neural network in impulsive noise environment. In Proceedings of the 2019 IEEE 19th International Conference on Communication Technology (ICCT), Xi’an, China, 16–19 October 2019; IEEE: New York, NY, USA, 2019; pp. 188–192. [Google Scholar]
- Kalikulov, N.; Zhussip, D.; Zhexenov, N.; Kizilirmak, R.C. Multipath diversity for OFDM based visible light communication systems Through fractional sampling. Wirel. Pers. Commun. 2020, 112, 2715–2724. [Google Scholar] [CrossRef]
- Dimitrov, D.; Abdo, H. Tight independent set neighborhood union condition for fractional critical deleted graphs and ID deleted graphs. Discret. Contin. Dyn. Syst. S 2019, 12, 711. [Google Scholar] [CrossRef]
- Xiao, B.; Wang, W.; Zhang, X.; Long, G.; Fan, J.; Chen, H.; Deng, L. A novel fractal solution for permeability and Kozeny-Carman constant of fibrous porous media made up of solid particles and porous fibers. Powder Technol. 2019, 349, 92–98. [Google Scholar] [CrossRef]
- Stojiljković, V.; Ramaswamy, R.; Abdelnaby, O.A.A.; Radenović, S. Some Novel Inequalities for LR-(k,h-m)-p Convex Interval Valued Functions by Means of Pseudo Order Relation. Fractal Fract. 2022, 6, 726. [Google Scholar] [CrossRef]
- Singh, J.; Ahmadian, A.; Rathore, S.; Kumar, D.; Baleanu, D.; Salimi, M.; Salahshour, S. An efficient computational approach for local fractional Poisson equation in fractal media. Numer. Methods Part. Differ. Equ. 2021, 37, 1439–1448. [Google Scholar] [CrossRef]
- Singh, J.; Jassim, H.K.; Kumar, D. An efficient computational technique for local fractional Fokker Planck equation. Phys. A: Stat. Mech. Its Appl. 2020, 555, 124525. [Google Scholar] [CrossRef]
- Yang, X.J.; Gao, F.; Srivastava, H.M. A new computational approach for solving nonlinear local fractional PDEs. J. Comput. Appl. Math. 2018, 339, 285–296. [Google Scholar] [CrossRef]
- Ziane, D.; Baleanu, D.; Belghaba, K.; Cherif, M.H. Local fractional Sumudu decomposition method for linear partial differential equations with local fractional derivative. J. King Saud Univ. Sci. 2019, 31, 83–88. [Google Scholar] [CrossRef]
- Wang, K.J.; Si, J. On the non-differentiable exact solutions of the (2+1)-dimensional local fractional breaking soliton equation on Cantor sets. Math. Methods Appl. Sci. 2023, 46, 1456–1465. [Google Scholar] [CrossRef]
- Yang, X.J.; Baleanu, D. Fractal heat conduction problem solved by local fractional variation iteration method. Therm. Sci. 2013, 17, 625–628. [Google Scholar] [CrossRef]
- Wang, K.J. Investigation to the local fractional Fokas system on Cantor set by a novel technology. Fractals 2022, 30, 2250112. [Google Scholar] [CrossRef]
- Yang, Y.J.; Baleanu, D.; Yang, X.J. Analysis of fractal wave equations by local fractional Fourier series method. Adv. Math. Phys. 2013, 2013, 632309. [Google Scholar] [CrossRef]
- Wang, K.L. A novel perspective to the local fractional bidirectional wave model on Cantor sets. Fractals 2022, 30, 2250107. [Google Scholar] [CrossRef]
- Wang, K.L. A novel perspective to the local fractional Zakharov-Kuznetsov-modified equal width dynamical model on Cantor sets. Math. Methods Appl. Sci. 2022, 46, 622–630. [Google Scholar] [CrossRef]
- Dubey, V.P.; Singh, J.; Alshehri, A.M.; Dubey, S.; Kumar, D. A comparative analysis of two computational schemes for solving local fractional Laplace equations. Math. Methods Appl. Sci. 2021, 44, 13540–13559. [Google Scholar] [CrossRef]
- Su, W.H.; Yang, X.J.; Jafari, H.; Baleanu, D. Fractional complex transform method for wave equations on Cantor sets within local fractional differential operator. Adv. Differ. Equ. 2013, 2013, 1–8. [Google Scholar] [CrossRef]
- Sang, X.; Zhang, Z.; Yang, H.; Han, X. Exact Traveling Wave Solutions of the Local Fractional Bidirectional Propagation System Equations. Fractal Fract. 2022, 6, 653. [Google Scholar] [CrossRef]
- Yang, X.J.; Baleanu, D.; Srivastava, H.M. Local Fractional Integral Transforms and Their Applications; Academic Press: Cambridge, MA, USA, 2015. [Google Scholar]
- Yang, X.J.; Gao, F.; Srivastava, H.M. Non-differentiable exact solutions for the nonlinear ODEs defined on fractal sets. Fractals 2017, 25, 1740002. [Google Scholar] [CrossRef]
- Ghanbari, B. Abundant exact solutions to a generalized nonlinear Schrödinger equation with local fractional derivative. Math. Methods Appl. Sci. 2021, 44, 8759–8774. [Google Scholar] [CrossRef]
- Yang, X.J.; Gasimov, Y.S.; Gao, F.; Allahverdiyeva, N. Travelling-wave solutions for Klein-Gordon and Helmholtz equations on cantor sets. Proc. Inst. Math. Mechanics. 2017, 43, 123–131. [Google Scholar]
- Ghanbari, B. On novel nondifferentiable exact solutions to local fractional Gardner’s equation using an effective technique. Math. Methods Appl. Sci. 2021, 44, 4673–4685. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, K.-J.; Shi, F. A New Perspective on the Exact Solutions of the Local Fractional Modified Benjamin–Bona–Mahony Equation on Cantor Sets. Fractal Fract. 2023, 7, 72. https://doi.org/10.3390/fractalfract7010072
Wang K-J, Shi F. A New Perspective on the Exact Solutions of the Local Fractional Modified Benjamin–Bona–Mahony Equation on Cantor Sets. Fractal and Fractional. 2023; 7(1):72. https://doi.org/10.3390/fractalfract7010072
Chicago/Turabian StyleWang, Kang-Jia, and Feng Shi. 2023. "A New Perspective on the Exact Solutions of the Local Fractional Modified Benjamin–Bona–Mahony Equation on Cantor Sets" Fractal and Fractional 7, no. 1: 72. https://doi.org/10.3390/fractalfract7010072
APA StyleWang, K.-J., & Shi, F. (2023). A New Perspective on the Exact Solutions of the Local Fractional Modified Benjamin–Bona–Mahony Equation on Cantor Sets. Fractal and Fractional, 7(1), 72. https://doi.org/10.3390/fractalfract7010072






