On the Stability Domain of a Class of Linear Systems of Fractional Order
Abstract
1. Introduction
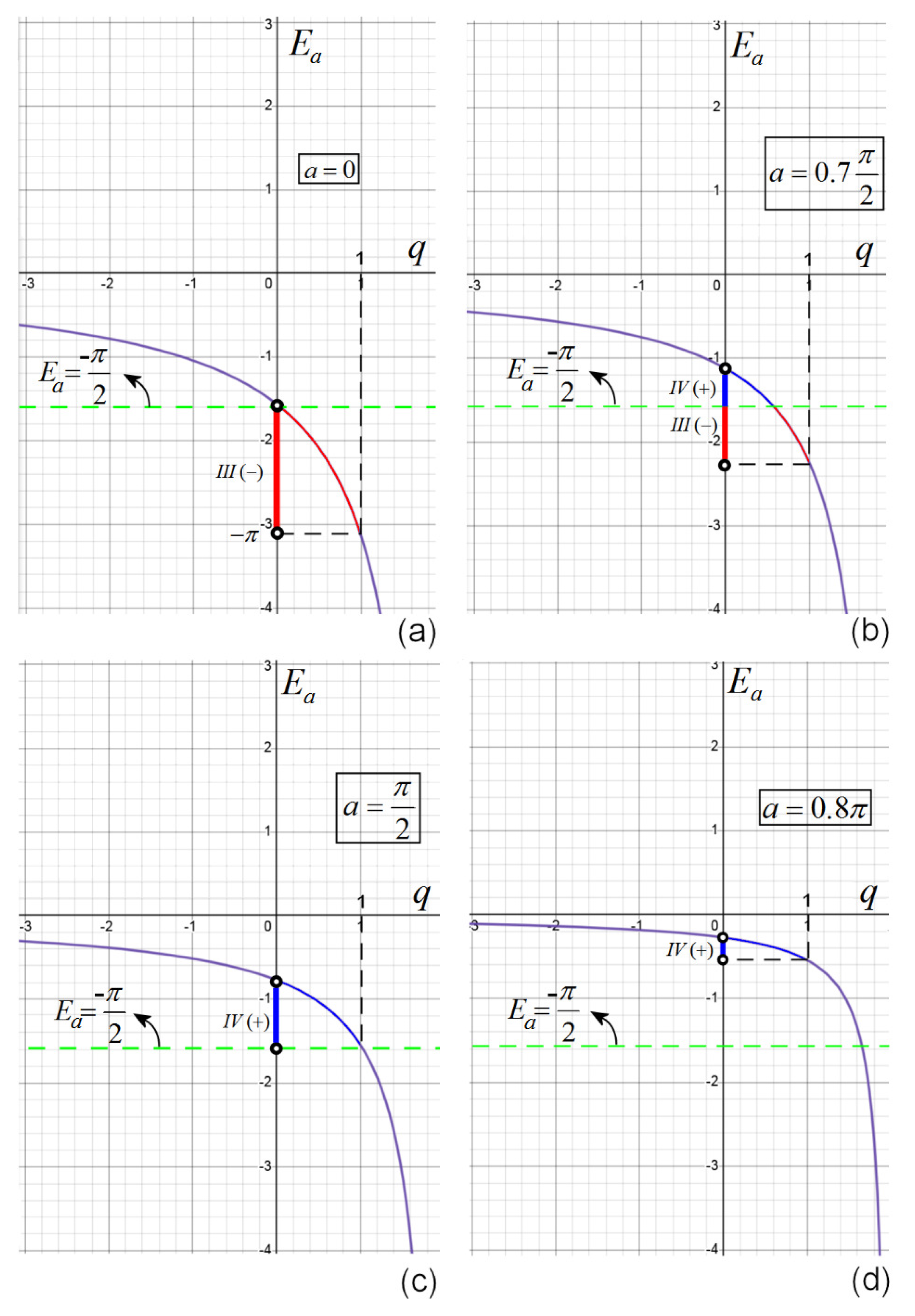
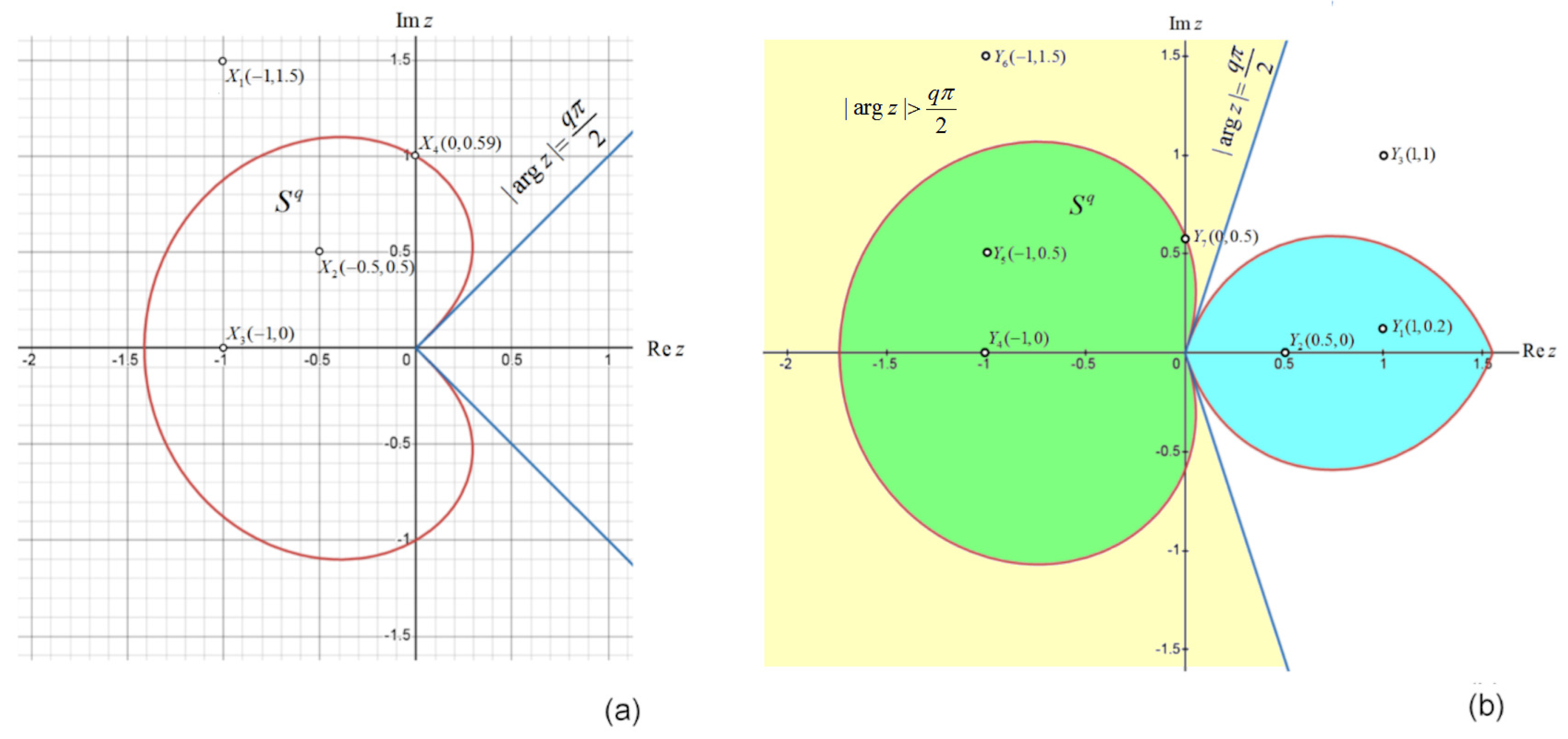
2. On the Shape of the Stability Area
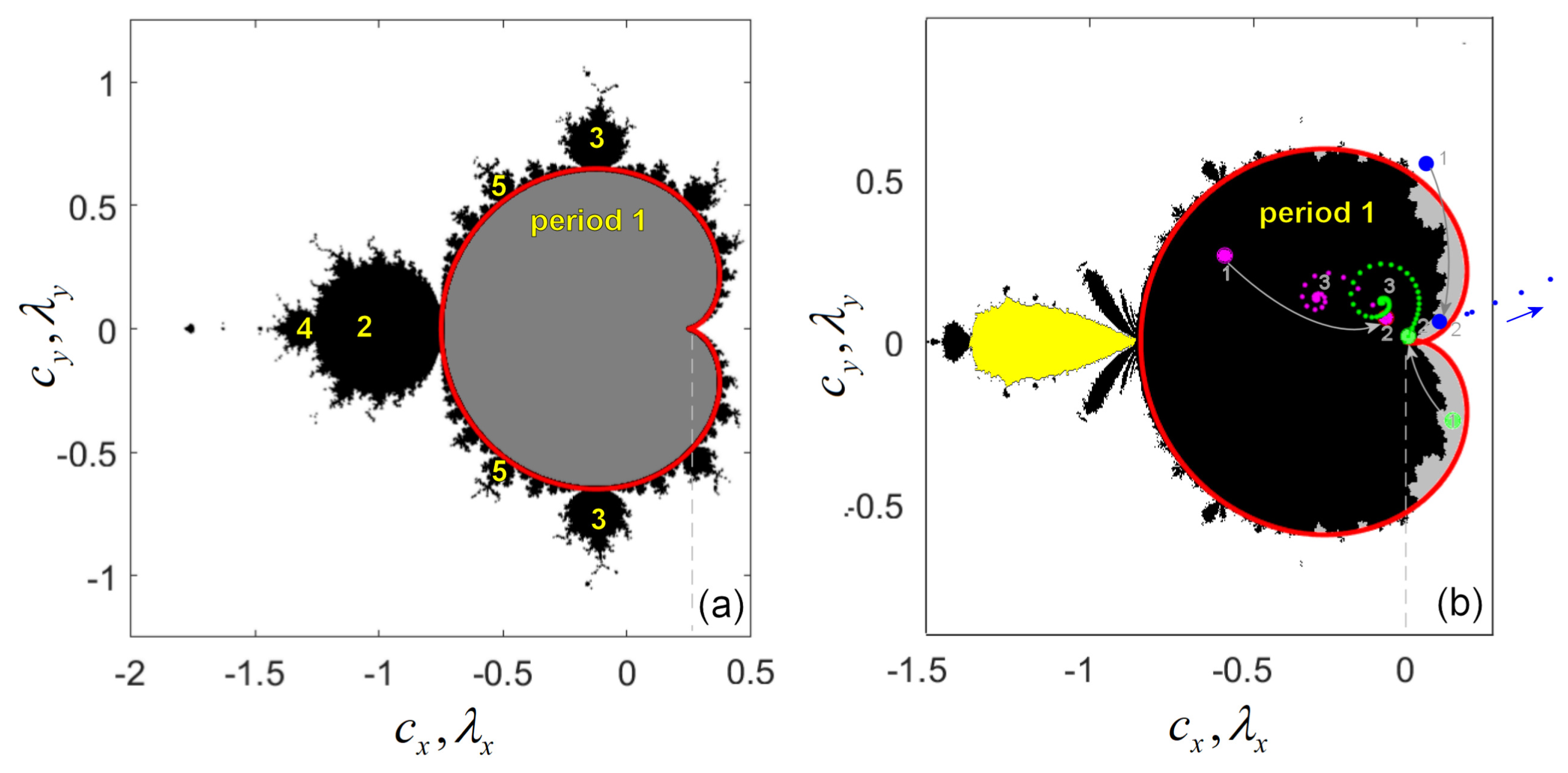
3. Stability of the Mandelbrot Map of Fractional Order
4. Conclusions
Supplementary Materials
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mozyrska, D.; Wyrwas, M. Stability of linear systems with Caputo fractional-, variable-order difference operator of convolution type. In Proceedings of the 2018 41st International Conference on Telecommunications and Signal Processing (TSP), Athens, Greece, 4–6 July 2018. [Google Scholar]
- Čermáak, J.; Kisela, T.; Nechváatal, L. Stability and asymptotic properties of a linear fractional difference equation. Adv. Differ. Equ. 2012, 2012, 122. [Google Scholar] [CrossRef]
- Hilfer, R. Applications of Fractional Calculus in Physics; World Scientific Publishing Company: Singapore, 2000. [Google Scholar]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; North-Holland Mathematics Studies, 204; Elsevier Science B. V.: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Ortigueira, M.D.; Coito, F.J.; Trujillo, J.J. Discrete-time differential systems. Signal Process. 2015, 107, 198–217. [Google Scholar] [CrossRef]
- Ostalczyk, P. Discrete Fractional Calculus. Applications in Control and Image Processing; Series in Computer Vision 4; World Scientific Publishing Co. Pte Ltd.: Singapore, 2016. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; Academic Press: Cambridge, MA, USA, 1999. [Google Scholar]
- Axtell, M.; Bise, E.M. Fractional calculus applications in control systems. In Proceedings of the IEE 1990 IEEE Conference on Aerospace and Electronics, Dayton, OH, USA, 21–25 May 1990; Volume 311, pp. 536–566. [Google Scholar]
- Bastos, N.R.O.; Ferreira, R.A.C.; Torres, D.F.M. Discrete-time fractional variational problems. Signal Process. 2011, 91, 513–524. [Google Scholar] [CrossRef]
- Vinagre, B.M.; Monje, C.A.; Caldero, A.J. Fractional order systems and fractional order actions. In Proceedings of the Tutorial Workshop#2: Fractional Calculus Applications in Automatic Control and Robotics, Las Vegas, NV, USA, 1 December 2002; pp. 1–23. [Google Scholar]
- Bahaa, G.M. Fractional optimal control problem for variable-order differential systems. Fract. Calc. Appl. Anal. 2017, 20, 1447–1470. [Google Scholar] [CrossRef]
- Mozyrska, D.; Ostalczyk, P. Variable-fractional-order Grünwald-Letnikov backward difference selected properties. In Proceedings of the 39th International Conference on Telecommunications and Signal Processing, Vienna, Austria, 27–29 June 2016. [Google Scholar]
- Brandibur, O.; Garrappa, R.; Kaslik, E. Stability of Systems of Fractional-Order Differential Equations with Caputo Derivatives. Mathematics 2021, 9, 914. [Google Scholar] [CrossRef]
- Čermáak, J.; Kisela, T. Asymptotic stability of dynamic equations with two fractional terms: Continuous versus discrete case. Fract. Calc. Appl. Anal. 2015, 18, 437–458. [Google Scholar] [CrossRef]
- Mozyrska, D.; Wyrwas, M. The Z-transform method and delta type fractional difference operators. Discret. Dyn. Nat. Soc. 2015, 2015, 25. [Google Scholar] [CrossRef]
- Sabatier, J.; Farges, M.M.C. LMI stability conditions for fractional order systems. Comput. Mater. Appl. 2010, 59, 1594–1609. [Google Scholar] [CrossRef]
- Goodrich, C.; Peterson, A.C. Discrete Fractional Calculus; Springer International Publishing: Cham, Switzerland, 2015. [Google Scholar]
- Huang, L.-L.; Wu, G.-C.; Baleanu, D.; Wang, H.Y. Discrete fractional calculus for interval-valued systems. Fuzzy Sets Syst. 2021, 404, 141–158. [Google Scholar] [CrossRef]
- Elsonbaty, A.; Elsadany, A.A. On discrete fractional-order Lotka-Volterra model based on the Caputo difference discrete operator. Math. Sci. 2021, 1–13. [Google Scholar] [CrossRef]
- Matignon, D. Stability results on fractional differential equations with applications to control processing. In Proceedings of the Computational Engineering in Systems and Application Multiconference, IMACS, IEEE-SMC, Lille, France, 9–12 July 1996; Volume 2, pp. 963–968. [Google Scholar]
- Sierociuk, D.; Dzielinski, A. Stability of discrete fractional order state-space systems. J. Vib. Control 2008, 14, 1543–1556. [Google Scholar]
- Čermáak, J.; Györi, I.; Nechvátal, L. On explicit stability conditions for a linear fractional difference system. Fract. Calc. Appl. Anal. 2015, 18, 651–672. [Google Scholar] [CrossRef]
- Abdeljawad, T. On delta and nabla Caputo fractional differences and dual Identities, in Recent Developments and Applications on Discrete Fractional Equations and Related Topic. Hindawi 2013, 2013, 406910. [Google Scholar]
- Anastassiou, G. Principles of delta fractional calculus on time scales and inequalities. Math. Comp. Model. 2010, 52, 556–566. [Google Scholar] [CrossRef]
- Abu-Saris, R.; Al-Mdallal, Q. On the asymptotic stability of linear system of fractional-order difference equations. Fract. Calc. Appl. Anal. 2013, 16, 613–629. [Google Scholar] [CrossRef]
- Beebe, N.H.F. The Mathematical-Function Computation Handbook, Programming Using the MathCW Portable Software Library; Springer: Berlin/Heidelberg, Germany, 2017. [Google Scholar]
- Available online: https://www.desmos.com/calculator/pi5ofejgt0?lang=en (accessed on 12 December 2022).
- Available online: https://wiki.analytica.com/index.php?title=Exponentiation_of_negative_numbers#Exponentiation_operator_.5E (accessed on 12 December 2022).
- Chen, F.; Luo, X.; Zhou, Y. Existence results for nonlinear fractional difference equations. Adv. Differ. Equ. 2011, 2011, 713201. [Google Scholar] [CrossRef]
- Atici, F.M.; Eloe, P.W. Initial value problems in discrete fractional calculus. Proc. Am. Math. Soc. 2009, 137, 981–989. [Google Scholar] [CrossRef]
- Fečkan, M.; Danca, M.-F. Non-periodicity of complex Caputo like fractional differences. In Advances in Fractional-Order Neural Networks; Volume II, submitted.
- Available online: https://www.mathworks.com/matlabcentral/fileexchange/121632-fo_mandelbrot (accessed on 12 December 2022).
- Peitgen, H.-O.; Richter, P.H. The Beauty of Fractals Images of Complex Dynamical Systems; Springer: Berlin/Heidelberg, Germany, 1986. [Google Scholar]
- Branner, B. The Mandelbrot Set. In Chaos and Fractals: The Mathematics behind the Computer Graphics; Devaney, R.L., Keen, L., Eds.; Amer Math Soc: Providence, RI, USA, 1989; pp. 75–105. [Google Scholar]
- Peitgen, H.-O.; Saupe, D. (Eds.) The science of Fractal Images; Springer: New York, NY, USA, 1988; pp. 178–179. [Google Scholar]
- Danca, M.-F.; Fečan, M. Mandelbrot set and Julia sets of fractional order. arXiv 2022, arXiv:2210.02037. [Google Scholar]
- Danca, M.-F. Fractional order logistic map: Numerical approach. Chaos Soliton Fract. 2022, 157, 111851. [Google Scholar] [CrossRef]
- Tavazoei, M.S. A note on fractional-order derivatives of periodic functions. Automatica 2010, 46, 945–948. [Google Scholar] [CrossRef]
- Diblík, J.; Fečkan, M.; Pospíšil, M. Nonexistence of periodic solutions and S-asymptotically periodic solutions in fractional difference equations. Appl. Math. Comput. 2015, 257, 230–240. [Google Scholar] [CrossRef]

| z | ||||
| 0.500 | −0.609 | −0.4142 | 0 |
| z | |||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Danca, M.-F. On the Stability Domain of a Class of Linear Systems of Fractional Order. Fractal Fract. 2023, 7, 49. https://doi.org/10.3390/fractalfract7010049
Danca M-F. On the Stability Domain of a Class of Linear Systems of Fractional Order. Fractal and Fractional. 2023; 7(1):49. https://doi.org/10.3390/fractalfract7010049
Chicago/Turabian StyleDanca, Marius-F. 2023. "On the Stability Domain of a Class of Linear Systems of Fractional Order" Fractal and Fractional 7, no. 1: 49. https://doi.org/10.3390/fractalfract7010049
APA StyleDanca, M.-F. (2023). On the Stability Domain of a Class of Linear Systems of Fractional Order. Fractal and Fractional, 7(1), 49. https://doi.org/10.3390/fractalfract7010049







