Spectra of Reduced Fractals and Their Applications in Biology
Abstract
1. Introduction
2. Reduced Fractals in Biology
3. Applications to Biology
3.1. Selected Biological Systems and Their Self-Similarity
3.2. Fractal Dimension and Box-Counting Method
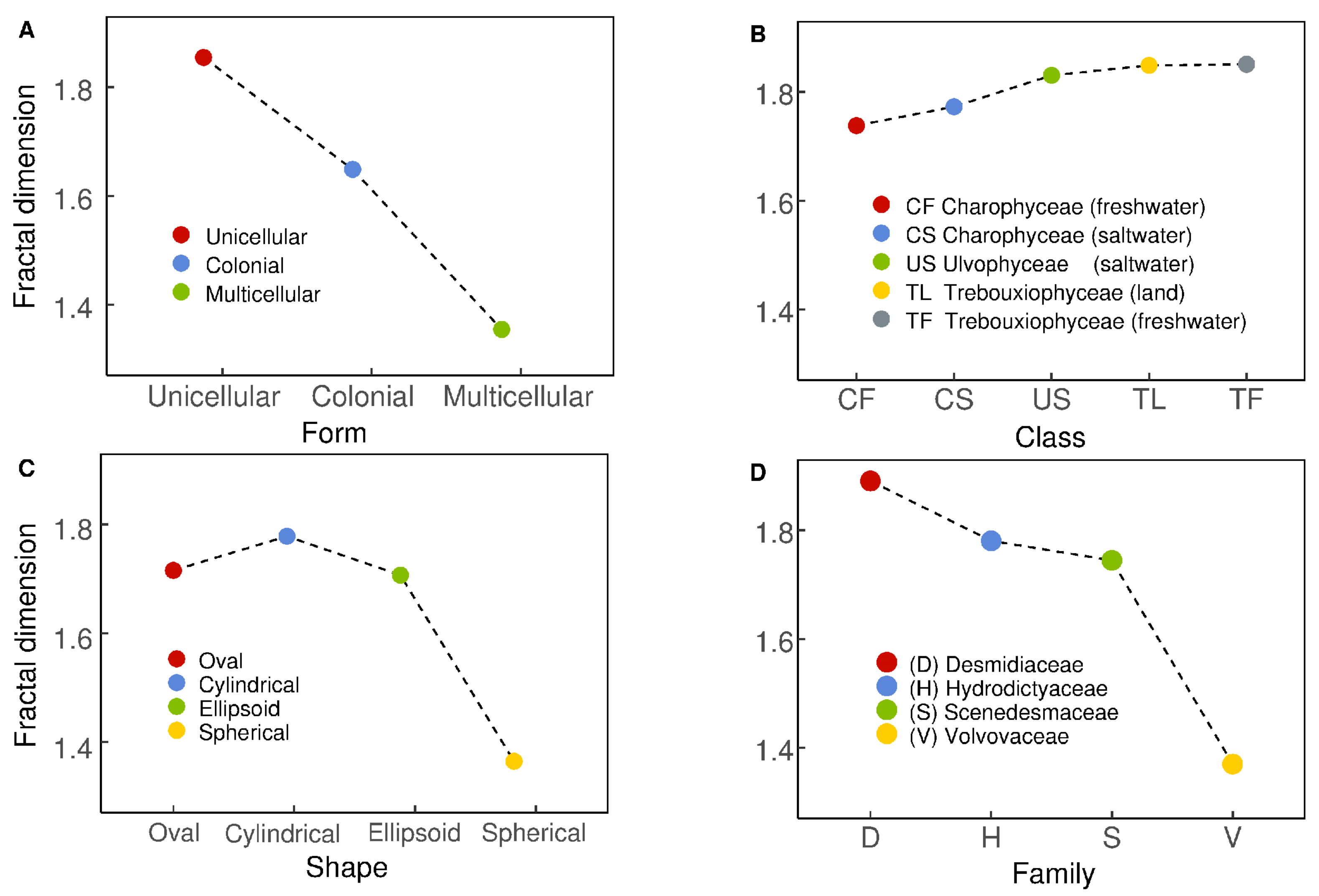
3.3. Fractal Dimension for Selected Algae
3.4. Spectra of Reduced Fractals for Selected Green Algae
3.5. Discussion of the Obtained Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mandelbrot, B. The Fractal Geometry of Nature; W.H. Freeman & Company: San Francisco, CA, USA, 1982. [Google Scholar]
- Schroeder, M. Fractals, Chaos, Power Laws; W.H. Freeman & Company: New York, NY, USA, 1991. [Google Scholar]
- Falconer, K. Fractal Geometry: Mathematical Foundations and Applications; John Wiley & Sons: New York, NY, USA, 1990. [Google Scholar]
- Peitgen, H.-O.; Jürgens, H.; Saupe, D. Chaos and Fractals; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Gouyet, J.-F. Physics and Fractal Structures; Springer: Berlin/Heidelberg, Germany, 1996. [Google Scholar]
- Bunde, A.; Havlin, S. (Eds.) Fractals in Science; Springer: Berlin/Heidelberg, Germany, 1994. [Google Scholar]
- Buldyrev, S.V. Fractals in Biology. In Mathematics of Complexity and Dynamical Systems; Meyers, R., Ed.; Springer: New York, NY, USA, 2012. [Google Scholar]
- Yates, E. Fractal Applications in Biology: Scaling Time in Biochemical Networks; Academic Press, Inc.: New York, NY, USA, 1992. [Google Scholar]
- Crisan, D.; Mina, C.; Voinea, V. Fractal techniques for classifying biologic system. In Curran Associates, Inc. Proceedings; Curran Associates, Inc.: Red Hook, NY, USA, 2009. [Google Scholar]
- Havlin, S.; Buldyrev, S.; Goldberger, A.; Mantegna, R.N.; Ossadnik, S.M.; Peng, C.K.; Simons, M.; Stanley, H.E. Fractals in biology and medicine. Chaos Solitons Fractals 1995, 6, 172–201. [Google Scholar] [CrossRef] [PubMed]
- Losa, G. Fractals and Their Contribution to Biology and Medicine. Medicographia 2012, 34, 365–374. [Google Scholar]
- Newman, T.; Antonovics, J.; Wilbur, H. Population Dynamics with a Refuge: Fractal Basins and the Suppression of Chaos. Theor. Popul. Biol. 2002, 62, 121–128. [Google Scholar] [CrossRef]
- Alados, C.; Pueyo, Y.; Navas, D.; Cabezudo, B.; Gonzalez, A.; Freeman, D.C. Fractal analysis of plant spatial patterns: A monitoring tool for vegetation transition shifts. Biodivers. Conserv. 2005, 14, 1453–1468. [Google Scholar] [CrossRef]
- Aldrich, P.; Horsley, R.; Ahmed, Y.; Williamson, J.J.; Turcic, S.M. Fractal topology of gene promoter networks at phase transitions. Gene Regul. Syst. Biol. 2010, 4, 5389. [Google Scholar] [CrossRef]
- Guevara, M.; Glass, L.; Shrier, A. Phase locking, period-doubling bifurcations, and irregular dynamics in periodically stimulated cardiac cells. Science 1981, 214, 1350–1353. [Google Scholar] [CrossRef] [PubMed]
- Ivanov, P.; Amaral, L.; Goldberger, A.; Havlin, S.; Rosenblum, M.G.; Struzik, Z.R.; Stanley, H.E. Multifractality in human heartbeat dynamics. Nature 1999, 399, 461–465. [Google Scholar] [CrossRef]
- Sernetz, M.; Wubbeke, J.; Wlczek, P. Three-dimensional image analysis and fractal characterization of kidney arterial vessels. Physica A 1992, 191, 13–16. [Google Scholar] [CrossRef]
- Bassingthwaighte, J.; Liebovitch, L.; West, B. Fractal Physiology; Springer: New York, NY, USA, 2013. [Google Scholar]
- Kniffki, K.; Pawlak, M.; Vahle-Hinz, C. Fractal dimensions and dendritic branching of neurons in the somatosensory thalamus. In Fractals in Biology and Medicine; Birkhauser: Basel, Switzerland, 1994; p. 221. [Google Scholar]
- Sztojanov, I.; Crisan; Mina, C.; Voinea, V. Image Processing in Biology Based on Fractal Analysis. In Image Processing; Chen, Y.-S., Ed.; INTECH: Zagreb, Croatia, 2009. [Google Scholar]
- Ieva, A.; Ebstan, F.; Grizzi, F.; Klonowski, W.; Martín-Landrove, M. Fractals in the neurosciences, part II: Clinical applications and future perspectives. Neuroscientist 2015, 21, 30–43. [Google Scholar] [CrossRef]
- Karaca, Y.; Moonis, M.; Baleanu, D. Fractal and multifractal-based predictive optimization model fro stroke subtypes’ classifications. Chaos Solitons Fractals 2020, 136, 199820. [Google Scholar] [CrossRef]
- Liao, S.; Shi, C. International Conference on Intelligent Processing; Beijing, China, 1997; p. 1419. [Google Scholar]
- Ogilvy, J. Theory of Wave Scattering from Random Rough Surfaces; Taylor and Francis: Philadelphia, PA, USA, 1991. [Google Scholar]
- Goff, J. The relationship between local-and global-scale scattering functions for fractal surfaces under a separation of scales hypothesis. J. Acoust. Soc. Am. 1995, 97, 1586–1595. [Google Scholar] [CrossRef]
- van der Hoek, C.; Mann, D.; Jahns, H. Algae An Introduction to Phycology; Cambridge Uni. Press: Cambridge, UK, 1995. [Google Scholar]
- Raven, P.; Evert, R.; Eichhorn, S. Biology of Plants; W.H. Freeman & Company: New York, NY, USA, 2015. [Google Scholar]
- Li, J.; Du, Q.; Sun, C. An improved box-counting method for image fractal dimension estimation. Pattern Recognit. 2009, 42, 2460–2469. [Google Scholar] [CrossRef]
- Gonzato, G. A practical implementation of the box counting algorithm. Comput. Geosci. 1998, 24, 95–100. [Google Scholar] [CrossRef]
- Bouda, M.; Caplan, J.; Saiers, J.E. Box-counting dimension revisited: Presenting an efficient method of minimizing quantization error and an assessment of the self-similarity of structural root systems. Front. Plant Sci. 2016, 7, 149. [Google Scholar] [CrossRef] [PubMed]
- Hooper, G.; Davenport, J. Epifaunal composition and fractal dimensions of intertidal marine macroalgae in relation to emersion. J. Mar. Biol. Assoc. UK 2006, 86, 1297–1304. [Google Scholar] [CrossRef]
- Corbit, J.; Garbary, D.J. Fractal dimension as a quantitative measure of complexity in plant development. Proc. R. Soc. Lond. B 1995, 262, 1–6. [Google Scholar]
- Gee, J.; Warwick, R.M. Metazoan community structure in relation to the fractal dimensions of marine macroalgae. Mar. Ecol. Prog. Ser. 1994, 103, 141–150. [Google Scholar] [CrossRef]
- Fielding, A. Applications of fractal geometry to biology. Bioinformatics 1992, 8, 359–366. [Google Scholar] [CrossRef]
- Yates, F.E. [30] fractal applications in biology: Scaling time in biochemical networks. Methods Enzymol. 1992, 210, 636–675. [Google Scholar]
- Kenkel, N.; Walker, D.J. Fractals in the biological sciences. Coenoses 1996, 11, 77–100. [Google Scholar]
- Kumar, D.; Arjunan, S.; Aliahmad, B. Fractals. Applications in Biological Signalling and Image Processing; CRS Publishing, Taylor and Francis Group: Boca Raton, FL, USA, 2021. [Google Scholar]
- Nai, G.A.; Martelli, C.A.T.; Medina, D.A.L.; de Oliveira, M.S.C.; Caldeira, I.D.; Henriques, B.C.; Marques, M.E.A. Fractal dimension analysis: A new tool for analyzing colony-forming units. MethodsX 2021, 8, 101228. [Google Scholar] [CrossRef] [PubMed]


| Genus sp. | Colony Size | Magnification |
|---|---|---|
| Eudorina elegans | 16, 32 | 2 |
| Desmodesmus magnus | 4, 8, 16 | 3 |
| Scenedesmus granlulatas | 2, 4, 8, 16, 32 | 5 |
| Pediastrum angulosum | 4, 8, 32, 64, 128 | 5 |
| Pediastrum clothratum | 8, 16, 32, 64 | 4 |
| Tetraedron minimum | 2, 4, 8, 16 | 4 |
| Genus Species | Cell Shape | Fractal Dimension |
|---|---|---|
| Euastrum oblongum | Ellipsoid | |
| Euastrum verrucosum | Ellipsoid | |
| Euastrum ansatum | Ellipsoid | |
| Euastrum humerosum | Ellipsoid | |
| Euastrum crissum | Ellipsoid | |
| Micrasterias americana | Spherical | |
| Micrasterias truncata | Spherical | |
| Micrasterias rotata | Spherical |
| Family | Genus sp. | Form | Cell Shape | Fractal Dimension |
|---|---|---|---|---|
| Volvocaceae | Volvox globator | Multicellular | Spherical | 1.2288 |
| Volvocaceae | Volvox aureus | Multicellular | Spherical | 1.3701 |
| Volvocaceae | Eudorina elegans | Colonial | Spherical | 1.6975 |
| Scenedesmaceae | Scenedesmus granulatus | Colonial | Ellipsoid | 1.7097 |
| Crescent | ||||
| Scenedesmaceae | Desmodesmus magnus | Colonial | Ellipsoid | 1.7447 |
| Hydrodictyaceae | Tetraedron minimum | Colonial | Ellipsoid | 1.7087 |
| Spherical | ||||
| Hydrodictyaceae | Pediastrum clothratum | Colonial | Oval | 1.7182 |
| Hydrodictyaceae | Pediastrum angulosum | Colonial | Cylindrical | 1.7806 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pham, D.T.; Musielak, Z.E. Spectra of Reduced Fractals and Their Applications in Biology. Fractal Fract. 2023, 7, 28. https://doi.org/10.3390/fractalfract7010028
Pham DT, Musielak ZE. Spectra of Reduced Fractals and Their Applications in Biology. Fractal and Fractional. 2023; 7(1):28. https://doi.org/10.3390/fractalfract7010028
Chicago/Turabian StylePham, Diana T., and Zdzislaw E. Musielak. 2023. "Spectra of Reduced Fractals and Their Applications in Biology" Fractal and Fractional 7, no. 1: 28. https://doi.org/10.3390/fractalfract7010028
APA StylePham, D. T., & Musielak, Z. E. (2023). Spectra of Reduced Fractals and Their Applications in Biology. Fractal and Fractional, 7(1), 28. https://doi.org/10.3390/fractalfract7010028









