Switching-Jumps-Dependent Quasi-Synchronization Criteria for Fractional-Order Memrisive Neural Networks
Abstract
1. Introduction
2. Preliminaries and Model Description
3. Main Results
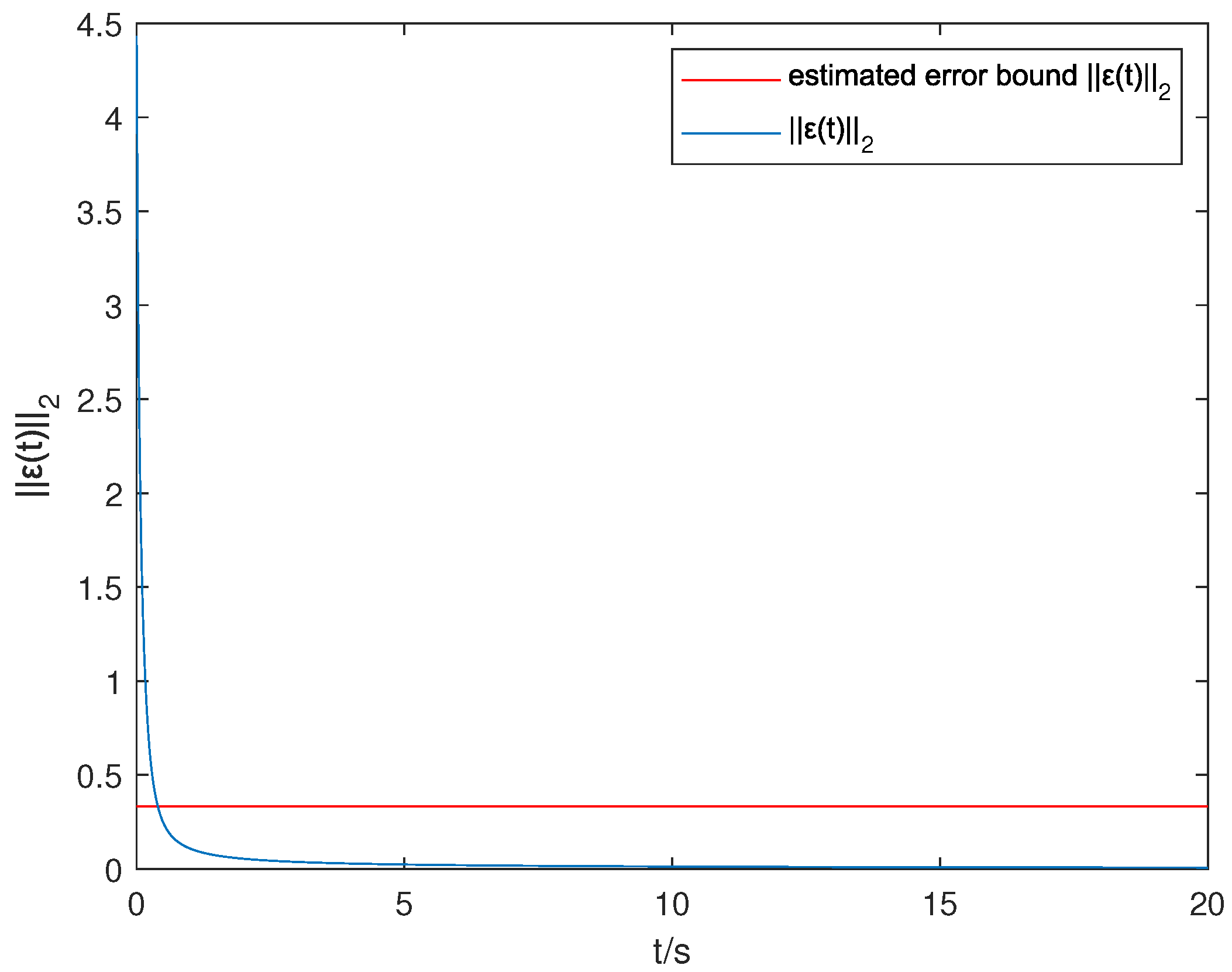
4. Numerical Examples
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| ASTI | asynchronous switching time interval |
| FMNNs | fractional-order memristive neural networks |
| SSTI | asynchronous switching time interval |
| LMI | linear matrix inequality |
References
- Xu, X.; Tan, M.; Corcoran, B.; Wu, J.; Boes, A.; Nguyen, T.G.; Chu, S.T.; Little, B.E.; Hicks, D.G.; Morotti, R.; et al. 11 TOPS photonic convolutional accelerator for optical neural networks. Nature 2021, 589, 44–51. [Google Scholar] [CrossRef]
- Xu, X.; Cao, D.; Zhou, Y.; Gao, J. Application of neural network algorithm in fault diagnosis of mechanical intelligence. Mech. Syst. Signal Process. 2020, 141, 106625. [Google Scholar] [CrossRef]
- Yao, Q.; Wang, R.; Fan, X.; Liu, J.; Li, Y. Multi-class arrhythmia detection from 12-lead varied-length ECG using attention-based time-incremental convolutional neural network. Inf. Fusion 2020, 53, 174–182. [Google Scholar] [CrossRef]
- Wang, M.; Yan, Z.; Wang, T.; Cai, P.; Gao, S.; Zeng, Y.; Wan, C.; Wang, H.; Pan, L.; Yu, J.; et al. Gesture recognition using a bioinspired learning architecture that integrates visual data with somatosensory data from stretchable sensors. Nat. Electron. 2020, 3, 563–570. [Google Scholar] [CrossRef]
- Raissi, M.; Yazdani, A.; Karniadakis, G.E. Hidden fluid mechanics: Learning velocity and pressure fields from flow visualizations. Science 2020, 367, 1026–1030. [Google Scholar] [CrossRef] [PubMed]
- Sun, Y.; Xue, B.; Zhang, M.; Yen, G.G. Evolving deep convolutional neural networks for image classification. IEEE Trans. Evol. Comput. 2020, 24, 394–407. [Google Scholar] [CrossRef]
- Wang, J.; Wang, J.; Han, Q. Multivehicle task assignment based on collaborative neurodynamic optimization with discrete hopfield networks. IEEE Trans. Neural Netw. Learn. Syst. 2021, 32, 5274–5286. [Google Scholar] [CrossRef]
- Kim, B.; Lee, S.; Kim, J. Inverse design of porous materials using artificial neural networks. Sci. Adv. 2020, 6, 1–7. [Google Scholar] [CrossRef]
- Cao, B.; Zhao, J.; Lv, Z.; Gu, Y.; Yang, P.; Halgamuge, S.K. Multiobjective evolution of fuzzy rough neural network via distributed parallelism for stock prediction. IEEE Trans. Fuzzy Syst. 2020, 28, 939–952. [Google Scholar] [CrossRef]
- Zhang, X.; Zhuo, Y.; Luo, Q.; Wu, Z.; Midya, R.; Wang, Z.; Song, W.; Wang, R.; Upadhyay, N.K.; Fang, Y.; et al. An artificial spiking afferent nerve based on Mott memristors for neurorobotics. Nat. Commun. 2020, 11, 51. [Google Scholar] [CrossRef] [PubMed]
- Si, X.; Wang, Z.; Song, Z.; Zhang, Z. Asymptotic Stabilization of Delayed Linear Fractional-Order Systems Subject to State and Control Constraints. Fractal Fract. 2022, 6, 67. [Google Scholar] [CrossRef]
- Yousefpour, A.; Jahanshahi, H.; Munoz-Pacheco, J.M.; Bekiros, S.; Wei, Z. A fractional-order hyper-chaotic economic system with transient chaos. Chaos Solitons Fractals 2020, 130, 109400. [Google Scholar] [CrossRef]
- Tuan, L.A. Neural observer and adaptive fractional-order back-stepping fast terminal sliding mode control of RTG cranes. IEEE Trans. Ind. Electron. 2021, 68, 434–442. [Google Scholar] [CrossRef]
- Chua, L. Memristor-The missing circuit element. IEEE Trans. Circuit Theory 1971, 18, 507–519. [Google Scholar] [CrossRef]
- Strukov, D.B.; Snider, G.S.; Stewart, D.R.; Williams, R.S. The missing memristor found. Nature 2008, 453, 80–83. [Google Scholar] [CrossRef]
- Chen, C.; Zhu, S.; Wei, Y.; Chen, C. Finite-Time stability of delayed memristor-based fractional-order neural networks. IEEE Trans. Cybern. 2020, 50, 1607–1616. [Google Scholar] [CrossRef]
- Chen, L.; Huang, T.; Machado, J.; Lopes, M.; Chai, Y.; Wu, R. Delay-dependent criterion for asymptotic stability of a class of fractional-order memristive neural networks with time-varying delays. Neural Netw. 2019, 118, 289–299. [Google Scholar] [CrossRef]
- Song, C.; Cao, J.; Abdel-Aty, M. New Results on Robust Synchronization for Memristive Neural Networks with Fractional Derivatives via Linear Matrix Inequality. Fractal Fract. 2022, 6, 585. [Google Scholar] [CrossRef]
- Chen, J.; Chen, B.; Zeng, Z. Global asymptotic stability and adaptive ultimate Mittag-Leffler synchronization for a fractional-order complex-valued memristive neural networks with delays. IEEE Trans. Syst. Man Cybern. Syst. 2019, 49, 2519–2535. [Google Scholar] [CrossRef]
- Jia, J.; Zeng, Z.; Wang, F. Pinning synchronization of fractional-order memristor-based neural networks with multiple time-varying delays via static or dynamic coupling. J. Frankl. Inst. 2021, 358, 895–933. [Google Scholar] [CrossRef]
- Ali, M.S.; Hymavathi, M.; Senan, S.; Shekher, V.; Arik, S. Global asymptotic synchronization of impulsive fractional-order complex-valued memristor-based neural networks with time varying delays. Commun. Nonlinear Sci. Numer. Simul. 2019, 78, 104869. [Google Scholar]
- Gu, Y.; Yu, Y.; Wang, H. Projective synchronization for fractional-order memristor-based neural networks with time delays. Neural Comput. Appl. 2019, 31, 6039–6054. [Google Scholar] [CrossRef]
- Xiong, P.; Jahanshahi, H.; Alcaraz, R.; Chu, Y.; Gómez-Aguilar, J.F.; Alsaadi, F.E. Spectral entropy analysis and synchronization of a multi-stable fractional-order chaotic system using a novel neural network-based chattering-free sliding mode technique. Chaos Solitons Fractals 2021, 144, 110576. [Google Scholar] [CrossRef]
- Fan, Y.; Huang, X.; Wang, Z.; Li, Y. Global dissipativity and quasi-synchronization of asynchronous updating fractional-order memristor-based neural networks via interval matrix method. J. Frankl. Inst. 2018, 355, 5998–6025. [Google Scholar] [CrossRef]
- Liu, S.; Yu, Y.; Zhang, S. Robust synchronization of memristor-based fractional-order Hopfield neural networks with parameter uncertainties. Neural Comput. Appl. 2019, 31, 3533–3542. [Google Scholar] [CrossRef]
- Wu, A.; Zeng, Z. Global Mittag-Leffler stabilization of fractional-order memristive neural networks. IEEE Trans. Neural Netw. Learn. Syst. 2017, 28, 206–217. [Google Scholar] [CrossRef] [PubMed]
- Bao, H.; Park, J.H.; Cao, J. Adaptive synchronization of fractional-order memristor-based neural networks with time delay. Nonlinear Dyn. 2015, 82, 1343–1354. [Google Scholar] [CrossRef]
- Chen, L.; Cao, J.; Wu, R.; Machado, J.T.; Lopes, A.M.; Yang, H. Stability and synchro- nization of fractional-order memristive neural networks with multiple delays. Neural Netw. 2017, 94, 76–85. [Google Scholar] [CrossRef]
- Zhang, L.; Yang, Y. Lag synchronization for fractional-order memristive neural networks via period intermittent control. Nonlinear Dyn. 2017, 89, 367–381. [Google Scholar] [CrossRef]
- Huang, T.; Li, C.; Yu, W.; Chen, G. Synchronization of delayed chaotic systems with parameter mismatches by using intermittent linear state feedback. Nonlinearity 2009, 22, 569–584. [Google Scholar] [CrossRef]
- Filippov, A.F. Differential Equations with Discontinuous Righthand Sides; Kluwer Academic Publishers: Boston, MA, USA, 1988. [Google Scholar]
- Henderson, J.; Ouahab, A. Fractional functional differential inclusions with finite delay. Nonlinear Anal. 2009, 70, 2091–2105. [Google Scholar] [CrossRef]
- Aubin, J.P.; Cellina, A. Differential Inclusions: Set-Valued Maps and Viability Theory; Springer: Berlin, Germany, 1984. [Google Scholar]
- Shen, J.; Lam, J. Non-existence of finite-time stable equilibria in fractional-order nonlinear systems. Automatica 2014, 50, 547–551. [Google Scholar] [CrossRef]
- Fan, Y.; Huang, X.; Wang, Z. Local Stabilization of Delayed Fractional-Order Neural Networks Subject to Actuator Saturation. Fractal Fract. 2022, 6, 451. [Google Scholar] [CrossRef]
- Zhang, S.; Yu, Y.; Wang, H. Mittag-Leffler stability of fractional-order Hopfield neural networks. Nonlinear Anal. Hybrid Syst. 2015, 16, 104–121. [Google Scholar] [CrossRef]
- Duarte-Mermoud, M.A.; Aguila-Camacho, N.; Gallegos, J.A.; Castro-Linares, R. Using general quadratic Lyapunov functions to prove Lyapunov uniform stability for fractional order systems. Commun. Nonlinear Sci. Numer. Simul. 2015, 22, 650–659. [Google Scholar] [CrossRef]
- Clarke, F.H.; Ledyaev, Y.S.; Stern, R.J.; Wolenski, P.R. Nonsmooth Analysis and Control Theory; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Wang, D.; Xiao, A.; Liu, H. Dissipativity and stability analysis for fractional functional differential equations. Fract. Calc. Appl. Anal. 2015, 18, 1399–1422. [Google Scholar] [CrossRef]




| Notation | Description |
|---|---|
| the maximum eigenvalue of matrix Q | |
| transpose (or inverse) of matrix Q | |
| * | the symmetric element |
| Q is a positive | |
| (or | definite (or semi-definite) matrix |
| diag(…) | a block diagonal matrix |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fan, Y.; Wei, Z.; Li, M. Switching-Jumps-Dependent Quasi-Synchronization Criteria for Fractional-Order Memrisive Neural Networks. Fractal Fract. 2023, 7, 12. https://doi.org/10.3390/fractalfract7010012
Fan Y, Wei Z, Li M. Switching-Jumps-Dependent Quasi-Synchronization Criteria for Fractional-Order Memrisive Neural Networks. Fractal and Fractional. 2023; 7(1):12. https://doi.org/10.3390/fractalfract7010012
Chicago/Turabian StyleFan, Yingjie, Zhongliang Wei, and Meixuan Li. 2023. "Switching-Jumps-Dependent Quasi-Synchronization Criteria for Fractional-Order Memrisive Neural Networks" Fractal and Fractional 7, no. 1: 12. https://doi.org/10.3390/fractalfract7010012
APA StyleFan, Y., Wei, Z., & Li, M. (2023). Switching-Jumps-Dependent Quasi-Synchronization Criteria for Fractional-Order Memrisive Neural Networks. Fractal and Fractional, 7(1), 12. https://doi.org/10.3390/fractalfract7010012






