New Exact Solutions of Some Important Nonlinear Fractional Partial Differential Equations with Beta Derivative
Abstract
1. Introduction
- ,
- ,
- ,
- ,
2. F-Expansion Method
3. Applications to Fractional Equations with Beta Derivatives
| set1 | set2 | set3 |
| (*) | (**) | (***) |
| set1 | set2 | set3 |
| (*) | (**) | (***) |
4. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Elwakil, S.; El-Labany, S.; Zahran, M.; Sabry, R. Modified extended tanh-function method for solving nonlinear partial differential equations. Phys. Lett. A 2002, 299, 179. [Google Scholar] [CrossRef]
- Abdou, M.; Soliman, A. New applications of variational iteration method. Physica D 2005, 211, 1. [Google Scholar] [CrossRef]
- Wazwaz, A.M. Multiple-soliton solutions for the KP equation by Hirota’s bilinear method and by the tanh–coth method. Appl. Math. Comput. 2007, 190, 633. [Google Scholar] [CrossRef]
- Kudryashov, N.A. One method for finding exact solutions of nonlinear differential equations. Commun. Nonlinear Sci. Numer. Simulat. 2012, 17, 2048. [Google Scholar] [CrossRef]
- Yildirim, O.; Uzun, M. On the numerical solutions of high order stable difference schemes for the hyperbolic multipoint nonlocal boundary value problems. Appl. Math. Comput. 2015, 254, 210. [Google Scholar] [CrossRef]
- Yildirim, O.; Caglak, S. Lie point symmetries of difference equation for nonlinear sine-Gordon equation. Phys. Scr. 2019, 94, 085219. [Google Scholar] [CrossRef]
- Inc, M.; Miah, M.; Chowdhury, A.; Ali, S.; Rezazadeh, H.; Akinlar, M.A.; Chu, Y. New exact solutions for the Kaup-Kupershmidt equation. AIMS Math. 2020, 5, 6726. [Google Scholar] [CrossRef]
- Yildirim, O.; Uzun, M. Weak solvability of the unconditionally stable difference scheme for the coupled sine-Gordon system. Nonlinear Anal. Model. Control 2020, 25, 997. [Google Scholar] [CrossRef]
- Gómez, C.A.; Jhangeer, A.; Rezazadeh, H.; Talarposhti, R.C.; Bekir, A. Closed Form Solutions of the Perturbed Gerdjikov-Ivanov Equation with Variable Coefficients. East Asian J. Appl. Math. 2020, 11, 207. [Google Scholar]
- Hashemi, M.S.; Rezazadeh, H.; Almusawa, H.; Ahmad, H. A Lie group integrator to solve the hydromagnetic stagnation point flow of a second grade fluid over a stretching sheet. AIMS Math. 2021, 6, 13392. [Google Scholar] [CrossRef]
- Leta, T.D.; Liu, W.; Rezazadeh, H.; Ding, J.; Achab, A.E. Analytical Traveling Wave and Soliton Solutions of the (2 + 1) Dimensional Generalized Burgers–Huxley Equation. Qual. Theory Dyn. Syst. 2021, 20, 1–23. [Google Scholar] [CrossRef]
- Gomez-Argular, J.F. Space-time fractional diffusion equation using a derivative with non-singular and regular kernel. Phys. A Stat. Mech. Appl. 2017, 465, 562. [Google Scholar] [CrossRef]
- Kumar, D.; Singh, J.; Baleanu, D. A hybrid computational approach for Klein-Gordon equations on Cantor sets. Nonlinear Dyn. 2017, 87, 511. [Google Scholar] [CrossRef]
- Owolabi, K.M.; Atangana, A. Numerical simulations of chaotic and complex spatiotemporal patterns in fractional reaction diffusion systems. Comput. Appl. Math. 2018, 37, 2166–2189. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Kumar, D.; Singh, J. An efficient analytical technique for fractional model of vibration equation. Appl. Math. Model. 2017, 45, 192. [Google Scholar] [CrossRef]
- Owolabi, K.M.; Atangana, A. Numerical simulation of non-integer order system in subdiffusive, diffusive and superdiffusive scenarios. J. Comput. Nonlinear Dyn. 2017, 12, 1. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Metzler, R.; Klafter, J. The random walks guide to anomalous diffusion: A fractional dynamics approach. Phys. Rep. 2000, 339, 1. [Google Scholar] [CrossRef]
- Caputo, M.; Fabrizio, M. A new definition of fractional derivative without singular kernel. Progr. Fract. Differ. Appl. 2015, 1, 73. [Google Scholar]
- Jumarie, G. Modified Riemann-Liouville derivative and fractional Taylor series of nondifferentiable functions further results. Comput. Math. Appl. 2006, 51, 1367. [Google Scholar] [CrossRef]
- Atangana, A.; Baleanu, D. New fractional derivatives with nonlocal and non-singular kernel: Theory and application to heat transfer model. Therm. Sci. 2016, 20, 763. [Google Scholar] [CrossRef]
- Zhang, S.; Zhang, H.Q. Fractional sub-equation method and its applications to nonlinear fractional PDEs. Phys. Lett. A 2011, 375, 1069. [Google Scholar] [CrossRef]
- Lu, B. The first integral method for some sime fractional differential equations. J. Math. Anal. Appl. 2012, 395, 684. [Google Scholar] [CrossRef]
- Tala-Tebue, E.; Korkmaz, A.; Rezazadeh, H.; Raza, N. New auxiliary equation approach to derive solutions of fractional resonant Schrödinger equation. Anal. Math. Phys. 2021, 11, 167. [Google Scholar] [CrossRef]
- Pandir, Y.; Gurefe, Y.; Misirli, E. New exact solutions of the time-fractional Nonlinear dispersive KdV equation. Int. J. Model. Opt. 2013, 3, 349. [Google Scholar] [CrossRef][Green Version]
- Das, N.; Singh, R.; Wazwaz, A.M.; Kumar, J. An algorithm based on the variational iteration technique for the Bratu-type and the Lane-Emden problems. J. Math. Chem. 2016, 54, 527. [Google Scholar] [CrossRef]
- Zhou, M.; Kanth, A.S.V.R.; Aruna, K.; Raghavendar, K.; Rezazadeh, H.; Inc, M.; Aly, A. Numerical Solutions of Time Fractional Zakharov-Kuznetsov Equation via Natural Transform Decomposition Method with Nonsingular Kernel Derivatives. J. Funct. Spaces 2021, 2021, 9884027. [Google Scholar] [CrossRef]
- Khalil, R.; Horani, O.A.; Yousef, A.; Sabableh, M. A new definition of fractional derivative. J. Comput. Appl. Math. 2014, 264, 65. [Google Scholar] [CrossRef]
- Cenesiz, Y.; Kurt, A. The extented trial equation method for some time-fractional differential equations. Discret. Dyn. Nat. Soc. 2013, 2013, 491359. [Google Scholar]
- Atangana, A.; Baleanu, D.; Alsaedi, A. New properties of conformable derivative. Open Math. 2015, 13, 889–898. [Google Scholar] [CrossRef]
- Atangana, A.; Baleanu, D.; Alsaedi, A. Analysis of time-fractional Hunter-Saxton equation: A model of neumatic liquid crystal. Open Phys. 2016, 14, 145–149. [Google Scholar] [CrossRef]
- Yepez-Martinez, H.; Gomez-Aguilar, J.F.; Atangana, A. First integral method for non-linear differential equations with conformable derivative. Math. Model Nat. Phenom. 2018, 13, 1. [Google Scholar] [CrossRef]
- Zayed, E.M.E.; Amer, Y.A.; Shohib, R.M.A. The Jacobi elliptic function expansion method and its applications for solving the higher order dispersive nonliear Schrödinger equation. Sci. J. Math. Res. 2014, 4, 53–72. [Google Scholar]
- Zayed, E.M.; Al-Nowehy, A.G. Exact solutions for the perturbed nonlinear Schrödinger equation with power law nonlinearity and Hamiltonian perturbed terms. Optik 2017, 139, 123–144. [Google Scholar] [CrossRef]
- Mirzazadeh, M.; Ekici, M.; Sonmezoglu, A. On the Solutions of the Space and Time Fractional Benjamin–Bona–Mahony Equation. Iran. J. Sci. Technol. Trans. A Sci. 2017, 41, 819–836. [Google Scholar] [CrossRef]
- Uddin, M.H.; Akbar, M.A.; Khan, M.A.; Haque, M.A. New exact solitary wave solutions to the space-time fractional differential equations with conformable derivative. AIMS Math. 2019, 4, 199. [Google Scholar] [CrossRef]
- Gurefe, Y. The generalized Kudryashov method for the nonlinear fractional partial differential equations with the beta-derivative. Rev. Mex. Fis. 2020, 66, 771. [Google Scholar] [CrossRef]
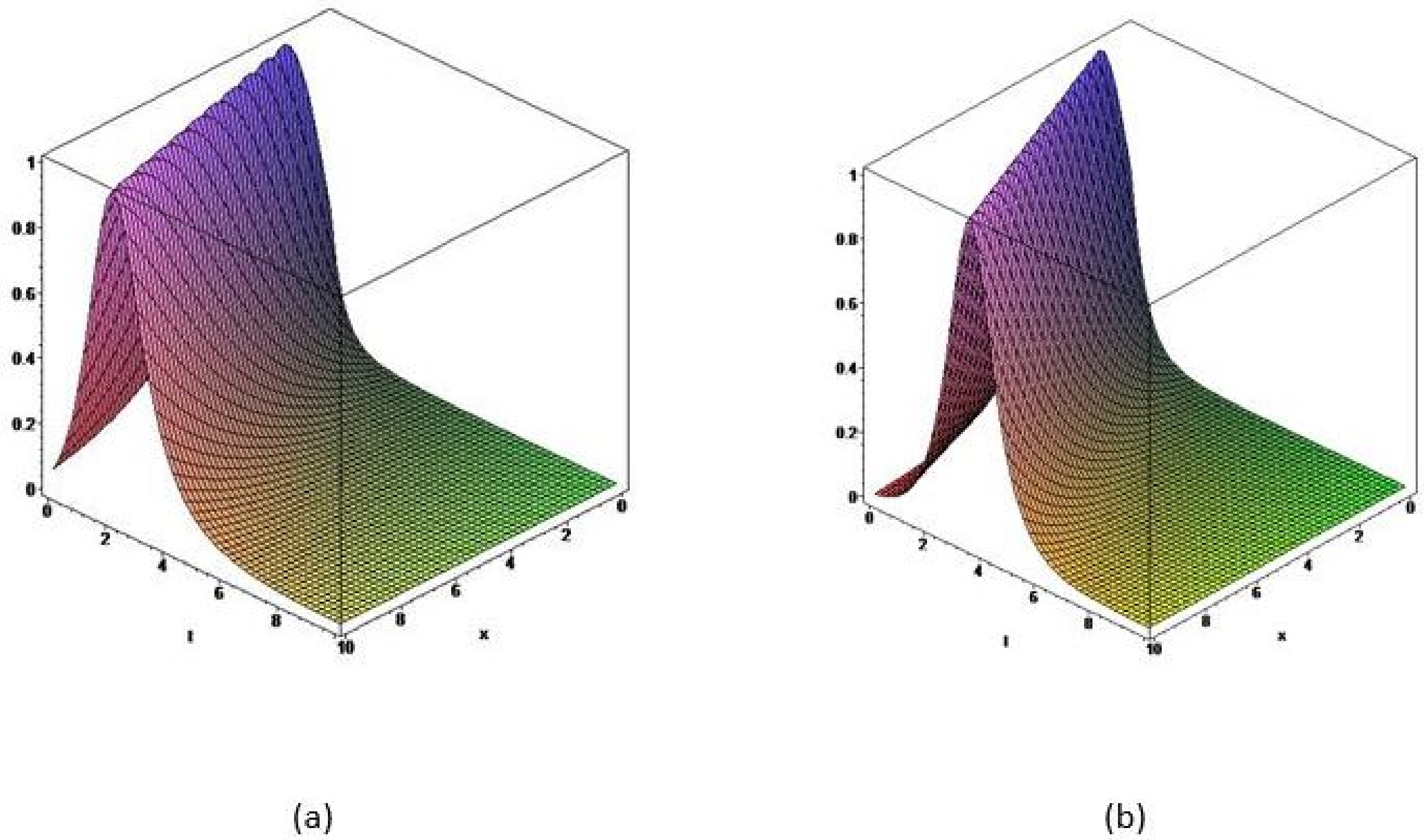
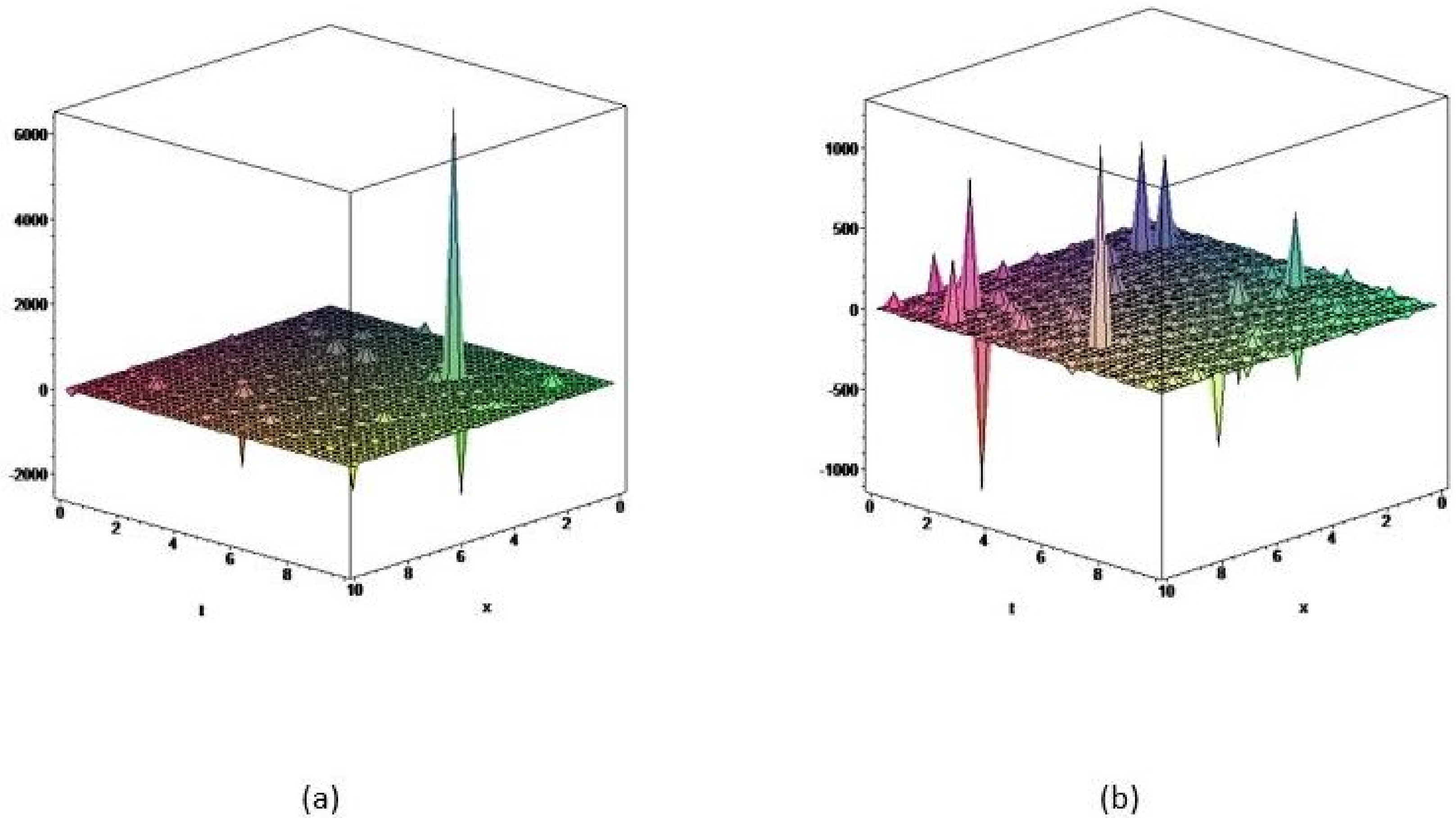
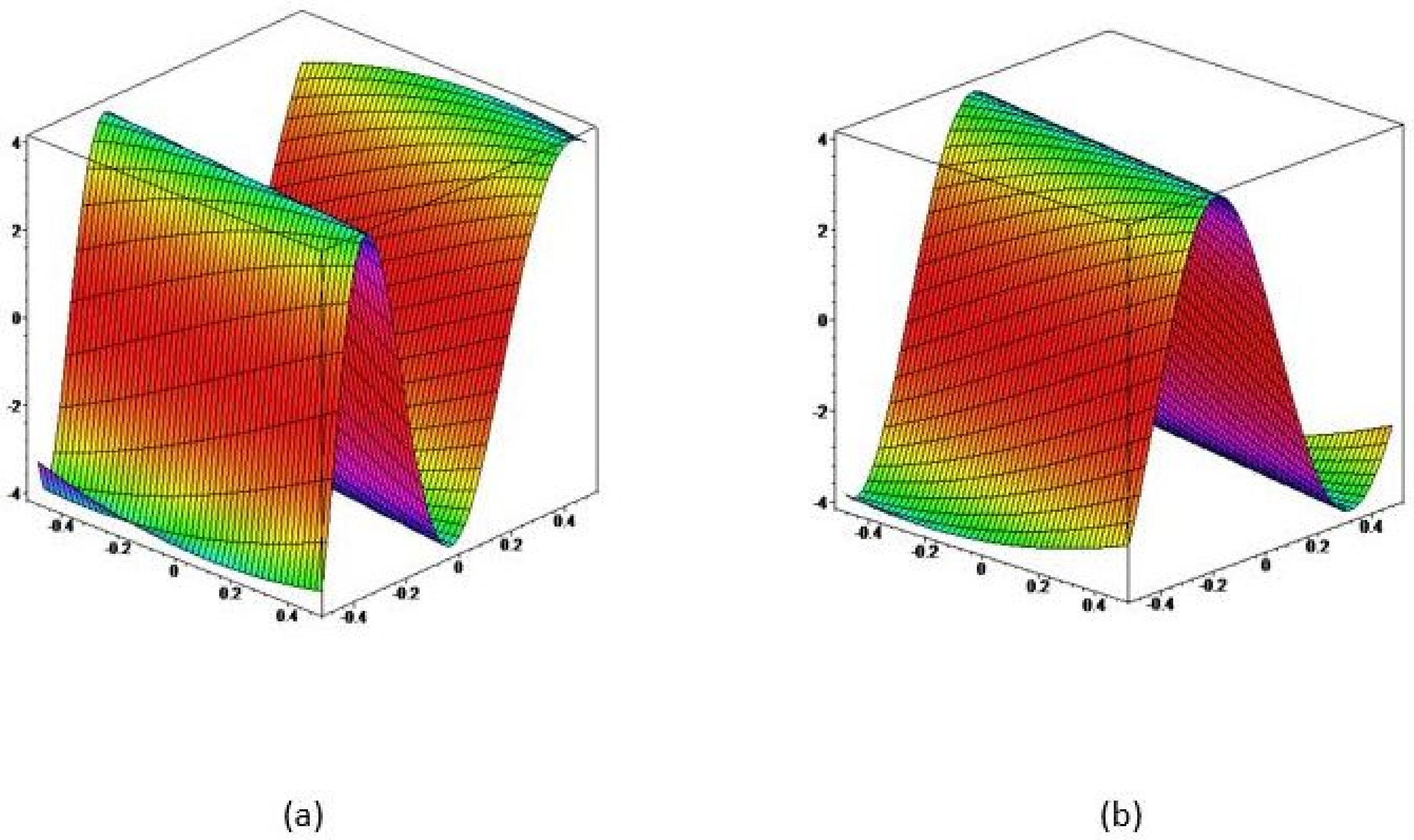

| Case | ) | |||
|---|---|---|---|---|
| 1 | 1 | or | ||
| 2 | cn() | |||
| 3 | −1 | dn() | ||
| 4 | 1 | ns() or dc() | ||
| 5 | nc() | |||
| 6 | −1 | nd() | ||
| 7 | 1 | sc() | ||
| 8 | 1 | sc() | ||
| 9 | 1 | cs() | ||
| 10 | 1 | ds() | ||
| 11 | nc() ± sc() or | |||
| 12 | mcn() ± dn() | |||
| 13 | ||||
| 14 |
| m→ 0 | m→ 1 |
|---|---|
| sn() = | sn() = |
| cd() = ) | cn() = |
| cn() = ) | dn() = |
| ns() = | ns() = |
| cs() = | cs() = |
| ds() = | ds() = |
| sc() = | sc() = |
| sd() = | sd() = |
| nc() = | ns() = ) |
| dn() = 1 | cd() = 1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ozkan, E.M. New Exact Solutions of Some Important Nonlinear Fractional Partial Differential Equations with Beta Derivative. Fractal Fract. 2022, 6, 173. https://doi.org/10.3390/fractalfract6030173
Ozkan EM. New Exact Solutions of Some Important Nonlinear Fractional Partial Differential Equations with Beta Derivative. Fractal and Fractional. 2022; 6(3):173. https://doi.org/10.3390/fractalfract6030173
Chicago/Turabian StyleOzkan, Erdogan Mehmet. 2022. "New Exact Solutions of Some Important Nonlinear Fractional Partial Differential Equations with Beta Derivative" Fractal and Fractional 6, no. 3: 173. https://doi.org/10.3390/fractalfract6030173
APA StyleOzkan, E. M. (2022). New Exact Solutions of Some Important Nonlinear Fractional Partial Differential Equations with Beta Derivative. Fractal and Fractional, 6(3), 173. https://doi.org/10.3390/fractalfract6030173






