Switched Fractional Order Multiagent Systems Containment Control with Event-Triggered Mechanism and Input Quantization
Abstract
:1. Introduction
2. Preliminaries
2.1. Fractional Calculus
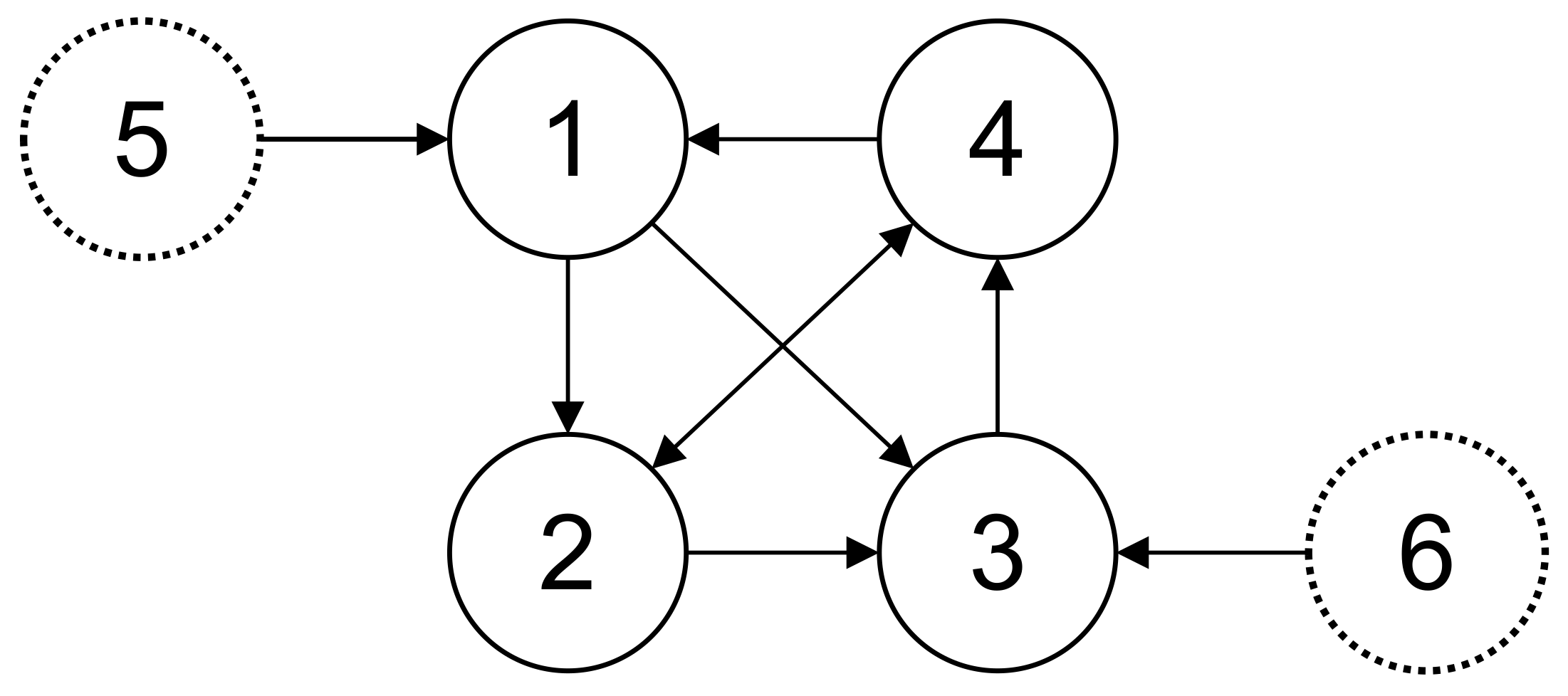
2.2. Problem Formulation
2.3. Hysteresis Quantizer
2.4. Graph Theory
2.5. Neural Network Approximation
3. Main Results
3.1. Observer Design
3.2. Controller Design
3.3. Stability Analysis
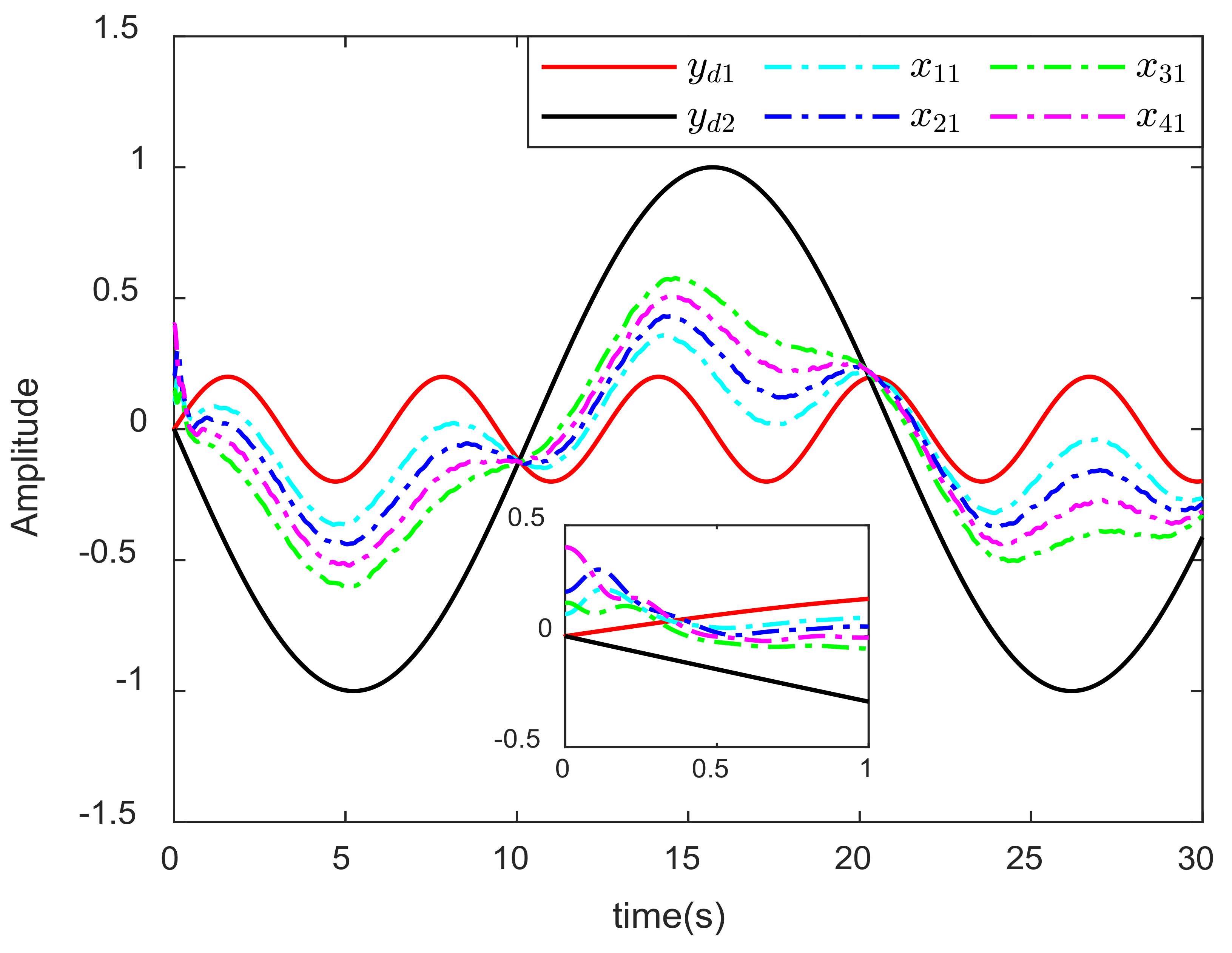
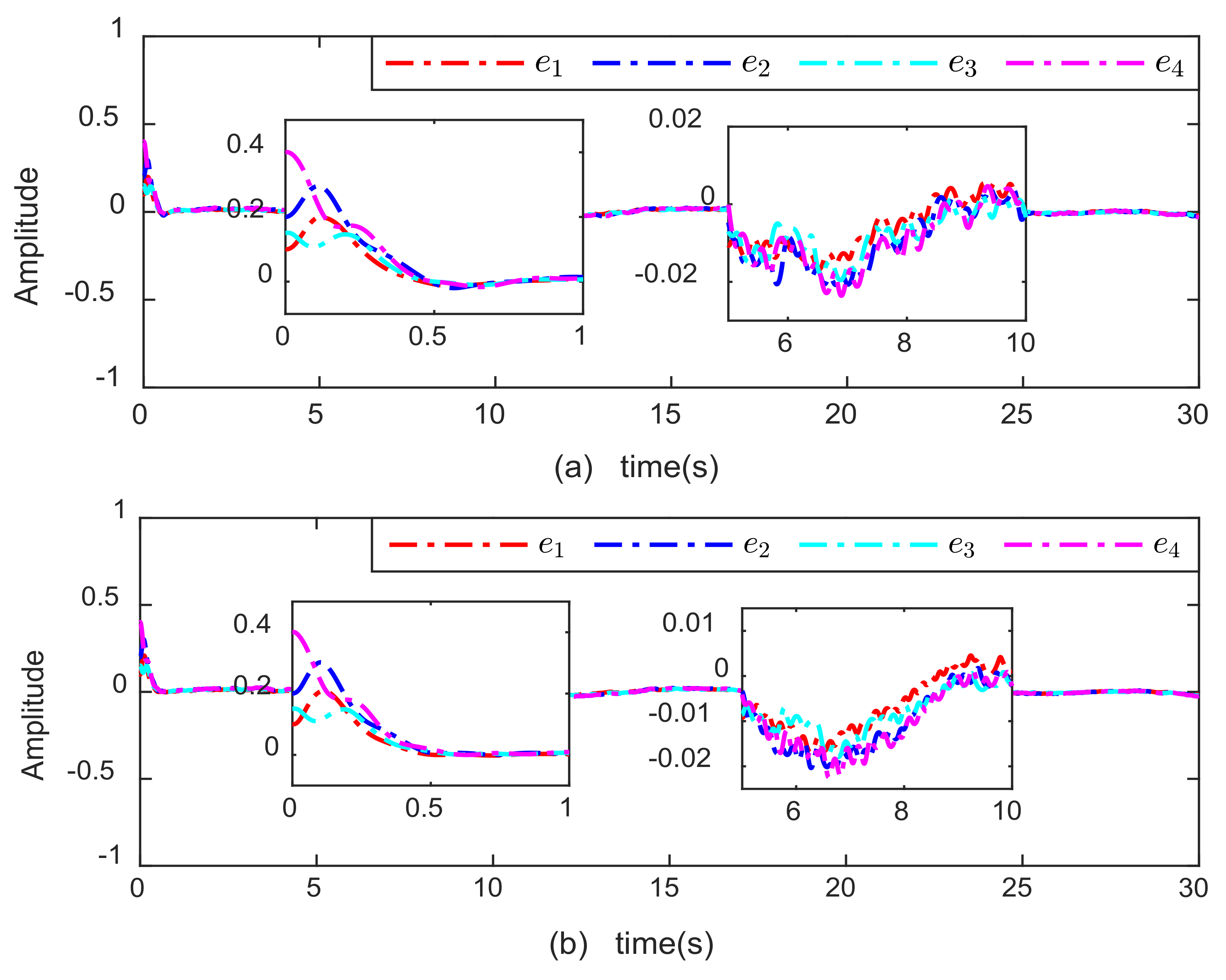
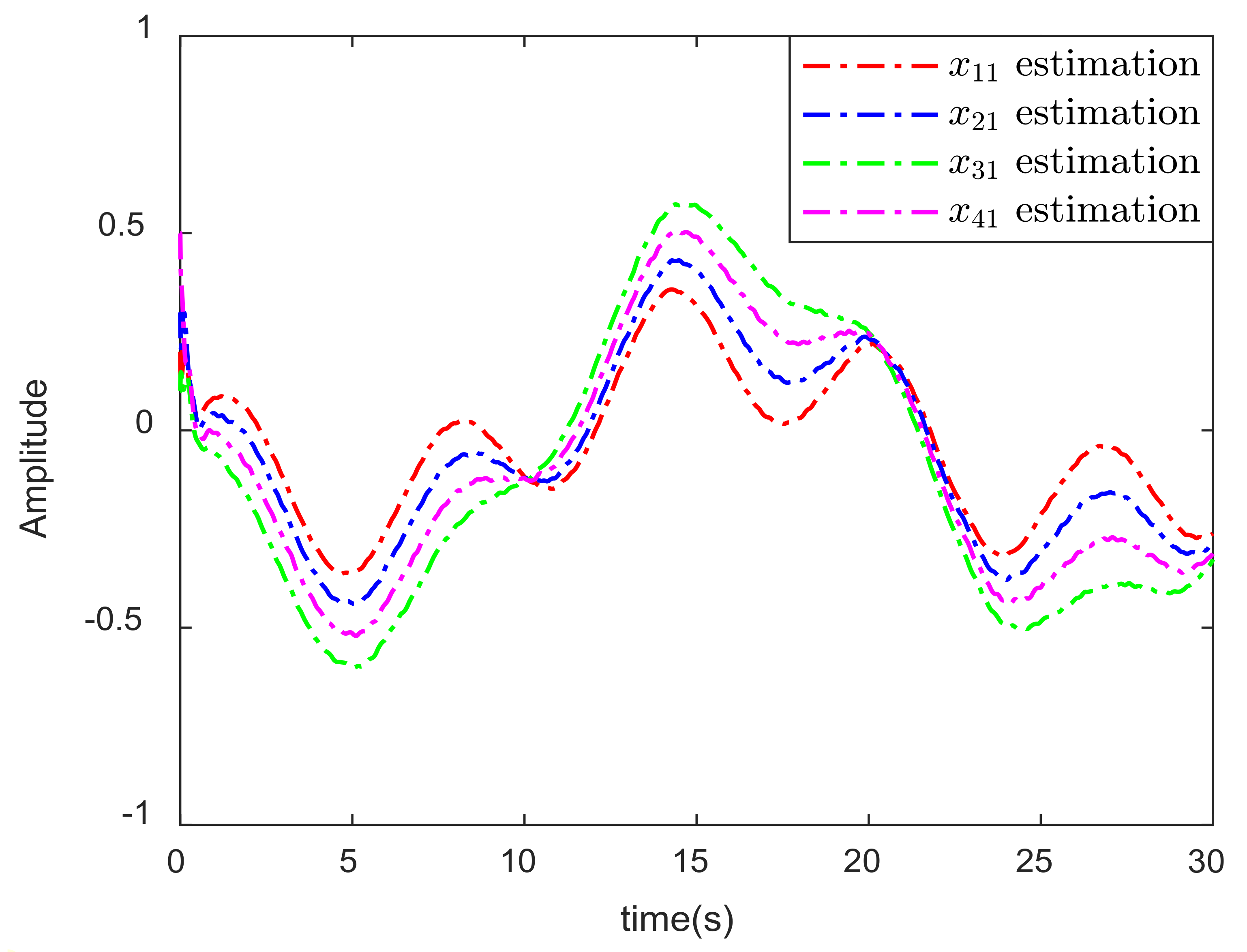
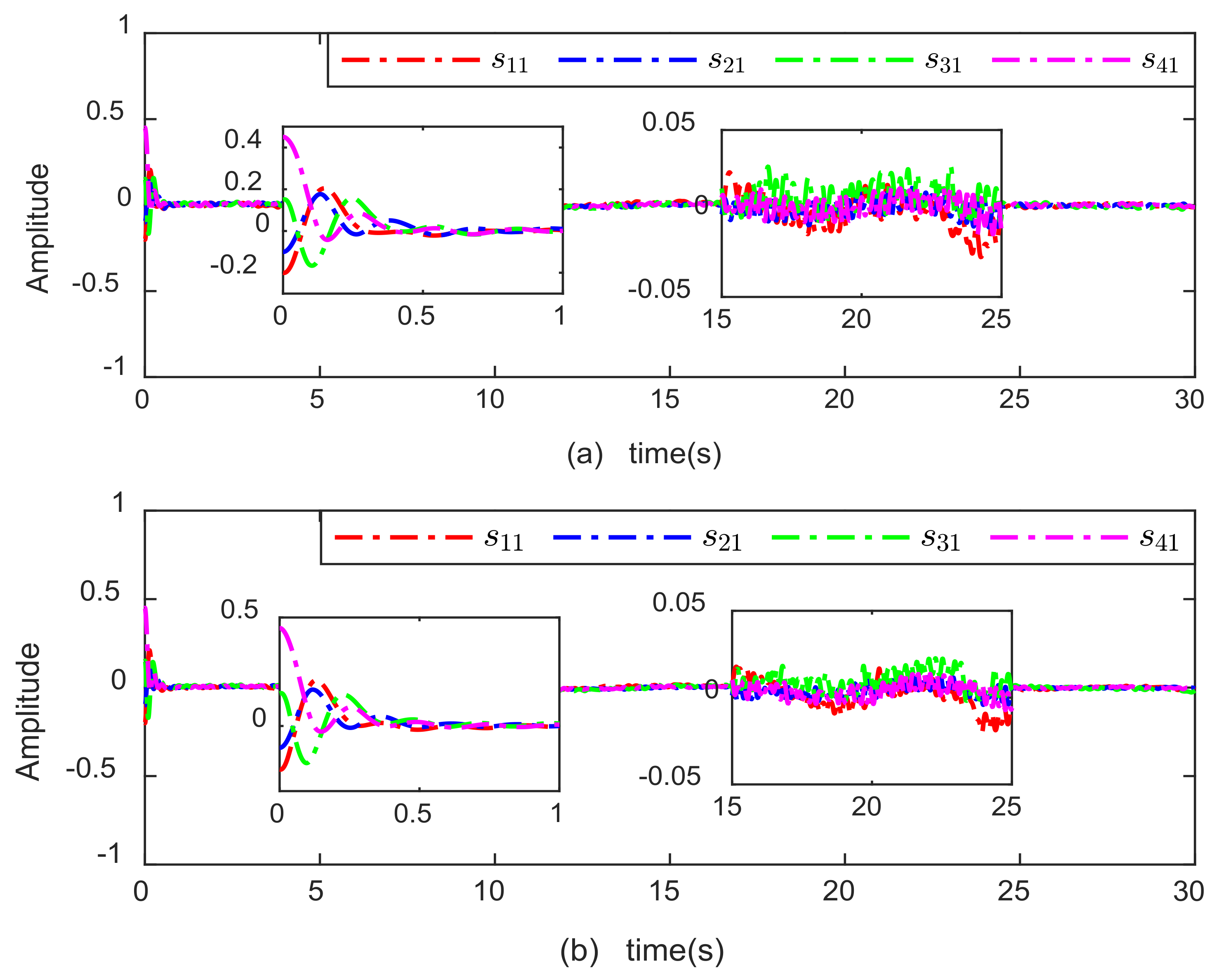
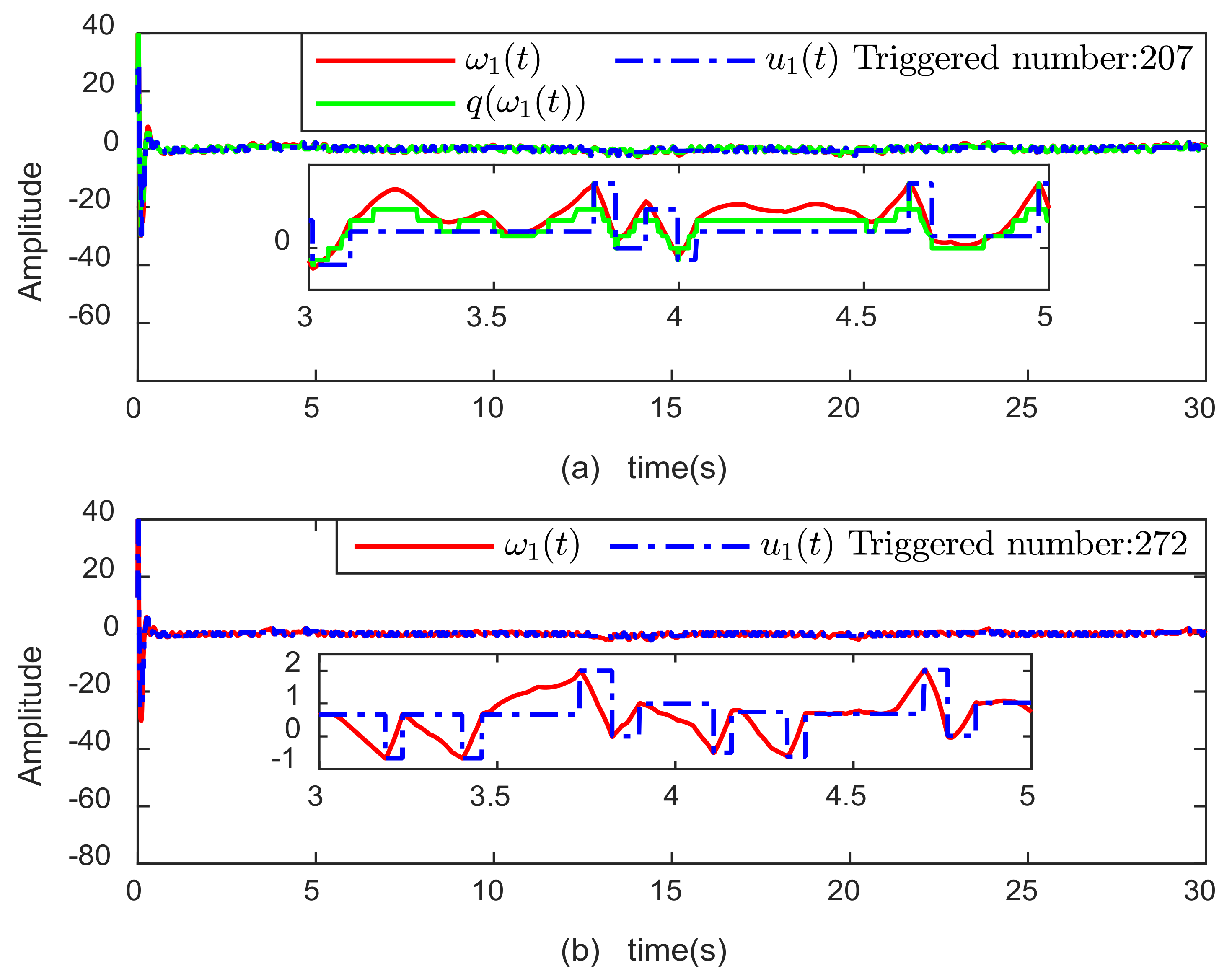
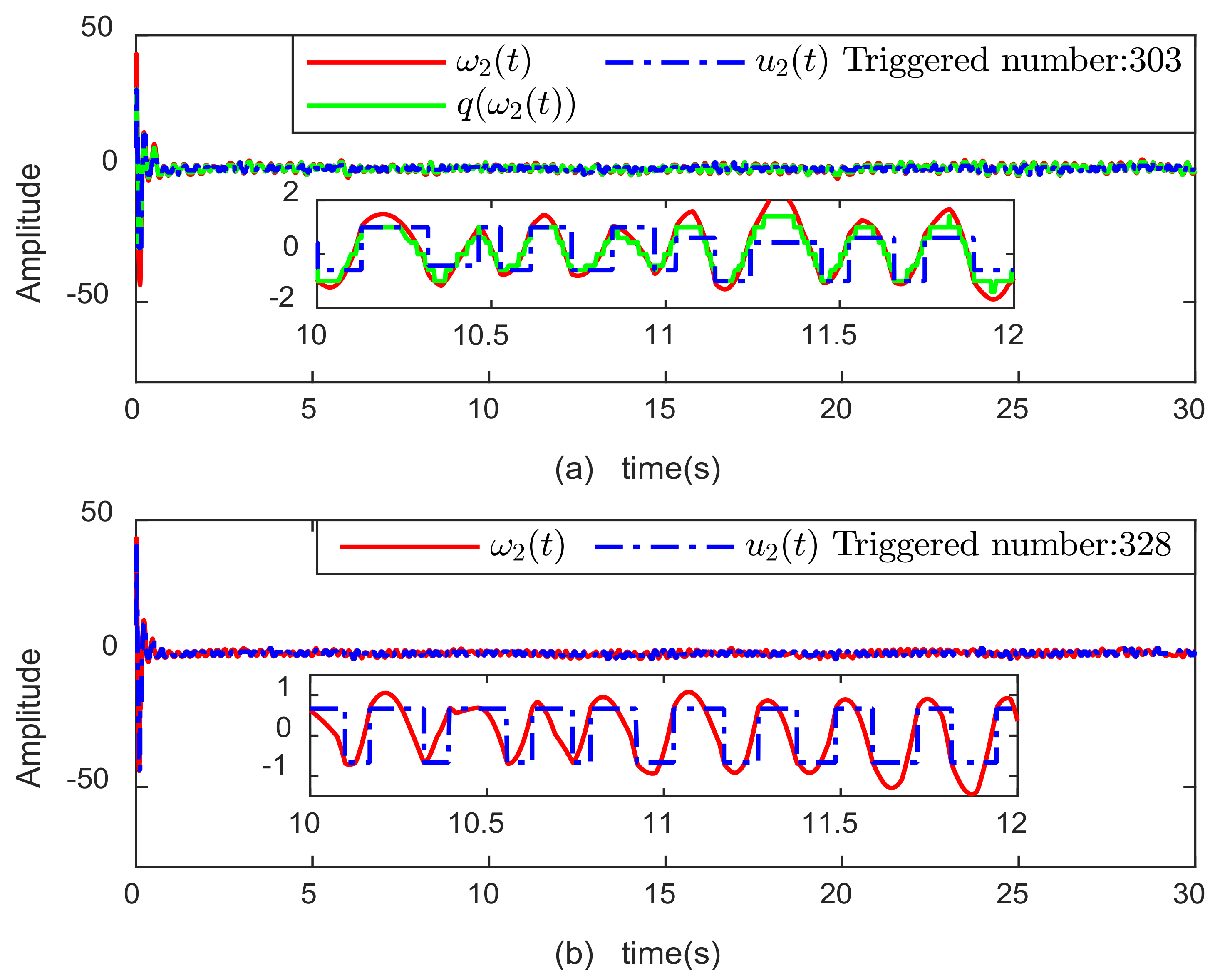
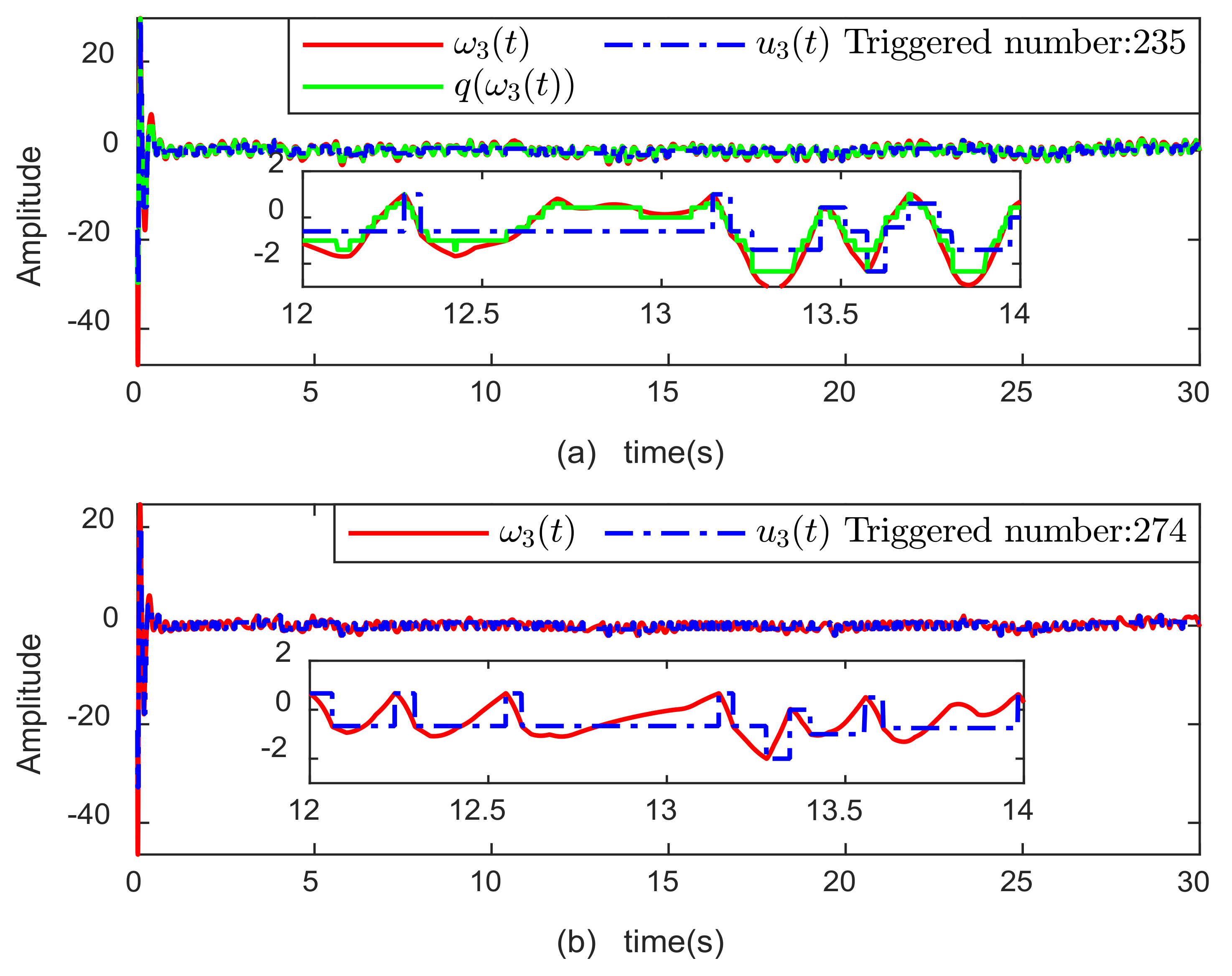
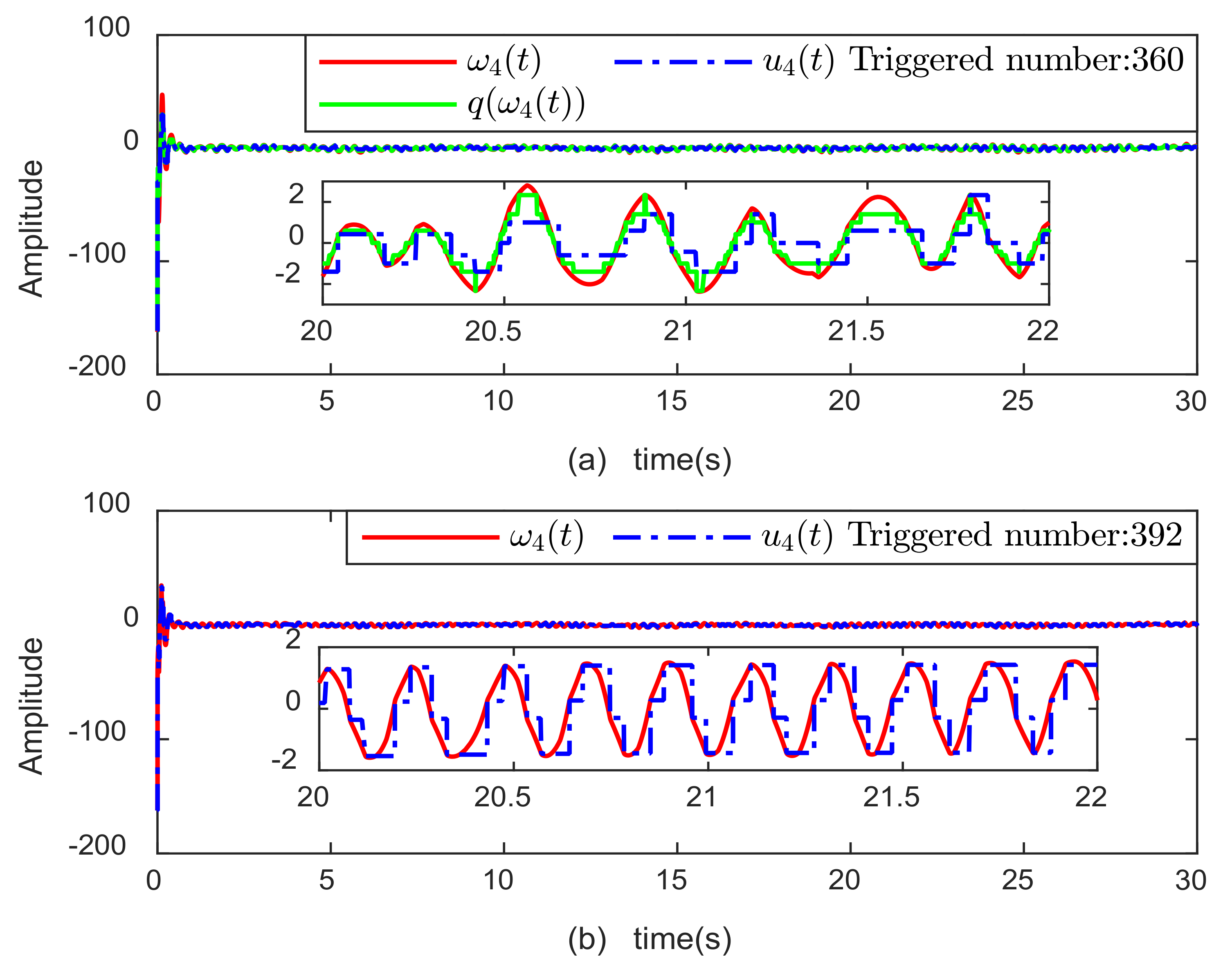
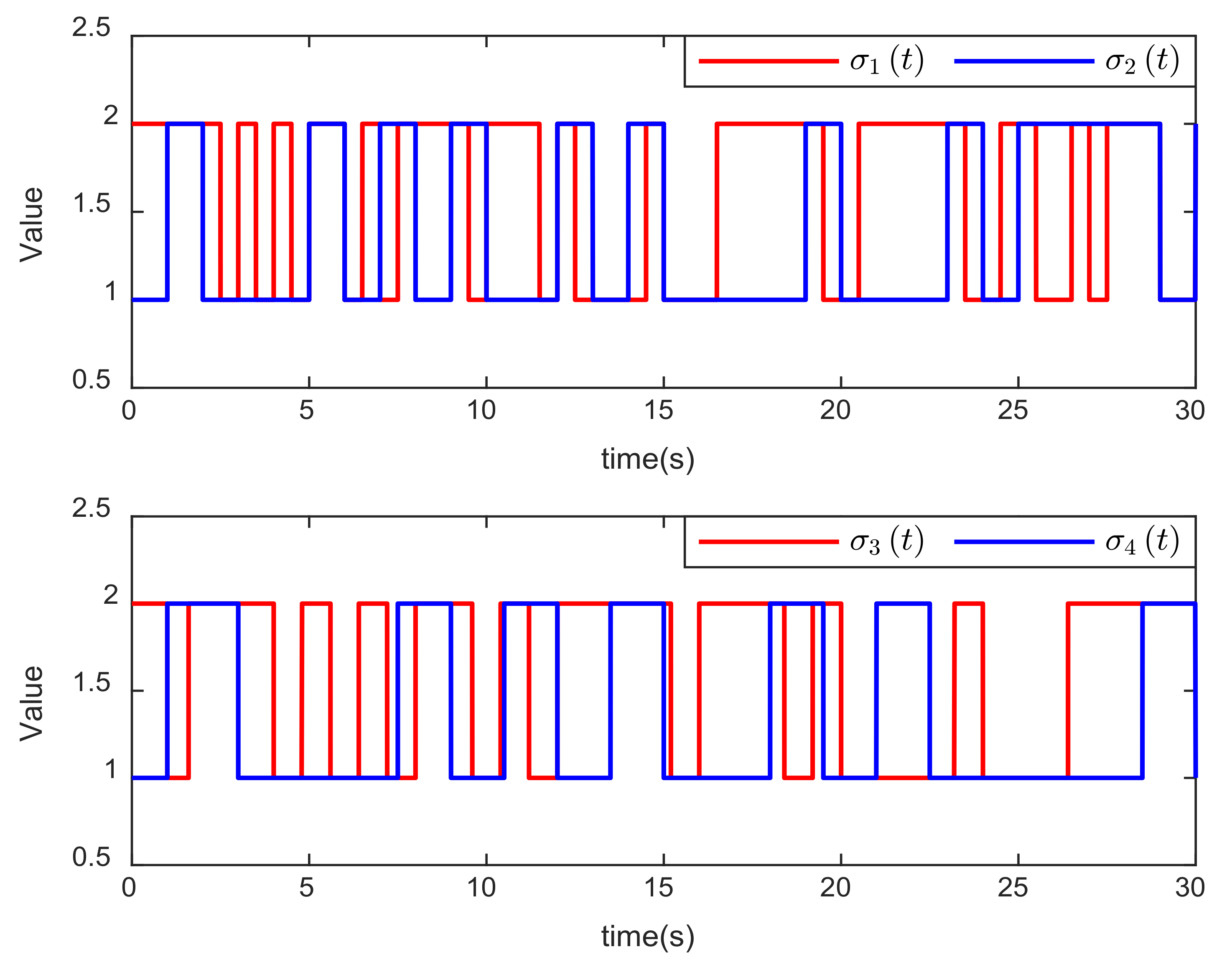
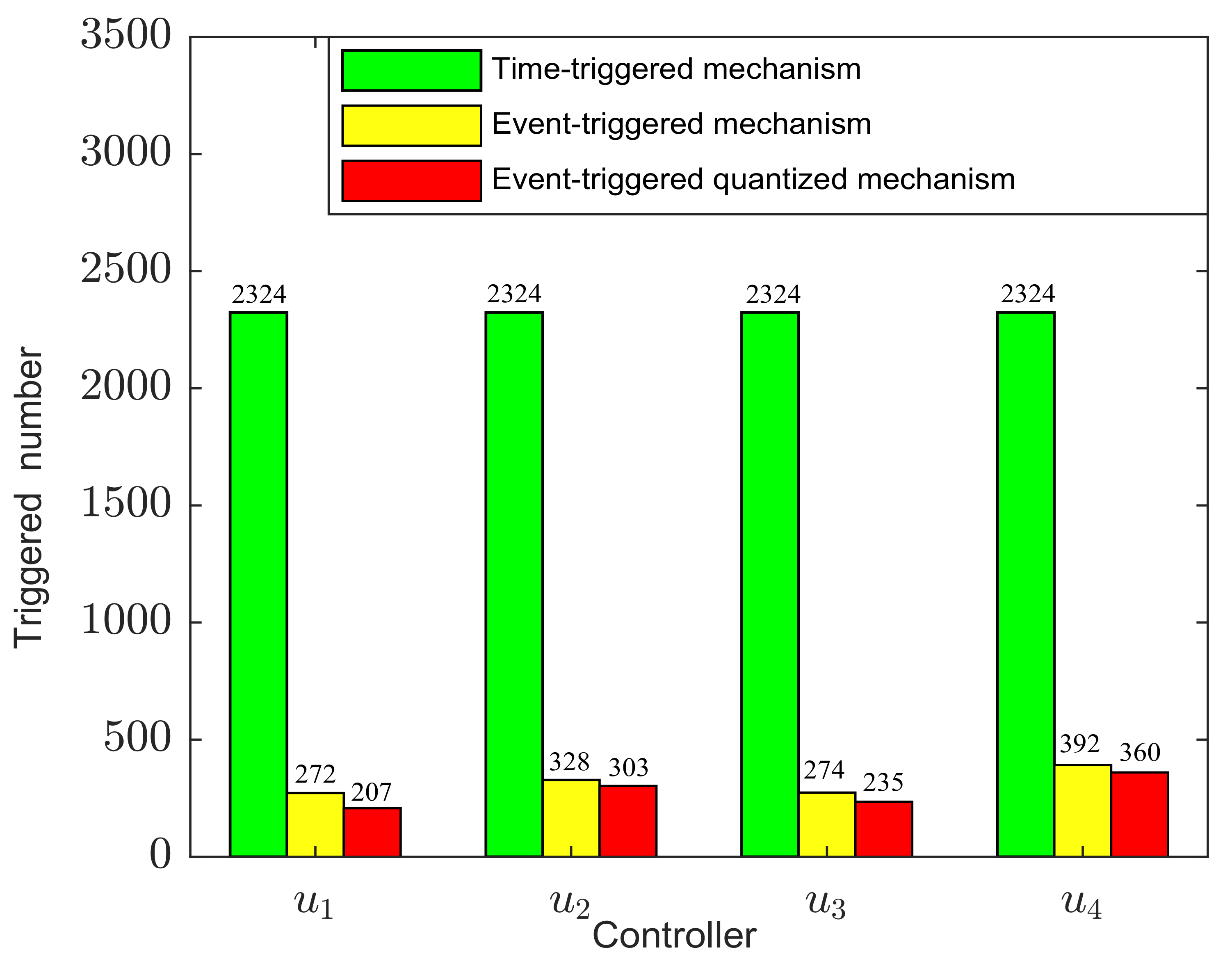
4. Simulation
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sun, F.; Lei, C.; Kurths, J. Consensus of heterogeneous discrete-time multi-agent systems with noise over Markov switching topologies. Int. J. Robust Nonlinear Control 2021, 31, 1530–1541. [Google Scholar] [CrossRef]
- Ma, L.; Wang, Z.; Lam, H.K. Event-triggered mean-square consensus control for time-varying stochastic multi-agent system with sensor saturations. IEEE Trans. Autom. Control 2016, 62, 3524–3531. [Google Scholar] [CrossRef] [Green Version]
- Mao, J.; Yan, T.; Huang, S.; Li, S.; Jiao, J.g. Sampled-data output feedback leader-following consensus for a class of nonlinear multi-agent systems with input unmodeled dynamics. Int. J. Robust Nonlinear Control 2021, 31, 4203–4226. [Google Scholar] [CrossRef]
- Deng, F.; Guo, S.; Zhou, R.; Chen, J. Sensor multifault diagnosis with improved support vector machines. IEEE Trans. Autom. Sci. Eng. 2015, 14, 1053–1063. [Google Scholar] [CrossRef]
- Yao, D.; Dou, C.; Yue, D.; Zhao, N.; Zhang, T. Adaptive neural network consensus tracking control for uncertain multi-agent systems with predefined accuracy. Nonlinear Dyn. 2020, 101, 2249–2262. [Google Scholar] [CrossRef]
- Guo, X.; Liang, H.; Pan, Y. Observer-Based Adaptive Fuzzy Tracking Control for Stochastic Nonlinear Multi-Agent Systems with Dead-Zone Input. Appl. Math. Comput. 2020, 379, 125269. [Google Scholar] [CrossRef]
- Tian, Y.; Xia, Q.; Chai, Y.; Chen, L.; Lopes, A.M.; Chen, Y. Guaranteed Cost Leaderless Consensus Protocol Design for Fractional-Order Uncertain Multi-Agent Systems with State and Input Delays. Fractal Fract. 2021, 5, 141. [Google Scholar] [CrossRef]
- Chen, T.; Yuan, J.; Yang, H. Event-triggered adaptive neural network backstepping sliding mode control of fractional-order multi-agent systems with input delay. J. Vib. Control 2021. [Google Scholar] [CrossRef]
- Yang, Y.; Liu, F.; Yang, H.; Li, Y.; Liu, Y. Distributed Finite-Time Integral Sliding-Mode Control for Multi-Agent Systems with Multiple Disturbances Based on Nonlinear Disturbance Observers. J. Syst. Sci. Complex. 2021, 34, 995–1013. [Google Scholar] [CrossRef]
- Shahvali, M.; Azarbahram, A.; Naghibi-Sistani, M.B.; Askari, J. Bipartite consensus control for fractional-order nonlinear multi-agent systems: An output constraint approach. Neurocomputing 2020, 397, 212–223. [Google Scholar] [CrossRef]
- González, A.; Aragüés, R.; López-Nicolás, G.; Sagüés, C. Weighted predictor-feedback formation control in local frames under time-varying delays and switching topology. Int. J. Robust Nonlinear Control 2020, 30, 3484–3500. [Google Scholar] [CrossRef]
- Cui, G.; Xu, S.; Chen, X.; Lewis, F.L.; Zhang, B. Distributed containment control for nonlinear multiagent systems in pure-feedback form. Int. J. Robust Nonlinear Control 2018, 28, 2742–2758. [Google Scholar] [CrossRef]
- Deng, X.; Cui, Y. Adaptive fuzzy containment control for nonlinear multi-agent systems with input delay. Int. J. Syst. Sci. 2021, 52, 1633–1645. [Google Scholar] [CrossRef]
- Cui, Y.; Liu, X.; Deng, X.; Wang, L. Adaptive Containment Control for Nonlinear Strict-Feedback Multi-Agent Systems with Dynamic Leaders. Int. J. Control 2020, 1–20. [Google Scholar] [CrossRef]
- Li, Y.; Hua, C.; Wu, S.; Guan, X. Output feedback distributed containment control for high-order nonlinear multiagent systems. IEEE Trans. Cybern. 2017, 47, 2032–2043. [Google Scholar] [CrossRef]
- Parsa, M.; Danesh, M. Containment control of high-order multi-agent systems with heterogeneous uncertainties, dynamic leaders, and time delay. Asian J. Control 2021, 23, 799–810. [Google Scholar] [CrossRef]
- Pan, H.; Yu, X.; Yang, G.; Xue, L. Robust consensus of fractional-order singular uncertain multi-agent systems. Asian J. Control 2020, 22, 2377–2387. [Google Scholar] [CrossRef]
- Lü, H.; He, W.; Han, Q.L.; Ge, X.; Peng, C. Finite-time containment control for nonlinear multi-agent systems with external disturbances. Inf. Sci. 2020, 512, 338–351. [Google Scholar] [CrossRef]
- Xu, C.; Liao, M.; Li, P.; Yao, L.; Qin, Q.; Shang, Y. Chaos Control for a Fractional-Order Jerk System via Time Delay Feedback Controller and Mixed Controller. Fractal Fract. 2021, 5, 257. [Google Scholar] [CrossRef]
- Jahanzaib, L.S.; Trikha, P.; Matoog, R.T.; Muhammad, S.; Al-Ghamdi, A.; Higazy, M. Dual Penta-Compound Combination Anti-Synchronization with Analysis and Application to a Novel Fractional Chaotic System. Fractal Fract. 2021, 5, 264. [Google Scholar] [CrossRef]
- İlknur, K.; AKÇETİN, E.; Yaprakdal, P. Numerical approximation for the spread of SIQR model with Caputo fractional order derivative. Turk. J. Sci. 2020, 5, 124–139. [Google Scholar]
- Dokuyucu, M.A. Caputo and atangana-baleanu-caputo fractional derivative applied to garden equation. Turk. J. Sci. 2020, 5, 1–7. [Google Scholar]
- Butt, S.I.; Nadeem, M.; Farid, G. On Caputo fractional derivatives via exponential s-convex functions. Turk. J. Sci. 2020, 5, 140–146. [Google Scholar]
- Zhao, S.; Butt, S.I.; Nazeer, W.; Nasir, J.; Umar, M.; Liu, Y. Some Hermite–Jensen–Mercer type inequalities for k-Caputo-fractional derivatives and related results. Adv. Differ. Equ. 2020, 2020, 262. [Google Scholar] [CrossRef]
- Baleanu, D.; Fernandez, A.; Akgül, A. On a fractional operator combining proportional and classical differintegrals. Mathematics 2020, 8, 360. [Google Scholar] [CrossRef] [Green Version]
- Sabir, Z.; Raja, M.A.Z.; Guirao, J.L.; Shoaib, M. A novel design of fractional Meyer wavelet neural networks with application to the nonlinear singular fractional Lane-Emden systems. Alex. Eng. J. 2021, 60, 2641–2659. [Google Scholar] [CrossRef]
- Jiang, J.; Chen, H.; Cao, D.; Guirao, J.L. The global sliding mode tracking control for a class of variable order fractional differential systems. Chaos Solitons Fractals 2022, 154, 111674. [Google Scholar] [CrossRef]
- Chen, J.; Guan, Z.H.; Yang, C.; Li, T.; He, D.X.; Zhang, X.H. Distributed containment control of fractional-order uncertain multi-agent systems. J. Frankl. Inst. 2016, 353, 1672–1688. [Google Scholar] [CrossRef]
- Yuan, X.L.; Mo, L.P.; Yu, Y.G.; Ren, G.J. Distributed containment control of fractional-order multi-agent systems with double-integrator and nonconvex control input constraints. Int. J. Control Autom. Syst. 2020, 18, 1728–1742. [Google Scholar] [CrossRef]
- Yang, W.; Yu, W.; Zheng, W.X. Fault-Tolerant Adaptive Fuzzy Tracking Control for Nonaffine Fractional-Order Full-State-Constrained MISO Systems With Actuator Failures. IEEE Trans. Cybern. 2021, 1–14. [Google Scholar] [CrossRef]
- Gong, P.; Lan, W. Adaptive robust tracking control for multiple unknown fractional-order nonlinear systems. IEEE Trans. Cybern. 2018, 49, 1365–1376. [Google Scholar] [CrossRef]
- Wang, Y.; Yuan, Y.; Liu, J. Finite-time leader-following output consensus for multi-agent systems via extended state observer. Automatica 2021, 124, 109133. [Google Scholar] [CrossRef]
- Yuan, X.; Mo, L.; Yu, Y. Observer-based quasi-containment of fractional-order multi-agent systems via event-triggered strategy. Int. J. Syst. Sci. 2019, 50, 517–533. [Google Scholar] [CrossRef]
- Huo, X.; Ma, L.; Zhao, X.; Zong, G. Observer-based fuzzy adaptive stabilization of uncertain switched stochastic nonlinear systems with input quantization. J. Frankl. Inst. 2019, 356, 1789–1809. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, Z. Stability and robust stabilization of uncertain switched fractional order systems. ISA Trans. 2020, 103, 1–9. [Google Scholar] [CrossRef]
- Tang, X.; Zhai, D.; Fu, Z.; Wang, H. Output Feedback Adaptive Fuzzy Control for Uncertain Fractional-Order Nonlinear Switched System with Output Quantization. Int. J. Fuzzy Syst. 2020, 22, 943–955. [Google Scholar] [CrossRef]
- Li, Y.; Tong, S. Adaptive neural networks prescribed performance control design for switched interconnected uncertain nonlinear systems. IEEE Trans. Neural Netw. Learn. Syst. 2017, 29, 3059–3068. [Google Scholar] [CrossRef]
- Sui, S.; Chen, C.L.P.; Tong, S. Neural-Network-Based Adaptive DSC Design for Switched Fractional-Order Nonlinear Systems. IEEE Trans. Neural Netw. Learn. Syst. 2021, 32, 4703–4712. [Google Scholar] [CrossRef]
- Liu, W.; Ma, Q.; Xu, S.; Zhang, Z. Adaptive finite-time event-triggered control for nonlinear systems with quantized input signals. Int. J. Robust Nonlinear Control 2021, 31, 4764–4781. [Google Scholar] [CrossRef]
- Liu, G.; Pan, Y.; Lam, H.K.; Liang, H. Event-triggered fuzzy adaptive quantized control for nonlinear multi-agent systems in nonaffine pure-feedback form. Fuzzy Sets Syst. 2021, 416, 27–46. [Google Scholar] [CrossRef]
- Choi, Y.H.; Yoo, S.J. Quantized-Feedback-Based Adaptive Event-Triggered Control of a Class of Uncertain Nonlinear Systems. Mathematics 2020, 8, 1603. [Google Scholar] [CrossRef]
- Xing, X.; Liu, J. Event-triggered neural network control for a class of uncertain nonlinear systems with input quantization. Neurocomputing 2021, 440, 240–250. [Google Scholar] [CrossRef]
- Zhou, Q.; Wang, W.; Liang, H.; Basin, M.V.; Wang, B. Observer-Based Event-Triggered Fuzzy Adaptive Bipartite Containment Control of Multiagent Systems With Input Quantization. IEEE Trans. Fuzzy Syst. 2021, 29, 372–384. [Google Scholar] [CrossRef]
- Li, Y.; Chen, Y.; Podlubny, I. Mittag–Leffler stability of fractional order nonlinear dynamic systems. Automatica 2009, 45, 1965–1969. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications; Elsevier: Amsterdam, The Netherlands, 1998. [Google Scholar]
- Duarte-Mermoud, M.A.; Aguila-Camacho, N.; Gallegos, J.A.; Castro-Linares, R. Using general quadratic Lyapunov functions to prove Lyapunov uniform stability for fractional order systems. Commun. Nonlinear Sci. Numer. Simul. 2015, 22, 650–659. [Google Scholar] [CrossRef]
- Gao, H.; Zhang, T.; Xia, X. Adaptive neural control of stochastic nonlinear systems with unmodeled dynamics and time-varying state delays. J. Frankl. Inst. 2014, 351, 3182–3199. [Google Scholar] [CrossRef]
- Wang, X.; Chen, Z.; Yang, G. Finite-time-convergent differentiator based on singular perturbation technique. IEEE Trans. Autom. Control 2007, 52, 1731–1737. [Google Scholar] [CrossRef]
- Liu, W.; Lim, C.C.; Shi, P.; Xu, S. Backstepping fuzzy adaptive control for a class of quantized nonlinear systems. IEEE Trans. Fuzzy Syst. 2016, 25, 1090–1101. [Google Scholar] [CrossRef]
- Algahtani, O.J.J. Comparing the Atangana–Baleanu and Caputo–Fabrizio derivative with fractional order: Allen Cahn model. Chaos Solitons Fractals 2016, 89, 552–559. [Google Scholar] [CrossRef]
- Caputo, M.; Fabrizio, M. A new definition of fractional derivative without singular kernel. Prog. Fract. Differ. Appl. 2015, 1, 1–13. [Google Scholar]
- Deepika, D.; Kaur, S.; Narayan, S. Uncertainty and disturbance estimator based robust synchronization for a class of uncertain fractional chaotic system via fractional order sliding mode control. Chaos Solitons Fractals 2018, 115, 196–203. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuan, J.; Chen, T. Switched Fractional Order Multiagent Systems Containment Control with Event-Triggered Mechanism and Input Quantization. Fractal Fract. 2022, 6, 77. https://doi.org/10.3390/fractalfract6020077
Yuan J, Chen T. Switched Fractional Order Multiagent Systems Containment Control with Event-Triggered Mechanism and Input Quantization. Fractal and Fractional. 2022; 6(2):77. https://doi.org/10.3390/fractalfract6020077
Chicago/Turabian StyleYuan, Jiaxin, and Tao Chen. 2022. "Switched Fractional Order Multiagent Systems Containment Control with Event-Triggered Mechanism and Input Quantization" Fractal and Fractional 6, no. 2: 77. https://doi.org/10.3390/fractalfract6020077
APA StyleYuan, J., & Chen, T. (2022). Switched Fractional Order Multiagent Systems Containment Control with Event-Triggered Mechanism and Input Quantization. Fractal and Fractional, 6(2), 77. https://doi.org/10.3390/fractalfract6020077






