Impact of Non-Uniform Periodic Magnetic Field on Unsteady Natural Convection Flow of Nanofluids in Square Enclosure
Abstract
1. Introduction
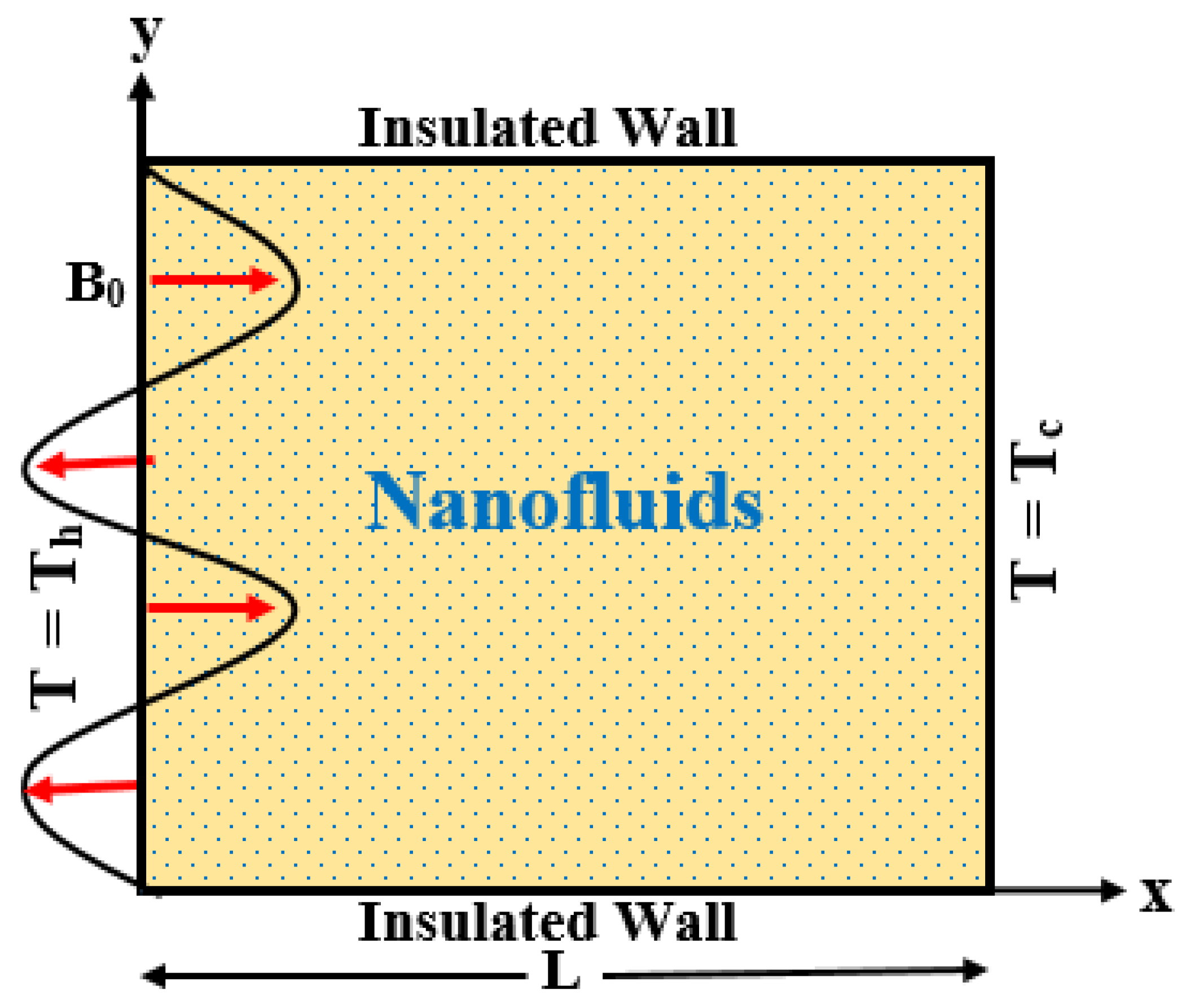
2. Physical and Mathematical Model
2.1. Dimensional Boundary Conditions
2.2. Physical and Thermal Properties of Nanofluids
2.3. Dimensional Analysis
3. Computational Procedure
3.1. Grid Independence Test
3.2. Validation of Code
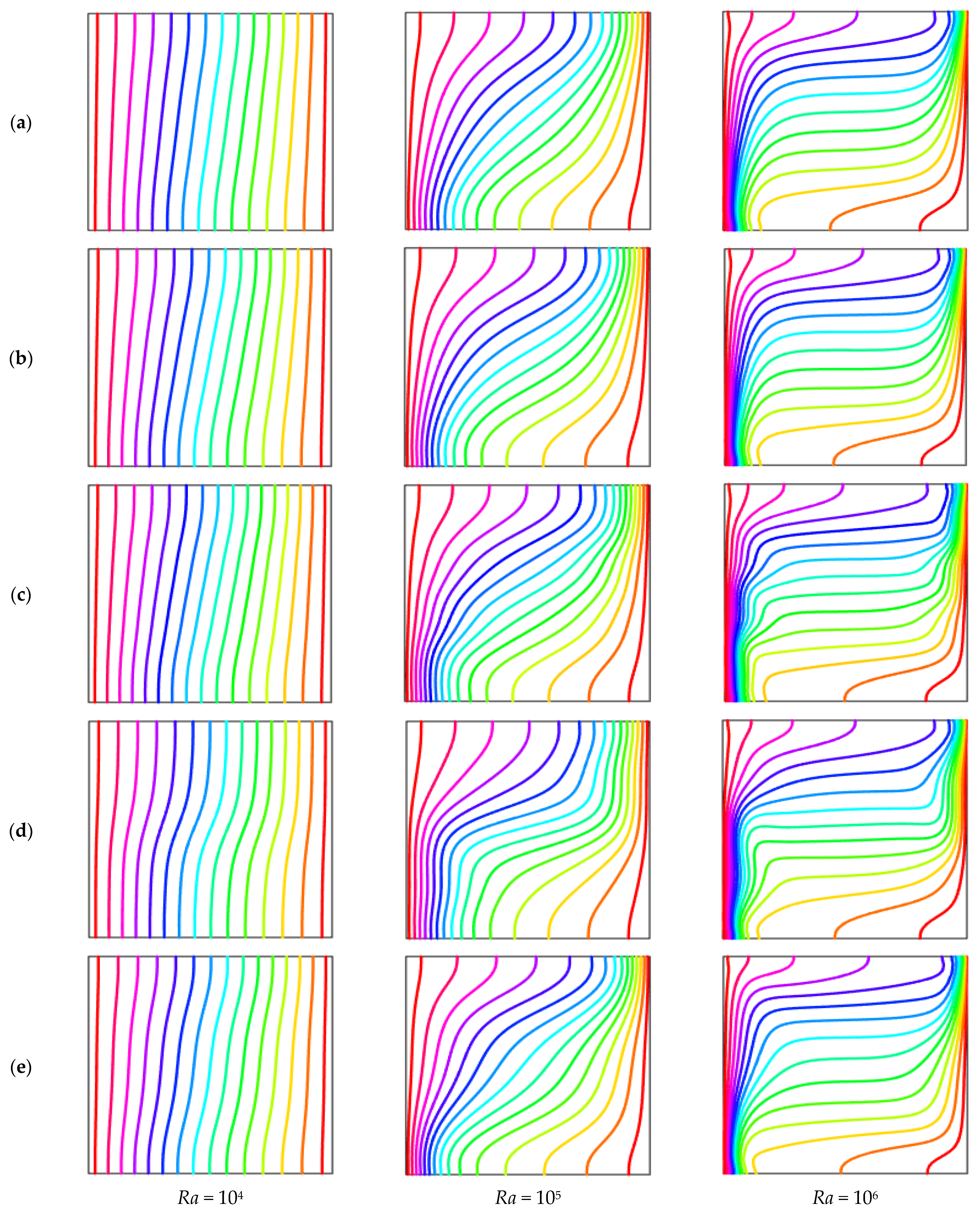
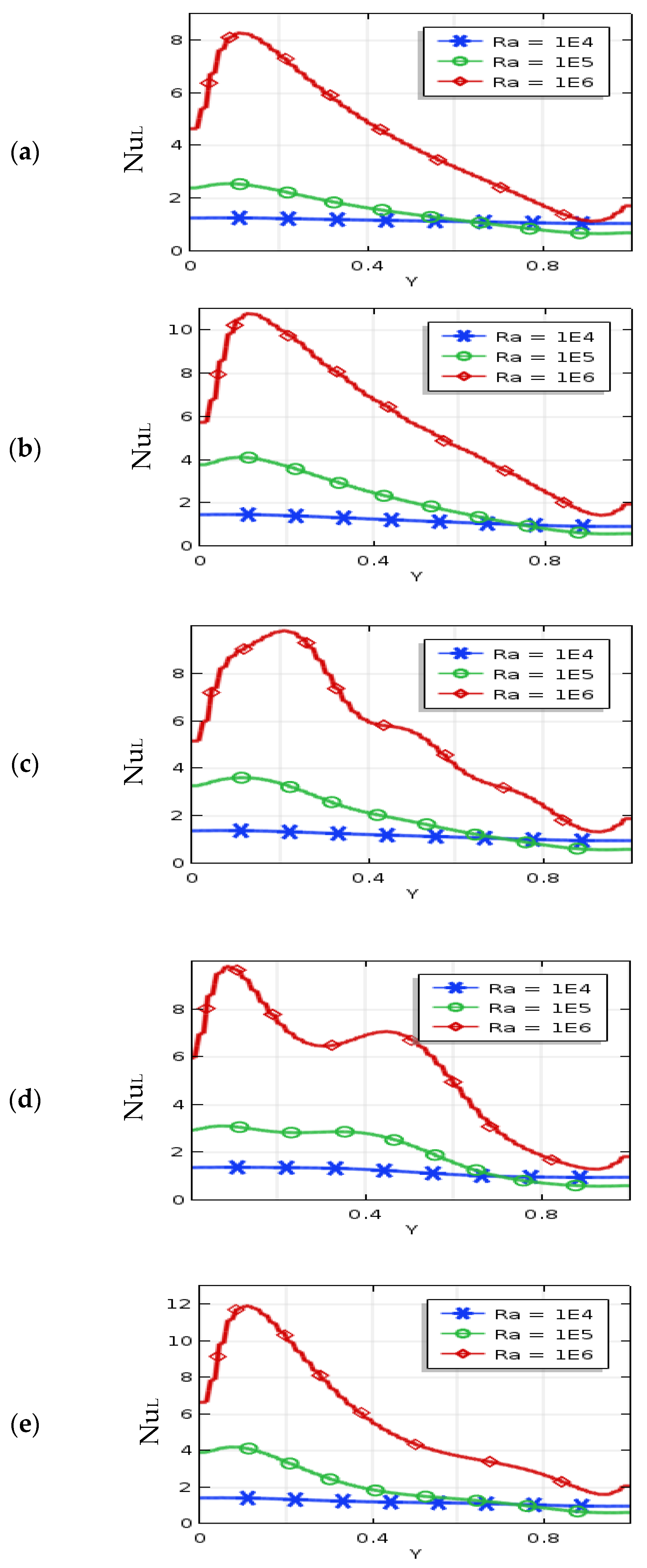
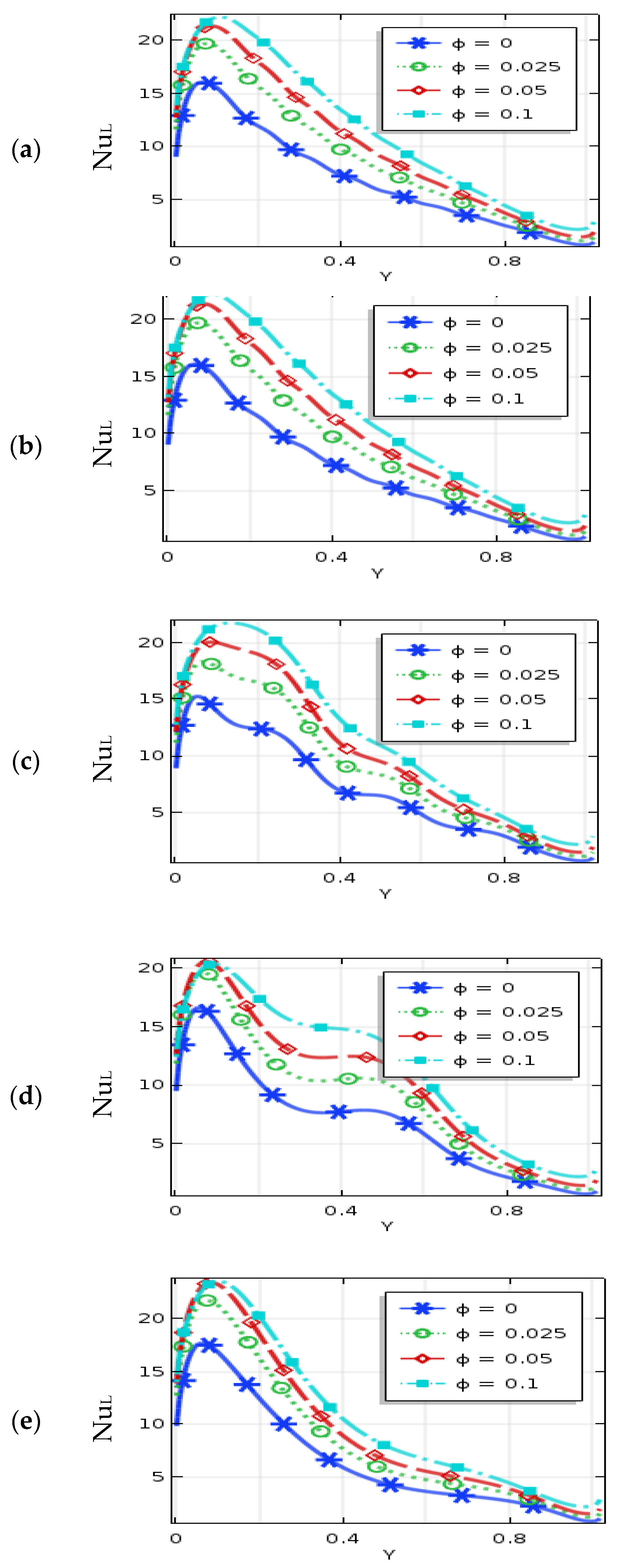
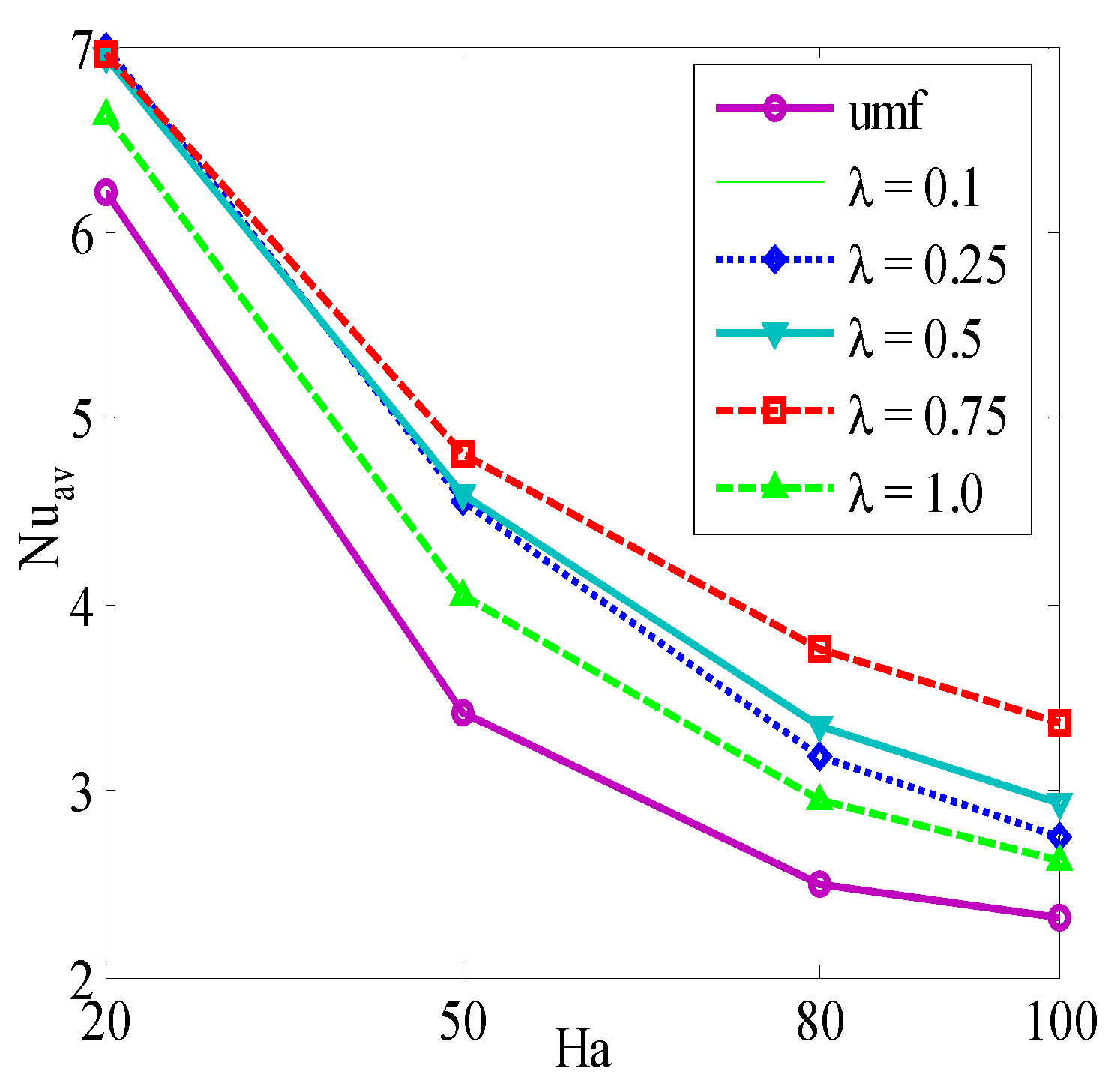
4. Results and Discussion
5. Conclusions
- The non-uniform magnetic effect has a significant impact on controlling the nanofluid flow and temperature transport, which provides a greater temperature transfer rate than the uniform magnetic field;
- The nanoparticles significantly improve heat transport rate even with 1% of nanoparticles volume fraction. The heat transfer rate rises by 18.71% for Cu-water nanofluid with 1% nanoparticles volume;
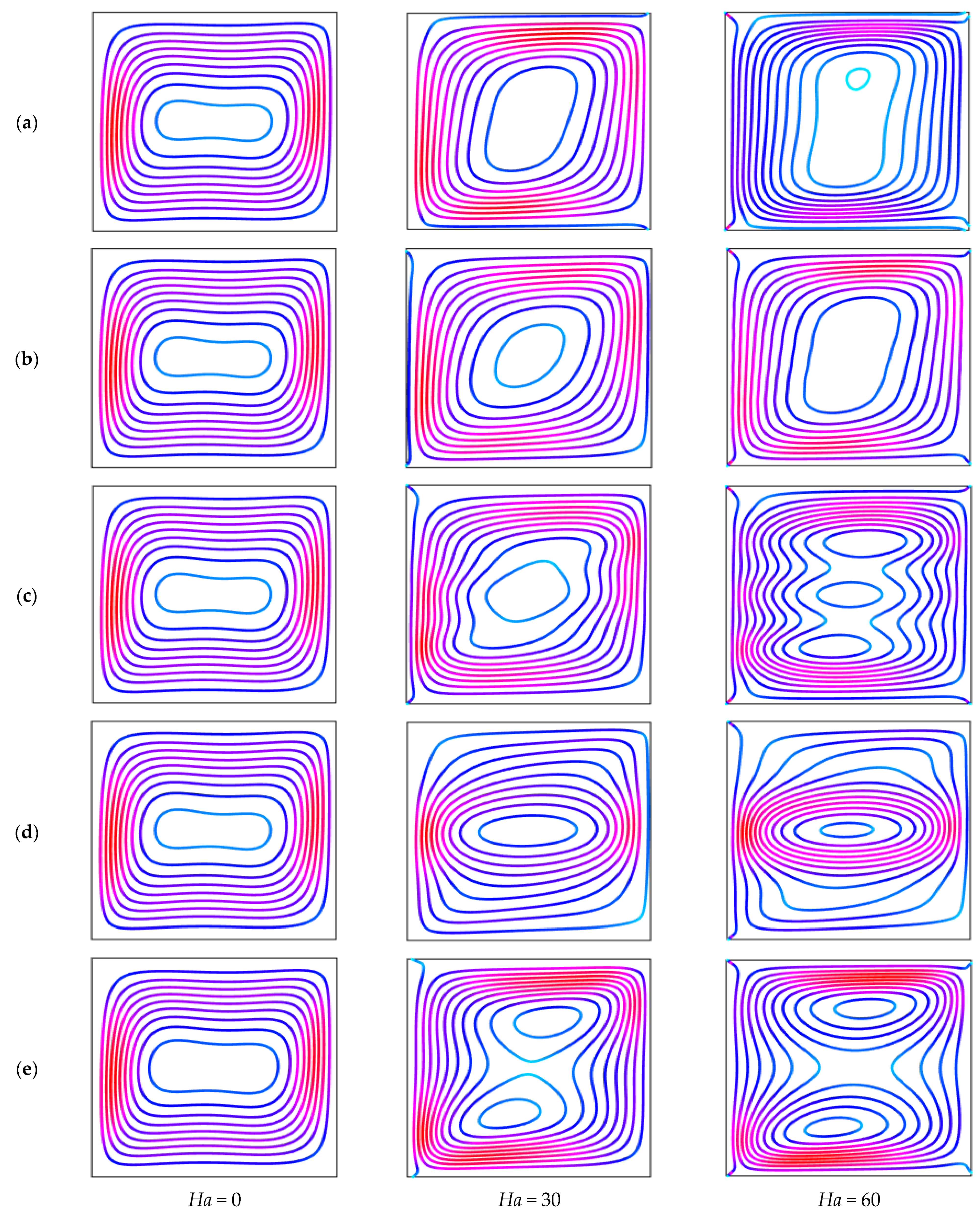
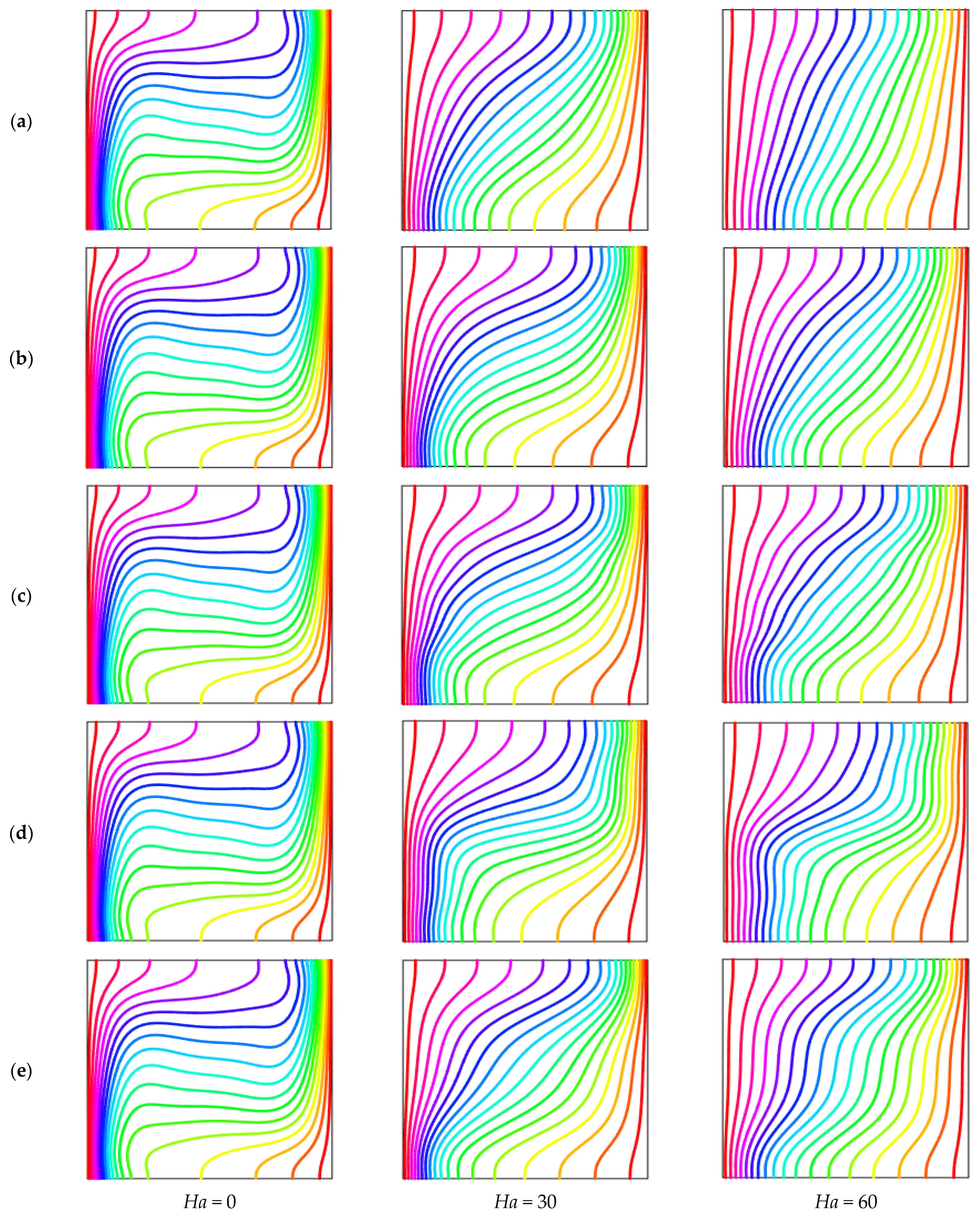
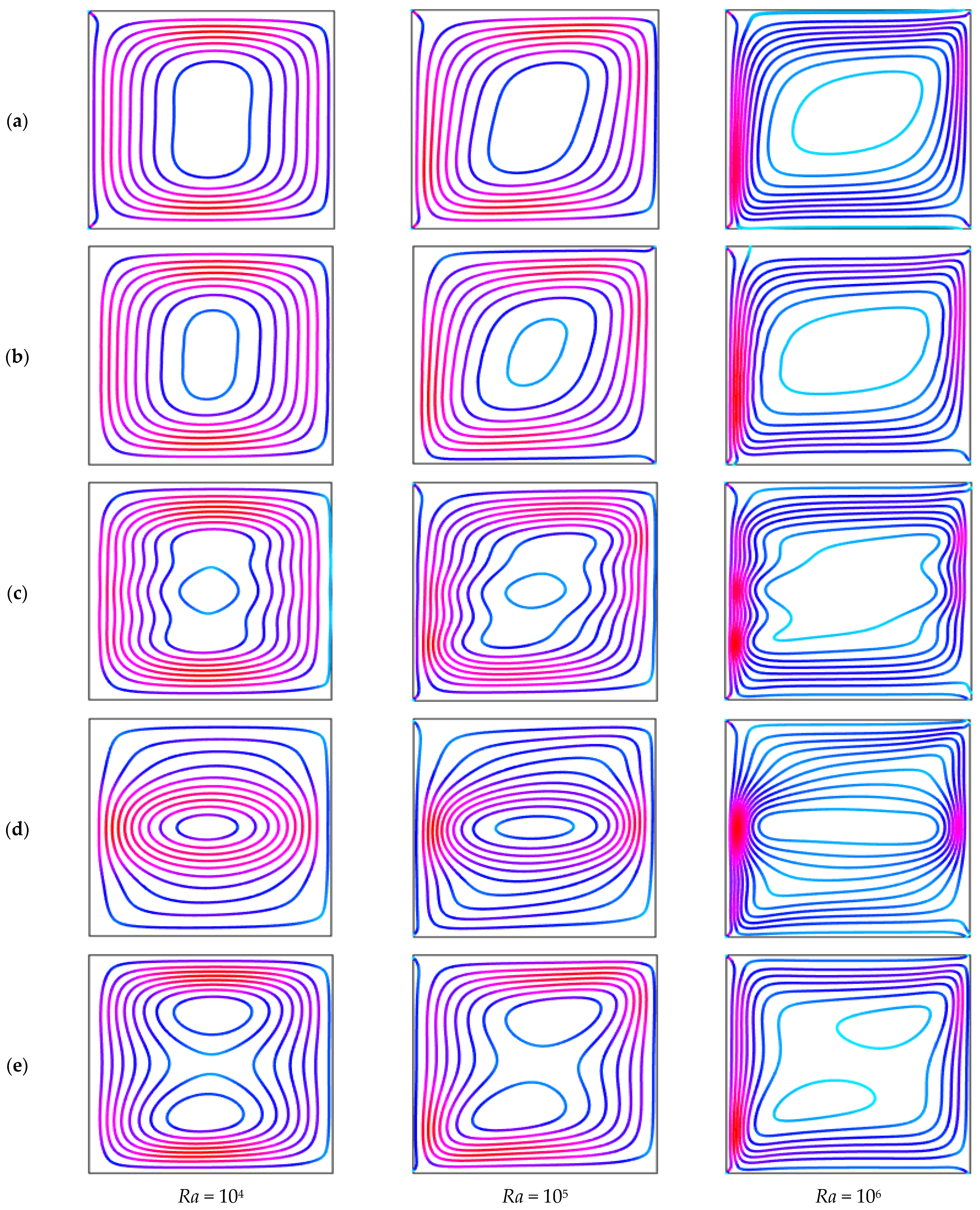
- A higher Rayleigh number represents a higher free convective heat transfer and fluid flow, whereas a higher Hartmann number diminishes the heat transport rate;
- The intensity of flow changes significantly with the variation of the magnetic field period. The streamlines and isotherms are almost identical at the low period (λ = 0.1) and uniform magnetic field. The period λ = 0.75 represents the highest heat transport rate;
- The nanoparticle’s diameter has an influential effect on making the nanofluid stable. The heat transfer rate is more significant for a smaller size of nanoparticles (0–20 nm);
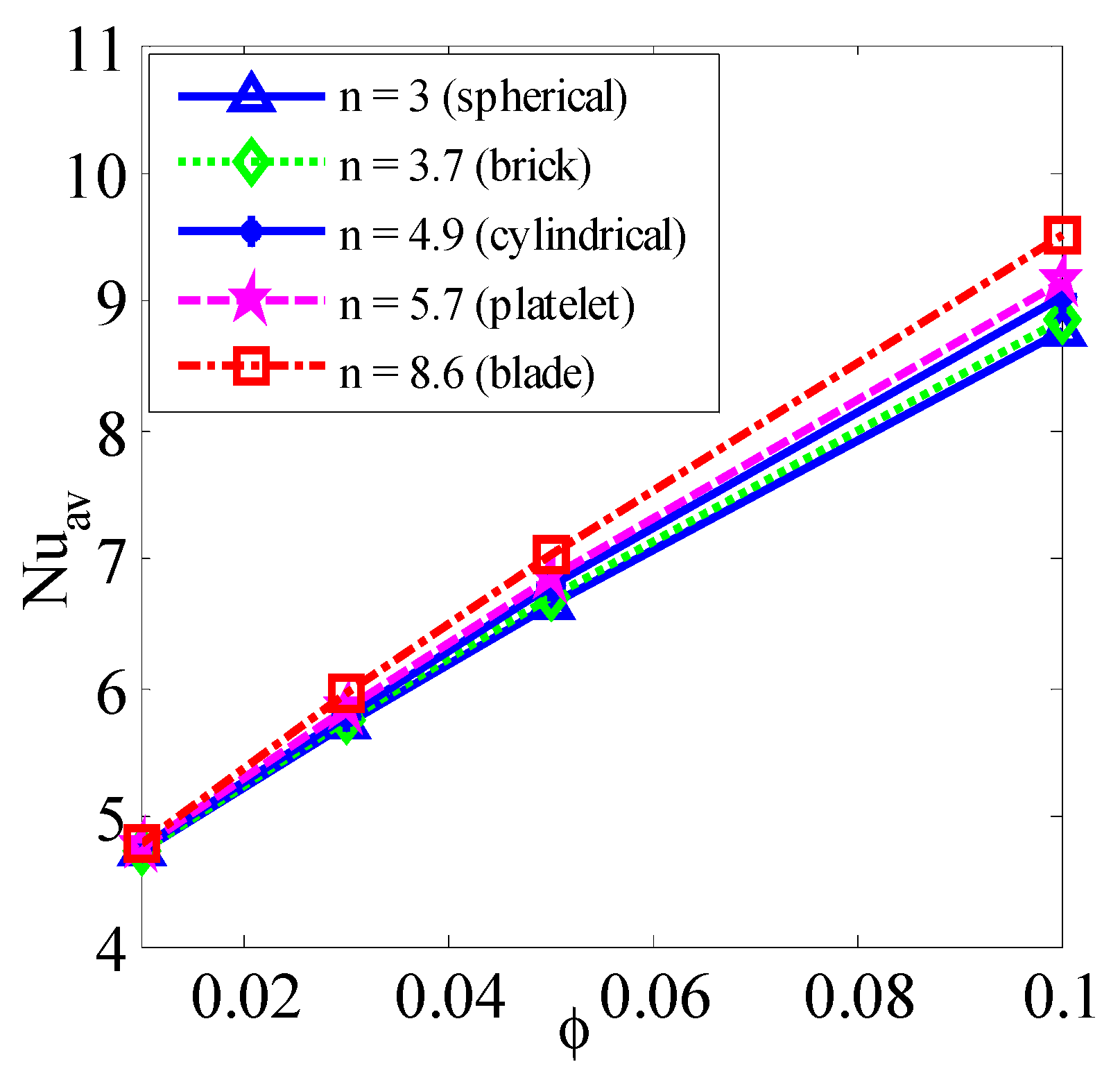
- The average heat transport is greater for blade-shaped nano-sized particles than other shapes. Therefore, shape and size of nanoparticles significantly influences the thermal performance;
- The highest heat transfer is obtained for Fe3O4-water and Cu-water nanofluids compared to other nanofluids. The heat transport rate increases by 20.14% for Fe3O4-water nanofluids, whereas it increases by 5.21% for CNT-water nanofluid;
- Brownian movement of nanoparticles has an influential role on heat transport.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| B0 | magnitude of magnetic field [kg s−2 A−1] | |
| cp | specific heat at constant pressure [J kg−1 K−1] | |
| g | gravitational acceleration [m s−2] | |
| dp | nanoparticles diameter [m] | |
| Ha | Hartmann number | |
| k | thermal conductivity [W m−1 K−1] | |
| KB | Boltzmann constant [J K−1] | |
| L | length of the enclosure [m] | |
| Nuav | average Nusselt number | |
| NuL | local Nusselt number | |
| p | dimensional fluid pressure [Pa] | |
| P | dimensionless fluid pressure | |
| Pr | Prandtl number | |
| Ra | Rayleigh number | |
| T | fluid temperature [K] | |
| t | dimensional time [s] | |
| u, v | dimensional velocity components [m s−1] | |
| U, V | dimensionless velocity component | |
| x, y | dimensional coordinates | |
| X, Y | non-dimensional coordinates | |
| Greek symbols | ||
| α | thermal diffusivity [m2 s−1] | |
| β | thermal expansion coefficient [K−1] | |
| ϕ | Solid volume fraction | |
| μ | dynamic viscosity [kg m−1 s−1] | |
| υ | kinematic viscosity [m2 s−1] | |
| θ | non-dimensional temperature | |
| ρ | density [kgm−3] | |
| σ | electric conductivity | |
| ψ | stream function | |
| λ0 | period number | |
| λ | period of magnetic field | |
| τ | dimensionless time | |
| Subscript | ||
| h | heat surface | |
| c | cold surface | |
| nf | nanofluid | |
| bf | basefluid | |
| sp | solid particle | |
| av | average | |
References
- Choi, S.U.S. Enhancing Thermal Conductivity of Fluids with Nanoparticles, Developments and Applications of Non-Newtonian Flows; ASME. FED-231/MD-66; Springer, D.A., Wang, H.P., Eds.; Argonne National Lab.: Argonne, IL, USA, 1995; pp. 99–105. [Google Scholar]
- Choi, S.U.S. Nanofluid Technology: Current Status and Future Research; Energy Technology Division, Argonne National Laboratory: Argonne, IL, USA, 1999; pp. 604–639. [Google Scholar]
- Saidur, R.; Leong, K.Y.; Mohammed, H.A. A review on applications and challenges of nanofluids. Renew. Sustain. Energy Rev. 2011, 15, 1646–1668. [Google Scholar] [CrossRef]
- Wen, D.; Lin, G.; Vafaei, S.; Zhang, K. Review of nanofluids for heat transfer applications. Particuology 2009, 7, 141–150. [Google Scholar] [CrossRef]
- Yu, W.; France, D.M.; Routbort, J.L.; Choi, S.U.S. Review and Comparison of Nanofluid Thermal Conductivity and Heat Transfer Enhancements. Heat Transf. Eng. 2008, 29, 432–460. [Google Scholar] [CrossRef]
- Wong, K.V.; De Leon, O. Applications of nanofluids: Current and future. Adv. Mech. Eng. 2010, 11, 519659. [Google Scholar] [CrossRef]
- Buongiorno, J. Convective Transport in Nanofluids. ASME J. Heat Transf. 2006, 128, 240–250. [Google Scholar] [CrossRef]
- Khan, M.I.; Alzahrani, F.; Hobiny, A. Simulation and modeling of second order velocity slip flow of micropolar ferrofluid with Darcy–Forchheimer porous medium. J. Mater. Res. Technol. 2020, 9, 7335–7340. [Google Scholar] [CrossRef]
- Khan, M.I.; Alzahrani, F. Binary chemical reaction with activation energy in dissipative flow of non-Newtonian nanomaterial. J. Theor. Comput. Chem. 2020, 19, 2040006. [Google Scholar] [CrossRef]
- Khan, M.I.; Alsaedi, A.; Hayat, T.; Khan, N.B. Modeling and computational analysis of hybrid class nanomaterials subject to entropy generation. Comput. Methods Programs Biomed. 2019, 179, 104973. [Google Scholar] [CrossRef]
- Hayat, T.; Tamoor, M.; Khan, M.I.; Alsaedi, A. Numerical simulation for nonlinear radiative flow by convective cylinder. Results Phys. 2016, 6, 1031–1035. [Google Scholar] [CrossRef]
- Hayat, T.; Ahmad, S.; Khan, M.I.; Alsaedi, A. Simulation of ferromagnetic nanomaterial flow of Maxwell fluid. Results Phys. 2018, 8, 34–40. [Google Scholar] [CrossRef]
- Mahian, O.; Kolsi, L.; Amani, M.; Estellé, P.; Ahmadi, G.; Kleinstreuer, C.; Marshall, J.S.; Siavashi, M.; Taylor, R.A.; Niazmand, H.; et al. Recent advances in modeling and simulation of nanofluid flows-Part I: Fundamentals and theory. Phys. Rep. 2019, 790, 1–48. [Google Scholar] [CrossRef]
- Mahian, O.; Kolsi, L.; Amani, M.; Estellé, P.; Ahmadi, G.; Kleinstreuer, C.; Marshall, J.S.; Taylor, R.A.; Abu-Nada, E.; Rashidi, S.; et al. Recent advances in modeling and simulation of nanofluid flows—Part II: Applications. Phys. Rep. 2019, 791, 1–59. [Google Scholar] [CrossRef]
- Shah, Z.; Ullah, A.; Bonyah, E.; Ayaz, M.; Islam, S.; Khan, I. Hall effect on Titania nanofluids thin film flow and radiative thermal behavior with different base fluids on an inclined rotating surface. AIP Adv. 2019, 9, 055113. [Google Scholar] [CrossRef]
- Rahman, M.; Alam, M.; Al-Salti, N.; Eltayeb, I. Hydromagnetic natural convective heat transfer flow in an isosceles triangular cavity filled with nanofluid using two-component nonhomogeneous model. Int. J. Therm. Sci. 2016, 107, 272–288. [Google Scholar] [CrossRef]
- Kalbani, K.S.A.; Rahman, M.M.; Alam, M.S.; Salti, N.A.; Eltayeb, I.A. Buoyancy induced heat transfer flow inside a tilted square enclosure filled with nanofluids in the presence of oriented magnetic field. Heat Transf. Eng. 2017, 39, 511–525. [Google Scholar] [CrossRef]
- Mojumder, S.; Rabbi, K.M.; Saha, S.; Hasan, M.N.; Saha, S.C. Magnetic field effect on natural convection and entropy gener-ation in a half-moon shaped cavity with semi-circular bottom heater having different ferrofluid inside. J. Magn. Magn. Mater. 2016, 407, 412–424. [Google Scholar] [CrossRef]
- Parveen, R.; Mahapatra, T. Numerical simulation of MHD double diffusive natural convection and entropy generation in a wavy enclosure filled with nanofluid with discrete heating. Heliyon 2019, 5, e02496. [Google Scholar] [CrossRef]
- Dogonchi, A.S.; Ismael, M.A.; Chamkha, A.J.; Ganji, D.D. Numerical analysis of natural convection of Cu–water nanofluid filling triangular cavity with semicircular bottom wall. J. Therm. Anal. 2019, 135, 3485–3497. [Google Scholar] [CrossRef]
- Billah, M.; Rahman, M.; Razzak, M.; Saidur, R.; Mekhilef, S. Unsteady buoyancy-driven heat transfer enhancement of nanofluids in an inclined triangular enclosure. Int. Commun. Heat Mass Transf. 2013, 49, 115–127. [Google Scholar] [CrossRef]
- Li, Z.; Hussein, A.K.; Younis, O.; Afrand, M.; Feng, S. Natural convection and entropy generation of a nanofluid around a circular baffle inside an inclined square cavity under thermal radiation and magnetic field effects. Int. Commun. Heat Mass Transf. 2020, 116, 104650. [Google Scholar] [CrossRef]
- Basak, T.; Roy, S.; Babu, S.K.; Balakrishnan, A. Finite element analysis of natural convection flow in a isosceles triangular enclosure due to uniform and non-uniform heating at the side walls. Int. J. Heat Mass Transf. 2008, 51, 4496–4505. [Google Scholar] [CrossRef]
- Al-Weheibi, S.M.; Rahman, M.; Alam, M.; Vajravelu, K. Numerical simulation of natural convection heat transfer in a trapezoidal enclosure filled with nanoparticles. Int. J. Mech. Sci. 2017, 131-132, 599–612. [Google Scholar] [CrossRef]
- Alam, M.S. Natural convective heat transfer of cobalt-kerosene nanofluid inside a quarter-circular enclosure with uniform and non-uniform heated bottom wall using two-component nonhomogeneous model. Thammasat Int. J. Sci. Technol. 2017, 22, 45–66. [Google Scholar]
- Mehryan, S.; Izadi, M.; Chamkha, A.J.; Sheremet, M.A. Natural convection and entropy generation of a ferrofluid in a square enclosure under the effect of a horizontal periodic magnetic field. J. Mol. Liq. 2018, 263, 510–525. [Google Scholar] [CrossRef]
- Haq, R.U.; Aman, S. Water functionalized CuO nanoparticles filled in a partially heated trapezoidal cavity with inner heated obstacle: FEM approach. Int. J. Heat Mass Transf. 2019, 128, 401–417. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Rashad, A.M.; Armaghani, T.; Mansour, M.A. Effects of partial slip on entropy generation and MHD combined convection in a lid-driven porous enclosure saturated with a Cu–water nanofluid. J. Therm. Anal. 2018, 132, 1291–1306. [Google Scholar] [CrossRef]
- Uddin, M.J. Numerical simulation on free convective heat transfer in a copper-water nanofluid filled semi-circular annulus with the magnetic field. Tec. Ital.-Ital. J. Eng. Sci. 2018, 62, 119–129. [Google Scholar] [CrossRef]
- Zahan, I.; Nasrin, R.; Alim, M.A. MHD effect on conjugate heat transfer in a nanofluid filled rectangular enclosure. Int. J. Petrochem. Sci. Eng. 2018, 3, 114–123. [Google Scholar] [CrossRef][Green Version]
- Abedini, A.; Armaghani, T.; Chamkha, A.J. MHD free convection heat transfer of a water–Fe3O4 nanofluid in a baffled C-shaped enclosure. J. Therm. Anal. 2018, 135, 685–695. [Google Scholar] [CrossRef]
- Marzougui, S.; Mebarek-Oudina, F.; Assia, A.; Magherbi, M.; Shah, Z.; Ramesh, K. Entropy generation on magneto-convective flow of copper–water nanofluid in a cavity with chamfers. J. Therm. Anal. 2021, 143, 2203–2214. [Google Scholar] [CrossRef]
- Balushi, L.M.A.; Rahman, M.M. Convective heat transfer utilizing magnetic nanoparticles in the presence of a sloping magnetic field inside a square enclosure. J. Therm. Sci. Eng. Appl. 2019, 11, 1–19. [Google Scholar]
- Al-Kalbani, K.S.; Rahman, M.M. Convective Heat Transfer in the Flow of a Nanofluid in an Inclined Square Enclosure. J. Eng. Phys. 2019, 92, 1150–1170. [Google Scholar] [CrossRef]
- Dutta, S.; Goswami, N.; Pati, S.; Biswas, A.K. Natural convection heat transfer and entropy generation in a porous rhombic enclosure: Influence of non-uniform heating. J. Therm. Anal. 2021, 144, 1493–1515. [Google Scholar] [CrossRef]
- Uddin, M.; Rasel, S. Numerical analysis of natural convective heat transport of copper oxide-water nanofluid flow inside a quadrilateral vessel. Heliyon 2019, 5, e01757. [Google Scholar] [CrossRef]
- Uddin, M.; Rasel, S.; Rahman, M.; Vajravelu, K. Natural convective heat transfer in a nanofluid-filled square vessel having a wavy upper surface in the presence of a magnetic field. Therm. Sci. Eng. Prog. 2020, 19, 100660. [Google Scholar] [CrossRef]
- Islam, T.; Akter, N.; Jahan, N. MHD free convective heat transfer in a triangular enclosure filled with copper-water nanofluid. Int. J. Mater. Math. Sci. 2020, 2, 29–38. [Google Scholar]
- Islam, T.; Parveen, N.; Asad, M.F.A. Hydromagnetic natural convection heat transfer of copper-water nanofluid within a right-angled triangular cavity. Int. J. Sci. Technol. 2020, 7, 070304. [Google Scholar]
- Islam, T.; Alam, N.; Asjad, M.I.; Parveen, N.; Chu, Y.-M. Heatline visualization of MHD natural convection heat transfer of nanofluid in a prismatic enclosure. Sci. Rep. 2021, 11, 1–18. [Google Scholar] [CrossRef]
- Mullick, S.H.; Kumar, A.; Kundu, P.K. Numerical Study of Natural Convection Inside a Square Cavity with Non-uniform Heating from Top. J. Inst. Eng. Ser. C 2020, 101, 1043–1050. [Google Scholar] [CrossRef]
- Rostami, S.; Ellahi, R.; Oztop, H.F.; Goldanlou, A.S. A study on the effect of magnetic field and the sinusoidal boundary con-dition on free convective heat transfer of non-Newtonian power-law fluid in a square enclosure with two constant-temperature obstacles using lattice Boltzmann method. J. Therm. Anal. Calorim. 2020, 144, 1–17. [Google Scholar]
- Alsabery, A.I.; Ismael, M.; Chamkha, A.J.; Hashim, I. Mixed convection of Al2O3-water nanofluid in a double lid-driven square cavity with a solid inner insert using Buongiorno’s two-phase model. Int. J. Heat Mass Transf. 2018, 119, 939–961. [Google Scholar] [CrossRef]
- Uddin, M.; Rahman, M. Heat Transportation in Copper Oxide-Water Nanofluid Filled Triangular Cavities. Int. J. Heat Technol. 2020, 38, 106–124. [Google Scholar] [CrossRef]
- Qayyum, S.; Khan, M.I.; Hayat, T.; Alsaedi, A. Comparative investigation of five nanoparticles in flow of viscous fluid with Joule heating and slip due to rotating disk. Phys. B Condens. Matter 2018, 534, 173–183. [Google Scholar] [CrossRef]
- Gireesha, B.; Sowmya, G.; Khan, M.I.; Öztop, H.F. Flow of hybrid nanofluid across a permeable longitudinal moving fin along with thermal radiation and natural convection. Comput. Methods Programs Biomed. 2020, 185, 105166. [Google Scholar] [CrossRef]
- Waqas, M.; Khan, M.I.; Hayat, T.; Gulzar, M.M.; Alsaedi, A. Transportation of radiative energy in viscoelastic nanofluid con-sidering buoyancy forces and convective conditions. Chaos Solitons Fractals 2020, 130, 109415. [Google Scholar] [CrossRef]
- Mansour, M.A.; Gorla, R.S.R.; Siddiqa, S.; Rashad, A.M.; Salah, T. Unsteady MHD natural convection flow of a nanofluid inside an inclined square cavity containing a heated circular obstacle. Int. J. Nonlinear Sci. Numer. Simul. 2021. [Google Scholar] [CrossRef]
- Alsoy-Akgün, N. Effect of an uniform magnetic field on unsteady natural convection of nanofluid. J. Taibah Univ. Sci. 2019, 13, 1073–1086. [Google Scholar] [CrossRef]
- Ghasemi, B.; Aminossadati, S.; Raisi, A. Magnetic field effect on natural convection in a nanofluid-filled square enclosure. Int. J. Therm. Sci. 2011, 50, 1748–1756. [Google Scholar] [CrossRef]
- Martínez-Farías, F.J.; Alvarado-Sánchez, A.; Rangel-Cortes, E.; Hernández-Hernández, A. Bi-dimensional crime model based on anomalous diffusion with law enforcement effect. Math. Model. Numer. Simul. Appl. 2022, 2, 26–40. [Google Scholar] [CrossRef]
- Asad, M.F.A.; Alam, M.N.; Rashad, A.M.; Sarker, M.M.A. Impact of undulation on magneto-free convective heat transport in an enclosure having vertical wavy sides. Int. Comm. Heat Mass Trans. 2021, 127, 105579. [Google Scholar] [CrossRef]
- Sene, N. Second-grade fluid with Newtonian heating under Caputo fractional derivative: Analytical investigations via Laplace transforms. Math. Model. Numer. Simul. Appl. 2022, 2, 13–25. [Google Scholar] [CrossRef]
- Fayz-Al-Asad, M.; Alam, N.; Ahmad, H.; Sarker, M.; Alsulami, M.; Gepreel, K.A. Impact of a closed space rectangular heat source on natural convective flow through triangular cavity. Results Phys. 2021, 23, 104011. [Google Scholar] [CrossRef]
- Khan, A.; Khan, A.; Sinan, M. Ion temperature gradient modes driven soliton and shock by reduction perturbation method for electron-ion magneto-plasma. Math. Model. Numer. Simul. Appl. 2022, 2, 1–12. [Google Scholar] [CrossRef]
- Asad, M.F.A.; Alam, M.N.; Tunç, C.; Sarker, M.M.A. Heat transport exploration of free convection flow inside enclosure having vertical wavy walls. J. Appl. Comput. Mech. 2021, 7, 520–527. [Google Scholar]
- Asad, M.F.A.; Yavuz, M.; Alam, M.N.; Sarker, M.M.A.; Bazighifan, O. Influence of fin length on magneto-combined con-vection heat transfer performance in a lid-driven wavy cavity. Fractal Fract. 2021, 5, 107. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Taylor, R.L. The Finite Element Method, 4th ed.; McGraw-Hill: New York, NY, USA, 1991. [Google Scholar]






| Base Fluid/Nanoparticles | cp (J kg−1 K−1) | ρ (kg m−3) | k (W m−1 K−1) | μ (kg m−1 s−1) | β × 10−5 (K−1) | σ (S m−1) | Pr |
|---|---|---|---|---|---|---|---|
| Water (H2O) | 4179 | 997.1 | 0.613 | 0.001003 | 21 | 5.5 × 10−6 | 6.8377 |
| Kerosene (Ke) | 2090 | 780 | 0.149 | 0.00164 | 99 | 6 × 10−10 | 23.004 |
| Ethylene Glycol (EG) | 2382.1 | 1117.48 | 0.2492 | 0.022 | 57 | 1.07 × 10−6 | 210.3 |
| Copper (Cu) | 385 | 8933 | 400 | - | 1.67 | 5.96 × 107 | - |
| Zink (Zn) | 387 | 7135 | 116 | - | 3.02 | 1.69 × 103 | - |
| Ferrosoferric oxide (Fe3O4) | 670 | 5180 | 80.4 | - | 20.6 | 0.112 × 106 | - |
| Alumina (Al2O3) | 765 | 3970 | 40 | - | 0.85 | 3.5 × 107 | - |
| Titanium oxide | 686.2 | 4250 | 8.9538 | 0.90 | 2.6 × 106 | - | |
| CNT | 650 | 1350 | 3500 | - | 4.2 | 1.0 × 107 | - |
| Ha | ϕ = 0 | ϕ = 0.02 | ||
|---|---|---|---|---|
| Ghasemi et al. [50] | Present Study | Ghasemi et al. [50] | Present Study | |
| 0 | 4.738 | 4.721 | 4.820 | 4.717 |
| 15 | 4.143 | 4.127 | 4.179 | 4.105 |
| 30 | 3.150 | 3.138 | 3.138 | 3.097 |
| 45 | 2.369 | 2.359 | 2.342 | 2.318 |
| 60 | 1.851 | 1.843 | 1.831 | 1.815 |
| Nanofluids | Increase (%) | Nanofluids | Increase (%) | ||||
|---|---|---|---|---|---|---|---|
| Fe3O4-water | 0.0 | 8.243898 | - | Zn-water | 0.0 | 8.243898 | - |
| 0.01 | 9.904465 | 20.14 | 0.01 | 9.508525 | 15.34 | ||
| 0.05 | 15.38697 | 55.35 | 0.05 | 13.47574 | 41.72 | ||
| 0.10 | 20.68821 | 34.45 | 0.10 | 16.89489 | 25.37 | ||
| Al2O3-water | 0.0 | 8.243898 | - | TiO2-water | 0.0 | 8.243898 | - |
| 0.01 | 9.561033 | 15.98 | 0.01 | 8.980775 | 8.94 | ||
| 0.05 | 13.48560 | 41.05 | 0.05 | 11.28278 | 25.63 | ||
| 0.10 | 16.57418 | 22.90 | 0.10 | 13.14243 | 16.48 | ||
| Cu-water | 0.0 | 8.243898 | - | CNT-water | 0.0 | 8.243898 | - |
| 0.01 | 9.786020 | 18.71 | 0.01 | 8.673405 | 5.210 | ||
| 0.05 | 14.52538 | 48.43 | 0.05 | 10.02884 | 15.63 | ||
| 0.10 | 18.52136 | 27.51 | 0.10 | 11.10022 | 10.68 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Islam, T.; Yavuz, M.; Parveen, N.; Fayz-Al-Asad, M. Impact of Non-Uniform Periodic Magnetic Field on Unsteady Natural Convection Flow of Nanofluids in Square Enclosure. Fractal Fract. 2022, 6, 101. https://doi.org/10.3390/fractalfract6020101
Islam T, Yavuz M, Parveen N, Fayz-Al-Asad M. Impact of Non-Uniform Periodic Magnetic Field on Unsteady Natural Convection Flow of Nanofluids in Square Enclosure. Fractal and Fractional. 2022; 6(2):101. https://doi.org/10.3390/fractalfract6020101
Chicago/Turabian StyleIslam, Tarikul, Mehmet Yavuz, Nazma Parveen, and Md. Fayz-Al-Asad. 2022. "Impact of Non-Uniform Periodic Magnetic Field on Unsteady Natural Convection Flow of Nanofluids in Square Enclosure" Fractal and Fractional 6, no. 2: 101. https://doi.org/10.3390/fractalfract6020101
APA StyleIslam, T., Yavuz, M., Parveen, N., & Fayz-Al-Asad, M. (2022). Impact of Non-Uniform Periodic Magnetic Field on Unsteady Natural Convection Flow of Nanofluids in Square Enclosure. Fractal and Fractional, 6(2), 101. https://doi.org/10.3390/fractalfract6020101








