Analysis of the Multi-Dimensional Navier–Stokes Equation by Caputo Fractional Operator
Abstract
1. Introduction
2. Pre-Requisites
2.1. Caputo Fractional Differential Operator
2.2. Caputo Fractional Integral Operator
2.3. Sumudu Transform
2.4. Sumudu Transform of the Caputo Fractional Derivative
3. Iteration Method in the Caputo Derivative by Using the Sumudu Transformation
4. Existence of Solution in Caputo Case
5. Uniqueness of Solution
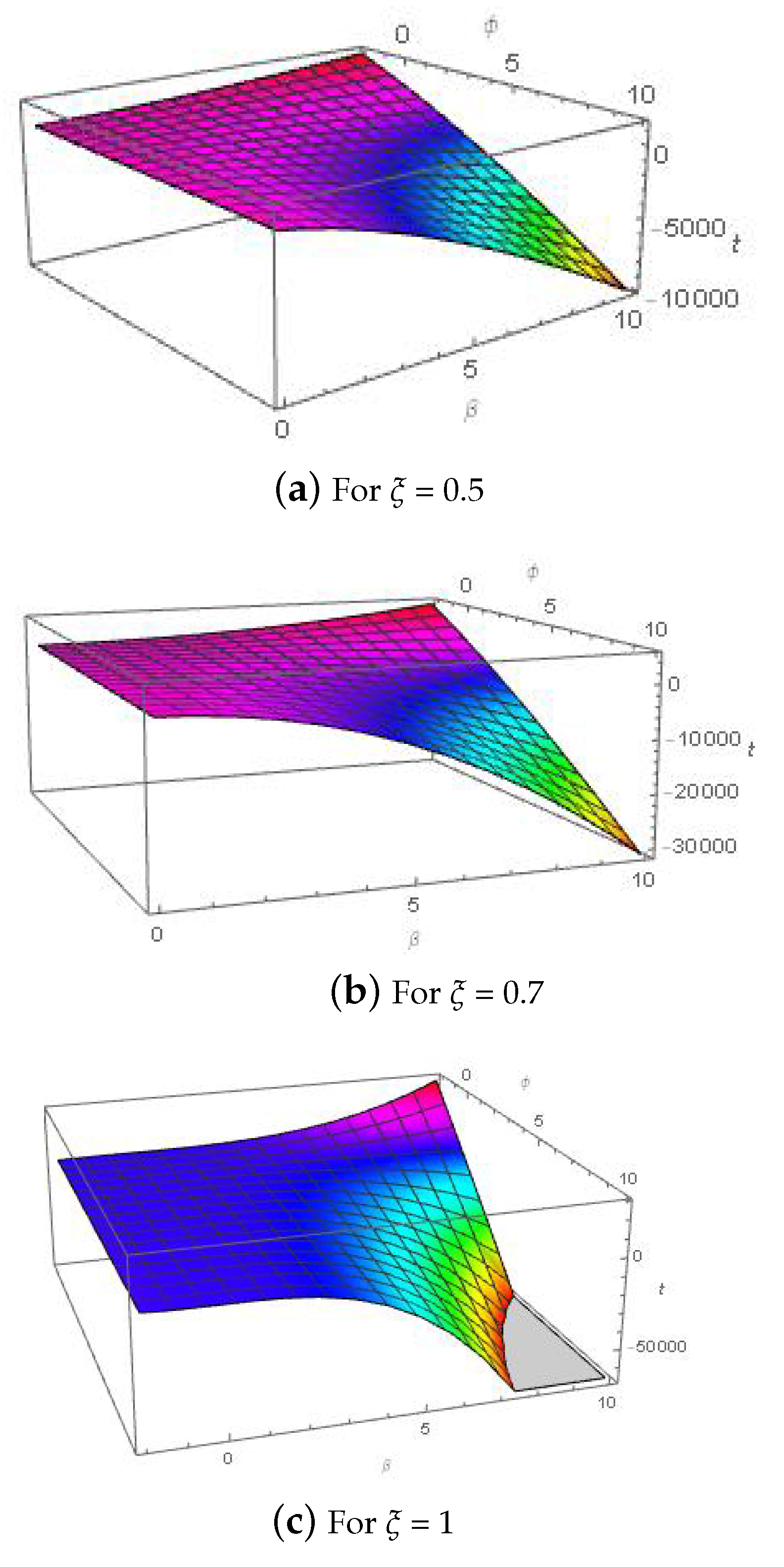
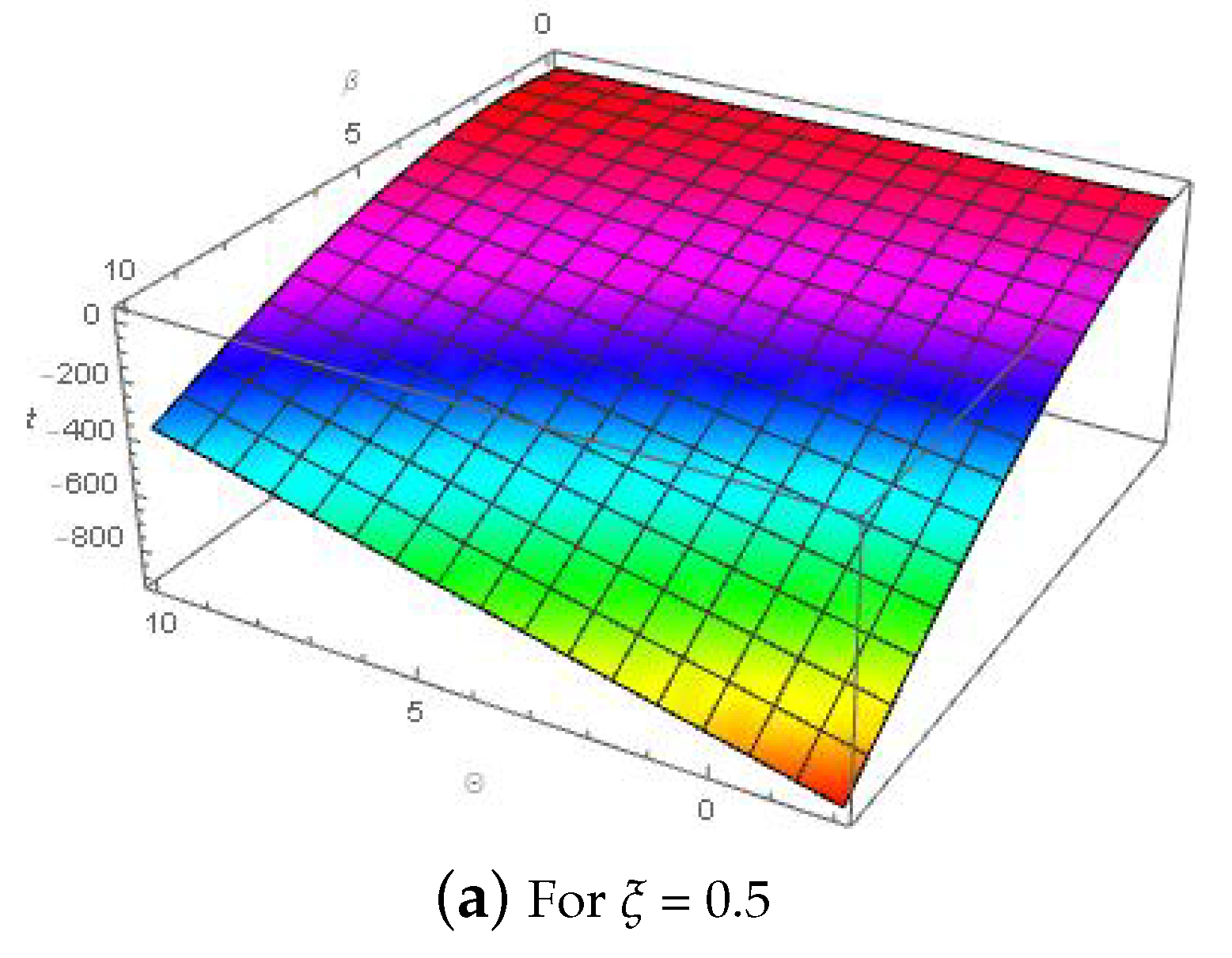
6. Solution of the Model by Sumudu Transform Method with Caputo Fractional Derivative
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Temam, R. Navier–Stokes Equations: Theory and Numerical Analysis; American Mathematical Society: Providence, RI, USA, 2001; Volume 343. [Google Scholar]
- Doering, C.R.; Gibbon, J.D. Applied Analysis of the Navier–Stokes Equations (No. 12); Cambridge University Press: Cambridge, MA, USA, 1995. [Google Scholar]
- Constantin, P.; Foias, C. Navier–Stokes Equations; University of Chicago Press: Chicago, IL, USA, 2020. [Google Scholar]
- Jin, X.; Cai, S.; Li, H.; Karniadakis, G.E. NSFnets (Navier–Stokes flow nets): Physics-informed neural networks for the incompressible Navier–Stokes equations. J. Comput. Phys. 2021, 426, 109951. [Google Scholar] [CrossRef]
- Kumar, S.; Ghosh, S.; Samet, B.; Goufo, E.F.D. An analysis for heat equations arises in diffusion process using new Yang-Abdel-Aty-Cattani fractional operator. Math. Methods Appl. Sci. 2020, 43, 6062–6080. [Google Scholar] [CrossRef]
- Kumar, S.; Nisar, K.S.; Kumar, R.; Cattani, C.; Samet, B. A new Rabotnov fractional-exponential function-based fractional derivative for diffusion equation under external force. Math. Methods Appl. Sci. 2020, 43, 4460–4471. [Google Scholar] [CrossRef]
- Atangana, A.; Akgül, A. Can transfer function and Bode diagram be obtained from Sumudu transform. Alex. Eng. J. 2020, 59, 1971–1984. [Google Scholar] [CrossRef]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; Elsevier: Amsterdam, The Netherlands, 2006; Volume 204. [Google Scholar]
- Hoult, R.E.; Kovtun, P. Stable and causal relativistic Navier–Stokes equations. J. High Energy Phys. 2020, 2020, 67. [Google Scholar] [CrossRef]
- Cheskidov, A.; Luo, X. Sharp nonuniqueness for the Navier–Stokes equations. arXiv 2020, arXiv:2009.06596. [Google Scholar] [CrossRef]
- Coppola, G.; Capuano, F.; de Luca, L. Discrete energy-conservation properties in the numerical simulation of the Navier–Stokes equations. Appl. Mech. Rev. 2019, 71, 010803. [Google Scholar] [CrossRef]
- Mahmood, S.; Shah, R.; Khan, H.; Arif, M. Laplace adomian decomposition method for multi dimensional time fractional model of Navier–Stokes equation. Symmetry 2019, 11, 149. [Google Scholar] [CrossRef]
- Zhang, C.; Li, Q.; Fu, S.; Wang, Z.J. A third-order gas-kinetic CPR method for the Euler and Navier–Stokes equations on triangular meshes. J. Comput. Phys. 2018, 363, 329–353. [Google Scholar] [CrossRef]
- Mukhtar, S.; Shah, R.; Noor, S. The Numerical Investigation of a Fractional-Order Multi-Dimensional Model of Navier–Stokes Equation via Novel Techniques. Symmetry 2022, 14, 1102. [Google Scholar] [CrossRef]
- Shah, R.; Khan, H.; Baleanu, D.; Kumam, P.; Arif, M. The analytical investigation of time-fractional multi-dimensional Navier–Stokes equation. Alex. Eng. J. 2020, 59, 2941–2956. [Google Scholar] [CrossRef]
- Chu, Y.M.; Shah, N.A.; Agarwal, P.; Chung, J.D. Analysis of fractional multi-dimensional Navier–Stokes equation. Adv. Differ. Equ. 2021, 2021, 91. [Google Scholar] [CrossRef]
- Singh, B.K.; Kumar, P. FRDTM for numerical simulation of multi-dimensional, time-fractional model of Navier–Stokes equation. Ain Shams Eng. J. 2018, 9, 827–834. [Google Scholar] [CrossRef]
- Kavvas, M.L.; Ercan, A. Generalizations of incompressible and compressible Navier–Stokes equations to fractional time and multi-fractional space. Sci. Rep. 2022, 12, 19337. [Google Scholar] [CrossRef] [PubMed]
- Dubey, R.S.; Baleanu, D.; Mishra, M.N.; Goswami, P. Solution of Modified Bergman Minimal Blood Glucose-Insulin Model Using Caputo-Fabrizio Fractional Derivative. CMES Comput. Model. Eng. Sci. 2021, 128, 1247–1263. [Google Scholar]
- Golmankhaneh, A.K.; Tunç, C. Sumudu transform in fractal calculus. Appl. Math. Comput. 2019, 350, 386–401. [Google Scholar] [CrossRef]
- Prakash, A.; Kumar, M.; Baleanu, D. A new iterative technique for a fractional model of nonlinear Zakharov–Kuznetsov equations via Sumudu transform. Appl. Math. Comput. 2018, 334, 30–40. [Google Scholar] [CrossRef]
- Peter, O.J.; Shaikh, A.S.; Ibrahim, M.O.; Nisar, K.S.; Baleanu, D.; Khan, I.; Abioye, A.I. Analysis and dynamics of fractional order mathematical model of COVID-19 in Nigeria using atangana-baleanu operator. Comput. Mater. Contin. 2021, 66, 1823–1848. [Google Scholar] [CrossRef]
- Baleanu, D.; Abadi, M.H.; Jajarmi, A.; Vahid, K.Z.; Nieto, J.J. A new comparative study on the general fractional model of COVID-19 with isolation and quarantine effects. Alex. Eng. J. 2022, 61, 4779–4791. [Google Scholar] [CrossRef]
- Gómez-Aguilar, J.F. Chaos in a nonlinear Bloch system with Atangana–Baleanu fractional derivatives. Numer. Methods Partial. Differ. Equ. 2018, 34, 1716–1738. [Google Scholar] [CrossRef]
- Yang, X.J.; Abdel-Aty, M.; Cattani, C. A new general fractional-order derivataive with Rabotnov fractional-exponential kernel applied to model the anomalous heat transfer. Therm. Sci. 2019, 23, 1677–1681. [Google Scholar] [CrossRef]
- Haq, I.; Khan, A.; Ahmad, S.; Ali, A.; Rahman, M.U. Modeling and analysis of a fractional anthroponotic cutaneous leishmania model with Atangana-Baleanu derivative. Comput. Methods Biomech. Biomed. Eng. 2022, 25, 1722–1743. [Google Scholar] [CrossRef] [PubMed]
- Kumar, P.; Erturk, V.S.; Vellappandi, M.; Trinh, H.; Govindaraj, V. A study on the maize streak virus epidemic model by using optimized linearization-based predictor-corrector method in Caputo sense. Chaos Solitons Fractals 2022, 158, 112067. [Google Scholar] [CrossRef]
- Malyk, I.V.; Gorbatenko, M.; Chaudhary, A.; Sharma, S.; Dubey, R.S. Numerical Solution of Nonlinear Fractional Diffusion Equation in Framework of the Yang–Abdel–Cattani Derivative Operator. Fractal Fract. 2021, 5, 64. [Google Scholar] [CrossRef]
- Shrahili, M.; Dubey, R.S.; Shafay, A. Inclusion of fading memory to Banister model of changes in physical condition. Discret. Contin. Dyn. Syst. S 2020, 13, 881. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Albalawi, K.S.; Mishra, M.N.; Goswami, P. Analysis of the Multi-Dimensional Navier–Stokes Equation by Caputo Fractional Operator. Fractal Fract. 2022, 6, 743. https://doi.org/10.3390/fractalfract6120743
Albalawi KS, Mishra MN, Goswami P. Analysis of the Multi-Dimensional Navier–Stokes Equation by Caputo Fractional Operator. Fractal and Fractional. 2022; 6(12):743. https://doi.org/10.3390/fractalfract6120743
Chicago/Turabian StyleAlbalawi, Kholoud Saad, Manvendra Narayan Mishra, and Pranay Goswami. 2022. "Analysis of the Multi-Dimensional Navier–Stokes Equation by Caputo Fractional Operator" Fractal and Fractional 6, no. 12: 743. https://doi.org/10.3390/fractalfract6120743
APA StyleAlbalawi, K. S., Mishra, M. N., & Goswami, P. (2022). Analysis of the Multi-Dimensional Navier–Stokes Equation by Caputo Fractional Operator. Fractal and Fractional, 6(12), 743. https://doi.org/10.3390/fractalfract6120743






