Fractal Characteristics of River Networks Induced by Dynamical Stochastic Replication
Abstract
:1. Introduction
2. Nonstationary Markovian Replication Process
3. Stochastic Model with Dynamic Replications for River Networks
4. Methods
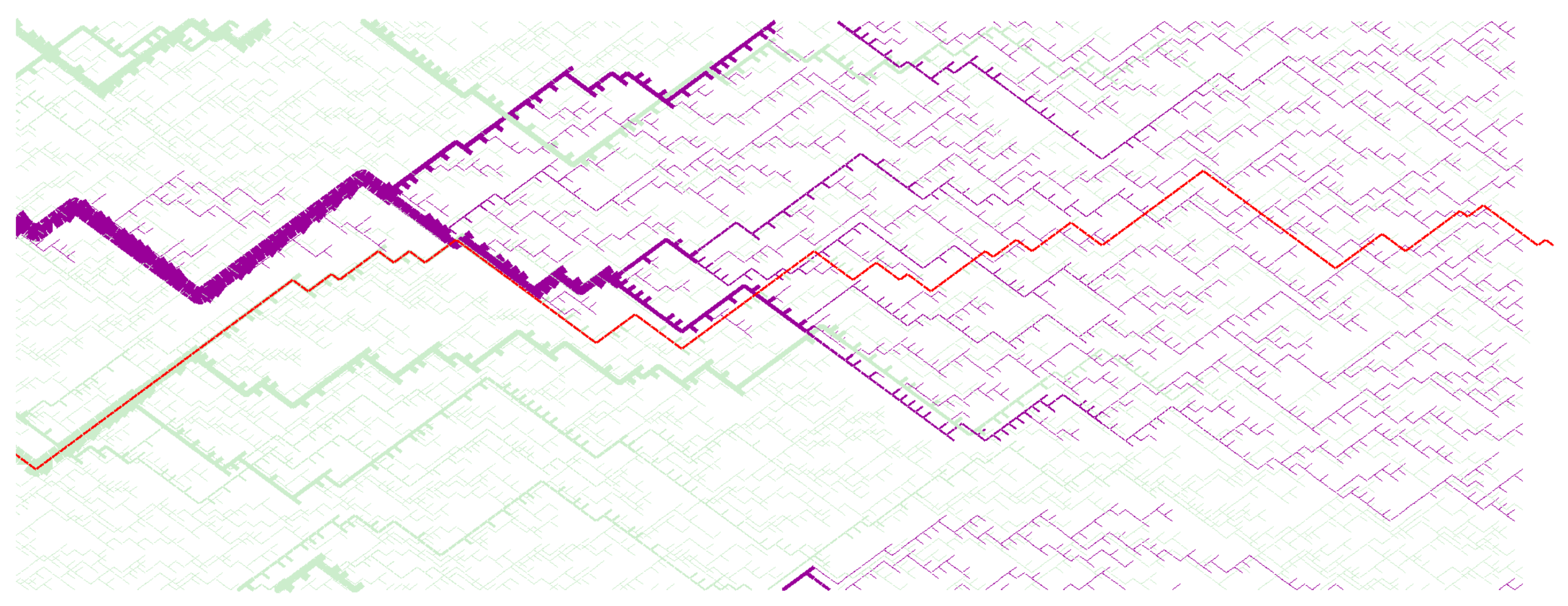
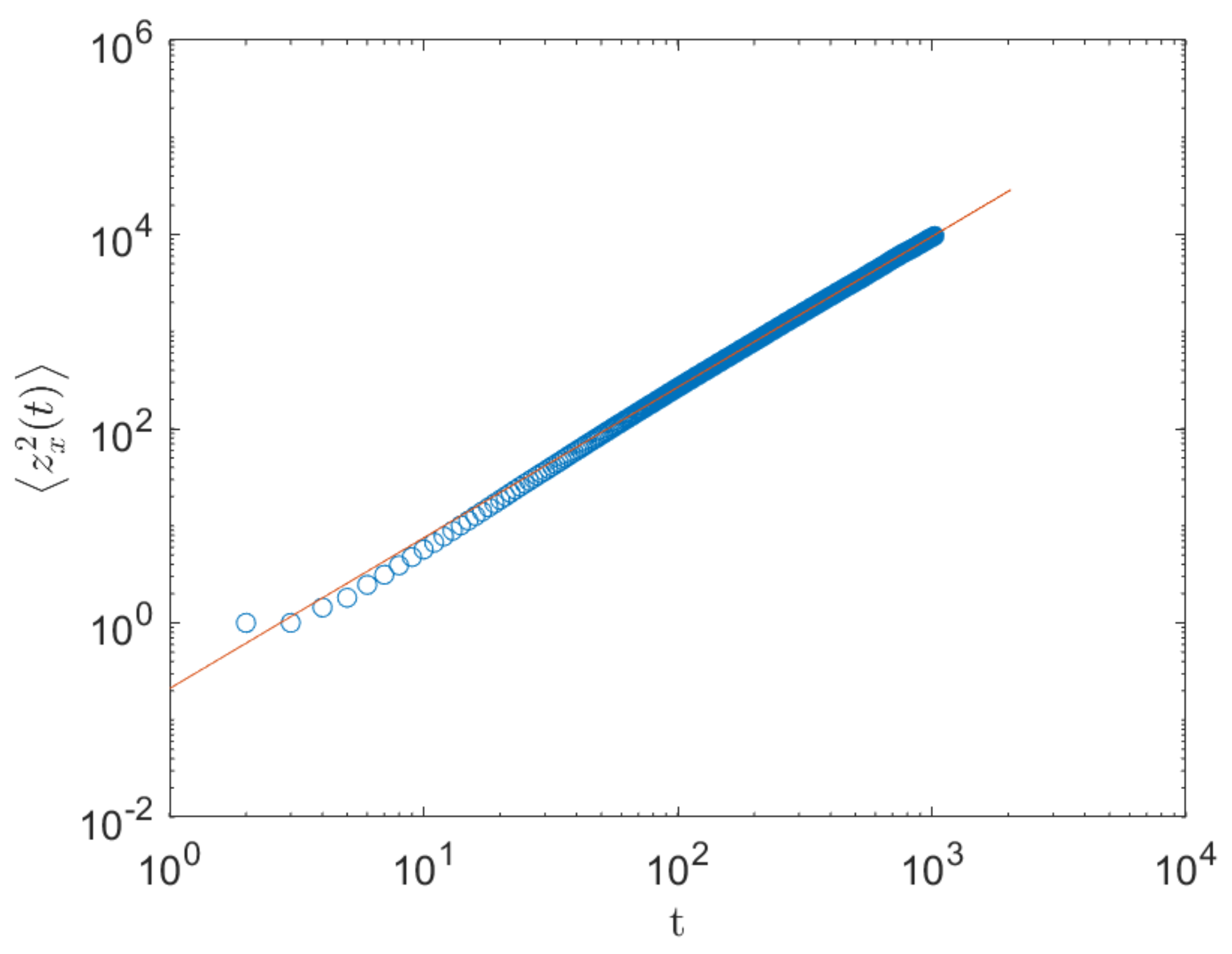
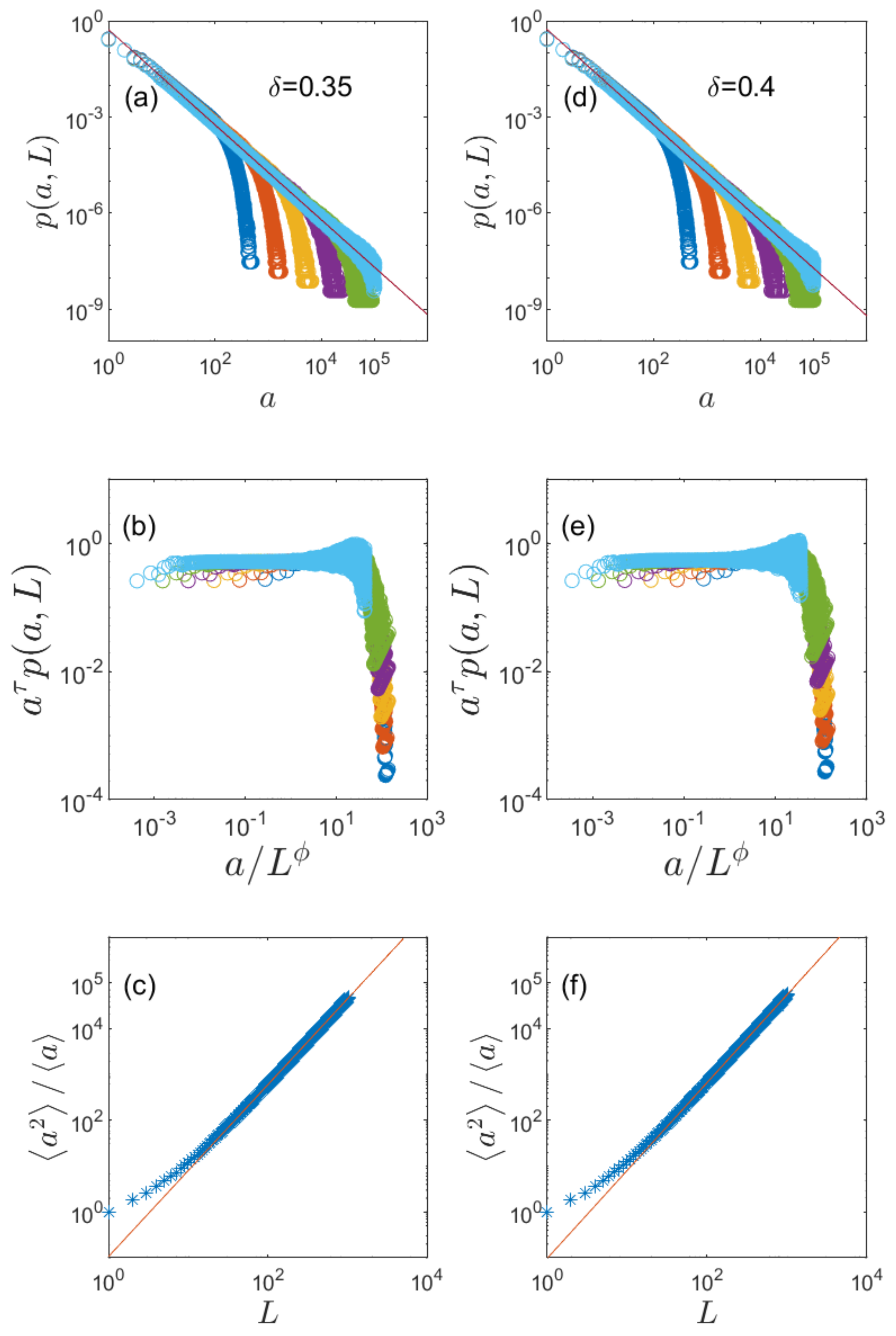
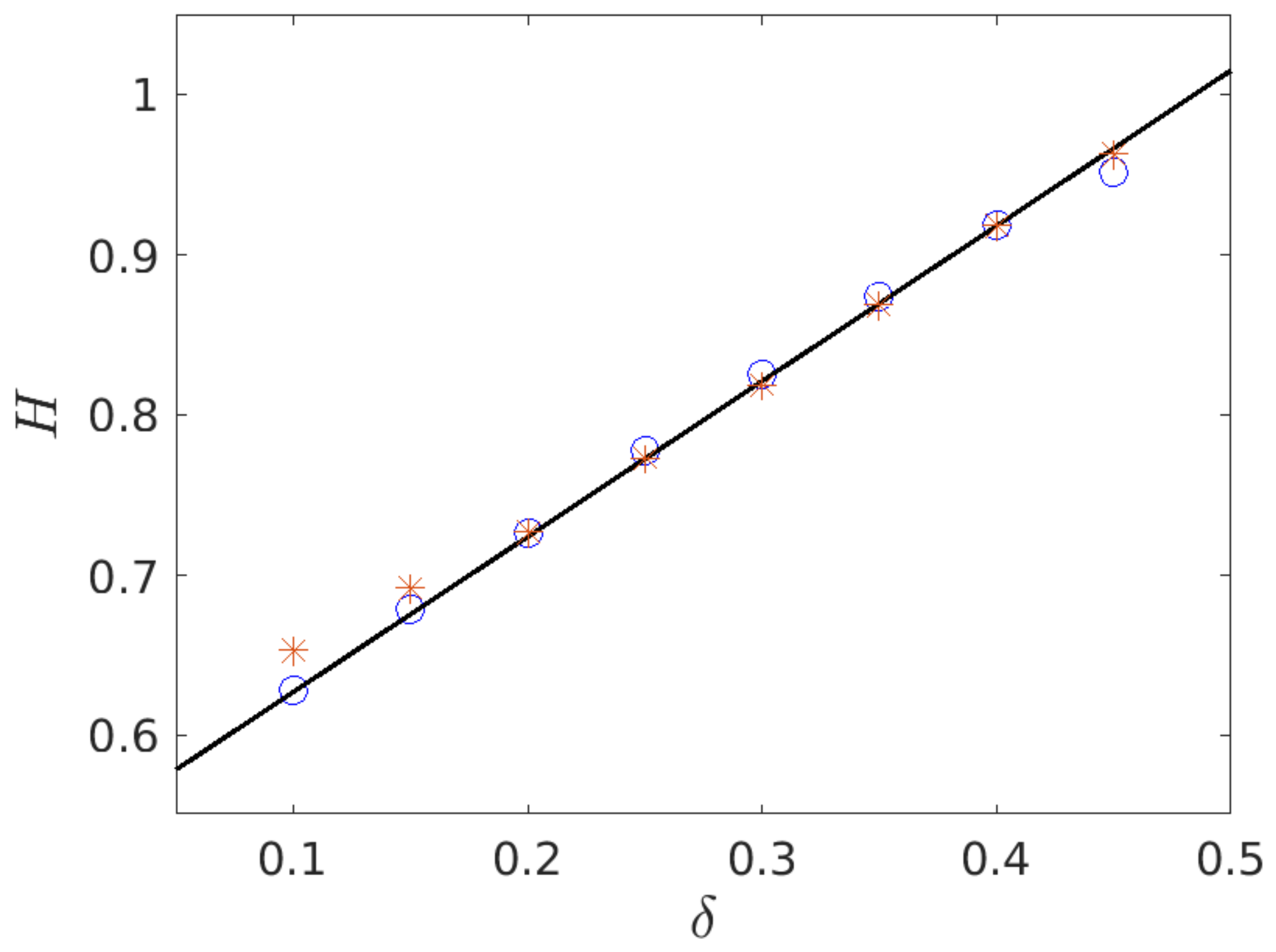
5. Results
6. Discussion
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mandelbrot, B.B. The Fractal Geometry of Nature; Freeman: New York, NY, USA, 1983. [Google Scholar]
- Rodríguez-Iturbe, I.; Rinaldo, A. Fractal River Basins: Chance and Self-Organization; Cambridge Univ Press: Cambridge, UK, 1997. [Google Scholar]
- Rinaldo, A.; Rigon, R.; Banavar, J.R.; Maritan, A.; Rodriguez-Iturbe, I. Evolution and selection of river networks: Statics, dynamics, and complexity. Proc. Natl. Acad. Sci. USA 2014, 111, 2417–2424. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Maritan, A.; Rinaldo, A.; Rigon, R.; Giacometti, A.; Rodriguez-Iturbe, I. Scaling laws for river networks. Phys. Rev. E 1996, 53, 1510. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dodds, P.S.; Rothman, D.H. Unified view of scaling laws for river networks. Phys. Rev. E 1999, 59, 4865. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hack, J.T. Studies of Longitudinal Stream-Profiles in Virginia and Maryland. U.S. Geol. Surv. Prof. Paper 1957, 294, 45–97. [Google Scholar]
- Caldarelli, G. Cellular models for river networks. Phys. Rev. E 2001, 63, 021118. [Google Scholar] [CrossRef] [Green Version]
- Rinaldo, A.; Rodriguez-Iturbe, I.; Rigon, R.; Ijjasz-Vasquez, E.; Bras, R.L. Self-organized fractal river networks. Phys. Rev. Lett. 1993, 70, 822. [Google Scholar] [CrossRef]
- Rodríguez-Iturbe, I.; Rinaldo, A.; Rigon, R.; Bras, R.L.; Marani, A.; Ijjász-Vásquez, E. Energy dissipation, runoff production, and the three-dimensional structure of river basins. Water Resour. Res. 1992, 28, 1095–1103. [Google Scholar] [CrossRef]
- Rodriguez-Iturbe, I.; Rinaldo, A.; Rigon, R.; Bras, R.L.; Ijjasz-Vasquez, E.; Marani, A. Fractal structures as least energy patterns: The case of river networks. Geophys. Res. Lett. 1992, 19, 889–892. [Google Scholar] [CrossRef]
- Rinaldo, A.; Rodriguez-Iturbe, I.; Rigon, I.R.; Bras, R.L.; Ijjasz-Vasquez, E.; Marani, A. Minimum energy and fractal structures of drainage networks. Water Resour. Res. 1992, 28, 2183–2195. [Google Scholar] [CrossRef]
- Briggs, L.A.; Krishnamoorthy, M. Exploring network scaling through variations on optimal channel networks. Proc. Natl. Acad. Sci. USA 2013, 110, 19295. [Google Scholar] [CrossRef] [Green Version]
- Takayasu, H.; Inaoka, H. New type of self-organized criticality in a model of erosion. Phys. Rev. Lett. 1992, 68, 966. [Google Scholar] [CrossRef]
- Inaoka, H.; Takayasu, H. Water erosion as a fractal growth process. Phys. Rev. E 1993, 47, 899. [Google Scholar] [CrossRef]
- Kramer, S.; Marder, M. Evolution of river networks. Phys. Rev. Lett. 1992, 68, 205. [Google Scholar] [CrossRef]
- Leheny, R.L.; Nagel, S.R. Model for the evolution of river networks. Phys. Rev. Lett. 1993, 71, 1470. [Google Scholar] [CrossRef]
- Giacometti, A.; Maritan, A.; Banavar, J.R. Continuum model for river networks. Phys. Rev. Lett. 1995, 577, 577. [Google Scholar] [CrossRef] [Green Version]
- Banavar, J.R.; Colaiori, F.; Flammini, A.; Giacometti, A.; Maritan, A.; Rinaldo, A. Sculpting of a Fractal River Basin. Phys. Rev. Lett. 1997, 78, 4522. [Google Scholar]
- Somfai, E.; Sander, L.M. Scaling and river networks: A Landau theory for erosion. Phys. Rev. E 1997, 56, R5. [Google Scholar] [CrossRef] [Green Version]
- Kim, H.-J.; Kim, I.-M.; Kim, J.M. River networks on the slope-correlated landscape. Phys. Rev. E 2000, 62, 3121. [Google Scholar] [CrossRef]
- Bak, P.; Tang, C.; Wiesenfeld, K. Self-organized criticality: An explanation of the 1/f noise. Phys. Rev. Lett. 1987, 59, 381. [Google Scholar] [CrossRef]
- Bak, P.; Tang, C.; Wiesenfeld, K. Self-organized criticality. Phys. Rev. A 1988, 38, 364. [Google Scholar] [CrossRef]
- Hergarten, S.; Neugebauer, H.J. Self-organized critical drainage networks. Phys. Rev. Lett. 2001, 86, 2689. [Google Scholar] [CrossRef] [PubMed]
- Scheidegger, A.E. Horton’s laws of stream lengths and drainage areas. Water Resour. Res. 1968, 4, 1015. [Google Scholar] [CrossRef]
- Abouagwa, M.; Aljoufi, L.S.; Bantan, R.A.R.; Khalaf, A.D.; Elgarhy, M. Mixed Neutral Caputo Fractional Stochastic Evolution Equations with Infinite Delay: Existence, Uniqueness and Averaging Principle. Fractal Fract. 2022, 6, 105. [Google Scholar] [CrossRef]
- Khalaf, A.D.; Saeed, T.; Abu-Shanab, R.; Almutiry, W.; Abouagwa, M. Estimating Drift Parameters in a Sub–Fractional Vasicek–Type Process. Entropy 2022, 24, 594. [Google Scholar] [CrossRef] [PubMed]
- Mandelbrot, B.B.; van Ness, J.W. Fractional Brownian motions, fractional noises and applications. SIAM Rev. 1968, 10, 422–437. [Google Scholar] [CrossRef]
- Metzler, R.; Klafter, J. The random walk’s guide to anomalous diffusion: A fractional dynamics approach. Phys. Rep. 2000, 39, 1–77. [Google Scholar] [CrossRef]
- Metzler, R.; Klafter, J. The restaurant at the end of the random walk: Recent developments in the description of anomalous transport by fractional dynamics. Phys. A: Math. Gen. 2004, 37, R161. [Google Scholar] [CrossRef]
- Klafter, J.; Sokolov, I.M. First Steps in Random Walks, 1st ed.; Oxford University Press: New York, NY, USA, 2011. [Google Scholar]
- Zaburdaev, V.; Denisov, S.; Klafter, J. Lévy walks. Rev. Mod. Phys. 2015, 87, 483. [Google Scholar] [CrossRef] [Green Version]
- Dybiec, B.; Gudowska-Nowak, E.; Barkai, E.; Dubkov, A.A. Lévy flights versus Lévy walks in bounded domains. Phys. Rev. E 2017, 95, 052102. [Google Scholar] [CrossRef] [Green Version]
- Fedotov, S.; Korab, N. Emergence of Lévy walks in systems of interacting individuals. Phys. Rev. E 2017, 95, 030107. [Google Scholar] [CrossRef] [Green Version]
- Zaburdaev, V.; Fouxon, I.; Denisov, S.; Barkai, E. Superdiffusive Dispersals Impart the Geometry of Underlying Random Walks. Phys. Rev. Lett. 2016, 117, 270601. [Google Scholar] [CrossRef] [Green Version]
- Boyer, D.; Pineda, I. Slow Lévy flights. Phys. Rev. E 2016, 93, 022103. [Google Scholar] [CrossRef]
- Rebenshtok, A.; Denisov, S.; Hänggi, P.; Barkai, E. Infinite densities for Lévy walks. Phys. Rev. E 2014, 90, 062135. [Google Scholar] [CrossRef] [Green Version]
- Godec, A.; Metzler, R. Linear response, fluctuation-dissipation, and finite-system-size effects in superdiffusion. Phys. Rev. E 2013, 88, 012116. [Google Scholar] [CrossRef] [Green Version]
- Schutz, G.M.; Trimper, S. Elephants can always remember: Exact long-range memory effects in a non-Markovian random walk. Phys. Rev. E 2004, 70, 045101. [Google Scholar] [CrossRef] [Green Version]
- Boyer, D.; Solis-Salas, C. Random Walks with Preferential Relocations to Places Visited in the Past and their Application to Biology. Phys. Rev. Lett. 2014, 112, 240601. [Google Scholar] [CrossRef] [Green Version]
- Boyer, D.; Romo-Cruz, J.C.R. Solvable random-walk model with memory and its relations with Markovian models of anomalous diffusion. Phys. Rev. E 2014, 90, 042136. [Google Scholar] [CrossRef] [Green Version]
- Cressoni, J.C.; da Silva, M.A.A.; Viswanathan, G.M. Amnestically induced persistence in random walks. Phys. Rev. Lett. 2007, 98, 070603. [Google Scholar] [CrossRef] [Green Version]
- Liemert, A.; Sandev, T.; Kantz, H. Generalized Langevin equation with tempered memory kernel. Physica A 2017, 466, 356–369. [Google Scholar] [CrossRef]
- Budini, A.A. Memory-induced diffusive-superdiffusive transition: Ensemble and time-averaged observables. Phys. Rev. E 2017, 95, 052110. [Google Scholar] [CrossRef] [Green Version]
- Budini, A.A. Inhomogeneous diffusion and ergodicity breaking induced by global memory effects. Phys. Rev. E 2016, 94, 052142. [Google Scholar] [CrossRef] [Green Version]
- Budini, A.A. Weak ergodicity breaking induced by global memory effects. Phys. Rev. E 2016, 94, 022108. [Google Scholar] [CrossRef] [PubMed]
- Kürsten, R. Random recursive trees and the elephant random walk. Phys. Rev. E 2016, 93, 032111. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kumar, N.; Harbola, U.; Lindenberg, K. Memory-induced anomalous dynamics: Emergence of diffusion, subdiffusion, and superdiffusion from a single random walk model. Phys. Rev. E 2010, 82, 021101. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kim, H.-J. Anomalous diffusion induced by enhancement of memory. Phys. Rev. E 2014, 90, 012103. [Google Scholar] [CrossRef] [Green Version]
- Choi, Y.; Kim, H.-J. Nonstationary Markovian replication process causing diverse diffusions. Phys. Rev. E 2017, 96, 042144. [Google Scholar] [CrossRef] [Green Version]
- Kim, D.; Kim, H.-J. Transition phenomena in diffusive motions due to the change of memory effects. Mod. Phys. Lett. B 2019, 96, 2050013. [Google Scholar] [CrossRef]
- Goldstein, S. On diffusion by discontinuous movements, and on the telegraph equation. Q. J. Mech. Appl. Math. 1951, 4, 129–156. [Google Scholar] [CrossRef]
- Kac, M. A stochastic model related to the telegrapher’s equation. Rocky Mt. J. Math. 1974, 4, 497–509. [Google Scholar] [CrossRef]
- Masoliver, J.; Lindenberg, K.; Weiss, G.H. A continuous-time generalization of the persistent random walk. Physica A 1989, 157, 891–898. [Google Scholar] [CrossRef]
- Masoliver, J.; Weiss, G.H. Finite-velocity diffusion. Eur. J. Phys. 1996, 190, 17. [Google Scholar] [CrossRef]
- Masoliver, J.; Weiss, G.H. Telegrapher’s equations with variable propagation speeds. Phys. Rev. E 1994, 49, 3852. [Google Scholar] [CrossRef]
- Weiss, G.H. Some applications of persistent random walks and the telegrapher’s equation. Physica A 2002, 311, 381–410; [Google Scholar] [CrossRef]
- Masoliver, J. Fractional telegrapher’s equation from fractional persistent random walks. Phys. Rev. E 2016, 93, 052107. [Google Scholar] [CrossRef] [Green Version]
- Wang, K.G. Long-time-correlation effects and biased anomalous diffusion. Phys. Rev. E 1992, 45, 833. [Google Scholar] [CrossRef]
- Wang, K.G.; Lung, C.W. Long-time correlation effects and fractal Brownian motion. Phys. Lett. A 1990, 151, 119–121. [Google Scholar] [CrossRef]
- Lim, S.C.; Muniandy, S.V. Self-similar Gaussian processes for modeling anomalous diffusion. Phys. Rev. E 2002, 66, 021114. [Google Scholar] [CrossRef]
- Thiel, F.; Sokolov, I.M. Scaled Brownian motion as a mean-field model for continuous-time random walks. Phys. Rev. E 2014, 89, 012115. [Google Scholar] [CrossRef] [Green Version]
- Horton, R.E. Erosional development of streams and their drainage basins; hydrophysical approach to quantitative morphology. Geol. Soc. Am. Bull. 1945, 56, 275–370. [Google Scholar] [CrossRef] [Green Version]
- Kirchner, J.W. Statistical inevitability of Horton’s laws and the apparent randomness of stream channel networks. Geology 1993, 21, 591. [Google Scholar] [CrossRef]
- Beer, T.; Borgas, M. Horton’s laws and the fractal nature of streams. Water Resour. Res. 1993, 29, 1475. [Google Scholar] [CrossRef]
- Rosso, R.; Bacchi, B.; La Barbera, P. Fractal relation of mainstream length to catchment area in river networks. Water Resour. Res. 1991, 27, 381–387. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, H.-J. Fractal Characteristics of River Networks Induced by Dynamical Stochastic Replication. Fractal Fract. 2022, 6, 569. https://doi.org/10.3390/fractalfract6100569
Kim H-J. Fractal Characteristics of River Networks Induced by Dynamical Stochastic Replication. Fractal and Fractional. 2022; 6(10):569. https://doi.org/10.3390/fractalfract6100569
Chicago/Turabian StyleKim, Hyun-Joo. 2022. "Fractal Characteristics of River Networks Induced by Dynamical Stochastic Replication" Fractal and Fractional 6, no. 10: 569. https://doi.org/10.3390/fractalfract6100569
APA StyleKim, H.-J. (2022). Fractal Characteristics of River Networks Induced by Dynamical Stochastic Replication. Fractal and Fractional, 6(10), 569. https://doi.org/10.3390/fractalfract6100569




