Chaos Control for a Fractional-Order Jerk System via Time Delay Feedback Controller and Mixed Controller
Abstract
:1. Introduction
- Control the chaotic behavior of system (5) via designing a suitable time delay feedback controller;
- Control the chaotic behavior of system (5) via designing an appropriate mixed controller which includes time delay feedback controller and fractional-order PDσ controller. Up to now, there have been very few papers that deal with the chaos control via this mixed controller.
- Based on the previous publications, we build a novel Jerk system.
- A suitable time delay feedback controller is successfully designed to suppress the chaotic behavior of Jerk system (5);
- A suitable mixed controller which includes time delay feedback controller and fractional-order PDσ controller is successfully designed to suppress the chaotic behavior of the Jerk system (5);
- The research idea can also be applied to deal with the chaos control issue for numerous other fractional-order differential systems in many areas.
2. Preliminary Theory
3. Chaos Control via Time Delay Feedback Controller
4. Chaos Control via Fractional-Order PD Controller
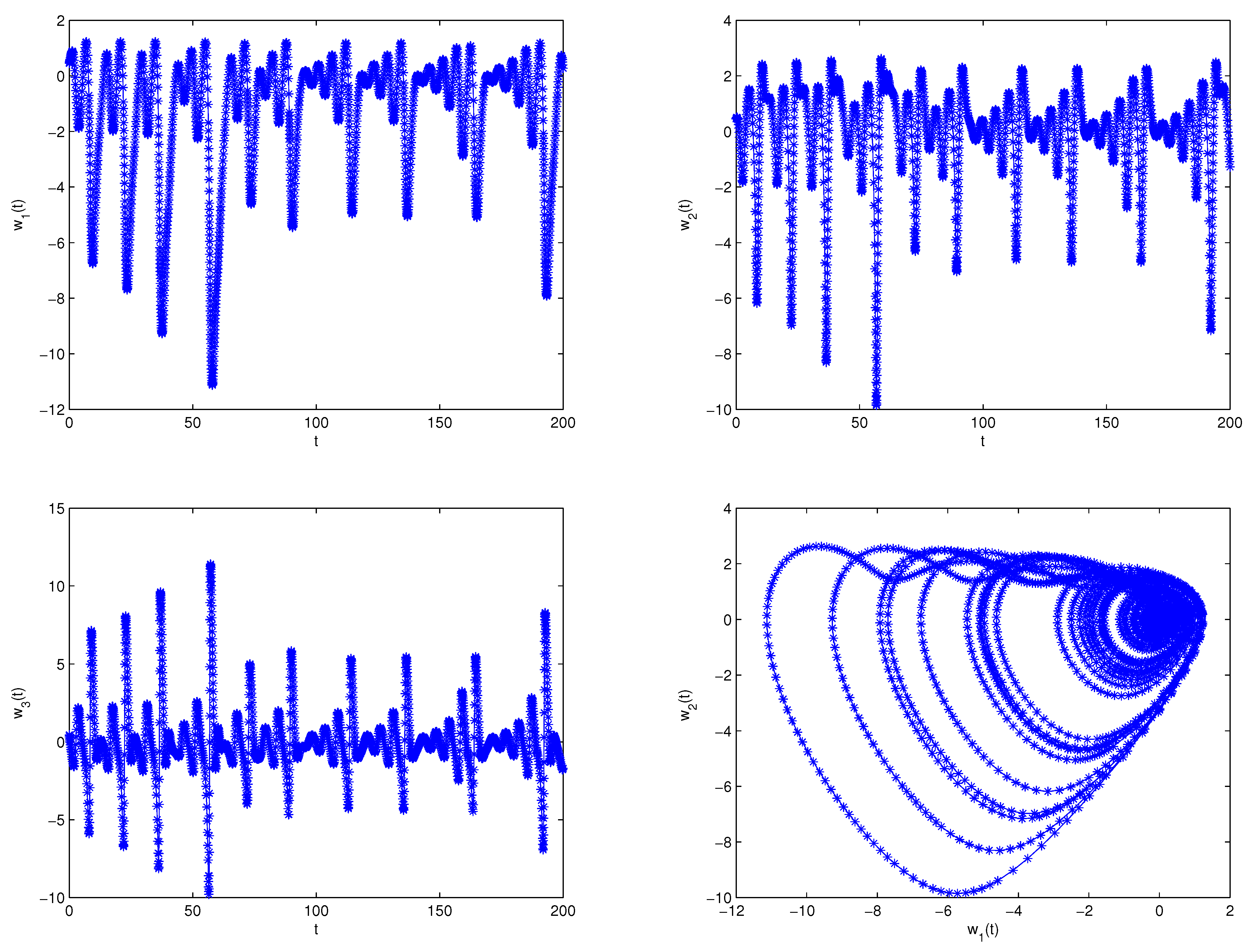
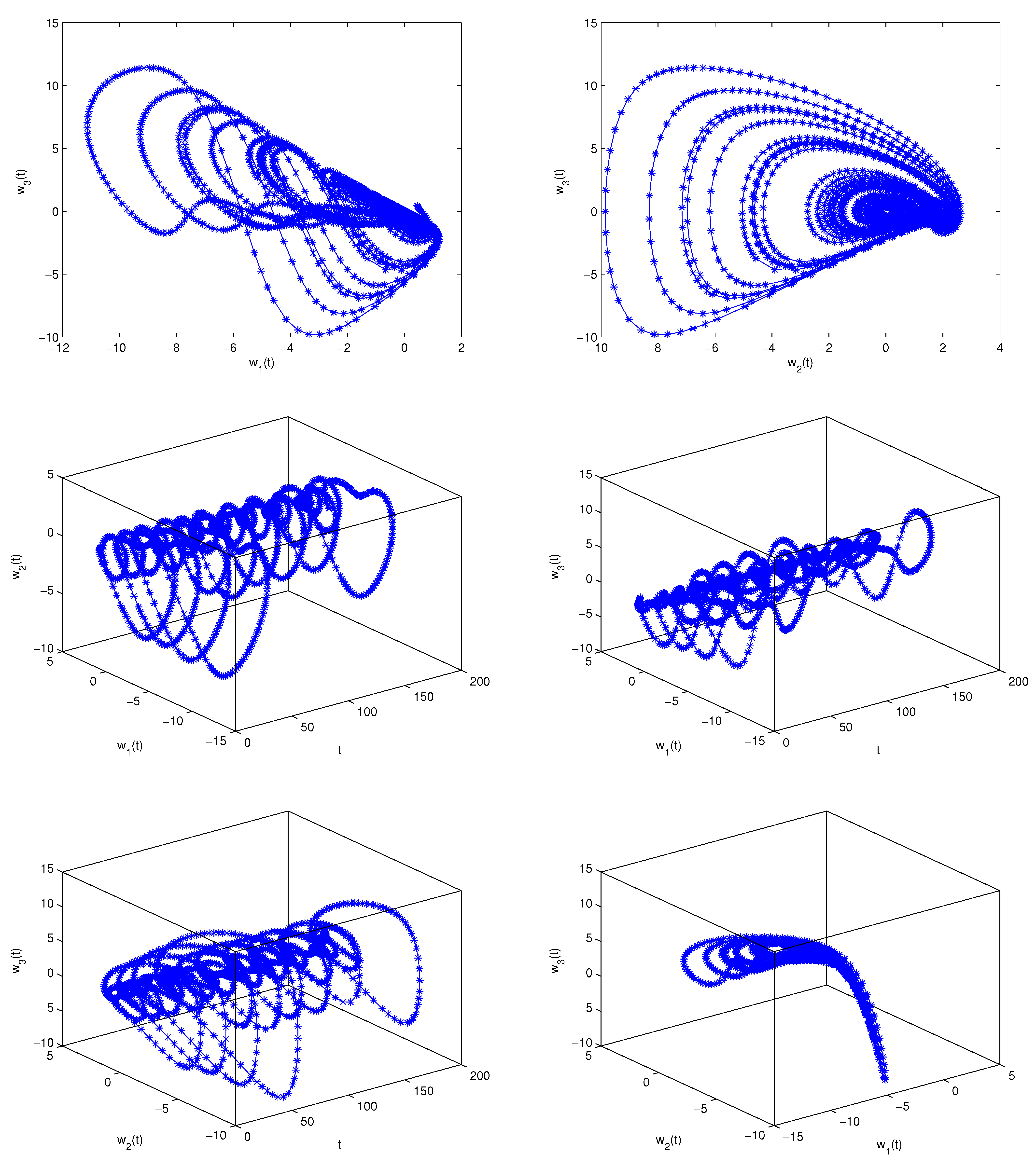
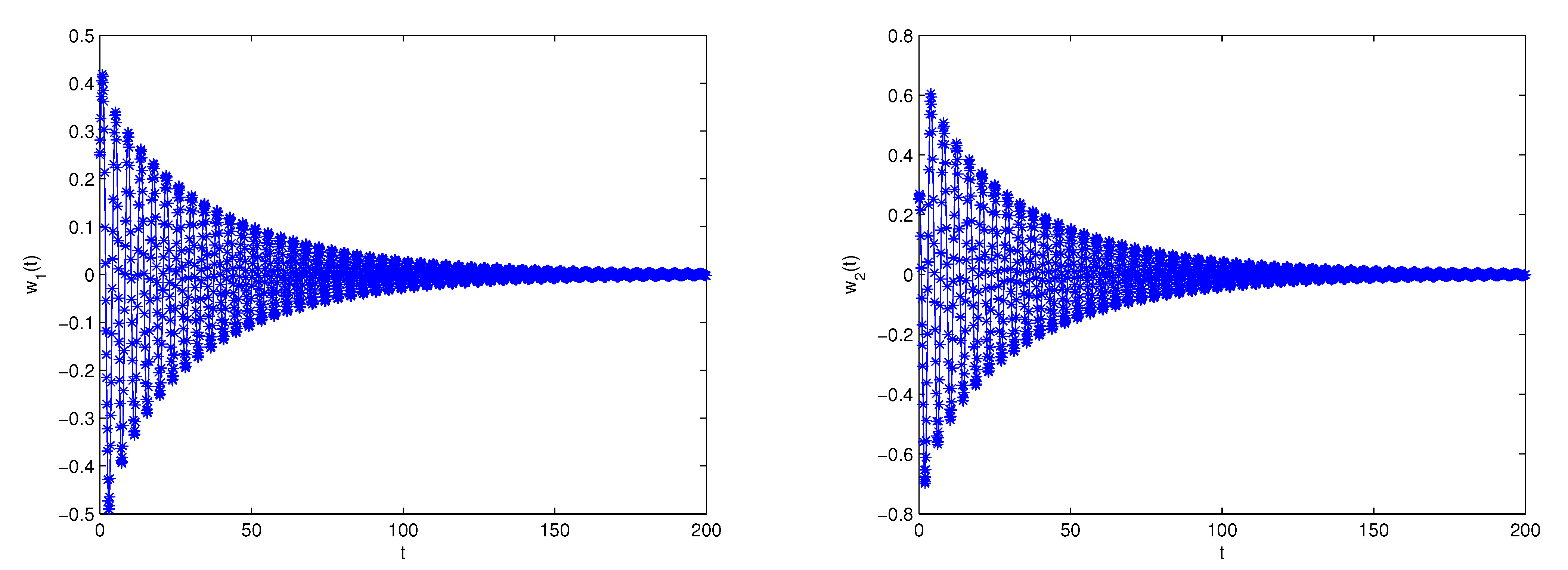
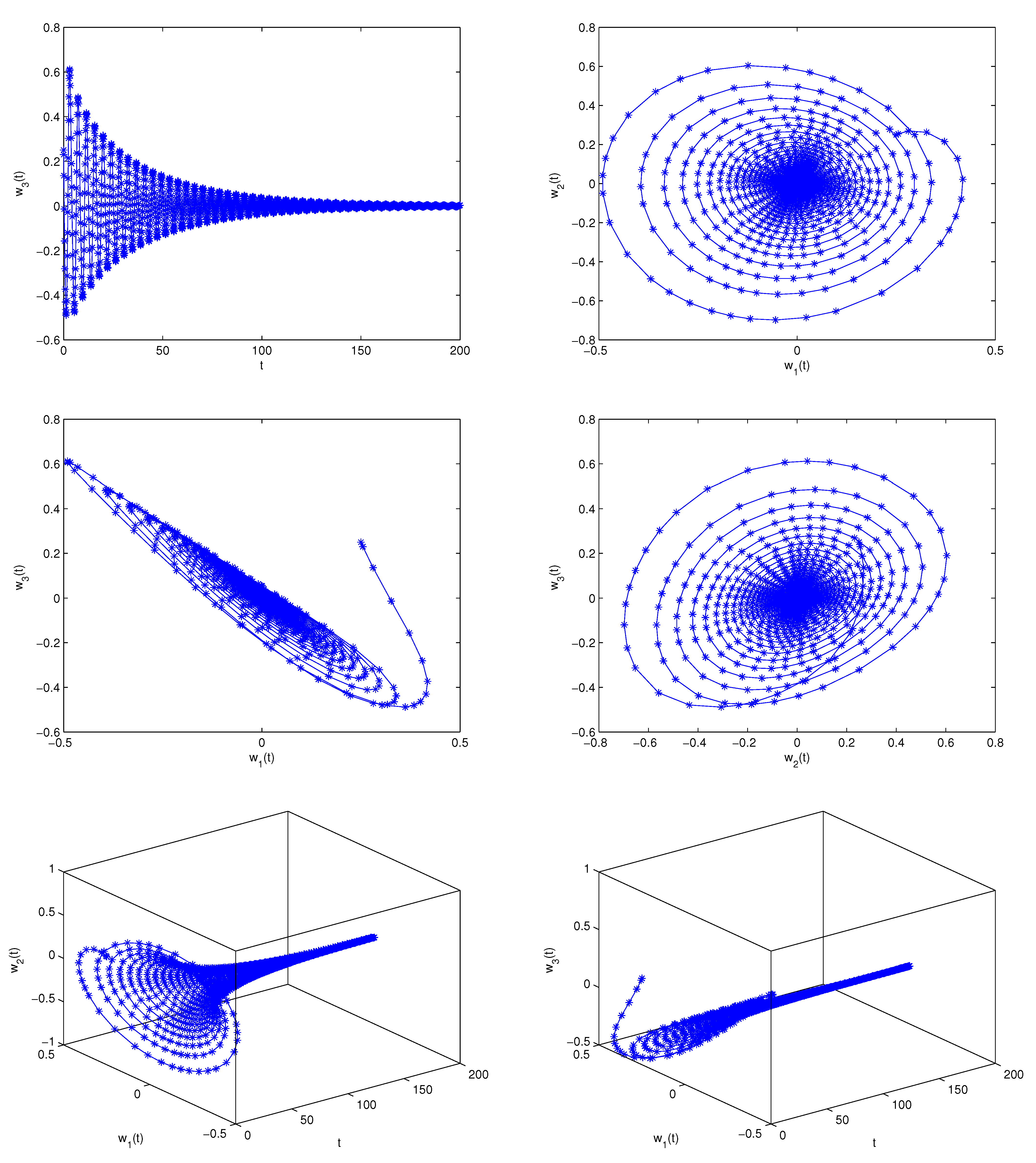
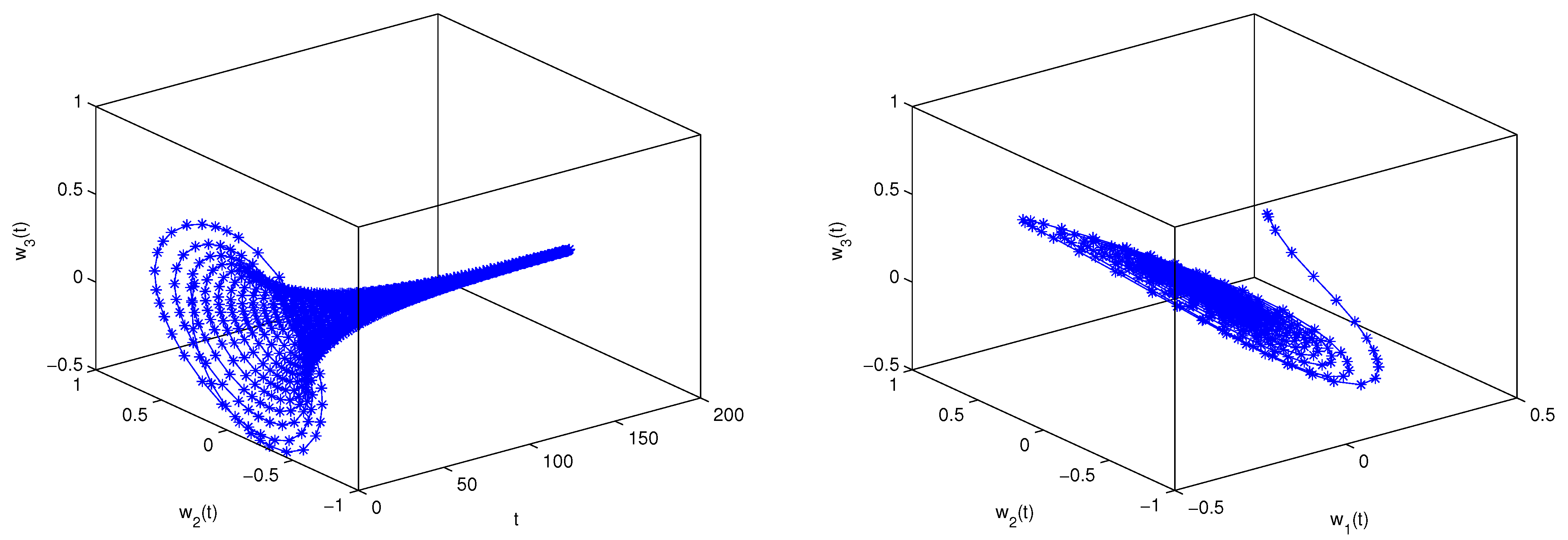
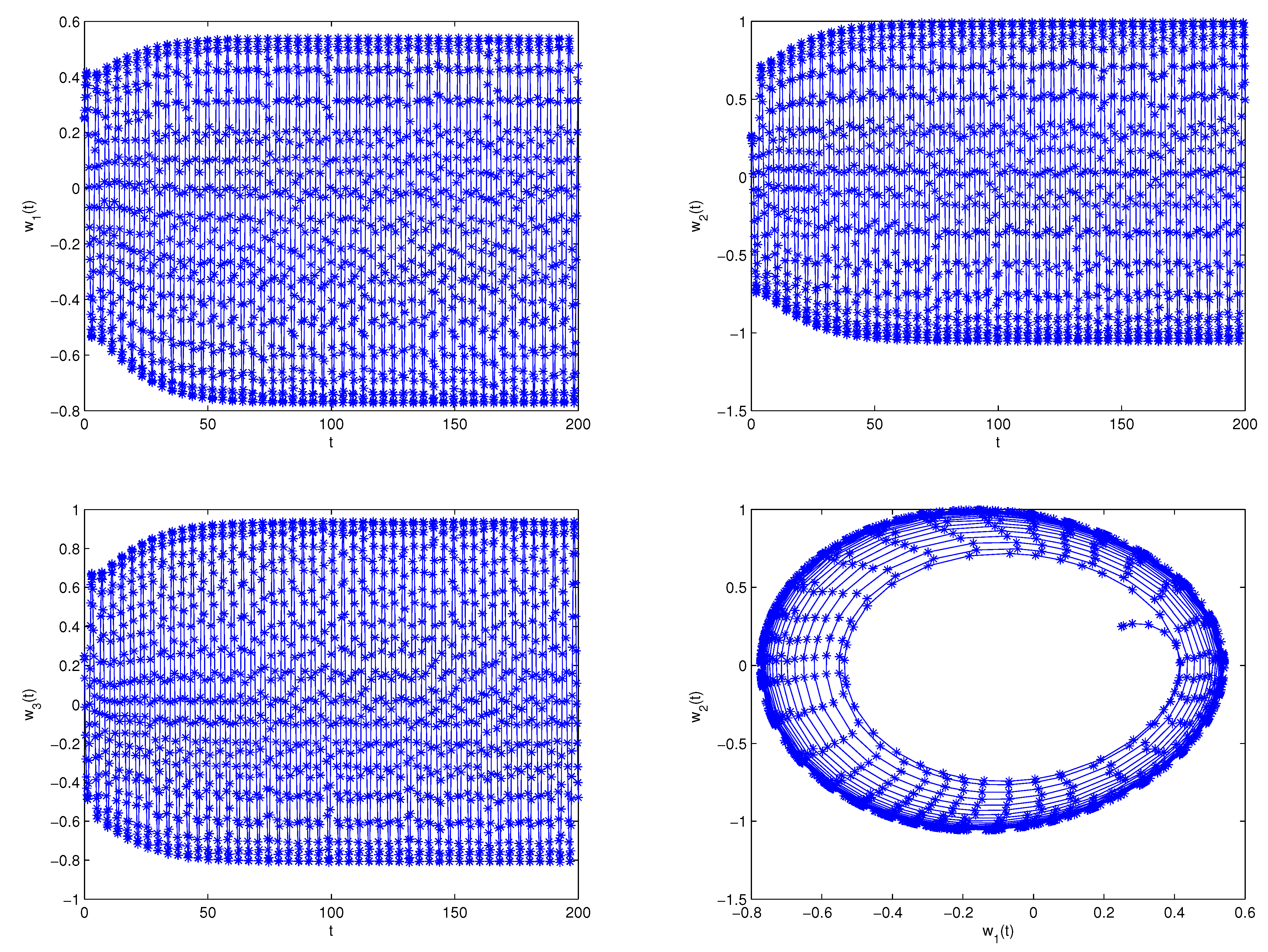
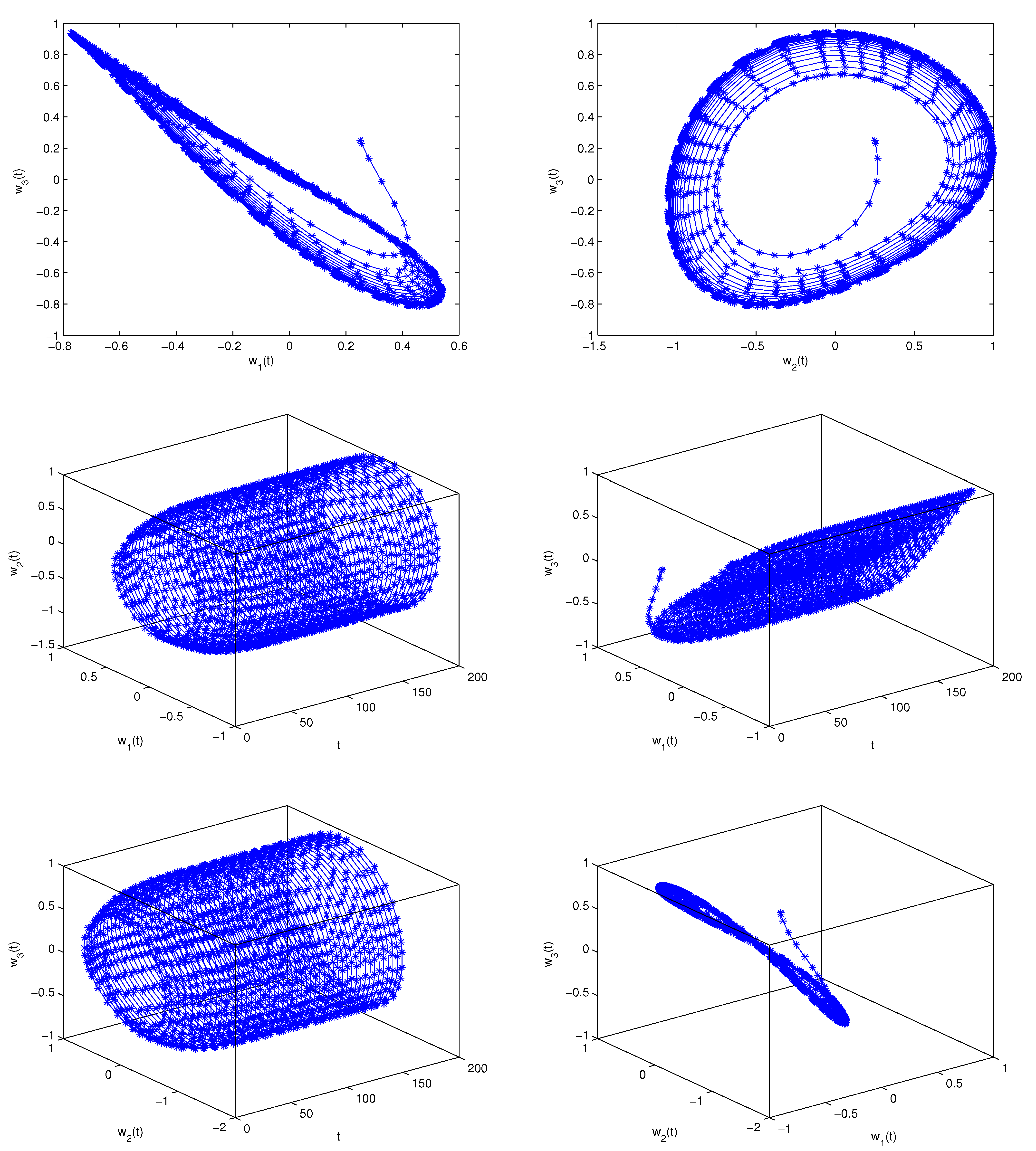
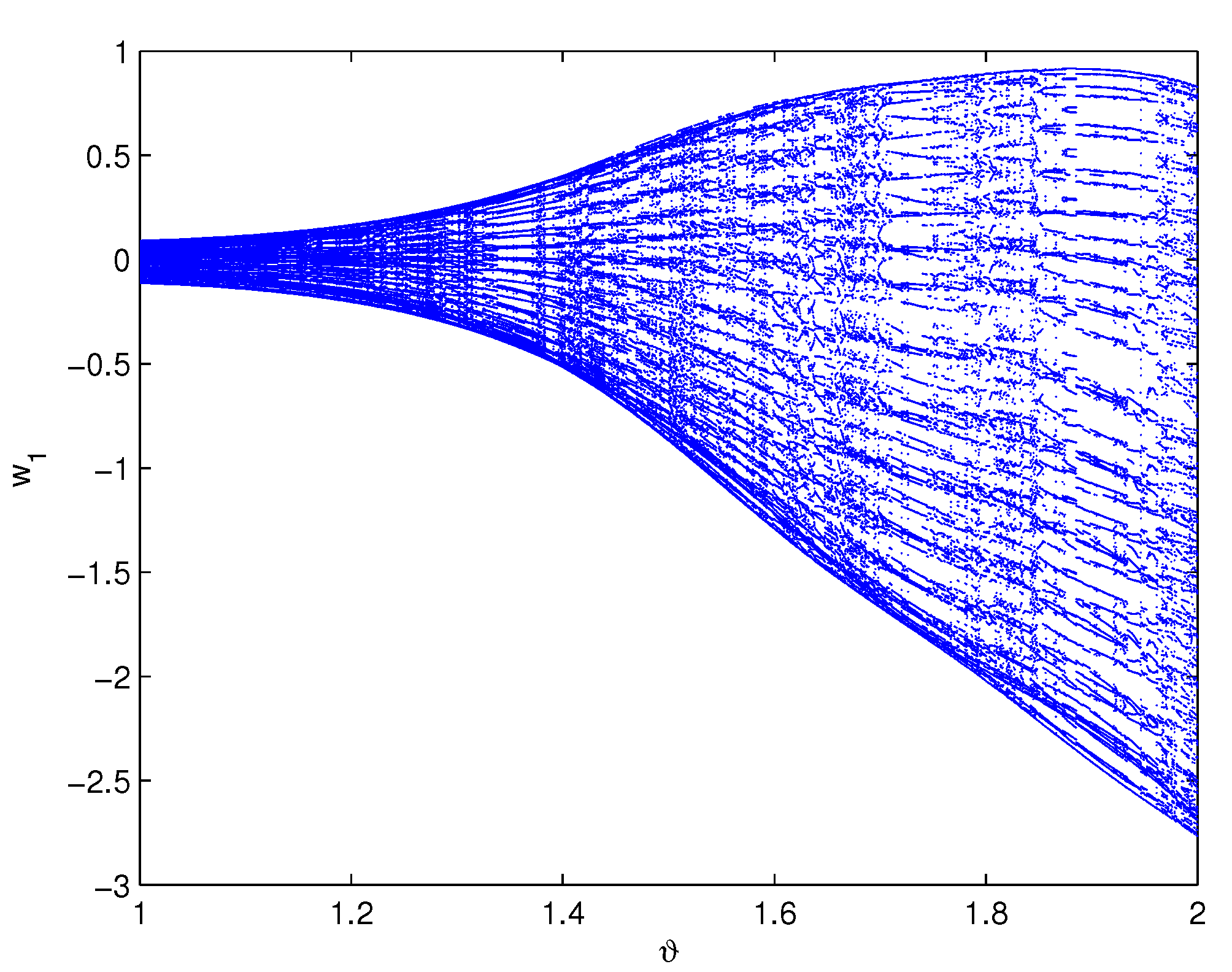
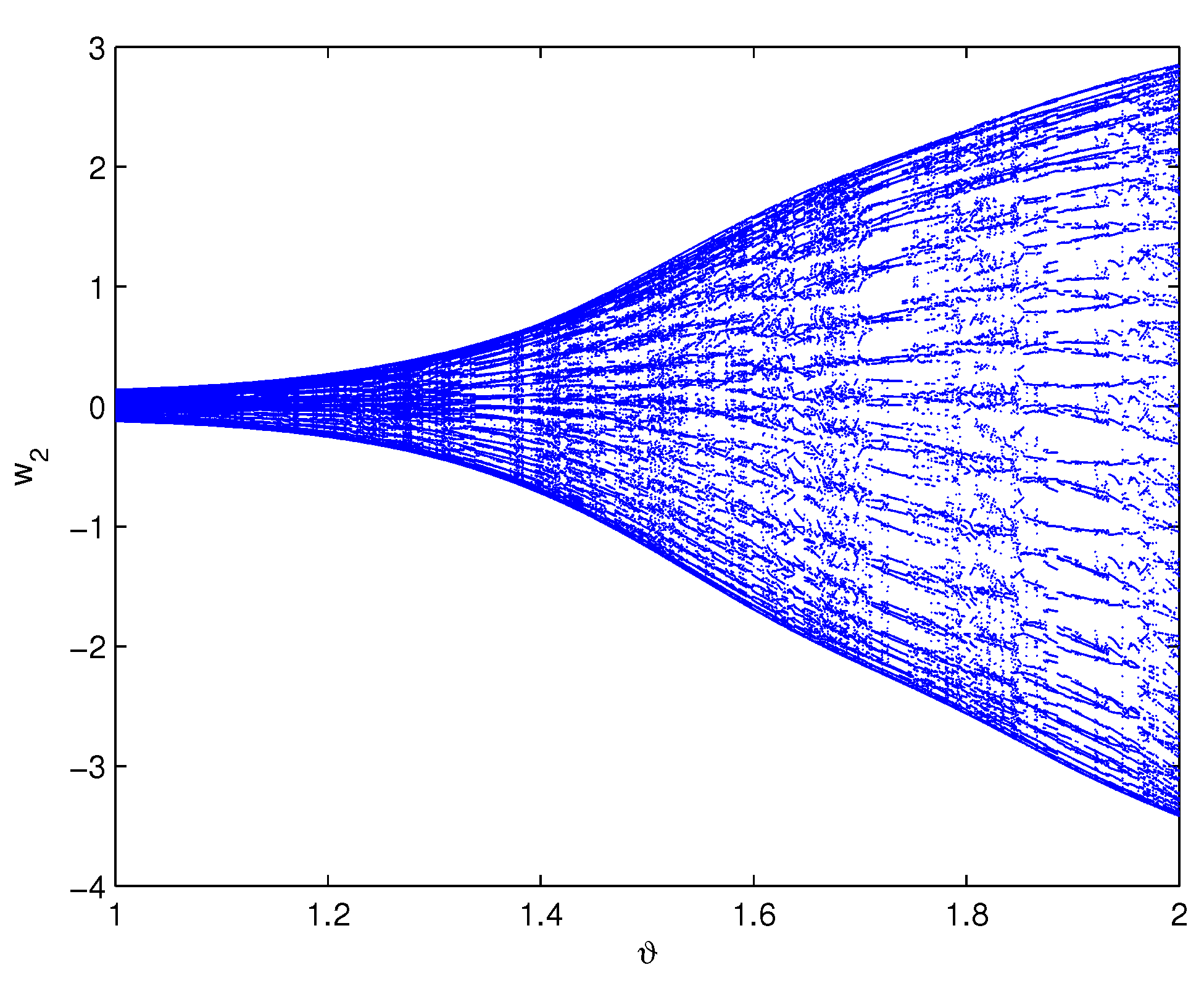
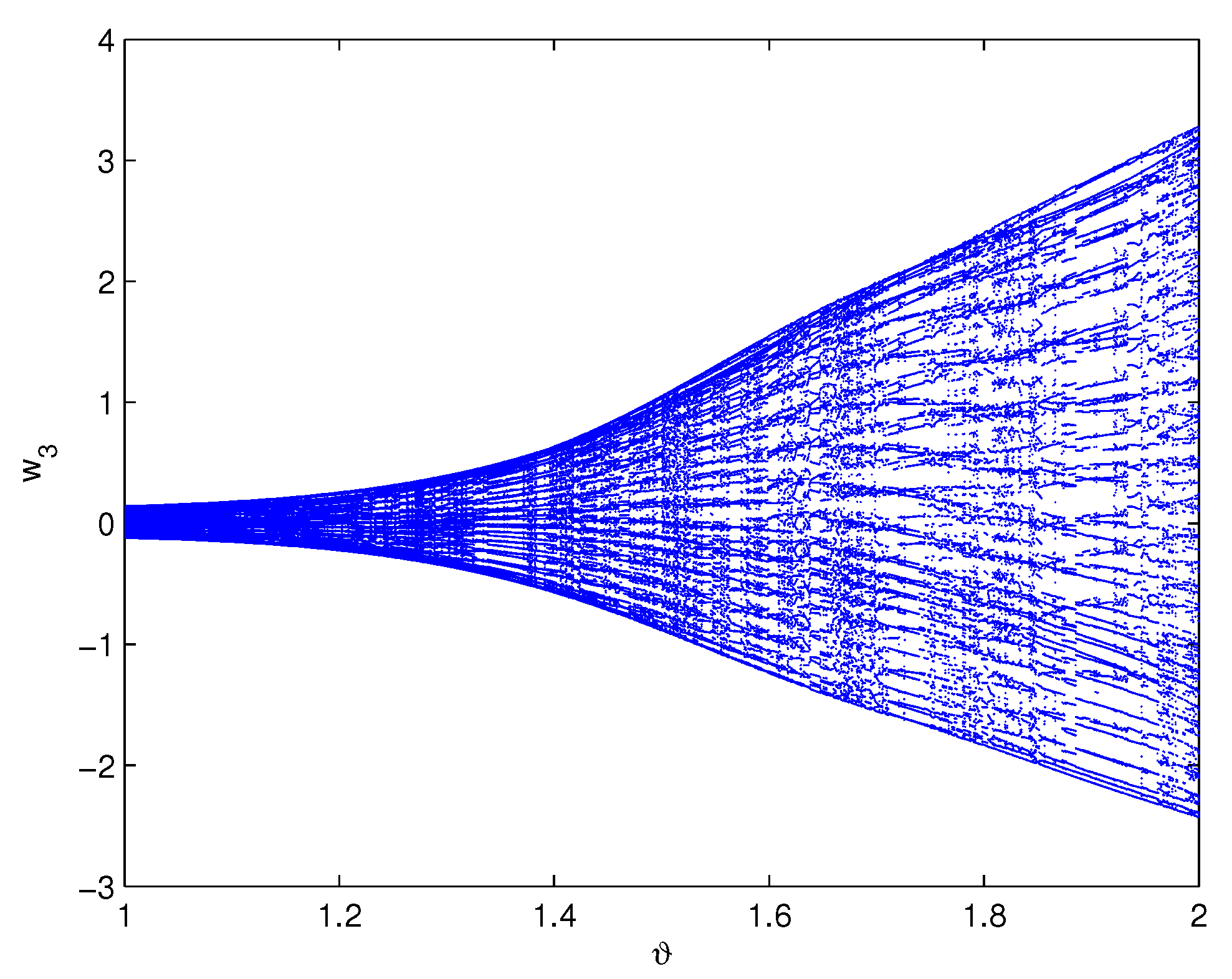
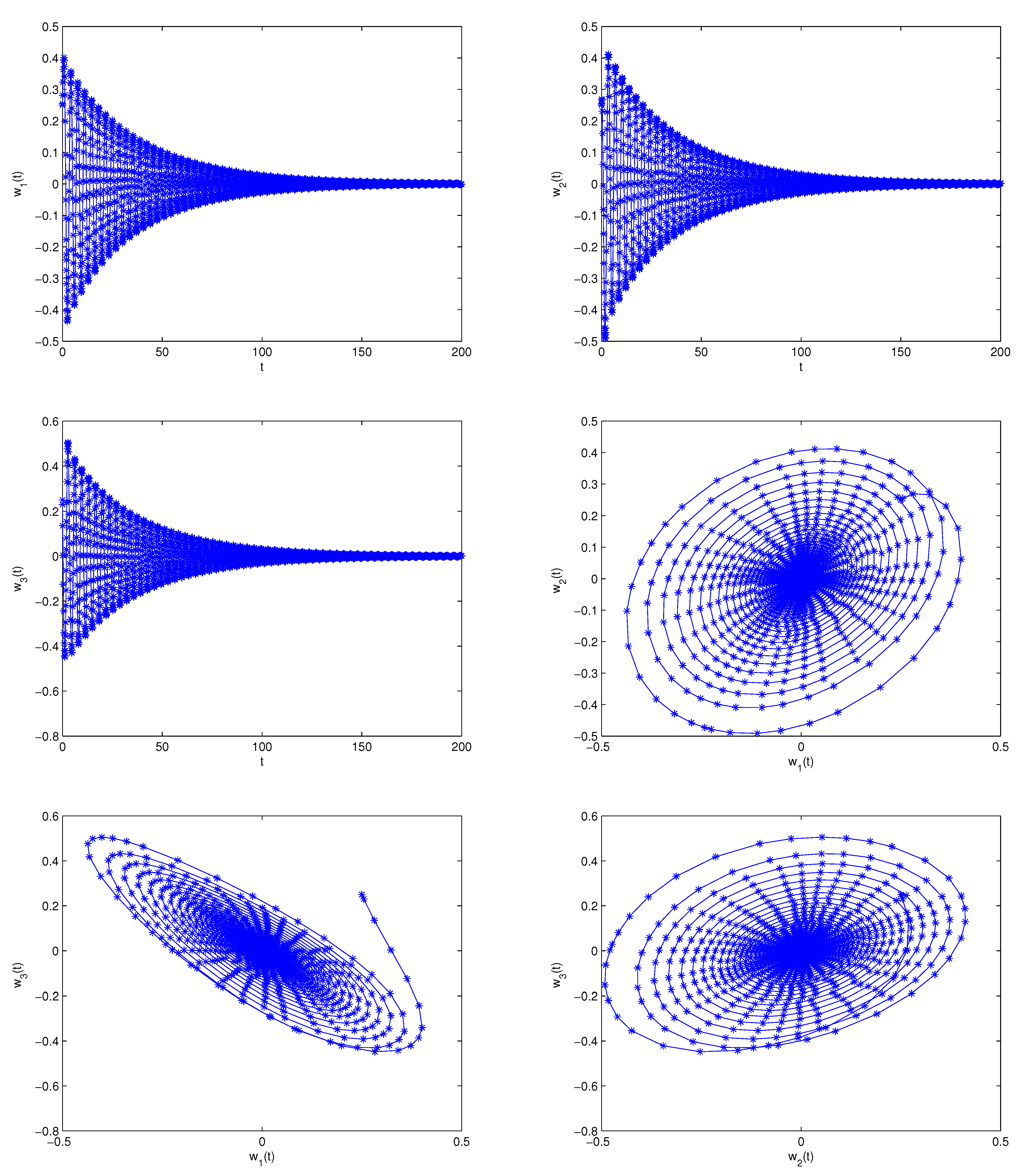
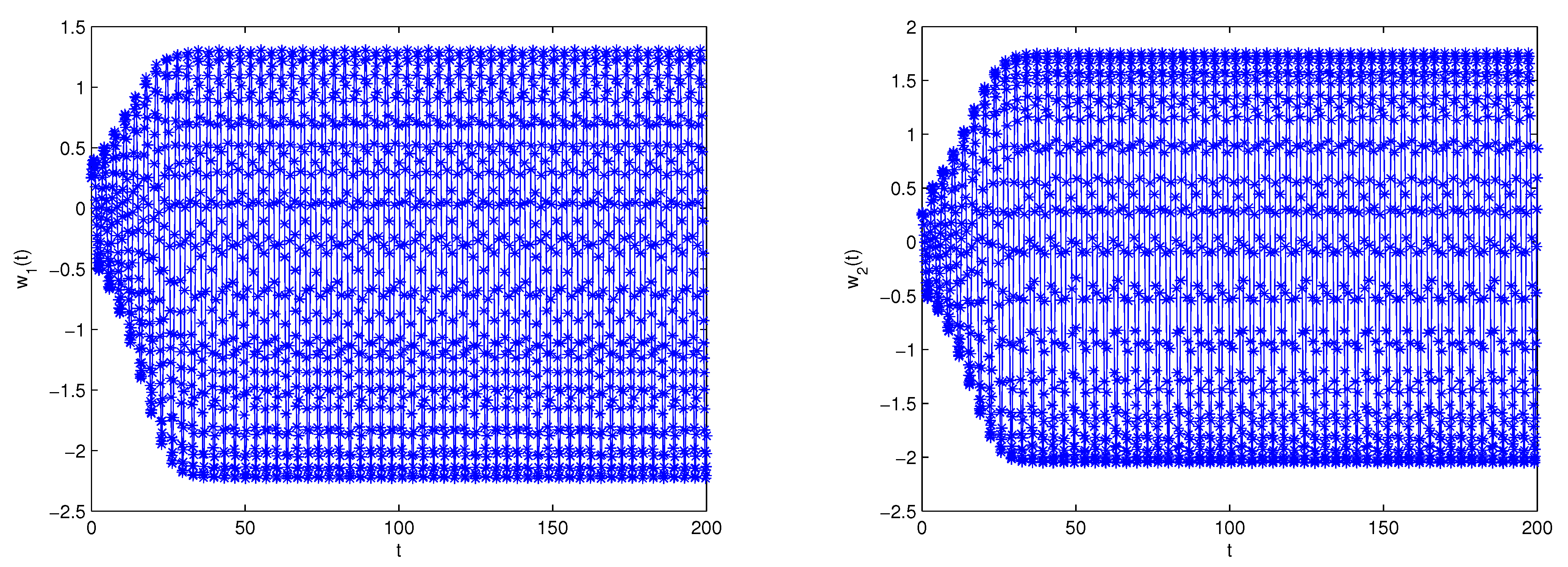
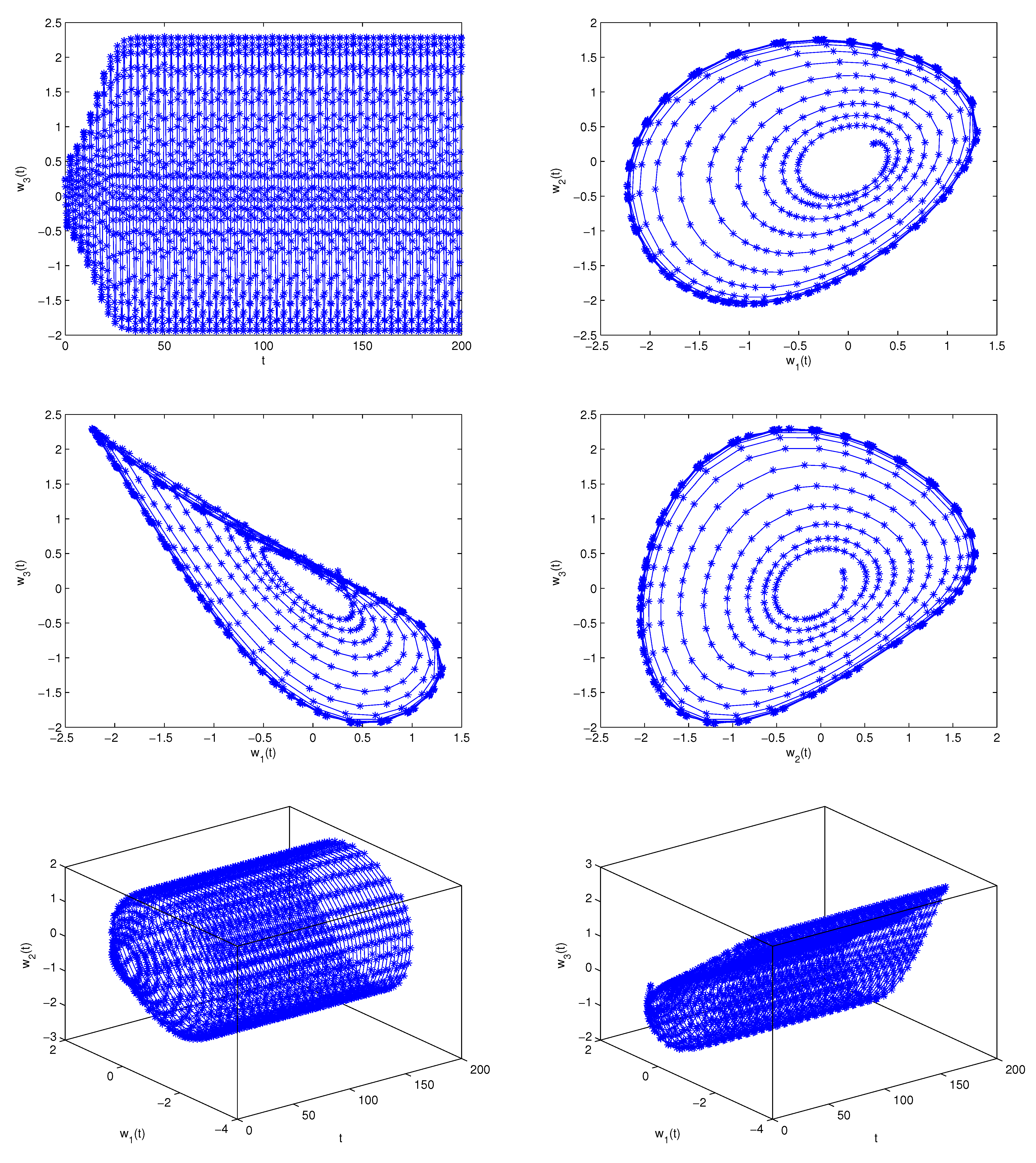
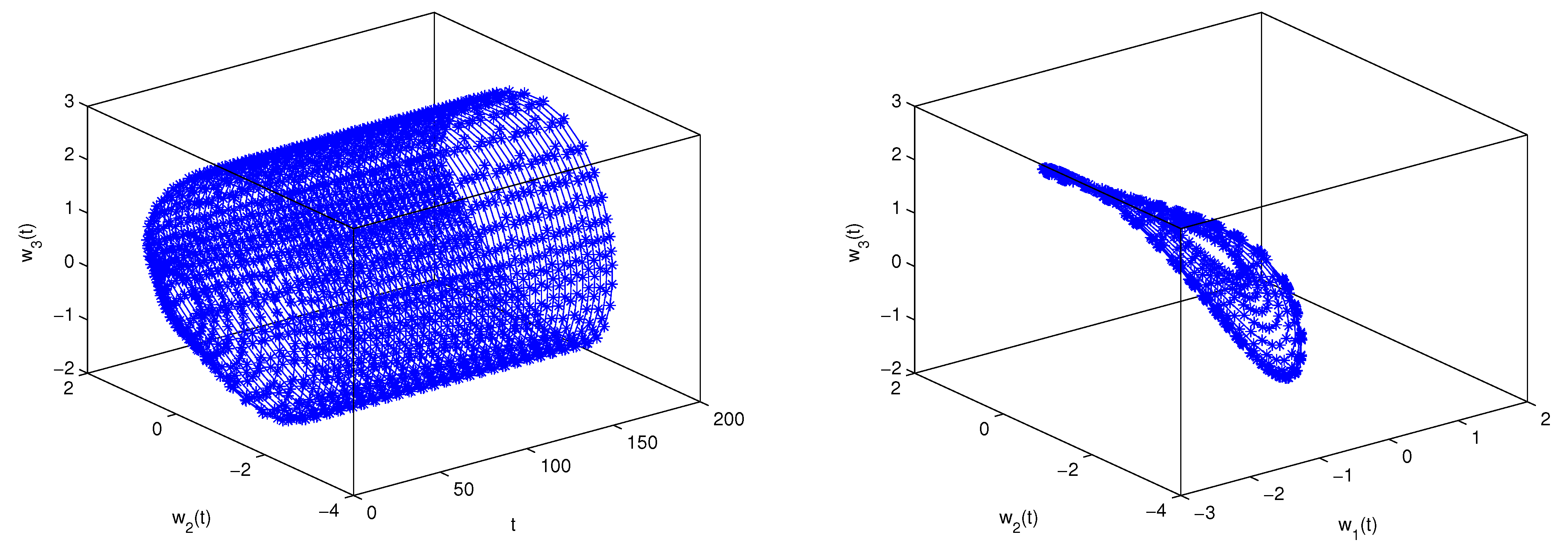
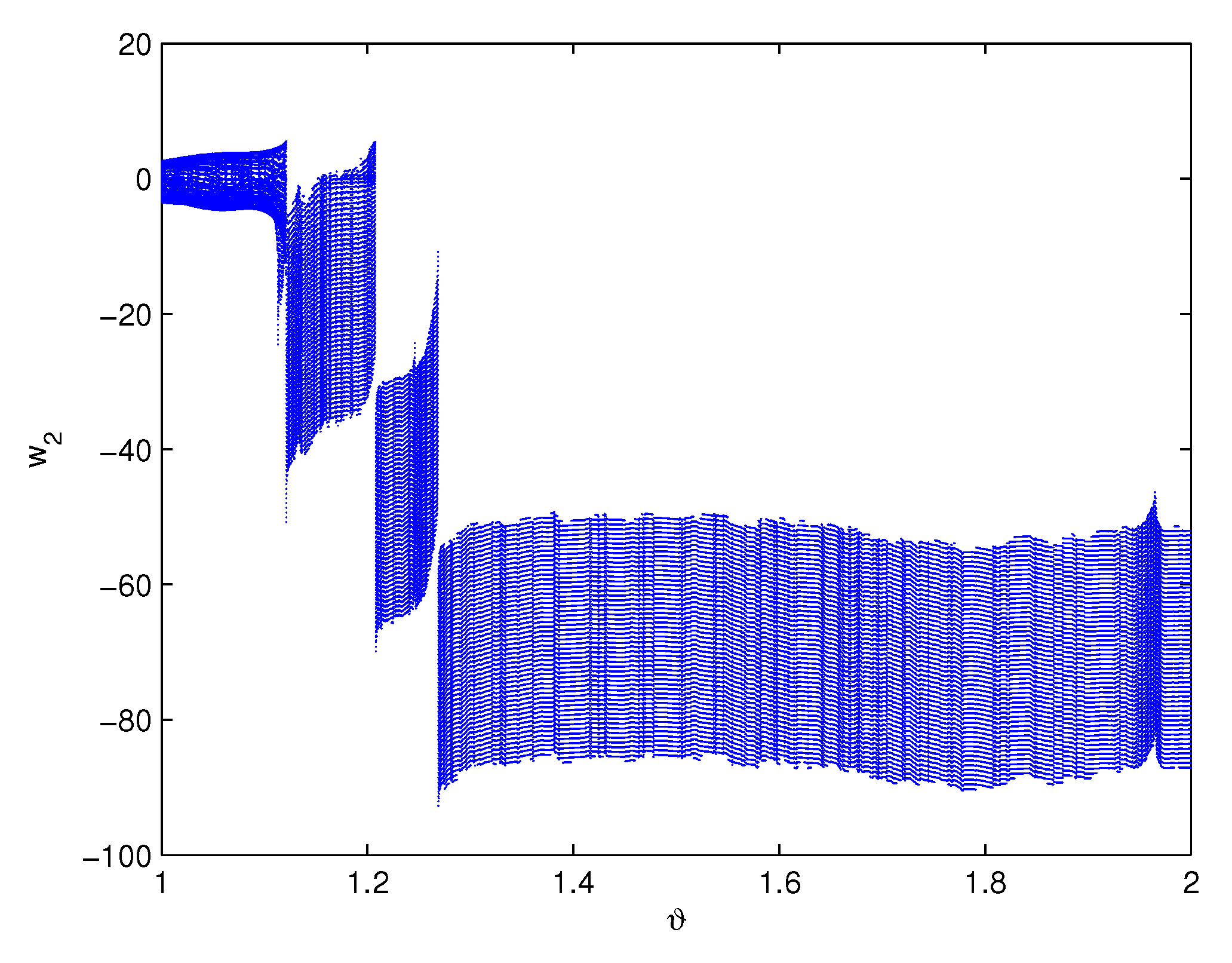
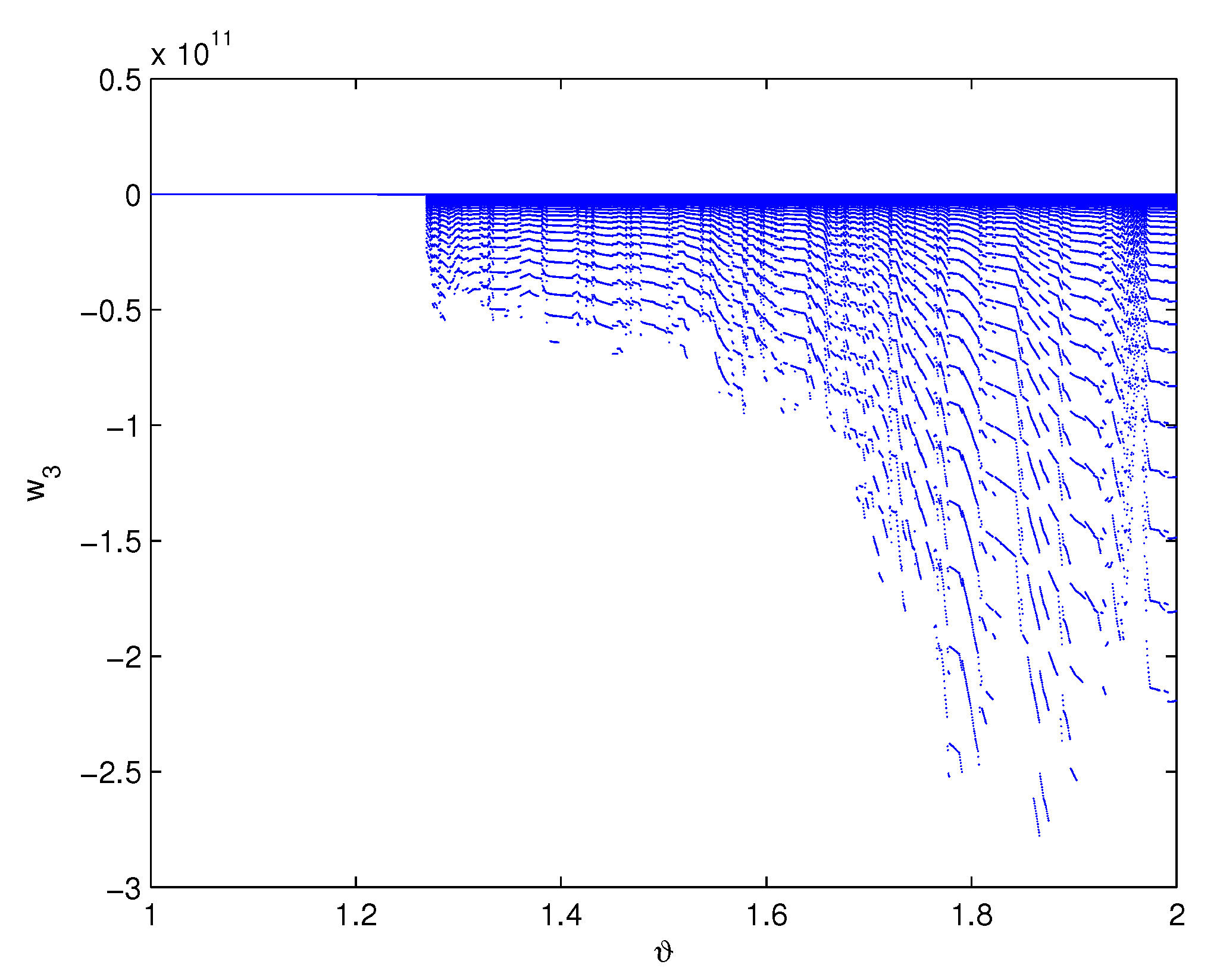
5. Examples
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhou, L.Q.; Chen, F.Q. Chaos of the RayleighCDuffing oscillator with a non-smooth periodic perturbation and harmonic excitation. Math. Comput. Simul. 2022, 192, 1–18. [Google Scholar] [CrossRef]
- Akhtar, S.; Ahmed, R.; Batool, M.; Shah, N.A.; Chung, J.D. Stability, bifurcation and chaos control of a discretized Leslie prey-predator model. Chaos Solitons Fractals 2021, 152, 111345. [Google Scholar] [CrossRef]
- Pietrych, L.; Sandubete, J.E.; Escot, L. Solving the chaos model-data paradox in the cryptocurrency market. Commun. Nonlinear Sci. Numer. Simul. 2021, 102, 105901. [Google Scholar] [CrossRef]
- Wojtusiak, A.M.; Balanov, A.G.; Savelev, S.E. Intermittent and metastable chaos in a memristive artificial neuron with inertia. Chaos Solitons Fractals 2021, 142, 110383. [Google Scholar] [CrossRef]
- Ma, C.; Wang, X.Y. Hopf bifurcation and topological horseshoe of a novel finance chaotic system. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 721–730. [Google Scholar] [CrossRef]
- Boccaletti, S.; Grebogi, C.; Lai, Y.C.; Mancini, H.; Maza, D. The control of chaos: Theory and application. Phys. Rep. 2000, 329, 103–197. [Google Scholar] [CrossRef] [Green Version]
- Corron, N.J.; Pethel, S.D.; Hopper, B.A. Controlling chaos with simple limiters. Phys. Rev. Lett. 2000, 84, 3835–3838. [Google Scholar] [CrossRef] [PubMed]
- Zheng, J.L. A simple universal adaptive feedback controller for chaos and hyperchaos control. Comput. Math. Appl. 2011, 61, 2000–2004. [Google Scholar] [CrossRef] [Green Version]
- Ott, E.; Grebogi, C.; Yorke, J.A. Controlling chaos. Phys. Rev. Lett. 1990, 64, 1196–1199. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.B.; Lu, W.; Gao, L.F.; Zhang, J.S. Nonlinear state feedback control of chaos system of brushless DC motor. Procedia Comput. Sci. 2021, 183, 636–640. [Google Scholar] [CrossRef]
- Du, J.G.; Huang, T.W.; Sheng, Z.H.; Zhang, H.B. A new method to control chaos in an economic system. Appl. Math. Comput. 2020, 217, 2370–2380. [Google Scholar] [CrossRef]
- Zhao, M.C.; Wang, J.W. H∞ control of a chaotic finance system in the presence of external disturbance and input time-delay. Appl. Math. Comput. 2014, 233, 320–327. [Google Scholar] [CrossRef]
- Higazy, M.; Hamed, Y.S. Dynamics, circuit implementation and control of new caputo fractional order chaotic 5-dimensions hyperjerk model. Alex. Eng. J. 2021, 60, 4177–4190. [Google Scholar] [CrossRef]
- Mahmoud, E.E.; Trikha, P.; Jahanzaib, L.S.; Eshmawi, A.A.; Matoog, R.T. Chaos control and Penta-compound combination anti-synchronization on a novel fractional chaotic system with analysis and application. Results Phys. 2021, 24, 104130. [Google Scholar] [CrossRef]
- Holyst, J.A.; Urbanowicz, K. Chaos control in economical model by time-delayed feedback method. Phys. A 2000, 287, 587–598. [Google Scholar] [CrossRef]
- Liu, M.; Sang, B.; Wang, N.; Ahmad, I. Choatic dynamics by some quadratic Jerk system. Axioms 2021, 10, 227. [Google Scholar] [CrossRef]
- Nie, X.B.; Liu, P.P.; Liang, J.L.; Cao, J.D. Exact coexistence and locally asymptotic stability of multiple equilibria for fractional-order delayed Hopfield neural networks with Gaussian activation function. Neural Netw. 2021, 142, 690–700. [Google Scholar] [CrossRef]
- Ke, L. Mittag-Leffler stability and asymptotic ω-periodicity of fractional-order inertial neural networks with time-delays. Neurocomputing 2021, 465, 53–62. [Google Scholar] [CrossRef]
- Zhang, F.H.; Huang, T.W.; Wu, Q.J.; Zeng, Z.G. Multistability of delayed fractional-order competitive neural networks. Neural Netw. 2021, 140, 325–335. [Google Scholar] [CrossRef]
- Rihan, F.A.; Rajivganthi, C. Dynamics of fractional-order delay differential model of prey-predator system with Holling-type III and infection among predators. Chaos Solitons Fractals 2020, 141, 110365. [Google Scholar] [CrossRef]
- Alidousti, J.; Ghafari, E. Dynamic behavior of a fractional order prey-predator model with group defense. Chaos Solitons Fractals 2020, 134, 109688. [Google Scholar] [CrossRef]
- Huang, C.D.; Liu, H.; Chen, P.; Zhang, M.S.; Ding, L.; Cao, J.D.; Alsaedi, A. Dynamic optimal control of enhancing feedback treatment for a delayed fractional order predator-prey model. Phys. A Stat. Mech. Its Appl. 2020, 554, 124136. [Google Scholar] [CrossRef]
- Wang, W.T.; Khan, M.A. Analysis and numerical simulation of fractional model of bank data with fractal-fractional Atangana-Baleanu derivative. J. Comput. Appl. Math. 2020, 369, 112646. [Google Scholar] [CrossRef]
- Xu, C.J.; Liao, M.X.; Li, P.L.; Guo, Y.; Liu, Z.X. Bifurcation properties for fractional order delayed BAM neural networks. Cogn. Comput. 2021, 13, 322–356. [Google Scholar] [CrossRef]
- Xu, C.J.; Liu, Z.X.; Liao, M.X.; Li, P.L.; Xiao, Q.M.; Yuan, S. Fractional-order bidirectional associate memory (BAM) neural networks with multiple delays: The case of Hopf bifurcation. Math. Comput. Simul. 2021, 182, 471–494. [Google Scholar] [CrossRef]
- Djilali, S.; Ghanbari, B.; Bentout, S.; Mezouaghi, A. Turing-Hopf bifurcation in a diffusive mussel-algae model with time-fractional-order derivative. Chaos Solitons Fractals 2020, 138, 109954. [Google Scholar] [CrossRef]
- Xiao, M.; Zheng, W.X.; Lin, J.X.; Jiang, G.P.; Zhao, L.D.; Cao, J.D. Fractional-order PD control at Hopf bifurcations in delayed fractional-order small-world networks. J. Frankl. Inst. 2017, 354, 7643–7667. [Google Scholar] [CrossRef]
- Xu, C.J.; Liao, M.X.; Li, P.L.; Yuan, S. Impact of leakage delay on bifurcation in fractional-order complex-valued neural networks. Chaos Solitons Fractals 2021, 142, 110535. [Google Scholar] [CrossRef]
- Huang, C.D.; Liu, H.; Shi, X.Y.; Chen, X.P.; Xiao, M.; Wang, Z.X.; Cao, J.D. Bifurcations in a fractional-order neural network with multiple leakage delays. Neural Netw. 2020, 131, 115–126. [Google Scholar] [CrossRef] [PubMed]
- Xu, C.; Liao, M.X.; Li, P.L.; Yuan, S. New insights on bifurcation in a fractional-order delayed competition and cooperation model of two enterprises. J. Appl. Anal. Comput. 2021, 11, 1240–1258. [Google Scholar] [CrossRef]
- Xu, C.J.; Aouiti, C. Comparative analysis on Hopf bifurcation of integer order and fractional order two-neuron neural networks with delay. Int. J. Circuit Theory Appl. 2020, 48, 1459–1475. [Google Scholar] [CrossRef]
- Xu, C.J.; Liu, Z.X.; Yao, L.Y.; Aouiti, C. Further exploration on bifurcation of fractional-order six-neuron bi-directional associative memory neural networks with multi-delays. Appl. Math. Comput. 2021, 410, 126458. [Google Scholar] [CrossRef]
- Xu, C.J.; Aouiti, C.; Liu, Z.X. A further study on bifurcation for fractional order BAM neural networks with multiple delays. Neurocomputing 2020, 417, 501–515. [Google Scholar] [CrossRef]
- Xu, C.J.; Liao, M.X.; Li, P.L. Bifurcation control of a fractional-order delayed competition and cooperation model of two enterprises. Sci. China Technol. Sci. 2019, 62, 2130–2143. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations; Academic Press: New York, NY, USA, 1999. [Google Scholar]
- Matignon, D. Stability results for fractional differential equations with applications to control processing. In Proceedings of the Computational Engineering in Systems and Application Multi-Conference, IMACS, Lille, France, 9–12 July 1996; pp. 963–968. [Google Scholar]
- Yu, P.; Chen, G.R. Hopf bifurcation control using nonlinear feedback with polynomial functions. Int. J. Bifurc. Chaos 2004, 14, 1683–1704. [Google Scholar] [CrossRef]
- Ding, D.W.; Zhang, X.Y.; Cao, J.D.; Wang, N.A.; Liang, D. Bifurcation control of complex networks model via PD controller. Neurocomputing 2016, 175, 1–9. [Google Scholar] [CrossRef]
- Tang, Y.H.; Xiao, M.; Jiang, G.P.; Lin, J.X.; Cao, J.D.; Zheng, W.X. Fractional-order PD control at Hopf bifurcations in a fractional-order congestion control system. Nonlinear Dyn. 2017, 90, 2185–2198. [Google Scholar] [CrossRef]
- Sun, Q.S.; Xiao, M.; Tao, B.B. Local bifurcation analysis of a fractional-order dynamic model of genetic regulatory networks with delays. Neural Process. Lett. 2018, 47, 1285–1296. [Google Scholar] [CrossRef]
- Hammouch, Z.; Yavuz, M.; Ozdemir, N. Numerical solutions and synchronization of a variable-order fractional chaotic system. Math. Model. Numer. Simul. Appl. 2021, 1, 11–23. [Google Scholar] [CrossRef]
- Xu, C.J.; Zhang, W.; Aouiti, C.; Liu, Z.X.; Liao, M.X.; Li, P.L. Further investigation on bifurcation and their control of fractional-order BAM neural networks involving four neurons and multiple delays. Math. Methods Appl. Sci. 2021, in press. [Google Scholar] [CrossRef]
- Xu, C.J.; Liao, M.X.; Li, P.L.; Guo, Y.; Xiao, Q.M.; Yuan, S. Influence of multiple time delays on bifurcation of fractional-order neural networks. Appl. Math. Comput. 2019, 361, 565–582. [Google Scholar] [CrossRef]
- Xu, C.J.; Zhang, W.; Liu, Z.X.; Yao, L.Y. Delay-induced periodic oscillation for fractional-order neural networks with mixed delays. Neurocomputing 2021. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, C.; Liao, M.; Li, P.; Yao, L.; Qin, Q.; Shang, Y. Chaos Control for a Fractional-Order Jerk System via Time Delay Feedback Controller and Mixed Controller. Fractal Fract. 2021, 5, 257. https://doi.org/10.3390/fractalfract5040257
Xu C, Liao M, Li P, Yao L, Qin Q, Shang Y. Chaos Control for a Fractional-Order Jerk System via Time Delay Feedback Controller and Mixed Controller. Fractal and Fractional. 2021; 5(4):257. https://doi.org/10.3390/fractalfract5040257
Chicago/Turabian StyleXu, Changjin, Maoxin Liao, Peiluan Li, Lingyun Yao, Qiwen Qin, and Youlin Shang. 2021. "Chaos Control for a Fractional-Order Jerk System via Time Delay Feedback Controller and Mixed Controller" Fractal and Fractional 5, no. 4: 257. https://doi.org/10.3390/fractalfract5040257
APA StyleXu, C., Liao, M., Li, P., Yao, L., Qin, Q., & Shang, Y. (2021). Chaos Control for a Fractional-Order Jerk System via Time Delay Feedback Controller and Mixed Controller. Fractal and Fractional, 5(4), 257. https://doi.org/10.3390/fractalfract5040257




