The Proof of a Conjecture Relating Catalan Numbers to an Averaged Mandelbrot-Möbius Iterated Function
Abstract
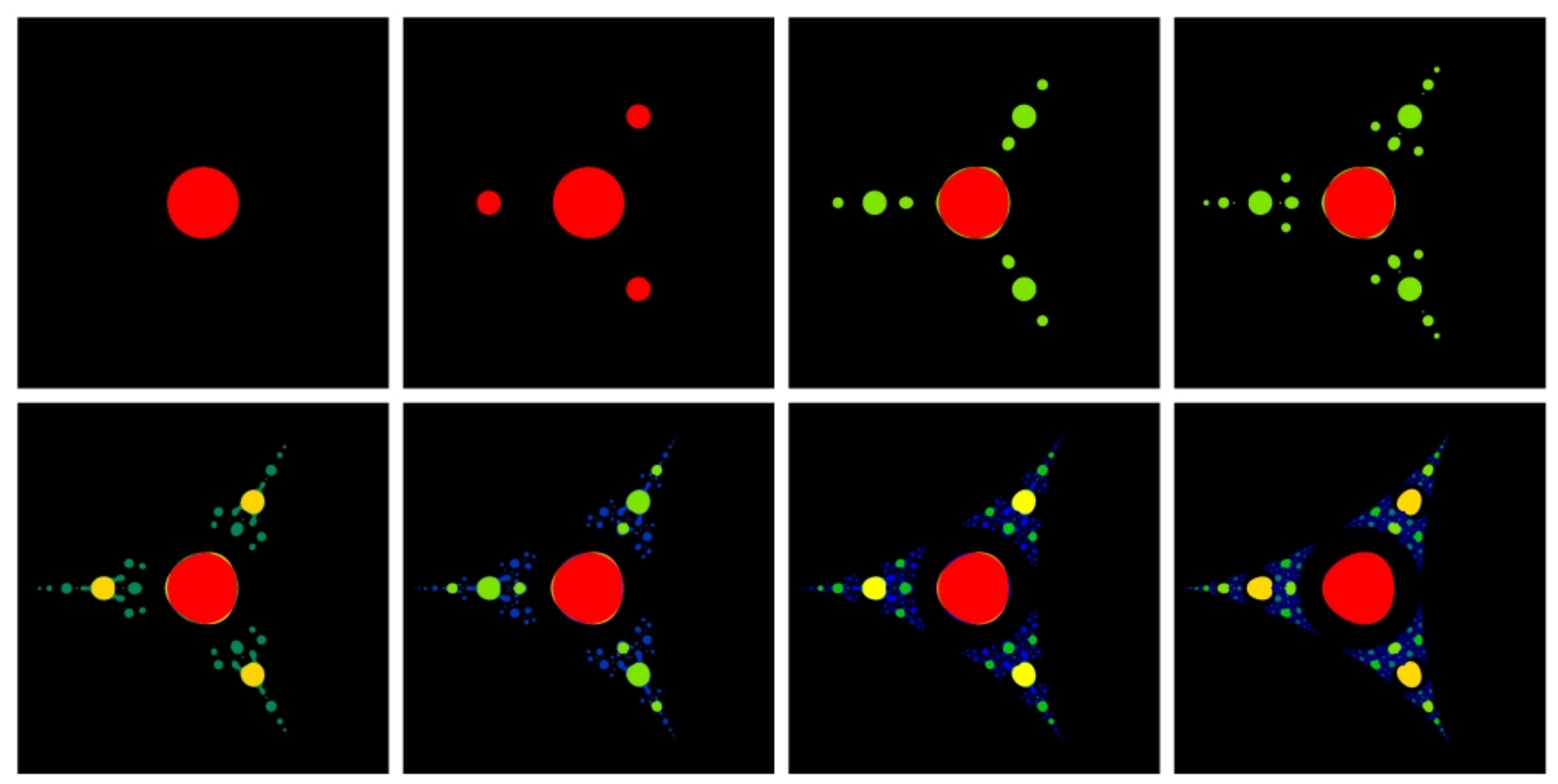
1. Introduction
| n | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 |
| 1 | 1 | 2 | 5 | 14 | 42 | 132 | 429 | 1430 | 4862 | 16,796 | 58,786 | 208,012 | 742,900 | 2,674,440 |
2. Auxiliary Results
- (i)
- If is defined by the recurrence,with , then , for all .
- (ii)
- If is defined by the recurrence,with , then , for all .
3. The Proof of the Theorem 1
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yang, G. Some Geometric Properties of Julia Sets and filled-in Julia Sets of Polynomials. Complex Var. Theory Appl. 2002, 47, 383–391. [Google Scholar] [CrossRef]
- Falconer, K.J. Fractal Geometry: Mathematical Foundations and Applications, 2nd ed.; John Wiley and Sons: Chichester, UK, 2003. [Google Scholar]
- Berry, M.V.; Lewis, Z.V.; Nye, J.F. On the Weierstrass-Mandelbrot fractal function. Proc. R. Soc. Lond. 1980, 370, 459–484. [Google Scholar] [CrossRef]
- Guariglia, E. Primality, Fractality, and Image Analysis. Entropy 2019, 21, 304. [Google Scholar] [CrossRef] [PubMed]
- Berteloot, F.; Dinh, T.C. The Mandelbrot set is the shadow of a Julia set. Discret. Contin. Dyn. Syst. 2020, 40, 6611–6633. [Google Scholar] [CrossRef]
- Sun, T.; Wang, D. The Symmetry in the Noise-Perturbed Mandelbrot Set. Symmetry 2019, 11, 577. [Google Scholar] [CrossRef]
- Blankers, V.; Rendfrey, T.; Shukert, A.; Shipman, P.D. Julia and Mandelbrot Sets for Dynamics over the Hyperbolic Numbers. Fractal Fract. 2019, 3, 6. [Google Scholar] [CrossRef]
- Zhou, H.; Tanveer, M.; Li, J. Comparative study of some fixed-point methods in the generation of Julia and Mandelbrot sets. J. Math. 2020, 2020, 7020921. [Google Scholar] [CrossRef]
- Abbas, M.; Iqbal, H.; De la Sen, M. Generation of Julia and Mandelbrot Sets via Fixed Points. Symmetry 2020, 12, 86. [Google Scholar] [CrossRef]
- Tanveer, M.; Ahmed, I.; Raza, A.; Nawaz, S.; Lv, Y.P. New escape conditions with general complex polynomial for fractals via new fixed point iteration. AIMS Math. 2021, 6, 5563–5580. [Google Scholar] [CrossRef]
- Mork, L.K.; Vogt, T.; Sullivan, K.; Rutherford, D.; Ulness, D.J. Exploration of Filled-In Julia Sets Arising from Centered Polygonal Lacunary Functions. Fractal Fract. 2019, 3, 42. [Google Scholar] [CrossRef]
- Kahane, J.-P. Lacunary Taylor and Fourier series. Bull. Am. Math. Soc. 1964, 70, 199–213. [Google Scholar] [CrossRef][Green Version]
- Gaposhkin, V.F. Lacunary series and independent functions. Uspekhi Matematicheskikh Nauk 1966, 21, 3–82. [Google Scholar] [CrossRef]
- Hille, E. Analytic Function Theory; Ginn and Company: Boston, MA, USA, 1959; Volume I. [Google Scholar]
- Mork, L.K.; Sullivan, K.; Ulness, D.J. Lacunary Möbius Fractals on the Unit Disk. Symmetry 2021, 13, 91. [Google Scholar] [CrossRef]
- Mork, L.K.; Ulness, D.J. Visualization of Mandelbrot and Julia Sets of Möbius Transformations. Fractal Fract. 2021, 5, 73. [Google Scholar] [CrossRef]
- Wolfram, S. The Mathematica Book, 4th ed.; Wolfram Media/Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Sloane, N.J.A. The On-Line Encyclopedia of Integer Sequences. Available online: https://oeis.org/ (accessed on 31 July 2021).
- Koshy, T. Catalan Numbers with Applications; Oxford University Press: Oxford, UK, 2009. [Google Scholar]
- Dutton, R.D.; Brigham, R.C. Computationally Efficient Bounds for the Catalan Numbers. Eur. J. Comb. 1986, 7, 211–213. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Trojovský, P.; Venkatachalam, K. The Proof of a Conjecture Relating Catalan Numbers to an Averaged Mandelbrot-Möbius Iterated Function. Fractal Fract. 2021, 5, 92. https://doi.org/10.3390/fractalfract5030092
Trojovský P, Venkatachalam K. The Proof of a Conjecture Relating Catalan Numbers to an Averaged Mandelbrot-Möbius Iterated Function. Fractal and Fractional. 2021; 5(3):92. https://doi.org/10.3390/fractalfract5030092
Chicago/Turabian StyleTrojovský, Pavel, and K Venkatachalam. 2021. "The Proof of a Conjecture Relating Catalan Numbers to an Averaged Mandelbrot-Möbius Iterated Function" Fractal and Fractional 5, no. 3: 92. https://doi.org/10.3390/fractalfract5030092
APA StyleTrojovský, P., & Venkatachalam, K. (2021). The Proof of a Conjecture Relating Catalan Numbers to an Averaged Mandelbrot-Möbius Iterated Function. Fractal and Fractional, 5(3), 92. https://doi.org/10.3390/fractalfract5030092