Design of Cascaded and Shifted Fractional-Order Lead Compensators for Plants with Monotonically Increasing Lags
Abstract
:1. Introduction
- (A)
- The CS-FLECs are multistage compensators, in which each stage is in its turn a fractional-order lead compensator (FLEC). Each FLEC stage is frequency-scaled with respect to the previous one, so that it provides phase lead values with a nearly flat phase on an adjacent interval. When compared with a cascade of identical FLECs that are superposed on the same frequency interval, a CS-FLEC exhibits a nearly flat phase in a much larger frequency interval. This is a direct result of the serial structure of the shifted stages.
- (B)
- To implement a CS-FLEC, each stage needs a rational TF approximation. As the paper shows, a second-order TF provides a satisfactory approximation in the frequency interval of each stage of the cascade. The values of the coefficients in each second-order TF ensure low sensitivity in both analogue and digital realizations.
- (C)
- The paper shows how to apply CS-FLEC structures to compensate a class of plants that are notoriously difficult to control [49,50]. The approach is especially suited for plants that are characterized by monotonically increasing lags. The fundamental ideas underlying the design pattern are simple and refer to formulas based on the properties of the CS-FLEC. The paper also brings out the limits of the CS-FLEC in controlling the chosen benchmarks.
2. Cascaded, Shifted, Fractional-Order Lead Compensator
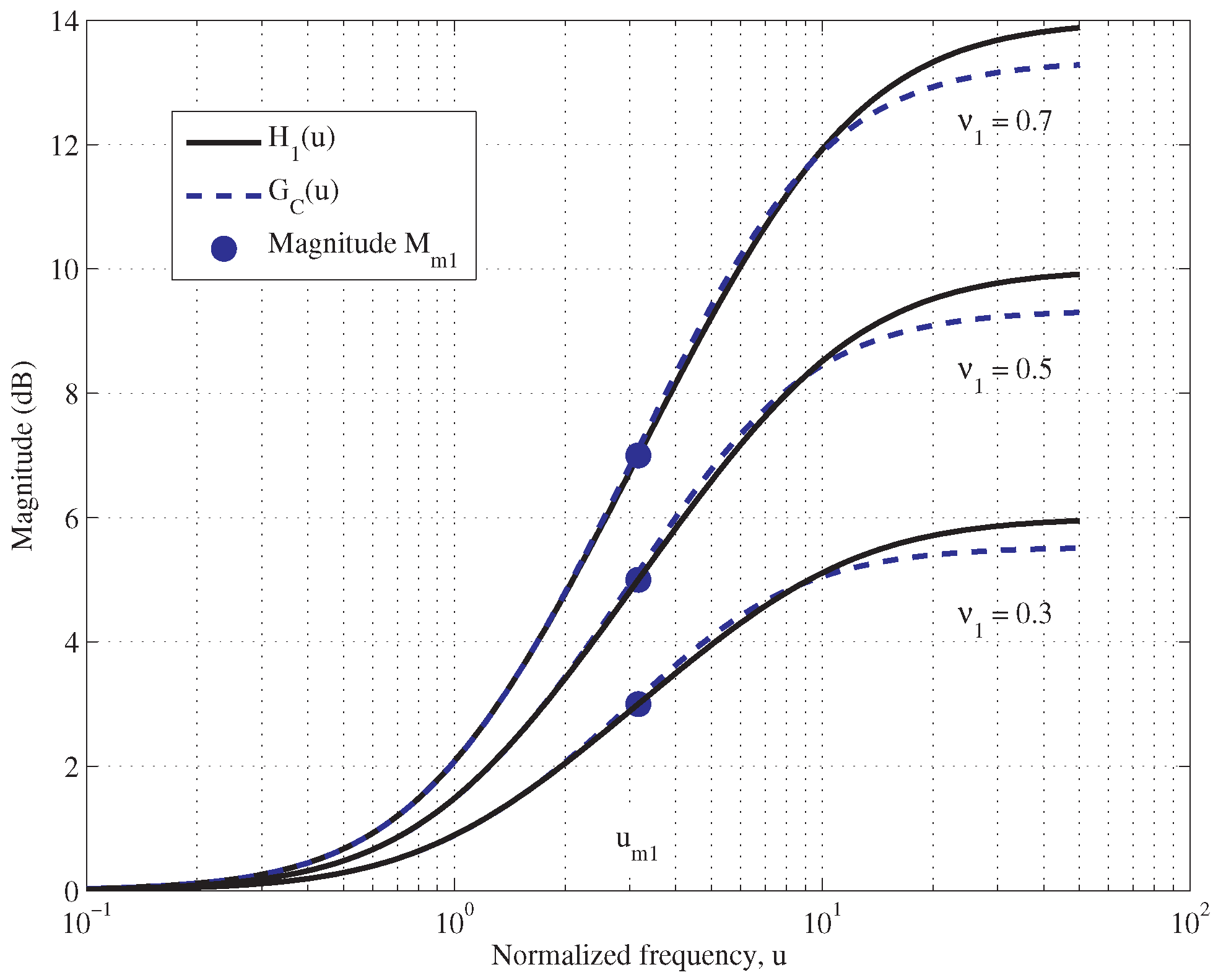
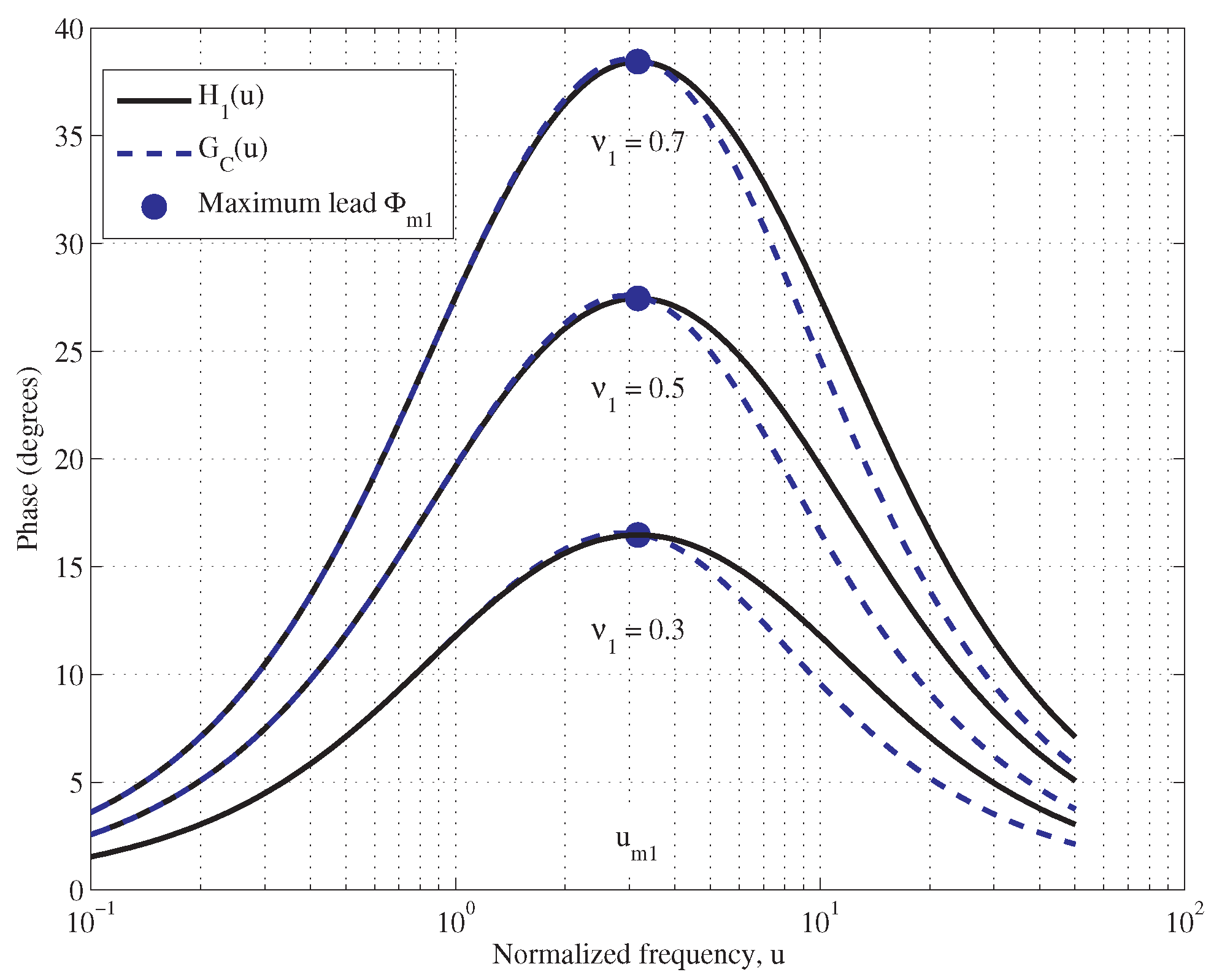
2.1. The First Compensator Stage
2.2. The Second Compensator Stage
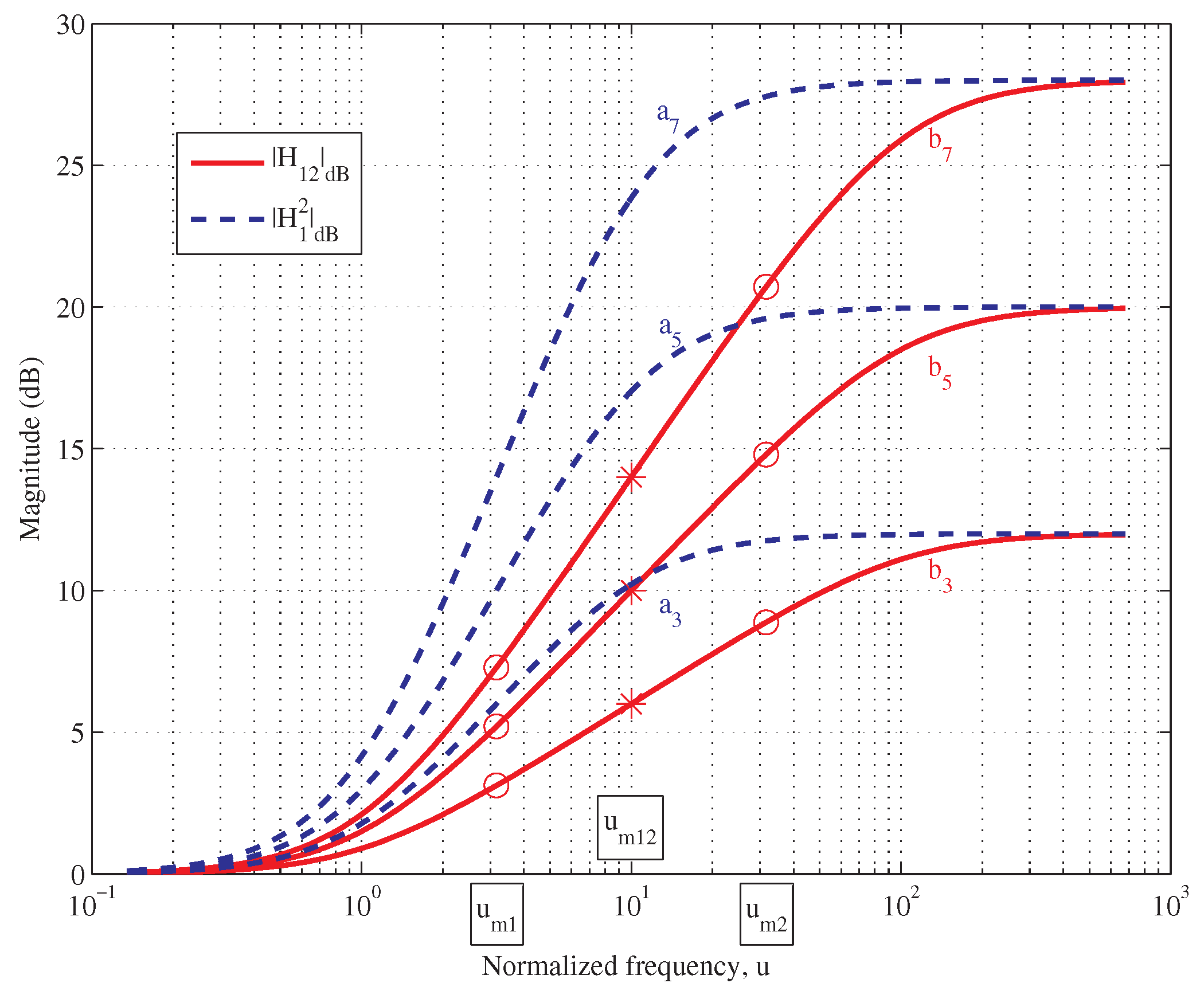
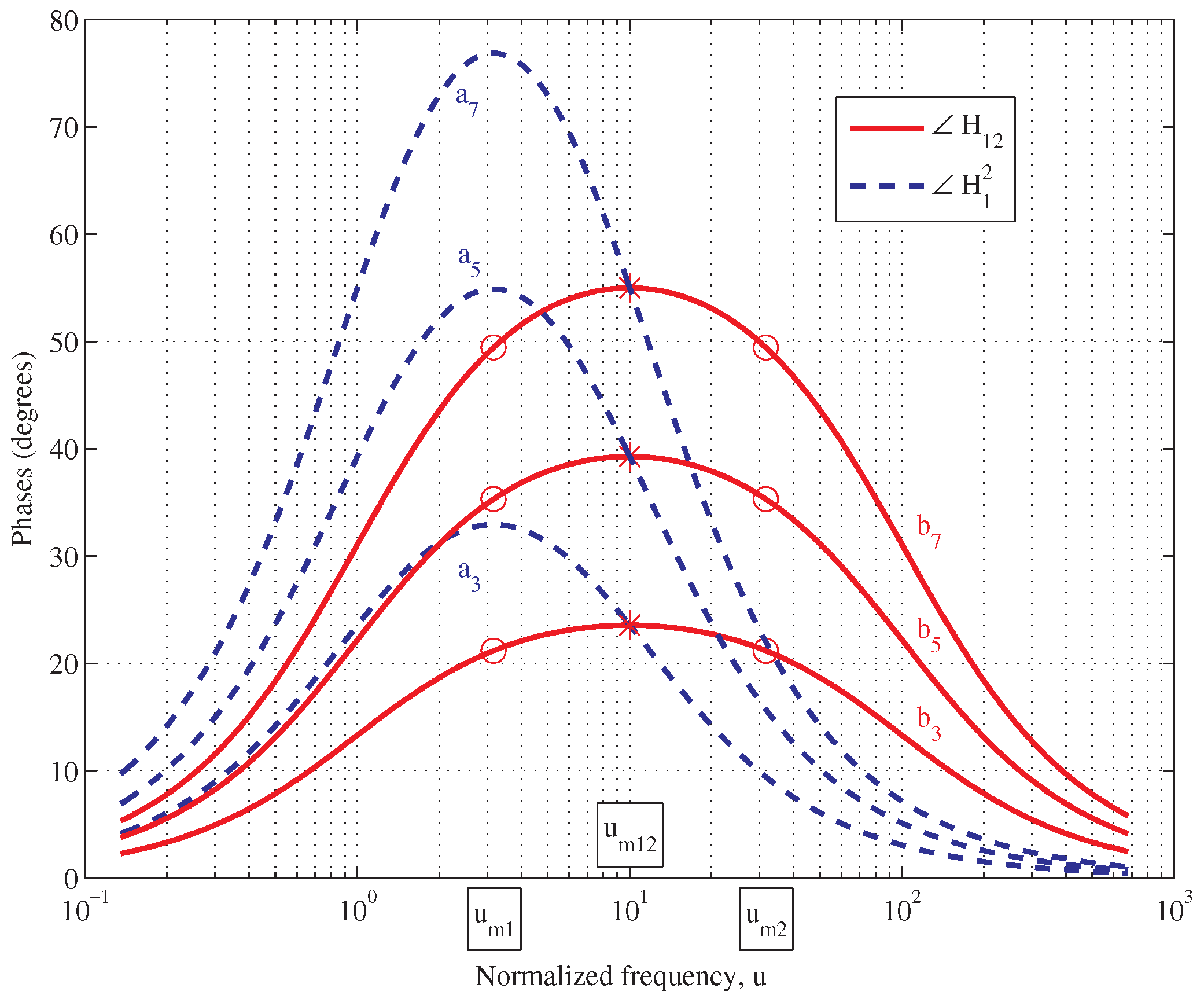
2.3. The Serial Compensator
3. Realization
4. The Design Method
- a.
- The features of the CS-FLEC are suitable for controlling plants with phase lags which are monotonic increasing with the frequency . Afterwards, putting , the phase margin of the plant at its gain crossover frequency , , is often negative.
- b.
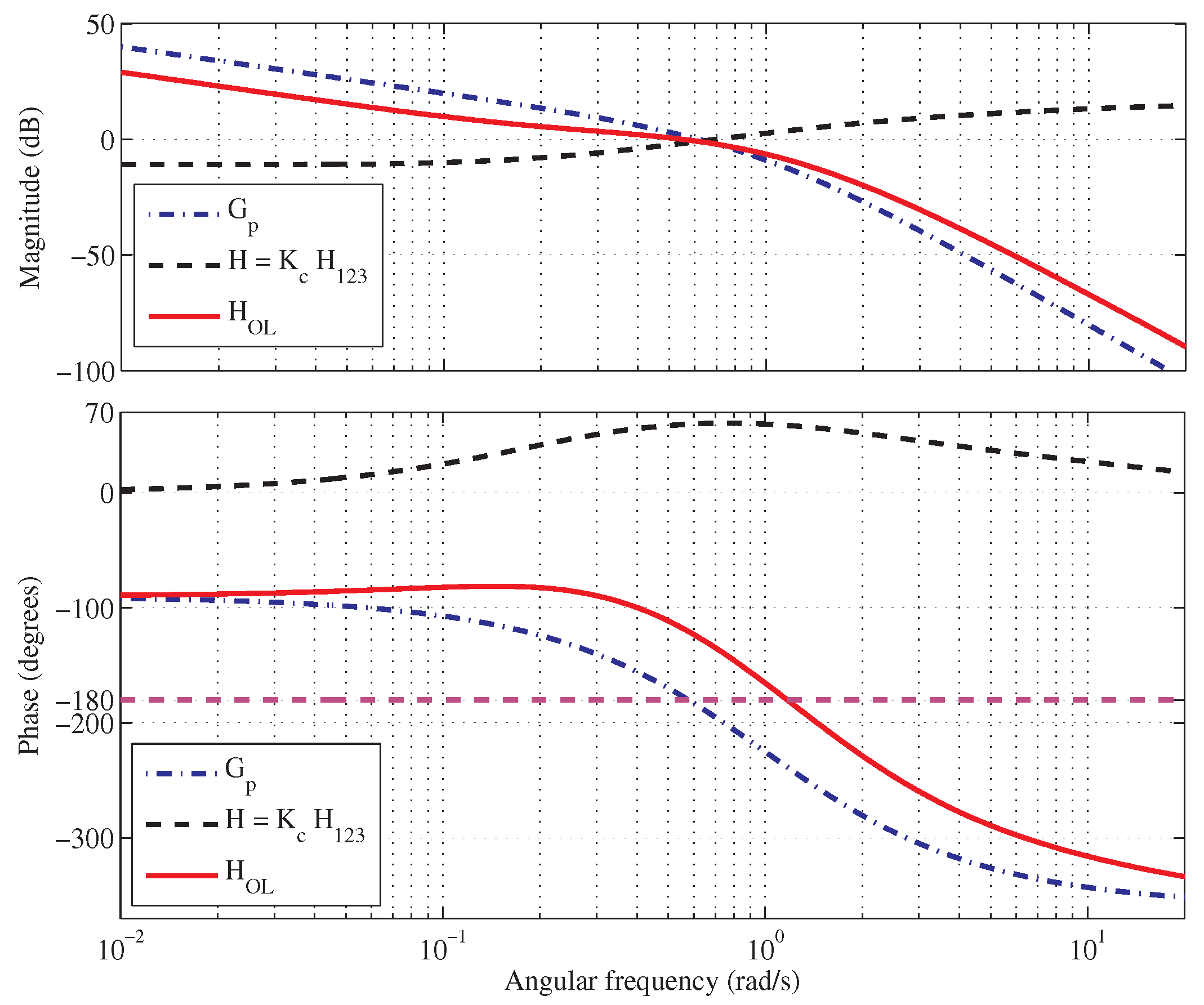
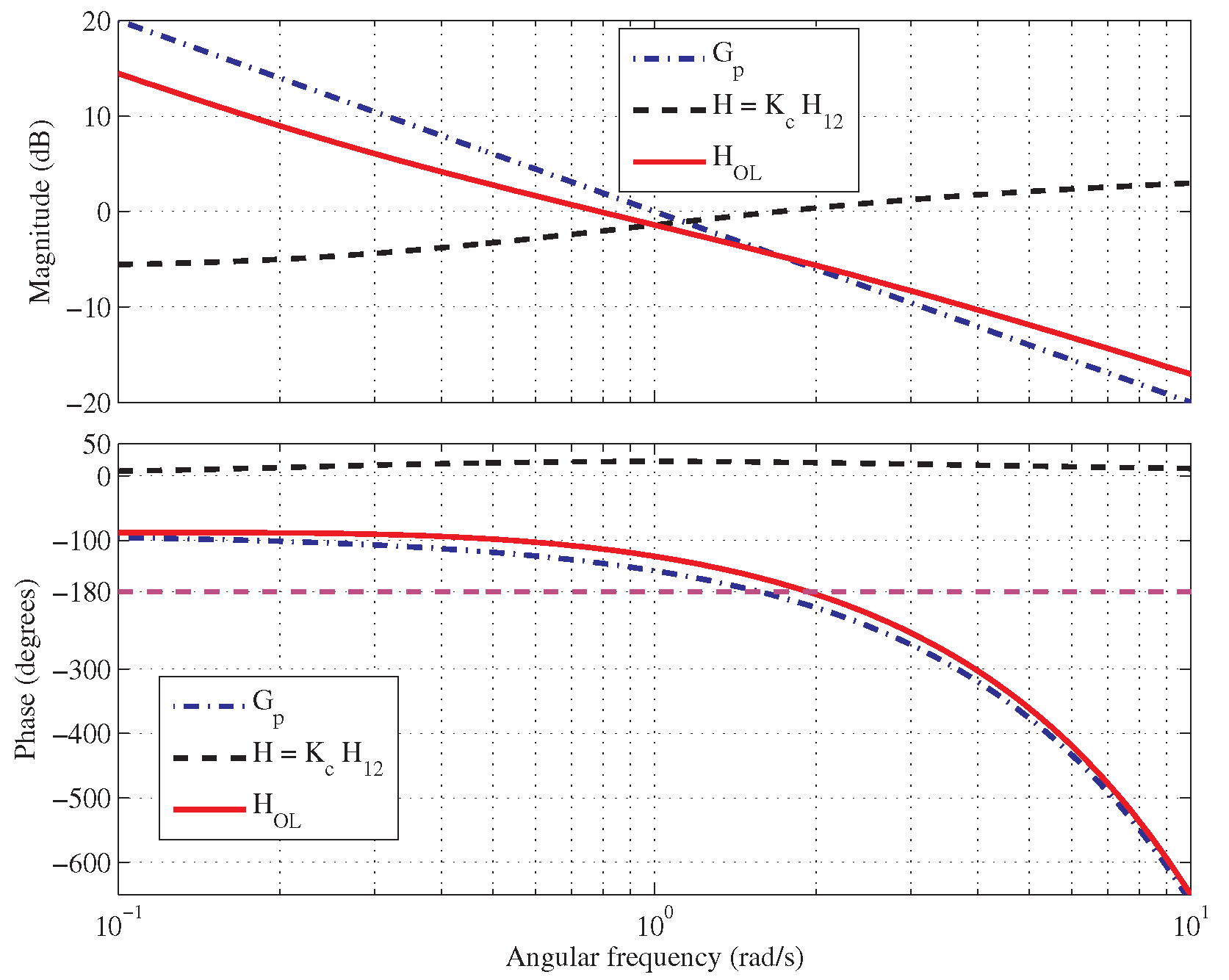
- The compensator will introduce a phase lead compensating the lag of the plant so as to obtain the flattest possible phase trend in the range surrounding , i.e., the gain crossover frequency of . However, the CS-FLEC shifts to the right of . Accordingly, if and decrease sharply beyond , cannot introduce leads balancing the greater lags (see the plant phase diagrams in Figure 7 and Figure 8). For this reason, the value of gain must be properly adjusted to make or even if these frequencies are not too far from each other.
- c.
- To satisfy the previous requirements, is chosen by composing and , having phase plots in shifted frequency ranges. More preciselywhere and is the scaling factor. The strategy is that introduces the larger lead amount, while improves the flatness. It holds .
- d.
- The compensated system must show an adequate phase margin (e.g., ) with a phase decay rate beyond that is less than that of the corresponding plant. Finally, from must be , without excessively reducing the system bandwidth.
- e.
- Let and define the slope . Afterwards, to improve the robustness to gain variations in a frequency range around , the slope must decrease as much as possible. Since the value of estimates the robustness to gain variations, some approaches are proposed for evaluating this parameter [43]. In the present case, simple calculations give (see Appendix B)It can be verified that , , and make , where provides the plant slope.
- I.
- By Equation (2), the time constant can be easily determined in terms of , the frequency where occurs. However, due to the lead contribution of , the controller reaches the maximum phase lead at frequency . Hence, to put , is chosen, so that with . The parameter h can be determined by few successive attempts. A first one can be .
- II.
- To set and , the phase margin specification on is considered. Hence, since and , the following approximate relation can be used:where by Equation (4) and is computed by Equation (5). Note that, if the plant shows a phase lag rapidly increasing with the frequency, can be negative. Moreover, it is suitable to choose , otherwise large values for would increase the magnitude of , that shifts the crossover of too far beyond . A rule of thumb, which is tested by numerical experiments, indicates . Moreover, Equation (19) shows that values of allow to compensate in order to obtain and achieve a right compromise between robustness and dynamic response.
- III.
- is set, so that . The gain crossover frequency , indeed, can also assume values that are less than . This choice is convenient if a greater phase margin is required and if decreases. However, must be quite close (to the left) to .
- IV.
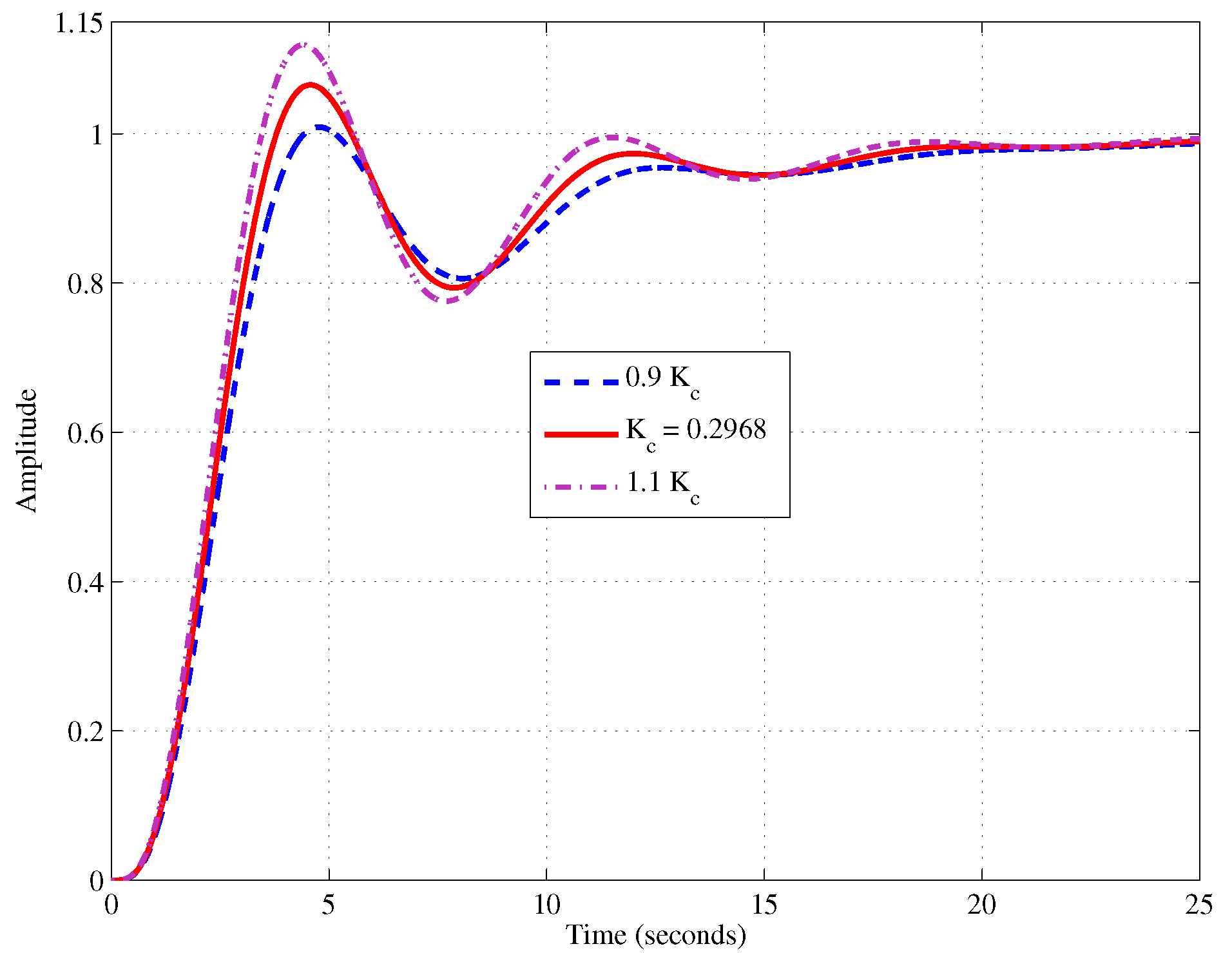
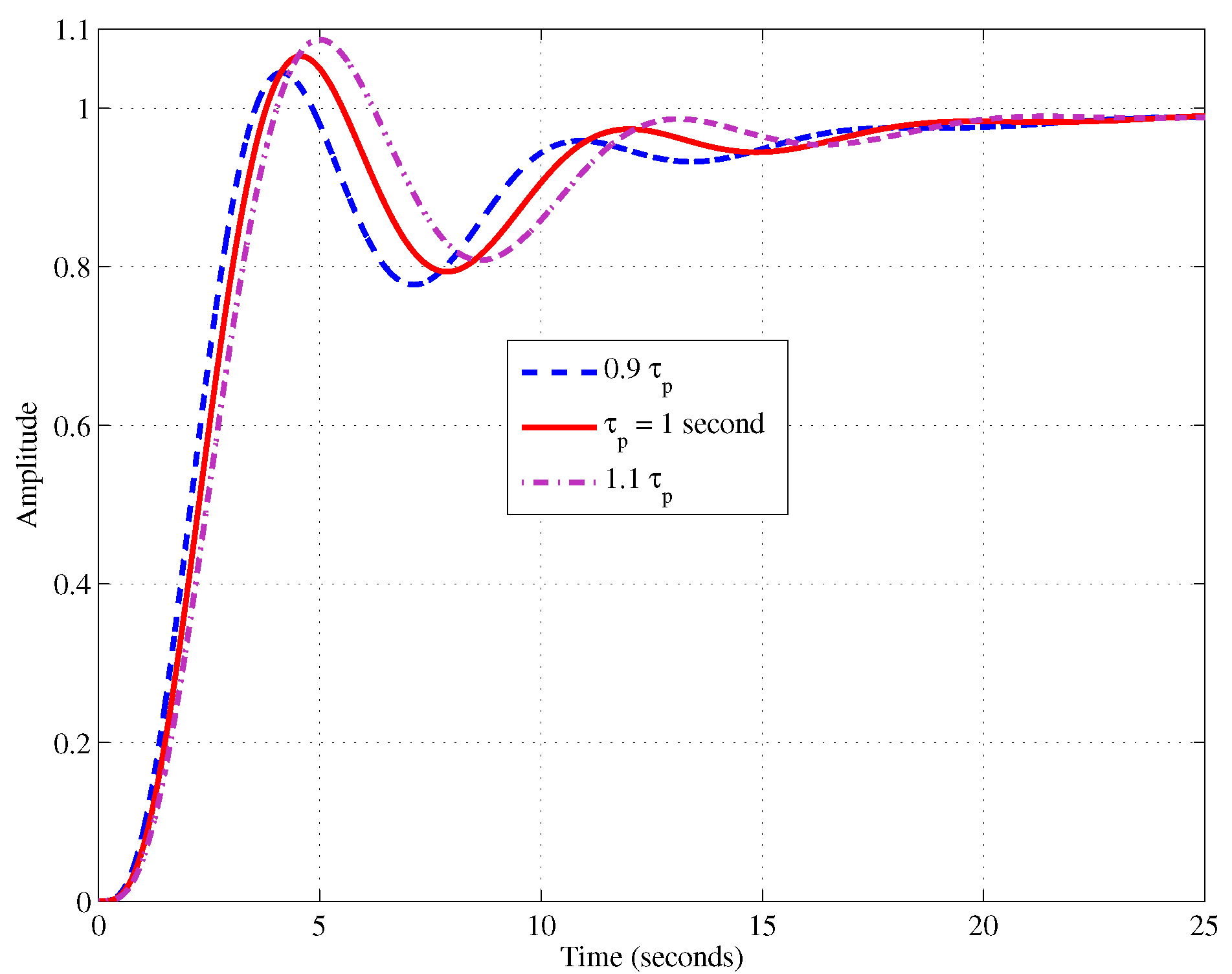
- The performance is verified. If specifications are not met, then the procedure must be repeated by going back to step I. (or III.), decreasing (or ), then changing and so on.
5. Two Illustrative Benchmark Examples
5.1. First Example
- –
- Step I: s
- –
- Step II: and
- –
- Step III:
- –
- Step IV: the obtained phase margin is in rad/s. The slope of the phase plot is . The step response shows a overshoot and settling time of s.
- –
- Step I: s
- –
- Step II: and
- –
- Step III:
- –
- Step IV: the obtained phase margin is in rad/s. Moreover, , which is a good improvement. The step response shows a overshoot and settling time of s.
- –
- Step I: s
- –
- Step II:
- –
- Step III:
- –
- Step IV: the obtained phase margin is in rad/s.
5.2. Second Example
- (a)
- The initial choice in the design procedure, and , is sufficient for small delays .
- (b)
- The positions and are applied to delays .
- (c)
- The design choice and can be used to satisfy the specifications and obtain good performance for delays (see Table 2).
- (d)
- The relations and are used for delays that are more difficult to compensate.
6. Conclusions
Funding
Conflicts of Interest
Abbreviations
| PID | Proportional-Integral-Derivative |
| FOC | Fractional-Order Controller |
| FPID | Fractional-Order PID |
| FPI | Fractional-Order PI |
| PSO | Particle Swarm Optimization |
| ITAE | Integral Time Absolute Error |
| ISE | Integral Square Error |
| IAE | Integral Absolute Error |
| FLEC | Fractional-Order Lead Compensator |
| CS-FLEC | Cascaded Shifted Fractional-Order Lead Compensator |
| TF | Transfer Function |
| PM | Phase Margin |
Appendix A. The Approximation Method
Appendix B. Computation of Slopes in the Bode Phase Diagrams
References
- Oldham, K.B.; Spanier, J. The Fractional Calculus: Integrations and Differentiations of Arbitrary Order; Academic Press: New York, NY, USA, 1974. [Google Scholar]
- Samko, S.; Kilbas, A.; Marichev, O. Fractional Integrals and Derivatives: Theory and Applications; Cambridge University Press: Cambridge, UK, 1993. [Google Scholar]
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations; John Wiley & Sons, Inc.: New York, NY, USA, 1993. [Google Scholar]
- Kiryakova, V.S. Generalized Fractional Calculus and Applications; Longman Scientific & Technical: Harlow, UK, 1994. [Google Scholar]
- Podlubny, I. Fractional Differential Equations—An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Magin, R.L. Fractional Calculus in Bioengineering; Begell House Publishers: Redding, CT, USA, 2006. [Google Scholar]
- Tarasov, V.E. Fractional Dynamics: Applications of Fractional Calculus to Dynamics of Particles, Fields and Media; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Uchaikin, V.E. Fractional Derivatives for Physicists and Engineers: Background and Theory; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Sapuppo, F.; Bucolo, M.; Intaglietta, M.; Fortuna, L.; Arena, P. A cellular nonlinear network: Real-time technology for the analysis of microfluidic phenomena in blood vessels. Nanotechnology 2006, 17, S54–S63. [Google Scholar]
- Cairone, F.; Gagliano, S.; Bucolo, M. Experimental study on the slug flow in a serpentine microchannel. Exp. Therm. Fluid Sci. 2016, 76, 34–44. [Google Scholar]
- Lino, P.; Maione, G.; Saponaro, F. Fractional-order modeling of high-pressure fluid-dynamic flows: An automotive application. In Proceedings of the 8th Vienna International Conference on Mathematical Modelling, Vienna, Austria, 18–20 February 2013; IFAC-PapersOnLine. Volume 8, Part 1. pp. 382–387. [Google Scholar]
- Garrappa, R.; Lino, P.; Maione, G.; Saponaro, F. Model optimization and flow rate prediction in electro-injectors of Diesel injection systems. In Proceedings of the 8th IFAC International Symposium on Advances in Automotive Control (AAC 2016), Norrköping, Sweden, 19–23 June 2016; IFAC-PapersOnLine. Volume 49, pp. 484–489. [Google Scholar]
- Kapetina, M.N.; Lino, P.; Maione, G.; Rapaić, M.R. Estimation of non-integer order models to represent the pressure dynamics in common-rail natural gas engines. In Proceedings of the 20th IFAC World Congress, Toulouse, France, 9–14 July 2017; IFAC-PapersOnLine. Volume 50, pp. 14551–14556. [Google Scholar]
- Åström, K.J.; Hägglund, T. PID Controllers: Theory, Design, and Tuning, 2nd ed.; Instrument Society of America: Research Triangle Park, NC, USA, 1995. [Google Scholar]
- Oustaloup, A. La Commande CRONE. Commande Robuste d’Ordre Non Entiér; Hermés: Paris, France, 1991. [Google Scholar]
- Chen, Y.Q. Ubiquitous fractional order controls? In Proceedings of the Second IFAC Symposium on Fractional Derivatives and Its Applications (IFAC FDA06), Porto, Portugal, 19–21 July 2006; pp. 481–492. [Google Scholar]
- Monje, C.A.; Vinagre, B.M.; Feliu, V.; Chen, Y.Q. Tuning and auto-tuning of fractional order controllers for industry applications. Control Eng. Pract. 2008, 16, 798–812. [Google Scholar]
- Monje, C.A.; Chen, Y.Q.; Vinagre, B.M.; Xue, D.; Feliu, V. Fractional Order Systems and Controls. Fundamentals and Applications; Springer: London, UK, 2010. [Google Scholar]
- Efe, M.O. Fractional order systems in industrial automation. A survey. IEEE Trans. Ind. Inform. 2011, 7, 582–591. [Google Scholar]
- Caponetto, R.; Dongola, G.; Pappalardo, F.; Tomasello, V. Auto-tuning and fractional order controller implementation on hardware in the loop system. J. Optim. Theory Appl. 2013, 156, 141–152. [Google Scholar]
- Caponetto, R.; Maione, G.; Pisano, A.; Rapaić, M.R.; Usai, E. Analysis and shaping of the self-sustained oscillations in relay controlled fractional-order systems. Fract. Calc. Appl. Anal. 2013, 16, 93–108. [Google Scholar]
- Caponetto, R.; Dongola, G. A numerical approach for computing stability region of FO-PID controller. J. Frankl. Inst. 2013, 350, 871–889. [Google Scholar]
- Xue, D.; Chen, Y.Q. A comparative introduction of four fractional order controllers. In Proceedings of the 4th World Congress on Intelligent Control and Automation, Shanghai, China, 10–14 June 2002; pp. 3228–3235. [Google Scholar]
- Lurie, B.J. Three-Parameter Tunable Tilt-Integral-Derivative (TID) Controller. U.S. Patent 5371670, 6 December 1994. [Google Scholar]
- Oustaloup, A.; Mathieu, B.; Lanusse, P. The CRONE control of resonant plants: Application to a flexible transmission. Eur. J. Control 1995, 1, 113–121. [Google Scholar]
- Oustaloup, A.; Moreau, X.; Nouillant, M. The CRONE suspension. Control Eng. Pract. 1996, 4, 1101–1108. [Google Scholar]
- Podlubny, I. Fractional-order systems and PIλDμ-controllers. IEEE Trans. Autom. Control 1999, 44, 208–214. [Google Scholar]
- Raynaud, H.F.; Zergainoh, A. State-space representation for fractional order controllers. Automatica 2000, 36, 1017–1021. [Google Scholar]
- Chen, Y.Q.; Bhaskaran, T.; Xue, D. Practical tuning rules development for fractional order proportional and integral controllers. ASME J. Comput. Nonlinear Dyn. 2008, 3, 1–8. [Google Scholar]
- Barbosa, R.S.; Tenreiro Machado, J.A.; Ferreira, I. Tuning PID controllers based on Bode’s ideal transfer function. Nonlinear Dyn. 2004, 38, 305–321. [Google Scholar]
- Maiti, D.; Acharya, A.; Chakraborty, M.; Konar, A.; Janarthanan, R. Tuning PID and PIλDδ controllers using the integral time absolute error criterion. In Proceedings of the 4th International Conference on Information and Automation for Sustainability, Colombo, Sri Lanka, 12–14 December 2008; pp. 457–462. [Google Scholar]
- Chang, F.K.; Lee, C.H. Design of fractional PID control via hybrid of electromagnetism-like and genetic algorithms. In Proceedings of the Eighth International Conference on Intelligent Systems Design and Applications, Kaohsiung, Taiwan, 26–28 November 2008; pp. 525–530. [Google Scholar]
- Cao, J.Y.; Liang, J.; Cao, B.G. Optimization of fractional order PID based on genetic algorithms. In Proceedings of the Fourth International Conference on Machine Learning and Cybernetics, Guangzhou, China, 18–21 August 2005; pp. 5686–5689. [Google Scholar]
- Boškovic, M.; Rapaić, M.; Jeličić, Z. Particle swarm optimization of PID controller under constraints on performance and robustness. Int. J. Electr. Eng. Comput. 2018, 2, 1–10. [Google Scholar]
- Jakovljević, B.B.; Rapaić, M.R.; Jeličić, Z.D.; Šekara, T.B. Optimization of fractional PID controller by maximization of the criterion that combines the integral gain and closed-loop system bandwidth. In Proceedings of the 2014 18th International Conference on System Theory, Control and Computing (ICSTCC), Sinaia, Romania, 17–19 October 2014; pp. 64–69. [Google Scholar]
- Maione, G.; Punzi, A. Combining differential evolution and particle swarm optimization to tune and realize fractional order controllers. Math. Comput. Model. Dyn. Syst. 2013, 19, 277–299. [Google Scholar]
- Barbosa, R.S.; Tenreiro Machado, J.A.; Jesus, I.S. On the fractional PID control of a laboratory servo system. In Proceedings of the 17th IFAC World Congress, Seoul, Korea, 6–11 July 2008; pp. 15273–15278. [Google Scholar]
- Valerio, D.; Sá da Costa, J. Tuning of fractional PID controllers with Ziegler—Nichols-type rules. Signal Process. 2010, 86, 2771–2784. [Google Scholar]
- Maione, G.; Lino, P. New tuning rules for fractional PIα controllers. Nonlinear Dyn. 2007, 49, 251–257. [Google Scholar]
- Monje, C.A.; Calderon, A.J.; Vinagre, B.M.; Chen, Y.Q.; Feliu, V. On fractional PIλ controllers: Some tuning rules for robustness to plant uncertainties. Nonlinear Dyn. 2004, 38, 369–381. [Google Scholar]
- Luo, Y.; Chen, Y.Q.; Wang, C.Y.; Pi, Y.G. Tuning fractional order proportional integral controllers for fractional order systems. J. Process. Control 2010, 20, 823–831. [Google Scholar]
- Chen, Y.Q.; Moore, K.L.; Vinagre, B.M.; Podlubny, I. Robust PID controller autotuning with a phase shaper. In Proceedings of the First IFAC Workshop on Fractional Differentiation and Its Application (ENSEIRB), Bordeaux, France, 19–21 July 2004; pp. 162–167. [Google Scholar]
- Chen, Y.Q.; Moore, K.L. Relay feedback tuning of robust PID controllers with iso-damping property. IEEE Trans. Syst. Man Cybern. Part B Cybern. 2005, 35, 23–31. [Google Scholar]
- Monje, C.A.; Vinagre, B.M.; Chen, Y.Q.; Feliu, V.; Lanusse, P.; Sabatier, J. Proposals for fractional PIλDμ tuning. In Proceedings of the First IFAC Symposium on Fractional Differentiation and Its Application (FDA04), Bordeaux, France, 19–21 July 2004; pp. 115–120. [Google Scholar]
- Das, S.; Saha, S.; Das, S.; Gupta, A. On the selection of tuning methodology of FOPID controllers for the control of higher order processes. ISA Trans. 2011, 50, 376–388. [Google Scholar]
- Tavakoli-Kakhki, M.; Haeri, M. Fractional order model reduction approach based on retention the dominant dynamics: Application in IMC based tuning of FOPI and FOPID controllers. ISA Trans. 2011, 50, 431–441. [Google Scholar]
- Zhang, B.T.; Pi, Y.G.; Luo, Y. Fractional order sliding-mode control based on parameters auto-tuning for velocity control of permanent magnet synchronous motor. ISA Trans. 2012, 51, 649–656. [Google Scholar]
- Tavazoei, M.S.; Tavakoli-Kakhki, M. Compensation by fractional-order phase-lead/lag compensators. IET Control Theory Appl. 2014, 8, 319–329. [Google Scholar]
- Åström, K.J.; Panagopoulos, H.; Hägglund, T. Design of PI controllers based on non-convex optimization. Automatica 1998, 34, 585–601. [Google Scholar]
- Panagopoulos, H.; Åström, K.J.; Hägglund, T. Design of PID controllers based on constrained optimization. IEE Proc. Control Theory Appl. 2002, 149, 32–40. [Google Scholar]
- Monje, C.A.; Calderon, A.J.; Vinagre, B.M.; Feliu, V. The fractional order lead compensator. In Proceedings of the 2nd International Conference on Computational Cybernetics (ICCC 2004), Vienna, Austria, 30 August–1 September 2004; pp. 347–352. [Google Scholar]
- Seborg, D.E.; Edgar, T.F.; Mellichamp, D.A. Process Dynamics and Control, 2nd ed.; John Wiley & Sons, Inc.: New York, NY, USA, 2004. [Google Scholar]
- Carlson, G.E.; Halijak, C.A. Approximation of fractional capacitors (1/s)1/n by a regular Newton process. IEEE Trans. Circuit Theory 1964, 11, 210–213. [Google Scholar]
- Charef, A.; Sun, H.H.; Tsao, Y.Y.; Onaral, B. Fractal system as represented by singularity function. IEEE Trans. Autom. Control 1992, 37, 1465–1470. [Google Scholar]
- Matsuda, K.; Fujii, H. H∞ optimized wave-absorbing control: Analytical and experimental results. J. Guid. Control Dyn. 1993, 16, 1146–1153. [Google Scholar]
- Oustaloup, A.; Levron, F.; Mathieu, B.; Nanot, F.M. Frequency-band complex noninteger differentiator: Characterization and synthesis. IEEE Trans. Circuits Syst. Fundam. Theory Appl. 2000, 47, 25–39. [Google Scholar]
- Xue, D.; Zhao, C.; Chen, Y.Q. A modified approximation method of fractional order system. In Proceedings of the IEEE International Conference on Mechatronics and Automation, Luoyang, China, 25–28 June 2006; pp. 1043–1048. [Google Scholar]
- Vinagre, B.M.; Podlubny, I.; Hernández, A.; Feliu, V. Some approximations of fractional order operators used in control theory and applications. Fract. Calc. Appl. Anal. 2000, 3, 231–248. [Google Scholar]
- Podlubny, I.; Petráš, I.; Vinagre, B.M.; O’Leary, P.; Dorčák, L. Analogue realizations of fractional-order controllers. Nonlinear Dyn. 2002, 29, 281–296. [Google Scholar]
- Valério, D. Fractional Robust System Control. Ph.D. Thesis, Instituto Superior Técnico, Universidade Técnica de Lisboa, Lisbon, Portugal, 2005. [Google Scholar]
- Muñiz-Montero, C.; Sánchez-Gaspariano, L.A.; Sánchez-López, C.; González-Díaz, V.R.; Tlelo-Cuautle, E. On the electronic realizations of fractional-order phase-lead-lag compensators with OpAmps and FPAAs. In Fractional Order Control and Synchronization of Chaotic Systems, Studies in Computational Intelligence; Azar, A., Vaidyanathan, S., Ouannas, A., Eds.; Springer: Cham, Switzerland, 2017; Volume 688, pp. 131–164. [Google Scholar]
- Jadhav, S.P.; Chile, R.H.; Hamde, S.T. A simple method to design robust fractional-order lead compensator. Int. J. Control Autom. Syst. 2017, 15, 1236–1248. [Google Scholar]
- Yuan, J.; Zhenlong, W.; Shumin, F.; Chen, Y.Q. Hybrid model-based feedforward and fractional-order feedback control design for the benchmark refrigeration system. Ind. Eng. Chem. Res. 2019, 58, 17885–17897. [Google Scholar]
- Ozdemir, A.A.; Gumussoy, S. Transfer function estimation in system identification toolbox via vector fitting. IFAC-PapersOnLine 2017, 50, 6232–6237. [Google Scholar]
- Bingi, K.; Ibrahim, R.; Karsiti, M.N.; Hassam, S.M.; Harindran, V.R. Frequency response based curve fitting approximation of fractional-order PID controllers. Int. J. Appl. Math. Comput. Sci. 2019, 29, 311–326. [Google Scholar]
- Bingi, K.; Ibrahim, R.; Karsiti, M.N.; Hassan, S.M.; Harindran, V.R. Fractional-order Systems and PID Controllers—Using Scilab and Curve Fitting Based Approximation Techniques; Springer: Cham, Switzerland, 2020. [Google Scholar]
- Kapoulea, S.; Tsirimokou, G.; Psychalinos, C.; Elwakil, A.S. Employment of the Padé approximation for implementing fractional-order lead/lag compensators. AEU Int. J. Electron. Commun. 2020, 120, 153203. [Google Scholar]
- Bošković, M.Č.; Rapaić, M.R.; Šekara, T.B.; Mandić, P.D.; Lazarević, M.P.; Cvetković, B.; Lutovac, B.; Daković, M. On the rational representation of fractional order lead compensator using pade approximation. In Proceedings of the 2018 7th Mediterranean Conference on Embedded Computing (MECO), Budva, Montenegro, 10–14 June 2018; pp. 1–4. [Google Scholar]
- Maione, G. High-speed digital realizations of fractional operators in the delta domain. IEEE Trans. Autom. Control 2011, 56, 697–702. [Google Scholar]
- Caponetto, R.; Tomasello, V.; Lino, P.; Maione, G. Design and efficient implementation of digital non-integer order controllers for electro-mechanical systems. J. Vib. Control 2016, 22, 2196–2210. [Google Scholar]
- Maione, G. Continued fractions approximation of the impulse response of fractional order dynamic systems. IET Control Theory Appl. 2008, 2, 564–572. [Google Scholar]
- Middleton, R.H. Dealing with actuator saturation. In The Control Handbook; Levine, W.S., Ed.; CRC Press: Boca Raton, FL, USA, 1996; pp. 377–381. [Google Scholar]
- Bucolo, M.; Buscarino, A.; Fortuna, L.; Frasca, M. Forward action to make time-delay systems positive-real or negative-imaginary. Syst. Control Lett. 2019, 131, 104495. [Google Scholar]


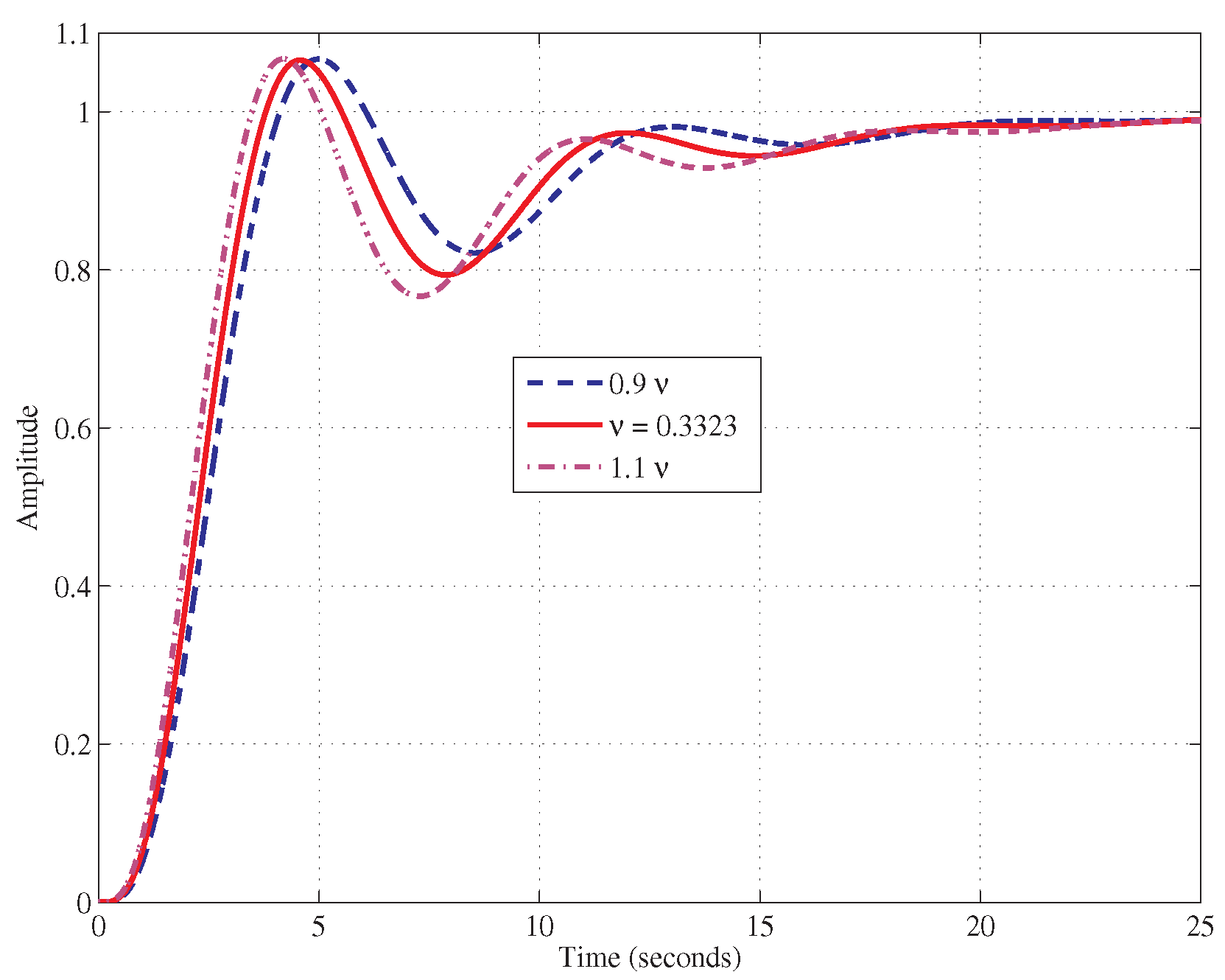


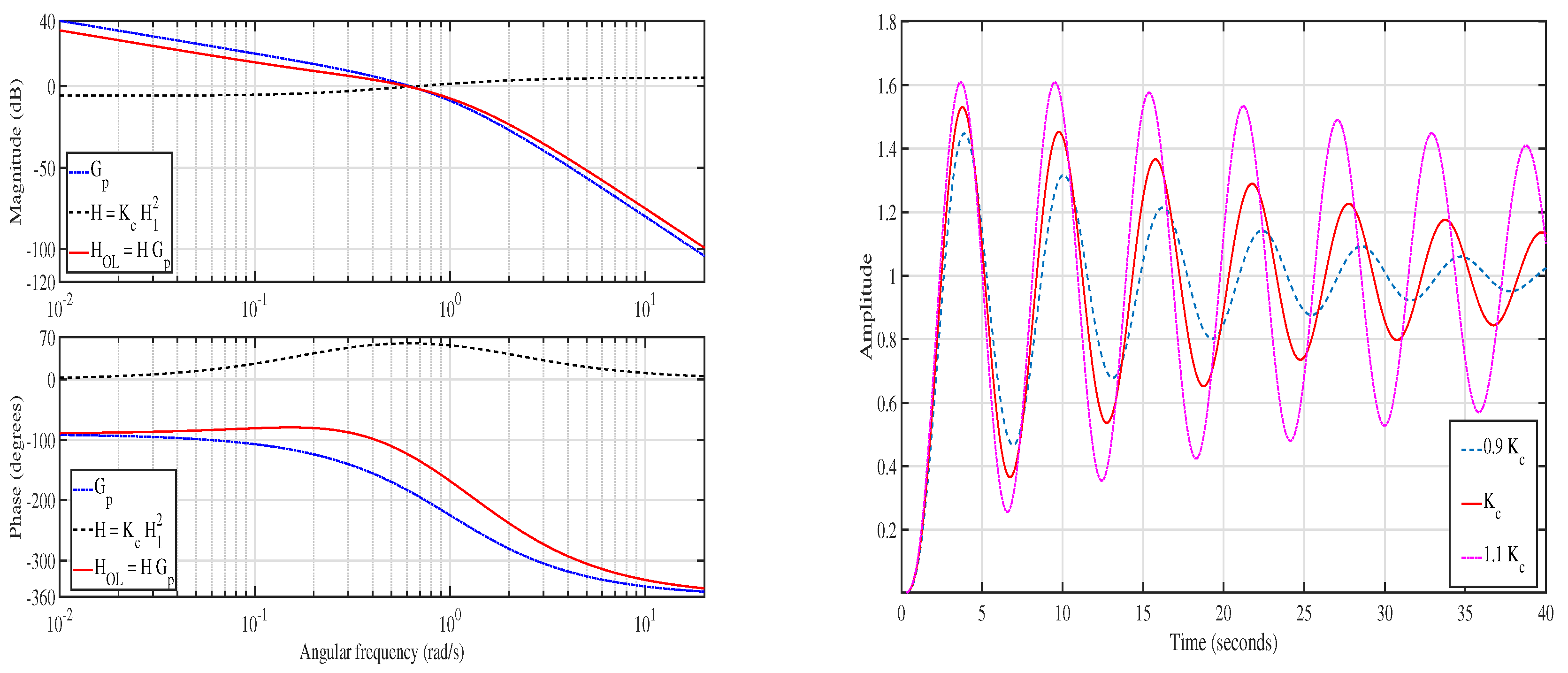

| First example | 0.9 | 2.46 | 0.90 | 21.47 | ||
| 2.19 | 6.55 | 18.50 | ||||
| 1.1 | 2.00 | 11.90 | 17.34 | |||
| 0.9 | 2.04 | 4.46 | 21.30 | |||
| 1.1 | 2.34 | 8.64 | 19.29 | |||
| 0.9 | 2.38 | 6.68 | 19.01 | |||
| 1.1 | 2.01 | 6.70 | 21.90 | |||
| Second example | 0.9 | – | 1.03 | 2.16 | 10.30 | |
| – | 0.89 | 10.89 | 9.40 | |||
| 1.1 | – | 0.78 | 19.71 | 9.03 | ||
| – | 0.9 | 0.95 | 8.47 | 9.56 | ||
| – | 1.1 | 0.82 | 13.76 | 11.47 |
| 0.9 | 1.01 | 3.01 | 12.95 | ||
| 0.87 | 12.65 | 12.65 | |||
| 1.1 | 0.76 | 22.38 | 12.58 | ||
| 0.9 | 0.96 | 8.87 | 10.15 | ||
| 1.1 | 0.78 | 17.24 | 12.50 | ||
| 0.9 | 1.00 | 4.04 | 14.12 | ||
| 0.85 | 14.54 | 13.32 | |||
| 1.1 | 0.73 | 25.12 | 16.29 | ||
| 0.9 | 0.96 | 9.05 | 13.60 | ||
| 1.1 | 0.74 | 21.23 | 16.06 |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maione, G. Design of Cascaded and Shifted Fractional-Order Lead Compensators for Plants with Monotonically Increasing Lags. Fractal Fract. 2020, 4, 37. https://doi.org/10.3390/fractalfract4030037
Maione G. Design of Cascaded and Shifted Fractional-Order Lead Compensators for Plants with Monotonically Increasing Lags. Fractal and Fractional. 2020; 4(3):37. https://doi.org/10.3390/fractalfract4030037
Chicago/Turabian StyleMaione, Guido. 2020. "Design of Cascaded and Shifted Fractional-Order Lead Compensators for Plants with Monotonically Increasing Lags" Fractal and Fractional 4, no. 3: 37. https://doi.org/10.3390/fractalfract4030037
APA StyleMaione, G. (2020). Design of Cascaded and Shifted Fractional-Order Lead Compensators for Plants with Monotonically Increasing Lags. Fractal and Fractional, 4(3), 37. https://doi.org/10.3390/fractalfract4030037





