Tempered Fractional Equations for Quantum Transport in Mesoscopic One-Dimensional Systems with Fractal Disorder
Abstract
:1. Introduction
2. Tempered Fractional Stable Distributions
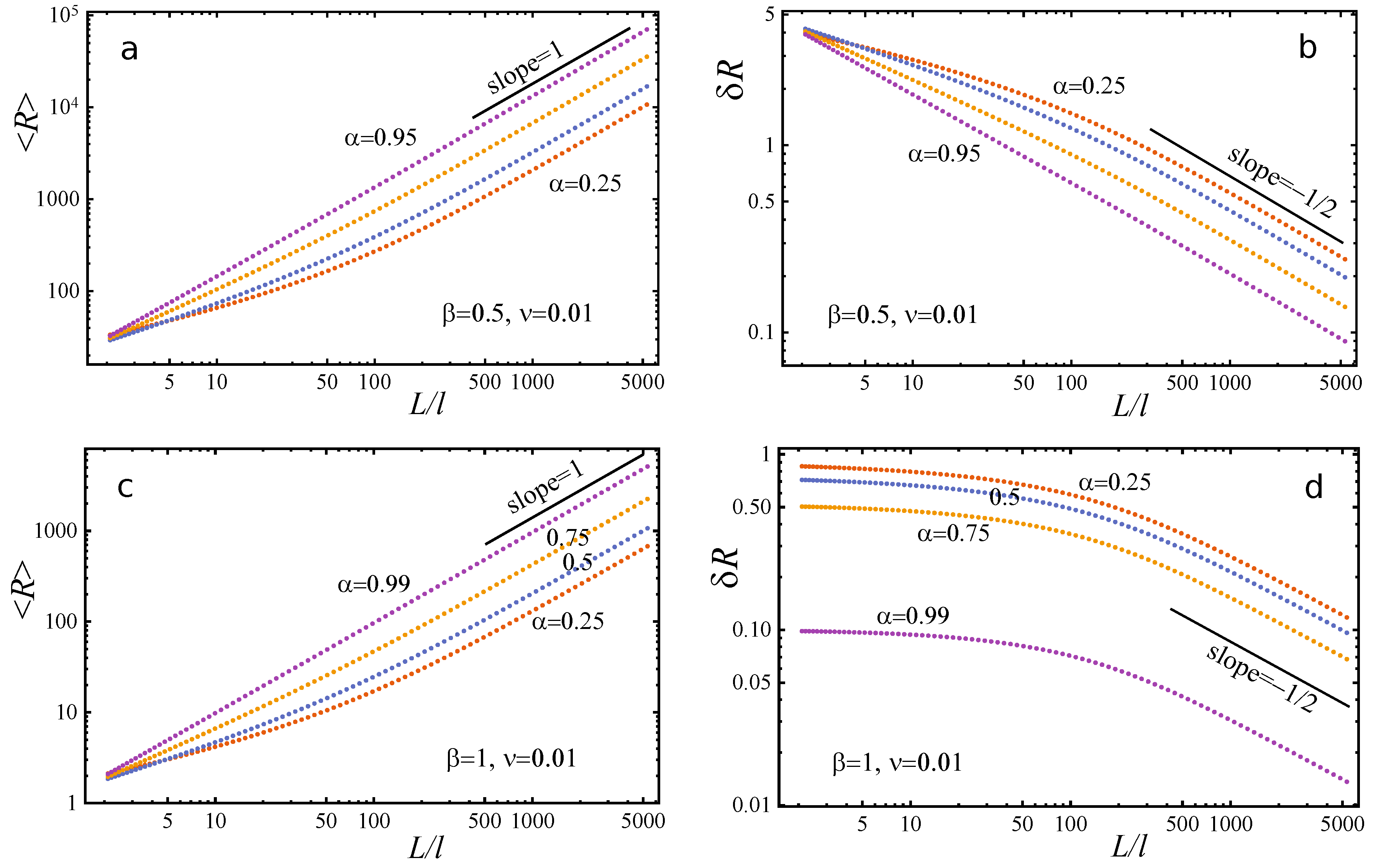
3. Weak Scattering in Mesoscopic Fractal Wires
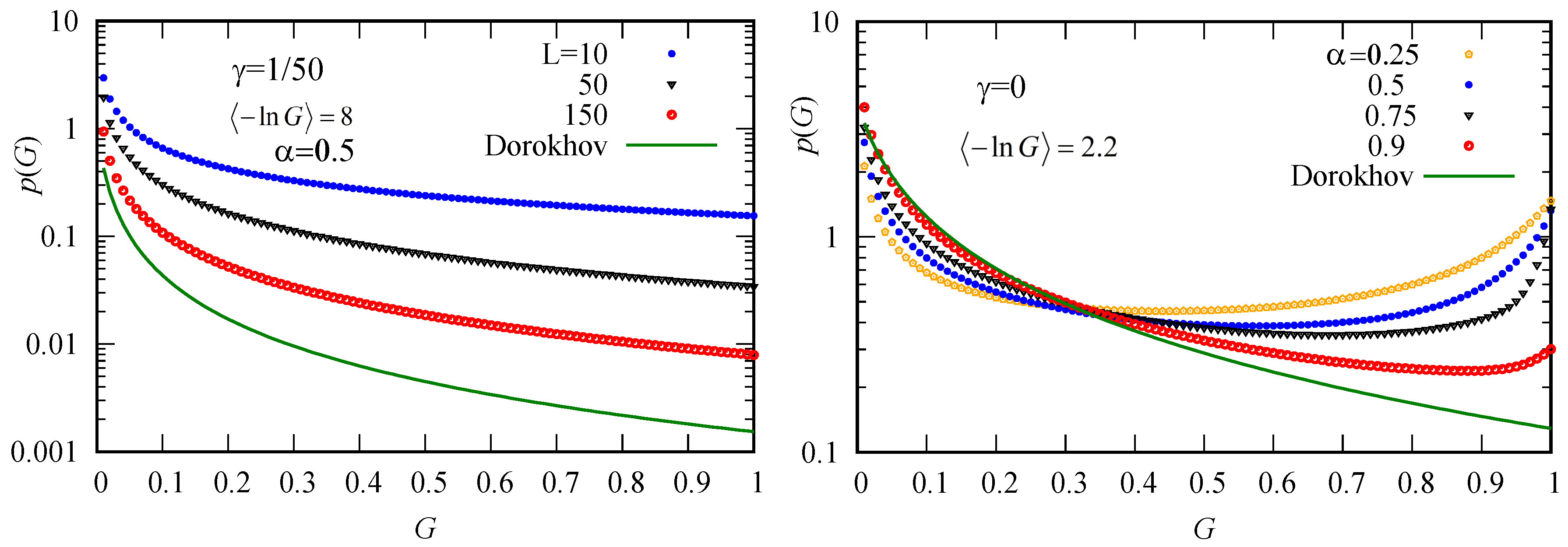
4. Incoherent Sequential Tunneling Through Wire with Lévy-Type Disorder
5. Concluding Remarks
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Pook, W.; Janßen, M. Multifractality and scaling in disordered mesoscopic systems. Zeitschrift für Physik B Condens. Matter 1991, 82, 295–298. [Google Scholar] [CrossRef]
- Hegger, H.; Huckestein, B.; Hecker, K.; Janssen, M.; Freimuth, A.; Reckziegel, G.; Tuzinski, R. Fractal conductance fluctuations in gold nanowires. Phys. Rev. Lett. 1996, 77, 3885. [Google Scholar] [CrossRef] [PubMed]
- Barthelemy, P.; Bertolotti, J.; Wiersma, D.S. A Lévy flight for light. Nature 2008, 453, 495. [Google Scholar] [CrossRef] [PubMed]
- Kohno, H.; Yoshida, H. Multiscaling in semiconductor nanowire growth. Phys. Rev. E 2004, 70, 062601. [Google Scholar] [CrossRef] [PubMed]
- Kohno, H. Self-organized nanowire formation of Si-based materials. In One-Dimensional Nanostructures; Springer: Berlin, Germany, 2008; pp. 61–78. [Google Scholar]
- Raboutou, A.; Peyral, P.; Lebeau, C.; Rosenblatt, J.; Burin, J.P.; Fouad, Y. Fractal vortices in disordered superconductors. Phys. A Stat. Mech. Appl. 1994, 207, 271–279. [Google Scholar] [CrossRef]
- Beenakker, C.; Groth, C.; Akhmerov, A. Nonalgebraic length dependence of transmission through a chain of barriers with a Lévy spacing distribution. Phys. Rev. B 2009, 79, 024204. [Google Scholar] [CrossRef]
- Falceto, F.; Gopar, V.A. Conductance through quantum wires with Lévy-type disorder: Universal statistics in anomalous quantum transport. Europhys. Lett. 2011, 92, 57014. [Google Scholar] [CrossRef]
- Sibatov, R.T. Distribution of the conductance of a linear chain of tunnel barriers with fractal disorder. JETP Lett. 2011, 93, 503–507. [Google Scholar] [CrossRef]
- Fernández-Marín, A.; Méndez-Bermúdez, J.; Gopar, V.A. Photonic heterostructures with Lévy-type disorder: Statistics of coherent transmission. Phys. Rev. A 2012, 85, 035803. [Google Scholar] [CrossRef]
- Amanatidis, I.; Kleftogiannis, I.; Falceto, F.; Gopar, V.A. Conductance of one-dimensional quantum wires with anomalous electron wave-function localization. Phys. Rev. B 2012, 85, 235450. [Google Scholar] [CrossRef]
- Burioni, R.; di Santo, S.; Lepri, S.; Vezzani, A. Scattering lengths and universality in superdiffusive Lévy materials. Phys. Rev. E 2012, 86, 031125. [Google Scholar] [CrossRef] [PubMed]
- Fernández-Marín, A.A.; Méndez-Bermúdez, J.; Carbonell, J.; Cervera, F.; Sánchez-Dehesa, J.; Gopar, V. Beyond Anderson localization in 1D: Anomalous localization of microwaves in random waveguides. Phys. Rev. Lett. 2014, 113, 233901. [Google Scholar] [CrossRef] [PubMed]
- Kotulski, M. Asymptotic distributions of continuous-time random walks: A probabilistic approach. J. Stat. Phys. 1995, 81, 777–792. [Google Scholar] [CrossRef]
- Montroll, E.W.; Weiss, G.H. Random walks on lattices. II. J. Math. Phys. 1965, 6, 167–181. [Google Scholar] [CrossRef]
- Kolokoltsov, V.; Korolev, V.; Uchaikin, V. Fractional stable distributions. J. Math. Sci. 2001, 105, 2569–2576. [Google Scholar] [CrossRef]
- Koponen, I. Analytic approach to the problem of convergence of truncated Lévy flights towards the Gaussian stochastic process. Phys. Rev. E 1995, 52, 1197. [Google Scholar] [CrossRef] [PubMed]
- Rosiński, J. Tempering stable processes. Stoch. Process. Their Appl. 2007, 117, 677–707. [Google Scholar] [CrossRef] [Green Version]
- Mantegna, R.N.; Stanley, H.E. Stochastic process with ultraslow convergence to a Gaussian: The truncated Lévy flight. Phys. Rev. Lett. 1994, 73, 2946. [Google Scholar] [CrossRef]
- Sibatov, R.T.; Uchaikin, V.V. Truncated Lévy statistics for dispersive transport in disordered semiconductors. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 4564. [Google Scholar] [CrossRef]
- Sibatov, R.T.; Morozova, E.V. Tempered fractional model of transient current in organic semiconductor layers. In Theory and Applications of Non-Integer Order Systems; Springer: Berlin, Germany, 2017. [Google Scholar]
- Saichev, A.I.; Zaslavsky, G.M. Fractional kinetic equations: solutions and applications. Chaos Interdiscip. J. Nonlinear Sci. 1997, 7, 753–764. [Google Scholar] [CrossRef] [Green Version]
- Metzler, R.; Klafter, J. The random walk’s guide to anomalous diffusion: A fractional dynamics approach. Phys. Rep. 2000, 339, 1–77. [Google Scholar] [CrossRef]
- Uchaikin, V.V.; Zolotarev, V.M. Chance and Stability: Stable Distributions and Their Applications; Walter de Gruyter: Berlin, Germany, 1999. [Google Scholar]
- Uchaikin, V.V.; Sibatov, R.T. Fractional Kinetics in Solids: Anomalous Charge Transport in Semiconductors, Dielectrics, and Nanosystems; World Scientific: Singapore, 2013. [Google Scholar]
- Sabzikar, F.; Meerschaert, M.M.; Chen, J. Tempered fractional calculus. J. Comput. Phys. 2015, 293, 14–28. [Google Scholar] [CrossRef] [PubMed]
- Gajda, J.; Magdziarz, M. Fractional Fokker–Planck equation with tempered α-stable waiting times: Langevin picture and computer simulation. Phys. Rev. E 2010, 82, 011117. [Google Scholar] [CrossRef] [PubMed]
- Kumar, A.; Vellaisamy, P. Inverse tempered stable subordinators. Stat. Probab. Lett. 2015, 103, 134–141. [Google Scholar] [CrossRef] [Green Version]
- Alrawashdeh, M.S.; Kelly, J.F.; Meerschaert, M.M.; Scheffler, H.P. Applications of inverse tempered stable subordinators. Comput. Math. Appl. 2017, 73, 892–905. [Google Scholar] [CrossRef] [Green Version]
- Cartea, Á.; del Castillo-Negrete, D. Fluid limit of the continuous-time random walk with general Lévy jump distribution functions. Phys. Rev. E 2007, 76, 041105. [Google Scholar] [CrossRef]
- Beenakker, C.W. Random-matrix theory of quantum transport. Rev. Mod. Phys. 1997, 69, 731. [Google Scholar] [CrossRef]
- Dorokhov, O. Transmission coefficient and the localization length of an electron in N bound disordered chains. JETP Lett. 1982, 36, 318–321. [Google Scholar]
- Mello, P.; Pereyra, P.; Kumar, N. Macroscopic approach to multichannel disordered conductors. Ann. Phys. 1988, 181, 290–317. [Google Scholar] [CrossRef]
- Muttalib, K.; Klauder, J. Generalized Fokker–Planck equation for multichannel disordered quantum conductors. Phys. Rev. Lett. 1999, 82, 4272. [Google Scholar] [CrossRef]
- Beenakker, C.; Rejaei, B. Nonlogarithmic repulsion of transmission eigenvalues in a disordered wire. Phys. Rev. Lett. 1993, 71, 3689. [Google Scholar] [CrossRef] [PubMed]
- Haubold, H.J.; Mathai, A.M.; Saxena, R.K. Mittag–Leffler functions and their applications. J. Appl. Math. 2011, 2011, 298628. [Google Scholar] [CrossRef]
- Valkó, P.P.; Abate, J. Comparison of sequence accelerators for the Gaver method of numerical Laplace transform inversion. Comput. Math. Appl. 2004, 48, 629–636. [Google Scholar] [CrossRef]
- Uchaikin, V.V. Self-similar anomalous diffusion and Lévy-stable laws. Phys. Uspekhi 2003, 46, 821. [Google Scholar] [CrossRef]


© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sibatov, R.T.; Sun, H. Tempered Fractional Equations for Quantum Transport in Mesoscopic One-Dimensional Systems with Fractal Disorder. Fractal Fract. 2019, 3, 47. https://doi.org/10.3390/fractalfract3040047
Sibatov RT, Sun H. Tempered Fractional Equations for Quantum Transport in Mesoscopic One-Dimensional Systems with Fractal Disorder. Fractal and Fractional. 2019; 3(4):47. https://doi.org/10.3390/fractalfract3040047
Chicago/Turabian StyleSibatov, Renat T., and HongGuang Sun. 2019. "Tempered Fractional Equations for Quantum Transport in Mesoscopic One-Dimensional Systems with Fractal Disorder" Fractal and Fractional 3, no. 4: 47. https://doi.org/10.3390/fractalfract3040047
APA StyleSibatov, R. T., & Sun, H. (2019). Tempered Fractional Equations for Quantum Transport in Mesoscopic One-Dimensional Systems with Fractal Disorder. Fractal and Fractional, 3(4), 47. https://doi.org/10.3390/fractalfract3040047





