State of the Art on Two-Phase Non-Miscible Liquid/Gas Flow Transport Analysis in Radial Centrifugal Pumps-Part A: General Considerations on Two-Phase Liquid/Gas Flows in Centrifugal Pumps
Abstract
1. Introduction
2. Two-Phase Parameters for Pump Applications
- Gas fraction x:
- 2.
- Void fraction α:
- 3.
- Slip ratio between phases SV:
- 4.
- Mass flux G:
- 5.
- Homogeneous two-phase density ρtp:
3. Dimensional Analysis Application in Pumps
- -
- A characteristic length of the pump, i.e., the impeller diameter dimp;
- -
- The rotational speed n;
- -
- The gas and liquid flow rates, respectively, QG and QL;
- -
- The gas and liquid densities ρG and ρL;
- -
- The gas and liquid kinematic viscosities υG and υL;
- -
- The surface tension σ;
- -
- The gravitation acceleration.
- -
- is related to the liquid phase Reynolds number. Typical values reach 2 × 106, which means that viscous effects are mostly concentrated inside boundary layers. Viscous effects can be neglected compared with inertia terms.
- -
- is related to the gas phase Reynolds number, and typical values are close to 1 × 105.
- -
- is related to the Weber number. Typical values are around 3 × 106. This means that the surface tension effects can be considered negligible compared with liquid inertia effects.
- -
- is related to the ratio between gravitation and centrifugal forces, often represented by the Froude number. The order of magnitude is about 5 × 10−2. The centrifugal acceleration can be considered the most important one compared with the gravitational one inside the rotating parts of the pump.
- -
- is close to 10−3, if an air–water mixture is considered. This suggests that the most important effects on the pressure field are dominated by the liquid phase, as already mentioned. Note that, in some cases, this density ratio may be controlled and may not be neglected.
4. Pumps Two-Phase Performance Representation—Basic Physical Aspects
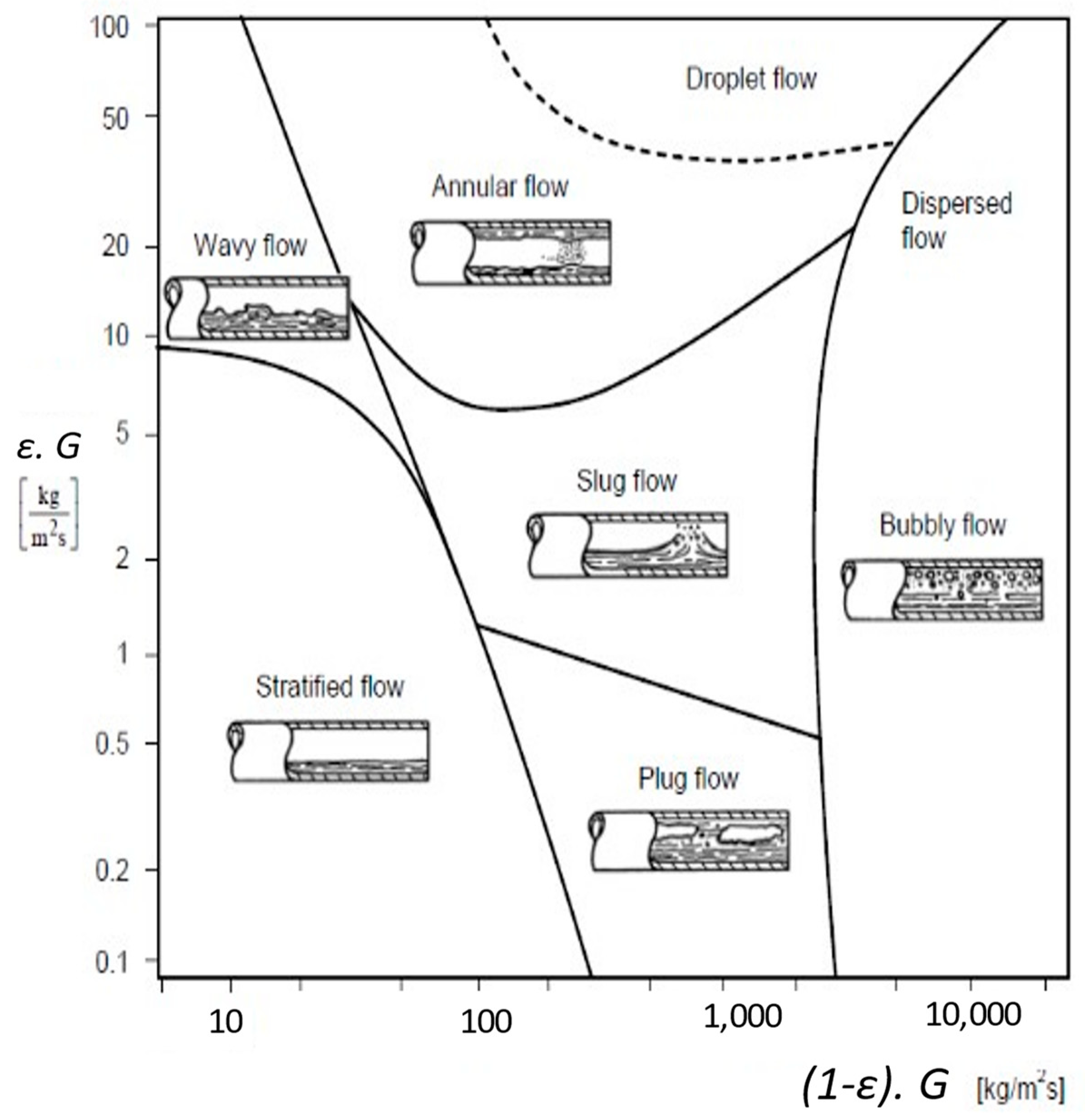
4.1. Two-Phase Flow Patterns Inside a Pump
4.2. Analogy with Flow Pattern inside a Tube
4.3. Physical Mechanism—Single Phase Conditions
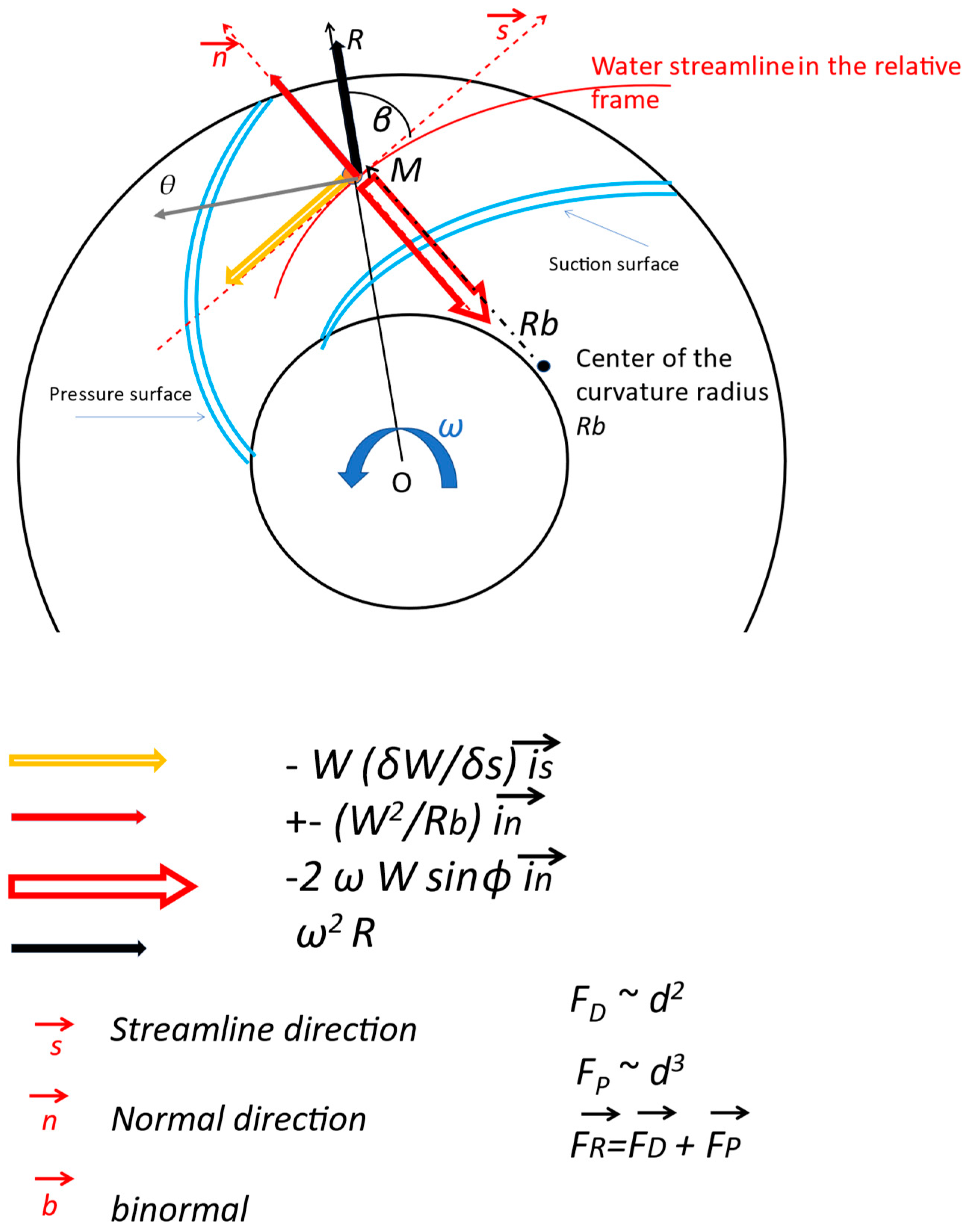
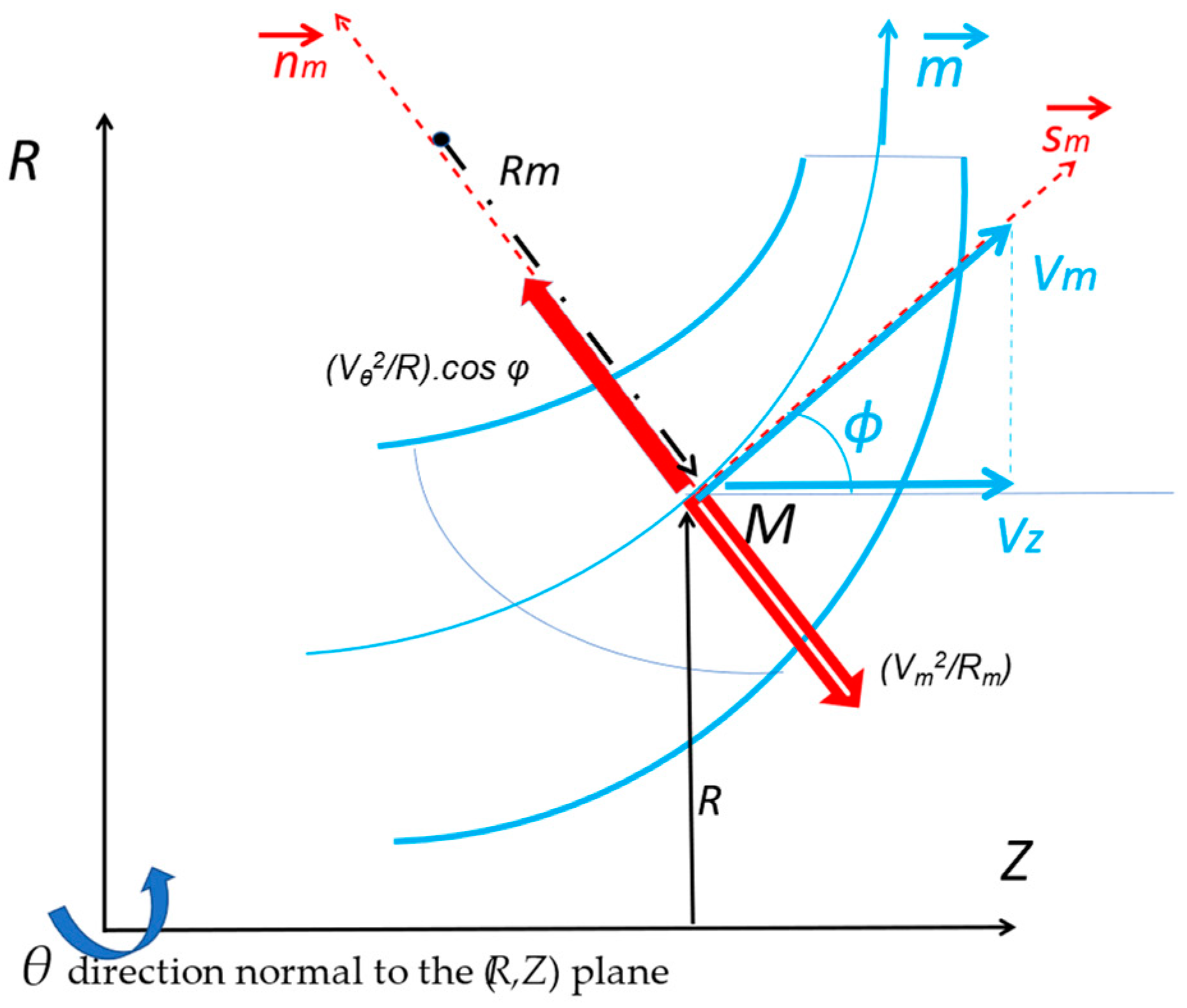
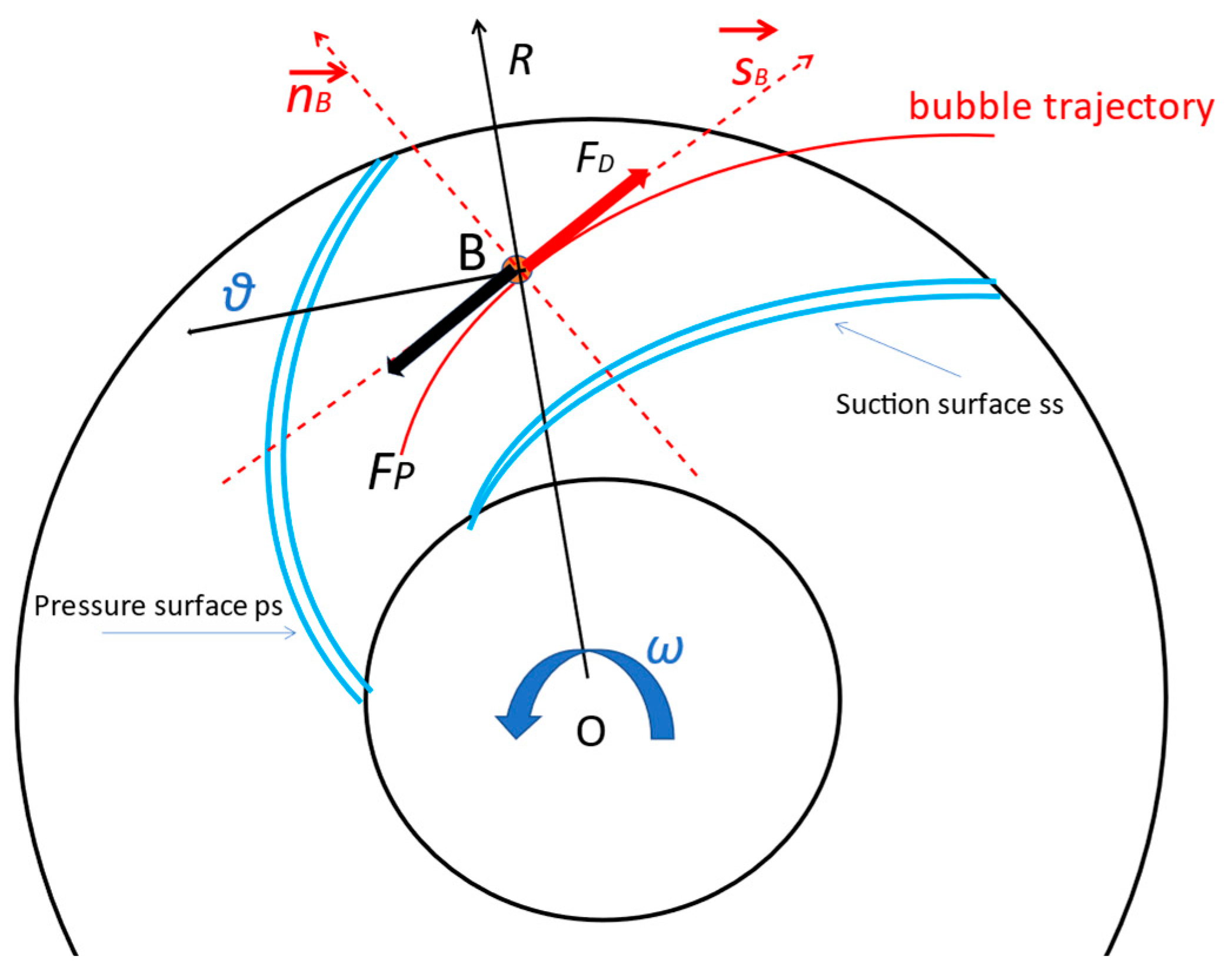
4.4. Physical Mechanism—Forces Acting on a Single Bubble inside the Main Core Flow in an Axial and a Centrifugal Impeller
5. Simplified Approaches for Pumps Two-Phase Performance Prediction
5.1. Semi-Empirical Correlation
- -
- fsp corresponds to the usual single-phase friction factor that depends on the pump Reynolds number and the wall roughness factor, as can be found on the well-known Moody diagram.
- -
- L is an approximated channel length (impeller or volute).
- -
- A is a characteristic pump area.
- -
- dhyd is the hydraulic diameter (impeller or volute).
- -
- -
- .
5.2. Analytical Approaches
- (a)
- An average mixture of homogeneous bubbly flow.
- (b)
- Considering separated phases called the two-phase modeling.
- (a)
- One-Dimensional Two-Phase Bubbly Flow Modeling
- (b)
- One-Dimensional Two-Fluid Modeling (Minemura et al. [14])
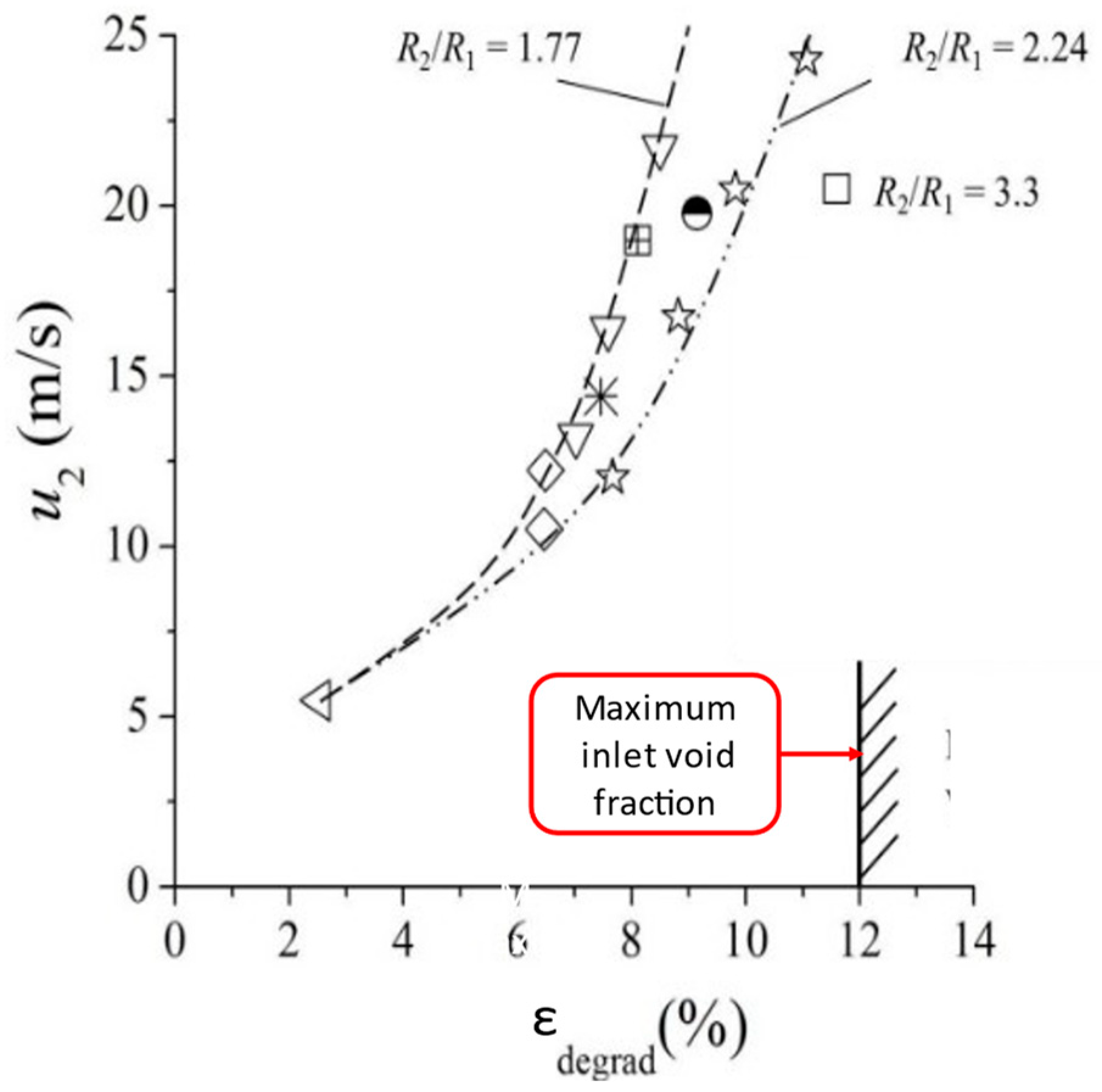
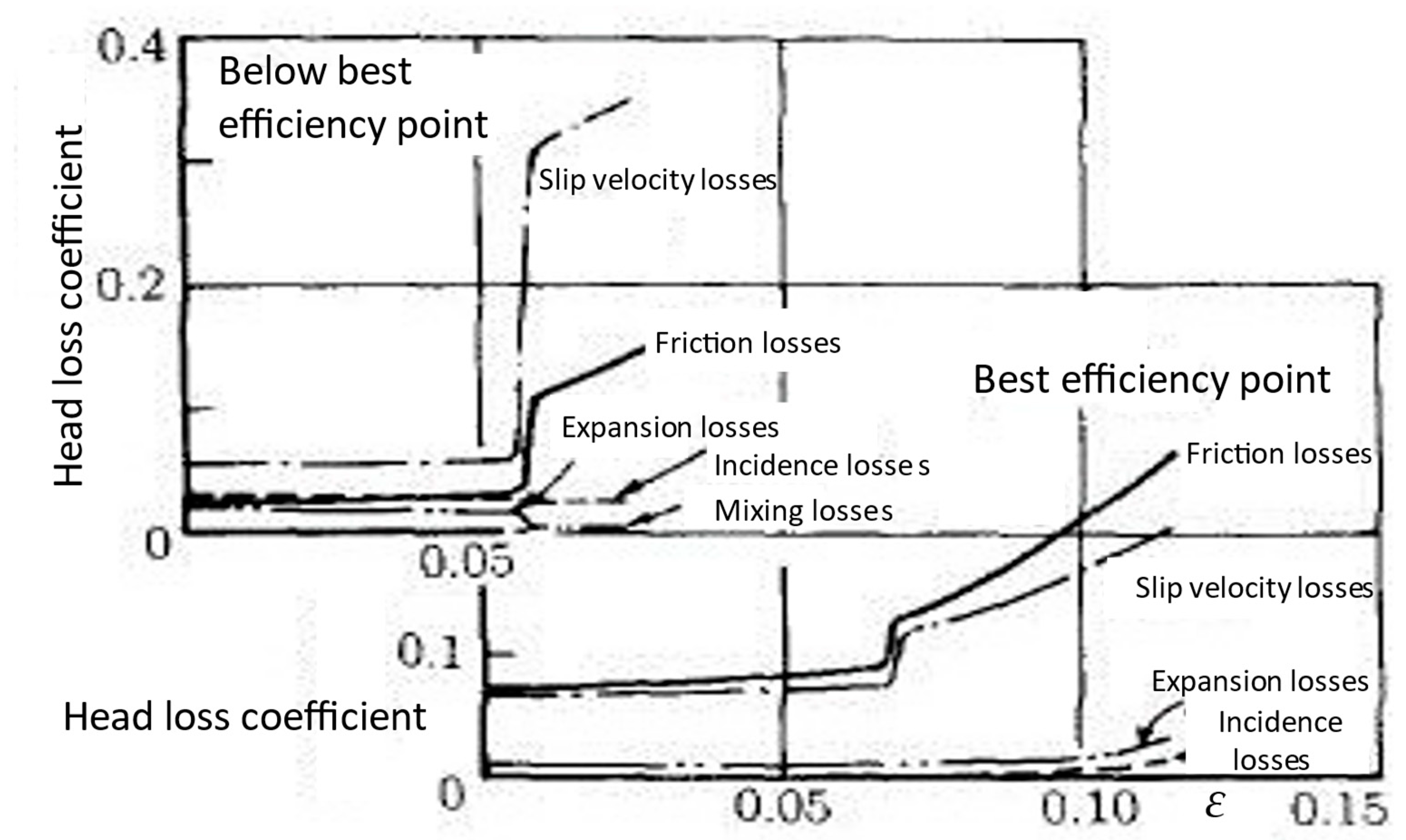
5.3. Surging Criteria Detection
6. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| a | two-phase function, |
| A | cross area |
| b | outlet impeller width- binormal direction (intrinsic coordinate system) |
| Reynolds dependent drag coefficient | |
| d | diameter |
| D | total derivative |
| Fx | function |
| G | mass flux |
| g | gravitational acceleration |
| head | |
| head loss ratio | |
| h | enthalpy |
| K | constant |
| mass flow rate per unit time | |
| m | mass |
| n | direction normal to s (intrinsic coordinate system) |
| n | rotational speed |
| p | pressure |
| Q | volume flow rate |
| R | radius |
| r | radial vector direction |
| s | direction of a streamline. (Intrinsic coordinate system) |
| SV | phase slip ratio |
| t | blade pitch, time |
| U | rotating velocity |
| V | absolute velocity |
| Ʋ | volume |
| W | relative velocity |
| x | gas fraction—gas quality |
| z | axial direction |
| α | local void fraction, absolute flow angle |
| β | gas to liquid ratio, relative flow angle |
| δ | derivative |
| Δ | increment, difference |
| ε | inlet void fraction |
| φ | flow coefficient, |
| φ | meridional angle |
| μ | dynamic viscosity |
| υ | kinematic viscosity = μ/ρ |
| ζ | loss coefficient |
| θ | tangential direction |
| ρ | density |
| ψ | head coefficient, |
| η | efficiency |
| ω | angular velocity |
| σ | surface tension |
| Indices | |
| be | best efficiency |
| B | bubble |
| cs | cross sectional |
| degrad | related to 50% head decrease. |
| D | drag |
| G | gas |
| H | homogenous |
| hyd | hydraulic |
| imp | impeller |
| L | liquid |
| LOSS | related to losses |
| m | meridional |
| opt | optimum |
| p | due to pressure gradient |
| ps | pressure side |
| R | resultant |
| rel | relative to the liquid phase |
| ss | suction side |
| sp | single phase |
| t | total |
| th | theoretical |
| tp | two-phase |
| v | volumetric |
| 0 | related to α = 0 |
| 1 | impeller inlet section |
| 2 | impeller outlet section |
Appendix A
Appendix A.1. Two-Phase Basic Definitions
- (a)
- Gas Fraction (or Gas Quality) x
- (b)
- Void Fraction
- (c)
- Cross-Sectional Void Fraction
- -
- Homogeneous model, which assumes the two phases travel at the same velocity.
- -
- One-dimensional models which account for differing velocities of the two phases.
- -
- Two-dimensional models incorporating the normal distribution of the local void fraction and velocities.
- -
- Models based on the physics of specific flow regimes.
- -
- Empirical and semi-empirical methods.
Appendix A.2. Homogeneous Model and Velocity Ratio
- (a)
- Homogeneous Void Fraction
- (b)
- Velocity Ratio: SV.
- (c)
- Mass Flux G.
- (d)
- Homogeneous Two-Phase Density ρtp
Appendix B
- Pressure force: ;
- Centrifugal force: ;
- Drag force: ;
- Virtual mass force: .
References
- Gamboa, G.; Prado, M. Experimental Study of Two-Phase Performance of an Electric Submersible Pump Stage. SPE Prod. Oper. 2012, 27, 414–421. [Google Scholar] [CrossRef]
- Estevam, V.; França, F.A.; Alhanati, F.J.S. Mapping the performance of centrifugal pumps under two-phase conditions. In Proceedings of the 17th Congress of Mechanical Engineering, COBEM 2003, Sao Paulo, Brasil, 10–14 November 2003. [Google Scholar]
- Barrios, L. Visualization of Multiphase Performance inside an Electrical Submersible Pump. Ph.D. Thesis, The University of Tulsa, Tulsa, OK, USA, 2007. [Google Scholar]
- Verde, W.M.; Biazussi, J.L.; Sassim, N.A.; Bannwart, A.C. Experimental study of gas-liquid two-phase flow patterns within centrifugal pumps impellers. Exp. Therm. Fluid Sci. 2017, 85, 37–51. [Google Scholar] [CrossRef]
- Zhu, Z.J.; Zhang, H.Q. A Review of Experiments and Modeling of Gas-Liquid Flow in Electrical Submersible Pumps. Energies 2018, 11, 180. [Google Scholar] [CrossRef]
- Jiang, Q.; Heng Liu, X.; Zhang, W.; Bois, G.; Si, Q. A Review of Design Considerations of Centrifugal Pump Capability for Handling Inlet Gas-Liquid Two-Phase Flows. Energies 2019, 12, 1078. [Google Scholar] [CrossRef]
- Baker, O. Design of pipelines for simultaneous oil and gas flow. Oil Gas J. 1954, 26. [Google Scholar]
- Shaoa, C.; Lia, C.; Zhoua, J. Experimental investigation of flow patterns and external performance of a centrifugal pump that transports gas-liquid two-phase mixtures. Int. J. Heat Fluid Flow 2018, 71, 460–469. [Google Scholar] [CrossRef]
- Gülich, J.F. Centrifugal Pumps; Springer: Berlin/Heidelberg, Germany, 2010; ISBN 978-3-642-12823-3. [Google Scholar] [CrossRef]
- Stel, H.; Ofuchi, E.M.; Sabino, R.H.G.; Ancajima, F.C.; Bertoldi, D.; Neto AM, M.; Morales RE, M. Investigation of the Motion of Bubbles in a Centrifugal Pump Impeller. ASME J. Fluids Eng. 2018, 141, 031203. [Google Scholar] [CrossRef]
- Ofuchi, E.M.; Silva, H.L.V.; Bertoldi, D.; Mancilla, E.; Stel, H.; Morales, R.E.M. Study of the bubble motion in a centrifugal rotor based on visualization in a rotating frame of reference. Chem. Eng. Sci. 2022, 259, 117829. [Google Scholar] [CrossRef]
- Mikielewicz, J.; Gordon Wilson, D.; Chan, T.; Goldfinch, A. A Method for Correlating the Characteristics of Centrifugal Pumping Two-Phase flow. J. Fluids Eng. Trans. ASME 1978, 100, 395–409. [Google Scholar] [CrossRef]
- Furuya, O. An Analytical Model for Prediction of Two-Phase (Non condensable) Flow Pump Performance. J. Fluids Eng. Trans. ASME 1985, 107, 139–147. [Google Scholar] [CrossRef]
- Minemura, K. Prediction of Air-Water Two-Phase Flow Performance of a Centrifugal Pump based on one-dimensional Two- Fluid Model. ASME J. Fluid Eng. 1998, 120, 327–334. [Google Scholar] [CrossRef]
- Hench, J.E.; Johnston, J.P. Two-Dimensional Diffuser Performance with Subsonic, Two-Phase, Air-Water Flow. ASME J. Basic Eng. 1972, 94, 105–121. [Google Scholar] [CrossRef]
- Zuber, N.; Hench, J.E. Steady State and Transient Void Fraction of Bubbling Systems and Their Operating Limits. (Part I, Steady State Operation); Technical Report No. 62GL100; General Electric Co.: Boston, MA, USA, 1962; 334p. [Google Scholar]
- Barrios, L.; Prado, M.G. Experimental Visualization of Two-Phase Flow Inside an Electrical Submersible Pump Stage. ASME J. Energy Resour. Technol. 2011, 133, 042901. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY-NC-ND) license (https://creativecommons.org/licenses/by-nc-nd/4.0/).
Share and Cite
Bois, G. State of the Art on Two-Phase Non-Miscible Liquid/Gas Flow Transport Analysis in Radial Centrifugal Pumps-Part A: General Considerations on Two-Phase Liquid/Gas Flows in Centrifugal Pumps. Int. J. Turbomach. Propuls. Power 2023, 8, 16. https://doi.org/10.3390/ijtpp8020016
Bois G. State of the Art on Two-Phase Non-Miscible Liquid/Gas Flow Transport Analysis in Radial Centrifugal Pumps-Part A: General Considerations on Two-Phase Liquid/Gas Flows in Centrifugal Pumps. International Journal of Turbomachinery, Propulsion and Power. 2023; 8(2):16. https://doi.org/10.3390/ijtpp8020016
Chicago/Turabian StyleBois, Gerard. 2023. "State of the Art on Two-Phase Non-Miscible Liquid/Gas Flow Transport Analysis in Radial Centrifugal Pumps-Part A: General Considerations on Two-Phase Liquid/Gas Flows in Centrifugal Pumps" International Journal of Turbomachinery, Propulsion and Power 8, no. 2: 16. https://doi.org/10.3390/ijtpp8020016
APA StyleBois, G. (2023). State of the Art on Two-Phase Non-Miscible Liquid/Gas Flow Transport Analysis in Radial Centrifugal Pumps-Part A: General Considerations on Two-Phase Liquid/Gas Flows in Centrifugal Pumps. International Journal of Turbomachinery, Propulsion and Power, 8(2), 16. https://doi.org/10.3390/ijtpp8020016






