Numerical Determination of the Equivalent Sand Roughness of a Turbopump’s Surface and Its Roughness Influence on the Pump Characteristics
Abstract
:1. Introduction
2. Materials and Methods
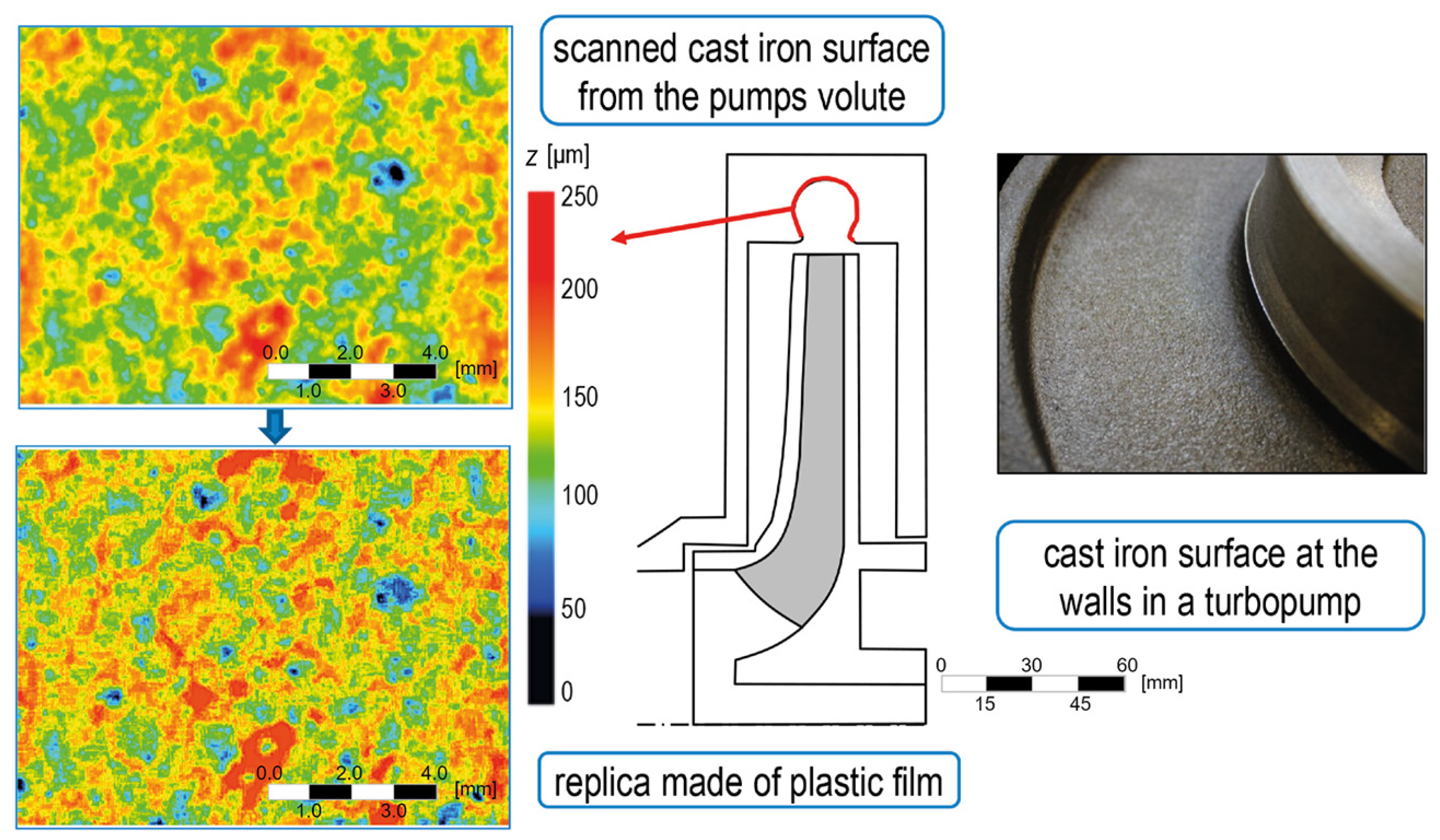
2.1. Generation of Identical and Reproducible Rough Walls for the Experiments
2.2. Experimental Setup of the Rough Channel Flow Simulations
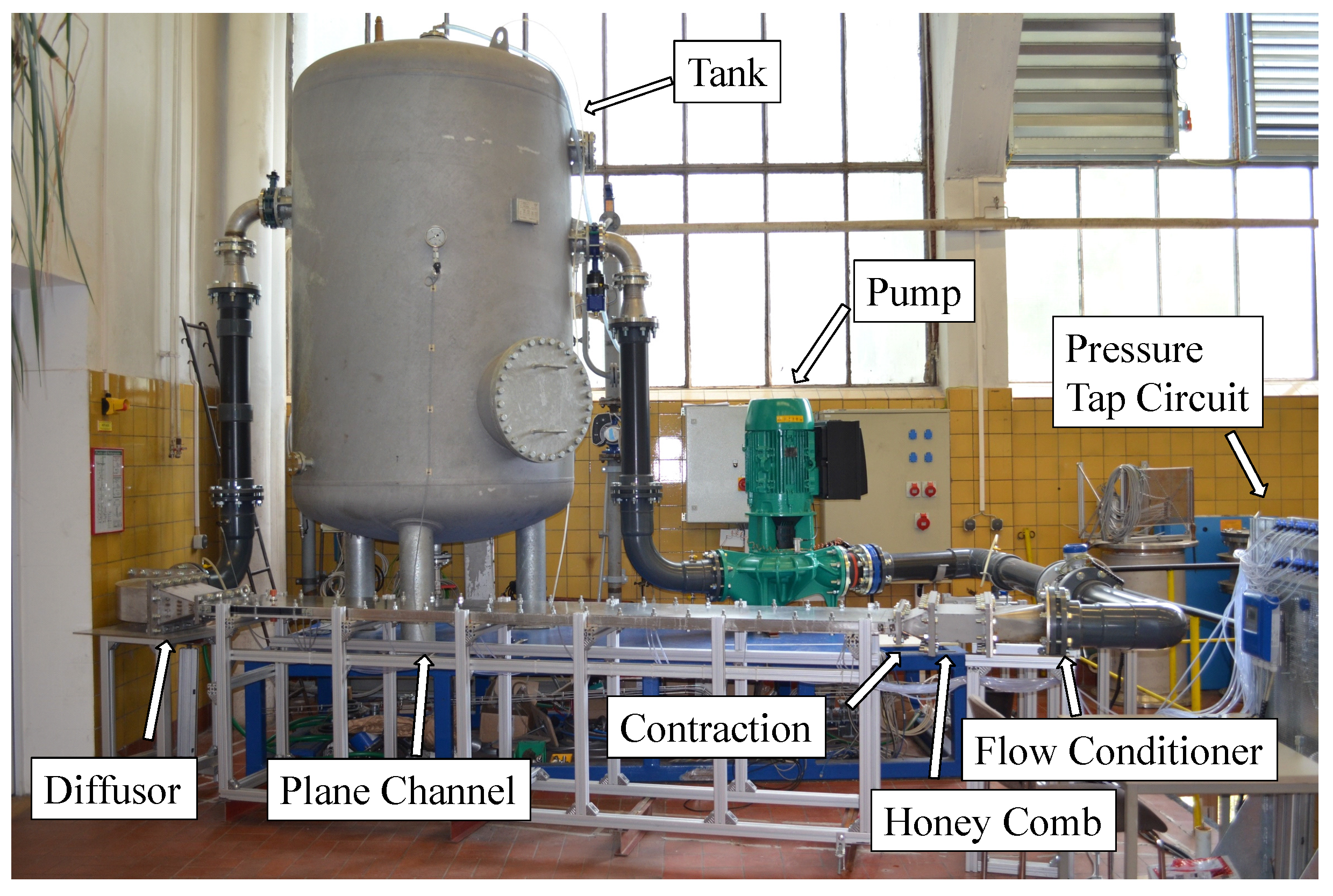
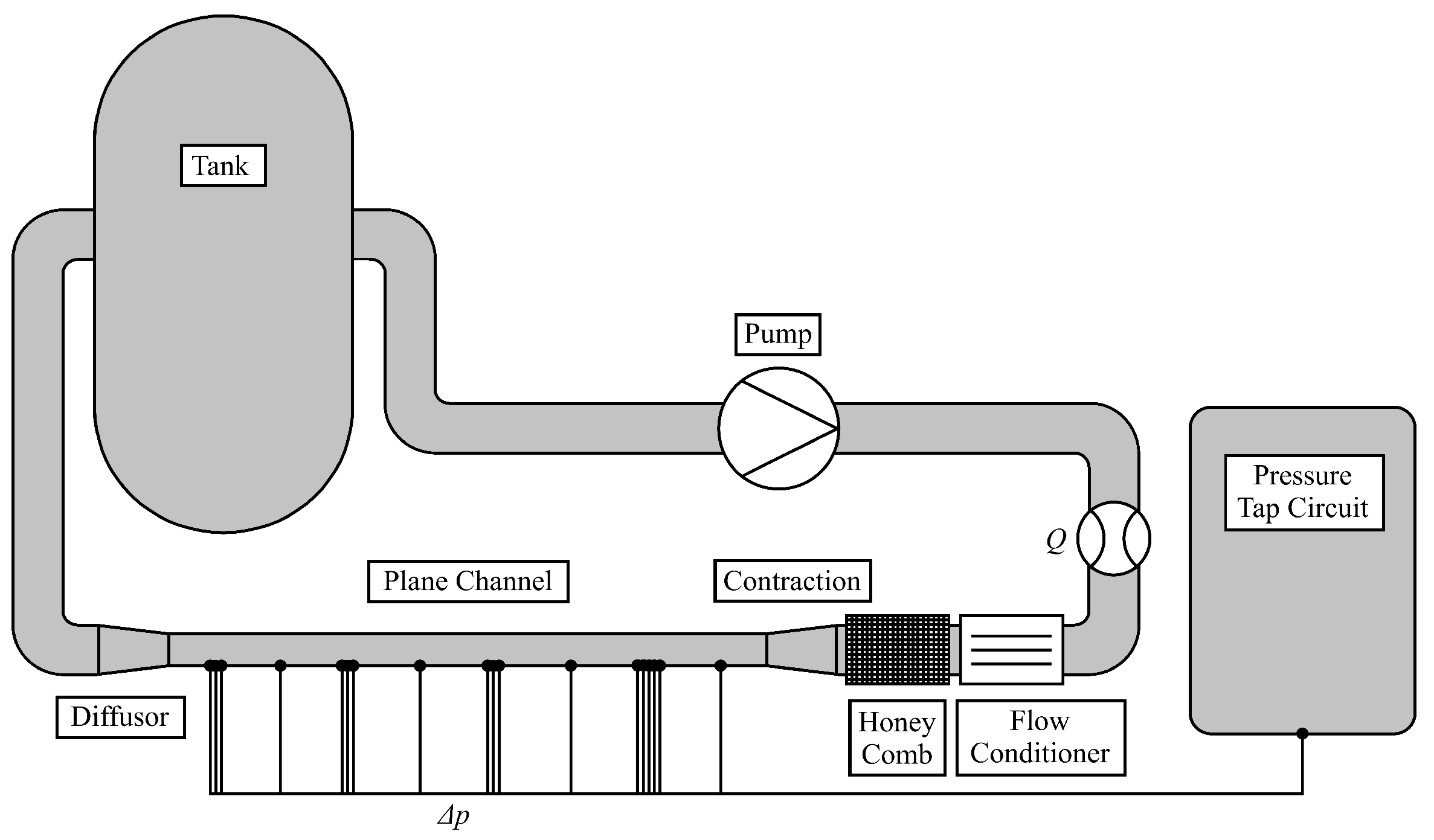
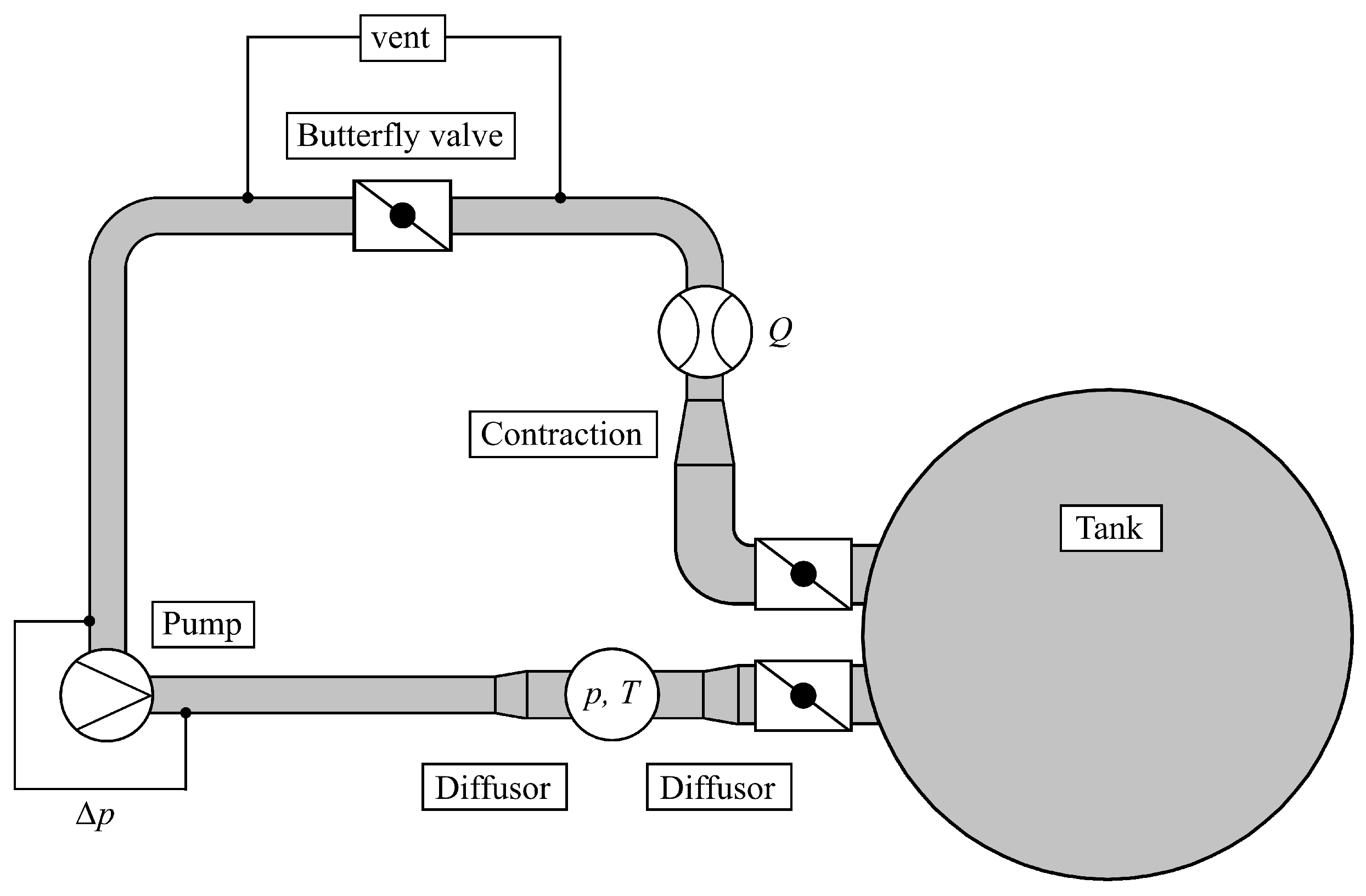
2.2.1. Test Section
2.2.2. Surface Roughness
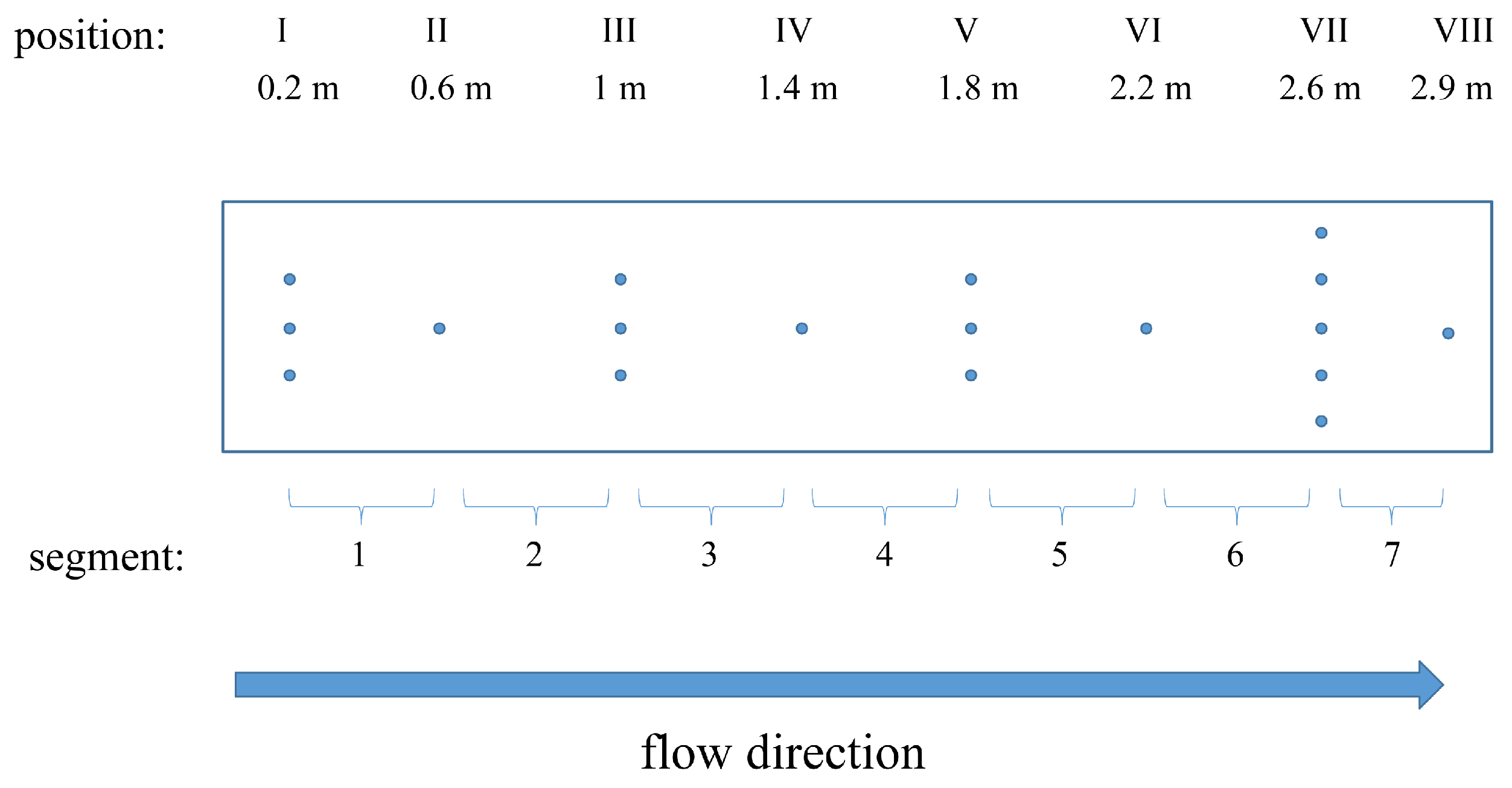
2.2.3. Measurement System and Procedure
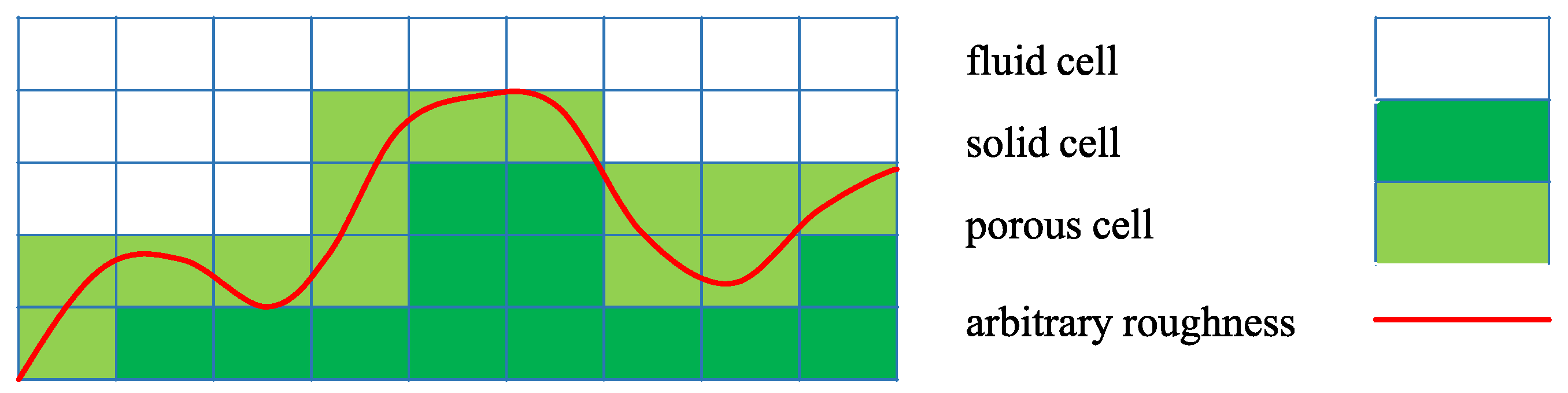
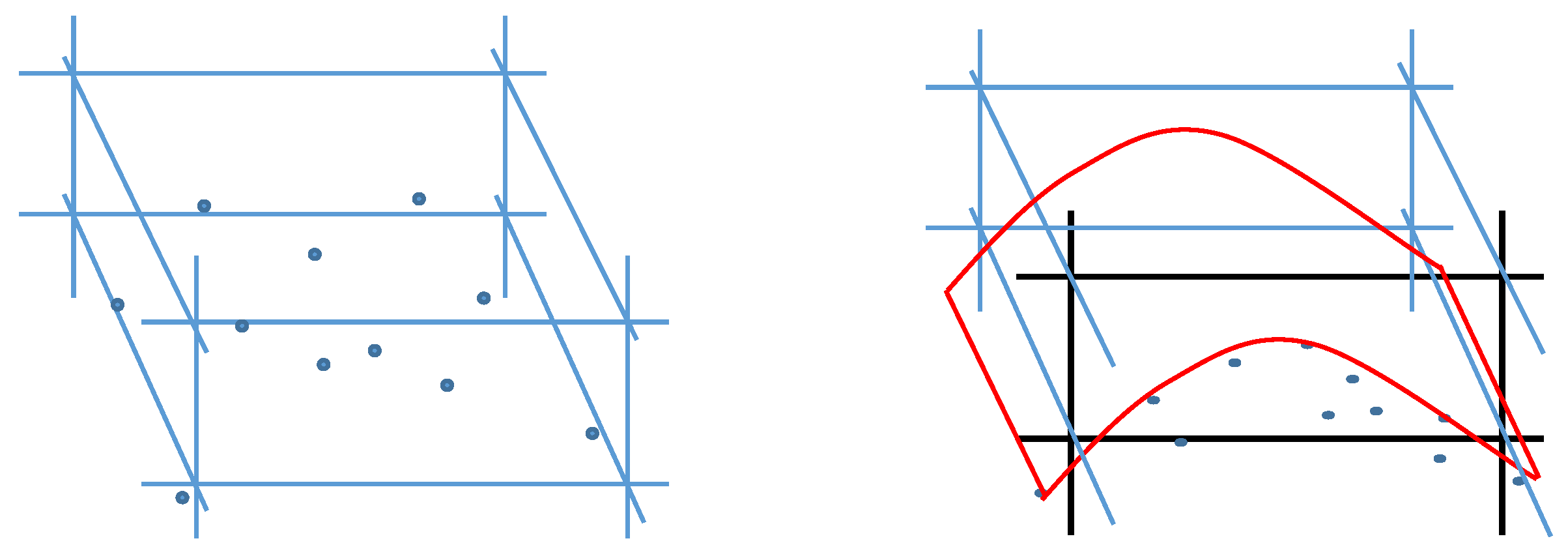
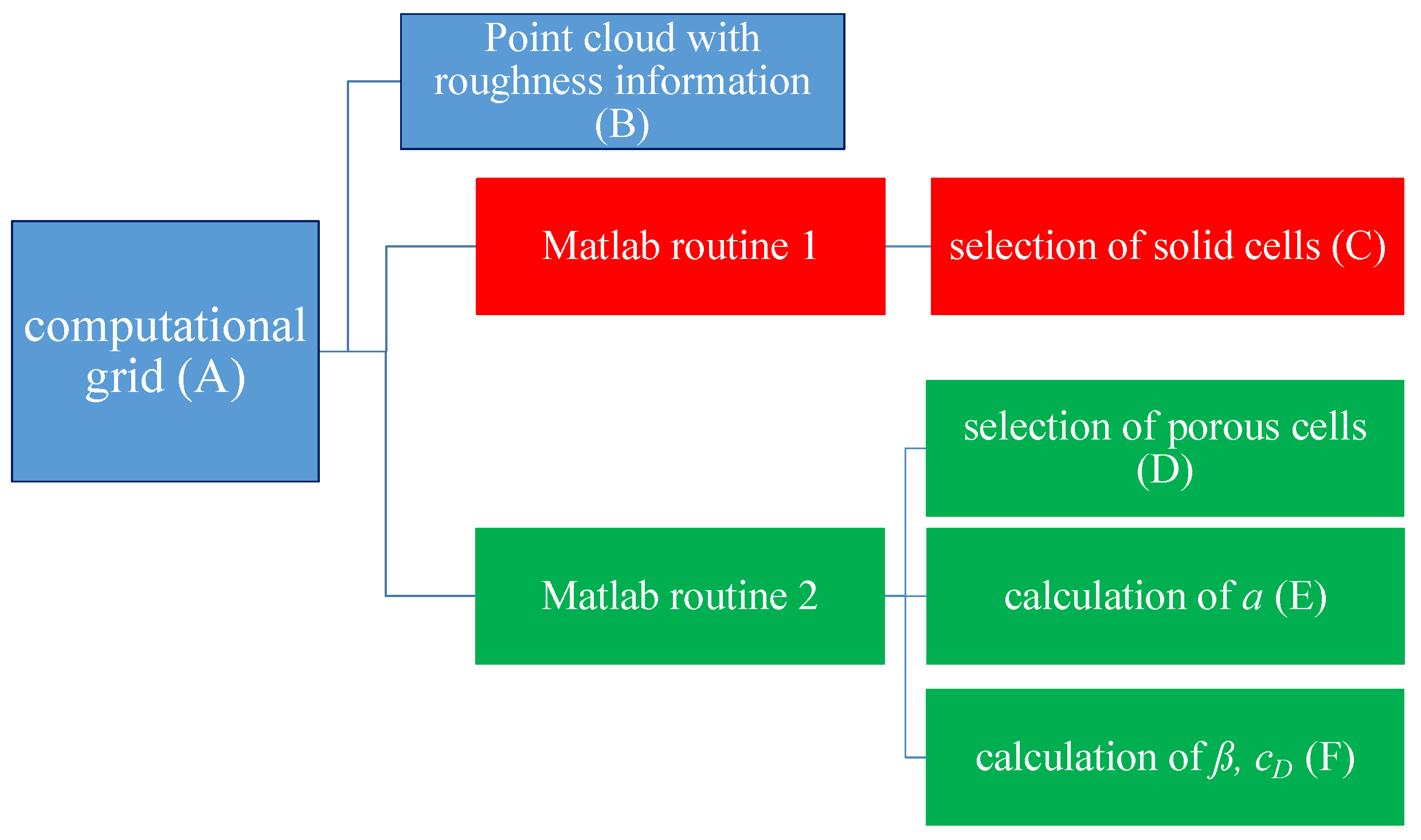
2.3. Numerical Treatment of Rough Surfaces: Discrete Porosity Method (DPM)
2.3.1. Rationale of the DPM Method
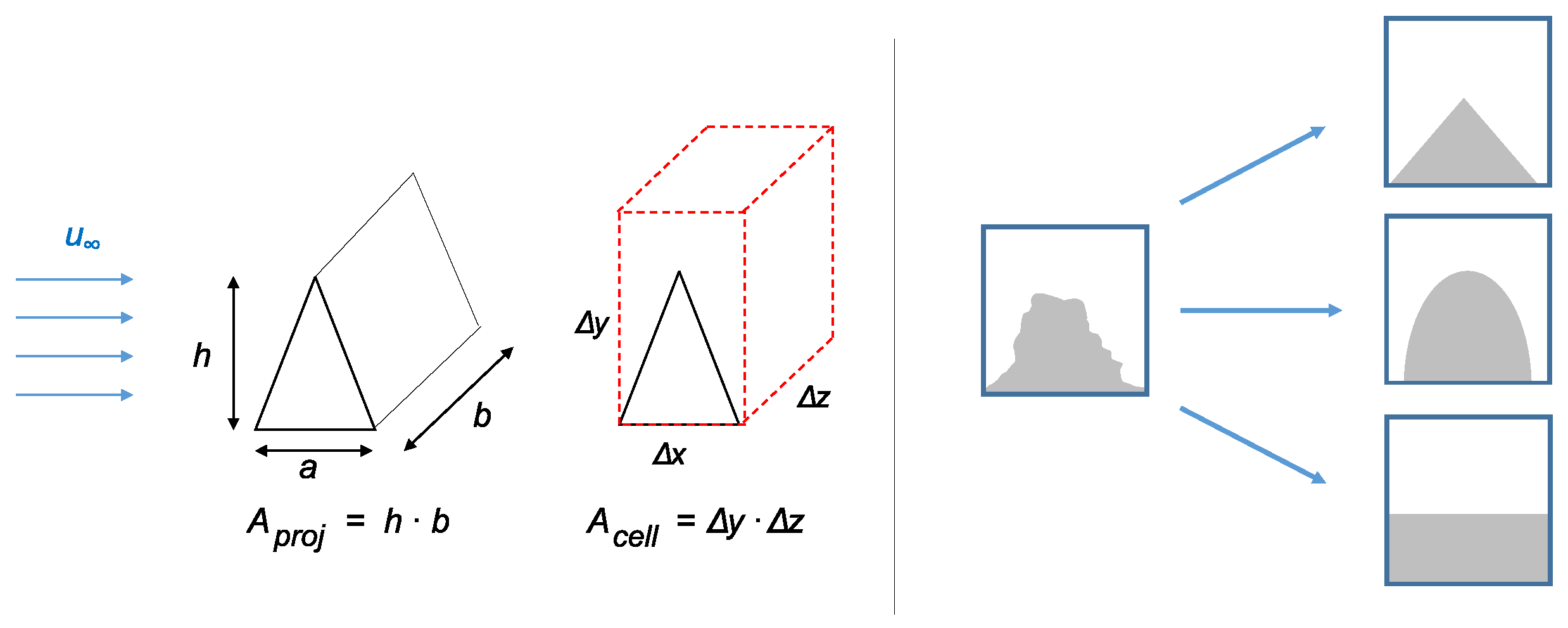
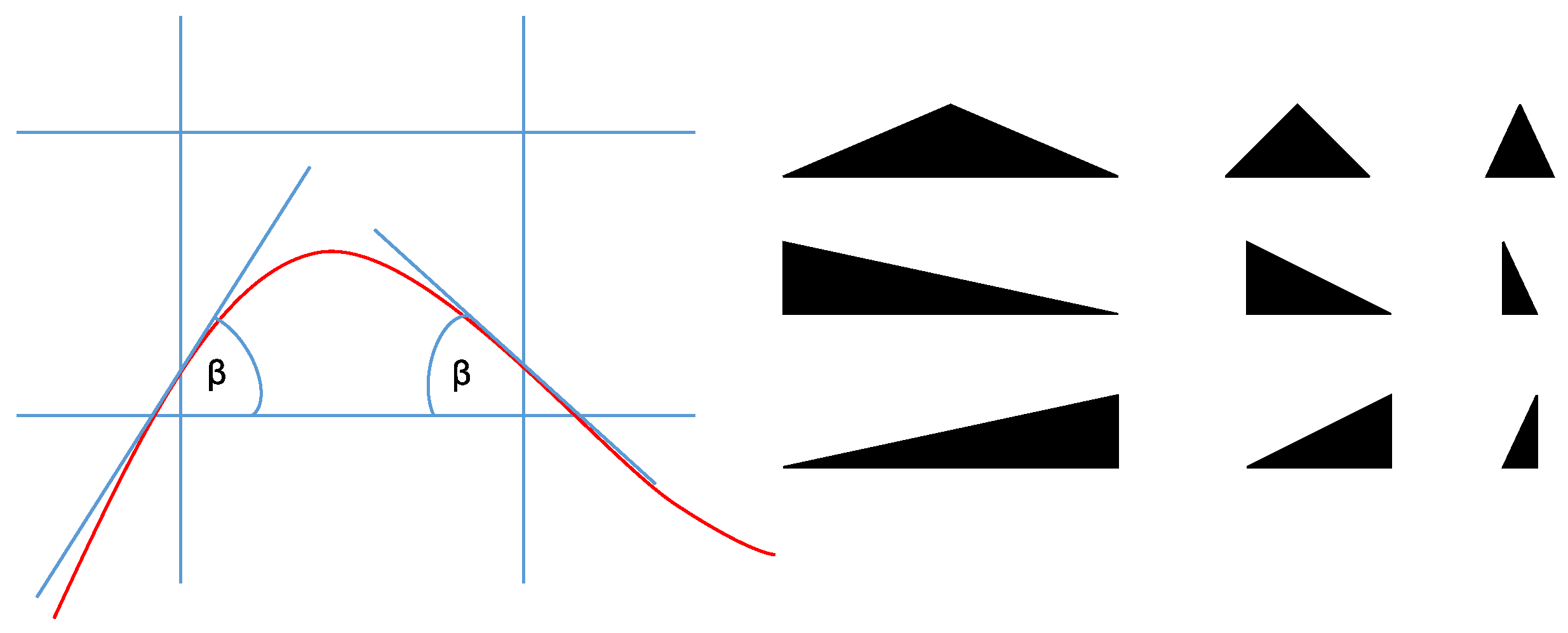
2.3.2. Determination of and
2.3.3. Implementation of the DPM
2.4. Numerical Setup for the Rough Channel Flow Simulations
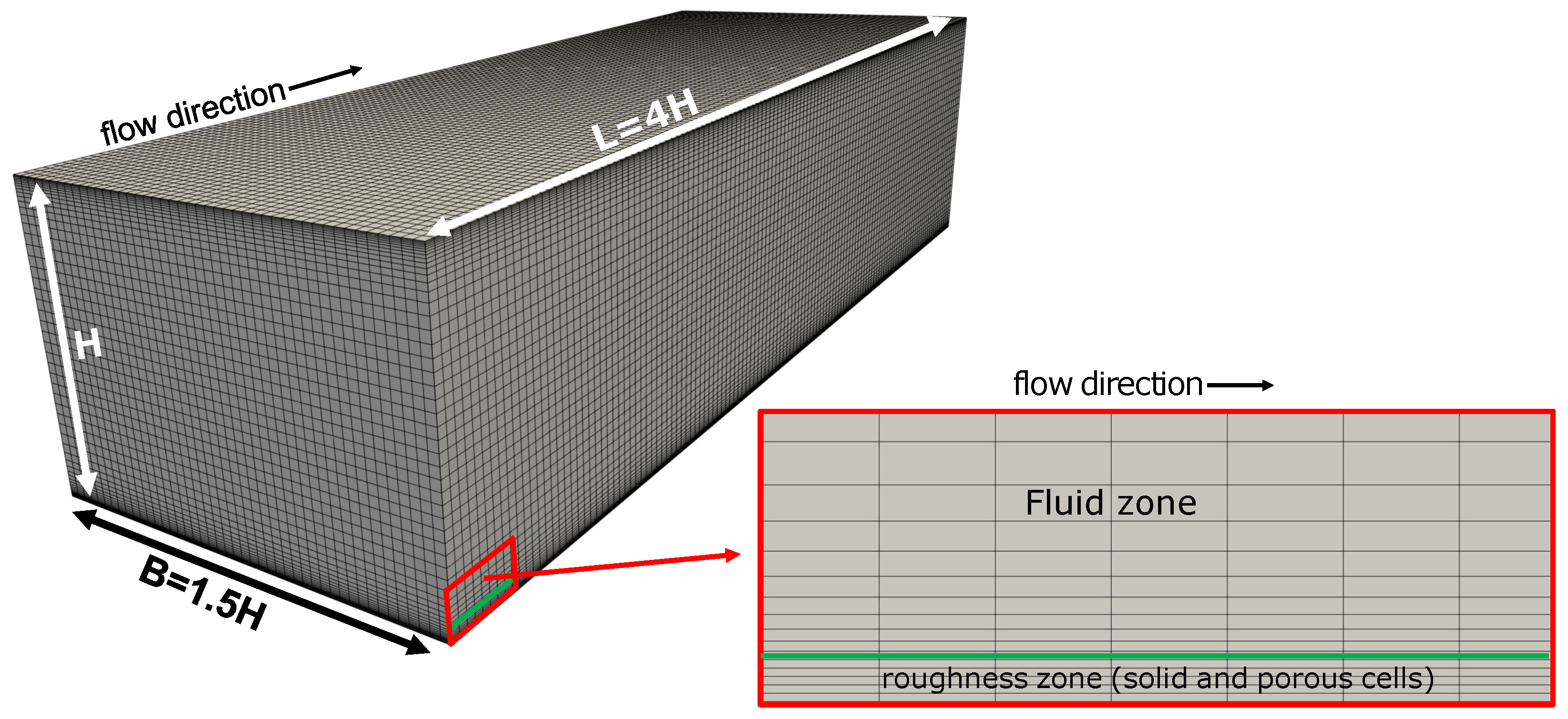
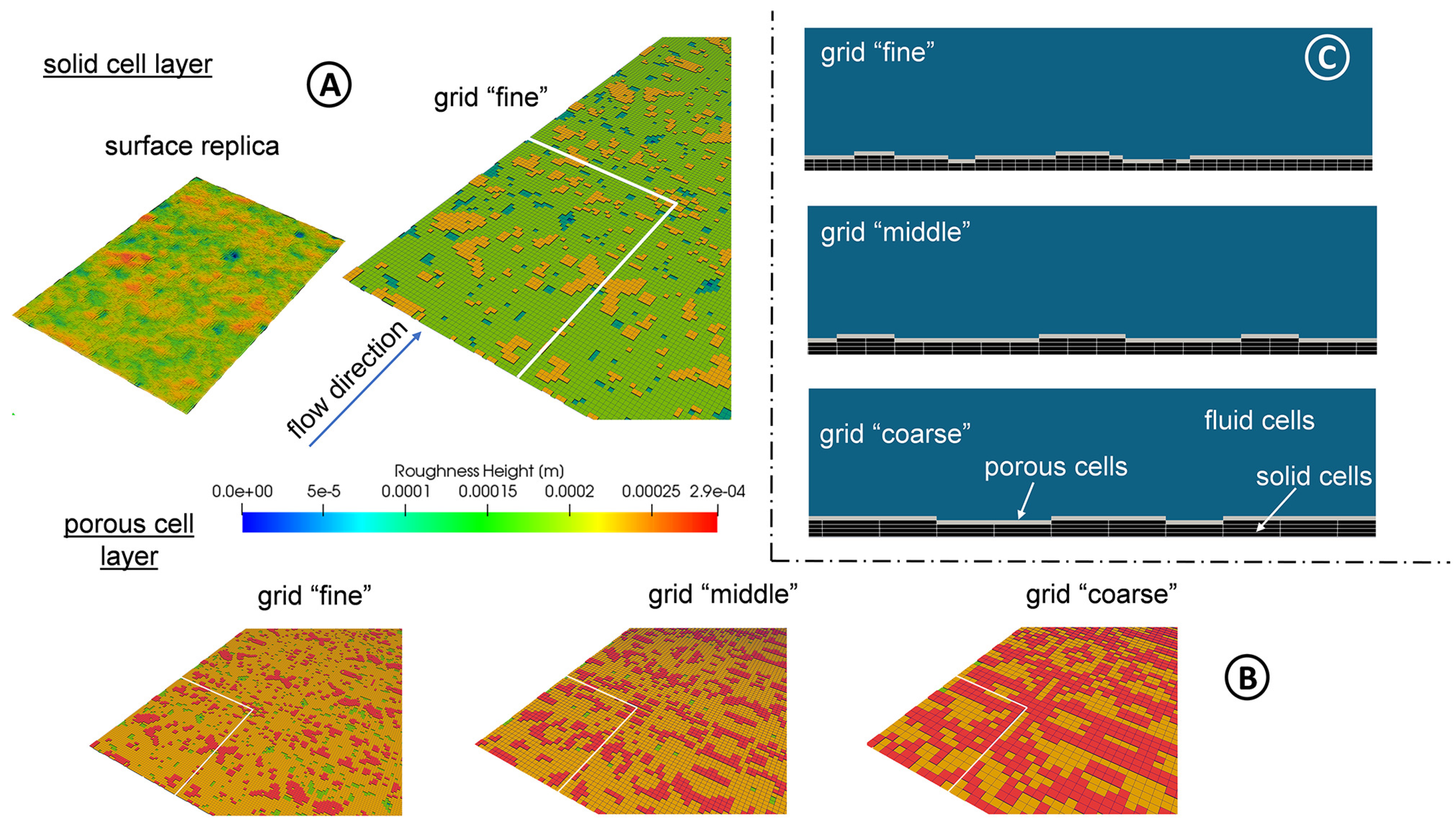
2.4.1. Computational Domain and Grid Generation
2.4.2. Consideration of the Roughness Zone (Solid and Porous Cells)
2.4.3. Numerical Setup for the DPM Simulations
2.5. Method for the Determination of the -Value for the Rough Surfaces from the DPM Simulations
2.6. Experimental and Numerical Setup for the Turbopump Investigation
2.6.1. Numerical Setup
2.6.2. Experimental Setup
3. Results and Discussion
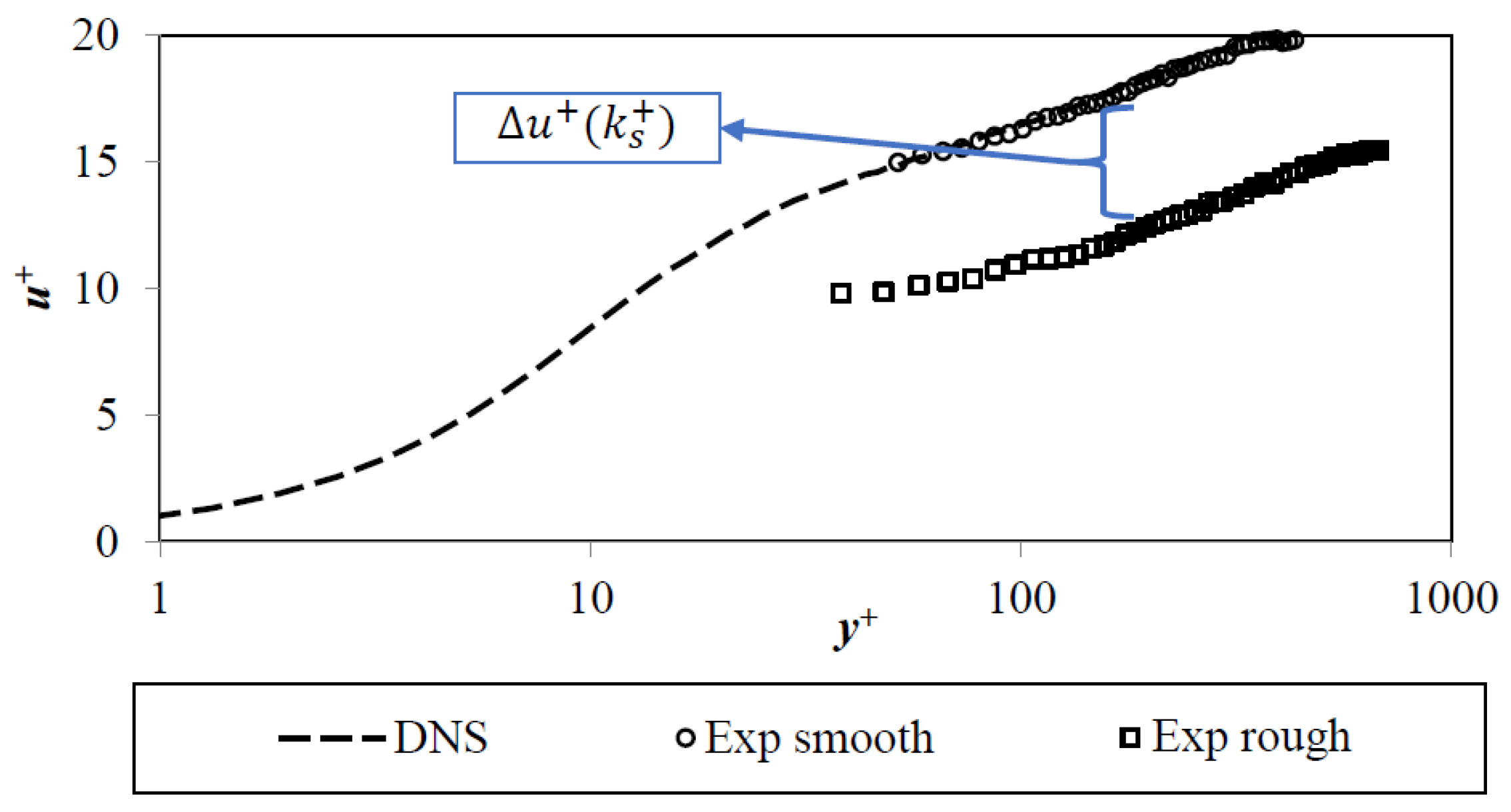
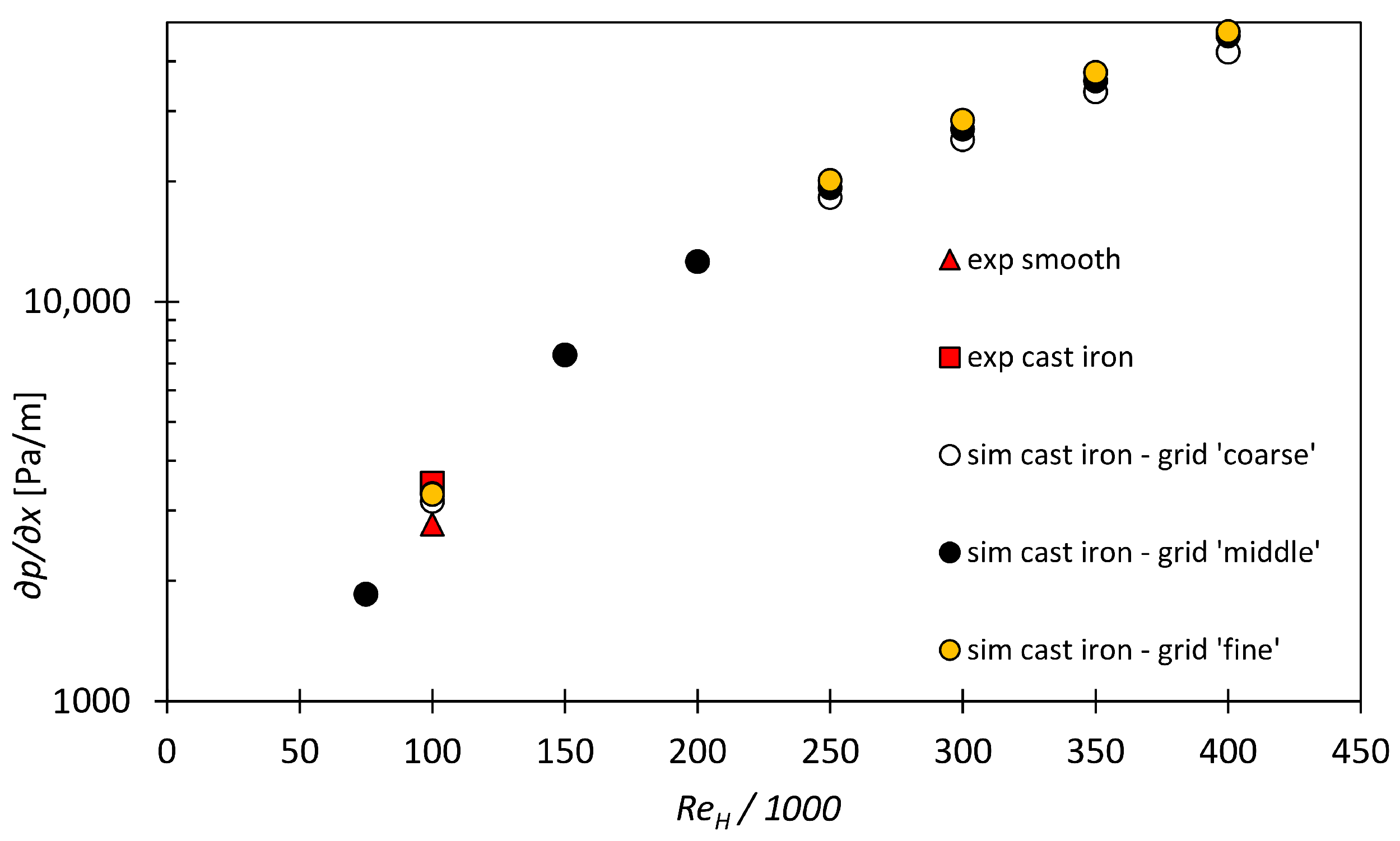
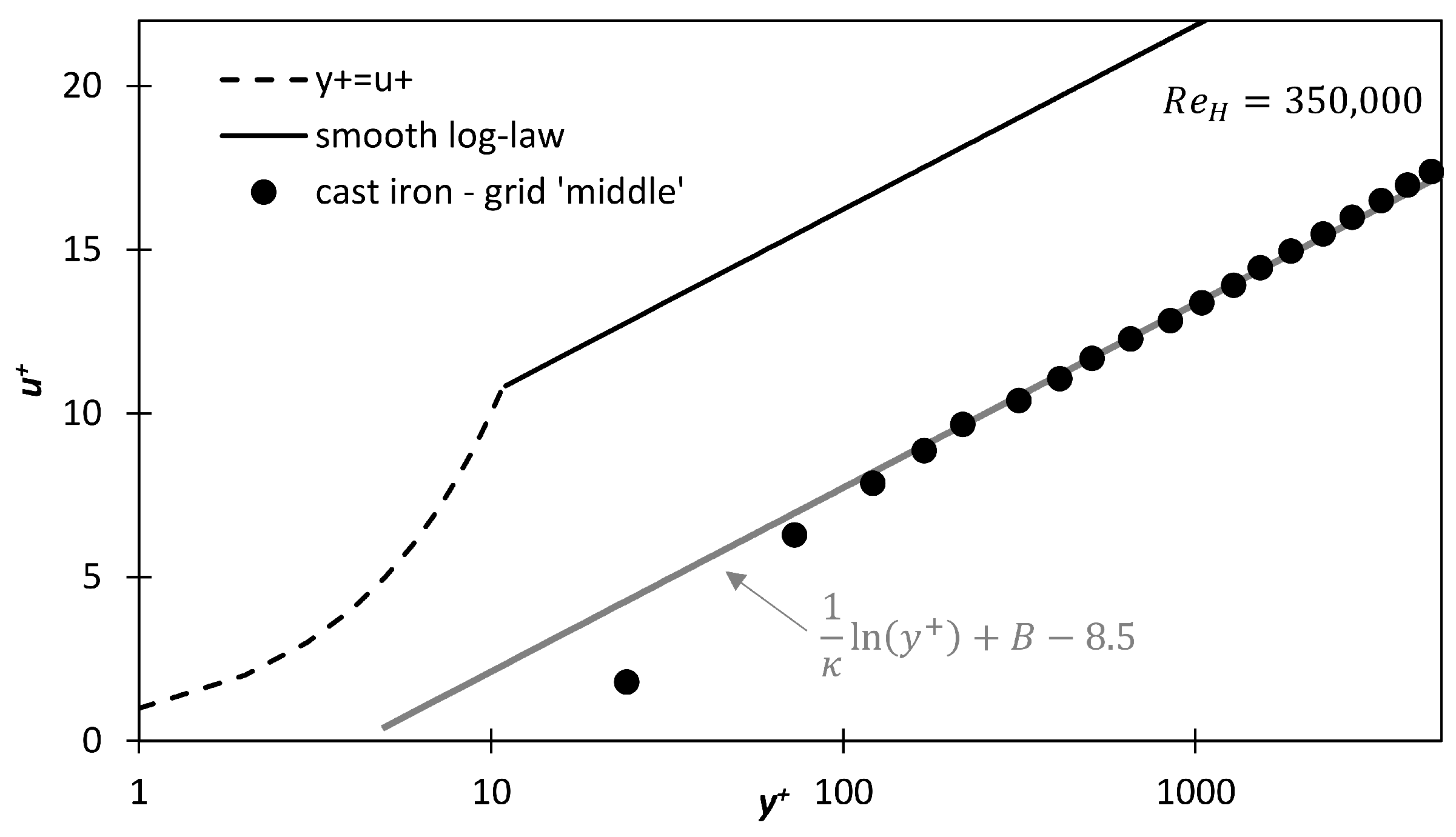
3.1. Pressure Gradients and Velocity Profiles in the Channel Flows with Wall Roughness
3.2. Determination of the Equivalent Sand Grain Roughness in the Fully Rough Regime
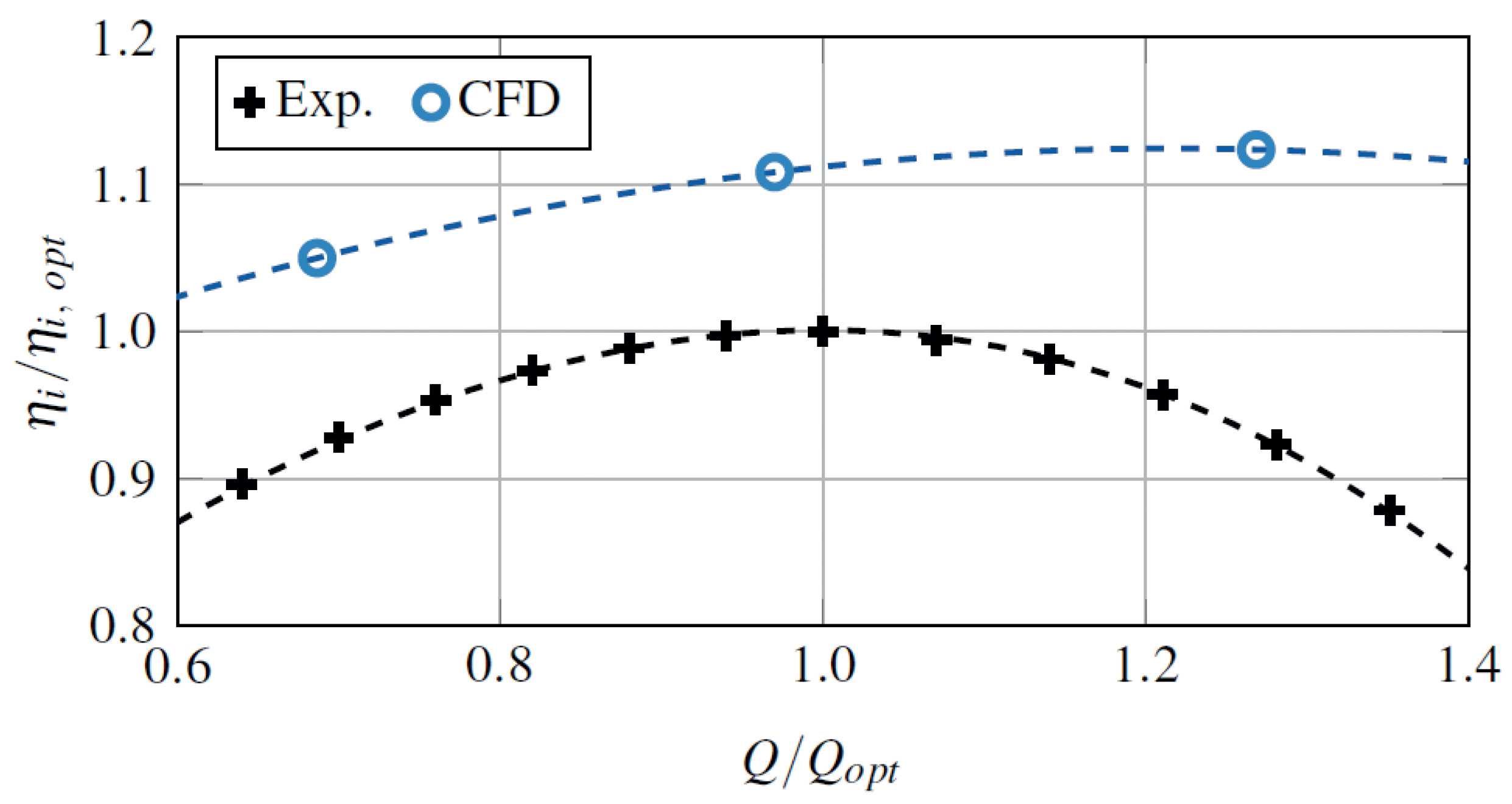
3.3. Simulation of the Performance Data in a Radial Turbopump with Wall Roughness
4. Limitations
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | area, m |
| B | constant, |
| b | width, m |
| drag coefficient, - | |
| effective slope, - | |
| unit vector parallel to z-axis, - | |
| drag force, N | |
| g | gravitational constant, m/s |
| H | channel height, m |
| pressure head, m | |
| h | height/elevation, m |
| k | turbulent kinetic energy, m/s |
| average roughness height, m | |
| peak-to-trough roughness height, m | |
| RMS roughness height, m | |
| equivalent sand grain roughness, m | |
| L | length, m |
| M | torque, Nm |
| mass flow, kg/s | |
| n | rotational speed, 1/min |
| specific speed, 1/min | |
| p | pressure, Pa |
| Q | flow rate, m/s |
| r | radius, m |
| channel Reynolds number , - | |
| S | source term, Pa/m |
| skewness, - | |
| time, s | |
| velocities, m/s | |
| bulk velocity, m/s | |
| friction velocity , m/s | |
| downshift due to roughness, - | |
| directions, m | |
| area ratio, - | |
| inflow and outflow angle, | |
| kinematic viscosity, m/s | |
| eddy viscosity, m/s | |
| density, kg/m | |
| wall shear stress, Pa | |
| specific dissipation rate, 1/s | |
| Sub-, Superscripts and Operators | |
| b | bulk |
| cell | |
| H | channel height |
| spatial directions | |
| projected | |
| optimal/BEP | |
| r | rough |
| root-mean-square | |
| s | smooth |
| total | |
| increment | |
| ∞ | free stream |
| → | vector |
| + | scaling with wall units (, ) |
| time-averaged quantity | |
| averaged quantity | |
| Abbreviations | |
| BEP | best efficiency point |
| BC | boundary condition |
| DNS | direct numerical simulation |
| DPM | discrete porosity method |
| Rel. Dev. | relative deviation |
| Re | Reynolds number |
| RMS | root-mean-square |
| URANS | unsteady Reynolds-averaged Navier-Stokes |
| 2D | two-dimensional |
| 3D | three-dimensional |
References
- Viet, D.D.; Kumar, J.; Wurm, F.H. Modelling of wall roughness in flow simulations. In Proceedings of the 4th International Rotating Equipment Conference, Wiesbaden, Germany, 23–24 September 2019; Paper No. 071. pp. 1–11. [Google Scholar]
- Juckelandt, K.; Bleeck, S.; Wurm, F.H. Analysis of Losses in Centrifugal Pumps with Low Specific Speed with Smooth and Rough Walls. In Proceedings of the 11th European Conference on Turbomachinery, Madrid, Spain, 23–26 March 2015; Martelli, F., Díaz, R.V., Eds.; 2015; pp. 1–10. [Google Scholar]
- Schlichting, H.; Gersten, K. Boundary-Layer Theory, 9th ed.; Springer: Berlin/Heidelberg, Germany, 2017. [Google Scholar]
- Schlichting, H. Experimentelle Untersuchungen zum Rauhigkeitsproblem. Ing.-Arch. 1936, 7, 1–34. [Google Scholar] [CrossRef]
- Rotta, J.C. Das in Wandnähe gültige Geschwindigkeitsgesetz turbulenter Strömungen. Ing.-Arch. 1950, 18, 277–280. [Google Scholar] [CrossRef]
- Flack, K.A.; Schultz, M.P.; Shapiro, T.A. Experimental support for Townsend’s Reynolds number similarity hypothesis on rough walls. Phys. Fluids 2005, 17, 35102. [Google Scholar] [CrossRef]
- Flack, K.A.; Schultz, M.P. Review of Hydraulic Roughness Scales in the Fully Rough Regime. J. Fluids Eng. 2010, 132, 41203. [Google Scholar] [CrossRef]
- Lechner, R.; Menter, F.R. Development of a Rough Wall Boundary Condition for Omega-Based Turbulence Models: TR-04-04, Rough Walls 1.1; ANSYS CFX: Otterfing, Germany, 2004. [Google Scholar]
- Apsley, D. CFD Calculation of Turbulent Flow with Arbitrary Wall Roughness. Flow Turbul. Combust. 2007, 78, 153–175. [Google Scholar] [CrossRef]
- Aupoix, B. Wall Roughness Modelling with k-w SST Model. In Proceedings of the 10th International ERCOFTAC Symposium on Engineering Turbulence Modelling and Measurements, Marbella, Spain, 17–19 September 2014; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Wilcox, D.C. Formulation of the k-omega Turbulence Model Revisited. AIAA J. 2008, 46, 2823–2838. [Google Scholar] [CrossRef]
- Knopp, T.; Eisfeld, B.; Calvo, J.B. A new extension for k– turbulence models to account for wall roughness. Int. J. Heat Fluid Flow 2009, 30, 54–65. [Google Scholar] [CrossRef]
- Wagner, W. Strömung und Druckverlust, 6th ed.; Vogel: Würzburg, Germany, 2008. [Google Scholar]
- Fried, E.; Idelcik, I.E. Flow Resistance: A Design Guide for Engineers, first ed.; Taylor & Francis: Philadelphia, PA, USA, 1997. [Google Scholar]
- Oertel jr., H.; Böhle, M.; Dohrmann, U. Strömungsmechanik: Grundlagen–Grundgleichungen–Lösungsmethoden—Softwarebeispiele, 5th ed.; Vieweg+Teubner: Wiesbaden, Germany, 2009. [Google Scholar]
- Gülich, J.F. Centrifugal Pumps, 3rd ed.; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- White, F.M. Fluid Mechanics, 4th ed.; McGraw-Hill: Boston, MA, USA, 1998. [Google Scholar]
- Bons, J.P. A Review of Surface Roughness Effects in Gas Turbines. J. Turbomach. 2010, 132, 021004. [Google Scholar] [CrossRef]
- Schultz, M.P.; Flack, K.A. Turbulent boundary layers on a systematically varied rough wall. Phys. Fluids 2009, 21, 015104. [Google Scholar] [CrossRef]
- Sigal, A.; Danberg, J.E. New correlation of roughness density effect on the turbulent boundary layer. AIAA J. 1990, 28, 554–556. [Google Scholar] [CrossRef]
- van Rij, J.A.; Belnap, B.J.; Ligrani, P.M. Analysis and Experiments on Three-Dimensional, Irregular Surface Roughness. J. Fluids Eng. 2002, 124, 671. [Google Scholar] [CrossRef]
- Chung, D.; Hutchins, N.; Schulz, M.P.; Flack, K.A. Predicting the Drag of Rough Surfaces. Annu. Rev. Fluid Mech. 2021, 53, 439–471. [Google Scholar] [CrossRef]
- Forooghi, P.; Stroh, A.; Magagnato, F.; Jakirlic, S.; Frohnapfel, B. Toward a universal roughness correlation. J. Fluids Eng. 2017, 139, 121201. [Google Scholar] [CrossRef]
- Chan, L.; MacDonald, M.; Chung, D.; Hutchins, N.; Ooi, A. A systematic investigation of roughness height and wavelength in turbulent pipe flow in he transitionally rough regime. J. Fluid Mech. 2015, 771, 743–777. [Google Scholar] [CrossRef]
- Yuan, J.; Piomelli, U. Estimation and prediction of the roughness function on realistic surfaces. J. Turbul. 2014, 15, 350–365. [Google Scholar] [CrossRef]
- Busse, A.; Tyson, C.J.; Sandham, N.D.; Lützner, M. DNS of turbulent channel flow over engineering rough surfaces. In Proceedings of the 8th International Symposium on Turbulence and Shear Flow Phenomena, Poitiers, France, 28–30 August 2013. [Google Scholar]
- Burattini, P.; Leonardi, S.; Orlandi, P.; Antonia, R.A. Comparison between experiments and direct numerical simulations in a channel flow with roughness on one wall. J. Fluid Mech. 2008, 600, 403–426. [Google Scholar] [CrossRef]
- Thakkar, M.; Busse, A.; Sandham, N.D. Surface correlations of hydrodynamic drag for transitionally rough engineering surfaces. J. Turbul. 2017, 18, 138–169. [Google Scholar] [CrossRef]
- Thakkar, M.; Busse, A.; Sandham, N.D. Direct numerical simulation of turbulent channel flow over a surrogate for Nikuradse-type roughness. J. Fluid Mech. 2018, 837, R1. [Google Scholar] [CrossRef]
- Busse, A.; Lützner, M.; Sandham, N. Direct numerical simulation of turbulent flow over a rough surface based on a surface scan. Comput. Fluids 2015, 116, 129–147. [Google Scholar] [CrossRef]
- Forooghi, P. Weidenlener, A.; Magagnato, F.; Böhm, B.; Kubach, H.; Koch, T.; Frohnapfel, B. DNS of momentum and heat transfer over rough surfaces based on realistic combustion chamber deposit geometries. Int. J. Heat Fluid Flow 2018, 69, 83–94. [Google Scholar] [CrossRef]
- Flack, K.A.; Schultz, M.P.; Rose, W.B. The onset of roughness effects in the transitionally rough regime. Int. J. Heat Fluid Flow 2012, 35, 160–167. [Google Scholar] [CrossRef]
- Flack, K.A.; Schultz, M.P. Roughness effects on wall-bounded turbulent flows. Phys. Fluids 2014, 26, 101305. [Google Scholar] [CrossRef]
- Flack, K.A.; Schultz, M.P.; Connelly, J.S. Examination of a critical roughness height for outer layer similarity. Phys. Fluids 2007, 19, 095104. [Google Scholar] [CrossRef]
- Schultz, M.P.; Flack, K.A. Reynolds-number scaling of turbulent channel flow. Phys. Fluids 2013, 25, 25104. [Google Scholar] [CrossRef]
- Busse, A.; Sandham, N.D. Parametric forcing approach to rough-wall turbulent channel flow. J. Fluid Mech. 2012, 712, 169–202. [Google Scholar] [CrossRef]
- Forooghi, P.; Frohnapfel, B.; Magagnato, F.; Busse, A. A modified Parametric Forcing Approach for modelling of roughness. Int. J. Heat Fluid Flow 2018, 17, 200–209. [Google Scholar] [CrossRef]
- Jones, D.C., Jr. An improvement in the calculation of turbulent friction in rectangular ducts. J. Fluids Eng. 1976, 98, 173–180. [Google Scholar] [CrossRef]
- EN ISO 5167-1:2003; Measurement of fluid flow by means of pressure differential devices inserted in circular cross-section conduits running full - Part 1: General principles and requirements (ISO 5167-1:2003). German version EN ISO 5167-1:2003; ISO: Geneva, Switzerland, 2003.
- Bell, J.H.; Metha, R.D. Contraction Design for small low-speed wind tunnels. JIAA Tr. 84. 1988. Available online: https://ntrs.nasa.gov/api/citations/19890004382/downloads/19890004382.pdf (accessed on 29 January 2023).
- Taylor, R.P.; Coleman, H.W.; Hodge, B.K. Prediction of turbulent rough-wall skin friction using a discrete element approach. J. Fluids Eng. 1985, 107, 251–257. [Google Scholar] [CrossRef]
- Magagnato, F.; Bühler, S.; Gabi, M. Modeling the wall roughness for RANS and LES using the Discrete Element Method. In Proceedings of the The International Congress of Theoretical and Applied Mechanics (ICTAM); Denier, J., Ed.; International Union of Theoretical and Applied Mechanics: Adelaide, Australia, 2008. [Google Scholar]
- Pritz, B.; Magagnato, F.; Gabi, M. Investigation of the Effect of Surface Roughness on the Pulsating Flow in Combustion Chambers with LES. In Springer Proceedings in Physics; Yoo, S.D., Ed.; Springer: Berlin/Heidelberg, Germany, 2008; pp. 69–76. [Google Scholar]
- Stripf, M.; Schulz, A.; Bauer, H.J. Modeling of Rough-Wall Boundary Layer Transition and Heat Transfer on Turbine Airfoils. J. Turbomach. 2008, 130, 21003. [Google Scholar] [CrossRef]
- Menter, F.R. Best Practice: Scale-Resolving Simulations in ANSYS CFD; ANSYS CFX: Otterfing, Germany, 2015. [Google Scholar]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Fröhlich, J. Large Eddy Simulation turbulenter Strömungen, 1st ed.; Teubner: Wiesbaden, Germany, 2006. [Google Scholar]
- Witte, M.; Torner, B.; Wurm, F.H. Analysis of Unsteady Flow Structures in a Radial Turbomachine by using Proper Orthogonal Decomposition. In Proceedings of the ASME Turbo Expo 2018, Oslo, Norway, 11–15 June 2018; ASME: New York, NY, USA, 2018. [Google Scholar]
- Juckelandt, K. Experimentelle und numerische Untersuchung der Strömung in Pumpen Kleiner Spezifischer Drehzahl unter Berücksichtigung des Rauheitseinflusses. Ph.D. Thesis, Universität Rostock, Rostock, Germany, 2017. [Google Scholar]
- Witte, M.; Bleeck, S.; Benz, C.; Wurm, F.H. Principal experimental study of the acoustic behavior of pumps. In Proceedings of the INTER-NOISE 2016—45th International Congress and Exposition on Noise Control Engineering: Towards a Quieter Future, Hamburg, Germany, 21–24 August 2016; pp. 7666–7674. [Google Scholar]
- Smirnov, P.E.; Menter, F.R. Sensitization of the SST Turbulence Model to Rotation and Curvature by Applying the Spalart–Shur Correction Term. J. Turbomach. 2009, 131, 041010. [Google Scholar] [CrossRef]
- Juckelandt, K.; Wurm, F.H. Applicability of Wall-Function Approach in Simulations of Turbomachines. In ASME Turbo Expo 2015; ASME: New York, NY, USA, 2015; pp. 1–10. [Google Scholar]
- DIN EN ISO 9906; Rotodynamic Pumps—Hydraulic Performance Acceptance Tests—Grades 1, 2 and 3 (ISO 9906:2012). German version EN ISO 9906:2012; ISO: Geneva, Switzerland, 2012.
- Nikuradse, J. Strömungsgesetze in rauhen Rohren. VDI-Forschungsheft 1933, 4. Available online: https://www.scirp.org/(S(351jmbntvnsjt1aadkozje))/reference/referencespapers.aspx?referenceid=2054940 (accessed on 18 October 2022).








| Reference | |
|---|---|
| Wagner [13] | 200–600 |
| Fried and Idelchick [14] | 250–1000 |
| Oertel et al. [15] | 200–3000 |
| Gülich [16] | 300–1000 |
| White [17] | |
| Schlichting and Gersten [3] | 250 |
| Roughness | ||||||
|---|---|---|---|---|---|---|
| Scan | 190 | 280 | 20.0 | 25.5 | −0.12 | 0.24 |
| Replica | 186 | 270 | 20.3 | 26.1 | −0.22 | 0.16 |
| Grid Parameter | Cast Iron | ||
|---|---|---|---|
| Grid ‘Coarse’ | Grid ‘Middle’ | Grid ‘Fine’ | |
| Grid size in M elements | 0.3 | 1.2 | 4.8 |
| Grid distribution in | |||
| max. aspect ratio | 13.9 | 7.0 | 4.1 |
| max. volume change | 1.2 | 1.2 | 1.2 |
| grid angle | 90 | 90 | 90 |
| Cast Iron | |||
|---|---|---|---|
| Grid ‘Coarse’ | Grid ‘Middle’ | Grid ‘Fine’ | |
| 75,000 | − | ✓ | − |
| 100,000 | ✓ | ✓ | ✓ |
| 150,000 | − | ✓ | − |
| 200,000 | − | ✓ | − |
| 250,000 | ✓ | ✓ | ✓ |
| 300,000 | ✓ | ✓ | ✓ |
| 350,000 | ✓ | ✓ | ✓ |
| 400,000 | ✓ | ✓ | ✓ |
| Re | Cast Iron |
|---|---|
| Grid ‘Middle’ | |
| 250,000 | μm |
| 300,000 | μm |
| 350,000 | μm |
| 400,000 | μm |
| average | μm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY-NC-ND) license (https://creativecommons.org/licenses/by-nc-nd/4.0/).
Share and Cite
Torner, B.; Duong, D.V.; Wurm, F.-H. Numerical Determination of the Equivalent Sand Roughness of a Turbopump’s Surface and Its Roughness Influence on the Pump Characteristics. Int. J. Turbomach. Propuls. Power 2023, 8, 5. https://doi.org/10.3390/ijtpp8010005
Torner B, Duong DV, Wurm F-H. Numerical Determination of the Equivalent Sand Roughness of a Turbopump’s Surface and Its Roughness Influence on the Pump Characteristics. International Journal of Turbomachinery, Propulsion and Power. 2023; 8(1):5. https://doi.org/10.3390/ijtpp8010005
Chicago/Turabian StyleTorner, Benjamin, Duc Viet Duong, and Frank-Hendrik Wurm. 2023. "Numerical Determination of the Equivalent Sand Roughness of a Turbopump’s Surface and Its Roughness Influence on the Pump Characteristics" International Journal of Turbomachinery, Propulsion and Power 8, no. 1: 5. https://doi.org/10.3390/ijtpp8010005
APA StyleTorner, B., Duong, D. V., & Wurm, F.-H. (2023). Numerical Determination of the Equivalent Sand Roughness of a Turbopump’s Surface and Its Roughness Influence on the Pump Characteristics. International Journal of Turbomachinery, Propulsion and Power, 8(1), 5. https://doi.org/10.3390/ijtpp8010005





