Impact of Urban Morphology on Energy Consumption of Vertical Mobility in Asian Cities—A Comparative Analysis with 3D City Models
Abstract
:1. Introduction
1.1. Literature on Urban form and Energy Use
1.2. Literature on Vertical Mobility and Energy Consumption
1.3. Research Gaps
- The studies were specific to particular building types and countries. They did not perform at the urban blocks or city scales, rather on a building-by-building basis.
- Investigation on the relationship between urban form and vertical mobility was not performed.
- The 3D city models illustrate detailed building geometry and form, which help to develop a more realistic model. None of the studies performed analyses based on the 3D city models.
- The methods were mostly based on real consumption, so generic model-based calculation was not possible.
- Some studies were carried out without any reference to national or international standards.
- In most cases, the validation of the modelling results was not performed.
- Some studies did not calculate energy consumption during the standby mode. Earlier research revealed that the standby model consumes a significant percentage of the total energy consumption. For example, Hui et al. [24] collected lift energy consumption data of 21 lift units and found out that the standby consumption represented around 10.4% to 98.5% of the total lift electricity consumption.
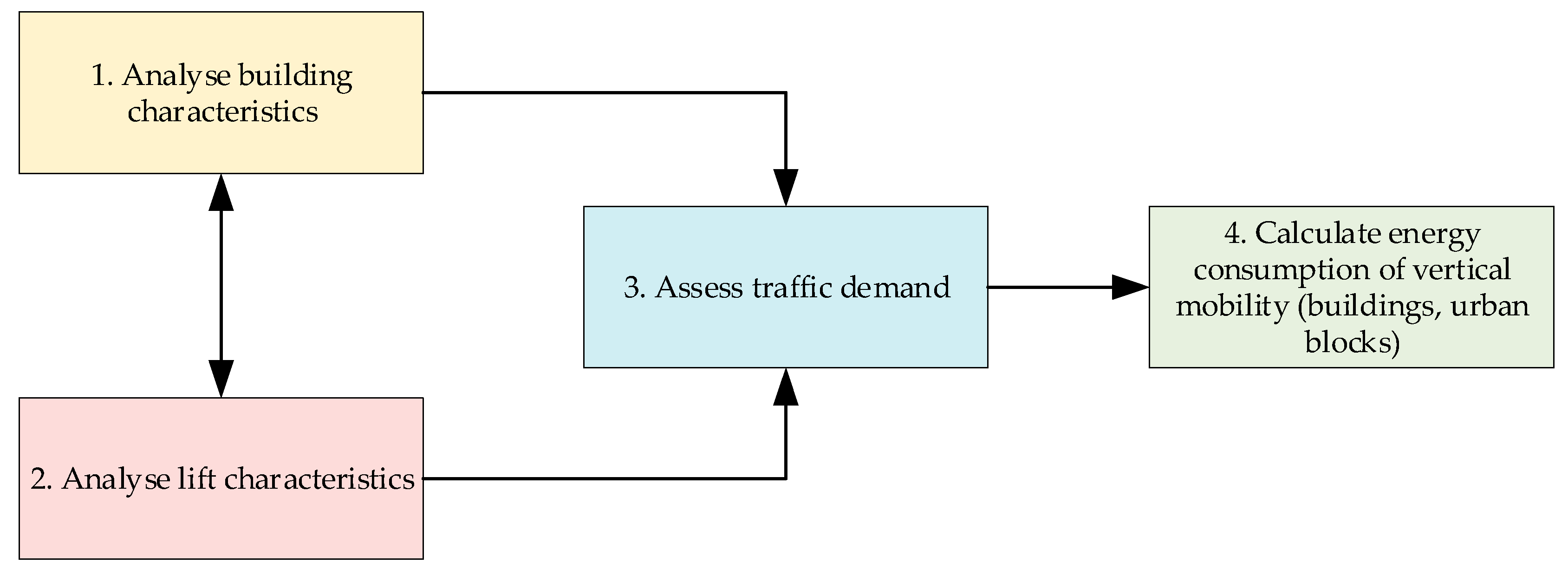
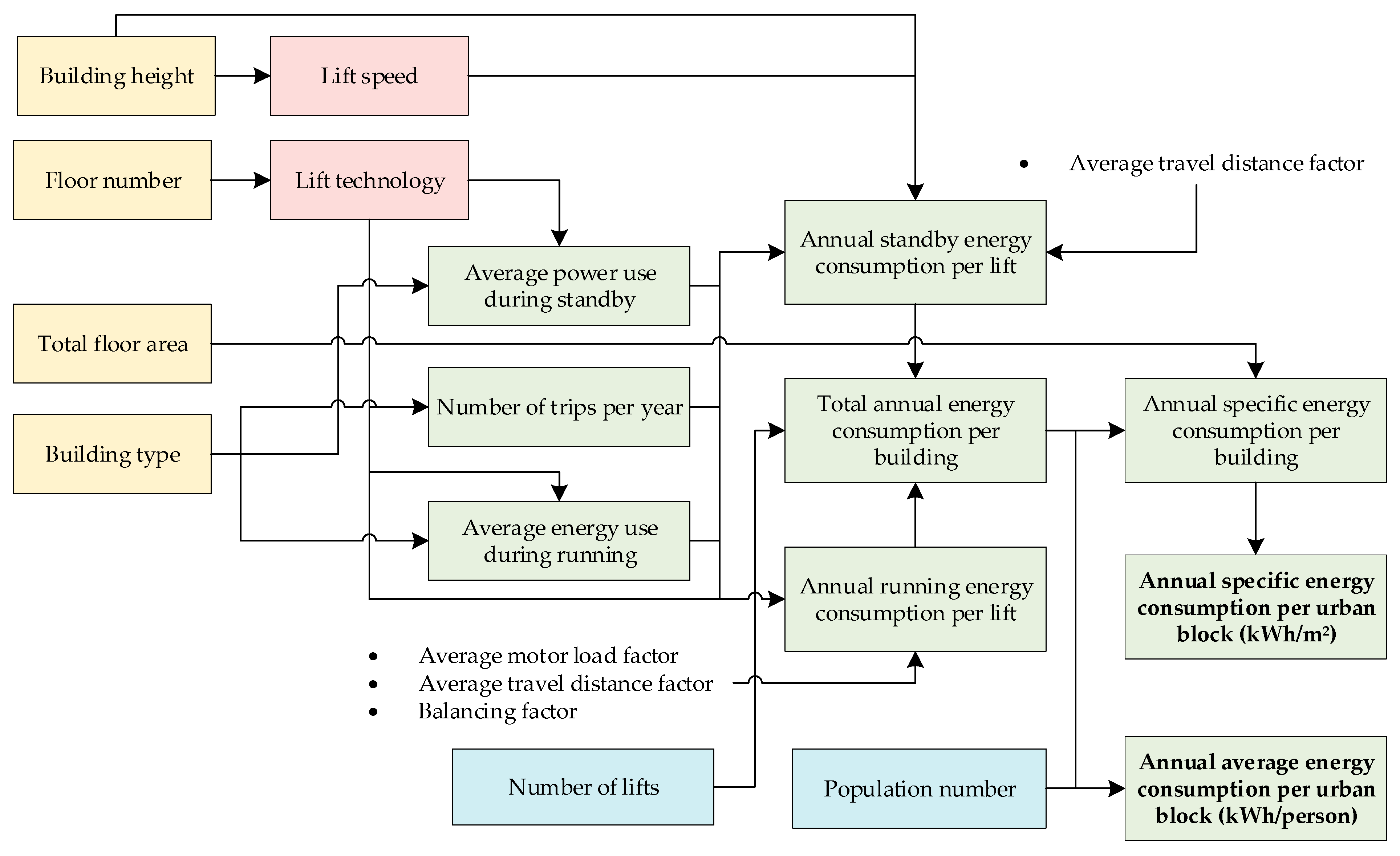
2. Description of Methodology
2.1. Building Characteristics
2.2. Lift Characteristics
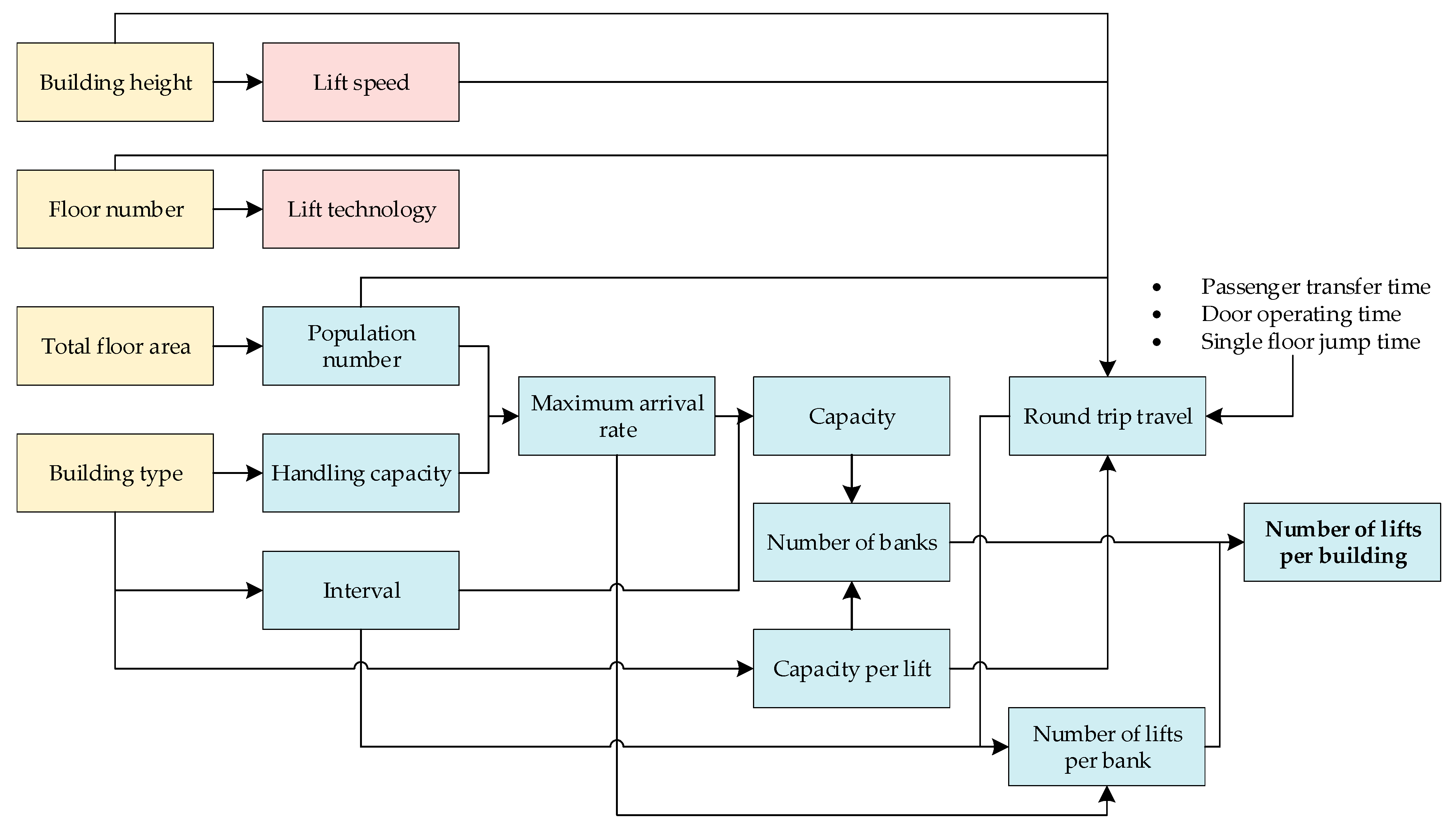
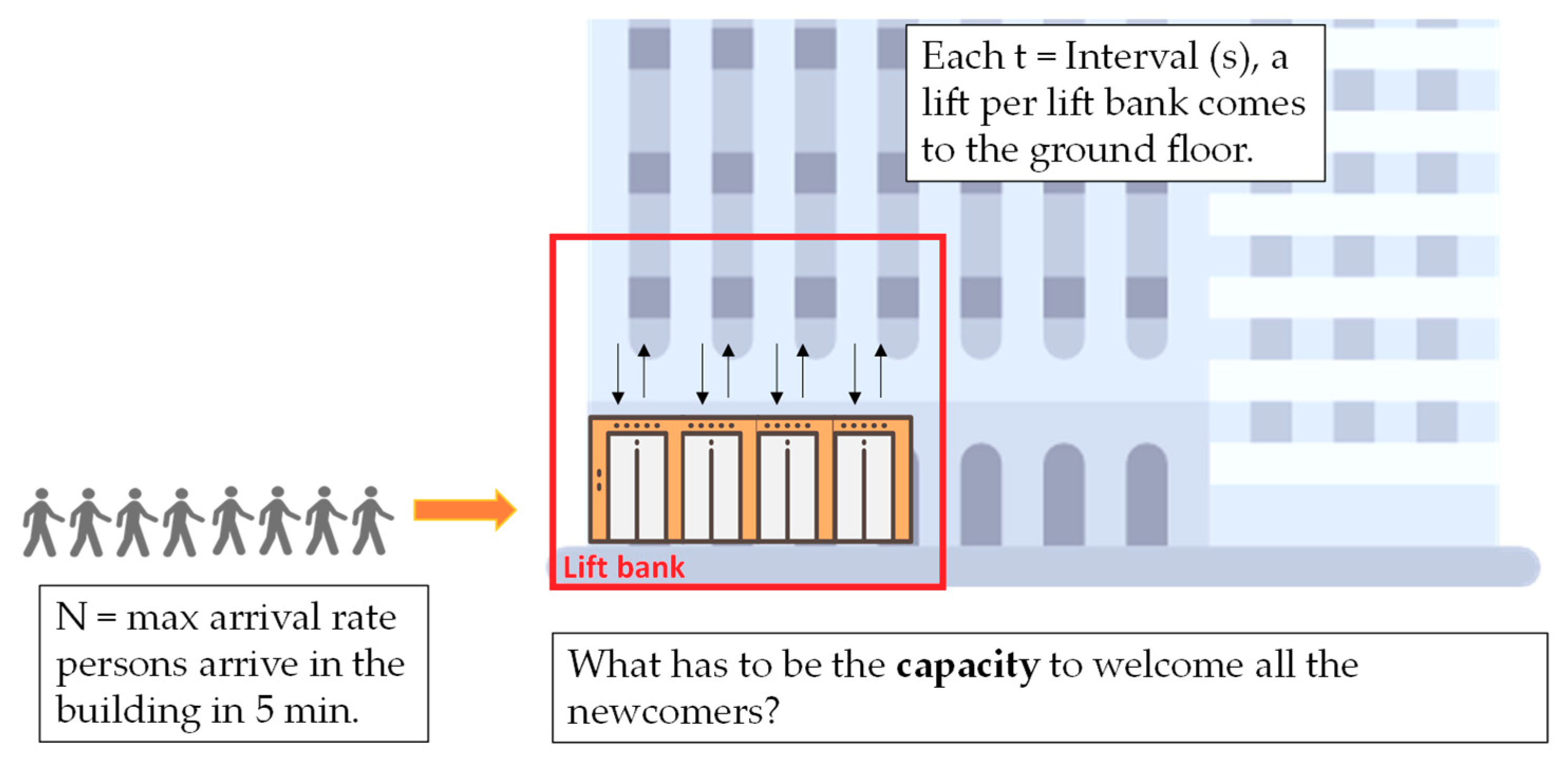
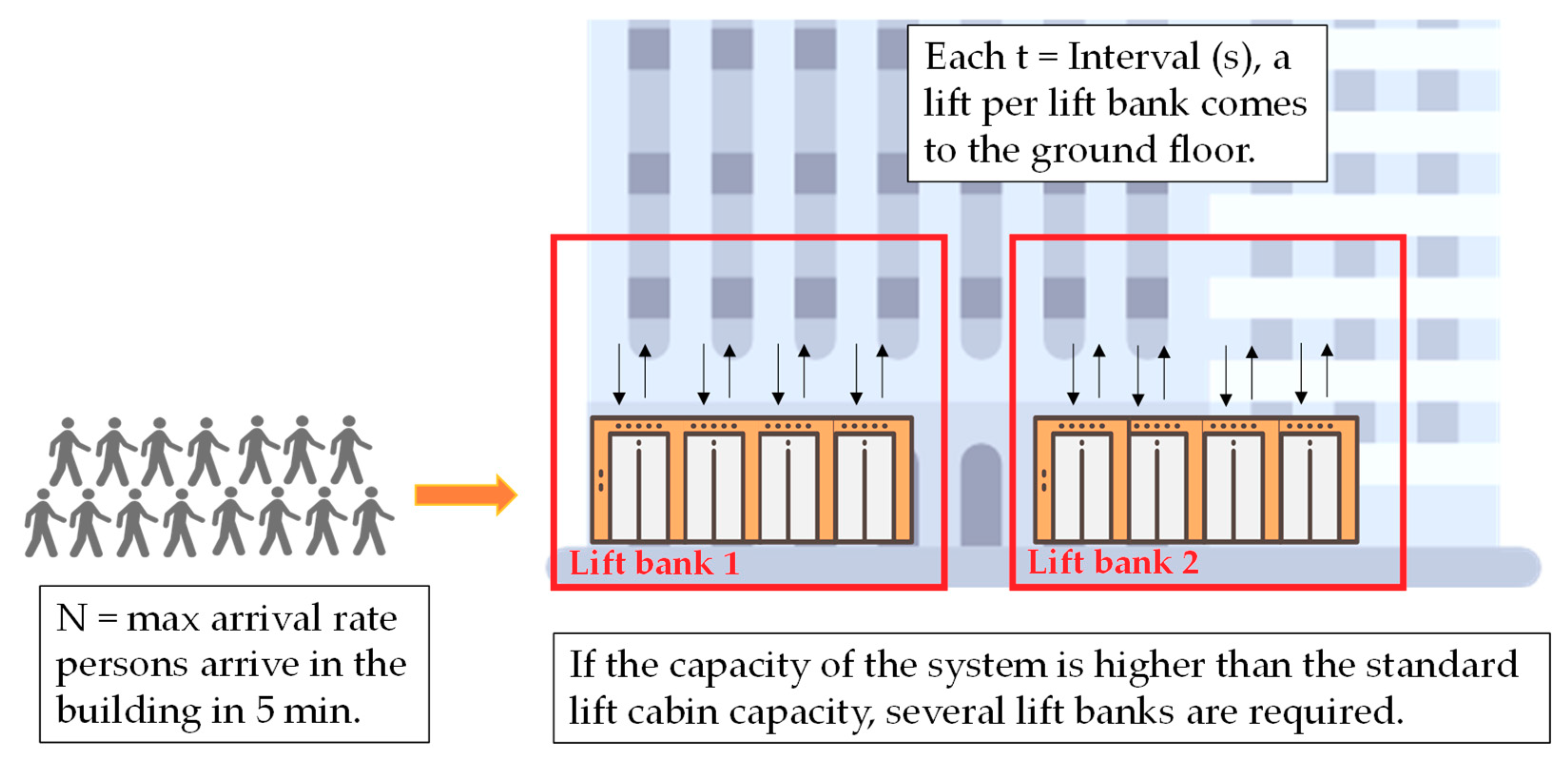
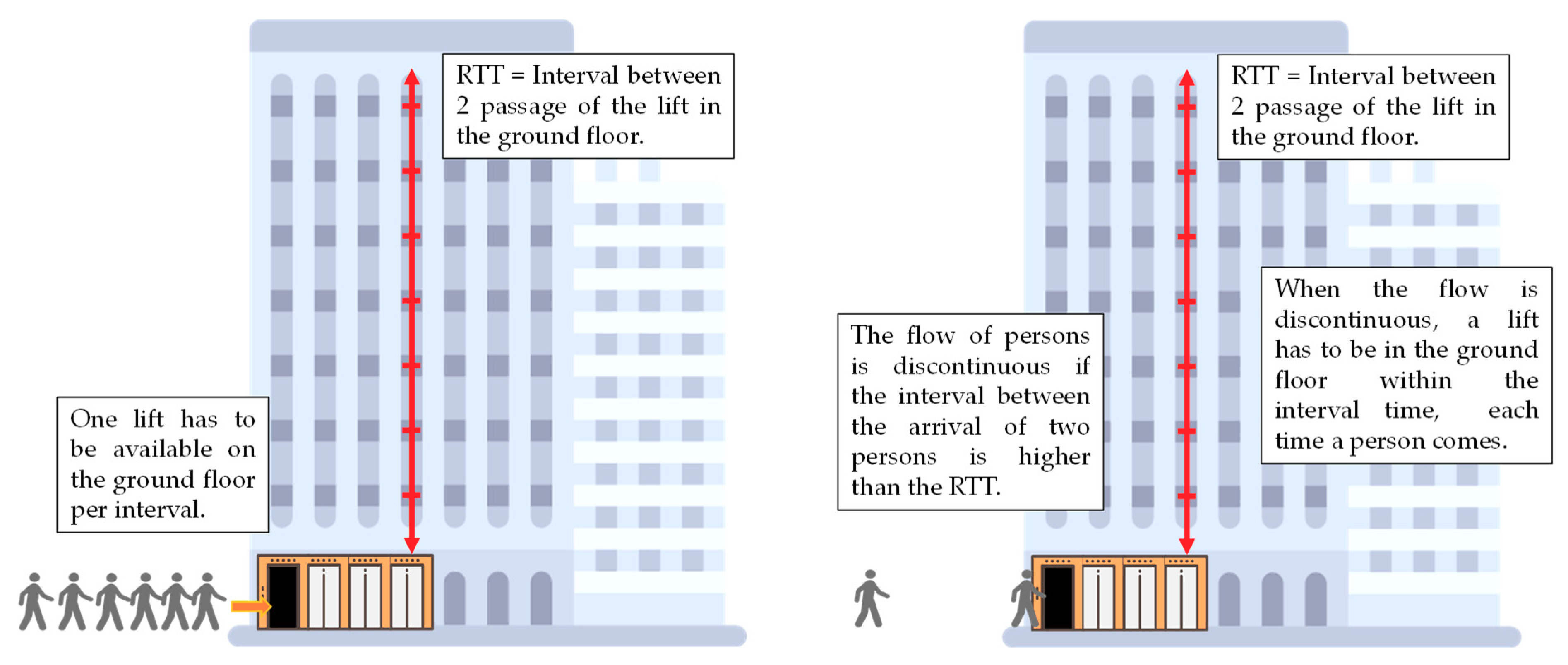
2.3. Traffic Demand Analysis
- highest reversal floor, approximated by the top floor [23], (p. 25)
- : interfloor/single floor jump time (in s) is the time required to travel between two adjacent floors. It is calculated by dividing the height of one floor (by diving the height of the building with the number of floors) with the speed of the lift [17], (p. 57)
- number of stops of the lift car during its round trip [23], (p. 25)
- : operating time is 3 s.
- number of passengers in the lift car [21], (p. 25)
- : passenger transfer time (per passenger) is 1.2 s.
2.4. Energy Consumption Calculation
2.4.1. Energy Consumption during Running Mode
- : average motor load factor is the ratio between the average motor load and the maximum possible motor load. It depends of the lift technology [19], p. 64
- : average travel distance factor depends on how often the lift stops during a trip between terminal floors, and the number of lifts in the building. Lifts are considered to have more than two stops [19], (p. 65)
- balancing factor considers the defect of the counterweight, when the technology used for the lift is traction [19], (p. 65)
- : number of trips per year [19], (p. 65)
2.4.2. Energy Consumption during Standby Mode
- : average travel distance factor depends on how often the lift stops during a trip between terminal floors, and the number of lifts in the building. Lifts are considered to have more than two stops [19], (p. 65)
- h: height of the building
- : number of trips of the lift per year [19], (p. 57)
- : speed of the lift [19], (p. 57)
- : power used by the lift when it is on “standby” mode [19], (p. 57)
2.4.3. Total Energy Consumption
3. Implementation of Method
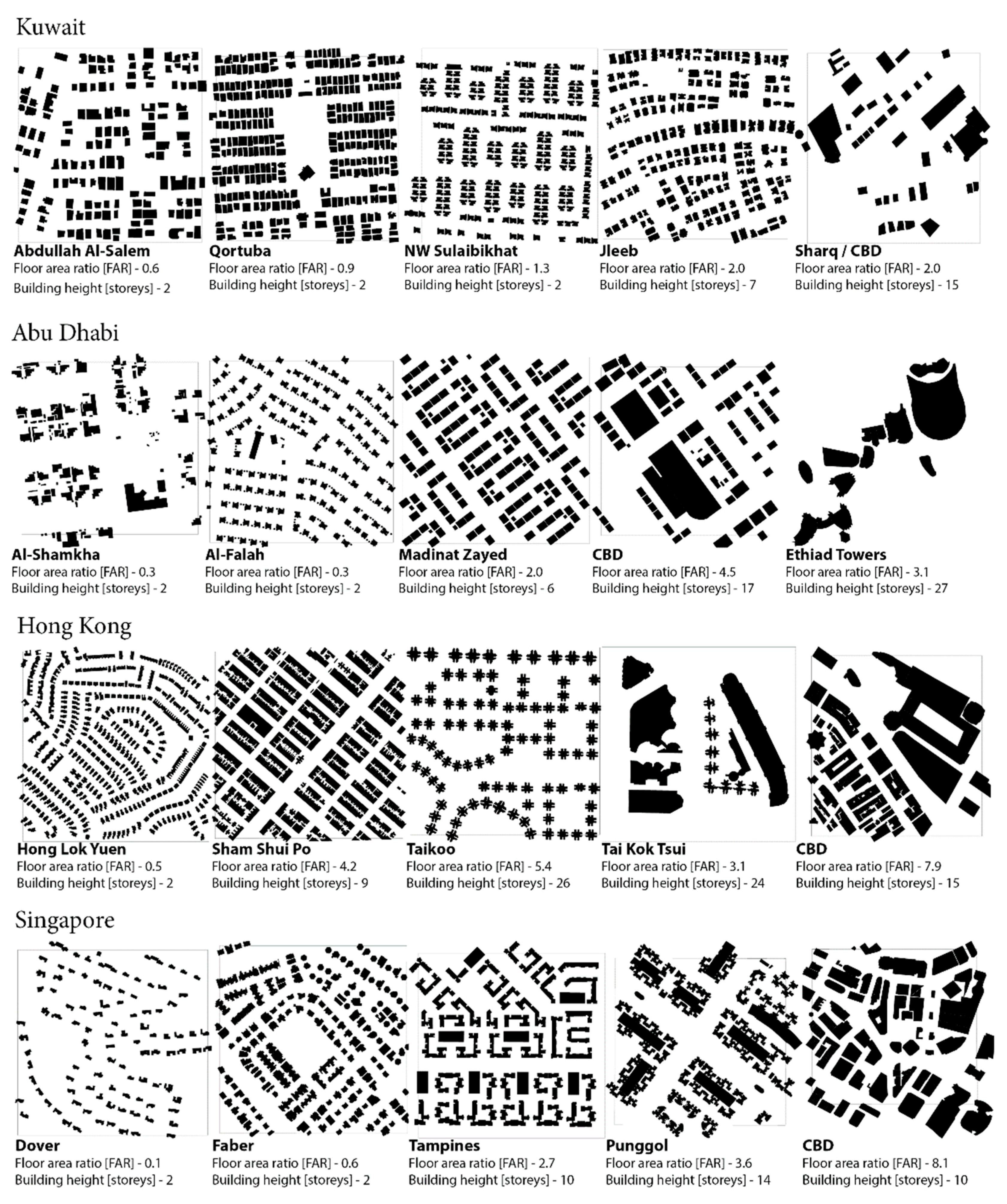
3.1. Selected Urban Morphologies
3.2. Required Data
3.3. Assumptions
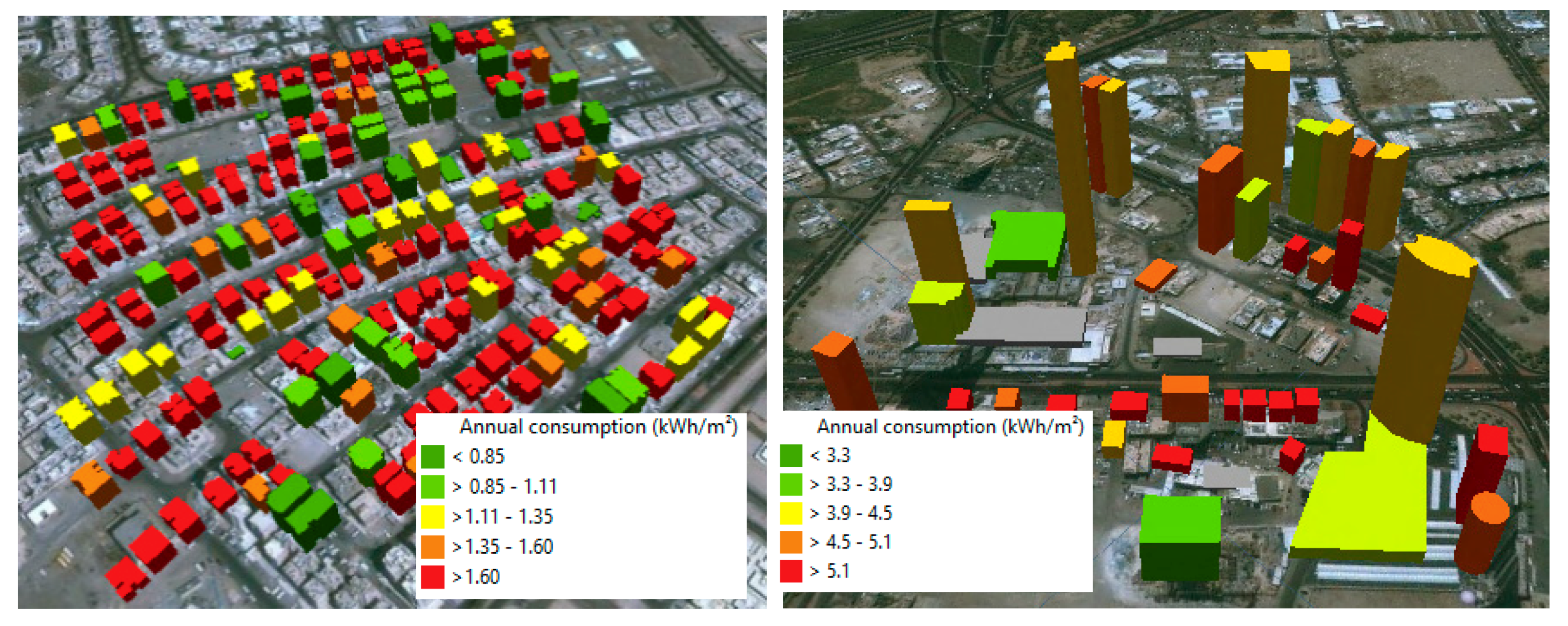
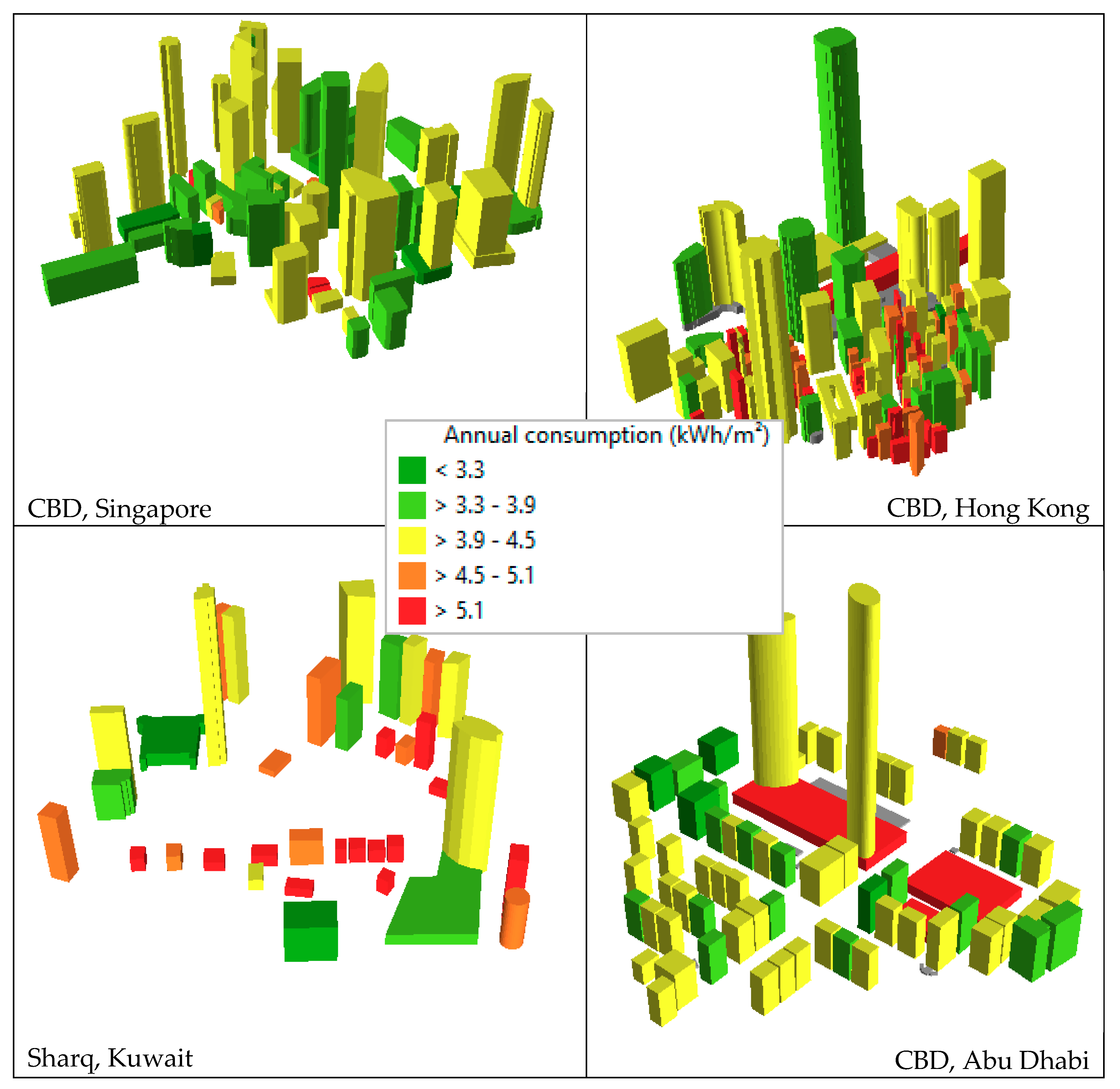
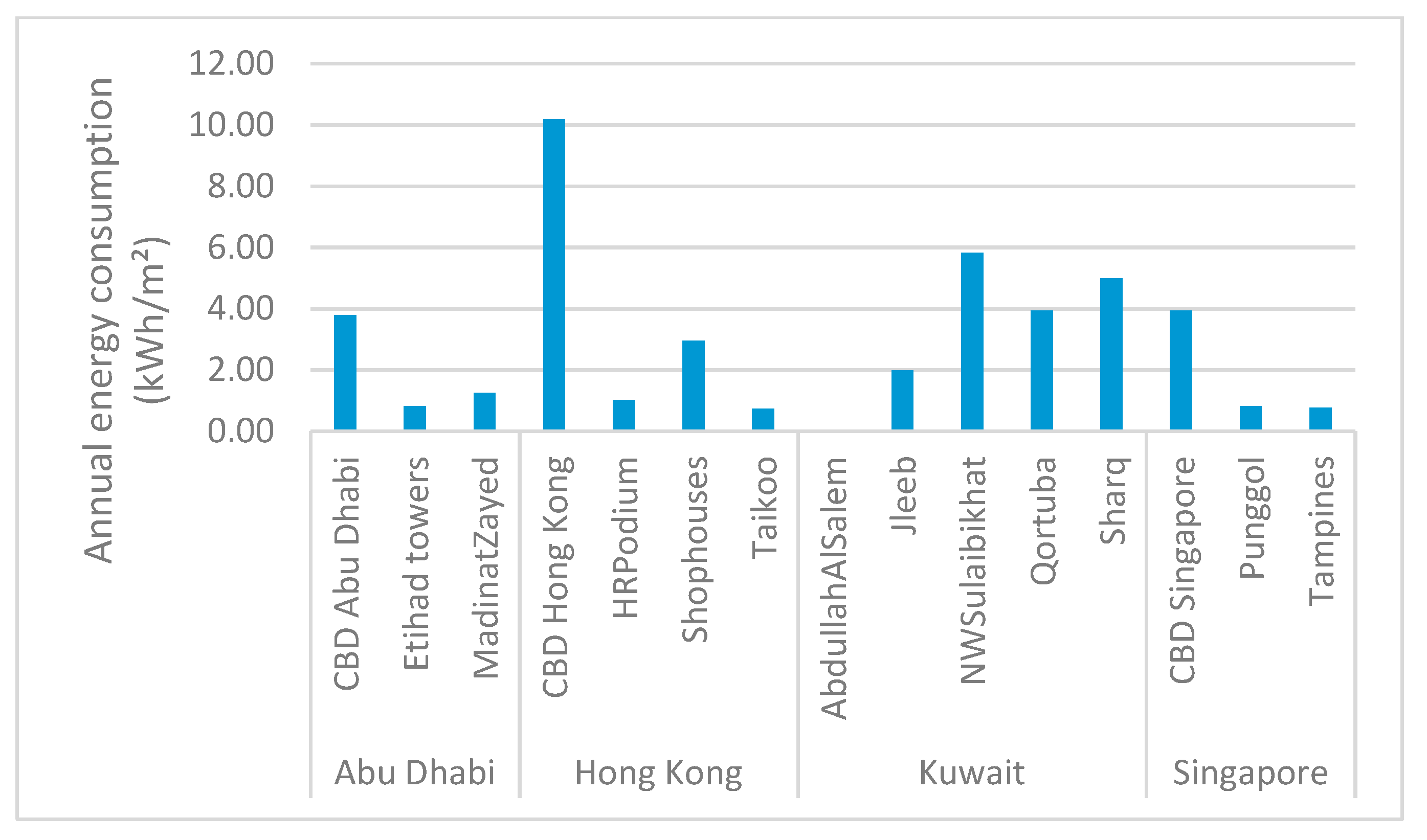
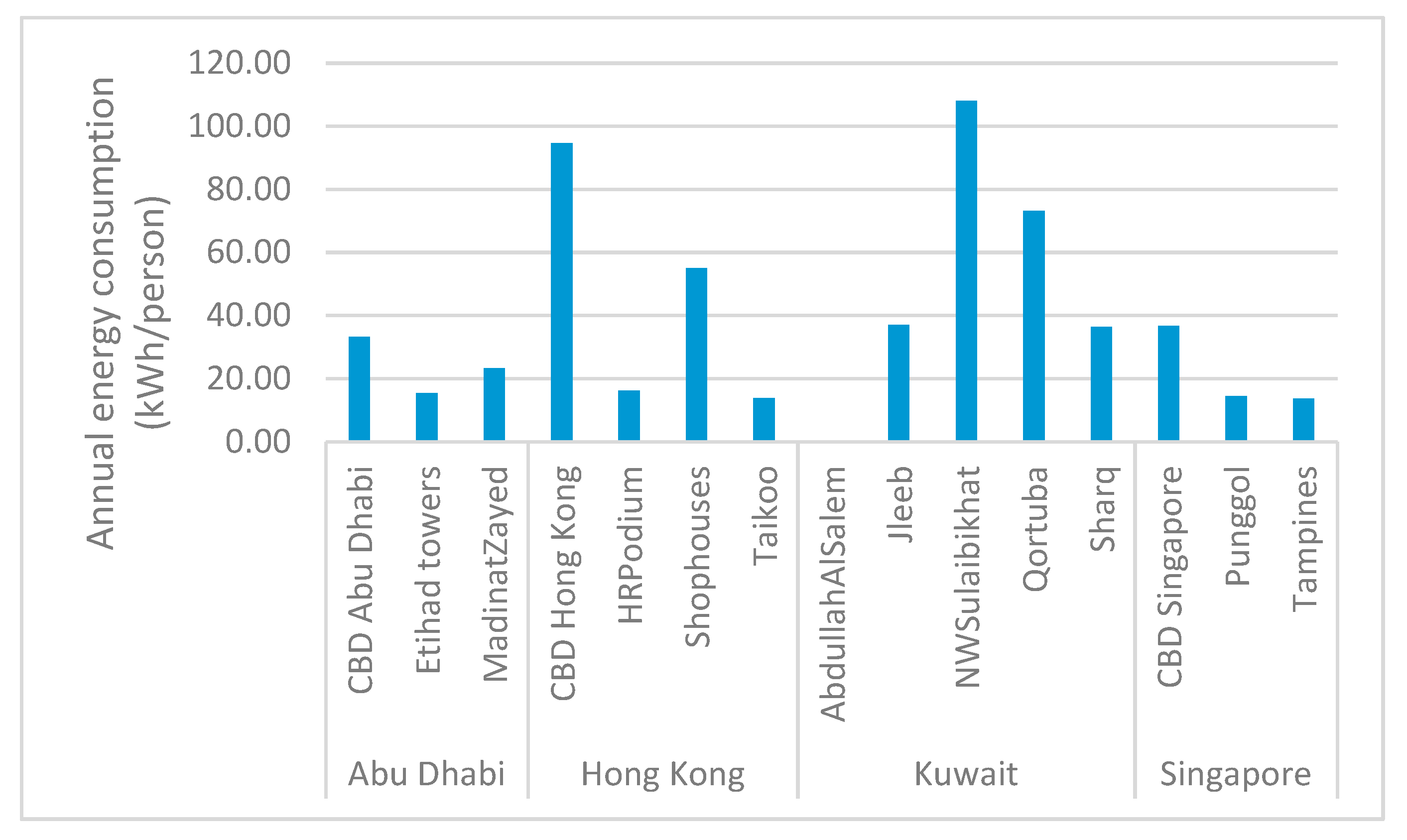
4. Discussion and Visualisation of Results
4.1. Evaluation in Each City and Morphology
4.1.1. Kuwait
4.1.2. Singapore
4.1.3. Hong Kong
4.1.4. Abu Dhabi
4.2. Comparative Analyses
5. Reliability of Data and Model Validation
5.1. Reliability of Data
5.2. Validation of the Results
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
| Building Typology | Lift Technology | Average Energy per Cycle (Wh) | Power Used during Standby Mode (W) | Number of Trips per Year | Lift Capacity (per pers) | Occupant Density (gross area/pers) | Handling Capacity (Average) | Interval (Average) (s) |
|---|---|---|---|---|---|---|---|---|
| Residential | Geared Traction | 50.4 | 163.8 | 62,300 | 4 | 18.58 | 6.25% | 65 |
| Residential | Gearless Traction | 33.07 | 249 | 131,000 | 6 | 18.58 | 6.25% | 65 |
| Residential | Hydraulic | 63.8 | 180.4 | 44,900 | 5 | 18.58 | 6.25% | 65 |
| Office | Geared Traction | 57.9 | 244.8 | 232,000 | 7 | 9.29 | 13.50% | 28.5 |
| Office | Gearless Traction | 43.9 | 320.7 | 242,000 | 8 | 9.29 | 13.50% | 28.5 |
| Office | Hydraulic | 83.1 | 182.9 | 164,000 | 7 | 9.29 | 13.50% | 28.5 |
| Hotel | Geared Traction | 77.7 | 198.5 | 199,000 | 9 | 4.645 | 14.00% | 32.5 |
| Hotel | Gearless Traction | 44.13 | 204.3 | 220,000 | 12 | 4.645 | 14.00% | 32.5 |
| Hotel | Hydraulic | 107.6 | 113.6 | 86,000 | 11 | 4.645 | 14.00% | 32.5 |
| Commercial | Geared Traction | 42.5 | 213.4 | 192,000 | 10 | 3.716 | 12.00% | 25 |
| Commercial | Gearless Traction | 24 | 235.1 | 224,000 | 10 | 3.716 | 12.00% | 25 |
| Commercial | Hydraulic | 92.9 | 177.3 | 142,000 | 10 | 3.716 | 12.00% | 25 |
| Car park | Geared Traction | 52.5 | 100 | 60,000 | 4 | 10 | 6.25% | 65.0 |
| Car park | Gearless Traction | 52.5 | 100 | 60,000 | 6 | 10 | 6.25% | 65.0 |
| Car park | Hydraulic | 52.5 | 100 | 60,000 | 5 | 10 | 6.25% | 65.0 |
References
- UN. The World’s Cities in 2016—Data Booklet (ST/ESA/ SER.A/392); United Nations, Department of Economic and Social Affairs, Population Division: New York, NY, USA, 2016. [Google Scholar]
- Zhao, J. Analysis of Urban Form Parameters with a Focus on Energy Consumption. Ph.D. Thesis, Technische Universität Dortmund, Dortmund, Germany, 2016. [Google Scholar]
- Ko, Y. Urban form and residential energy use: A review of design principles and research findings. J. Plan. Lit. 2013, 28, 327–351. [Google Scholar] [CrossRef]
- Bian, C.; Lin, L.; Zhang, Q. Effects of China’s Urban Form on Urban Energy Consumption. In Proceedings of the 40th Annual IAEE International Conference, Singapore, 18–21 June 2017; p. 9. [Google Scholar]
- Rode, P.; Keim, C.; Robazza, G.; Viejo, P.; Schofield, J. Cities and energy: Urban morphology and residential heat-energy demand. Environ. Plan. B Plan. Des. 2014, 41, 138–162. [Google Scholar] [CrossRef]
- Rode, P.; Burdett, R.; Robazza, G.; Schofield, J.; Keim, C.; Avci, N.; Dimitrova, S.; Garcia, P.V.; Murshed, S.M.; Bahu, J.-M. Cities and Energy: Urban Morphology and Heat Energy Demand; Final Report; LSE Cities, The London School of Economics and Political Science: London, UK, February 2014; p. 232. [Google Scholar]
- Mindali, O.; Raveh, A.; Salomon, I. Urban density and energy consumption: A new look at old statistics. Transp. Res. Part A Policy Pract. 2004, 38, 143–162. [Google Scholar] [CrossRef]
- Maharjan, S.; Tsurusaki, N.; Divigalpitiya, P. Influencing Mechanism Analysis of Urban Form on Travel Energy Consumption—Evidence from Fukuoka City, Japan. Urban Sci. 2018, 2, 15. [Google Scholar] [CrossRef]
- Liu, C.; Shen, Q. An empirical analysis of the influence of urban form on household travel and energy consumption. Comput. Environ. Urban Syst. 2011, 35, 347–357. [Google Scholar] [CrossRef]
- Liu, C. Exploring the Influence of Urban form on Travel and Energy Consumption, Using Structural Equation Modeling. Ph.D. Thesis, University of Maryland, College Park, MD, USA, 2012. [Google Scholar]
- Osorio, B.; McCullen, N.; Walker, I.; Coley, D. Understanding the relationship between energy consumption and urban form. Athens J. Sci. 2016, 4, 115–141. [Google Scholar] [CrossRef]
- Yin, Y.; Mizokami, S.; Maruyama, T. An analysis of the influence of urban form on energy consumption by individual consumption behaviors from a microeconomic viewpoint. Energy Policy 2013, 61, 909–919. [Google Scholar] [CrossRef]
- Adak, M.F.; Duru, N.; Duru, H.T. Elevator simulator design and estimating energy consumption of an elevator system. Energy Build. 2013, 65, 272–280. [Google Scholar] [CrossRef]
- Al-Sharif, L. Lift energy consumption: General overview (1974–2001). Elevator World 2004, 52, 61–67. [Google Scholar]
- Ahmed, S.S.; Iqbal, A.; Sarwar, R.; Salam, M.S. Modeling the energy consumption of a lift. Energy Build. 2014, 71, 61–67. [Google Scholar] [CrossRef]
- Barney, G. Energy Models for Lifts. In Proceedings of the 1st Symposium of Lift and Escalator Technologies, Northampton, UK, 29 September 2011; pp. 22–31. [Google Scholar]
- Nipkow, J.; Schalcher, M. Energy Consumption and Efficiency Potentials of Lifts; Swiss Agency for Efficient Energy use S.A.F.E.: Zurich, Switzerland; HTW Chur University of Applied Sciences: Chur, Switzerland, 2006; p. 3. [Google Scholar]
- Tukia, T. Determining and Modeling the Energy Consumption of Elevators. Master’s Thesis, Aalto University School of Electrical Engineering: Espoo, Finland, 2014. [Google Scholar]
- De Almeida, A.T.; Patrão, C.; Fong, J.; Araújo, R.; Nunes, U.; Rivet, L.; Lindegger, U.; Nanetti, M.; Cariani, W.; Disi, A.; et al. E4 Project Energy Efficient Elevators and Escalators; ISR—University of Coimbra: Coimbra, Portugal, March 2010; p. 122. [Google Scholar]
- Tukia, T.; Uimonen, S.; Siikonen, M.-L.; Hakala, H.; Donghi, C.; Lehtonen, M. Explicit method to predict annual elevator energy consumption in recurring passenger traffic conditions. J. Build. Eng. 2016, 8, 179–188. [Google Scholar] [CrossRef]
- Bannister, P.B.; Bloomfield, C.; Chen, H. Empirical Prediction of Office Building Lift Energy Consumption. In Proceedings of the Building Simulation 2011: 12th Conference of International Building Performance Simulation Association, Sydney, Australia, 14–16 November 2011; pp. 2635–2642. [Google Scholar]
- Energy Calculator. thyssenkrupp Elevator Corporation © 2018, United States. Available online: https://www.thyssenkruppelevator.com/Tools/energy-calculator (accessed on 30 July 2018).
- EMSD. Guidelines on Energy Efficiency of Lift & Escalator Installations; Electrical and Mechanical Services Department: Hong Kong, China, 2007; p. 31.
- Hui, C.M.; Yeung, C.Y. Analysis of standby power consumption for lifts and escalators. In Proceedings of the 7th Greater Pearl River Delta Conference on Building Operation and Maintenance, Hong Kong, China, 6 December 2016. [Google Scholar]
- EMSD. Code of Practice for Energy efficiency of Lift and Escalators Installations; Electrical and Mechanical Services Department: Hong Kong, China, 2007.
- Energy Performance Of Lifts And Escalators—Part 1: Energy Measurement And Conformance; ISO/DIS 25745-1; International Organization for Standardization: Geneva, Switzerland, 2008.
- Siikonen, M.-L. On traffic planning methodology. Elevator Technol. 2000, 27, 267–274. [Google Scholar]
- Peters, R.D. Improvement to the Up Peak Round Trip Time Calculation. Int. J. Elevator Eng. 2000, 3. [Google Scholar]
- ISO. Energy performance of lifts, escalators and moving walks—Part 2: Energy calculation and classification for lifts (elevators). In ISO 25745-2:2012; International Organization for Standardization: Geneva, Switzerland, 2012. [Google Scholar]
- Rode, P.; Gomes, A.; Adeel, M.; Sajjad, F.; McArthur, J.; Alshalfan, S.; Schwinger, P.; Montagne, C.; Tunas, D.; Lange, C.; et al. Resource Urbanisms: Asia’s Divergent City Models of Kuwait, Abu Dhabi, Singapore and Hong Kong; LSE Cities, School of Economics and Political Science: London, UK, 2017; p. 71. [Google Scholar]
- Wendel, J.; Simons, A.; Nichersu, A.; Murshed, S.M. Rapid development of semantic 3D city models for urban energy analysis based on free and open data sources and software. In Proceedings of the 3rd ACM SIGSPATIAL Workshop on Smart Cities and Urban Analytics, Redondo Beach, CA, USA, 7 November 2017; p. 7. [Google Scholar]
- Murshed, S.M.; Al-Hyari, A.; Wendel, J.; Ansart, L. Design and Implementation of a 4D Web Application for Analytical Visualization of Smart City Applications. ISPRS Int. J. Geo-Inf. 2018, 7, 276. [Google Scholar] [CrossRef]
- Murshed, S.M.; Lindsay, A.; Picard, S.; Simons, A. PLANTING: Computing High Spatio-Temporal Resolutions of Photovoltaic Potential of 3D City Models. In Geospatial Technologies for All, Lecture Notes in Geoinformation and Cartography; Mansourian, A., Pilesjö, P., Harrie, L., van Lammere, R., Eds.; Springer International Publishing AG: Cham, Switzerland, 2018; in press. [Google Scholar]
- Murshed, S.M.; Picard, S.; Koch, A. Modelling, Validation and Quantification of Climate and Other Sensitivities of Building Energy Model on 3D City Models. ISPRS Int. J. Geo-Inf. 2018, 7, 447. [Google Scholar] [CrossRef]
- International Code Council. International Code Council. International Building Code 2015 (IBC 2015). In Chapter 3 Use and Occupancy Classification; International Code Council, Inc.: Washington, DC, USA, 2015. [Google Scholar]
- Djuric, N.; Novakovic, V.; Frydenlund, F. Electricity use in two low energy office buildings in Norway. REHVA Eur. HVAC J. 2012, 49, 30–34. [Google Scholar]
- Lobato, C.; Pless, S.; Sheppy, M.; Torcellini, P. Reducing plug and process loads for a large scale, low energy office building: Nrel’s research support facility. In Proceedings of the ASHRAE Winter Conference, Las Vegas, NV, USA, 29 January–2 February 2011; pp. 1–2. [Google Scholar]
- Wang, X.; Huang, C.; Cao, W. Energy audit of building: A case study of a commercial building in Shanghai. In Proceedings of the Power and Energy Engineering Conference (APPEEC), Chengdu, China, 28–31 March 2010; pp. 1–4. [Google Scholar]
- Schroeder, J. Energy Consumption and Power Requirements of Elevators; Springer: Boston, MA, USA, 1988. [Google Scholar]
- Venables, J.; Monaghan, E. Smarter Buildings: Real-World Energy Use of Lifts/Elevators in Contemporary Office Buildings and Its Mitigation through Stair-Use Promotion; StepJockey and SVM Associates: London/Birmingham, UK, May 2016; p. 36. [Google Scholar]
- Al-Sharif, L. Lift and Excalator Energy Consumption. In Proceedings of the CIBSE/ASHRAE Joint National Conference, Harrogate, UK, 29 September–1 October 1996; pp. 231–239. [Google Scholar]
- Koch, A.; Zech, D. Impact analysis of heat consumption: User behaviour and the consumption of heat energy. In The Nature of Sustainable Consumption and How to Achieve It: Results from the Focal Topic “From Knowledge to Action–New Paths Towards Sustainable Consumption; Defila, R., Giulio, A.D., Kaufmann-Hayoz, R., Eds.; Oekom Verlag: München, Germany, 2012; pp. 353–365. [Google Scholar]

| Category | Variable | Source | Use of Data | Use of Method |
|---|---|---|---|---|
| Building characteristics | Floor area | Database | x | |
| Building type | Database | x | ||
| Number of floors | Database | x | ||
| Height of the building | Database | x | ||
| Lift characteristics | Lift technology | [19], p. 57 | x | |
| Speed | [23], p. 16 | x | ||
| Number of trips per year | [19], p. 57 | x | ||
| Traffic analysis | Population | [35], Table 1004.1.1 | x | |
| Handling capacity | [27], Table 1 | x | ||
| Maximum arrival rate | [23], p. 25 | x | ||
| Interval | [27], Table 1 | x | ||
| Capacity | [23], p. 25 | x | ||
| Capacity per lift | [19], p. 57 | x | ||
| Number of lift banks | [23], p. 25 | x | ||
| Round trip travel | [23], p. 25 | x | ||
| Number of lift car per lift bank | [23], p.25 | x | ||
| Number of lift | [23,25] | x | ||
| Energy consumption | Average energy use during running mode per cycle | [19], p. 78 | x | x |
| Annual running energy consumption per lift | [19,26] | x | ||
| Power use during standby mode | [19], p. 79 | x | x | |
| Annual energy for standby mode per lift | [19,26] | x |
| Variable | Reliability | Comments/to Improve |
|---|---|---|
| Floor Area | High | Calculated from building footprints. |
| Building Type | Medium | If building types are missing in database, assumptions are made. |
| Number of floors | High | Field survey through local experts. |
| Height of the building | High | Field survey through local experts. |
| Population | Medium | Calculated according to building type and floor area. |
| Handling capacity | Medium | Literature review. |
| Maximum arrival rate | Medium | Literature review. |
| Interval | Medium | Literature review. |
| Capacity | Medium | Literature review. |
| Number of lifts | Medium | Literature review. |
| Lift technology | Medium | Choice between hydraulic and geared lifts are made after literature. |
| Speed | Medium | Speed is defined based on the height of the building, and not the building category and the lift technology used (except for hydraulic lifts). |
| Capacity per lift, Number of trips per year, Average energy use per cycle, Power use during stand-by mode | Medium | Based on measurements on 87 lifts in Italy, Germany, Poland and Portugal [19]. We assume the same values for Asian cities. |
| References | Type of Data | Type of Building | Lift Information | Energy Consumption Value |
|---|---|---|---|---|
| [36] | Measured | Low energy office building in Trondheim (Norway), with 16,200 m2 floor area | 0.3 kWh/m2a | |
| [38] | Measured | Energy Audit of a commercial building in Shanghai (China), 26,000 m2 floor area | 4 lifts, power requirement of 22 kW and 15 kWh | 19 kWh/m2a |
| [39] | Requirements for high-rise building in 1988 | Office building | 4 kWh/m2a | |
| [41] | Value taken for calculation in an example | Lift and escalator energy consumption for office building of 20,000 m2 | 6 lifts | 2.12 kWh/m2a |
| [40] | Measured | Seven-storey (excluding ground) office building, serving 460 employees (UK) | 3 gearless lifts | between 14,716 kWh and 19,862 kWh (3.2 kWh/m2a and 4.4 kWh/m2a) * |
| [40] | Measured | Seven-storey (excluding ground) office building, serving 460 employees (UK) | 3 geared lifts | between 63,636 kWh and 98,852 kWh (14 kWh/m2a and 21 kWh/m2a) * |
| [40] | Measured | Two-storey (excluding ground) office building, serving 410 employees (UK) | 2 hydraulic lifts | between 121,962 kWh and 145,130 kWh (30 kWh/m2a and 36 kWh/m2a) * |
| [37] | Measured | Office building of 20,438 m2, completed in 2010 in Colorado (National Renewable Energy Laboratory building) 3 storeys | Hydraulic lifts | 2.52 kWh/m2a |
| [37] | Hypothesis of refurbishment | Office building of 20,438 m2, completed in 2010 in Colorado (National Renewable Energy Laboratory building) 3 storeys | Regenerative geared lifts | 1.73 kWh/m2a |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Murshed, S.M.; Duval, A.; Koch, A.; Rode, P. Impact of Urban Morphology on Energy Consumption of Vertical Mobility in Asian Cities—A Comparative Analysis with 3D City Models. Urban Sci. 2019, 3, 4. https://doi.org/10.3390/urbansci3010004
Murshed SM, Duval A, Koch A, Rode P. Impact of Urban Morphology on Energy Consumption of Vertical Mobility in Asian Cities—A Comparative Analysis with 3D City Models. Urban Science. 2019; 3(1):4. https://doi.org/10.3390/urbansci3010004
Chicago/Turabian StyleMurshed, Syed Monjur, Alice Duval, Andreas Koch, and Philipp Rode. 2019. "Impact of Urban Morphology on Energy Consumption of Vertical Mobility in Asian Cities—A Comparative Analysis with 3D City Models" Urban Science 3, no. 1: 4. https://doi.org/10.3390/urbansci3010004
APA StyleMurshed, S. M., Duval, A., Koch, A., & Rode, P. (2019). Impact of Urban Morphology on Energy Consumption of Vertical Mobility in Asian Cities—A Comparative Analysis with 3D City Models. Urban Science, 3(1), 4. https://doi.org/10.3390/urbansci3010004





