1. Introduction
Numerous types of economic networks can be distinguished, such as strategic alliances (joint venture, equity strategic alliance and non-equity strategic alliance), supply chains, industrial districts (Marshallian industrial district, hub-and-spoke industrial district, hybrid industrial district, satellite industrial district, Italian industrial district), as well as local networks and regional clusters [
1,
2,
3,
4,
5,
6,
7,
8]. Nowadays, a new form of business network is emerging that is regarded as coopetition [
9,
10]. The notion of coopetition (mixture of cooperation and competition) was coined to the terminology system of economics by Bradenburger and Nalebuff (1996) [
11] more than two decades ago. Coopetition refers to a dynamic interplay in which rivals cooperate and compete with each other simultaneously in business segments, in order to reach higher profit rates [
9,
12,
13,
14,
15]. Among competitors, a special partnership and collaboration can be found. The coopetition, as a new inter-firm relationship, is defined as a network; more precisely, it appears as a rivals’ network.
The core idea of the paper is that every type of economic network has finite geographical extension. The size, spatial structure, vertices and edges of the business networks (and the coopetitive networks, of course) are nested in the space; the linkages have physical distances and nodes can be localized geographically as well [
16,
17,
18,
19,
20,
21]. Business networks therefore have to be analyzed by advanced toolkits of spatial network analysis (SpNA) to gain a deeper understanding of spatial characteristics, functions and complex accomplishments of economic players and their connections [
22]. Interestingly, spatial aspects of economic networks have so far been scrutinized by methods of economics, spatial statistics or spatial econometrics [
23,
24,
25,
26,
27,
28,
29], but not by toolkits of SpNA. This paper attempts to void this gap.
Basically, spatial traits of economic networks have to be examined empirically to recognize the network space, circumference and spatial structure of linkages and territorial allocation of vertices, geographical length of inter-firm connections, spatial small world effects, network diameter, network density and spatial interconnectivity as well [
21,
22,
30,
31,
32]. To reach these aims, advanced methods of SpNA are synthesized in this paper.
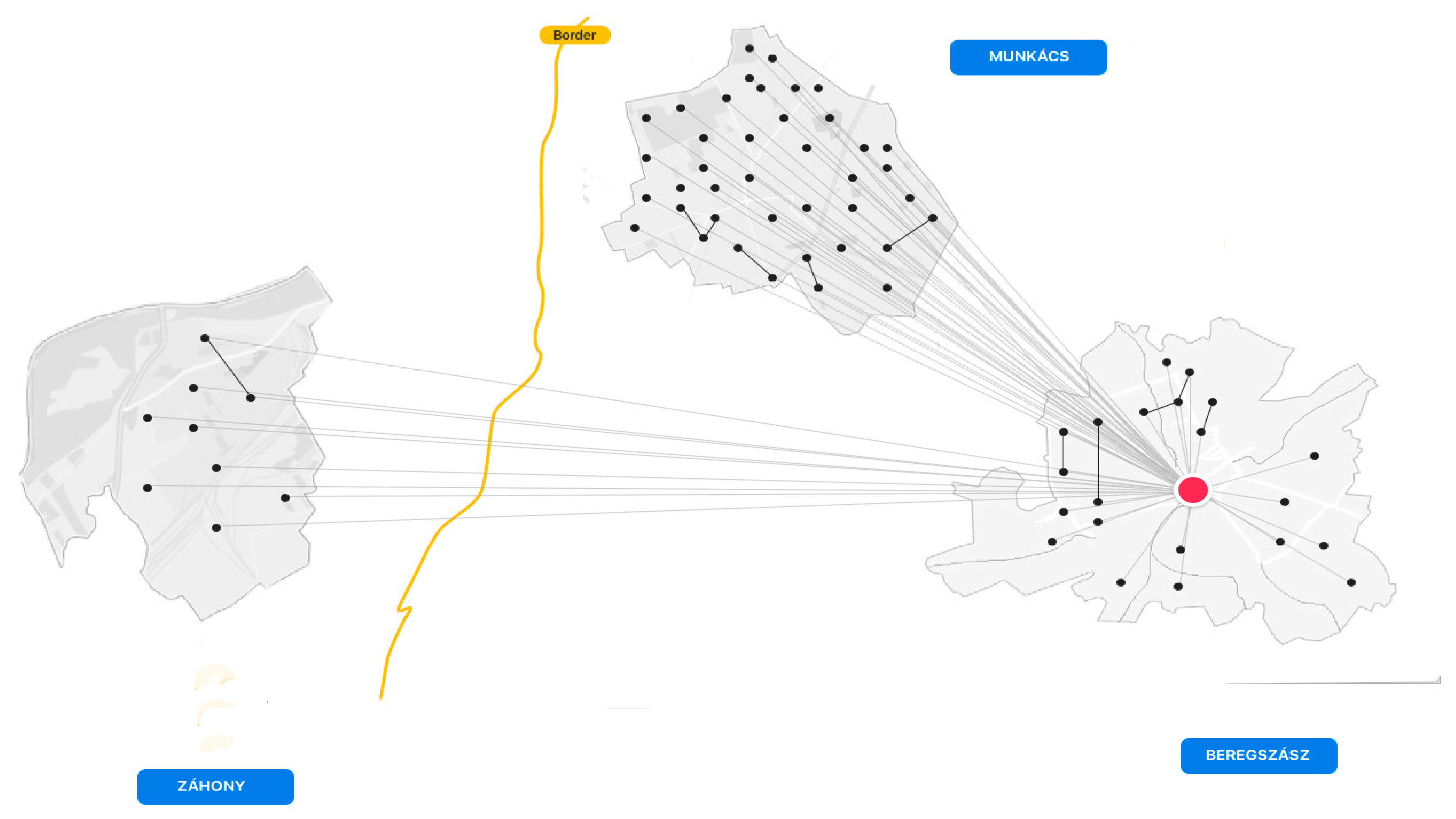
In this vein, the paper utilizing primary graph datasets describes and explains the spatial attributes of an informal, undirected (bidirectional links), unweighted, bottom-up coopetitive network functionalizing at transboundary level in Hungary and Ukraine. The interregional competitors’ network with 69 players can be found Záhony (nine agents) (Hungary), Beregszász (40 agents) (Ukraine) and Munkács (20 agents) (Ukraine) (network of Záhony-Beregszász-Munkács: NZBM).
As a whole, this empirical study could be defined as a cutting-edge inquiry. On one hand, a new type of economic network is dissected, and on the other hand, a new method (so-called SpNA) is utilized to unfold unknown segments of spatial patterns and the topology of a coopetitive network.
The paper is organized as follows. The section of theoretical background points out basic principles, experiences and operating mechanisms of a coopetition-based business strategy. Afterwards, the method section highlights how the primary network database was collected. Some relevant toolkits of SpNA are demonstrated, to assist in understanding the spatial dimensions of the new economic network. The results section details the core spatial traits of NZBM rooted from complex geospatial datasets. The final part of paper summarizes new empirical findings and recommends some options of intervention for the successful network-based regional economic development policy.
2. Materials and Methods
The coopeting partners could minimize the cost of transactions and mutual transportation, share risks, material and immaterial capacities, and resources (business information) to reach a higher profit rate. They could also realize the benefits of collective learning, knowledge spillover, product development and technological shifts. By employing a coopetition-based business approach, competitors are able to economize financial resources diminishing their expenditures. Thus, savings are realized and invested in further business developments contributing significantly to the regional economic growth. The paper stresses that competitors do not cooperate in every business sector within a framework of coopetition but only in some (two or maximum three) fields of business life. Coopetition does not destroy the main pillars of the market economy, since rivals’ cooperation has been built on the rules of the globalized free market by synthesizing collaborative and competitive advantages. Integrating both types of advantages, a higher profit rate can be realized, if only the rigorous and severe business competition rules would be preferred by the competitors [
33].
The coopetition is not just a nice theory; it functionalizes in the real economic scheme too. A large number of coopetitive examples have appeared at different regional levels, irrespective of the size of the company (from microenterprises to the multinational companies). Firstly, the coopetitive networks of transnational companies should be looked at. In 2005, the owners of Toyota and Citroën formed a coopetitive network in order to mutually improve and produce a new city car called the Peugeot 107. Since these rivals understood they would not able to separately finance the innovation background of the Peugeot 107, the costs of development and production were backed up by both multinational firms. Gain has been shared according to the partial of investments [
34]. Nevertheless, micro-, small- and medium- sized enterprises (SME) have started engaging in coopetition to maximize their profit rate. For example, in Germany, 279 rivals in the dairy industry have already established a formal inter-firm collaboration understanding that the coopetition-based business model could lead to raising gains [
35]. Likewise, agricultural advisory service providers working with more than 900 rivaling farmers started collaborating for a sustainable French agricultural branch from 2009 [
14]. Similar coopetitive networks can be found in the sector of steel, pharmaceuticals and semiconductors in order to utilize their capacities [
28]. Strese et al. (2016) [
36] analyze the empirically cross-functional coopetition of 392 German project leaders and department heads of the innovation industry, highlighting how rivals’ cooperation impacts exploitative innovation process. The notion of cross-functional coopetition describes intra-firm coopetition for material and immaterial assets appearing regularly in multinational companies. Bendig et al. (2018) [
13] also examine, employing a web-based survey, showing how in R&D sector 331, German managers’ cross-functional coopetition defines the accomplishment of companies day by day. By elaborating on expert interviews, Kraus-Schmid-Gast (2017) [
37] pinpointed how coopetitive networks of SMEs in the trust industry determine trends of innovation in Lichtenstein. Note that empirical findings reveal that intra-organizational coopetition determines the significantly and directly multilayer performance of business entities [
12,
33,
38,
39].
Additionally, in Tyrol, Austria, a formal coopetitive network (Leisure Card Tyrol) was introduced to integrate sectors of leisure business and tourism by 60 competitors (six museums, six ice skating rings owners, 13 bonus partners, 12 swimming pools owners and 23 mountain railway companies). The results of the qualitative graph database show that network agents have been able to reach a higher gain rate by applying coopetition business plans. Additionally, the local societal relationships (intangible asset) and the landscape (material asset) have also been reshaped basically and positively by the effects and externalities of this coopetitive network [
39]. Similar coopetitive networks can be recognized in the tourism sectors of Canton of Ticino (Switzerland) and Basilicata (Italy) as well [
40,
41].
A large number of coopetitive networks have been operating in the post-communist Poland. In the high-tech sector, 235 rivals [
42], and 66 competitors in the tourism branch [
33] created coopetitive networks in order to mutually allocate their resources to eliminate expenditures.
The competitors’ cooperation has been scrutinized by economics, mathematics, game theory, marketing and management science, notwithstanding. Its spatial perspectives and approaches were marginalized in the scientific inquiries. The paper attempts to fill this gap by demonstrating in the next sections the relevant spatial dimensions of two transboundary coopetitive networks based on primary, quantitative and qualitative network datasets.
The coopetitive network model (N) embraces a finite, nonempty set of vertices (V) and a finite, nonempty set of edges (E). The number of vertices in V is defined as i, calling the degree () of N and the number of edges in E is referred to as j. Moreover, in the coopetitive network model the node demonstrates the location of a firm. Meanwhile, the edge means undirected coopetitive interactions between rivals. In this paper, every vertex demonstrates the geographical position of a microenterprise, small- or medium- sized company. The coopetitive interaction that is depicted by an edge is regarded to be a business communication channel in which business information flows among competitors. The weight, strength and flow parameters of linkages are the same in both of networks. Furthermore, in both networks the coopetitive activities are planned and coordinated by emails. Thus, the linkage of length is defined as the Euclidian distance (non-spatial relationships); the connection between rivals is illustrated as crow flies in the maps of inter-firm relationships.
Whereas bottom-up coopetitive networks functionalize informally, I did not have a formal list about the geographical positions of network players. I encountered the first network agent, by coincidence, at a social event. He provided more information on rivals’ cooperation, and as a result, by applying a snow-ball sociological method I could map up the overarching coopetitive networks of local restaurant owners. Sociological, semi-structured interviews were made and questionnaires were undertaken separately with every restaurant owner (69 players). Altogether, 69 interviews and 69 questionnaires were mustered between September 2016 and October 2017. In this inquiry, quantitative and qualitative methods were synthesized to explore the depth and scope of territorial patterns of the inter-firm collaboration of competitors.
Firstly the networked place has to be delimited geographically. To this end, by following the Eulerian path of a network (i.e., a path that visits each node exactly once), the outermost vertices are connected in every network to each other, obtaining the networked place. Obviously, the networked placed is a two-dimensional, irregular polygon thus the circumference (
CI) of the network is obtained [
43,
44]:
where
demonstrates the physical distance between two nodes. Simply put, the outermost nodes of the network are interconnected to delimit the network. The network shape is recognized as an irregular two-dimensional polygon. The
CI of the irregular polygon could be calculated if the geographical lengths of sides of the polygon are added.
Afterward, the diameter (i.e., the largest spatial distance between two vertices) of a coopetitive network is calculated [
22]. As above, the coopetitive interactions between two players appear as non-spatial relationships that are gauged by Euclidian distance (or the shortest distance), applying kilometer (km). The Euclidian distance refers to the non-spatial distances, which are visualized and understood as crow flies with relative short straight lines. Thus, the connections in such a network are not circuitous and are not too long, relatively [
21]. The computing of Euclidian distance based on the Pythagorean theorem is obtained in a simple way:
where
means Euclidian distance between nodes [
17,
22]. In the practice, every length of linkages is measured separately, and the longest is regarded as the spatial diameter of the network.
Subsequently, the spatial topology of coopetitive networks is operationalized. The paper develops a new measurement model to gauge the empirically spatial structure of the coopetitive networks. This network measurement model simultaneously considers the linkages of spatial architecture and the vertices of geographical allocations as well. More precisely, the connections of the territorial structure are measured with degree distribution. This is in order to map up the spatial layout of the inter-firm relationships of rivals, and on the other hand, to recognize whether one or more hubs exist in the network [
43]. Nevertheless, the vertices of spatial distribution are gauged by using quadrat analysis (i.e., the quadrat analysis focuses on spatial patterns) and the allocation of players by comparing the number of vertices among the same sized cells; the size of a grid has no mathematical rules or theorems, it is defined by the researcher [
45,
46,
47]. Both techniques (degree distribution and quadrat analysis) are applied simultaneously to better understand the territorial framework of the nodes and edges of coopetitive networks.
Whereas the hub triggers spatial small world phenomenon (or six degrees of separation), referring to how a focal firm (or hub) is able to shortcut the distance nested in the space between players [
22,
43], so that competitors could collaborate in some business fields. The spatial small world (
SSW) can be estimated well
, where
means the average degree and
[
30,
43].
In addition, the spatial density (
SD) of coopetitive interactions presents how business information spread among economic actors within a certain territorial unit.
SD is expressed with a well-known formula:
comparing the actual connections to the potential connections [
48,
49].
Finally, the global connectivity index (
) is elaborated by [
44] gauging the spatial interconnectivity of networks. [
44] If an undirected vertex
i is linked in a region (
r) to a vertex
j in region (
p) (that is a transboundary network), this linkage can be expressed
, that takes the value of one if an edge is existing, or zero if otherwise. The total number of undirected, global connections of the network (
G) could be computed:
where
tr stands for total number of regions. Furthermore, if a vertex
i is connected to other nodes
j in the same region, the linkages are demonstrated by
that takes the value 1 if an edge is existing and zero is otherwise. The total number of undirected, local connection of the network (
L) is obtained:
Finally, value of the global connectivity index is computed:
The sociological, semi-structured primary interviews are elaborated with structured content analysis and longitudinal input-output analysis. Parts of the interviews are written in italics.
To sum up, firstly the circumferences, diameters and spatial shapes of both coopetitive networks are measured to describe their basic territorial traits. Subsequently, the spatial structures of coopetitive networks are dissected by synthesizing two special methods of SpNA. On one hand, the spatial topology of edges is measured by degree distribution, and on the other hand, the spatial framework of nodes and the hub are investigated and localized with quadrat analysis. Later, the territorial functions and features of the hub are examined by SSW detecting how the giant component shortcuts distance among nodes. Finally, spatial patterns of the interconnectivity of coopetitive networks are gauged by the spatial density and the global connectivity index as well.
3. Results
Firstly the general features of NZBM are demonstrated. The network agents, that are local microenterprises or/and SMEs, cooperate with each other only in two business segments. On one hand, they purchase different products together, the wholesalers thus provide discount to them; savings are realized by every coopeting enterprise because they purchase in a bulk. On the other hand, products are transported mutually that requires fewer trucks economizing financial resources. Shortly,
The coopetitive savings, which stem from two coopetitive activities, are invested in creating new jobs and/or increasing level of income. As a result, the coopetitive network contributes to the local economic development and growth significantly. Simply put, network agents purchase in bulk to receive discounts from wholesalers and transport products together. These acts permit to eliminate expenditures, and in parallel, increase revenue streams. The firms reduce their expenditures, but the price of goods is not diminished; finally their profit rate increase.
Furthermore, NZBM was established informally after the economic crisis of 2008. It can be explained that with growing unemployment and poverty rates, numerous firms lost an overwhelming element of capital. Meanwhile, some went out of the business structure and capital inflow was mitigated dramatically in the post-communist regions. Companies needed financial resources, but they did not have them. Some firms, therefore, formed coopetitive networks to reduce their expenditures and raise profit without any government interventions or other formal support. More generally, coopeting partners have converted their relational capital (immaterial asset) into economic capital (material asset) after the credit crisis [
50]. By following this business strategy, new financial resources have appeared in the developing regions without regional economic development policy or other foreign investors. More recently, a large number of developed regions have already managed to accept coopetition based businesses, and not just the emerging places. The territorial extension of NZBM can be seen in
Figure 1.
Besides, the NZBM incorporates a focal firm, representing it with a red point on the map. The focal firm is called the dominant firm, major firm, central firm or broker by economics and management science. This paper uses only the notion of focal firm for clear interpretation. The focal firm has meaningful spatial functions in the coopetitive network. This paper, unfortunately, cannot deal with these in detail but focuses on merely describing the core functions of it.
Rivals cooperate rarely with each other in the real economy due to their harmful, earlier experiences. Competitors do not trust in each other, but attempt to defeat each other, in accordance with the rules of the free market economy. The rivals loathe each other in the real economic life. The competitors, whereupon, do not interact with each other, but every rival has trust in the focal firm in NZBM. The focal firm bridges the gaps between network players, manages coopetitive activities and allocates business information among rivals. The interviews highlight that the focal firm works in the networks for charity and for free; the hub’s ability to acquire links is high.
‘I am a well-known and a successful businessman, I do not need more and more profit, I only want to maintain my own business circles. But I want to help to my region too so I use my personality and connections for assisting to other local entrepreneurs, competitors. I would be happy to help them, for example, I organize mutual transportation. It is my mission for my region.’
(second interview).
Obviously, the coopetitive network would not have been established and evolved if the focal firm did not mediate and interlink between competitors. In a nutshell, rivals in this network communicate and collaborate mostly through the focal firm, because they cannot stand each other but confide in the focal firm.
Since the mutual purchase and transportation emerge as a daily routine in the networks, it can already be organized easily by the focal firm. Regularly, the same amount of fruit, spices, salt and sugar, vegetables and meat are bought and transported together every weekday for mutual benefit. The focal firm is able to estimate the number of used trucks for mutual transportation. The 50 or maximum 60 percent of all the trucks is enough to transport different goods for all the networks. On the first day, a half of set of vehicles delivers products. The next day, another set of trucks transport what was missed previously, so every firm participates equally in the coopetitive activities. As a result, every rival could receive products and spend less time on buying goods. Inputs are purchased at a lower unit price, the profit rate can be increased and the extent of local pollution may be mitigated as well. The goods are delivered to a transshipment point in all three cities, where products are distributed among players.
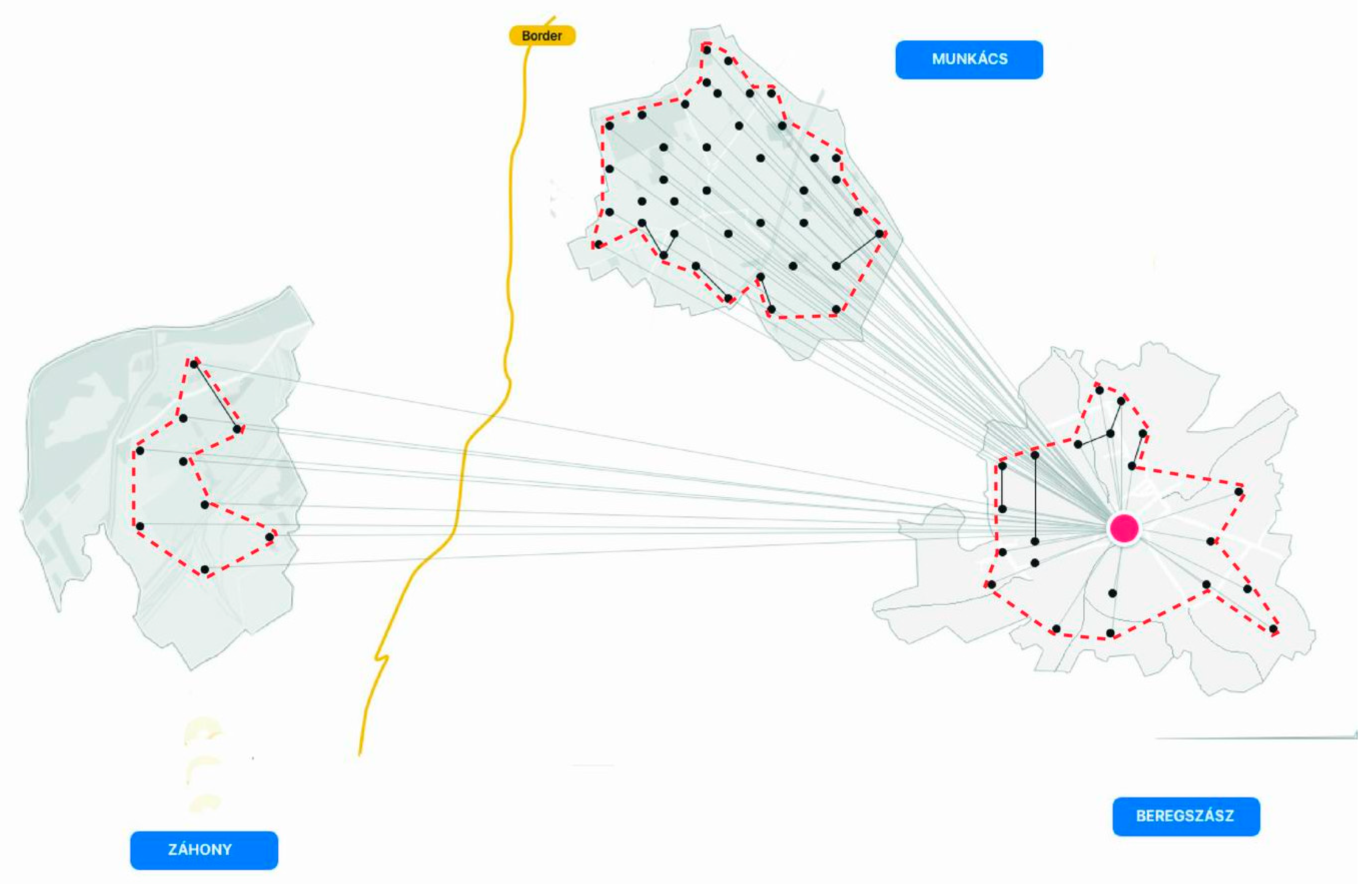
As above, the delimitation of the network can be carried out geographically if the outermost vertices of the network are tied to each other (Eulerian path) in every city. The border of the networked place is demonstrated with red dotted lines in
Figure 2.
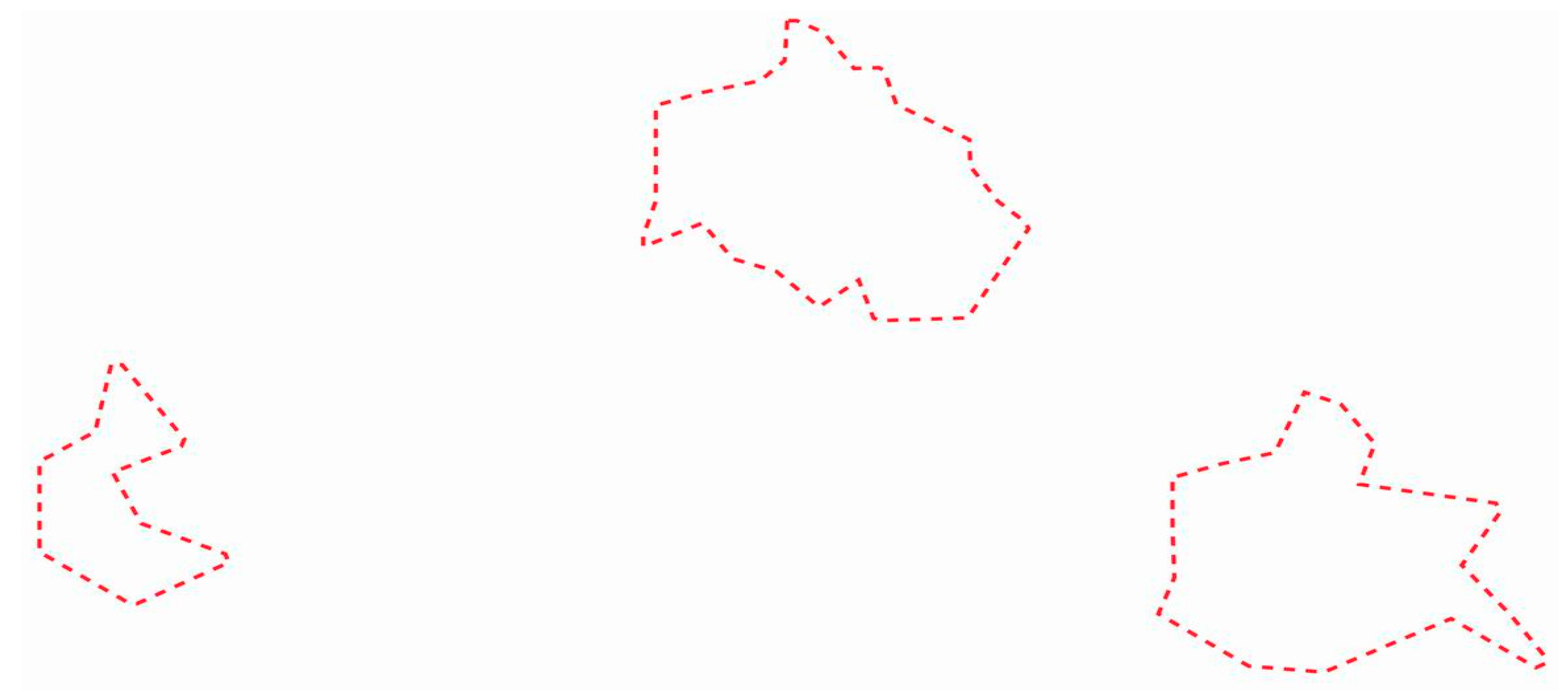
The red dotted lines depict the circumference of the network as well, that is recognized as a two-dimensional, irregular polygon (see
Figure 3); circumferences of the network can be calculated easily with the above mentioned method of SpNA.
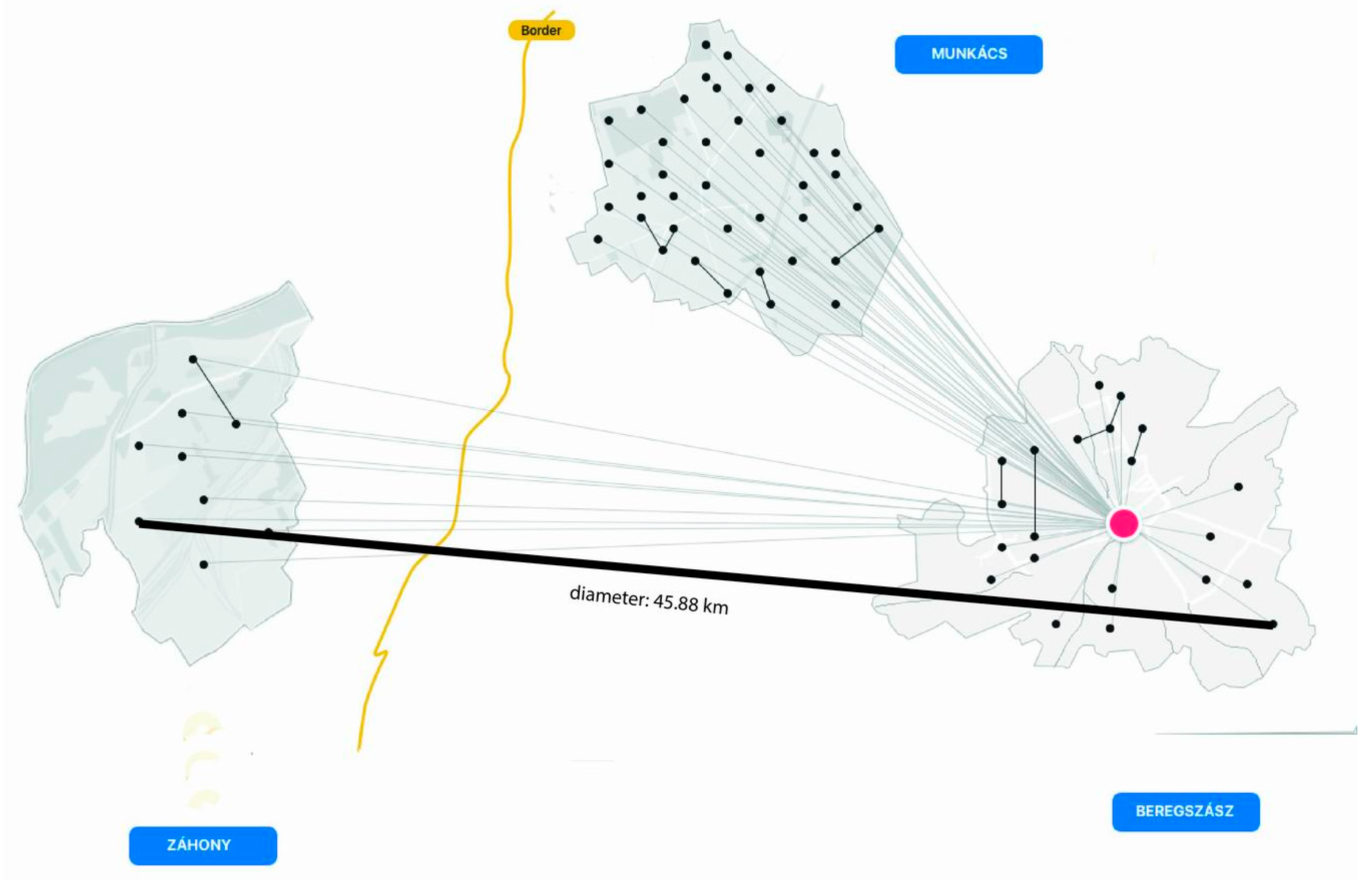
According to the empirical measurements, the circumference of NZBM is 42.01 km. Moreover, the diameter of NZBM is 45.88 km, crossing the Hungarian east national border and more statistical regional boundaries as well (see
Figure 4).
Table 1 displays the average spatial length of the connection of NZBM, which is 20.82 km long.
At this point, the question emerges: how does the highly connected focal firm sustain the coopetitive connection among competitors in the long run, using only emails or/and phone?
Responding to this sharp question, the focal firm said that initially the coopetitive network interactions were based on face-to-face relationships. Later, when more coopeting firms (restaurant owners) were attached to the network, the focal firm could not keep interacting with every agent in person. Recently, the coopetitive connection has therefore been built on emails or/and phone calls. The telecommunication devices permit the very strongly connected node to be able to interact with those network players that are set up far from the giant component.
In the network, there are not just hub-node connections; some node-node relationships can also be found. In the practice, the focal firm in the undirected hub-node interaction, writes an email to rivals and receives confirmation emails from competitors. They email back and forth. Nevertheless, the node-node coopetitive nexus is composed of just forwarded emails, in which rivals forward the focal firm’s email to each other, containing the details of next mutual purchase and transportation. The forwarded email does not include new business information, but merely repeats the hub’s message. As a whole, the coopetitive linkage incorporates the hub-node (new information) and node-node (forwarded email) relationships as well.
Shortly, the coopetitive linkages do not require nowadays face-to-face relationship irrespective of immense and huge distance (see result of diameter). The IT-based coopetitive interaction system has been functionalizing effectively and has proved to be sustainable in these post-communist regions.
‘For ages I have known A.B. (fictitious name of the focal firm in NZBM) in flash, he has an integrative personality that is why I trust him totally. I trust his abilities to arrange our mutual transportation, he is good in this field, he is an expert despite he did not learn such things at the school. We keep in touch in emails, earlier we talked to each other face-to face, but now we do not need it. We write emails to each other organizing mutual transportation. I have a restaurant another city than A. B. lives but it does not matter because we can manage mutual business using emails. The emails defeat the distance in the business.’
(37th interview).
As already mentioned, the spatial structures of coopetitive networks are computed by twofold ways. On one hand, spatial patterns of coopetitive interactions (edges) are gauged by degree distribution. On the other hand, the spatial allocation of locations of firms are examined with quadrat analysis. Both parameters have to be taken into account at the same time. Several papers have so far applied only degree distribution, because of the overlooked spatial dimensions of economic networks. This paper fills this gap and empirically studies the spatial allocation of both edges and nodes as well.
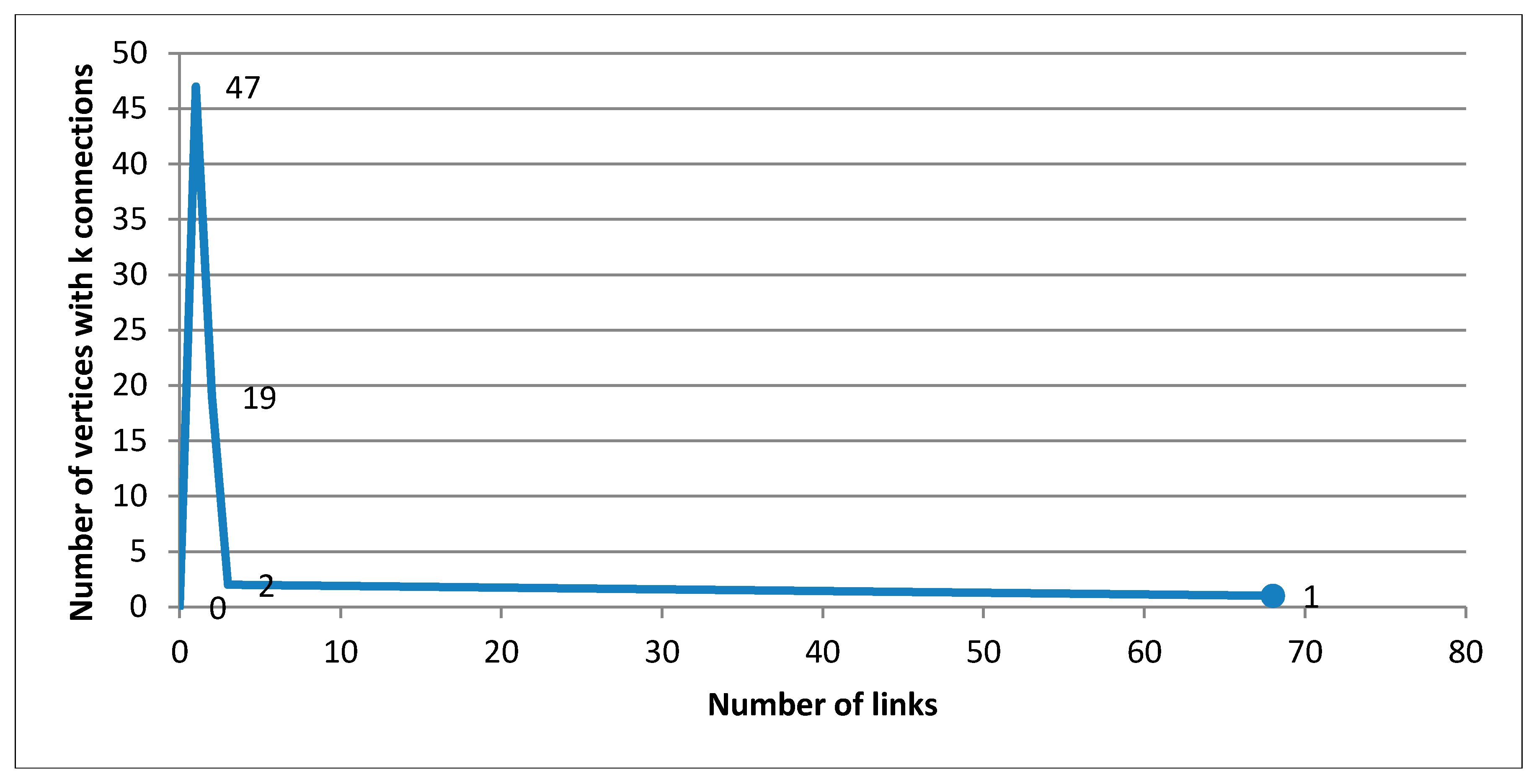
Firstly, the linkages of the spatial layout have to be operationalized, since interactions constitute network space. The results of degree distributions of coopetitive networks present the topology of linkages (see
Figure 5). The coopetitive network has hub-and-spoke-network architecture [
51]. This network structure refers to how every player connects to the focal firm, so the hub has numerous bonds in the network (more precisely, the hub has E-1 connections). In parallel, the most vertices (the small-degree nodes) have a few connections in the network [
18,
21,
22]. For example, in Beregszász every agent bonds to the focal firm, however, only five linkages can be found among other nodes, in the same town. The centralized framework of coopetitive networks similar to star graphs (i.e., a type of network scheme in which a hub links to every node but the vertices do not connect to each other (only to the hub), but it is not the same.
Besides, the hub-and-spoke network system indicates that the coopetitive networks have a relatively high robustness and, of course, the network vulnerability is relatively low. More specifically, if the coopetitive network is attacked
randomly, the network could continue to operate. This is because in all probability, those nodes will be removed out from the network that have only a few connections. In contrast, when the network is attacked
consciously, the focal firm will be removed from the graph. The economic network whereupon will collapse and may disappear rapidly [
43].
A further merit of the hub-and-spoke network framework is that the business information could remain clean due to the focal firm providing information to rivals, and after that they send confirmation emails or phone calls. Only the hub provides new information, the small-degree nodes do not give additional information; they merely may forward the hub’s messages to the rivals or send a confirmation email to the hub. Thus, disinformation cannot be added in the network and misunderstandings can be avoided by everyone. The function of the hub provides effective information circulation and less friction for the network players.
Notwithstanding, [
19,
20] highlights that dictatorial, hierarchical relationships may be formed between the focal firm and the nodes with a few connections; the focal firm may command to every agent and they obey to the focal firm. By contrast, results of the primary network datasets emphasize that the focal firm never dictates to anyone. It only manages, bridges and organizes among rivals; horizontal network structure functionalizes, and not vertical in both coopetitive networks. The hub emerges as the Achilles Heel in the coopetitive network.
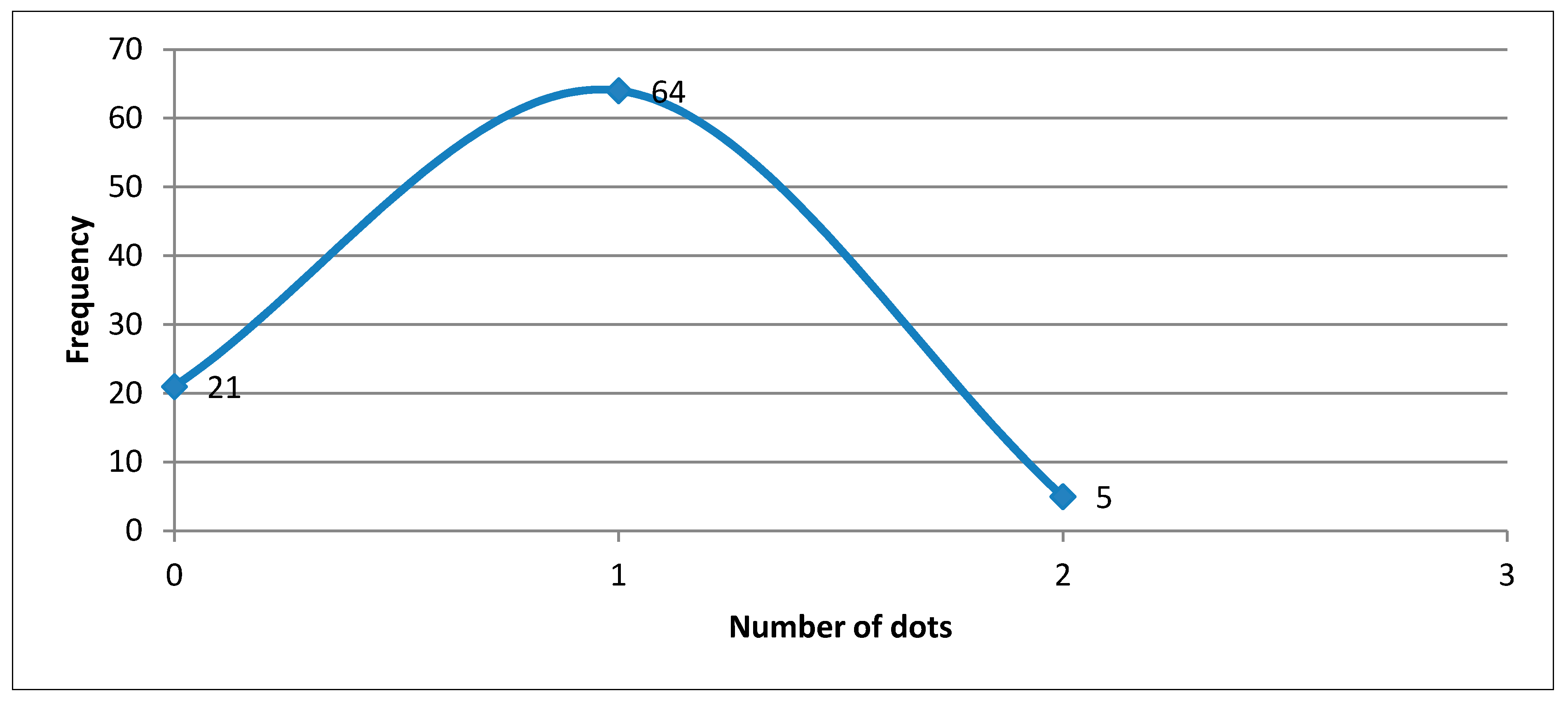
Subsequently, the vertices of spatial allocation have to be dissected by quadrat analysis. More precisely, a regular grid of squares is superimposed over the map of coopetitive network and the number of events falling in each grid area is counted. A cell covers a 350 × 350-m area in this study. Since the territories among cities are empty cells, only the networked places are studied in the cities where enterprises were established.
The results of quadrat analysis demonstrate that relatively big geographical distance can be found among rivals in the same city (
Figure 6). It proves that not always, but regularly, only one dot exists in a grid, sometimes, in turn, zero.
By concentrating on quadrat analysis (in which a grid depicts 350 × 350 m) of cities that involve the hubs, it can be seen that there are not clustered areas of nodes close to the hub (see
Figure 7). The paper presupposed that numerous vertices would allocate close to the hub, but the empirical gauges underscore that the intrinsic quality of the connection between the hub and nodes does not depend on the geographical proximity or distance.
To sum up, the degree distribution points out that the hub is tied to every rival while competitors rarely connect to each other; the coopetitive network follows hub-and-spoke spatial architecture. However, the quadrat analysis accentuates that the super-component of the network geographically and physically exist relatively far from clusters of nodes; the hub cannot be found in the clustered field of the networks. More precisely, the focal firms were established in the core of the cities but rivals and their clusters regularly were evolved in the periphery.
The
SSW describes how a focal firm as a super component shortcuts geographical, cognitive and organizational distance by bridging and mediating among rivals. According to the empirical results (see
Table 1), in NZBM (7.784) the value of
SSW is low. Therefore, the willingness towards coopetition cannot be called high, meaning the trust exists at a low level. Irrespective of this fact, the rivals’ inter-organizational collaboration operates positively and adequately because the focal firm shortcuts distances between them.
The global connectivity index (
) compares the interregional connectivity to the intraregional connectivity in the network. The empirical results illustrate that NZBM functionalize as sparse networks, referring to few linkages existing among rivals, meanwhile the hub has a tremendous number of ties so as to mediate among competitors (see
Table 1). The value of interconnectivity (283.4944) is high; referring to how the hubs have been able to cooperate and bridge at a transboundary level among competitors.