Transmission Bender as an Analyzer Device for MIEZE
Abstract
:1. Introduction
- Acceptance of beam divergence up to ±1.5
- High polarization ≥ 95%
- Unchanged beam direction, divergence, or homogeneity
- Stable polarization during long measurement times
- Physical dimensions and stray magnetic fields as small as possible
- Good transmission over a broad wavelength range from 4.5 Å to 15 Å

2. State of the Art
2.1. He Transmission Filter
2.2. Solid State Devices
2.3. Transmission Bender Device for MIEZE Applications
Simulations
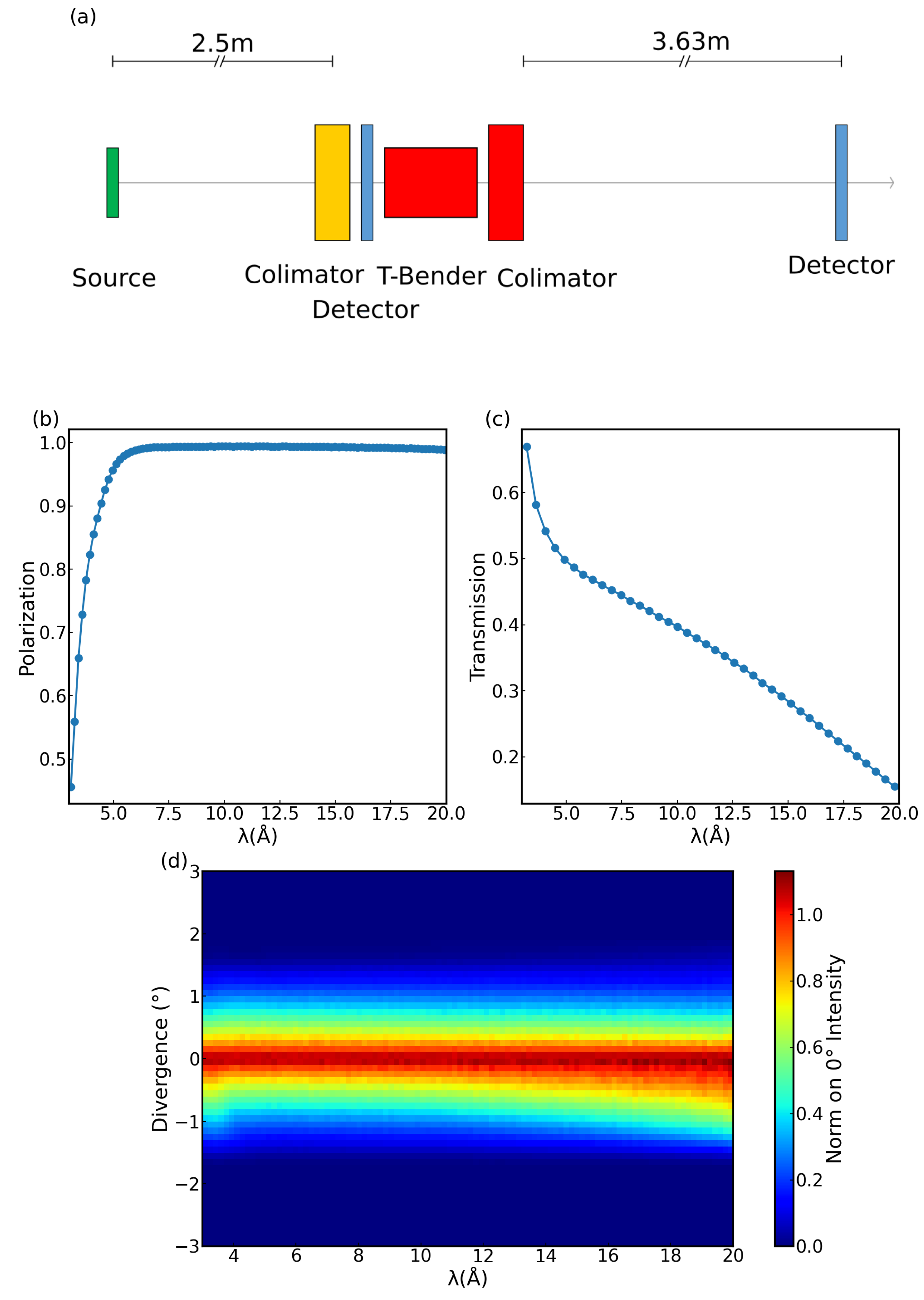
3. Experimental Tests
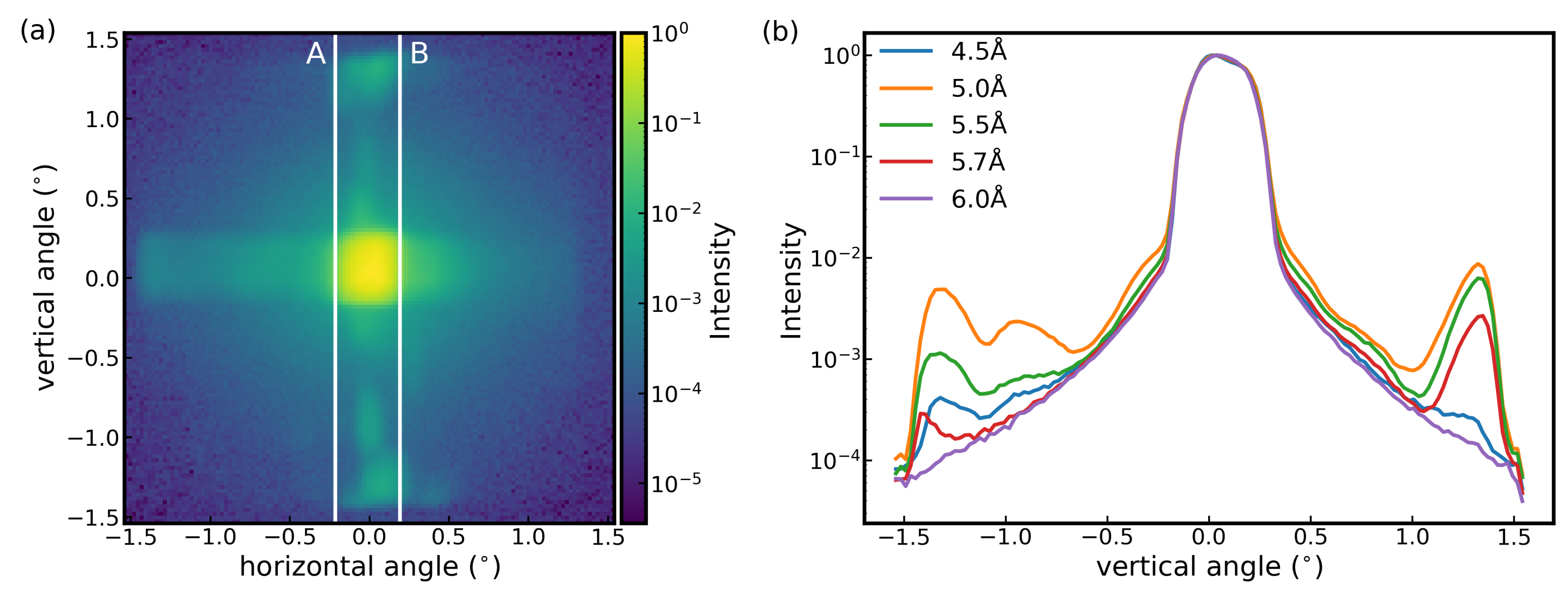
3.1. Commissioning

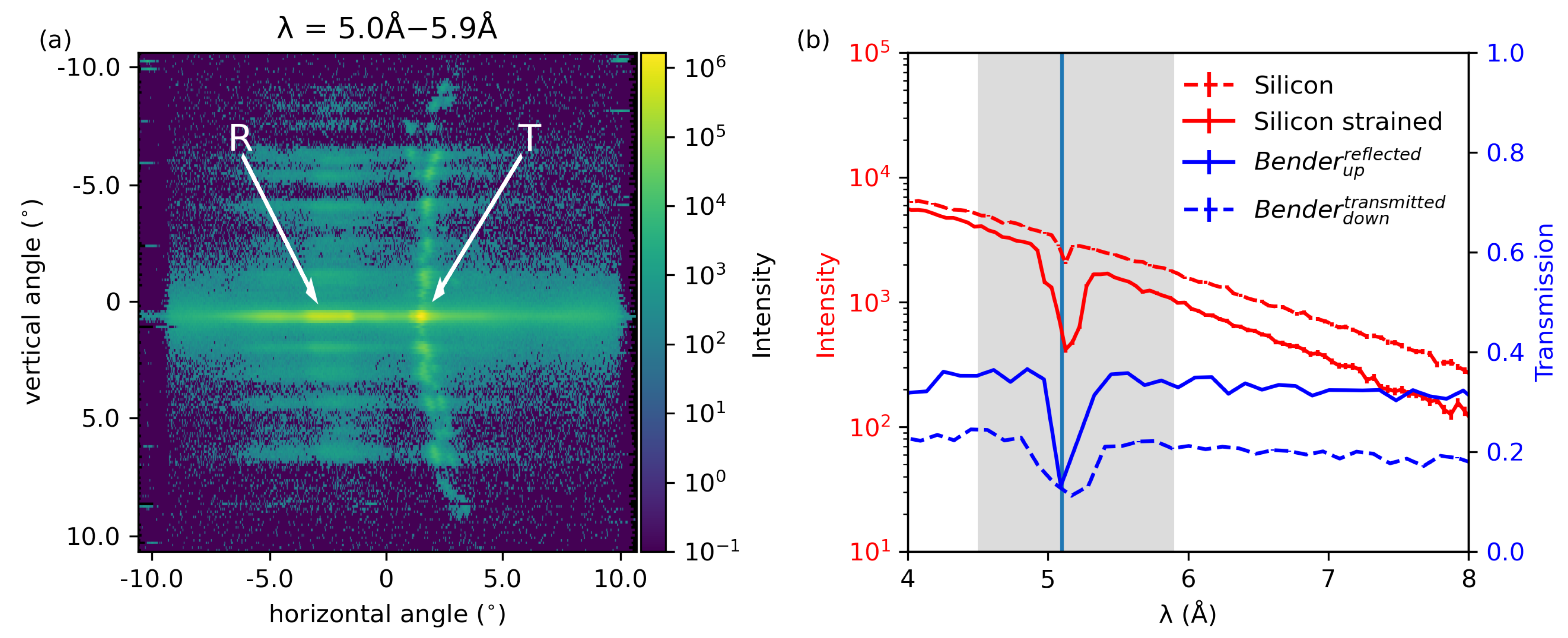
3.2. Spurious Scattering
3.2.1. Experiment
3.2.2. Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| MIEZE | Modulation of IntEnsity with Zero Effort |
| rf | radio frequency |
| SANS | Small angle neutron scattering |
| SEOP | spin exchange optical pumping |
| MEOP | metastability–exchange optical pumping |
References
- Honecker, D.; Bersweiler, M.; Erokhin, S.; Berkov, D.; Chesnel, K.; Venero, D.A.; Qdemat, A.; Disch, S.; Jochum, J.K.; Michels, A.; et al. Using small-angle scattering to guide functional magnetic nanoparticle design. Nanoscale Adv. 2022, 4, 1026–1059. [Google Scholar] [CrossRef]
- Franz, C.; Soltwedel, O.; Fuchs, C.; Säubert, S.; Haslbeck, F.; Wendl, A.; Jochum, J.K.; Böni, P.; Pfleiderer, C. The longitudinal neutron resonant spin echo spectrometer RESEDA. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2019, 939, 22–29. [Google Scholar] [CrossRef]
- Jochum, J.K.; Hecht, A.; Soltwedl, O.; Fuchs, C.; Frank, J.; Faulhaber, E.; Leiner, J.F.K.C.; Pfleiderer, C.; Franz, C. Oscillatory magnetic fields for neutron resonance spin-echo spectroscopy. Meas. Sci. Technol. 2020, 32, 045902. [Google Scholar] [CrossRef]
- Jochum, J.K.; Wendl, A.; Keller, T.; Franz, C. Neutron MIEZE spectroscopy with focal length tuning. Meas. Sci. Technol. 2019, 31, 035902. [Google Scholar] [CrossRef]
- Cussen, L.D.; Goossens, D.J.; Hicks, T.J. 3He neutron polarising filters—Theoretical comparison with supermirrors and Heusler alloy polarisers. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2000, 440, 409–420. [Google Scholar] [CrossRef]
- Xu, J.; Atterving, M.; Skoulatos, M.; Ostermann, A.; Georgii, R.; Keller, T.; Böni, P. Design of a neutron polarizing bender for a cold triple-axis spectrometer. Nucl. Instrum. Methods Phys. Res. Sect. Accel. Spectrom. Detect. Assoc. Equip. 2022, 1031, 166526. [Google Scholar] [CrossRef]
- Kreuz, M.; Nesvizhevsky, V.; Petoukhov, A.; Soldner, T. The crossed geometry of two super mirror polarisers—A new method for neutron beam polarisation and polarisation analysis. Nucl. Instrum. Methods Phys. Res. Sect. Accel. Spectrom. Detect. Assoc. Equip. 2005, 547, 583–591. [Google Scholar] [CrossRef]
- Gentile, T.R.; Nacher, P.J.; Saam, B.; Walker, T.G. Optically polarized 3He. Rev. Mod. Phys. 2017, 89, 045004. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chen, W.C.; Gentile, T.R.; Ye, Q.; Walker, T.G.; Babcock, E. On the limits of spin-exchange optical pumping of 3He. J. Appl. Phys. 2014, 116, 014903. [Google Scholar] [CrossRef]
- Kindervater, J.; Martin, N.; Häußler, W.; Krautloher, M.; Fuchs, C.; Mühlbauer, S.; Lim, J.A.; Blackburn, E.; Böni, P.; Pfleiderer, C. Neutron Spin Echo Spectroscopy under 17 T Magnetic Field at RESEDA. EPJ Web Conf. 2015, 83, 03008. [Google Scholar] [CrossRef] [Green Version]
- Syromyatnikov, V.G.; Pusenkov, V.M. New compact neutron supermirror transmission polarizer. J. Phys. Conf. Ser. 2017, 862, 012028. [Google Scholar] [CrossRef] [Green Version]
- Krist, T.; Rucker, F.; Brandl, G.; Georgii, R. High performance, large cross-section S-bender for neutron polarization. Nucl. Instrum. Methods Phys. Res. Sect. Accel. Spectrom. Detect. Assoc. Equip. 2013, 698, 94–97. [Google Scholar] [CrossRef] [Green Version]
- Stunault, A.; Andersen, K.H.; Roux, S.; Bigault, T.; Ben-Saidane, K.; Rønnow, H.M. New solid state polarizing bender for cold neutrons. Phys. B Condens. Matter 2006, 385–386, 1152–1154. [Google Scholar] [CrossRef]
- Petukhov, A.K.; Nesvizhevsky, V.V.; Bigault, T.; Courtois, P.; Jullien, D.; Soldner, T. A project of advanced solid-state neutron polarizer for PF1B instrument at Institut Laue-Langevin. Rev. Sci. Instrum. 2019, 90, 085112. [Google Scholar] [CrossRef] [PubMed]
- Petukhov, A.; Nesvizhevsky, V.; Bigault, T.; Courtois, P.; Jullien, D.; Soldner, T. A concept of advanced broad-band solid-state supermirror polarizers for cold neutrons. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2016, 838, 33–38. [Google Scholar] [CrossRef] [Green Version]
- SwissNeutronics. Available online: https://www.swissneutronics.ch/products/neutron-supermirrors/ (accessed on 26 July 2022).
- Willendrup, P.K.; Lefmann, K. McStas (i): Introduction, use, and basic principles for ray-tracing simulations. J. Neutron Res. 2020, 22, 1–16. [Google Scholar] [CrossRef] [Green Version]
- Sears, V.F. Neutron scattering lengths and cross sections. Neutron News 1992, 3, 26–37. [Google Scholar] [CrossRef]
- Fredrikze, H.; van de Kruijs, R. Calibration of a polarized neutron reflectometer. Phys. B Condens. Matter 2001, 297, 143–147. [Google Scholar] [CrossRef]
- Lieutenant, K.; Cussen, L.D. Beam transport in double elliptic neutron guides. J. Neutron Res. 2015, 18, 127–134. [Google Scholar] [CrossRef]
- Renninger, M. “Umweganregung”, eine bisher unbeachtete Wechselwirkungserscheinung bei Raumgitterinterferenzen. Z. FüR Phys. 1937, 106, 141–176. [Google Scholar] [CrossRef]
- Barker, J.G.; Mildner, D.F.R. Survey of background scattering from materials found in small-angle neutron scattering. J. Appl. Crystallogr. 2015, 48, 1055–1071. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shah, V.R.; Washington, A.L.; Stonaha, P.; Ashkar, R.; Kaiser, H.; Krist, T.; Pynn, R. Optimization of a solid state polarizing bender for cold neutrons. Nucl. Instrum. Methods Phys. Res. Sect. Accel. Spectrom. Detect. Assoc. Equip. 2014, 768, 157–163. [Google Scholar] [CrossRef]
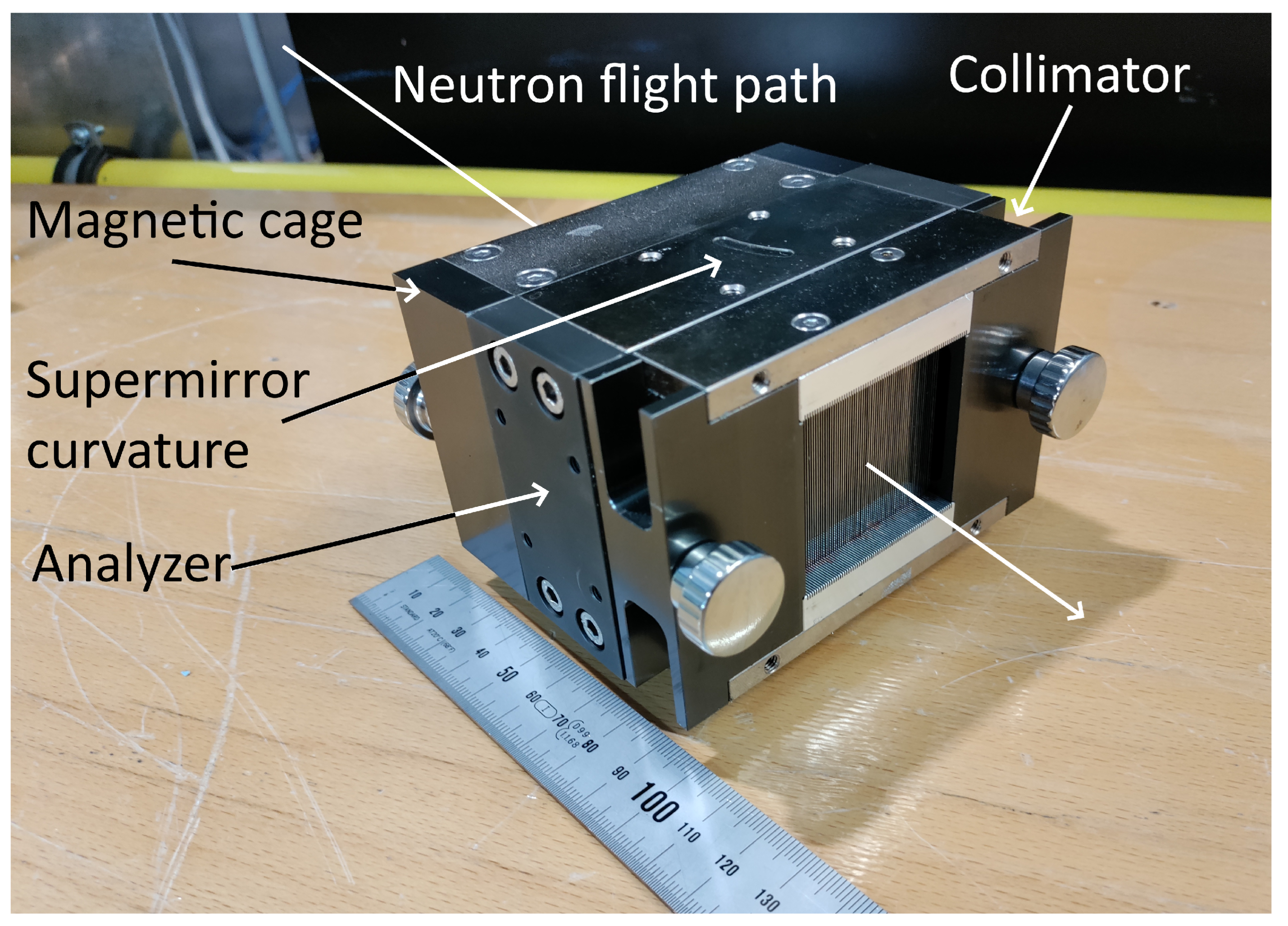

| Polarizing Bender with m = 5 | |
| Design | solid state |
| transmission bender | |
| coating | Fe/Si m = 5 |
| wafer thickness | 0.15 mm |
| radius of curvature | r = 0.56 m |
| length | 30 mm |
| critical wavelength | = 3.24 Å |
| magnetic field | B = 45 mT |
| Solid State Collimator | |
| horizontal collimation | 120 |
| material | Si |
| absorbing coating | Gd |
| wafer thickness | 0.3 mm |
| length | 10 mm |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jochum, J.K.; Cooper, J.F.K.; Vogl, L.M.; Link, P.; Soltwedel, O.; Böni, P.; Pfleiderer, C.; Franz, C. Transmission Bender as an Analyzer Device for MIEZE. Quantum Beam Sci. 2022, 6, 26. https://doi.org/10.3390/qubs6030026
Jochum JK, Cooper JFK, Vogl LM, Link P, Soltwedel O, Böni P, Pfleiderer C, Franz C. Transmission Bender as an Analyzer Device for MIEZE. Quantum Beam Science. 2022; 6(3):26. https://doi.org/10.3390/qubs6030026
Chicago/Turabian StyleJochum, Johanna K., Jos F. K. Cooper, Lukas M. Vogl, Peter Link, Olaf Soltwedel, Peter Böni, Christian Pfleiderer, and Christian Franz. 2022. "Transmission Bender as an Analyzer Device for MIEZE" Quantum Beam Science 6, no. 3: 26. https://doi.org/10.3390/qubs6030026
APA StyleJochum, J. K., Cooper, J. F. K., Vogl, L. M., Link, P., Soltwedel, O., Böni, P., Pfleiderer, C., & Franz, C. (2022). Transmission Bender as an Analyzer Device for MIEZE. Quantum Beam Science, 6(3), 26. https://doi.org/10.3390/qubs6030026







