Wolf Rock Lighthouse Long-Term Monitoring
Abstract
1. Introduction
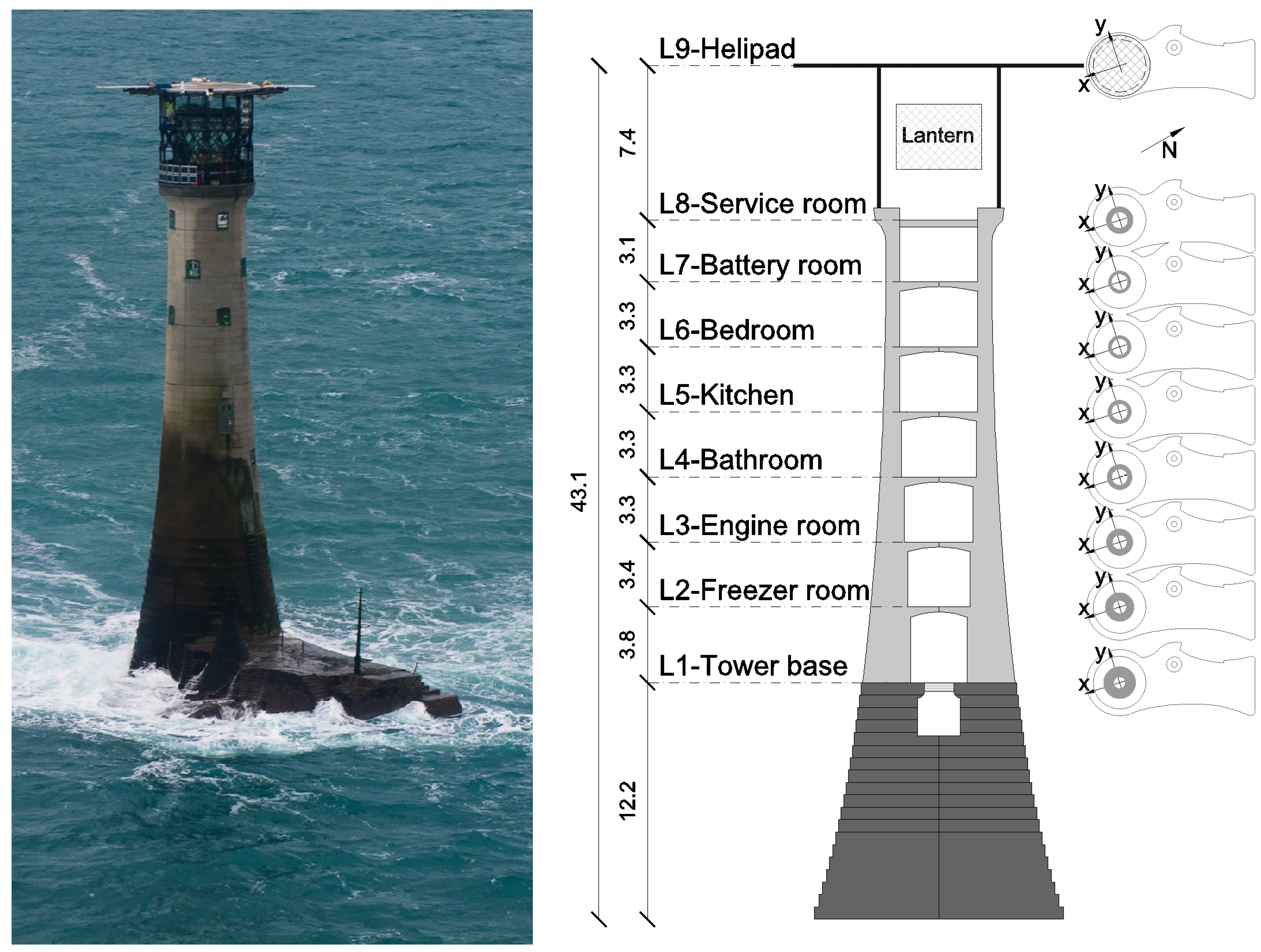
2. Description of Wolf Rock Lighthouse
3. Modal Tests
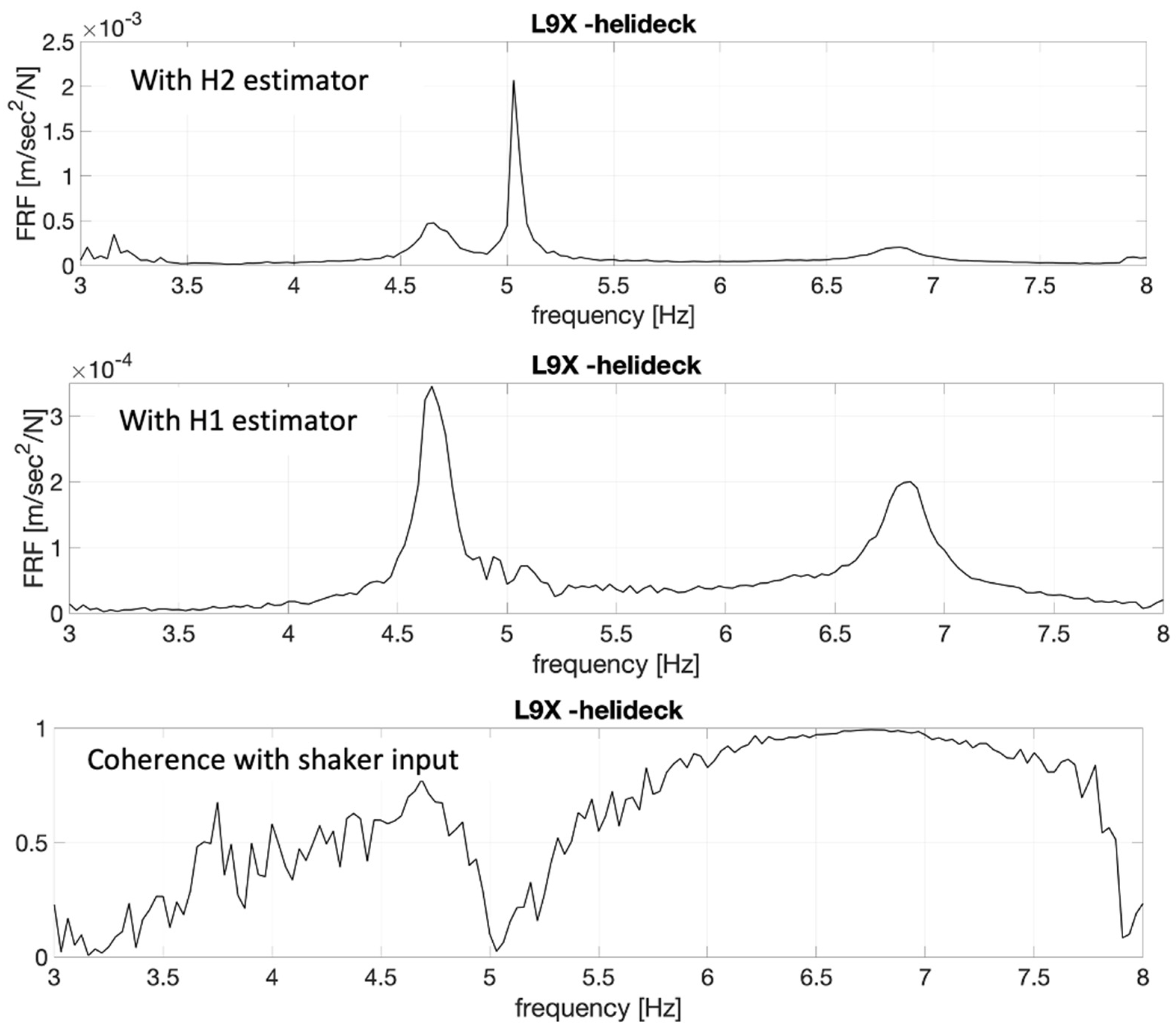
3.1. Forced Vibration Test
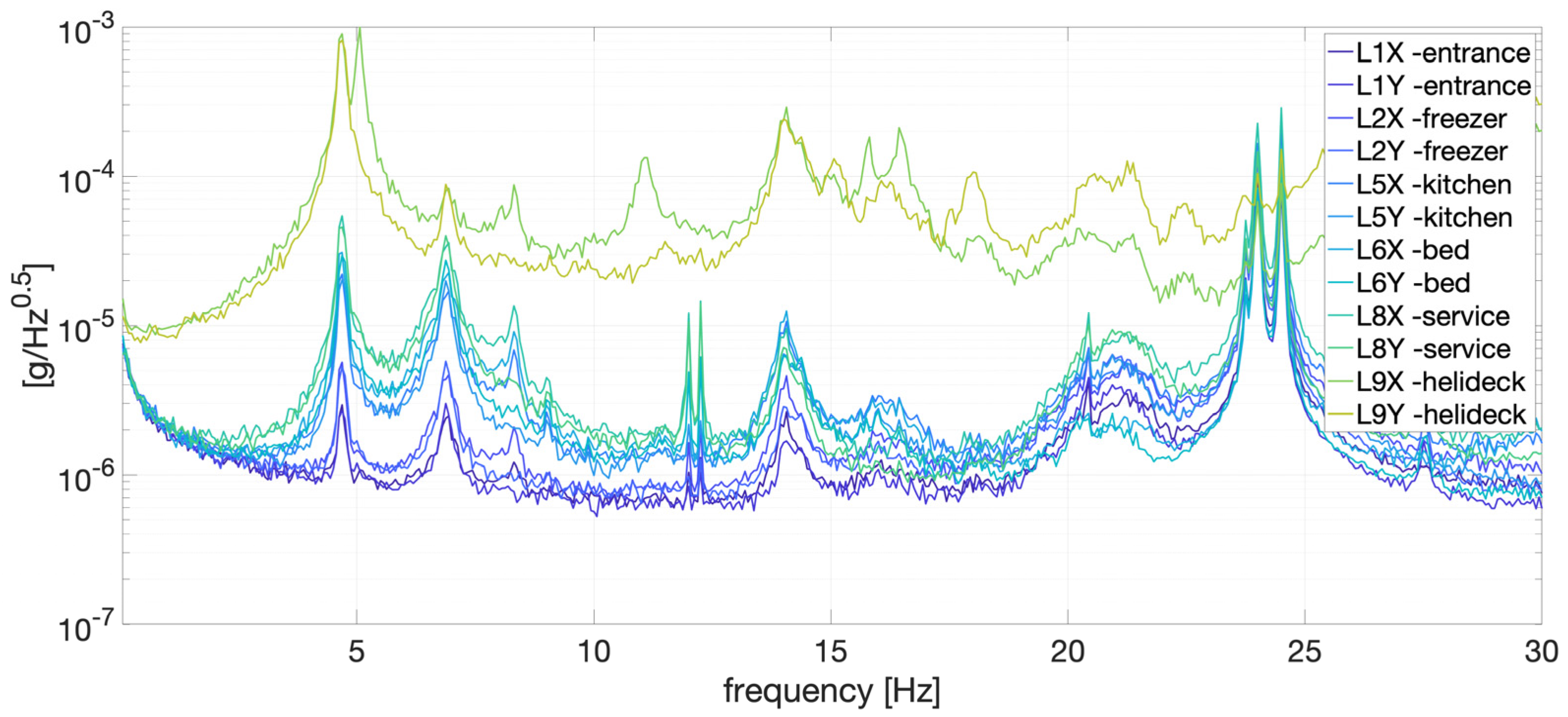
3.2. Ambient Vibration Survey
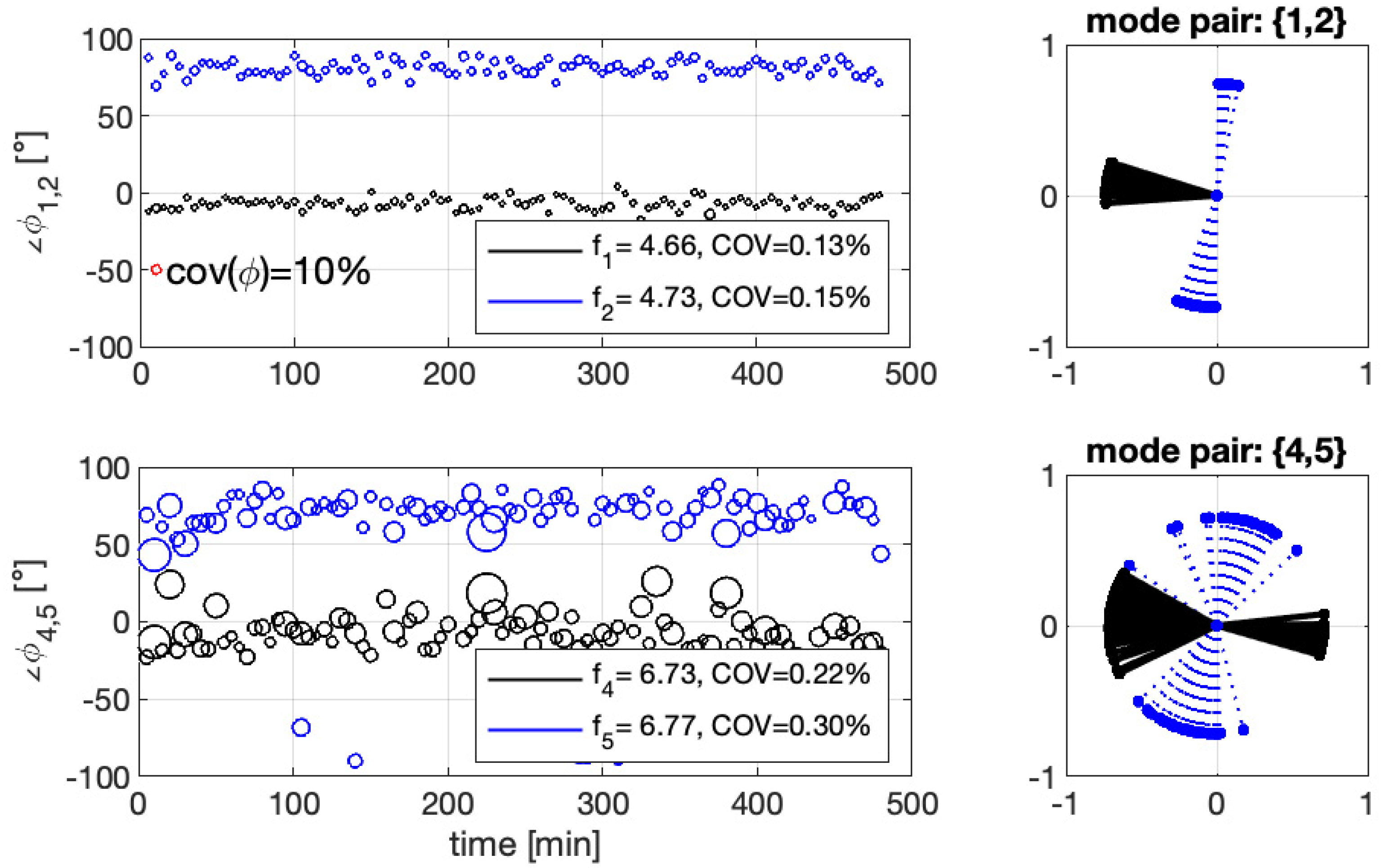
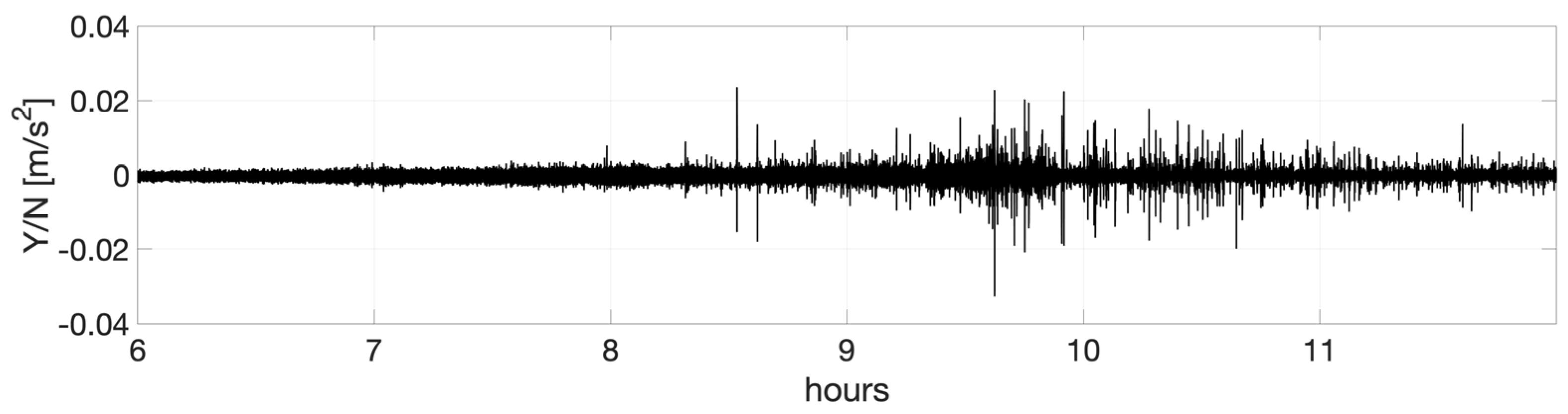
4. Monitoring
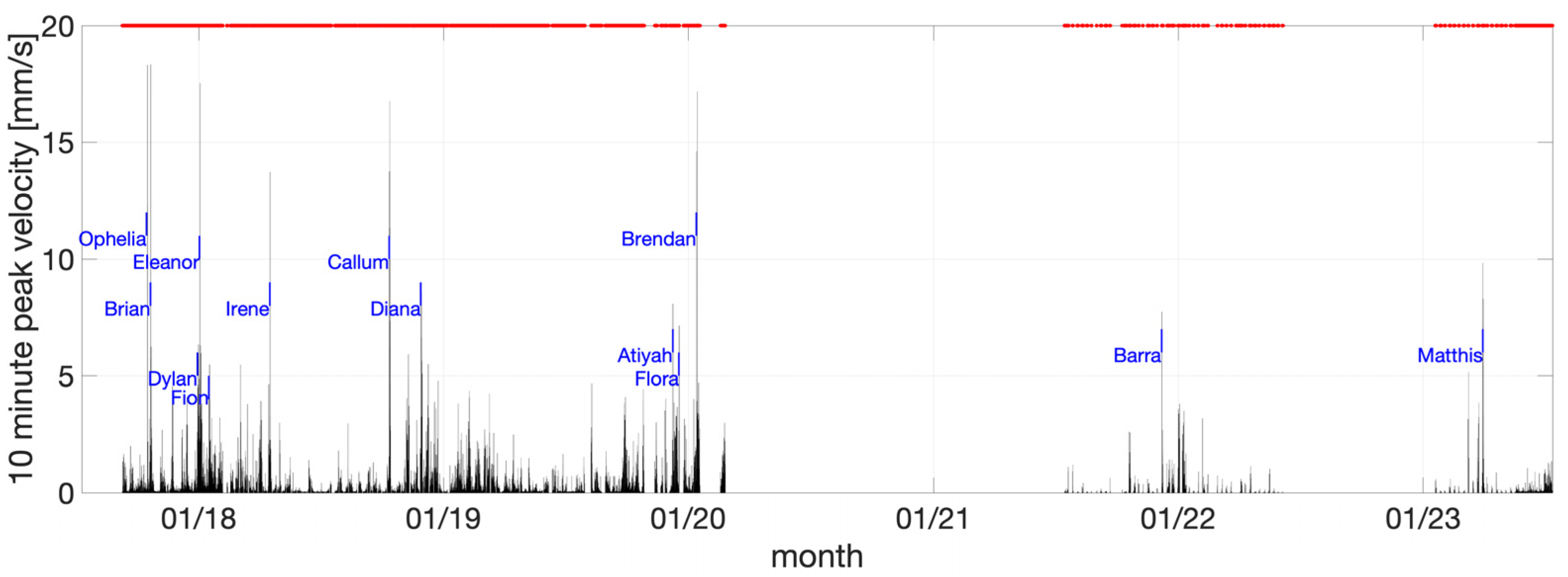
4.1. Wave Loading during Storms
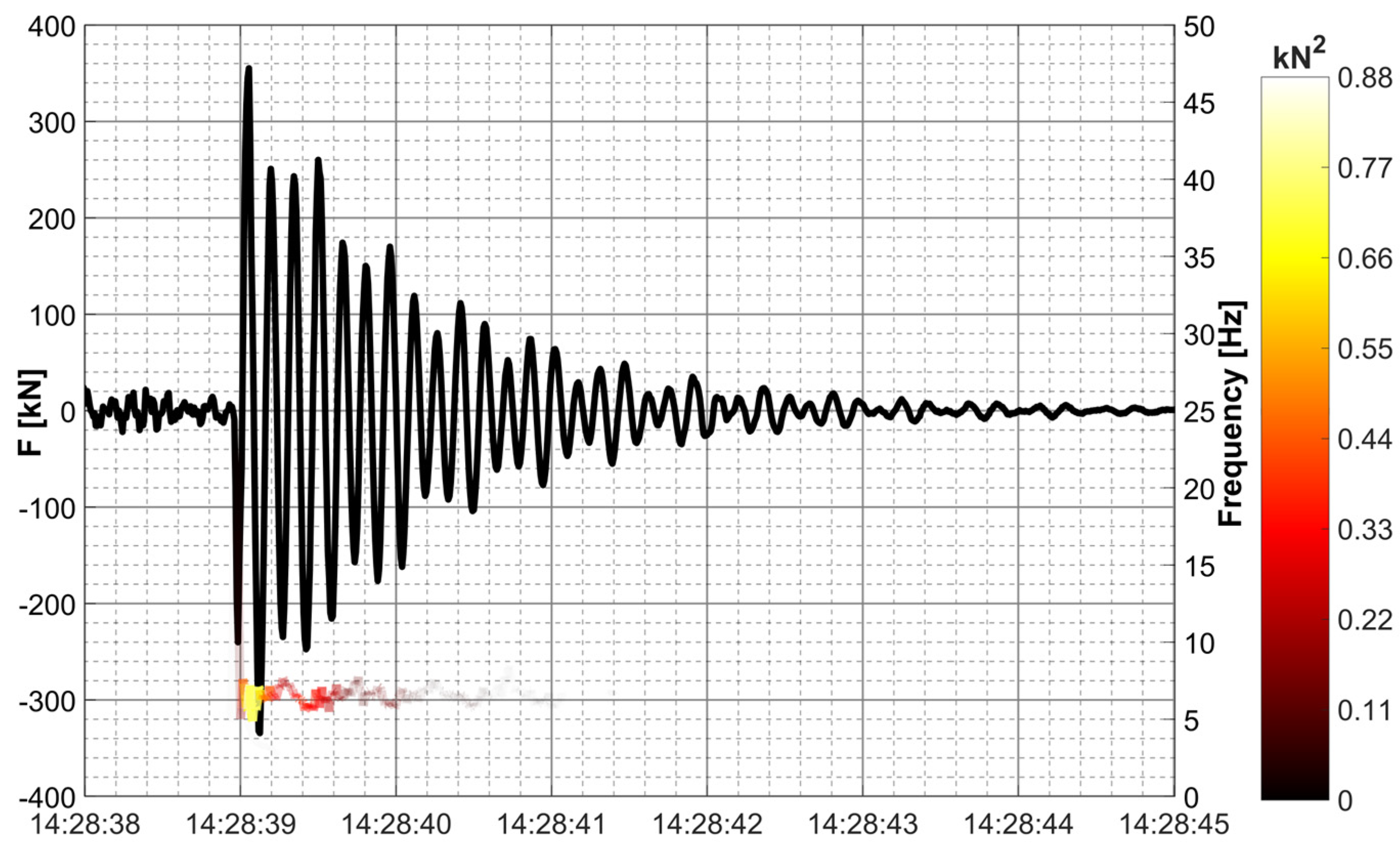
4.2. Helicopter Operations
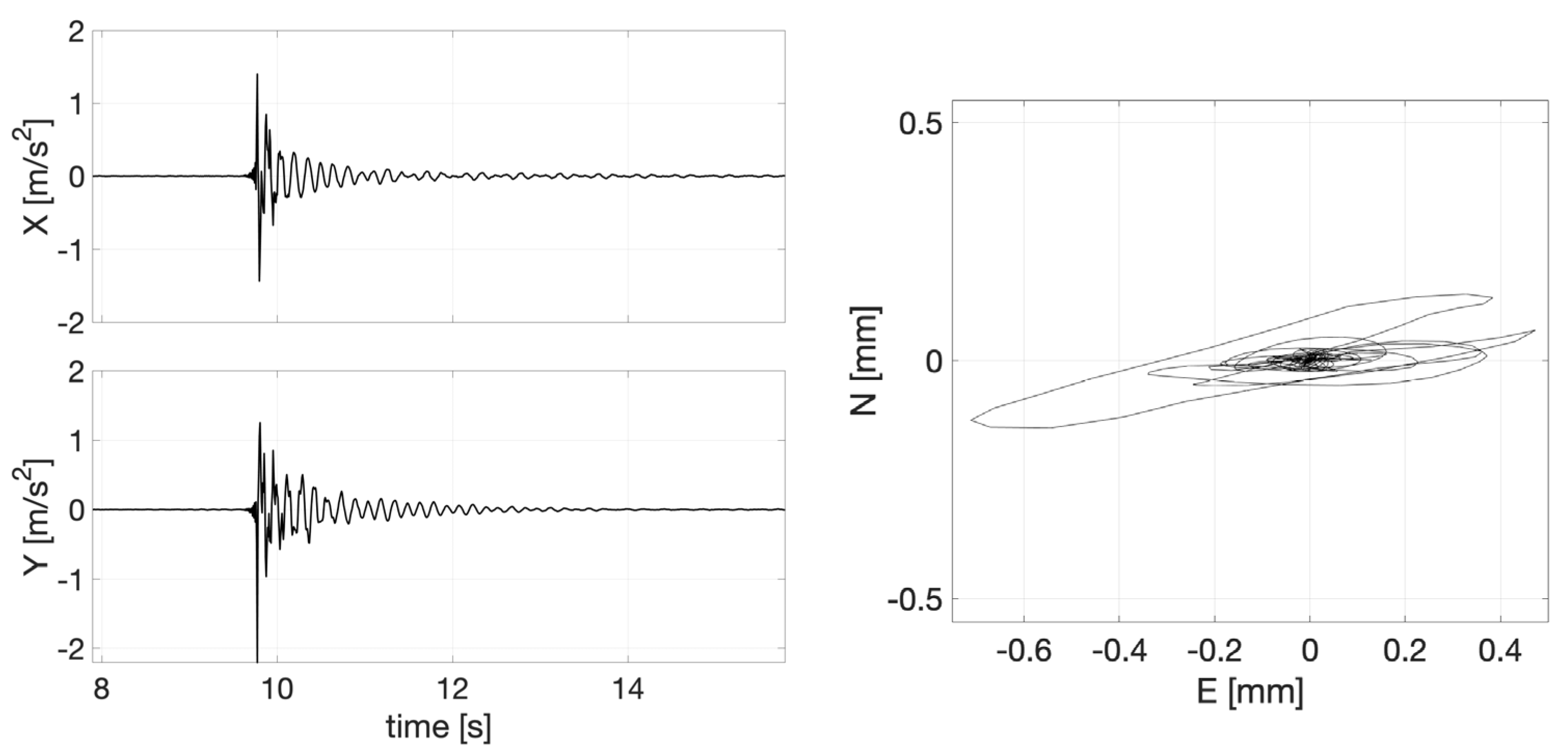
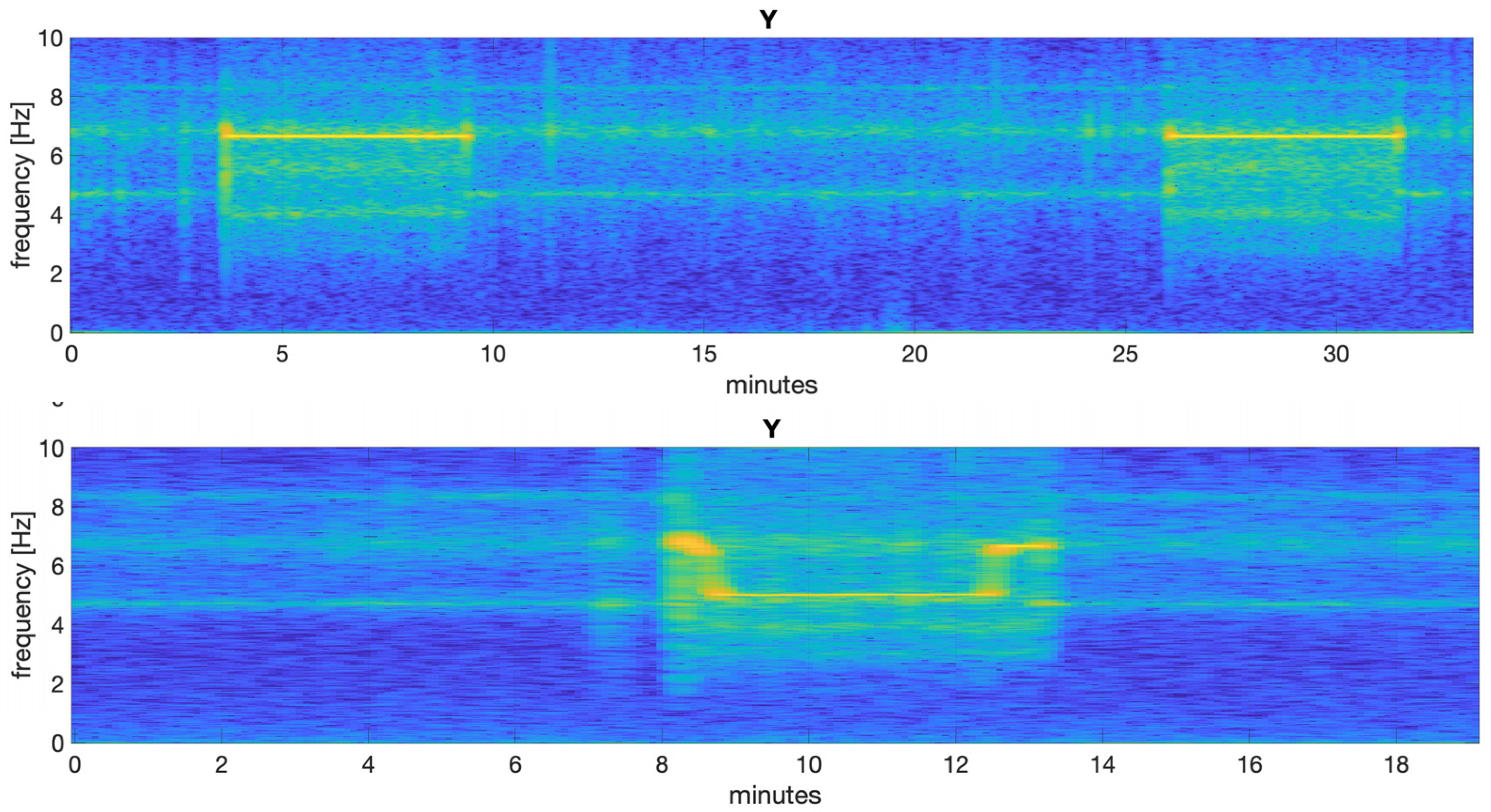
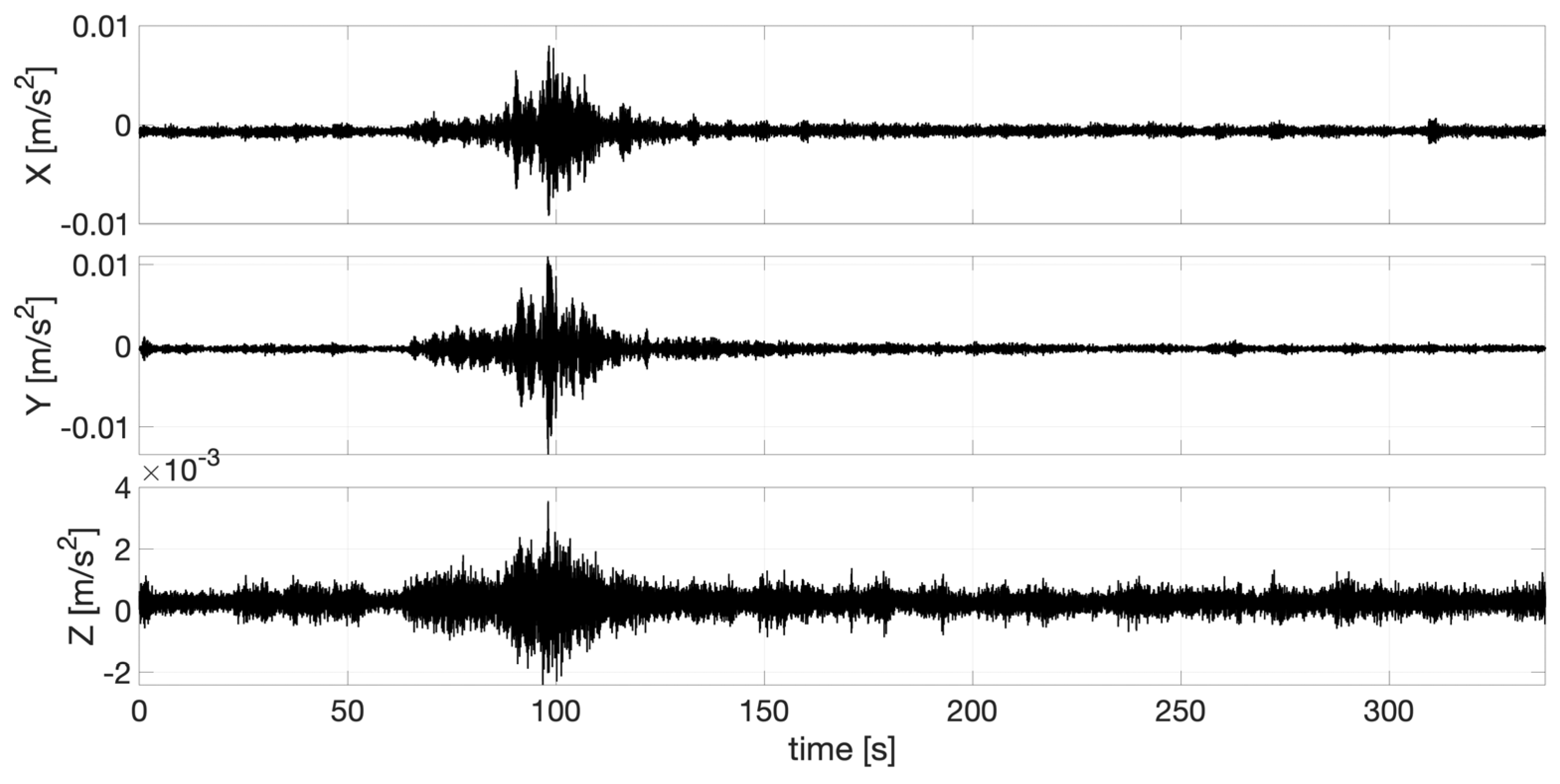
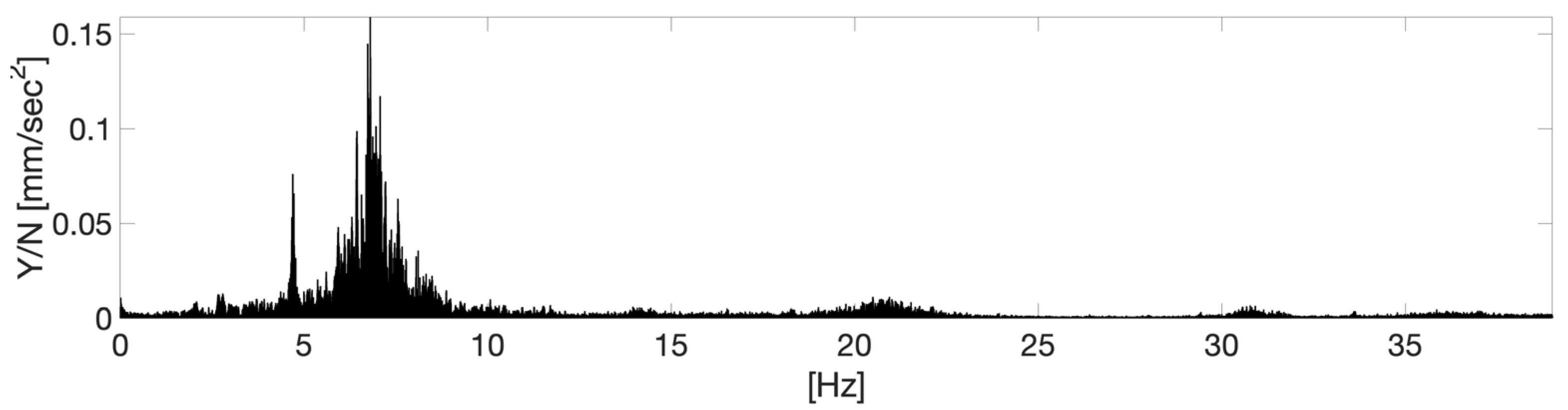
4.3. Earthquake
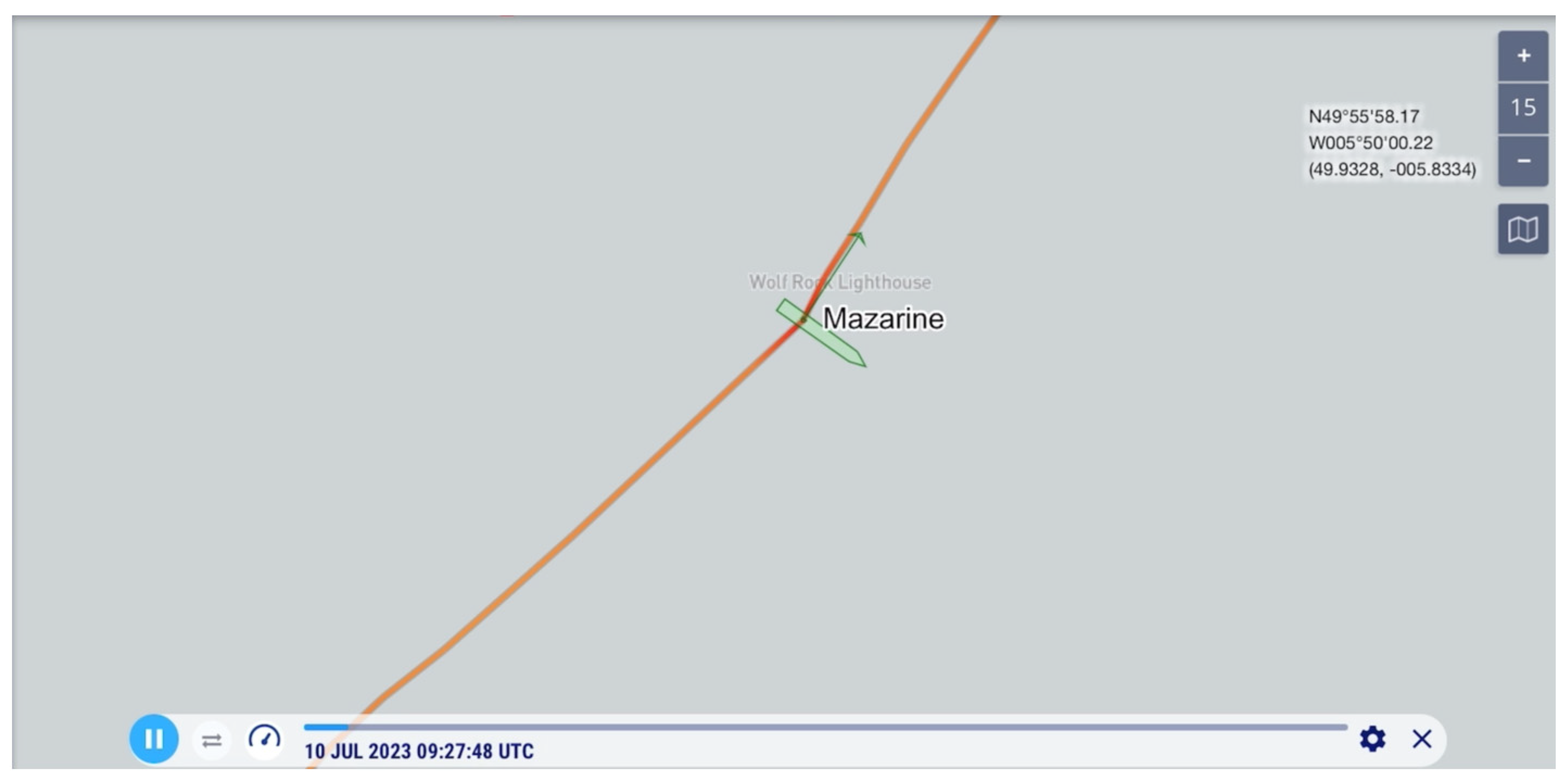
4.4. Ship Grounding
4.5. Vibration Monitoring of Other European Lighthouses
5. Final Comments
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Turner, N.M. Traditional Aids to Navigation: The Next 25 Years. J. Navig. 1997, 50, 234. [Google Scholar] [CrossRef]
- Douglass, W.T.; Webb, S.; Owen, G.W.; Redman, J.B.; Beazeley, M.; Harcourt, L.F.V.; Williams, P.; Inglis, J.C.; Rawlinson, S.R.; Brunton, R.H.; et al. Discussion. The new Eddystone Lighthouse. Minutes Proc. Inst. Civ. Eng. 1884, 75, 37–56. [Google Scholar] [CrossRef]
- Douglass, J.N. Note on the Eddystone Lighthouse. Minutes Proc. 1878, 53, 247–248. [Google Scholar] [CrossRef]
- Raby, A.; Bullock, G.N.; Banfi, D.; Rafiq, Y.; Cali, F. Wave loading on rock lighthouses. Proc. Inst. Civ. Eng. Marit. Eng. 2016, 169, 15–28. [Google Scholar] [CrossRef]
- Trinity House, Eddystone Lighthouse, (n.d.). Available online: https://www.trinityhouse.co.uk/lighthouses-and-lightvessels/eddystone-lighthouse (accessed on 21 February 2024).
- Douglass, W.T. The Bishop Rock Lighthouses. (Includes Plates). Minutes Proc. Inst. Civ. Eng. 1892, 108, 207–220. [Google Scholar] [CrossRef]
- Trinity House, Bishop Rock Lighthouse, (n.d.). Available online: https://www.trinityhouse.co.uk/lighthouses-and-lightvessels/bishop-rock-lighthouse (accessed on 21 February 2024).
- Stevenson, A. Account of the Skerryvore Lighthouse with Notes on the Illumination of Lighthouses; Adam and Charles Black: London, UK, 1848. [Google Scholar]
- Raby, A. STORMLAMP—STructural Behaviour of Rock Mounted Lighthouses at the Mercy of Impulsive Waves, (n.d.) EP/N022955/1. Available online: https://gow.epsrc.ukri.org/NGBOViewGrant.aspx?GrantRef=EP/N022947/1 (accessed on 26 March 2024).
- Douglass, J.N. The Wolf Rock Lighthouse. (Includes Plates). Minutes Proc. Inst. Civ. Eng. 1870, 30, 1–16. [Google Scholar] [CrossRef]
- Brownjohn, J.M.W.; Raby, A.; Bassitt, J.; Antonini, A.; Hudson, E.; Dobson, P. Experimental modal analysis of British rock lighthouses. Mar. Struct. 2018, 62, 1–22. [Google Scholar] [CrossRef]
- Pappas, A.; D’Ayala, D.; Antonini, A.; Brownjohn, J.M.W.; Raby, A. Numerical modelling of Fastnet Lighthouse based on experimental dynamic identification. In Proceedings of the ICACMS 2017 International Conference on Advances in Construction Materials and Systems, RILEM, Chennai, India, 3–8 September 2017. [Google Scholar]
- Au, S.-K.; Brownjohn, J.M.W.; Li, B.; Raby, A. Understanding and managing identification uncertainty of close modes in operational modal analysis. Mech. Syst. Signal Process. 2021, 147, 107018. [Google Scholar] [CrossRef]
- Zivanovic, S.; Pavic, A.; Reynolds, P. Modal testing and FE model tuning of a lively footbridge structure. Eng. Struct. 2006, 28, 857–868. [Google Scholar] [CrossRef]
- Pavic, A.; Reynolds, P. Modal testing and dynamic FE model correlation and updating of a prototype high-strength concrete floor. Cem. Concr. Compos. 2003, 25, 787–799. [Google Scholar] [CrossRef]
- Reynolds, P.; Pavic, A.; Carr, J. Experimental dynamic analysis of the Kingston Communications Stadium. Struct. Eng. 2007, 85, 33–39. [Google Scholar]
- Bouwkamp, J.G.; Stephen, R.M. Ambient and Forced Vibration Studies of a Multistory Pyramid-Shaped Building; Rome, Italy. 1974, pp. 260–269. Available online: https://www.iitk.ac.in/nicee/wcee/article/5_vol1_260.pdf (accessed on 15 April 2024).
- Da Rin, E.M. Dynamic structural testing of Torre Valdaliga Nord power plant 250m high multi-flue chimney. In Proceedings of the XXV CICIND Meeting, Venice, Spain, April 1986. [Google Scholar]
- Peeters, B. Reference based stochastic subspace identification for output-only modal analysis. Mech. Syst. Signal Process. 1999, 13, 855–878. [Google Scholar] [CrossRef]
- Brownjohn, J.M.W. Ambient vibration studies for system identification of tall buildings. Earthq. Eng. Struct. Dyn. 2003, 32, 71–95. [Google Scholar] [CrossRef]
- Ewins, D.J. Modal Testing: Theory and Practice; Research Studies Press Ltd.: Hertfordshire, UK; John Wiley and Sons Inc.: Taunton, UK, 1984. [Google Scholar]
- Formenti, D.L.; Richardson, M.H.; Formenti, D.L. Parameter estimation from frequency response measurements using rational fraction polynomials. In Proceedings of the 1st IMAC Conference, Orlando, FL, USA, 8–10 November 1982; pp. 167–181. [Google Scholar]
- Au, S.K. Operational Modal Analysis: Modeling, Bayesian Inference, Uncertainty Laws; Springer Nature: Singapore, 2017. [Google Scholar]
- De Wire, E. England’s Great Storm. Weatherwise 1996, 49, 34–38. [Google Scholar] [CrossRef]
- Antonini, A.; Brownjohn, J.M.W.; Dassanayake, D.; Raby, A.; Bassit, J.; Pappas, A.; D’Ayala, D. A Bayesian inverse dynamic approach for impulsive wave loading reconstruction: Theory, laboratory and field application. Coast. Eng. 2021, 168, 103920. [Google Scholar] [CrossRef]
- Raby, A.C.; Antonini, A.; Pappas, A.; Dassanayake, D.T.; Brownjohn, J.M.W.; D’Ayala, D. Wolf Rock lighthouse: Past developments and future survivability under wave loading. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2019, 377, 20190027. [Google Scholar] [CrossRef] [PubMed]
- Pappas, A.; D’Ayala, D.; Dassanayake, D.T.; Antonini, A.; Raby, A. Rocking of offshore lighthouses under extreme wave impacts: Limit analysis, analytic formulations and distinct element method. Eng. Struct. 2021, 228, 111534. [Google Scholar] [CrossRef]
- Khosroshahi, S.F.; Masina, M.; Antonini, A.; Ransley, E.; Brownjohn, J.M.W.; Dobson, P.; D’Ayala, D. A Multidisciplinary Computational Framework for Topology Optimisation of Offshore Helidecks. J. Mar. Sci. Eng. 2022, 10, 1180. [Google Scholar] [CrossRef]
- Galloway, D.D. Bulletin of British Earthquakes 2018; Report OR/19/002; UKRI: Edinburgh, Scotland, 2019. [Google Scholar]
- Antonini, A.; Raby, A.; Brownjohn, J.M.W.; Pappas, A.; D’Ayala, D. Survivability assessment of fastnet lighthouse. Coast. Eng. 2019, 150, 18–38. [Google Scholar] [CrossRef]
- Nord, T.S.; Øiseth, O.; Lourens, E.-M. Ice force identification on the Norströmsgrund lighthouse. Comput. Struct. 2016, 169, 24–39. [Google Scholar] [CrossRef]
- Guichard, J. La Jument, (n.d.). Available online: https://www.jean-guichard.com/en/photo/france/phares-de-france/la-jument-9 (accessed on 24 March 2024).
- Denarié, E.; Fady, N. Structural response of French offshore heritage lighthouses. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2019, 377, 20190011. [Google Scholar] [CrossRef] [PubMed]
- Filipot, J.-F.; Guimaraes, P.; Leckler, F.; Hortsmann, J.; Carrasco, R.; Leroy, E.; Fady, N.; Accensi, M.; Prevosto, M.; Duarte, R.; et al. La Jument lighthouse: A real-scale laboratory for the study of giant waves and their loading on marine structures. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2019, 377, 20190008. [Google Scholar] [CrossRef] [PubMed]
- Cheung, M.S.; Tadros, G.S.; Brown, T.; Dilger, W.H.; Ghali, A.; Lau, D.T. Field monitoring and research on performance of the Confederation Bridge. Can. J. Civ. Eng. 1997, 24, 951–962. [Google Scholar] [CrossRef]






| Run | Start | Duration | Locations | Shaker | Excitation | Comments |
|---|---|---|---|---|---|---|
| 2 | 12:35 | 784 s | 1, 2, 5, 6, 8, 9 | - | Ambient | Peaks at 4.7, 5.1, 6.8 Hz |
| 5 | 12:55 | 624 s | “ | x | Random 3–30 Hz | Peaks at 4.66, 5.06, 5.56, 6.94 Hz |
| 6 | 13:10 | 960 s | “ | x | Sweep 3–30 Hz | Good quality broadband |
| 8 | 13:30 | 576 s | “ | x | Sweep 3.4–8 Hz | Shaker cut out, 215 s |
| 9 | 13:45 | 336 s | “ | x | Sweep 3.4–8 Hz | Reduce shaker power, still cut out, 290 s. First setup |
| 10 | 14:20 | 144 s | “ | y | “ | Shaker cut out, 70 s |
| 12 | 14:30 | 384 s | “ | y | “ | Shaker cut out, 370 s. Second setup |
| 13 | 14:40 | 432 s | “ | y | “ | Shaker signal shorter than run 12 |
| 14 | 15:10 | 960 s | 3, 4, 6, 7, 8, 9 | - | Ambient | Large impulse after 12 min |
| 15 | 15:24 | 352 s | “ | y | Sweep 3.4–8 Hz | Shaker cut out, 330 s |
| 16 | 15:40 | 352 s | “ | y | “ | Shaker cut out, 270 s |
| 17 | 15:50 | 928 s | “ | y | “ | Perfect. Third setup |
| 18 | 16:10 | 624 s | “ | x | “ | Shaker cut out at 570 s, out of time. Fourth setup |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brownjohn, J.; Raby, A.; Bassitt, J.; Antonini, A.; Zhu, Z.; Dobson, P. Wolf Rock Lighthouse Long-Term Monitoring. Infrastructures 2024, 9, 77. https://doi.org/10.3390/infrastructures9040077
Brownjohn J, Raby A, Bassitt J, Antonini A, Zhu Z, Dobson P. Wolf Rock Lighthouse Long-Term Monitoring. Infrastructures. 2024; 9(4):77. https://doi.org/10.3390/infrastructures9040077
Chicago/Turabian StyleBrownjohn, James, Alison Raby, James Bassitt, Alessandro Antonini, Zuo Zhu, and Peter Dobson. 2024. "Wolf Rock Lighthouse Long-Term Monitoring" Infrastructures 9, no. 4: 77. https://doi.org/10.3390/infrastructures9040077
APA StyleBrownjohn, J., Raby, A., Bassitt, J., Antonini, A., Zhu, Z., & Dobson, P. (2024). Wolf Rock Lighthouse Long-Term Monitoring. Infrastructures, 9(4), 77. https://doi.org/10.3390/infrastructures9040077







