A New Water Film Depth Prediction Model for Pavement Surface Drainage
Abstract
1. Introduction
| Source | Equation Form |
|---|---|
| Ross and Russam (RRL) [14] | |
| Gallaway [15] | |
| Wambold [16] | (L = 11) |
| John Anderson [17] | |
| New Zealand modified [18] | |
| Empirical PAVDRN [20] | |
| J. Luo [28] | |
| M. Kane [12] | |
| Two-dimensional shallow water-governing equation [23] | |
| W. Luo [26] | |
| K. Wang [27] |
2. Intervening Factors on Pavement WFD
2.1. Drainage Length (L)
2.2. Rainfall Intensity (I)
2.3. Road Surface Gradient (i)
2.4. Initial Water Film Depth (h0)
2.5. Physical Parameters of Raindrops
3. Methodology
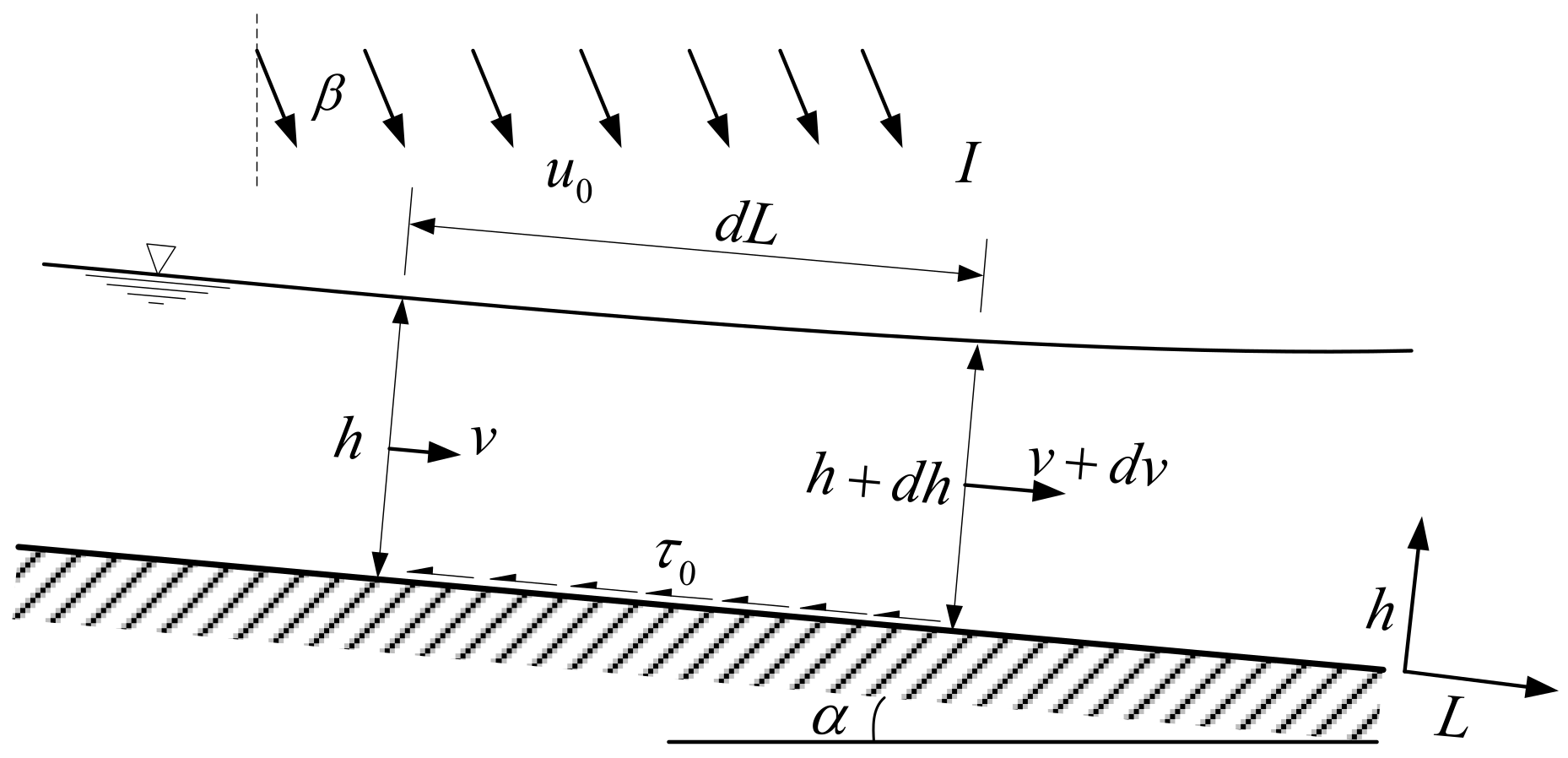
3.1. Theoretical Basis
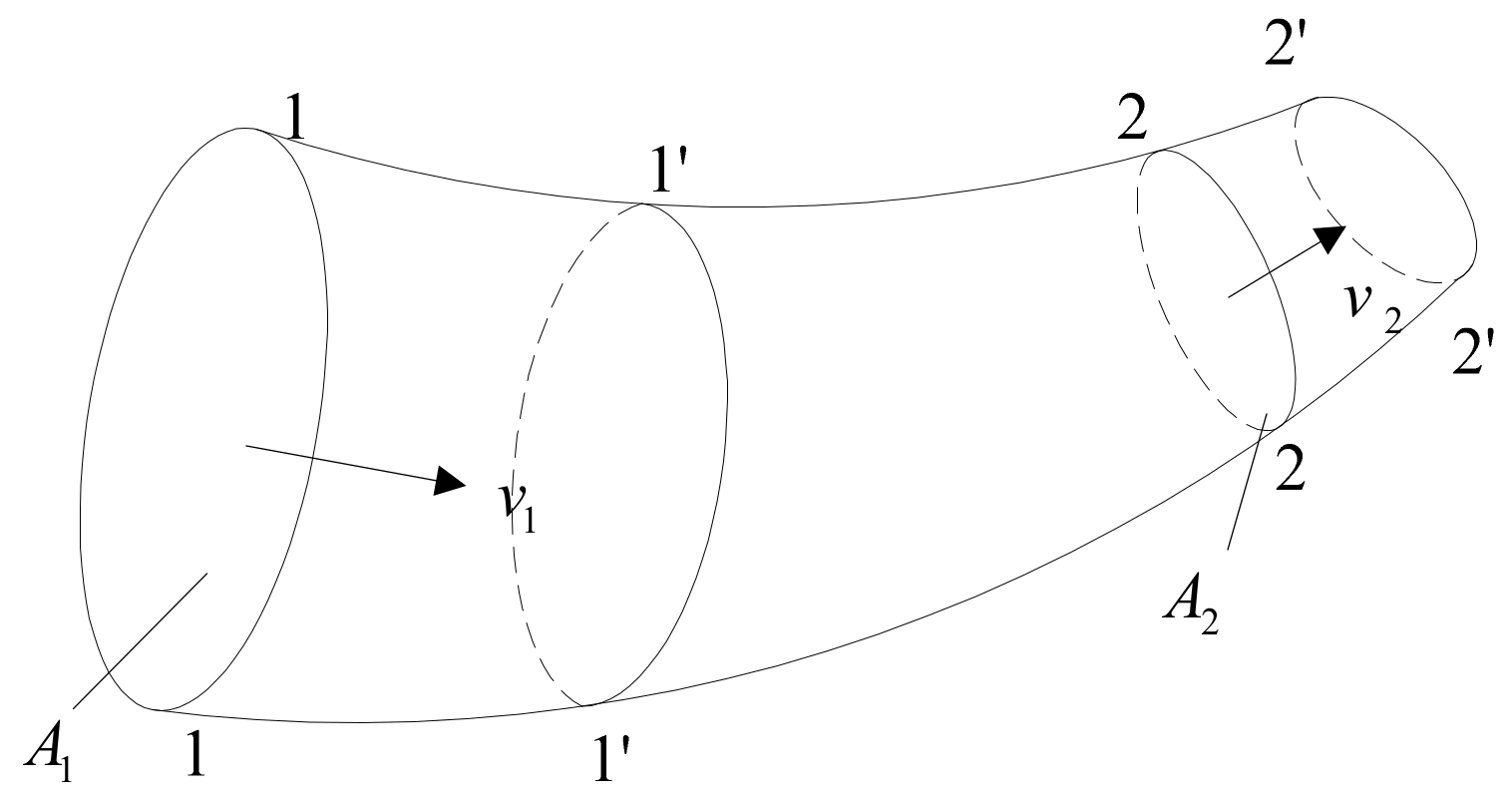
3.1.1. Conservation of Mass Equation
3.1.2. The Momentum Equation for Steady Total Flow
3.2. New WFD Model
4. Results and Discussion
4.1. Parametric Analysis
4.1.1. Relationship between WFD and Drainage Length of Road Surface
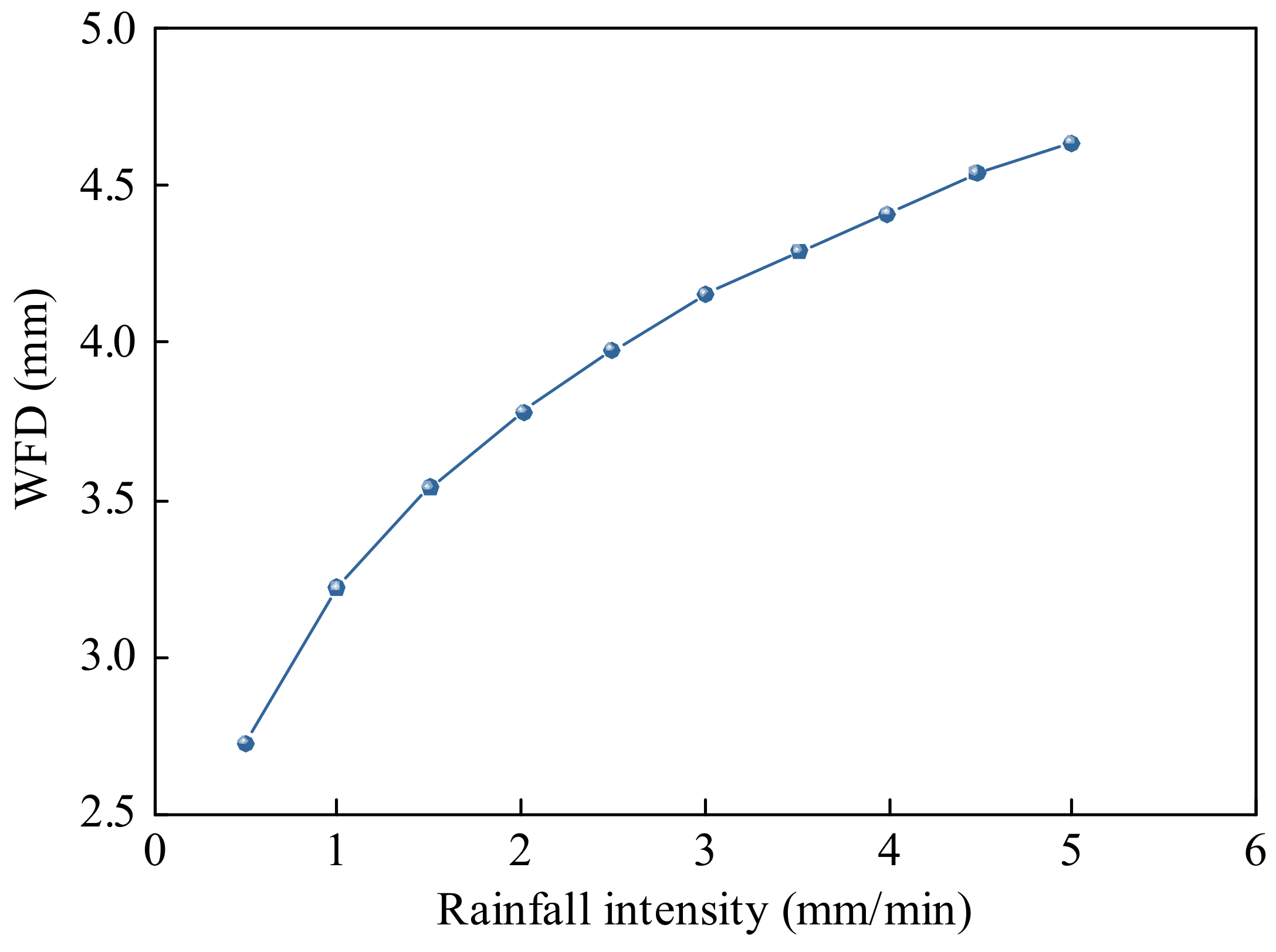
4.1.2. Relationship between WFD and Rainfall Intensity on Road Surface
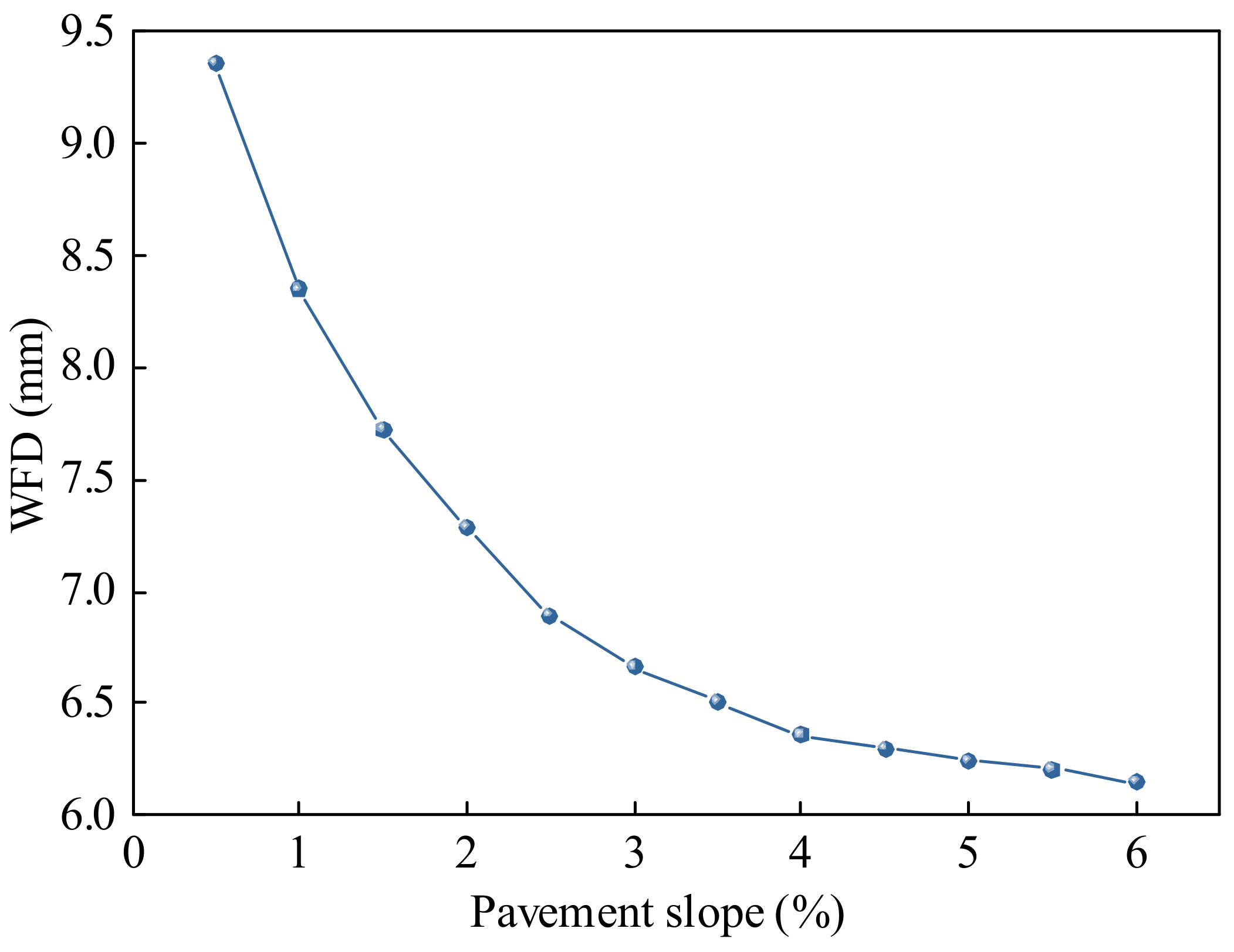
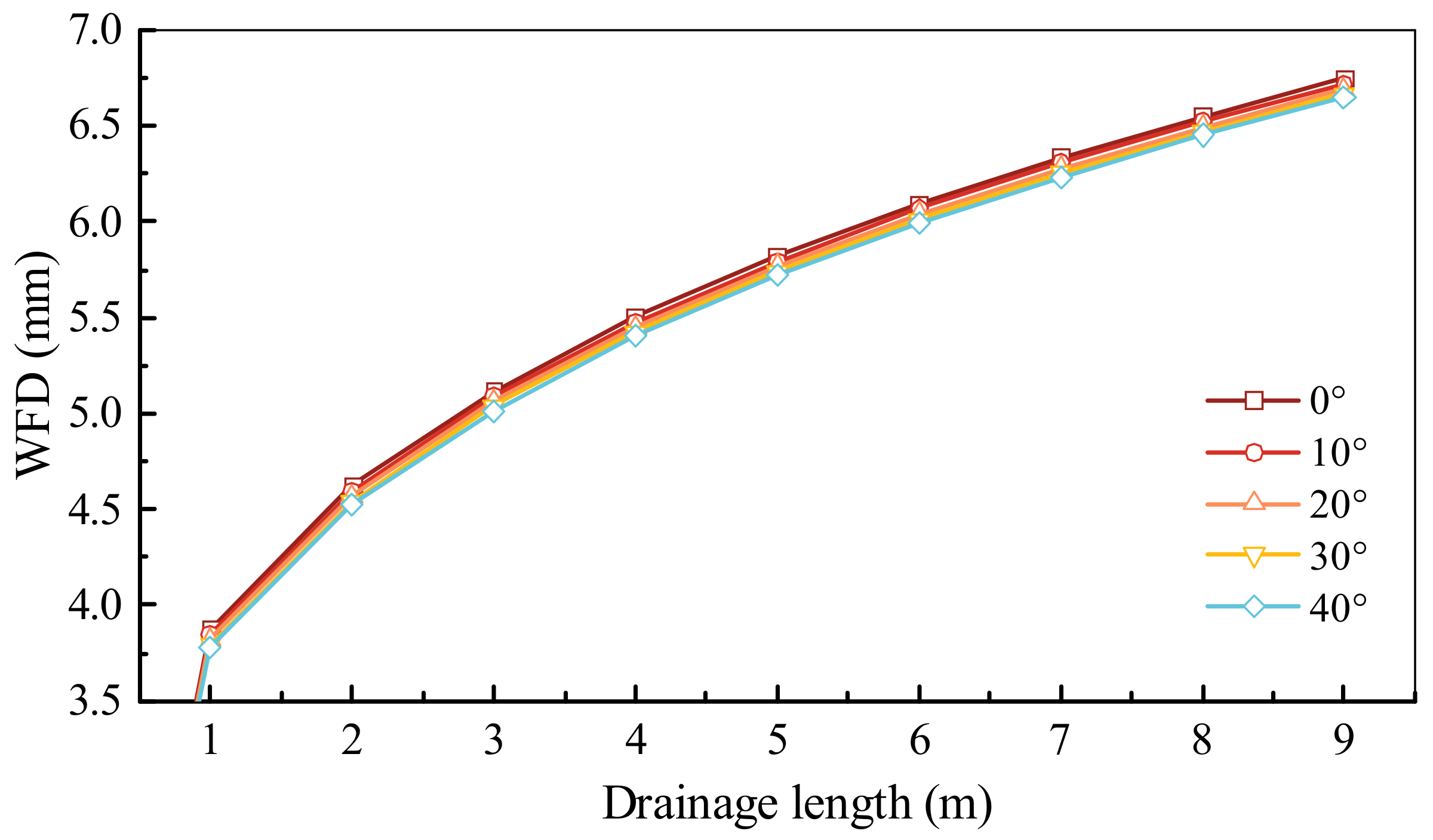
4.1.3. Relationship between WFD and Road Slope
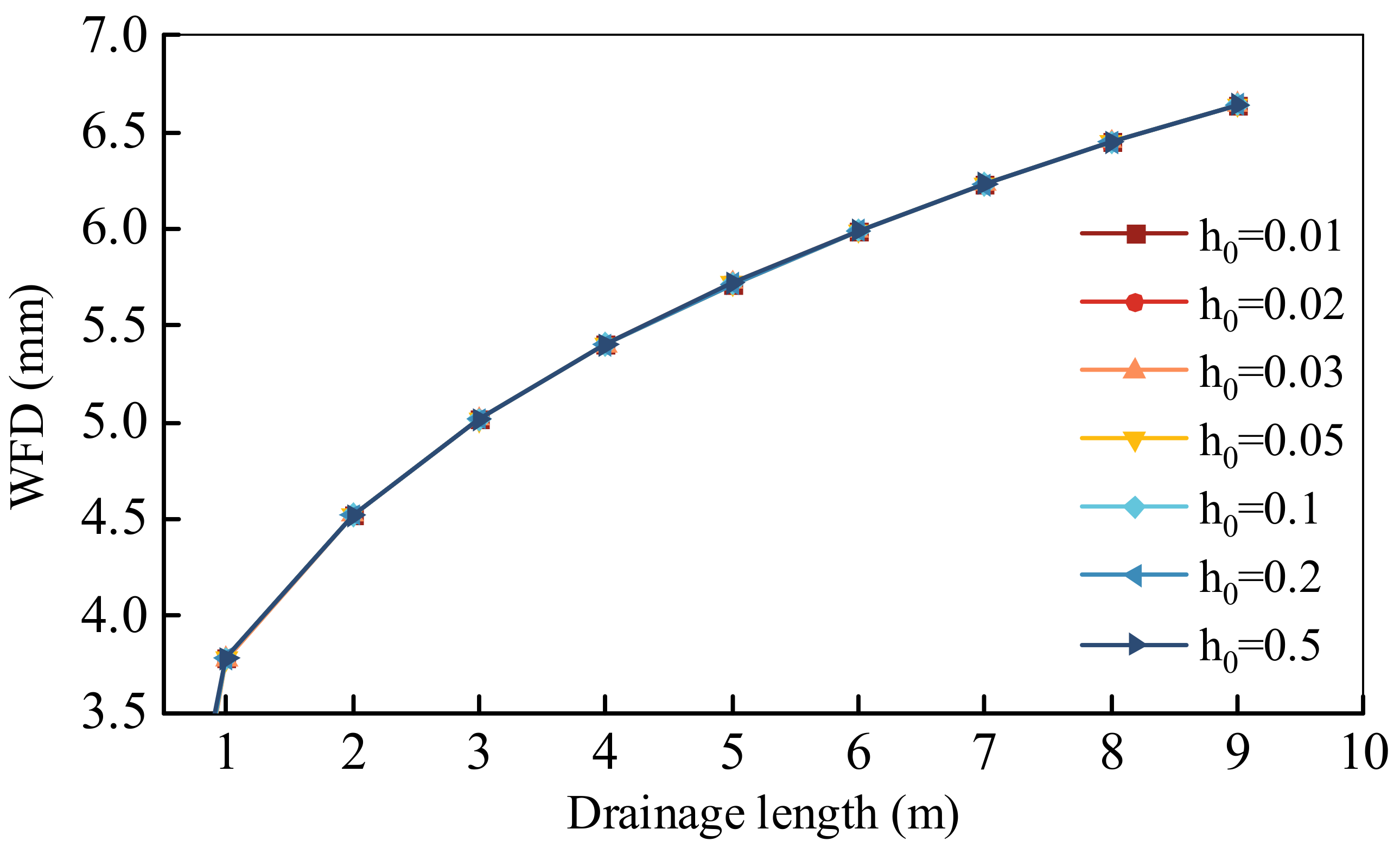
4.1.4. Relationship between WFD and the Initial Depth of Water Film
4.1.5. Relationship between WFD and Physical Parameters of Raindrops
4.2. Classic Empirical WFD Models
4.3. Comparison of Predictive Models
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Spitzhüttl, F.; Goizet, F.; Unger, T.; Biesse, F. The real impact of full hydroplaning on driving safety. Accid. Anal. Prev. 2020, 138, 105458. [Google Scholar] [CrossRef]
- Kassem, E.; Awed, A.; Masad, E.A.; Little, D.N. Development of predictive model for skid loss of asphalt pavements. Transp. Res. Rec. 2013, 2372, 83–96. [Google Scholar] [CrossRef]
- Li, Q.; Wang, J.; Lu, Y.; Zhou, X.; Hu, Y.; Sun, J.; Fu, G. Effect of dynamic water pressure on the adhesion behavior of recycled asphalt-aggregate interface by molecular dynamics method. Constr. Build. Mater. 2023, 382, 131296. [Google Scholar] [CrossRef]
- Wilson, D.J. The Effect of Rainfall and Contaminants on Road Pavement Skid Resistance; NZ Transport Agency: Wellington, New Zealand, 2013; p. 237. [Google Scholar]
- Zhao, K.; Song, S.; Wei, Y.; Li, G.; Guo, F. Adhesion properties of recycled high-viscosity asphalt–aggregate interface under dynamic water erosion. Materials 2023, 16, 6203. [Google Scholar] [CrossRef]
- Kogbara, R.B.; Masad, E.A.; Kassem, E.; Scarpas, A.T.; Anupam, K. A state-of-the-art review of parameters influencing measurement and modeling of skid resistance of asphalt pavements. Constr. Build. Mater. 2016, 114, 602–617. [Google Scholar] [CrossRef]
- Lee, H.S.; Carvajal, M.; Holzschuher, C.; Choubane, B. Florida department of transportation’s enhanced hydroplaning prediction tool. Transp. Res. Rec. 2021, 2675, 340–352. [Google Scholar] [CrossRef]
- Li, Q.; Wang, J.; Song, S.; Wang, R.; Jiang, J.; Yan, C. Study on the adhesion characteristics of asphalt-aggregate interface in cold recycled asphalt mixtures. J. Mater. Civ. Eng. 2023, 35, 04023283. [Google Scholar] [CrossRef]
- Cavdar, S.; Uyumaz, A. Hydrological considerations in designing roadways: Avoiding hydroplaning. Tek. Dergi 2022, 33, 12663–12676. [Google Scholar] [CrossRef]
- Cavdar, S.; Uyumaz, A. Impact of slope orientation on inlet spacing: Gutter flow analyses. Appl. Sci. 2022, 12, 11196. [Google Scholar] [CrossRef]
- Chen, X.; Wang, H. Analysis and mitigation of hydroplaning risk considering spatial-temporal water condition on the pavement surface. Int. J. Pavement Eng. 2023, 24, 2036988. [Google Scholar] [CrossRef]
- Kane, M.; Do, M.T. Kinematic wave approach to model water depth on road surfaces during and after rainfall events. Int. J. Pavement Eng. 2012, 13, 385–394. [Google Scholar] [CrossRef]
- Liu, X.; Cao, Q.; Wang, H.; Chen, J.; Huang, X. Evaluation of vehicle braking performance on wet pavement surface using an integrated tire-vehicle modeling approach. Transp. Res. Rec. 2019, 2673, 295–307. [Google Scholar] [CrossRef]
- Ross, N.; Russam, K. The depth of rain water on road surfaces. Road Research Laboratory: Crowthorne, UK, 1968. [Google Scholar]
- Gallaway, B.M.; Schiller, R.E.; Rose, J.G. The Effects of Rainfall Intensity, Pavement Cross Slope, Surface Texture, and Drainage Length on Pavement Water Depths; Federal Highway Administration Report N8138-5; Federal Highway Administration: Washington, DC, USA, 1971; p. 69. [Google Scholar]
- Wambold, J.C.; Henry, J.J.; Hegmon, R.R. Evaluation of pavement surface texture significance and measurement techniques. Wear 1982, 83, 351–368. [Google Scholar] [CrossRef]
- Anderson, J. Depth of rain water on road surfaces. Highw. Transp. 1995, 42, 45–49. [Google Scholar]
- Chesterton, J.; Nancekivell, N.; Tunnicliffe, N. The use of the Gallaway formula for aquaplaning evaluation in New Zealand. Transp. Purs. Excell. 2006, 8, 1–22. [Google Scholar]
- Jayasooriya, W.; Gunaratne, M. Evaluation of widely used hydroplaning risk prediction methods using Florida’s past crash data. Transp. Res. Rec. 2014, 2457, 140–150. [Google Scholar] [CrossRef]
- Huebner, R.S.; Anderson, D.A.; Warner, J.C.; Reed, J.R. Pavdrn: Computer model for predicting water film thickness and potential for hydroplaning on new and reconditioned pavements. Transp. Res. Rec. 1997, 1599, 128–131. [Google Scholar] [CrossRef]
- Pourhassan, A.; Gheni, A.A.; ElGawady, M.A. Water film depth prediction model for highly textured pavement surface drainage. Transp. Res. Rec. 2022, 2676, 100–117. [Google Scholar] [CrossRef]
- Kim, S.; Lee, J.; Yoon, T. Road surface conditions forecasting in rainy weather using artificial neural networks. Saf. Sci. 2021, 140, 105302. [Google Scholar] [CrossRef]
- Hubbard, M.; Baines, M. Conservative multidimensional upwinding for the steady two-dimensional shallow water equations. J. Comput. Phys. 1997, 138, 419–448. [Google Scholar] [CrossRef]
- Yaolu, M.; Yanfen, G.; Xianhua, C.; Yankun, L. Prediction for asphalt pavement water film thickness based on artificial neural network. J. Southeast Univ. Engl. Ed. 2017, 33, 490–495. [Google Scholar]
- Alber, S.; Schuck, B.; Ressel, W.; Behnke, R.; Canon Falla, G.; Kaliske, M.; Leischner, S.; Wellner, F. Modeling of surface drainage during the service life of asphalt pavements showing long-term rutting: A modular hydromechanical approach. Adv. Mater. Sci. Eng. 2020, 2020, 8793652. [Google Scholar] [CrossRef]
- Luo, W.; Li, L.; Wang, K.C.; Wei, C. Surface drainage evaluation of asphalt pavement using a new analytical water film depth model. Road Mater. Pavement Des. 2020, 21, 1985–2004. [Google Scholar] [CrossRef]
- Wang, K.; Li, P.; Ai, Q.; Wei, X. Study on water distribution characteristics of surface gathered water and water film thickness model. Alex. Eng. J. 2022, 61, 3293–3302. [Google Scholar] [CrossRef]
- Wang, R.; Wu, X.; Zhao, H.; Min, L.; Tao, Z.; Ji, X.; Luo, Y. Effects of erythropoietin combined with tissue plasminogen activator on the rats following cerebral ischemia and reperfusion. Brain Circ. 2016, 2, 54. [Google Scholar] [PubMed]
- Xiao, K.; Hui, B.; Qu, X.; Wang, H.; Diab, A.; Cao, M. Asphalt pavement water film thickness detection and prediction model: A review. J. Traffic Transp. Eng. Engl. Ed. 2023, 10, 349–367. [Google Scholar] [CrossRef]
- Xue, J.; Wang, J.; Yi, J.; Wei, Y.; Huang, K.; Ge, D.; Sun, R. Optimal parking path planning and parking space selection based on the entropy power method and bayesian network: A case study in an indoor parking lot. Sustainability 2023, 15, 8450. [Google Scholar] [CrossRef]
- Zhang, W.; Li, Q.; Wang, J.; Zhao, Z.; Jin, D. Physical and chemical evaluation of adhesion recovery property of aged high-viscosity asphalt based on specialized composite rejuvenators. J. Clean. Prod. 2023, 414, 137758. [Google Scholar] [CrossRef]
- Luo, W.; Li, L. Development of a new analytical water film depth (WFD) prediction model for asphalt pavement drainage evaluation. Constr. Build. Mater. 2019, 218, 530–542. [Google Scholar] [CrossRef]
- Luo, W.; Li, L. Estimation of water film depth for rutting pavement using imu and 3D laser imaging data. Int. J. Pavement Eng. 2021, 22, 1334–1349. [Google Scholar] [CrossRef]
- Luo, W.; Wang, K.C.; Li, L. Field test validation of water film depth (WFD) prediction models for pavement surface drainage. Int. J. Pavement Eng. 2019, 20, 1170–1181. [Google Scholar] [CrossRef]
- Serio, M.A.; Carollo, F.G.; Ferro, V. Raindrop size distribution and terminal velocity for rainfall erosivity studies. A review. J. Hydrol. 2019, 576, 210–228. [Google Scholar] [CrossRef]
- Kathiravelu, G.; Lucke, T.; Nichols, P. Rain drop measurement techniques: A review. Water 2016, 8, 29. [Google Scholar] [CrossRef]
- Edmar Schulz, H.; Edgar Curry, J.; Andrade Simões, A.L. Water films and hydroplaning on highways: Hydrodynamic aspects. J. Transp. Eng. Part B Pavements 2021, 147, 04021053. [Google Scholar] [CrossRef]
- Roth-Nebelsick, A.; Konrad, W.; Ebner, M.; Miranda, T.; Thielen, S.; Nebelsick, J.H. When rain collides with plants—Patterns and forces of drop impact and how leaves respond to them. J. Exp. Bot. 2022, 73, 1155–1175. [Google Scholar] [CrossRef] [PubMed]
- Angulo-Martínez, M.; Beguería, S.; Kyselý, J. Use of disdrometer data to evaluate the relationship of rainfall kinetic energy and intensity (KE-I). Sci. Total Environ. 2016, 568, 83–94. [Google Scholar] [CrossRef] [PubMed]
- Fox, N.I. The representation of rainfall drop-size distribution and kinetic energy. Hydrol. Earth Syst. Sci. 2004, 8, 1001–1007. [Google Scholar] [CrossRef]
- Sadeghi, S.H.; Abdollahi, Z.; Darvishan, A.K. Experimental comparison of some techniques for estimating natural raindrop size distribution on the south coast of the Caspian Sea, Iran. Hydrol. Sci. J. 2013, 58, 1374–1382. [Google Scholar] [CrossRef]




| Landing Altitude (m) | Landing Time (s) | Landing Speed (m/s) | Percentage of the Terminal Velocity (%) |
|---|---|---|---|
| 3.0 | 0.70 | 6.23 | 62.9% |
| 3.6 | 0.98 | 7.69 | 77.6% |
| 6.0 | 1.08 | 8.56 | 86.4% |
| 10.0 | 1.77 | 8.82 | 89.0% |
| ∞ | ∞ | 9.91 | 100% |
| Drainage Length/m | Water Film Initial Depth/mm | ||||||
|---|---|---|---|---|---|---|---|
| 0.01 | 0.02 | 0.03 | 0.05 | 0.1 | 0.2 | 0.5 | |
| 1 | 3.7768 | 3.7771 | 3.7758 | 3.7774 | 3.7774 | 3.7769 | 3.7767 |
| 2 | 4.5196 | 4.5200 | 4.5185 | 4.5204 | 4.5204 | 4.5197 | 4.5196 |
| 3 | 5.0169 | 5.0164 | 5.0159 | 5.0145 | 5.0147 | 5.0167 | 5.0147 |
| 4 | 5.4018 | 5.4021 | 5.3989 | 5.4016 | 5.4018 | 5.4020 | 5.4002 |
| 5 | 5.7159 | 5.7160 | 5.7198 | 5.7180 | 5.7176 | 5.7159 | 5.7194 |
| 6 | 5.9907 | 5.9914 | 5.9910 | 5.9928 | 5.9927 | 5.9910 | 5.9923 |
| 7 | 6.2291 | 6.2294 | 6.2334 | 6.2317 | 6.2314 | 6.2292 | 6.2331 |
| 8 | 6.4470 | 6.4478 | 6.4478 | 6.4495 | 6.4494 | 6.4473 | 6.4491 |
| 9 | 6.6426 | 6.6424 | 6.6436 | 6.6427 | 6.6425 | 6.6425 | 6.6434 |
| Drainage Length/m | β | ||||
|---|---|---|---|---|---|
| 0° | 10° | 20° | 30° | 40° | |
| 0 | 0.05 | 0.05 | 0.05 | 0.05 | 0.05 |
| 1 | 3.8754 | 3.8484 | 3.8205 | 3.7976 | 3.7774 |
| 2 | 4.6222 | 4.5919 | 4.566 | 4.5393 | 4.5204 |
| 3 | 5.1175 | 5.0882 | 5.0643 | 5.0387 | 5.0145 |
| 4 | 5.5052 | 5.4759 | 5.4478 | 5.4239 | 5.4016 |
| 5 | 5.8197 | 5.7943 | 5.766 | 5.7415 | 5.718 |
| 6 | 6.0931 | 6.0665 | 6.0386 | 6.0128 | 5.9928 |
| 7 | 6.3359 | 6.3081 | 6.2802 | 6.2544 | 6.2317 |
| 8 | 6.5497 | 6.5222 | 6.4936 | 6.4674 | 6.4495 |
| 9 | 6.7475 | 6.7185 | 6.6905 | 6.6651 | 6.6427 |
| Source | Equation Form |
|---|---|
| Ross and Russam (RRL) | |
| John Anderson | |
| Gallaway | |
| Wambold | (L = 11) |
| Drainage Length/m | New Model/mm | John Anderson Model/mm | RRL Model/mm |
|---|---|---|---|
| 1 | 4.14 | 2.85 | 1.20 |
| 2 | 4.96 | 4.02 | 1.67 |
| 3 | 5.51 | 4.93 | 2.02 |
| 4 | 5.94 | 5.69 | 2.31 |
| 5 | 6.28 | 6.36 | 2.56 |
| 6 | 6.59 | 6.97 | 2.79 |
| 7 | 6.86 | 7.53 | 3.00 |
| 8 | 7.09 | 8.05 | 3.20 |
| 9 | 7.31 | 8.54 | 3.38 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, K.; Zhou, Q.; Zhao, E.; Li, G.; Dou, Y. A New Water Film Depth Prediction Model for Pavement Surface Drainage. Infrastructures 2024, 9, 36. https://doi.org/10.3390/infrastructures9030036
Zhao K, Zhou Q, Zhao E, Li G, Dou Y. A New Water Film Depth Prediction Model for Pavement Surface Drainage. Infrastructures. 2024; 9(3):36. https://doi.org/10.3390/infrastructures9030036
Chicago/Turabian StyleZhao, Kang, Qiong Zhou, Enqiang Zhao, Guofen Li, and Yanan Dou. 2024. "A New Water Film Depth Prediction Model for Pavement Surface Drainage" Infrastructures 9, no. 3: 36. https://doi.org/10.3390/infrastructures9030036
APA StyleZhao, K., Zhou, Q., Zhao, E., Li, G., & Dou, Y. (2024). A New Water Film Depth Prediction Model for Pavement Surface Drainage. Infrastructures, 9(3), 36. https://doi.org/10.3390/infrastructures9030036







