Micro-Scale Experimental Approach for the Seismic Performance Evaluation of RC Frames with Improper Lap Splices
Abstract
1. Introduction
2. Code Requirements for Rebar Lap Splices
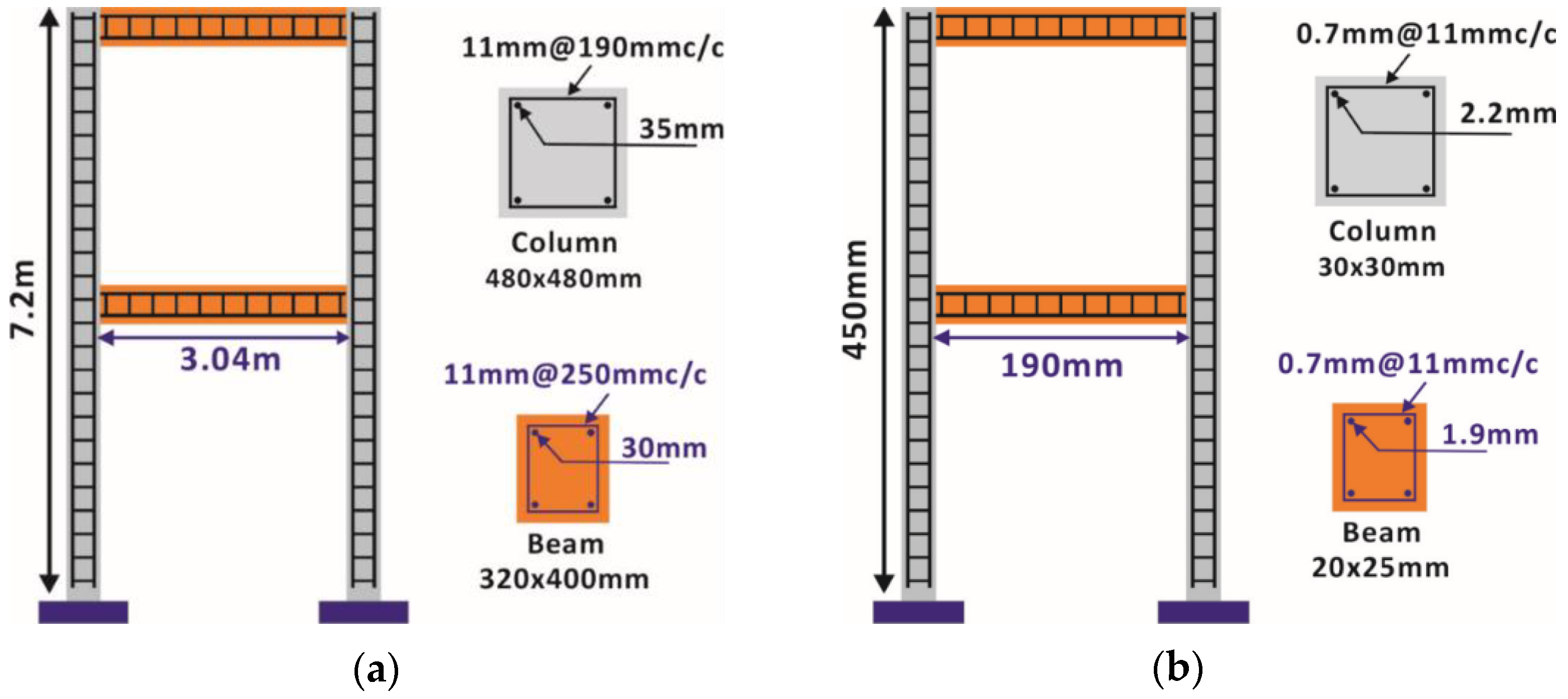
3. Design and Development of Model Structure
3.1. Design of Structure
3.2. Scaling of the Structure
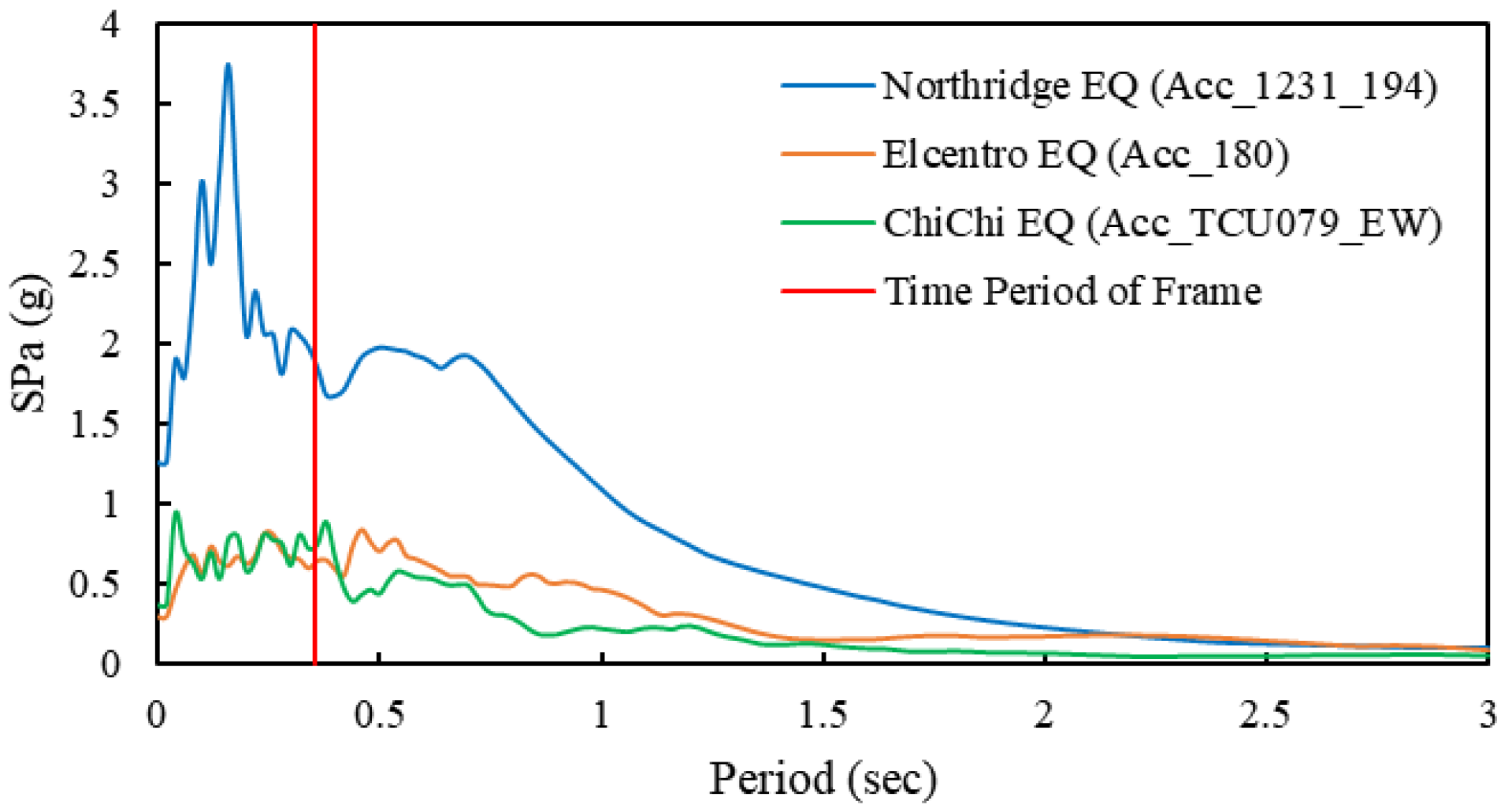
3.3. Verification of the Similitude Laws
3.3.1. Selection of Ground Motion
3.3.2. Applied Element Method (AEM) Results
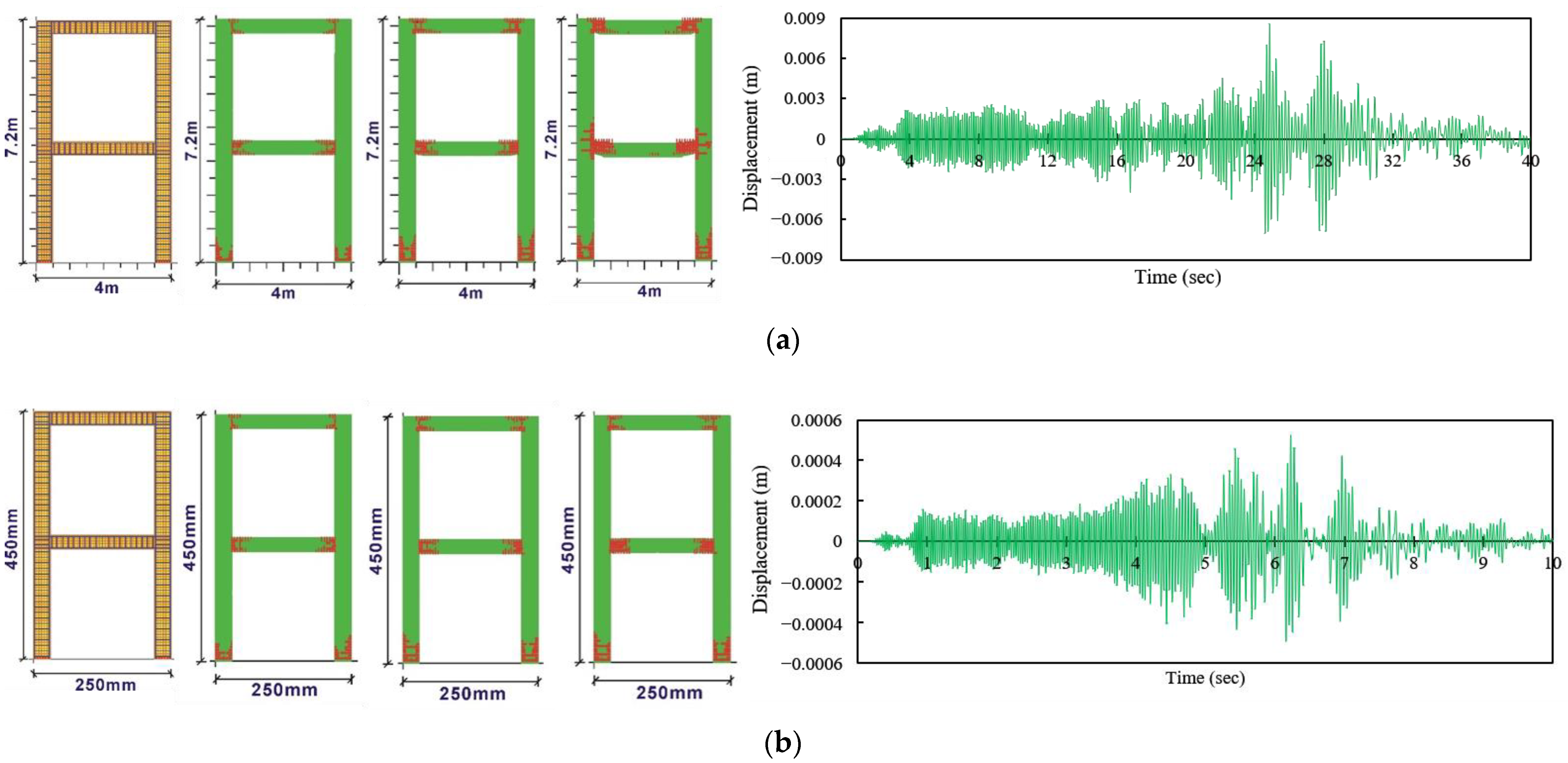
- For actual structure:
- Maximum displacement = 8.55 mmMax. Displacement/height of structure = 0.00119
- For scaled model:
- Maximum displacement = 0.453 mmMax. Displacement/height of structure = 0.00101
- Difference in results =
- 2.52%
3.4. Preparation of Model Structures
3.5. Location of Improper Lap Splices
4. Materials for Small-Scale Modelling
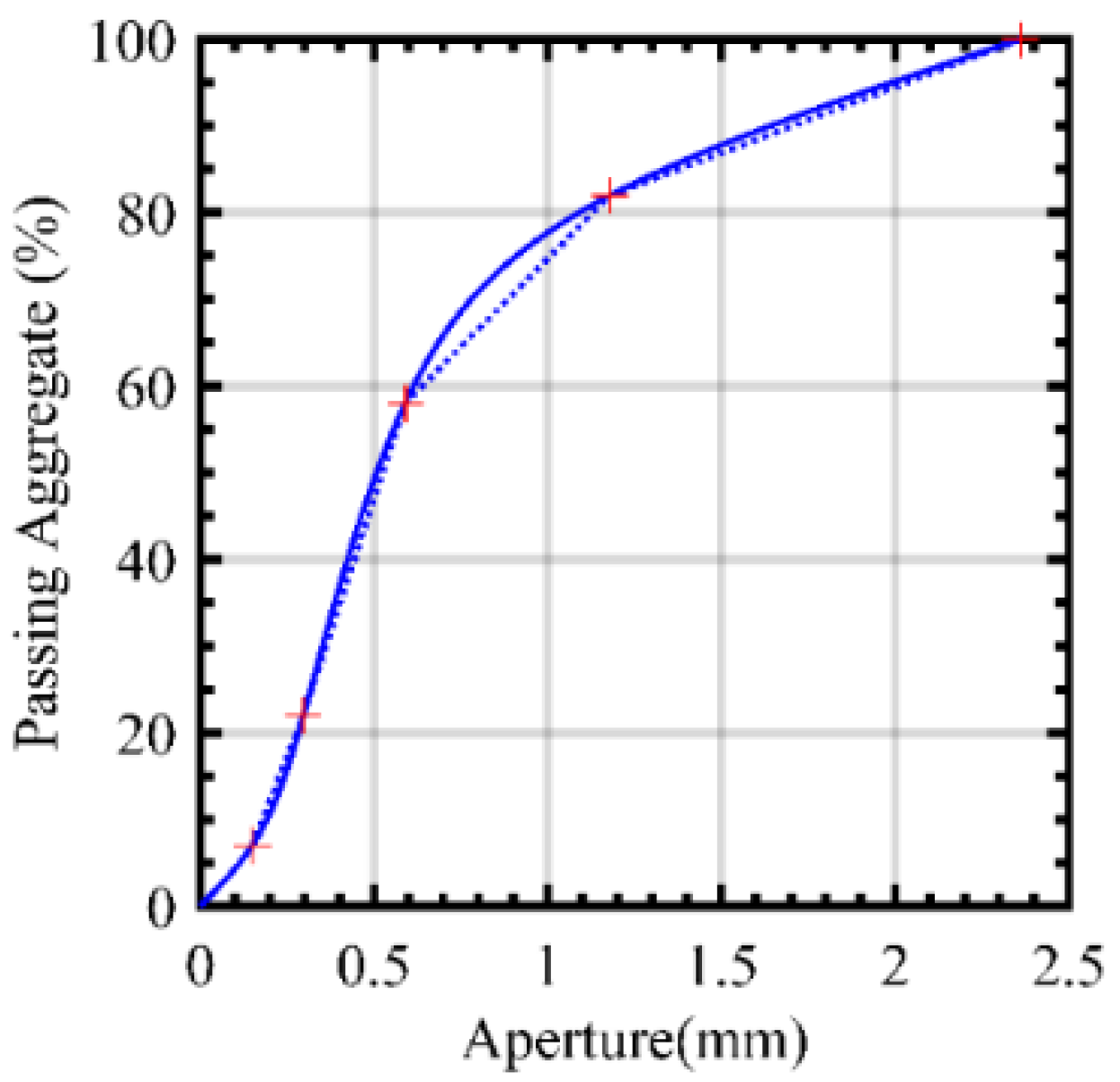
4.1. Sieve Analysis
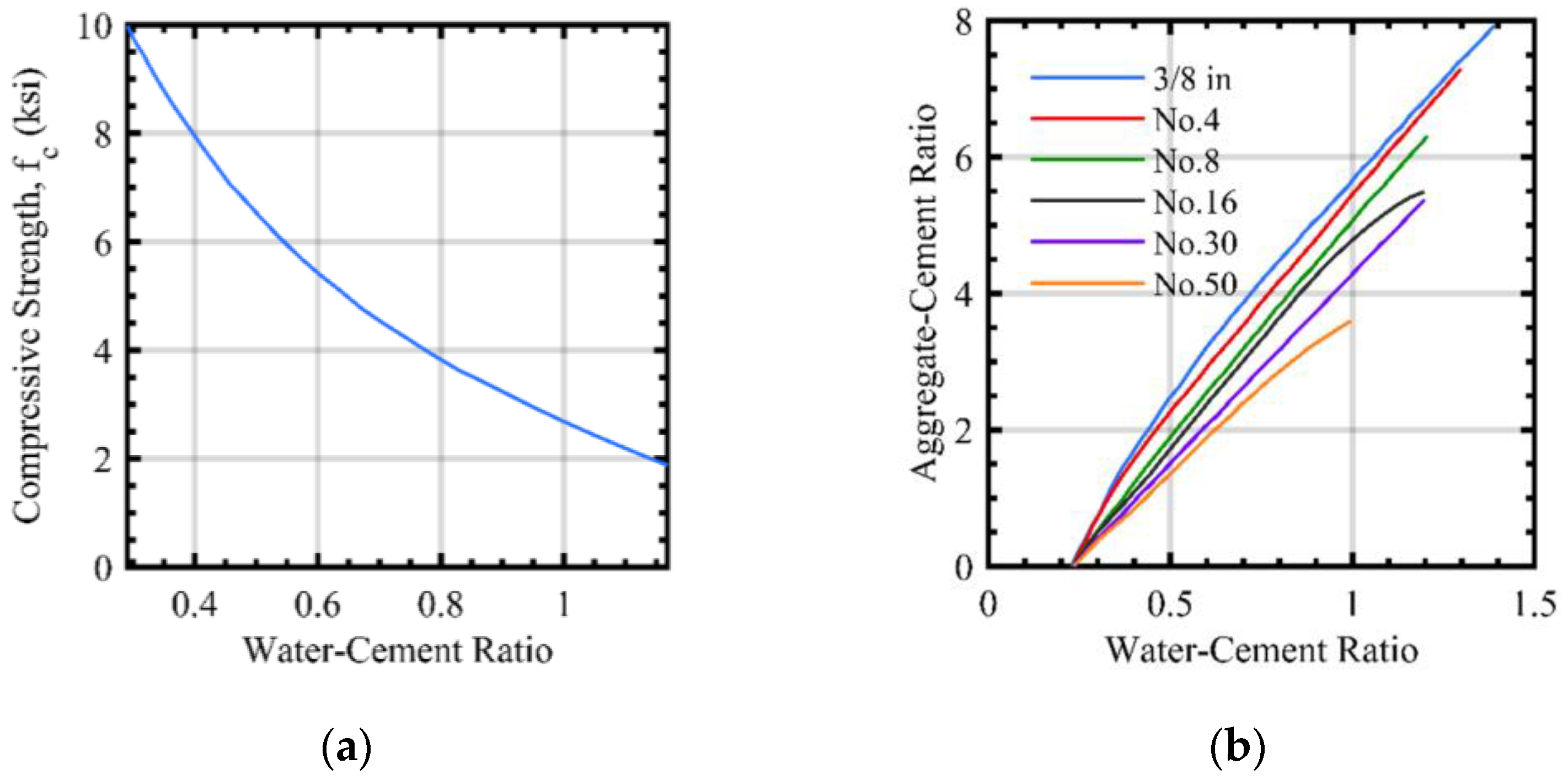
4.2. Selection of Aggregate-to-Cement and Water-to-Cement Ratios
4.3. Casting and Capping of Cylinders
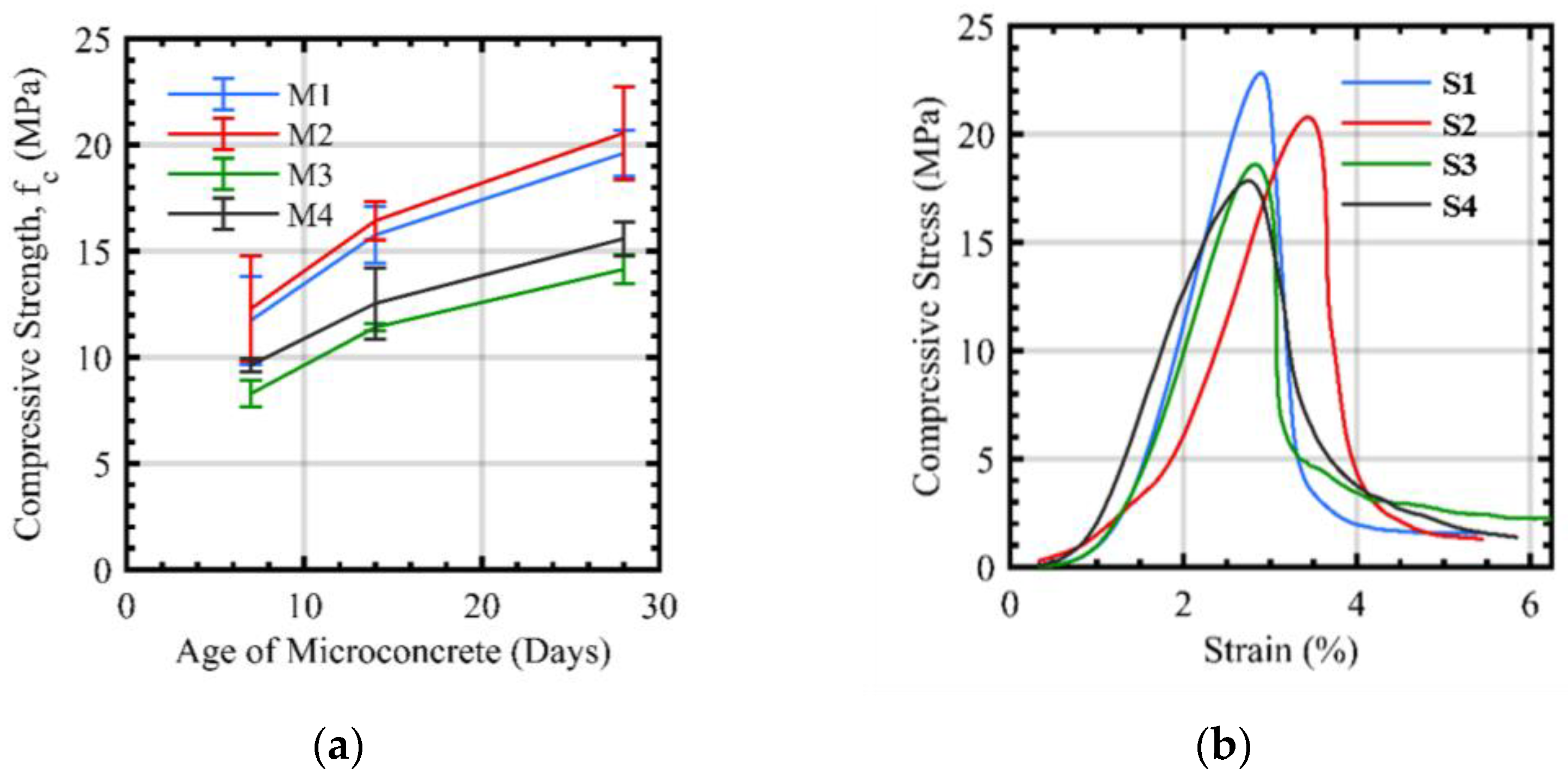
4.4. Compressive Strength of Micro-Concrete
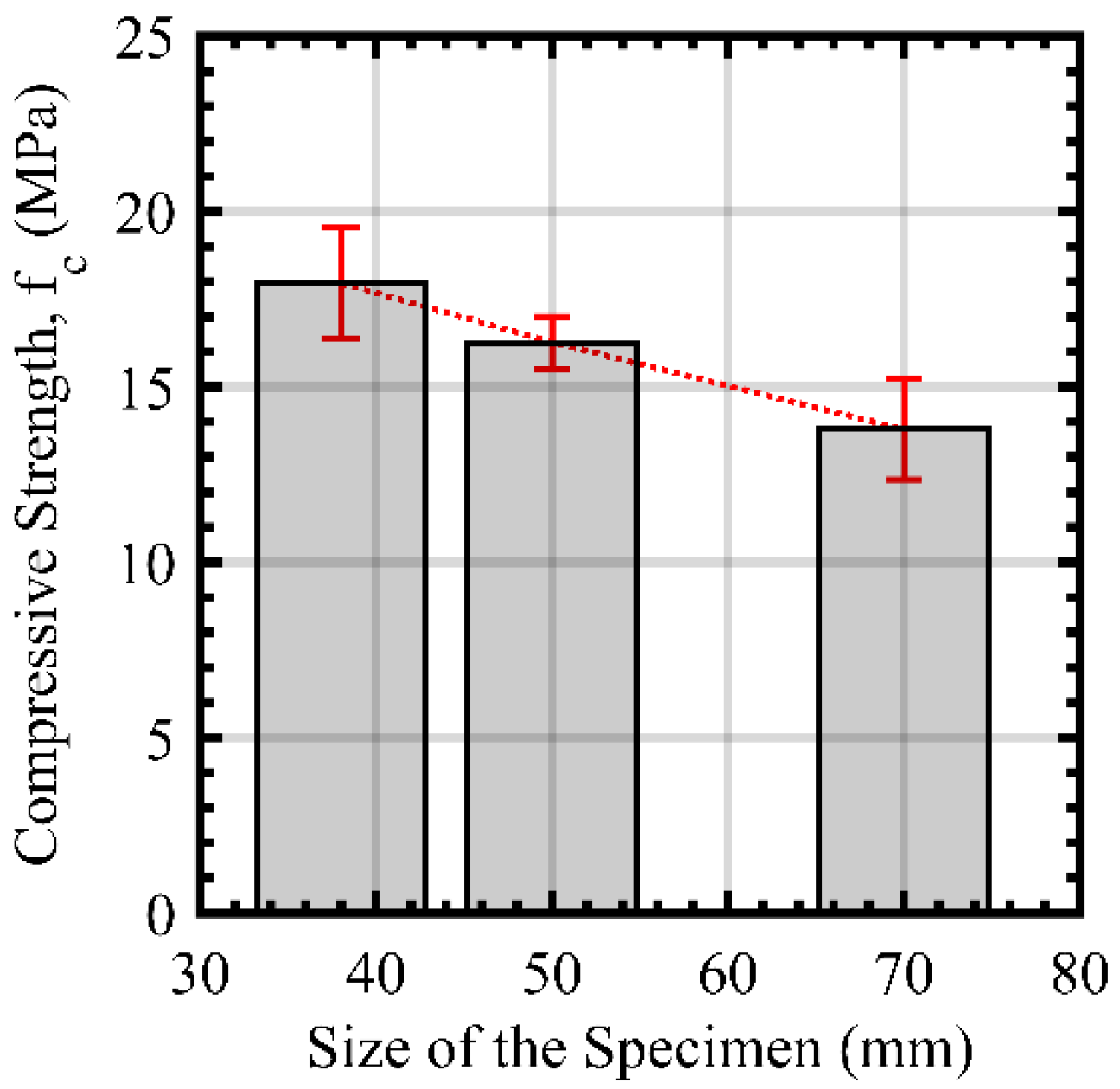
4.5. Size Effect in Compression
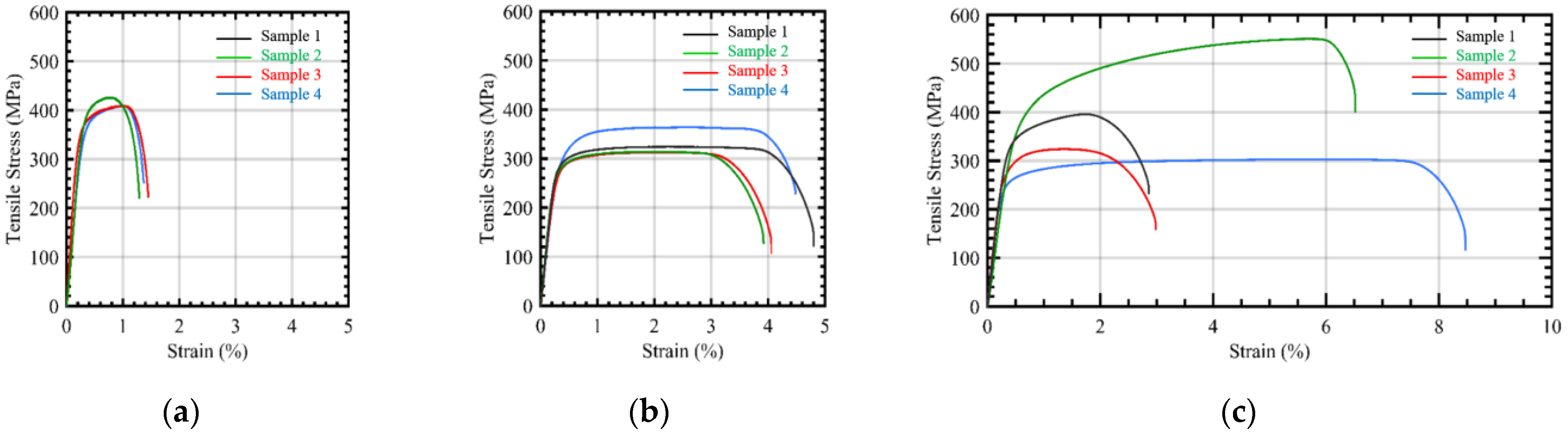
4.6. Tensile Strength of Scaled Rebars
5. Experimental Study
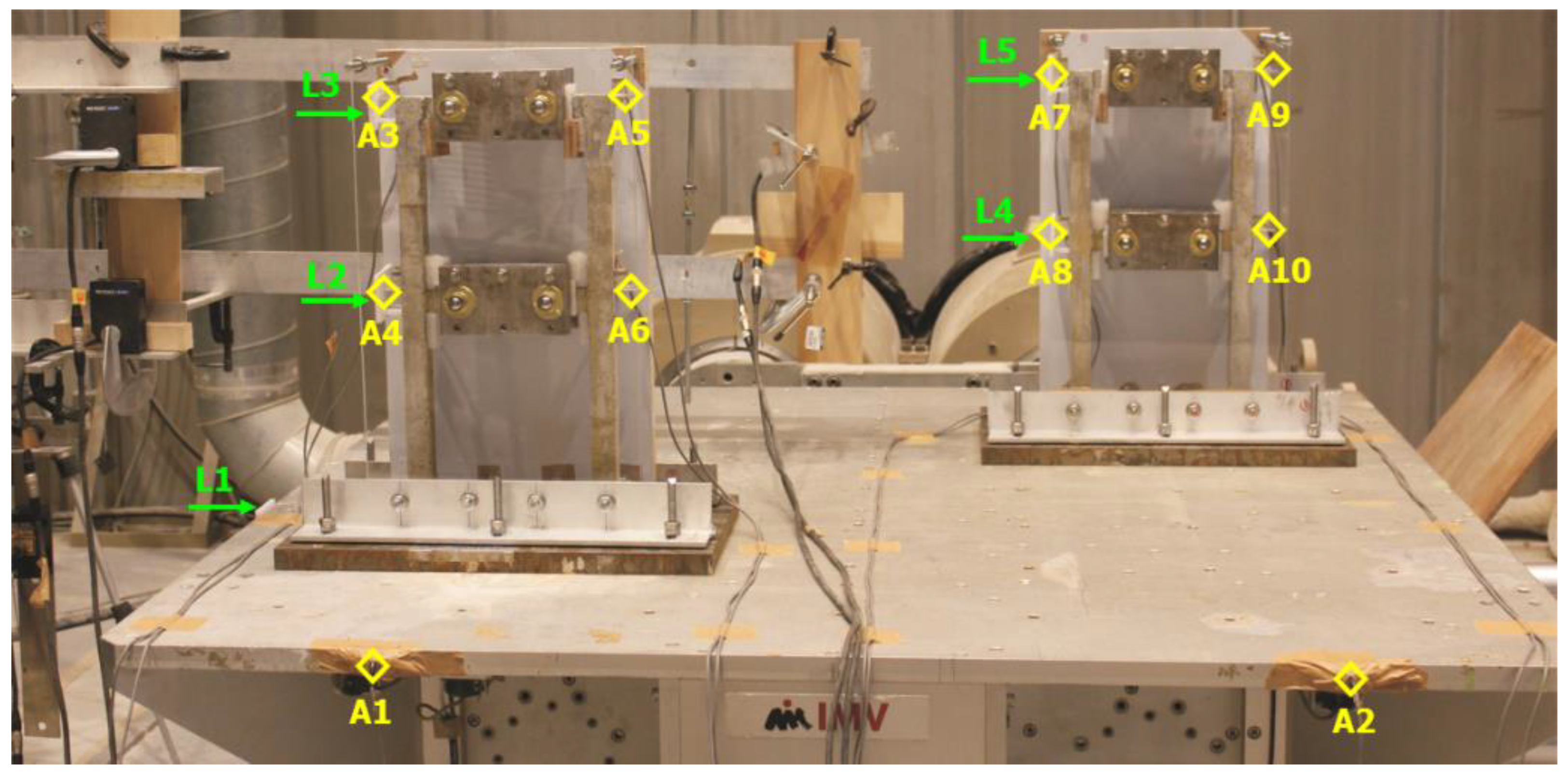
5.1. Instrumentation, Test Setup, and Testing Procedure
5.2. Shake Table Properties
5.3. Sinosoidal Motion Selection
- ▪
- By obtaining the maximum displacements, for all frequencies, in the proper frame through time history analysis in Staad.pro, which is a structural analysis and design software. The obtained values of displacements against all frequencies are shown in Table 11.
- ▪
- By judging and following the structure behavior against given inputs on the day of the test.
5.4. Calibration of Shake Table and Preparation of Data Files
6. Results and Discussions
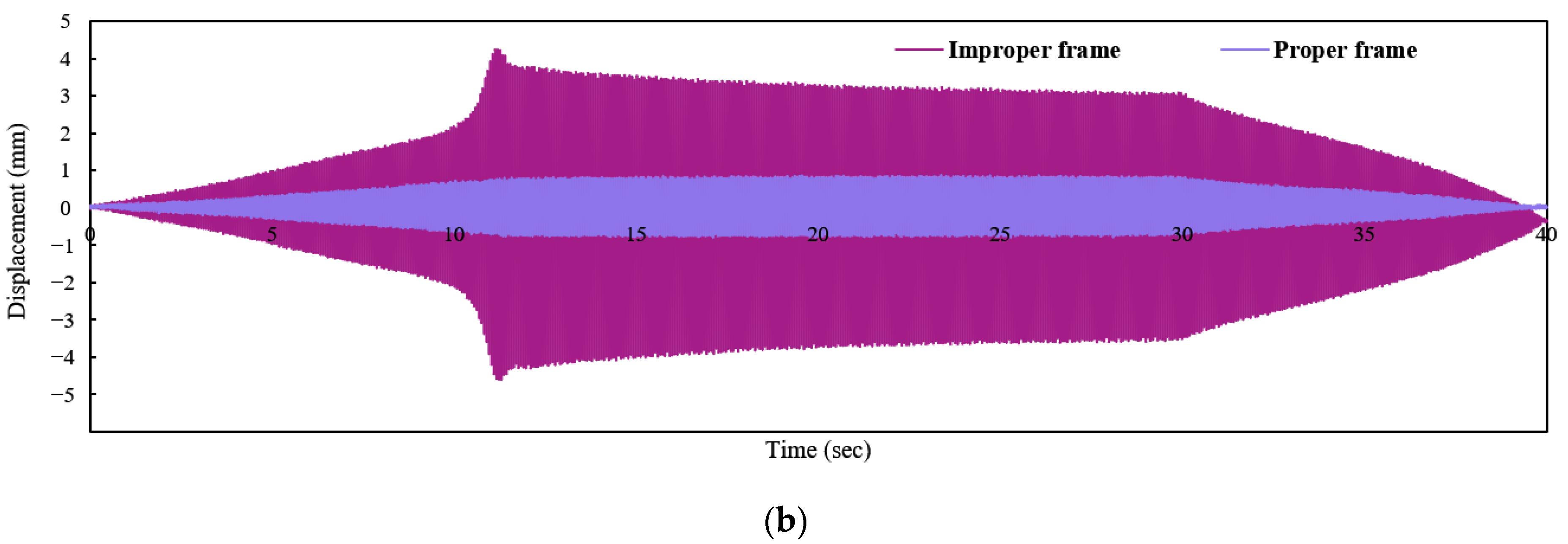
6.1. Crack Pattern and Energy Dissipation Mechanism
| Run | Description | |
| Proper Frame | Improper Frame | |
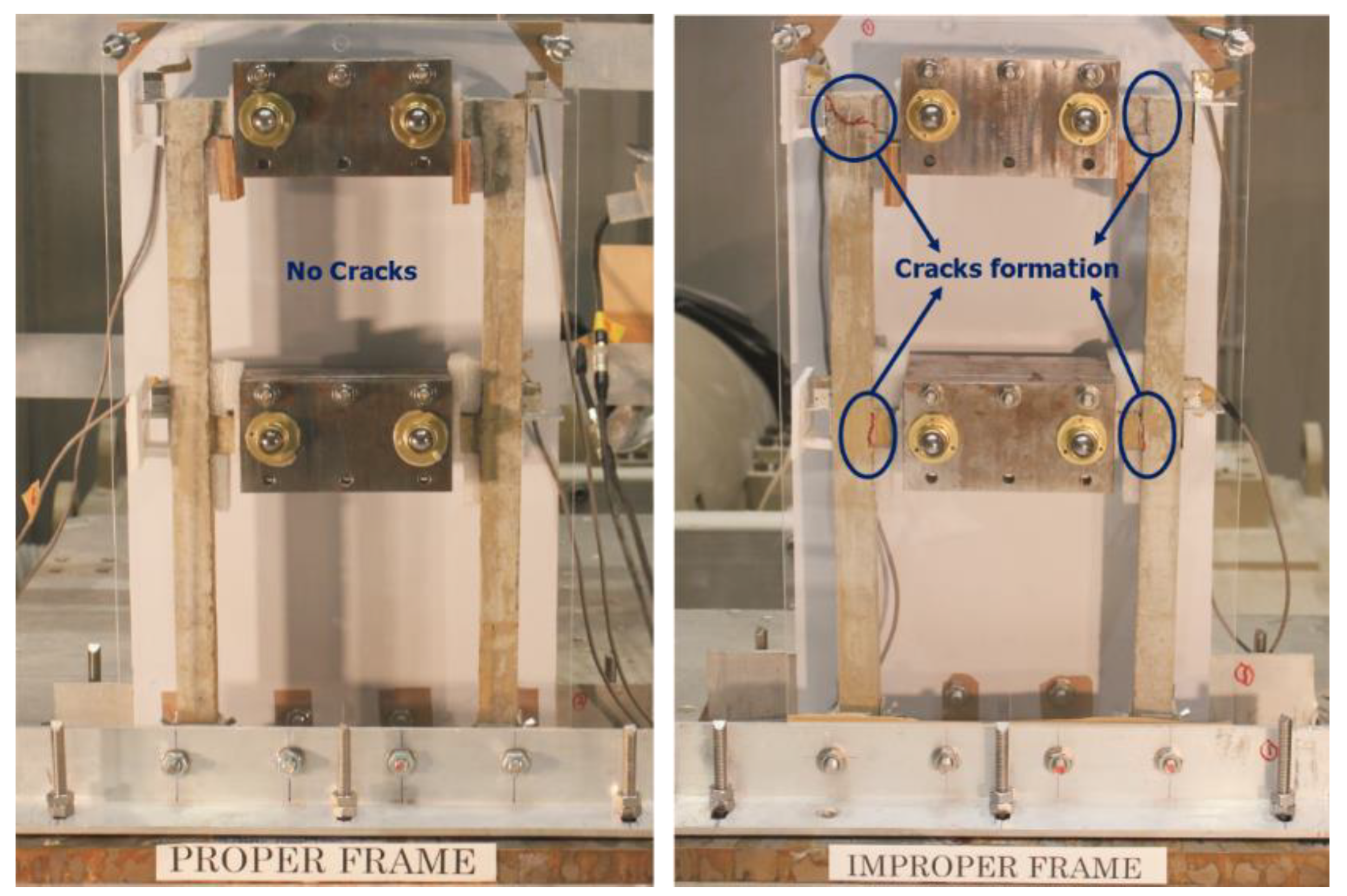
| 1–30 | No cracks | No significant cracks appear in the frame |
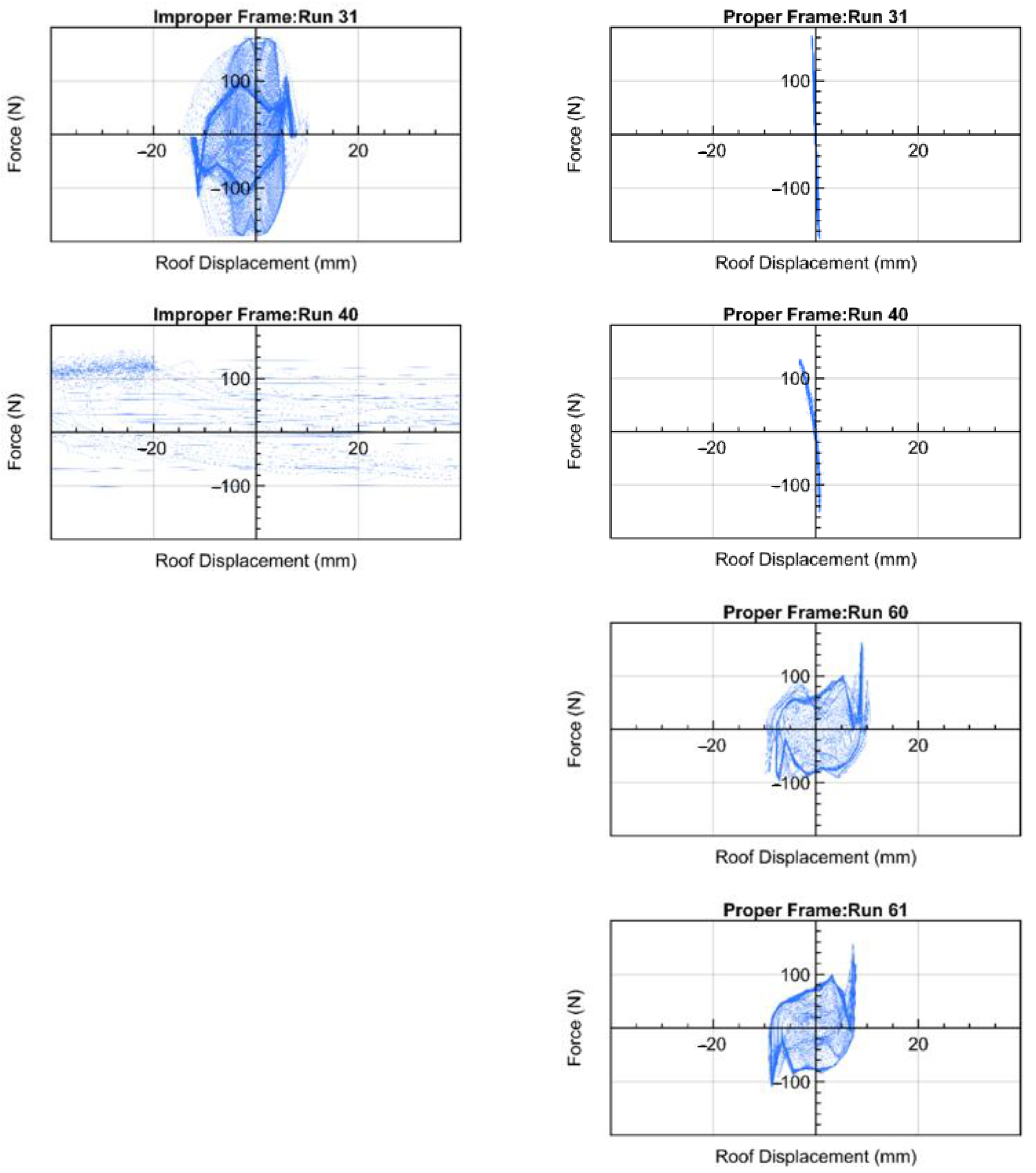
| 31 | No cracks | Significant cracks appeared in the beam–column joints when a 10 Hz frequency was applied for 40 s. The cracks in the frame are shown in Figure 17. Partial cracks appear in the base connection. |
| 32–38 | No cracks | No significant changes in the cracks. The frame was able to resist higher frequencies (i.e., 35 Hz and 40 Hz) and also low frequencies (i.e., 5 Hz and 2 Hz) with lesser (50%) amplitude. |
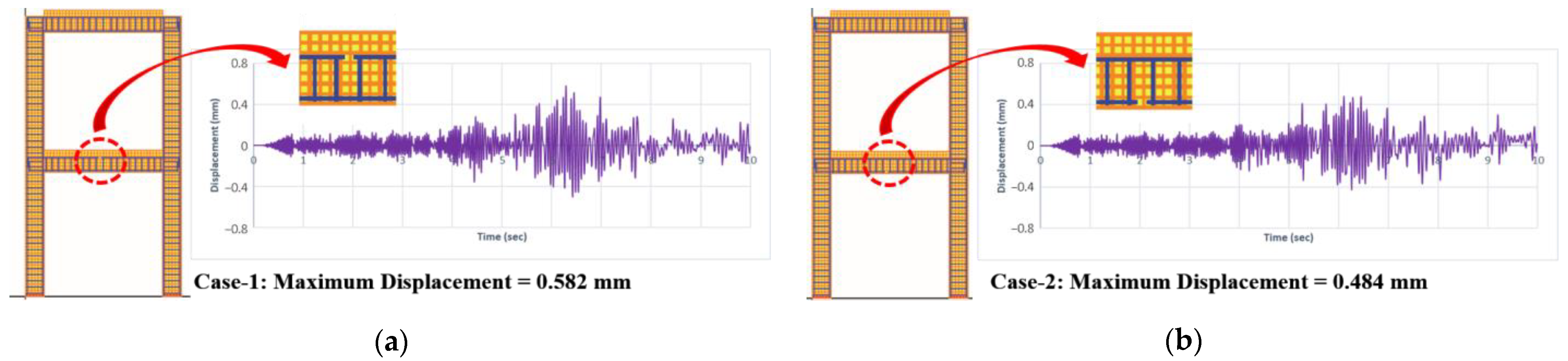
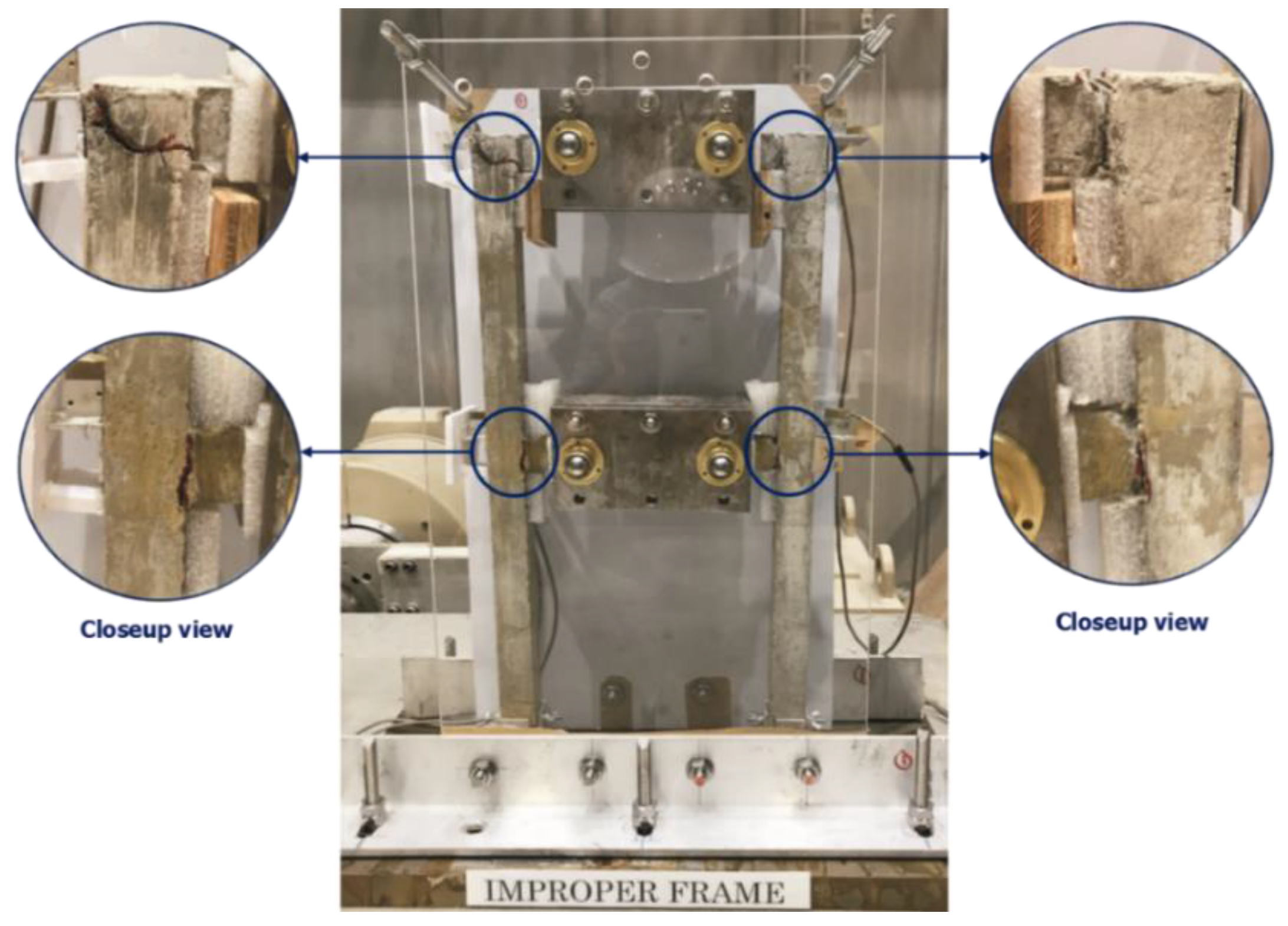
| 39 | No cracks | The cracks in the joints widen up severely, and additional cracks appear in the base connection. The reinforcement bars in the top right corner of the improper frame had a shear failure. The accelerometer A9 was detached, and reinforcement was visible. Its close-up view is shown in Figure 18. |
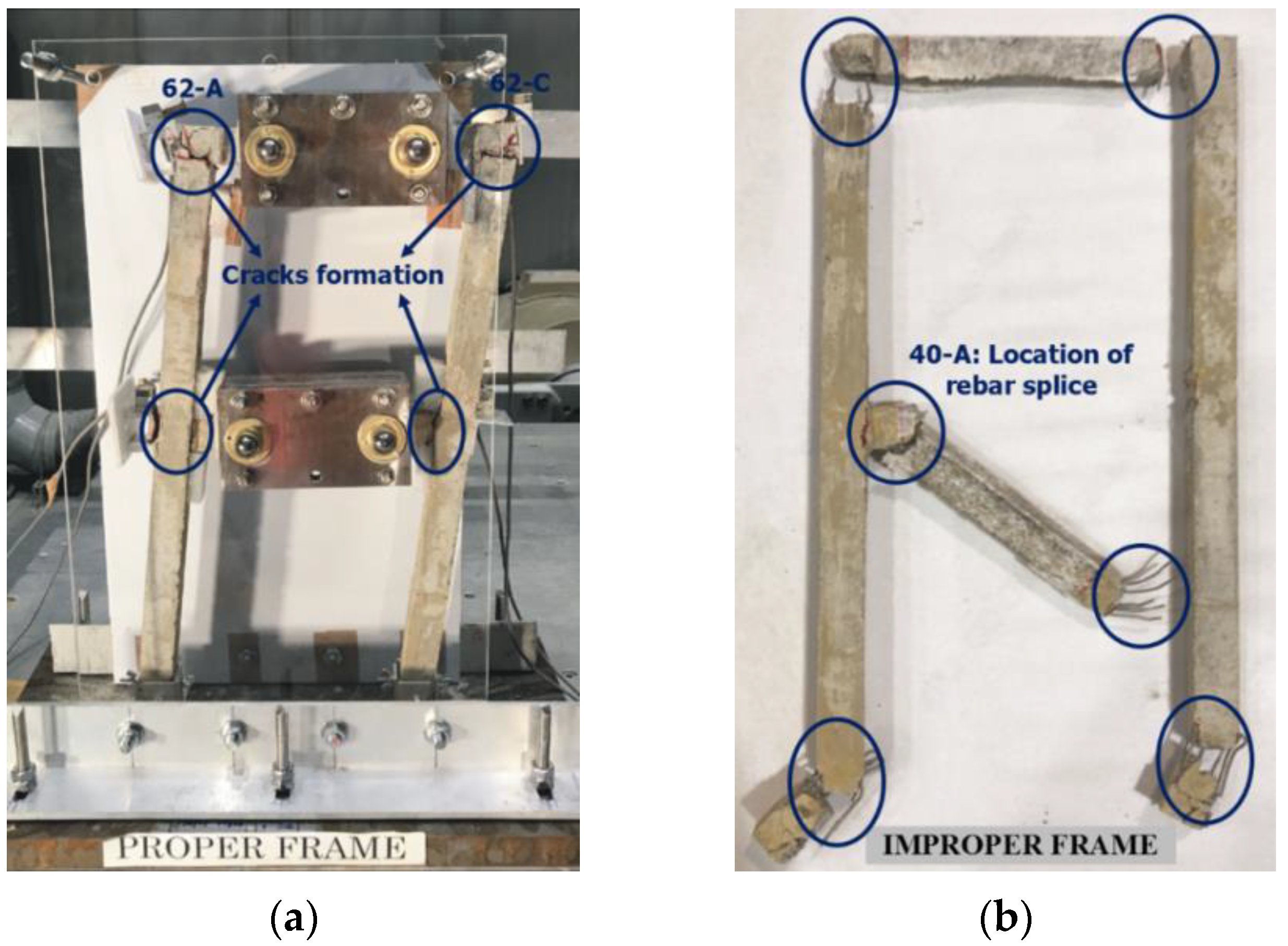
| 40 | No cracks | Complete failure of the improper frame. Both beams were damaged entirely as shown in Figure 19b. Although there were some cracks at the beam–column interface at point 40-A, but the improper lap splicing dominated the failure at this portion. At all other joints, the failure occurred at the beam–column joints. |
| 41–44 | No cracks were observed in the proper frame. After this stage, the 10 Hz frequency loading was repeated several times. | Collapsed |
| 45–58 | The 8 Hz, 10 Hz, and 12 Hz frequency waves were repeated several times, but no cracks were observed. | Collapsed |
| 59 | Significant cracks appear after repeating the 10 Hz frequency several times for 2 min. There were no significant cracks in the base connection. | Collapsed |
| 60,61 | No significant change in cracks. | Collapsed |
| 62 | Severe cracks appear in all joints, as shown in Figure 19a. The base connections also had severe cracks. The accelerometers were detached from points 62-a and 62-c. | Collapsed |
| 63 | Complete failure of the proper frame. None of the beams were completely detached, but all beam–column joints had severe damage. | Collapsed |
6.2. Hysteresis
7. Conclusions
- (1)
- The experimental results have proved that improper splicing details can reduce the capacity of reinforced concrete structures sufficiently, and the structure is highly vulnerable in case of an earthquake. The shake table test results demonstrate that the plastic hinges and damage points for the proper frame are the beam–column joints and the base connections as shown in Figure 19a, but if there is a provision of improper lap splice, then this can alter the damage point of the structure, triggering complete detachment of the beam with the improper lap splice as observed in Figure 19b at point 40-A.
- (2)
- The experimental results of this research also demonstrate the reduction in strength, stiffness, and energy dissipation of RC structures, which have been through multiple earthquakes, which implies the reduction in the age of the structure. Furthermore, the results from this experiment can be used for awareness among local communities about the vulnerability of structures against earthquakes due to these small, neglected defects. This could reduce poor construction practices in developing countries in future projects.
- (3)
- In addition to the scientific importance of this research, the authors would also like to emphasize the cost-effectiveness of this research. While increasing the burden on the financial component of experimentations, studying problems such as non-engineering defects could not be lucrative with the conventional methods of testing. Therefore, the testing mentioned in this research should pave the way for other research in micro-modeling to reduce the cost and study non-engineering problems extensively to find scientific and social solutions.
- (4)
- In this research, the shake table test was conducted by limiting the shaking to only one direction to avoid the out-of-plane failure of the 2D frames. Furthermore, it is also important to mention here that this research focuses mainly on the qualitative presentation of the problems caused by non-engineering defects. The idea of a reduction in the capacity of a structure with non-engineering defects can be achieved qualitatively through the numerical simulation presented in the article but the extent of the reduction in strength as compared to the proper structure could not be acquired at this stage. So, there is a need to further improve the numerical analysis techniques by using the experimental results.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zafar, A. Response Modification Factor of Reinforced Concrete Moment Resisting Frames in Developing Countries; University of Illinois at Urbana-Champaign: Urbana, IL, USA, 2009. [Google Scholar]
- Isler, O. Seismic Performances and Typical Damages of Beam-Column Joints in the RC Buildings. In Proceedings of the 14th World Conference on Earthquake Engineering, Beijing, China, 12–17 October 2008. [Google Scholar]
- Ates, S.; Kahya, V.; Yurdakul, M.; Adanur, S. Damages on reinforced concrete buildings due to consecutive earthquakes in Van. Soil Dyn. Earthq. Eng. 2013, 53, 109–118. [Google Scholar] [CrossRef]
- Arya, A.S. Non-engineered construction in developing countries—An approach toward earthquake risk preduction. In Proceedings of the 12th World Conference on Earthquake Engineering, Auckland, New Zeland, 30 January–4 February 2000; pp. 1–22. [Google Scholar]
- Castillo, A.; López-Almansa, F.; Pujades, L.G. Seismic risk analysis of urban non-engineered buildings: Application to an informal settlement in Mérida, Venezuela. Nat. Hazards 2011, 59, 891–916. [Google Scholar] [CrossRef]
- Arya, A.S.; Boen, T.; Ishiyama, Y. Guidelines for Earthquake Resistant Non-Engineered Construction; The United Nations Educational, Scientific and Cultural Organization (UNESCO): Paris, France, 2014. [Google Scholar]
- Alam, M.J.; Ahsan, R.; Akhter, F.; Paul, A. Earthquake Resistant Non-Engineered Building Construction for Rural Area in Bangladesh. In Proceedings of the 14th World Conference on Earthquake Engineering, Beijing, China, 12–17 October 2008. [Google Scholar]
- Ali, K.; Javed, A.; Mustafa, A.E.; Saleem, A. Blast-Loading Effects on Structural Redundancy of Long-Span Suspension Bridge Using a Simplified Approach. Pract. Period. Struct. Des. Constr. 2022, 27, 04022024. [Google Scholar] [CrossRef]
- Mustafa, A.E.; Javed, A.; Ali, K. Safety Assessment of Cables of Suspension Bridge under Blast Load. Struct. Congr. 2022, 2022, 79–93. [Google Scholar] [CrossRef]
- Chaudhary, M.T.A. Seismic Vulnerability Mitigation of Non-Engineered Buildings. Int. J. Civ. Struct. Constr. Archit. Eng. 2014, 8, 37–41. [Google Scholar]
- Boen, T.; Pribadi, K.S. Engineering of Non-Engineered Masonry Houses for Better Earthquake Resistance in Indonesia. Asian J. Environ. Disaster Manag. 2011, 3, 65–70. [Google Scholar] [CrossRef]
- Blondet, M.; Vargas-Neumann, J.; Tarque, N.; Soto, J.; Sosa, C.; Sarmiento, J. Seismic Reinforcement of Earthen Constructions. In Proceedings of the 16th World Conference on Earthquake Engineering, Santiago, Chile, 9–13 January 2017. [Google Scholar]
- Blondet, M.; Vargas, J.; Sosa, C.; Soto, J. Seismic Simulation Tests to Validate a Dual Technique for Repairing Adobe Historical Buildings Damaged by Earthquakes. In Proceedings of the Kerpic—New Generations Earthern Architecture, Istanbul, Turkey, 11–14 September 2013. [Google Scholar]
- Fahim, M.; Elkoly, S.; Morad, S. Efficient New Numerical Technique for RC Structures with Construction Defects under Monotonic and Cyclic Loading Conditions. Asian Trans. Eng. 2013, 2, 7–16. [Google Scholar]
- Elishakoff, I.; Ankitha, A.P.; Marzani, A. Rigorous versus naïve implementation of the Galerkin method for stepped beams. Acta Mech. 2019, 230, 3861–3873. [Google Scholar] [CrossRef]
- Elishakoff, I.; Amato, M.; Ankitha, A.P.; Marzani, A. Rigorous implementation of the Galerkin method for stepped structures needs generalized functions. J. Sound Vib. 2021, 490, 115708. [Google Scholar] [CrossRef]
- Javed, A.; Sadeghnejad, A.; Rehmat, S.; Yakel, A.; Azizinamini, A. Magnetic Flux Leakage (MFL) Method for Damage Detection in Internal Post-Tensioning Tendons; Florida International University: Miami, FL, USA, 2021. [Google Scholar] [CrossRef]
- Afzal, M.F.; Matsumoto, Y.; Nohmi, H.; Sakai, S.; Su, D.; Nagayama, T. Comparison of Radar Based Displacement Measurement Systems with Conventional Systems in Vibration Measurements at a Cable Stayed Bridge. 2016. Available online: https://www.researchgate.net/publication/307932143 (accessed on 31 January 2023).
- Hameed, A.; Rasool, A.M.; Ibrahim, Y.E.; Afzal, M.F.U.D.; Qazi, A.U.; Hameed, I. Utilization of Fly Ash as a Viscosity-Modifying Agent to Produce Cost-Effective, Self-Compacting Concrete: A Sustainable Solution. Sustainability 2022, 14, 11559. [Google Scholar] [CrossRef]
- Filiatrault, A.; Lachapelle, É.; Lamontagne, P. Seismic performance of ductile and nominally ductile reinforced concrete moment resisting frames. I. Experimental study. Can. J. Civ. Eng. 1998, 25, 331–341. [Google Scholar] [CrossRef]
- Caccese, V.; Harris, H.G. Earthquake simulation testing of small-scale reinforced concrete structures. Struct. J. 1990, 87, 72–80. [Google Scholar] [CrossRef]
- Žarnić, R.; Gostič, S.; Crewe, A.J.; Taylor, C.A. Shaking table tests of 1:4 reduced-scale models of masonry infilled reinforced concrete frame buildings. Earthq. Eng. Struct. Dyn. 2001, 30, 819–834. [Google Scholar] [CrossRef]
- Kim, W.; El-Attar, A.; White, R.N. Small-Scale Modeling Techniques for Reinforced Concrete Structures Subjected to Seismic Loads; Technical Report NCEER-88-0041; National Center for Earthquake Engineering Research: New York, NY, USA, 1988; p. 92. [Google Scholar]
- Noor, F.A.; Boswell, L.F. Small Scale Modelling of Concrete Structures; Elsevier Science Publishers Ltd.: New York, NY, USA, 2005. [Google Scholar] [CrossRef]
- Dejian, S.; Lu, X. Experimental study on dynamic compressive properties of microconcrete under different strain rate. In Proceedings of the 14th World Conference on Earthquake Engineering, Beijing, China, 12–17 October 2008. [Google Scholar]
- Boutemeur, R.; Demidem, M.; Bali, A.; Benyoussef, E.H. Modelling of Micro-Concrete Behaviour under Static and Dynamic Loading. Appl. Mech. Mater. 2014, 584–586, 1089–1096. [Google Scholar] [CrossRef]
- Tobar, R.; Bonelli, P. Test of micro-concrete model of a building damaged during the 1985 chile earthquake. In Proceedings of the Eleventh World Conference on Earthquake Engineering, Acapulco, Mexico, 23–28 June 1996. [Google Scholar]
- Opabola, E.A.; Mangalathu, S. Seismic fragility assessment of bridges with as-built and retrofitted splice-deficient columns. Bull. Earthq. Eng. 2022, 21, 583–603. [Google Scholar] [CrossRef]
- Opabola, E.A.; Elwood, K.J. Seismic assessment of reinforced concrete columns with short lap splices. Earthq. Spectra 2021, 37, 1726–1757. [Google Scholar] [CrossRef]
- Chronopoulos, P.; Trezos, C.; Chronopoulos, M. Behaviour of RC elements with inadequate lap splices, before and after upgrading by welding of reinforcement. In Proceedings of the 4th International Symposium “Bond in Concrete 2012”, Brescia, Italy, 17–20 June 2012. [Google Scholar]
- Elrakib, T.M.; Arafa, A.I. Experimental evaluation of the common defects in the execution of reinforced concrete beams under flexural loading. HBRC J. 2012, 8, 47–57. [Google Scholar] [CrossRef]
- Goksu, C.; Yilmaz, H.; Chowdhury, S.R.; Orakcal, K.; Ilki, A. The effect of lap splice length on the cyclic lateral load behavior of RC members with low-strength concrete and plain bars. Adv. Struct. Eng. 2014, 17, 639–658. [Google Scholar] [CrossRef]
- Cho, J.-Y.; Pincheira, J.A. Nonlinear modeling of RC columns with short lap splices. In Proceedings of the 13th World Conference on Earthquake Engineering, Vancouver, BC, Canada, 1–6 August 2004. [Google Scholar]
- Chowdhury, S.R.; Orakcal, K. Analytical modeling of columns with inadequate lap splices. ACI Struct. J. 2014, 110, 735–744. [Google Scholar] [CrossRef]
- Nawaz, W.; Yehia, S.; Elchalakani, M. Lap splices in confined self-compacting lightweight concrete. Constr. Build. Mater. 2020, 263, 120619. [Google Scholar] [CrossRef]
- Code CF; International Federation for Structural Concrete: Lausanne, Switzerland, 2010.
- ACI Committee 318, 318–319; Building Code Requirements for Structural Concrete and Commentary. ACI Committee: Farmington Hills, MI, USA, 2019. [CrossRef]
- ASCE. Seismic Evaluation and Retrofit of Existing Buildings ASCE/SEI 41-13; ASCE: Reston, VA, USA, 2014. [Google Scholar] [CrossRef]
- Okazaki, K.; Kusumastuti, D.; Probadi, K.; Saito, T. Comparison of Current Construction Practices of Non-Engineered Buildings in Developing Countries. In Proceedings of the 15th World Conference on Earthquake Engineering (15WCEE), Lisbon, Portugal, 24–28 September 2012; pp. 9962–9971. [Google Scholar]
- Rezaeepazhand, J.; Simitses, G.J.; Starnes, J.H. Scale models for laminated cylindrical shells subjected to axial compression. Compos. Struct. 1996, 34, 371–379. [Google Scholar] [CrossRef]
- Thomas, C.; Tamayo, P.; Setién, J.; Ferreño, D.; Polanco, J.A.; Rico, J. Effect of high temperature and accelerated aging in high density micro-concrete. Constr. Build. Mater. 2021, 272, 121920. [Google Scholar] [CrossRef]
- American Society of Civil Engineers. Minimum Design Loads and Associated Criteria for Buildings and Other Structures; American Society of Civil Engineers: Reston, VA, USA, 2017; pp. 1–889. [Google Scholar] [CrossRef]
- NFEC. Mix Design for Small-Scale Models of Concrete Structures: Technical Report R564; NFEC: Port Hueneme, CA, USA, 1968. [Google Scholar]
- Talaat, A.; Emad, A.; Tarek, A.; Masbouba, M.; Essam, A.; Kohail, M. Factors affecting the results of concrete compression testing: A review. Ain Shams Eng. J. 2021, 12, 205–221. [Google Scholar] [CrossRef]
- ASTM C 192/C 192M-02; Standard Practice for Making and Curing Concrete Test Specimens in the Laboratory. ASTM International, ASTM Committee: Farmington Hills, MI, USA, 2002; Volume 4, pp. 1–8.
- ASTM C 39/C 39M-01; Standard Test Method for Compressive Strength of Cylindrical Concrete Specimens. ASTM International, ASTM Committee: Farmington Hills, MI, USA, 2001; Volume 4, pp. 1–5.
- Yi, S.T.; Yang, E.I.; Choi, J.C. Effect of specimen sizes, specimen shapes, and placement directions on compressive strength of concrete. Nucl. Eng. Des. 2006, 236, 115–127. [Google Scholar] [CrossRef]
- Sabnis, G.M.; Mirza, S.M. Size Effect in Model Concretes? ASCE J. Struct. Div. 1979, 105, 1007–1020. [Google Scholar] [CrossRef]
- Elfahal, M.M.; Krauthammer, T.; Ohno, T.; Beppu, M.; Mindess, S. Size effect for normal strength concrete cylinders subjected to axial impact. Int. J. Impact Eng. 2005, 31, 461–481. [Google Scholar] [CrossRef]
- Elfahal, M.M.; Theodor, K. Dynamic Size Effect in Normal- and High-Strength Concrete Cylinders. Mater. J. 2005, 102, 77–85. [Google Scholar]




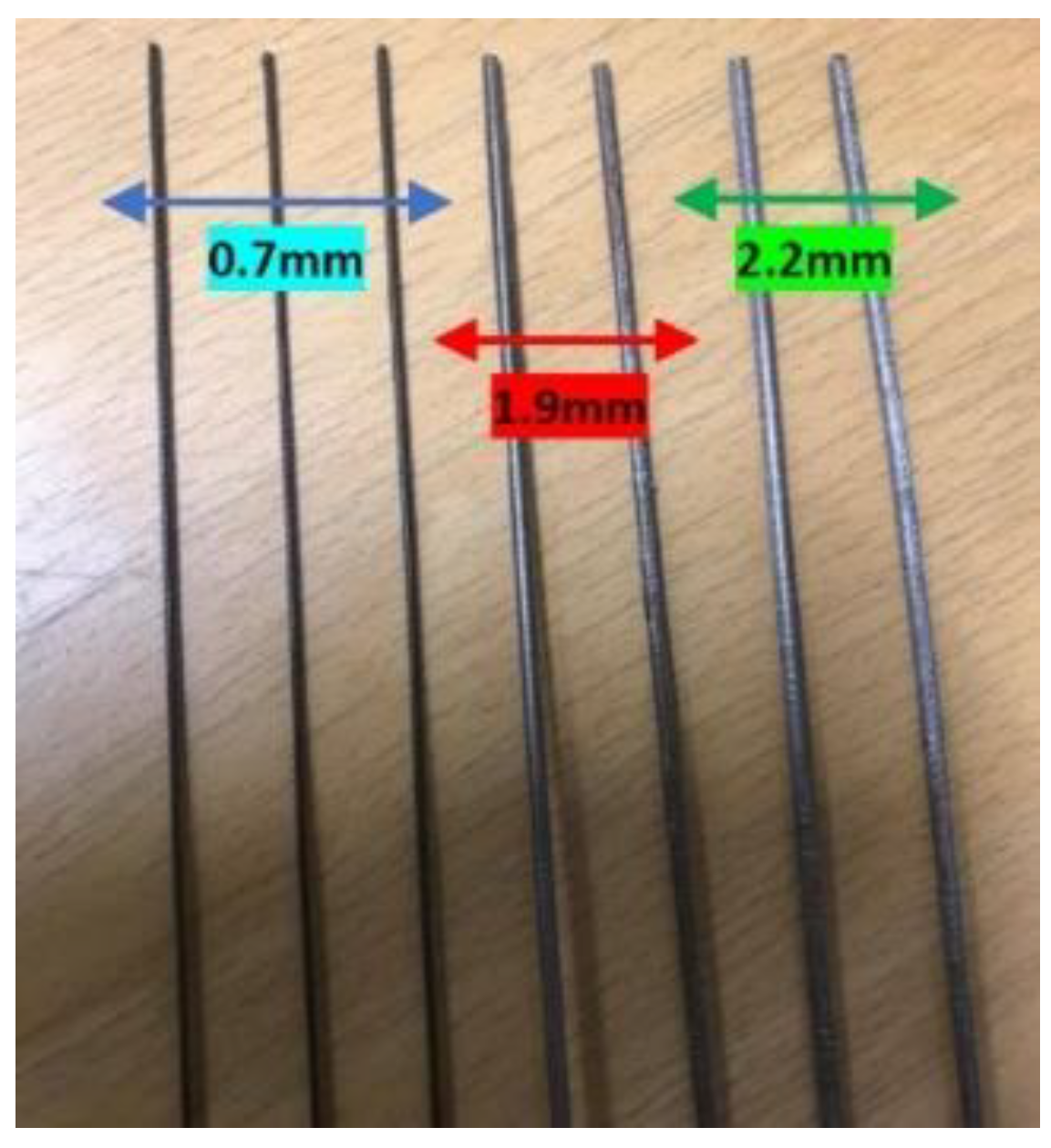



| Maximum Tensile Bar Stress | The Maximum Percent of Spliced within the Required Lap Length | |
| 50% | 50% | |
| Class A | Class B | |
| Class B | Class B | |
| (MPa) | 414 MPa | 414 MPa 550 MPa | 550 MPa |
| 21 | = Larger of (a) 0.071 (b) 300 mm | = Larger of (a) (0.13 24) (b) 300 mm | = Larger of (a) (0.13 24) (b) |
| 21 | = 1.333 × 0.071 | = 1.333 × (0.13 − 24) | = 1.333 × (0.13 − 24) |
| Parameter | 1/16 Scaled Value | Target Value |
| Length | 1/16 | 1/16 |
| Area | 1/162 | 1/162 |
| Mass | 1/163 | 1/163 |
| Time | 1/ | 1/ |
| Density | 1 | 1 |
| Stress | 1/16 | 1 |
| Strain | 1/16 | 1 |
| Model | Volume (m3) | Total Weight (kg) |
| Weight of actual size model | 4.096 | 9830 |
| Weight of scaled model | 0.001 | 2.4 |
| Sr. No. | Parameter | Value |
| 1 | Height of model | 450 mm |
| 2 | Width of model | 250 mm |
| 3 | Dimensions of columns | 30 mm × 30 mm |
| 4 | Dimensions of beams | 20 mm × 25 mm |
| Mix No. | Aggregate-to-Cement Ratio | Water-to-Cement Ratio |
| M-1 | 3.6 | 0.76 |
| M-2 | 3.6 | 0.72 |
| M-3 | 4.25 | 0.8 |
| M-4 | 4 | 0.78 |
| Mix No. | 7 Days | 14 Days | 28 Days |
| M1 | 12.32 | 15.82 | 19.27 |
| M2 | 12.59 | 16.28 | 19.89 |
| M3 | 8.18 | 11.22 | 14.39 |
| M4 | 9.63 | 12.24 | 15.39 |
| Cylinder Diameter | |||
| 38 mm | 50 mm | 75 mm | |
| Average (MPa) | 17.96 | 16.27 | 13.76 |
| Diameter (mm) | Stress (MPa) | Strain (%) |
| 0.7 | 414.7 | 1.51 |
| 1.9 | 328.7 | 4.32 |
| 2.2 | 393.28 | 5.42 |
| Property | Description |
| Frequency range | DC~100 Hz |
| Maximum load capacity | 2000 kg |
| Shake table dimensions | 1.5 m × 1.5 m |
| Maximum velocity (XYZ axis) | 1.1 m/s |
| Exciting force (XY axis) | Sine wave: 2000 kgf Pulse wave: 3000 kgf |
| Maximum displacement (XY axis) | ±100 mm |
| Maximum acceleration (XY axis) | Sine wave: 2.5 G and Pulse wave: 3.7 G (without load) Sine wave: 1.7 G and Pulse wave: 2.6 G (1000 kg) Sine wave: 1.2 G and Pulse wave: 1.0 G (2000 kg) |
| Maximum rotation angle | ±15° |
| Sr. No. | Frequency (Hz) | Maximum Displacement (mm) | |
| (1st Story) | (2nd Story) | ||
| 1 | 60 | 0.065 | 0.182 |
| 2 | 55 | 0.095 | 0.253 |
| 3 | 50 | 0.203 | 0.522 |
| 4 | 45 | 0.559 | 1.32 |
| 5 | 40 | 0.264 | 0.645 |
| 6 | 35 | 1.962 | 4.504 |
| 7 | 30 | 0.778 | 1.749 |
| 8 | 25 | 0.455 | 1.003 |
| 9 | 20 | 0.336 | 0.730 |
| 10 | 15 | 0.278 | 0.596 |
| 11 | 12 | 0.257 | 0.550 |
| 12 | 10 | 0.254 | 0.541 |
| 13 | 8 | 0.240 | 0.511 |
| 14 | 5 | 0.237 | 0.505 |
| 15 | 2 | 0.144 | 0.306 |
| 16 | 1.5 | 0.087 | 0.184 |
| Frequency | Max. Amplitude | Max. Velocity (m/s) | Max. Displacement (mm) |
| 60 | 2.0 g | 0.060 | 0.11 |
| 55 | 2.0 g | 0.065 | 0.14 |
| 50 | 2.0 g | 0.10 | 0.25 |
| 45 | 2.0 g | 0.080 | 0.24 |
| 40 | 2.0 g | 0.086 | 0.31 |
| 35 | 2.0 g | 0.10 | 0.40 |
| 30 | 2.0 g | 0.11 | 0.52 |
| 25 | 2.0 g | 0.14 | 0.85 |
| 20 | 2.0 g | 0.16 | 1.31 |
| 15 | 2.0 g | 0.22 | 2.30 |
| 12 | 2.0 g | 0.27 | 3.54 |
| 10 | 2.0 g | 0.33 | 5.21 |
| 8 | 2.0 g | 0.40 | 7.91 |
| 5 | 2.0 g | 0.65 | 20.6 |
| 2 | 1.28 g | 1.0 | 79.55 |
| 1.5 | 0.77 g | 0.80 | 88.50 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Javed, A.; Krishna, C.; Ali, K.; Afzal, M.F.U.D.; Mehrabi, A.; Meguro, K. Micro-Scale Experimental Approach for the Seismic Performance Evaluation of RC Frames with Improper Lap Splices. Infrastructures 2023, 8, 56. https://doi.org/10.3390/infrastructures8030056
Javed A, Krishna C, Ali K, Afzal MFUD, Mehrabi A, Meguro K. Micro-Scale Experimental Approach for the Seismic Performance Evaluation of RC Frames with Improper Lap Splices. Infrastructures. 2023; 8(3):56. https://doi.org/10.3390/infrastructures8030056
Chicago/Turabian StyleJaved, Ali, Chaitanya Krishna, Khawaja Ali, Muhammad Faheem Ud Din Afzal, Armin Mehrabi, and Kimiro Meguro. 2023. "Micro-Scale Experimental Approach for the Seismic Performance Evaluation of RC Frames with Improper Lap Splices" Infrastructures 8, no. 3: 56. https://doi.org/10.3390/infrastructures8030056
APA StyleJaved, A., Krishna, C., Ali, K., Afzal, M. F. U. D., Mehrabi, A., & Meguro, K. (2023). Micro-Scale Experimental Approach for the Seismic Performance Evaluation of RC Frames with Improper Lap Splices. Infrastructures, 8(3), 56. https://doi.org/10.3390/infrastructures8030056









