Comparative Assessment of Criticality Indices Extracted from Acoustic and Electrical Signals Detected in Marble Specimens
Abstract
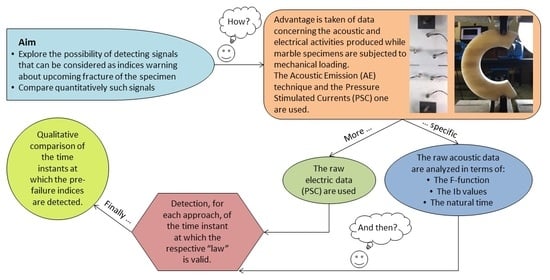
1. Introduction
2. Some Theoretical Preliminaries
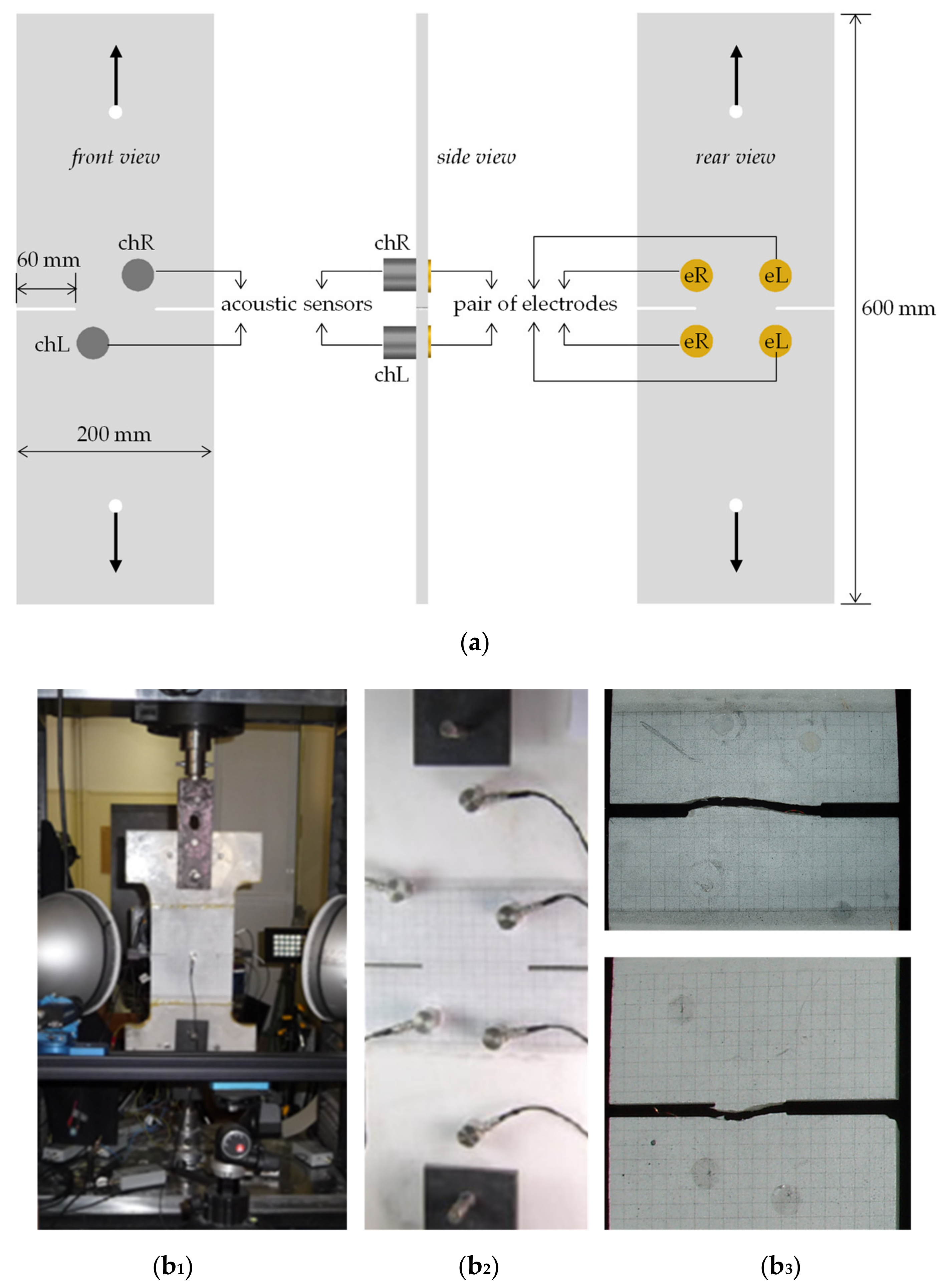
2.1. Sensing Techniques Based on Acoustic and Electric Emissions
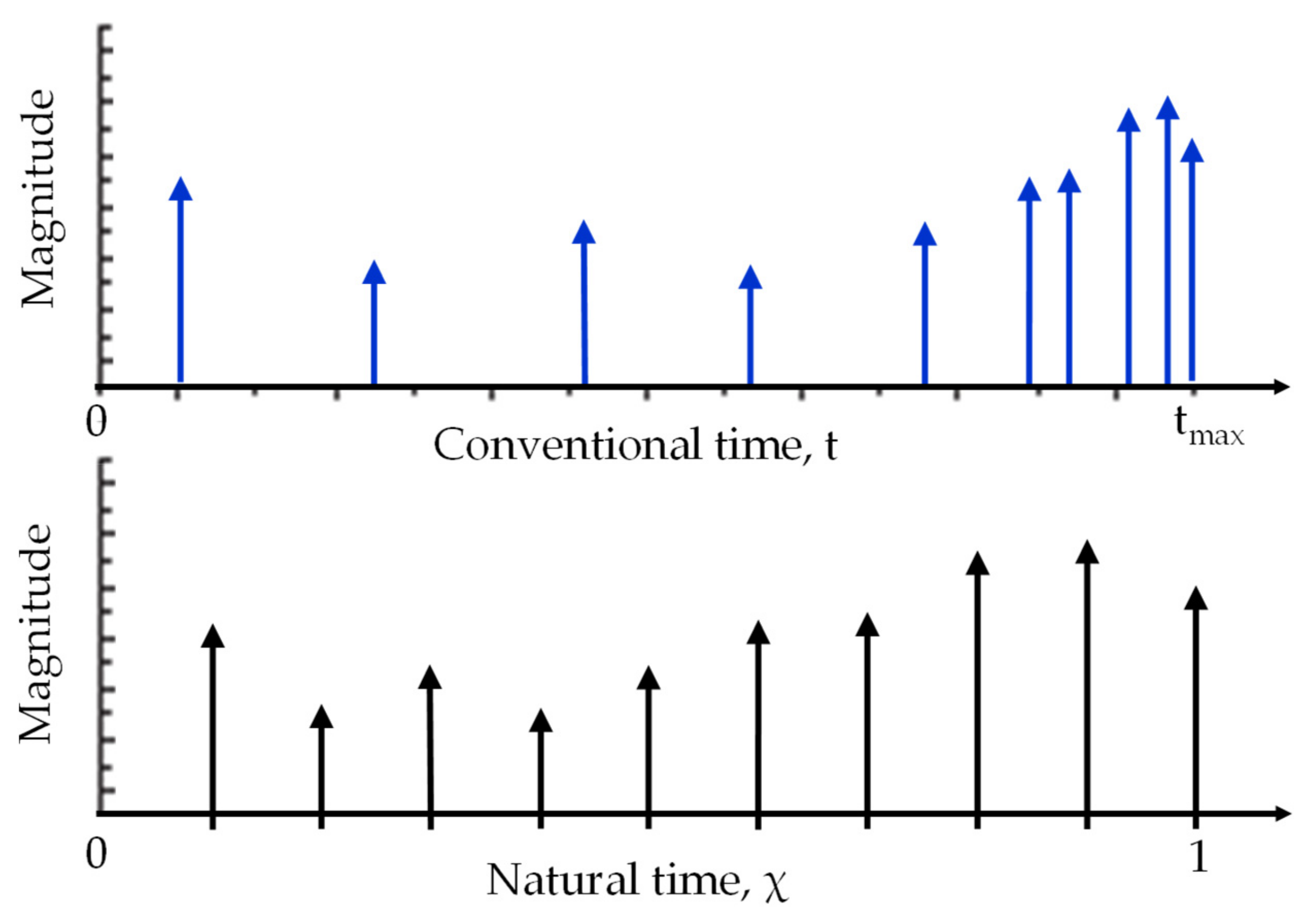
2.2. Elaborating the Data Concerning the Acoustic and Electric Emissions
3. Detecting Criticality Indices
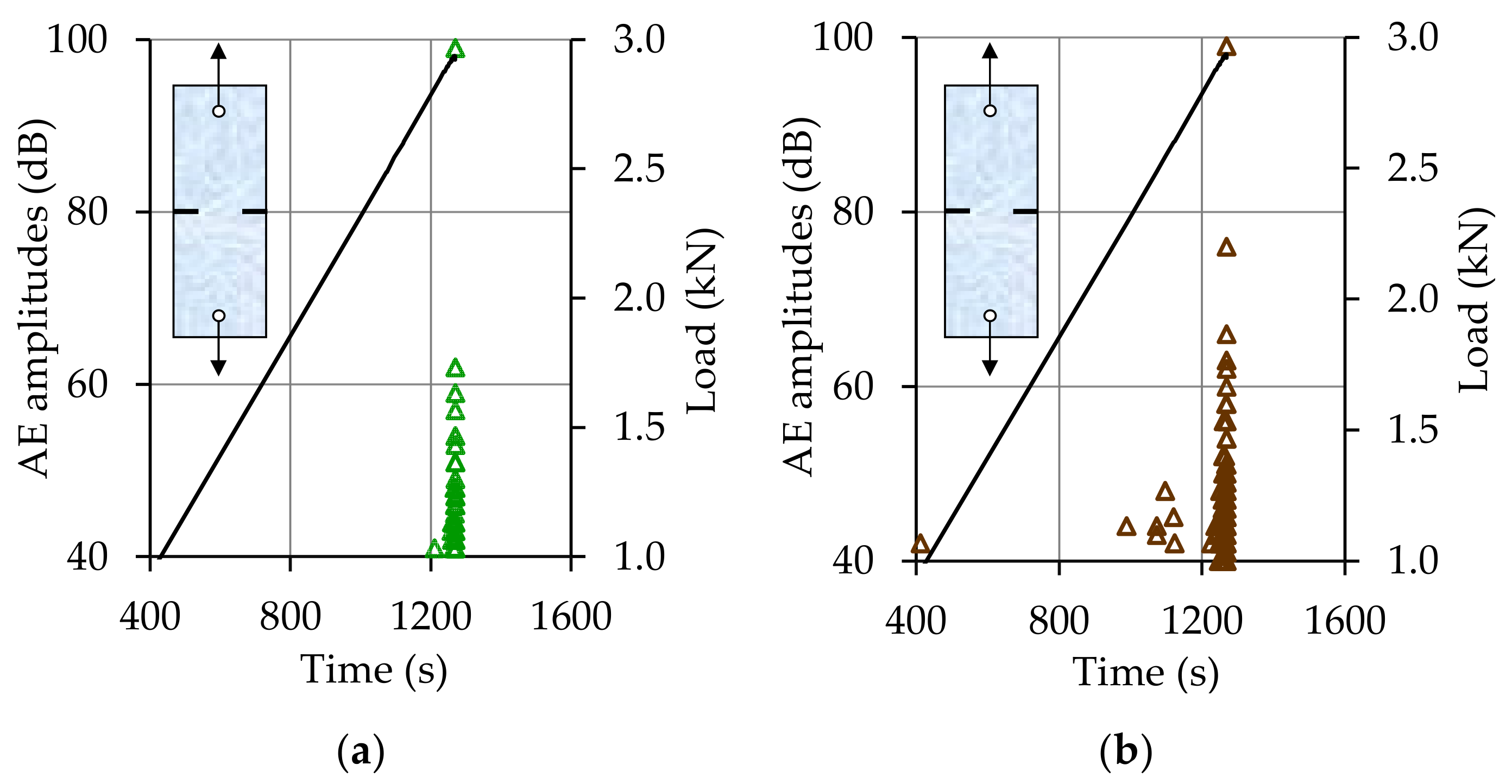
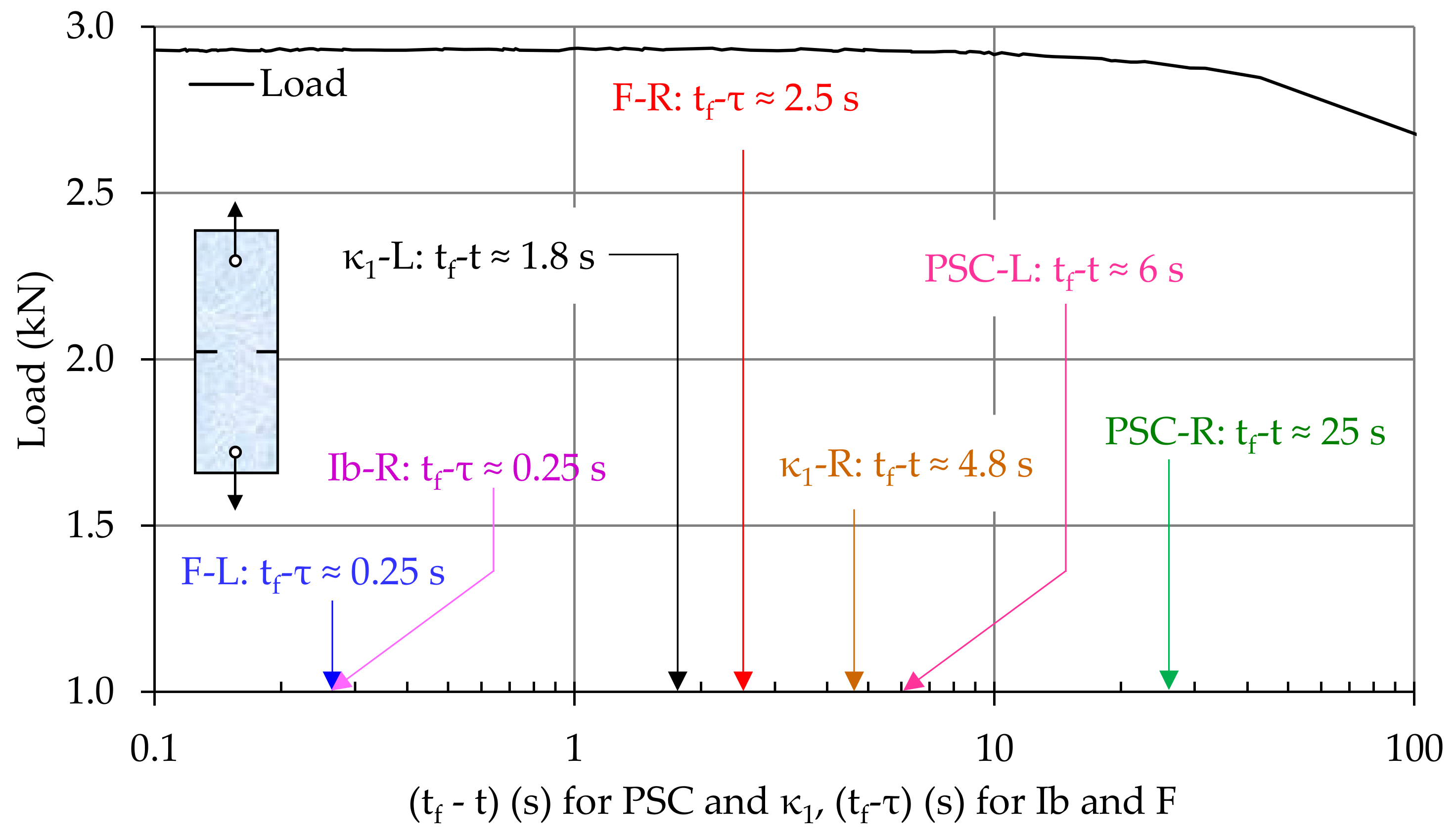
3.1. Direct Tension of Symmetrically Notched Marble Plates
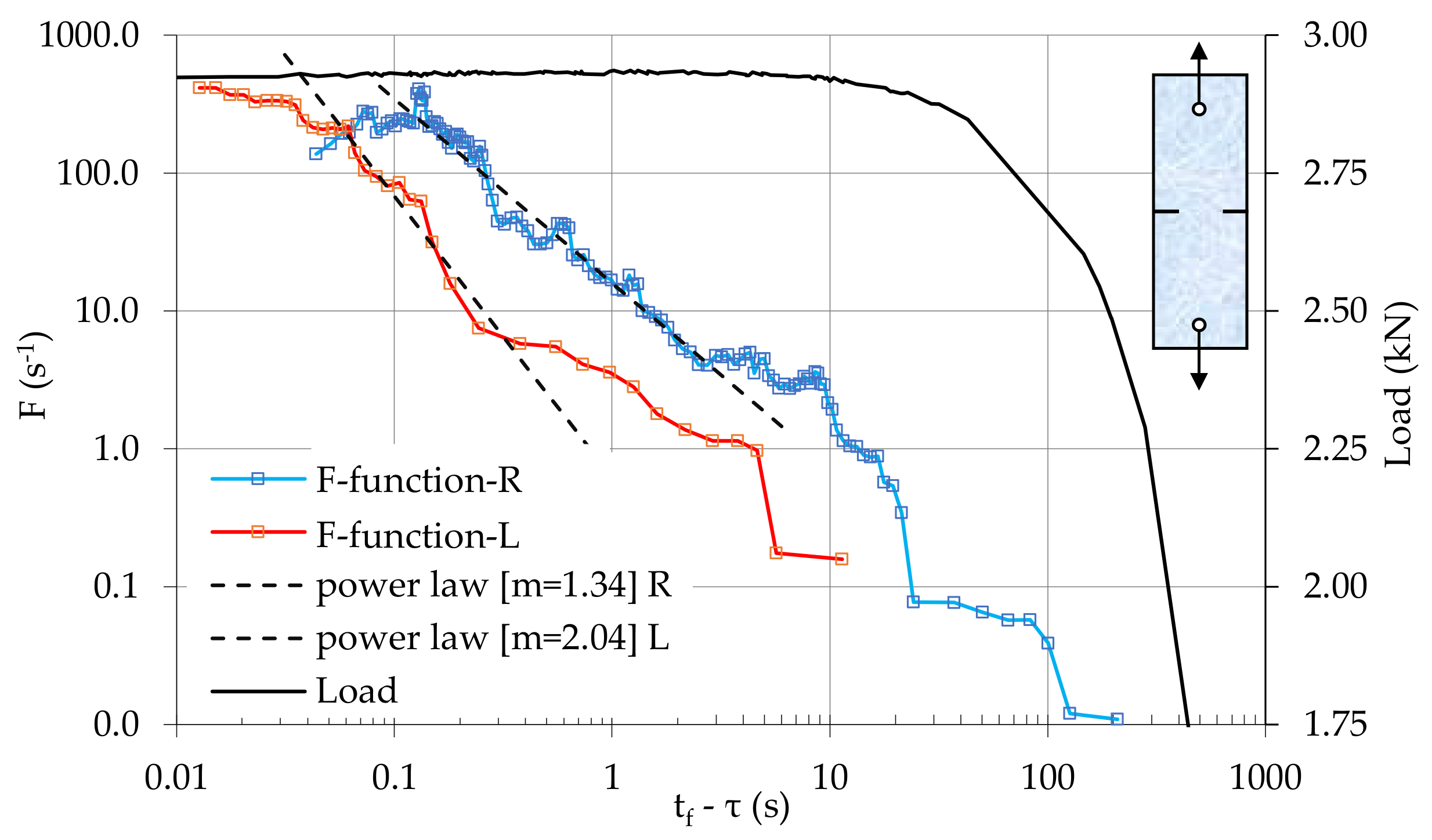
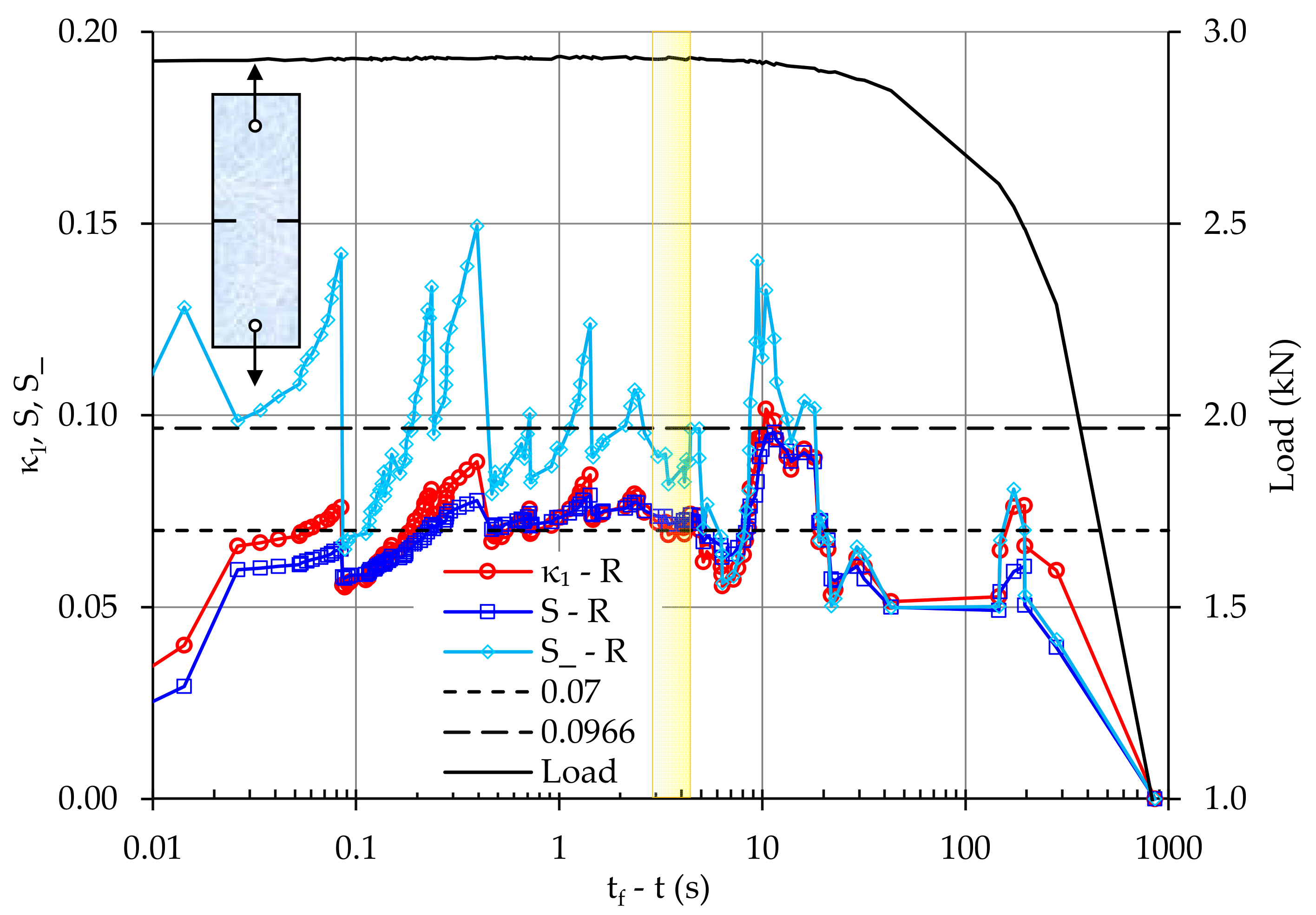
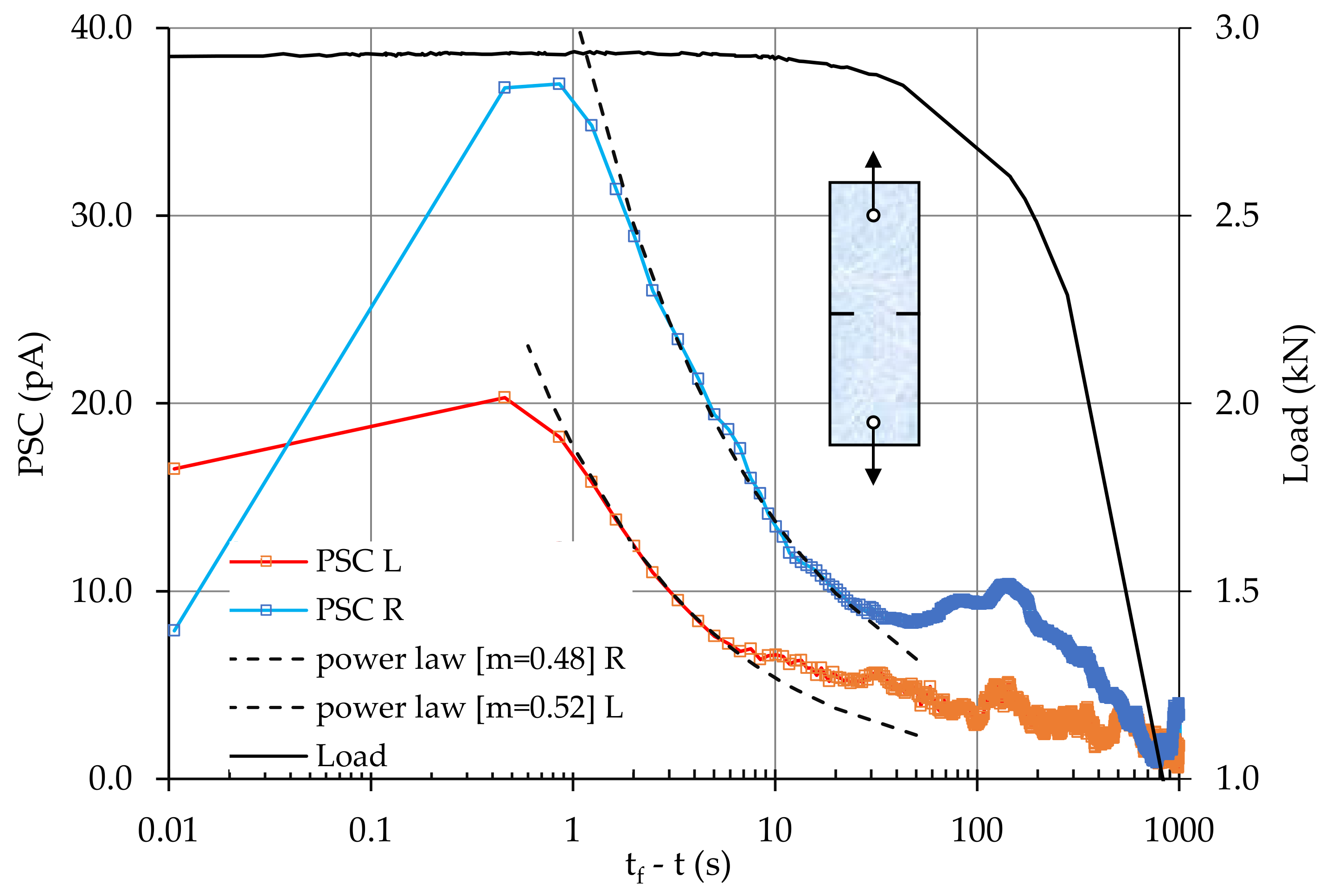
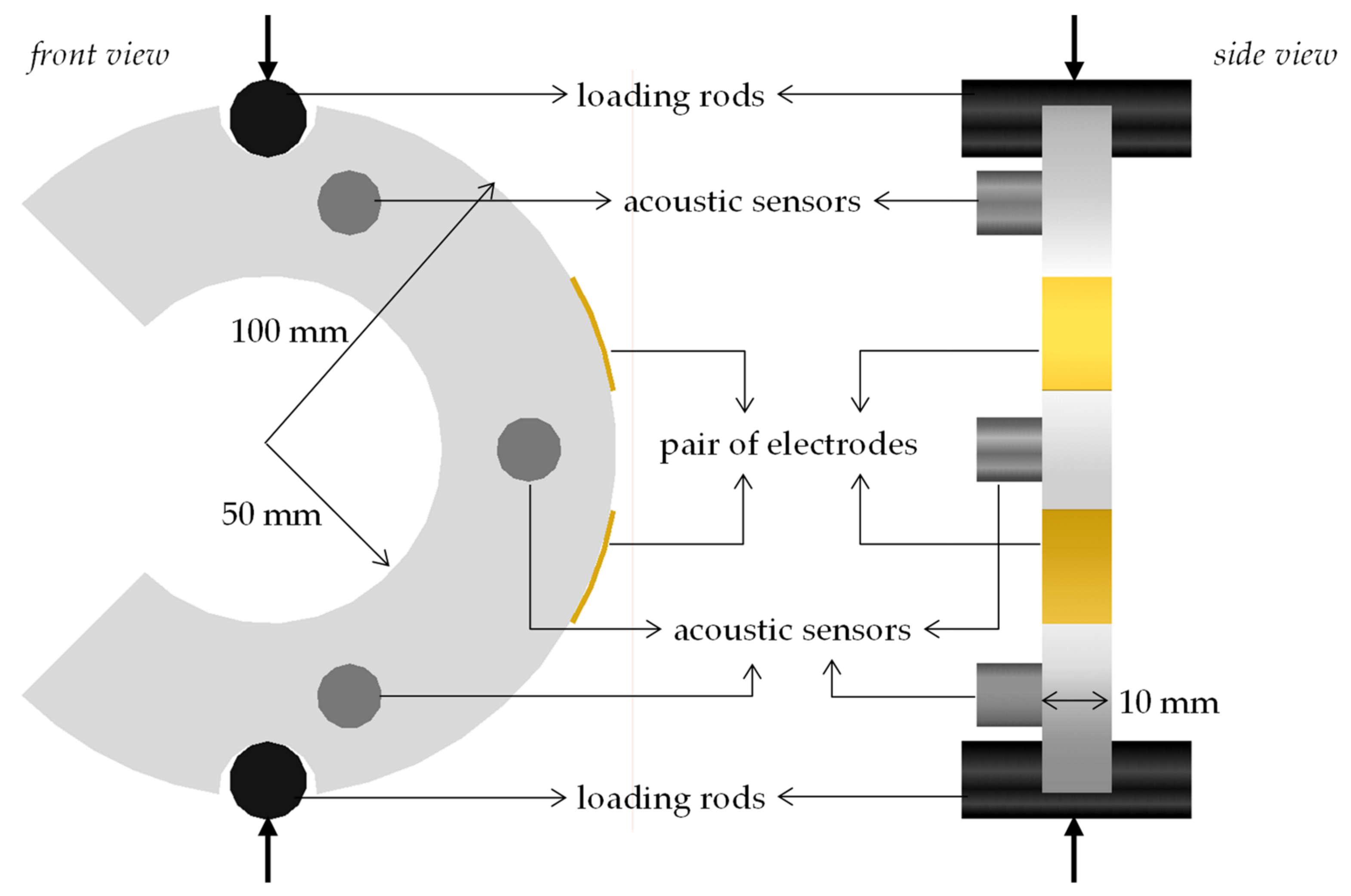
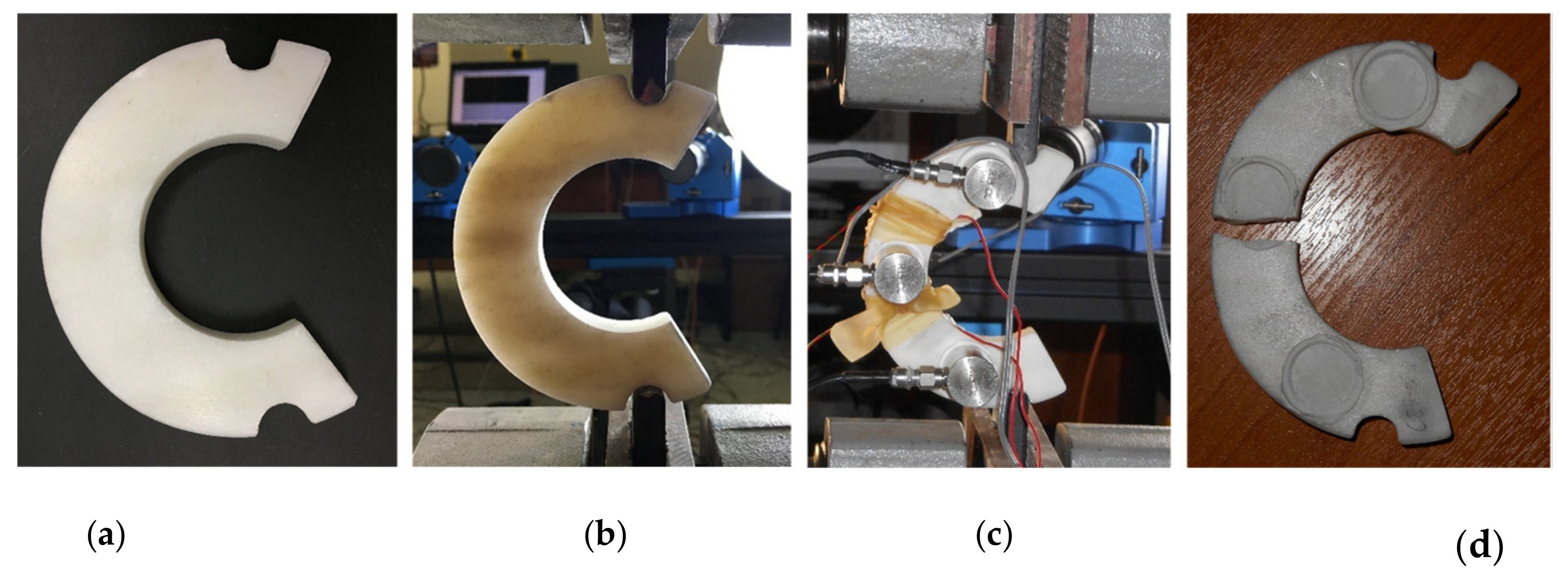
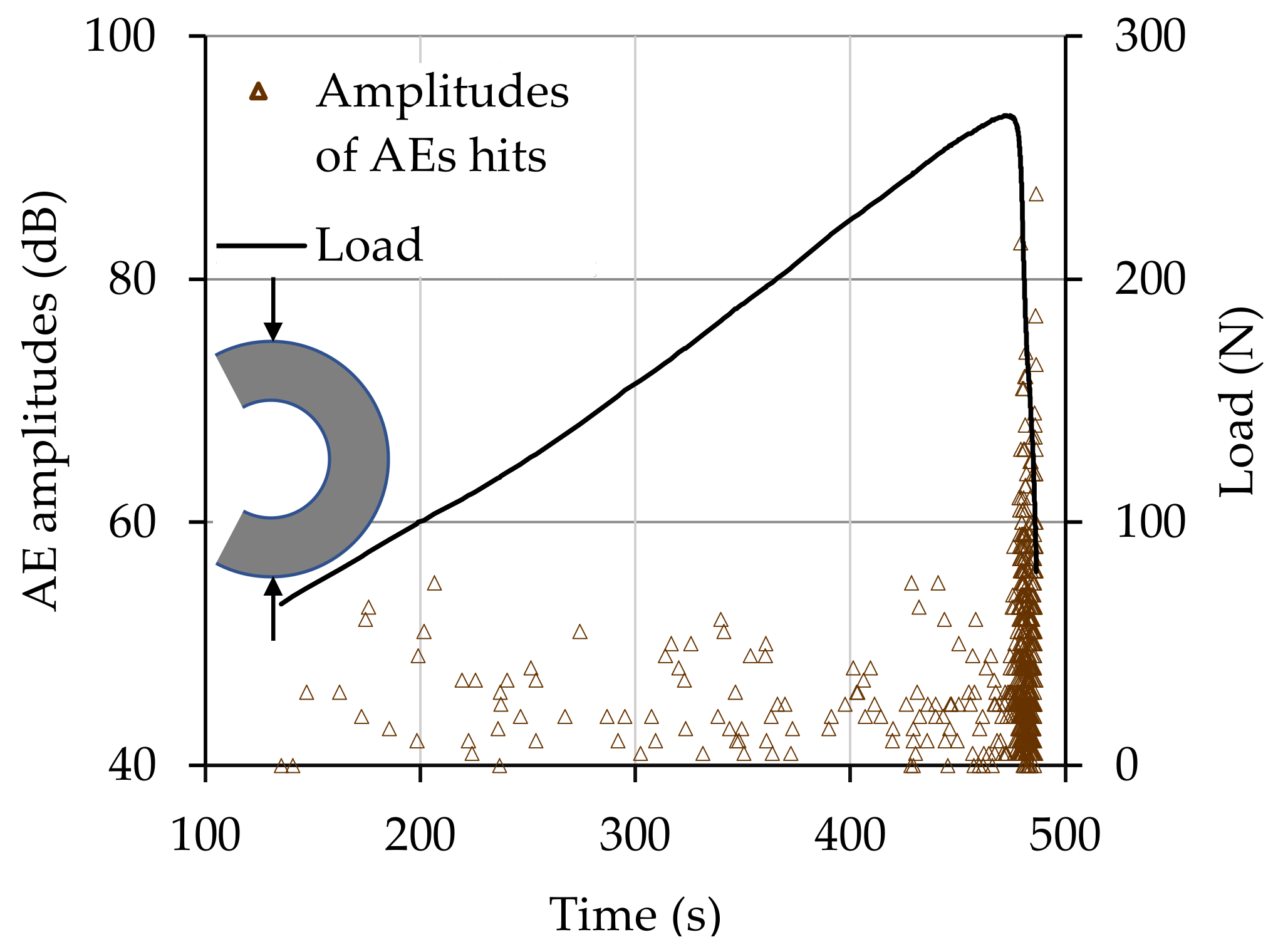
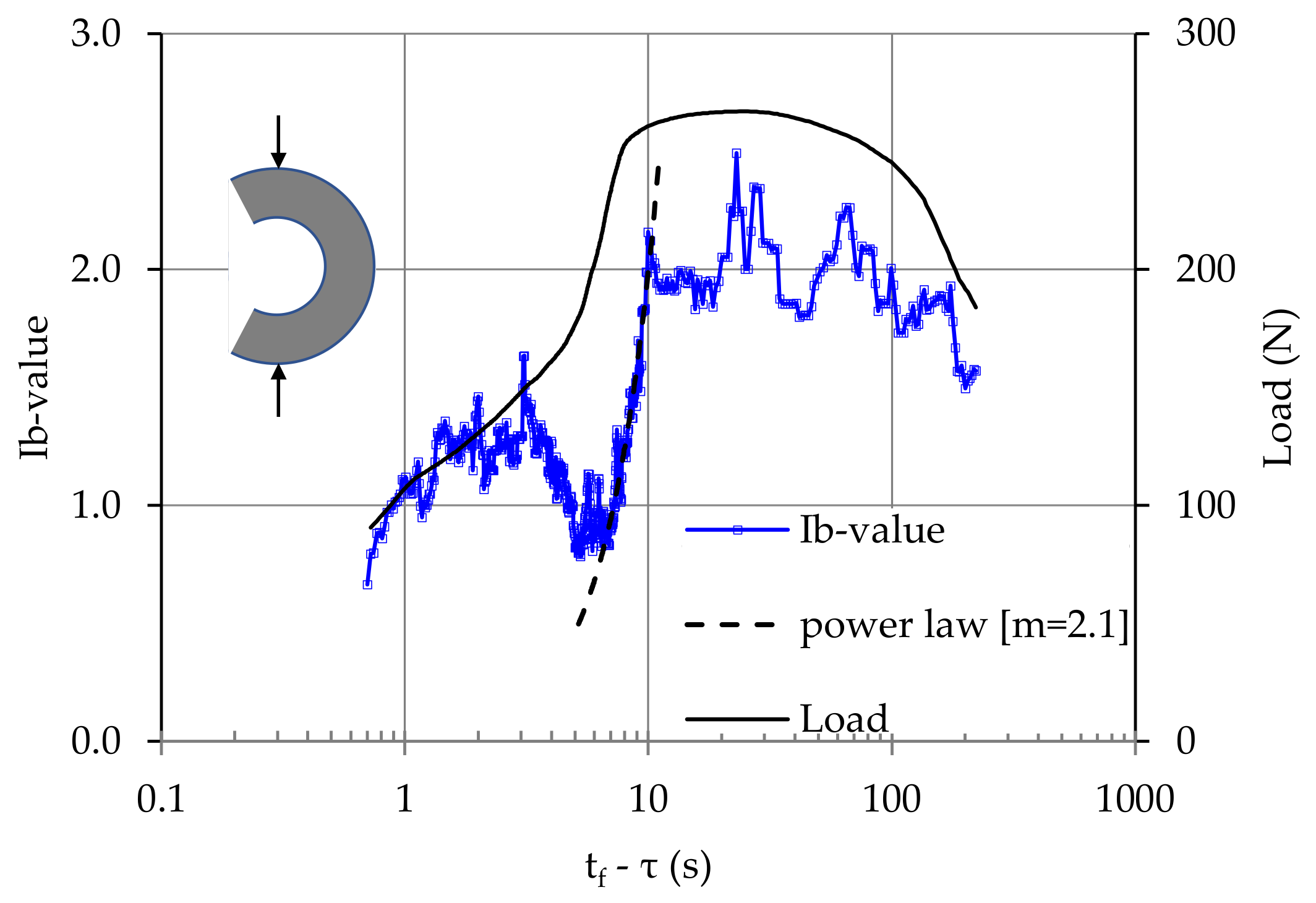
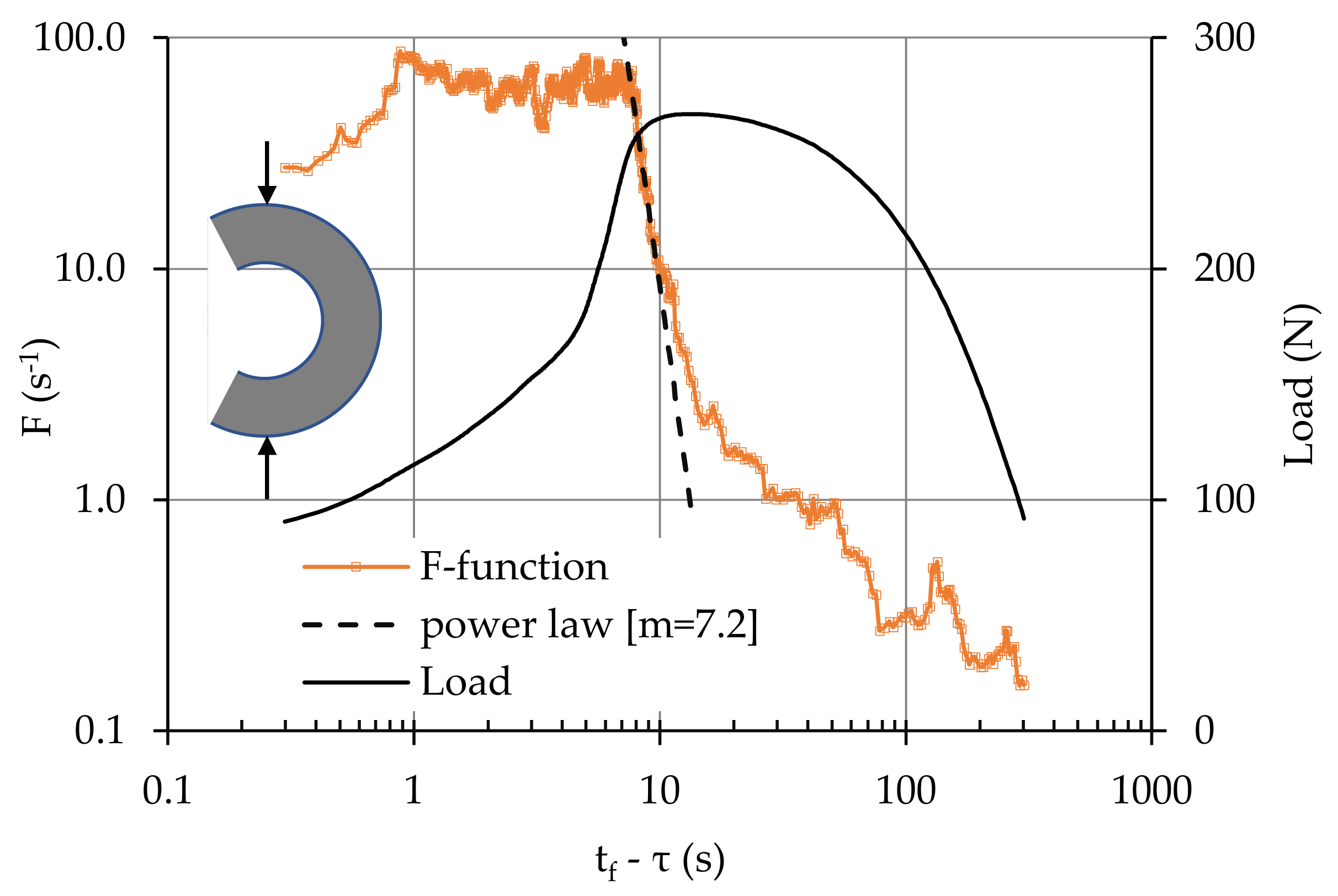
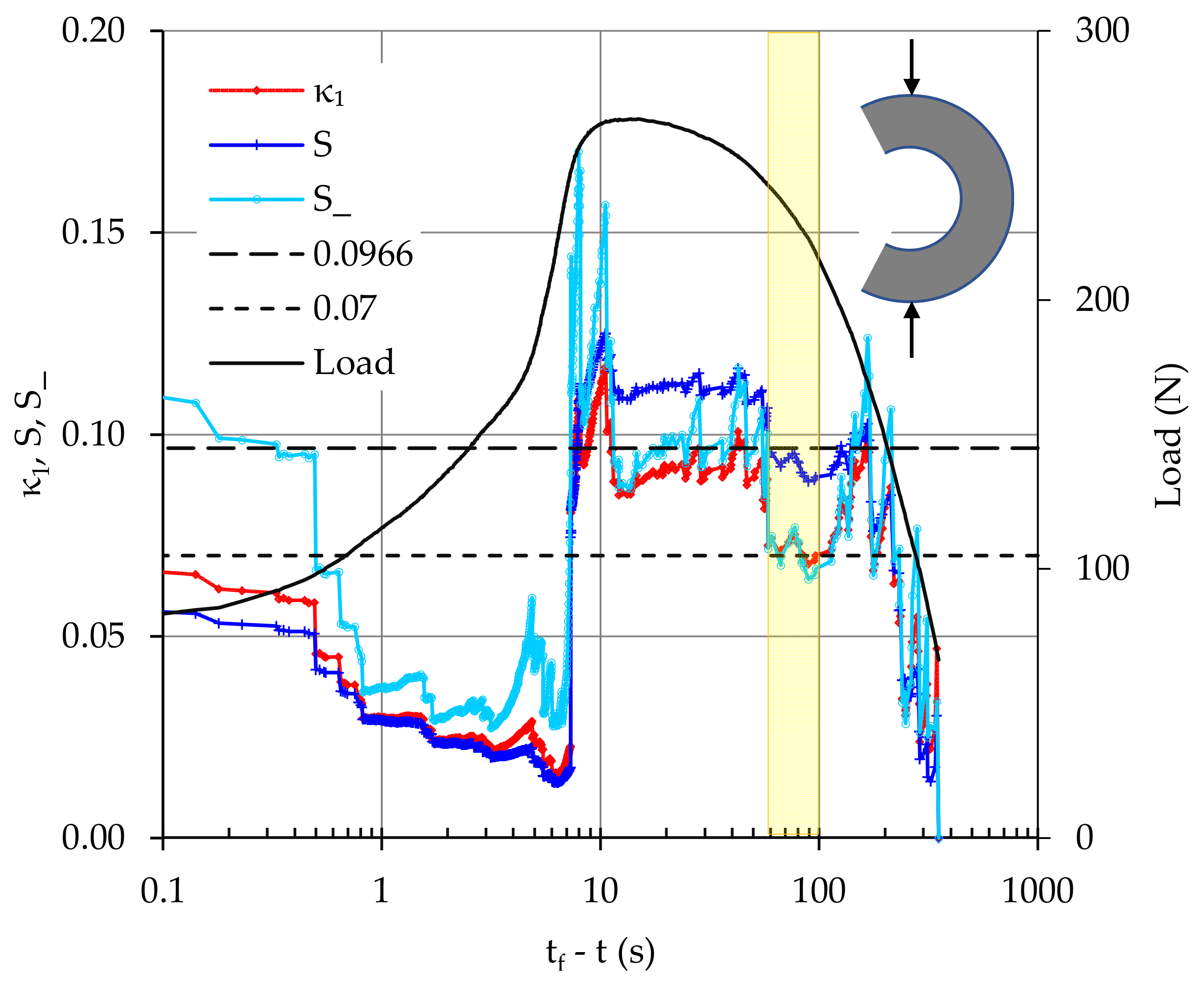
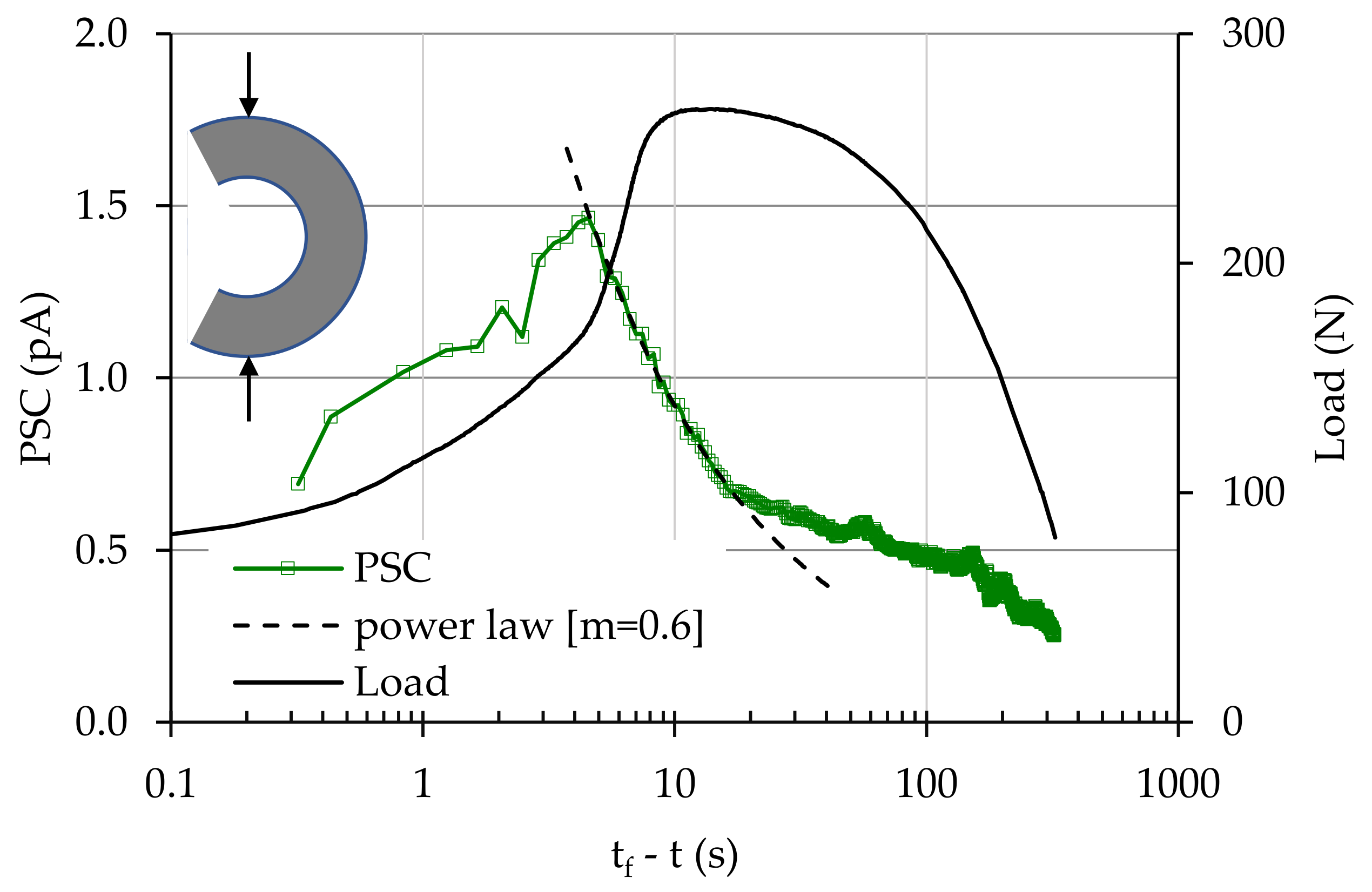
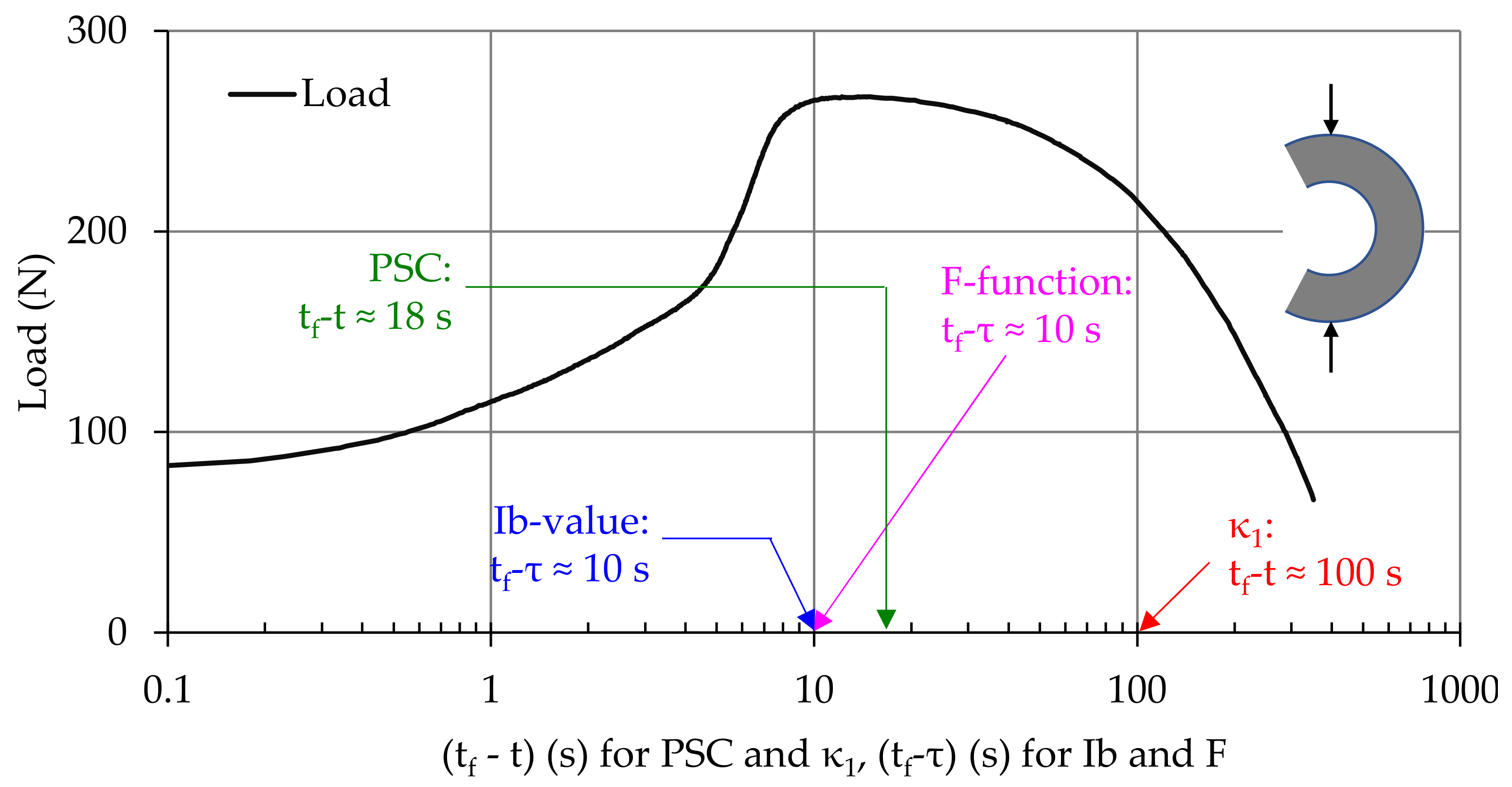
3.2. Diametral Compression of Circular Semi Rings
4. Discussion and Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Grosse, C.U.; Ohtsu, M. (Eds.) Acoustic Emission Testing; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Ohtsu, M. The history and development of acoustic emission in concrete engineering. Mag. Concr. Res. 1996, 48, 321–330. [Google Scholar] [CrossRef]
- Behnia, A.; Chai, H.K.; Shiotani, T. Advanced structural health monitoring of concrete structures with the aid of acoustic emission. Constr. Build. Mater. 2014, 65, 282–302. [Google Scholar] [CrossRef]
- Varotsos, P.A. The Physics of Seismic Electric Signals; TerraPub: Tokyo, Japan, 2005. [Google Scholar]
- Varotsos, P.A.; Sarlis, N.V.; Skordas, E.S. Attempt to distinguish electric signals of a dichotomous nature. Phys. Rev. E 2003, 68, 031106. [Google Scholar] [CrossRef] [PubMed]
- Surkov, V.; Hayakawa, M. Laboratory Study of Rock Deformation and Fracture. In Ultra and Extremely Low Frequency Electromagnetic Fields; Springer Geophysics: Tokyo, Japan, 2014; pp. 335–372. [Google Scholar]
- Vallianatos, F.; Triantis, D.; Tzanis, A.; Anastasiadis, C.; Stavrakas, I. Electric earthquake precursors: From laboratory results to field observations. Phys. Chem. Earth 2004, 29, 339–351. [Google Scholar] [CrossRef]
- Aggelis, D.G.; Soulioti, D.V.; Sapouridis, N.; Barkoula, N.M.; Paipetis, A.S.; Matikas, T.E. Acoustic emission characterization of the fracture process in fibre reinforced concrete. Constr. Build. Mater. 2011, 25, 4126–4131. [Google Scholar] [CrossRef]
- Kourkoulis, S.K.; Pasiou, E.D.; Dakanali, I.; Stavrakas, I.; Triantis, D. Notched marble plates under tension: Detecting pre-failure indicators and predicting entrance to the “critical stage”. Fatigue Fract. Eng. Mater. Struct. 2018, 41, 776–786. [Google Scholar] [CrossRef]
- Shearer, P.M. Introduction to Seismology; Cambridge University Press: Cambridge, UK, 1999; pp. 1–189. [Google Scholar]
- Colombo, S.; Main, I.G.; Forde, M.C. Assessing damage of reinforced concrete beam using ‘‘b-value’’ analysis of acoustic emission signals. J. Mater. Civ. Eng. 2003, 15, 280–286. [Google Scholar] [CrossRef]
- Vallianatos, F.; Michas, G.; Hloupis, G. Seismicity patterns prior to the Thessaly (Mw6. 3) strong earthquake on 3 March 2021 in terms of multiresolution wavelets and natural time analysis. Geosciences 2021, 11, 379. [Google Scholar] [CrossRef]
- Contoyiannis, Y.F.; Potirakis, S.M.; Diakonos, F.K. Wavelet-based detection of scaling behavior in noisy experimental data. Phys. Rev. E 2020, 101, 052104. [Google Scholar] [CrossRef]
- Varotsos, P.A.; Sarlis, N.V.; Skordas, E.S. Natural Time Analysis: The New View of Time: Precursory Seismic Electric Signals, Earthquakes and Other Complex Time Series; Springer: Heidelberg, Germany, 2011. [Google Scholar]
- Varotsos, P.A.; Sarlis, N.V.; Skordas, E.S.; Tanaka, H.K.; Lazaridou, M.S. Entropy of seismic electric signals: Analysis in natural time under time reversal. Phys. Rev. E 2006, 73, 0311144. [Google Scholar] [CrossRef]
- Tsallis, C. Possible generalization of Boltzmann-Gibbs statistics. J. Stat. Phys. 1988, 52, 479–487. [Google Scholar] [CrossRef]
- Tsallis, C. Nonadditive entropy Sq and nonextensive statistical mechanics: Applications in geophysics and elsewhere. Acta Geophys. 2012, 60, 502–525. [Google Scholar] [CrossRef]
- Triantis, D.; Pasiou, E.D.; Stavrakas, I.; Kourkoulis, S.K. Hidden affinities between electric and acoustic activity in brittle materials at near-fracture load levels. Rock Mech. Rock Eng. 2022, 1–18. [Google Scholar] [CrossRef]
- Tzanis, A.; Vallianatos, F. Distributed power-law seismicity changes and crustal deformation in the SW Hellenic ARC. Nat. Hazards Earth Syst. Sci. 2003, 3, 179–195. [Google Scholar] [CrossRef]
- Iowa State University, Nondestructive Evaluation Technique: History of Acoustic Emission Testing. Available online: https://www.nde-ed.org/NDETechniques/AcousticEmission/AE_History.xhtml (accessed on 8 December 2021).
- Kishinouye, F. An experiment on the progression of fracture. (A preliminary report). Jisin 6:24-31(1934) translated and published by Ono K. J. Acoust. Emiss. 1990, 9, 177–180. [Google Scholar]
- Förster, F. Akustische untersuchung der bildung von martensitnadeln (Acoustic study of the formation of martensile needels). Z. Für Met. 1936, 29, 245. [Google Scholar]
- Kaiser, E. A Study of Acoustic Phenomena in Tensile Test. Ph.D. Thesis, Technical University of Munich, Munich, Germany, 1950. [Google Scholar]
- Grosse, C.U.; Ohtsu, M.; Aggelis, D.G.; Shiotani, T. (Eds.) Acoustic Emission Testing: Basics for Research-Applications in Engineering; Springer Nature: Cham, Switzerland, 2021. [Google Scholar]
- Sause, M.G.R. In Situ Monitoring of Fiber-Reinforced Composites: Theory, Basic Concepts, Methods, and Applications; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar]
- Mohammad, I.; Huang, H. Monitoring fatigue crack growth and opening using antenna sensors. Smart Mater. Struct. 2010, 19, 055023. [Google Scholar] [CrossRef]
- Sharma, S.K.; Sivarathri, A.K.; Chauhan, V.S.; Sinapius, M. Effect of low temperature on electromagnetic radiation from soft PZT SP-5A under impact loading. J. Electron. Mater. 2018, 47, 5930–5938. [Google Scholar] [CrossRef]
- Stavrakas, I.; Anastasiadis, C.; Triantis, D.; Vallianatos, F. Piezo stimulated currents in marble samples: Precursory and concurrent-with-failure signals. Nat. Hazards Earth Syst. Sci. 2003, 3, 243–247. [Google Scholar] [CrossRef]
- Kyriazopoulos, A.; Anastasiadis, C.; Triantis, D.; Brown, C.J. Non-destructive evaluation of cement-based materials from pressure-stimulated electrical emission—Preliminary results. Constr. Build. Mater. 2011, 25, 1980–1990. [Google Scholar] [CrossRef]
- Enomoto, J.; Hashimoto, H. Emission of charged particles from indentation fracture of rocks. Nature 1990, 346, 641–643. [Google Scholar] [CrossRef]
- Ogawa, T.K.; Miura, T. Electromagnetic radiation from rocks. J. Geophys. Res. 1985, 90, 6245–6249. [Google Scholar] [CrossRef]
- Archer, J.W.; Dobbs, M.R.; Aydin, A.; Reeves, H.J.; Prance, R.J. Measurement and correlation of acoustic emissions and pressure stimulated voltages in rock using an electric potential sensor. Int. J. Rock Mech. Min. 2016, 89, 26–33. [Google Scholar] [CrossRef]
- Niu, Y.; Wang, C.J.; Wang, E.Y.; Li, Z.H. Experimental study on the damage evolution of gas-bearing coal and its electric potential response. Rock Mech. Rock Eng. 2019, 52, 4589–4604. [Google Scholar] [CrossRef]
- Triantis, D.; Anastasiadis, C.; Stavrakas, I. The correlation of electrical charge with strain on stressed rock samples. Nat. Hazards Earth Syst. 2008, 8, 1243–1248. [Google Scholar] [CrossRef]
- Zhang, X.; Li, Z.H.; Niu, Y.; Cheng, F.Q.; Ali, M.; Bacha, S. An experimental study on the precursory characteristics of EP before sandstone failure based on critical slowing down. J. Appl. Geophys. 2019, 170, 103818. [Google Scholar] [CrossRef]
- Whitworth, R.W. Charged dislocations in ionic crystals. Adv. Phys. 1975, 24, 203–304. [Google Scholar] [CrossRef]
- Varotsos, P.A.; Sarlis, N.V.; Skordas, E.S. Long-range correlations in the electric signals that precede rupture. Phys. Rev. E 2002, 66, 011902. [Google Scholar] [CrossRef]
- Saltas, V.; Vallianatos, F.; Triantis, D.; Stavrakas, I. Complexity in Laboratory Seismology: From Electrical and Acoustic Emissions, to Fracture. In Complexity Measurement and Application to Seismic Time Series, Measurement and Application; Elsevier: Amsterdam, The Netherlands, 2008; pp. 239–273. [Google Scholar]
- Takeuchi, A.; Aydan, Ö.; Sayanagi, K.; Nagao, T. Generation of electromotive force in igneous rocks subjected to non-uniform loading. Earthq. Sci. 2011, 24, 593–600. [Google Scholar] [CrossRef][Green Version]
- Slifkin, L. Seismic electric signals from displacement of charged dislocations. Tectonophysics 1993, 224, 149–152. [Google Scholar] [CrossRef]
- Vallianatos, F.; Tzanis, A. On possible scaling laws between electric earthquake precursors (EEP) and earthquake magnitude. Geophys. Res. Lett. 1999, 26, 2013–2016. [Google Scholar] [CrossRef]
- Sammonds, P.R.; Meredith, P.G.; Murrel, S.A.F.; Main, I.G. Modelling the damage evolution in rock containing pore fluid by acoustic emission. In Rock Mechanics in Petroleum Engineering; OnePetro: Delft, The Netherlands, 1994. [Google Scholar]
- Shiotani, T.; Yuyama, S.; Li, Z.W.; Othsu, M. Quantitative Evaluation of Fracture Process in Concrete by the Use of Improved b-value. In 5th International Symposium Non-Destructive Testing in Civil Engineering; Uohoto, T., Ed.; Elsevier Science: Amsterdam, The Netherlands, 2000; pp. 293–302. [Google Scholar]
- Triantis, D.; Kourkoulis, S.K. An alternative approach for representing the data provided by the acoustic emission technique. Rock Mech. Rock Eng. 2018, 51, 2433–2438. [Google Scholar] [CrossRef]
- Zhang, J.Z.; Zhou, X.P.; Zhou, L.S.; Berto, F. Progressive failure of brittle rocks with non-isometric flaws: Insights from acousto-optic-mechanical (AOM) data. Fatigue Fract. Eng. Mater. Struct. 2019, 42, 1787–1802. [Google Scholar] [CrossRef]
- Wang, X.; Wang, E.; Liu, X. Damage characterization of concrete under multi-step loading by integrated ultrasonic and acoustic emission techniques. Constr. Build. Mater. 2019, 221, 678–690. [Google Scholar] [CrossRef]
- Yao, W.; Yu, J.; Liu, X.; Zhou, X.; Cai, Y.; Zhu, Y.L. Study on acoustic emission characteristics and failure prediction of post-high-temperature granite. J. Test. Eval. 2019, 48, 2459–2473. [Google Scholar] [CrossRef]
- Ge, Z.; Sun, Q. Acoustic emission characteristics of gabbro after microwave heating. Rock Mech. Rock Eng. 2021, 138, 104616. [Google Scholar] [CrossRef]
- Varotsos, P.A.; Sarlis, N.V.; Tanaka, H.K.; Skordas, E.S. Similarity of fluctuations in correlated systems: The case of seismicity. Phys. Rev. E 2005, 72, 041103. [Google Scholar] [CrossRef] [PubMed]
- Uyeda, S.; Kamogawa, M.; Tanaka, H. Analysis of electrical activity and seismicity in the natural time domain for the volcanic-seismic swarm activity in 2000 in the Izu Island region, Japan. J. Geophys. Res. 2009, 114, B02310. [Google Scholar] [CrossRef]
- Vallianatos, F.; Michas, G.; Papadakis, G. Non-extensive and natural time analysis of seismicity before the Mw6.4, 12 October 2013 earthquake in the South West segment of the Hellenic Arc. Physica A 2014, 414, 163–173. [Google Scholar] [CrossRef]
- Varotsos, P.A.; Sarlis, N.V.; Skordas, E.S.; Lazaridou, M.S. Identifying sudden cardiac death risk and specifying its occurrence time by analyzing electrocardiograms in natural time. Appl. Phys. Lett. 2007, 91, 064106. [Google Scholar] [CrossRef]
- Baldoumas, G.; Peschos, D.; Tatsis, G.; Chronopoulos, S.K.; Christofilakis, V.; Kostarakis, P.; Varotsos, P.; Sarlis, N.V.; Skordas, E.S.; Bechlioulis, A.; et al. A prototype photoplethysmography electronic device that distinguishes congestive heart failure from healthy individuals by applying natural time analysis. Electronics 2019, 8, 1288. [Google Scholar] [CrossRef]
- Sarlis, N.V.; Skordas, E.S.; Varotsos, P.A. Heart rate variability in natural time and 1/f “noise”. Eur. Lett. 2009, 87, 18003. [Google Scholar] [CrossRef]
- Sarlis, N.V.; Varotsos, P.A.; Skordas, E.S. Flux avalanches in YBa2Cu3O7−x films and rice piles: Natural time domain analysis. Phys. Rev. B 2006, 73, 054504. [Google Scholar] [CrossRef]
- Vallianatos, F.; Michas, G.; Benson, P.; Sammonds, P. Natural time analysis of critical phenomena: The case of acoustic emissions in triaxially deformed Etna basalt. Physica A 2013, 392, 5172–5178. [Google Scholar] [CrossRef]
- Hloupis, G.; Stavrakas, I.; Pasiou, E.D.; Triantis, D.; Kourkoulis, S.K. Natural time analysis of acoustic emissions in double edge notched tension (DENT) marble specimens. Procedia Eng. 2015, 109, 248–256. [Google Scholar] [CrossRef]
- Loukidis, A.; Pasiou, E.D.; Sarlis, N.V.; Triantis, D. Fracture analysis of typical construction materials in natural time. Physica A 2020, 547, 123831. [Google Scholar] [CrossRef]
- Triantis, D.; Stavrakas, I.; Anastasiadis, C.; Kyriazopoulos, A.; Vallianatos, F. An analysis of pressure stimulated currents (PSC), in marble samples under mechanical stress. Phys. Chem. Earth 2006, 31, 234–239. [Google Scholar] [CrossRef]
- Cartwright-Taylor, A.; Vallianatos, F.; Sammonds, P. Superstatistical view of stress-induced electric current fluctuations in rocks. Physica A 2014, 414, 368–377. [Google Scholar] [CrossRef]
- Zhonghui, L.; Wang, E.; He, M. Laboratory studies of electric current generated during fracture of coal and rock in rock burst coal mine. J. Min. 2015, 235636. [Google Scholar]
- Zhang, X.; Li, Z.; Wang, E.; Li, B.; Song, J.; Niu, Y. Experimental investigation of ressure stimulated currents and acoustic emissions from sandstone and gabbro samples subjected to multi-stage uniaxial loading. Bull. Eng. Geol. Environ. 2021, 80, 7683–7700. [Google Scholar] [CrossRef]
- Li, D.; Wang, E.; Li, Z.; Ju, Y.; Wang, D.; Wang, X. Experimental investigations of pressure stimulated currents from stressed sandstone used as precursors to rock fracture. Int. J. Rock Mech. Min. 2021, 145, 104841. [Google Scholar] [CrossRef]
- Li, D.X.; Wang, E.Y.; Ju, Y.Q.; Wang, D.M. Laborator.ry investigations of a new method using pressure stimulated currents to monitor concentrated stress variations in coal. Nat. Resour. Res. 2021, 30, 707–724. [Google Scholar] [CrossRef]
- Aydin, A.; Prance, R.J.; Prance, H.; Harland, C.J. Observation of pressure stimulated voltages in rocks using an electric potential sensor. Appl. Phys. Lett. 2009, 95, 124102. [Google Scholar] [CrossRef]
- Dann, D.; Demikhova, A.; Fursa, T.; Kuimova, M. Research of electrical response communication parameters on the pulse mechanical impact with the stress-strain state of concrete under uniaxial compression. IOP Conf. Ser. Mater. Sci. Eng. 2014, 66, 012036. [Google Scholar] [CrossRef]
- Niu, Y.; Li, Z.; Kong, B.; Wang, E.; Lou, Q.; Qiu, L.; Kong, X.; Wang, J.; Dong, M.; Li, B. Similar simulation study on the characteristics of the electric potential response to coal mining. J. Geophys. Eng. 2017, 15, 42–50. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, X.; Wei, Y.; Ali, M. Experimental study of electric potential response characteristics of different lithological samples subject to uniaxial loading. Rock Mech. Rock Eng. 2021, 54, 397–408. [Google Scholar] [CrossRef]
- Pasiou, E.D.; Triantis, D. Correlation between the electric and acoustic signals emitted during compression of brittle materials. Frat. Ed. Integrità Strutt. 2017, 40, 41–51. [Google Scholar] [CrossRef]
- Castro, B.A.; Baptista, F.G.; Ardila Rey, J.A.; Ciampa, F. A chromatic technique for structural damage detection under noise effects based on impedance measurements. Meas. Sci. Technol. 2019, 30, 075601. [Google Scholar] [CrossRef]
- Vardoulakis, I.; Exadaktylos, G.; Kourkoulis, S.K. Bending of marble with intrinsic length scales: A gradient theory with surface energy and size effects. Le J. De Phys. IV 1998, 8, 399–406. [Google Scholar] [CrossRef]
- Kourkoulis, S.K.; Exadaktylos, G.E.; Vardoulakis, I. U-notched Dionysos-Pentelicon marble in three-point bending: The effect of nonlinearity, anisotropy and microstructure. Int. J. Fract. 1999, 98, 369–392. [Google Scholar] [CrossRef]
- Exadaktylos, G.E.; Vardoulakis, I.; Kourkoulis, S.K. Influence of nonlinearity and double elasticity on flexure of rock beams—II. Characterization of Dionysos marble. Int. J. Solids Struct. 2001, 38, 4119–4145. [Google Scholar] [CrossRef]
- De Souza Campos, F.; De Castro, B.A.; Budoya, D.E.; Baptista, F.G.; Ulson, J.A.C.; Andreoli, A.L. Feature extraction approach insensitive to temperature variations for impedance-based structural health monitoring. IET Sci. Meas. Technol. 2019, 13, 536–543. [Google Scholar] [CrossRef]
- Markides, C.H.F.; Pasiou, E.D.; Kourkoulis, S.K. A preliminary study on the potentialities of the Circular Semi-Ring test. Procedia Struct. Integr. 2018, 9, 108–115. [Google Scholar] [CrossRef]
- Markides, C.H.F.; Stavropoulou, M.; Pasiou, E.D.; Kourkoulis, S.K. Enlightening the role of critical parameters for the determination of the tensile strength by means of the Circular Semi Ring test. Procedia Struct. Integr. 2020, 25, 214–225. [Google Scholar] [CrossRef]
- Triantis, D.; Stavrakas, I.; Loukidis, A.; Pasiou, E.D.; Kourkoulis, S.K. Exploring the acoustic activity in brittle materials in terms of the position of the acoustic sources and the power of the acoustic signals—Part I: Founding the approach. Forces Mech. 2022; submitted. [Google Scholar]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kourkoulis, S.K.; Pasiou, E.D.; Loukidis, A.; Stavrakas, I.; Triantis, D. Comparative Assessment of Criticality Indices Extracted from Acoustic and Electrical Signals Detected in Marble Specimens. Infrastructures 2022, 7, 15. https://doi.org/10.3390/infrastructures7020015
Kourkoulis SK, Pasiou ED, Loukidis A, Stavrakas I, Triantis D. Comparative Assessment of Criticality Indices Extracted from Acoustic and Electrical Signals Detected in Marble Specimens. Infrastructures. 2022; 7(2):15. https://doi.org/10.3390/infrastructures7020015
Chicago/Turabian StyleKourkoulis, Stavros K., Ermioni D. Pasiou, Andronikos Loukidis, Ilias Stavrakas, and Dimos Triantis. 2022. "Comparative Assessment of Criticality Indices Extracted from Acoustic and Electrical Signals Detected in Marble Specimens" Infrastructures 7, no. 2: 15. https://doi.org/10.3390/infrastructures7020015
APA StyleKourkoulis, S. K., Pasiou, E. D., Loukidis, A., Stavrakas, I., & Triantis, D. (2022). Comparative Assessment of Criticality Indices Extracted from Acoustic and Electrical Signals Detected in Marble Specimens. Infrastructures, 7(2), 15. https://doi.org/10.3390/infrastructures7020015