Joint Layout Design: Finding the Strongest Connections within Segmental Masonry Arched Forms
Abstract
:1. Introduction
1.1. Segmentation of Masonry Curved Structures throughout History
1.2. Innovative Design and Construction Methods for Masonry Curved Structures
1.3. Research Objectives
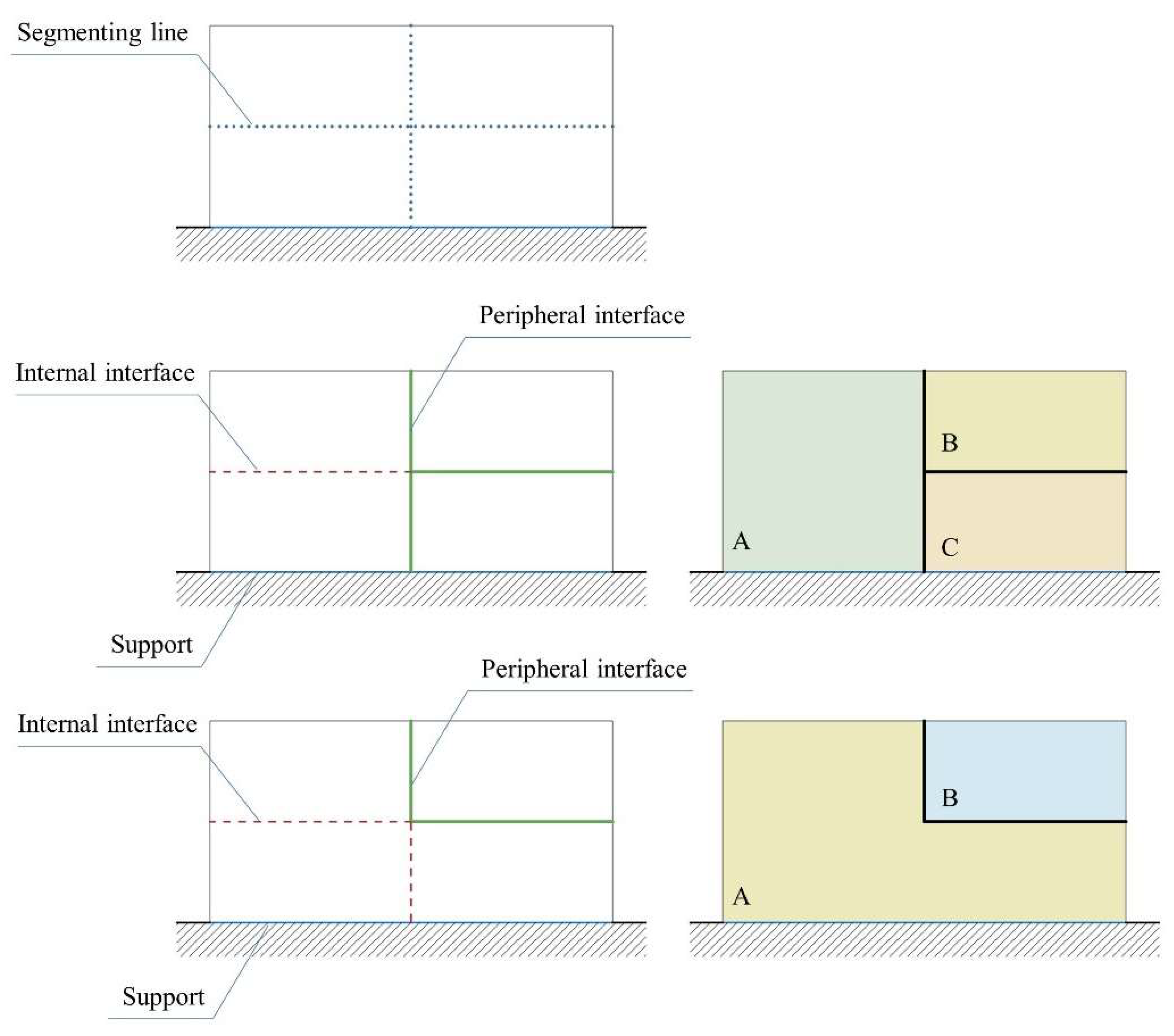
2. Joint Layout Optimization
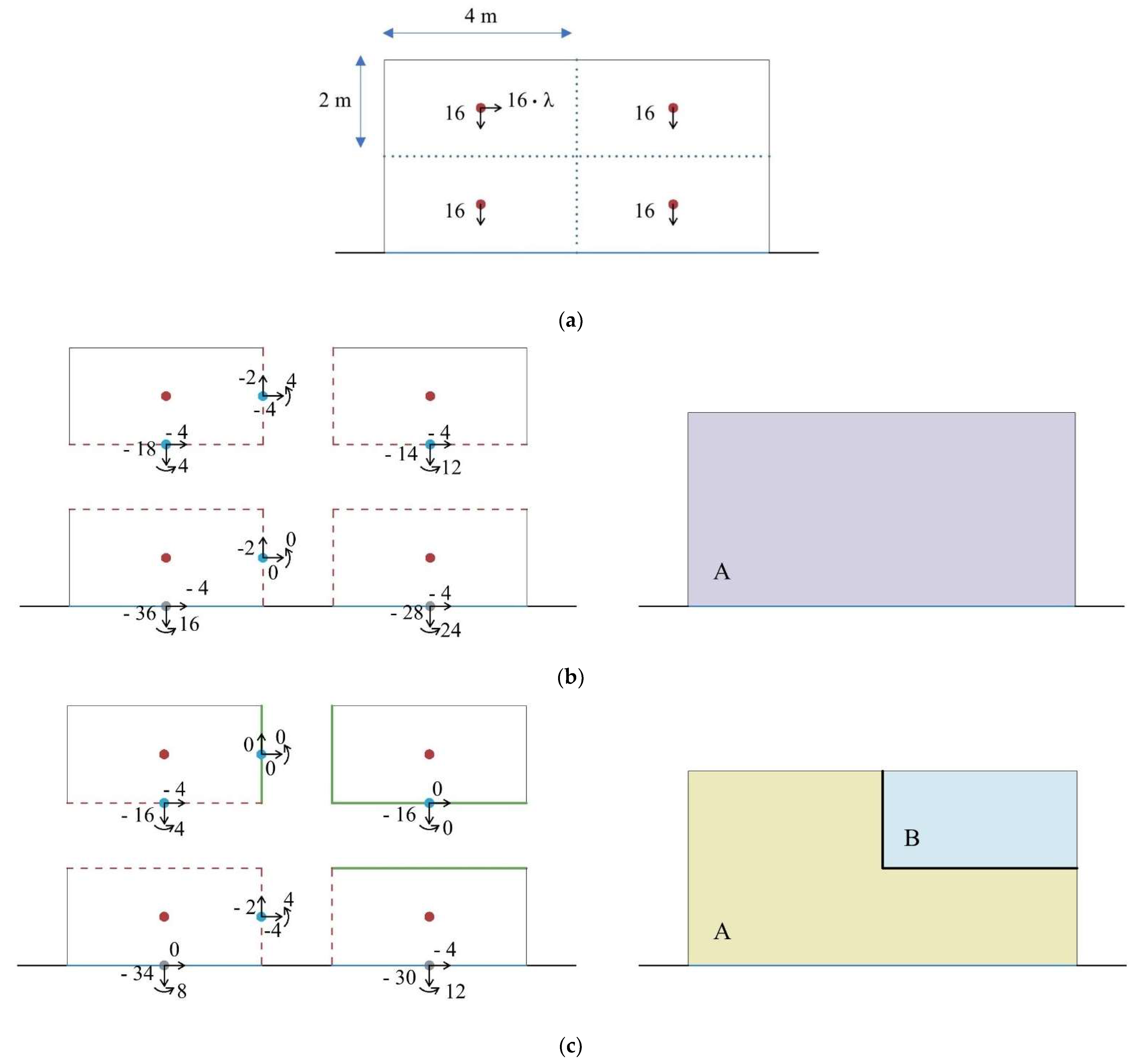
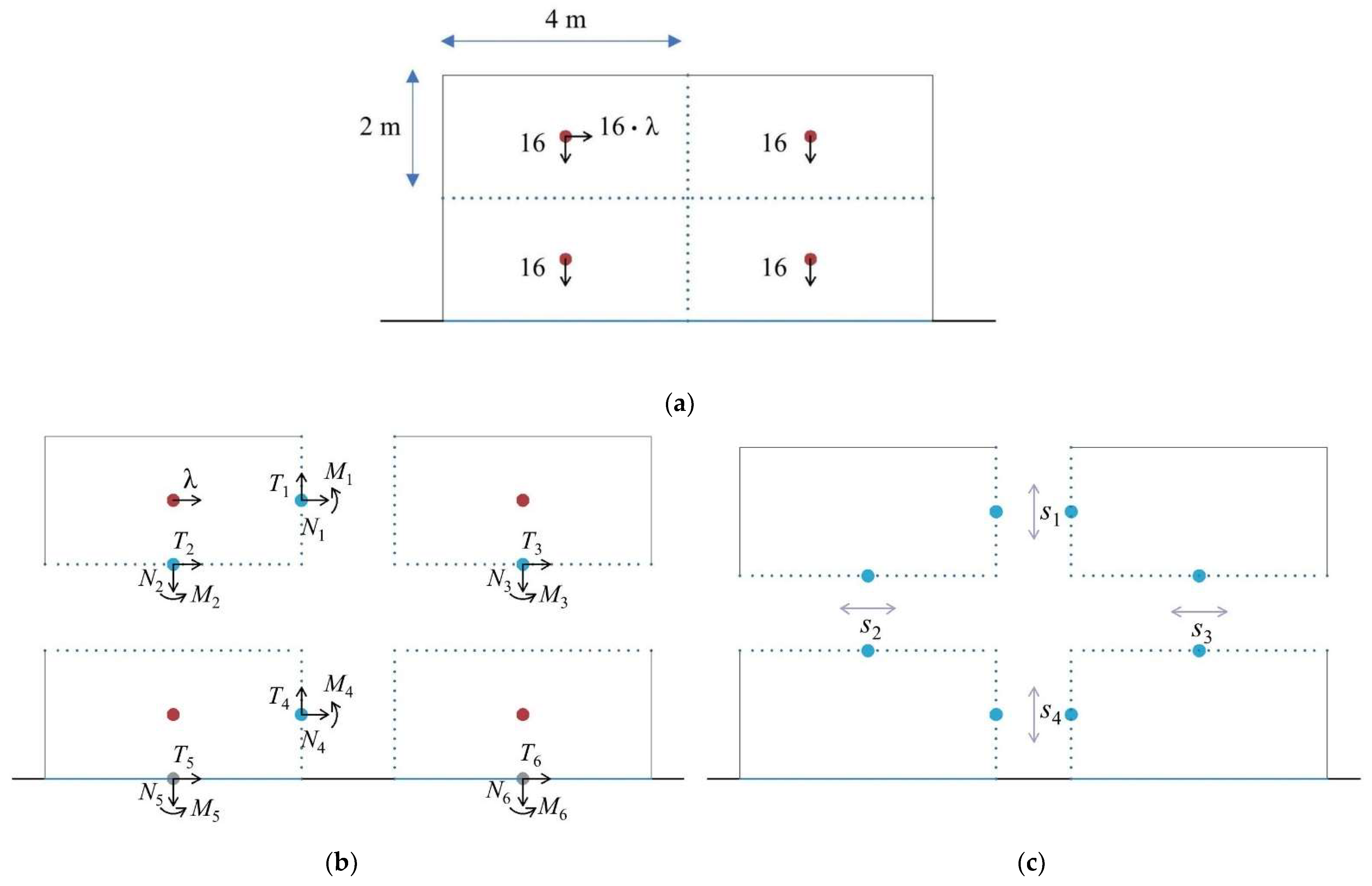
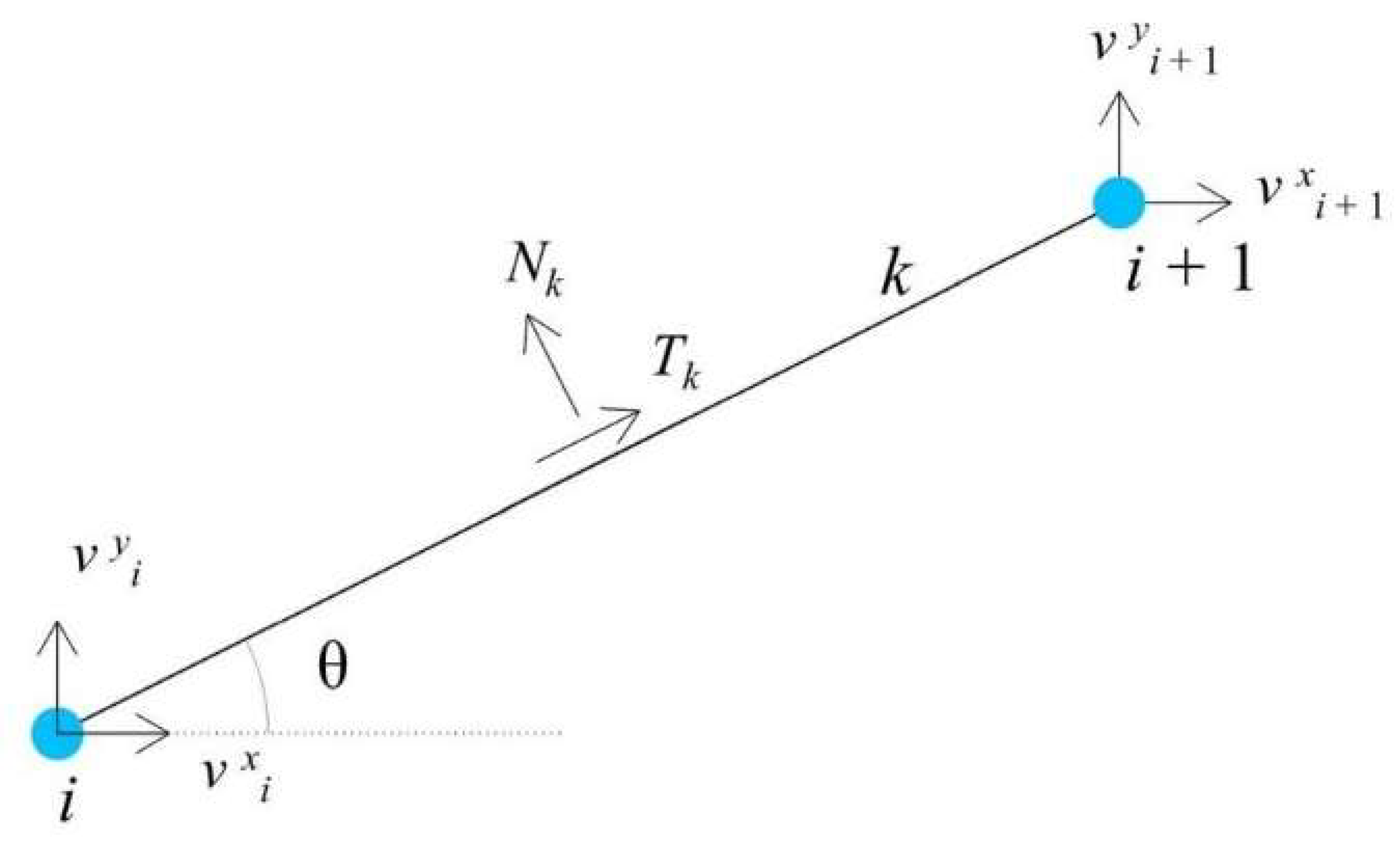
2.1. Load Bearing Capacity of a Segmental Model
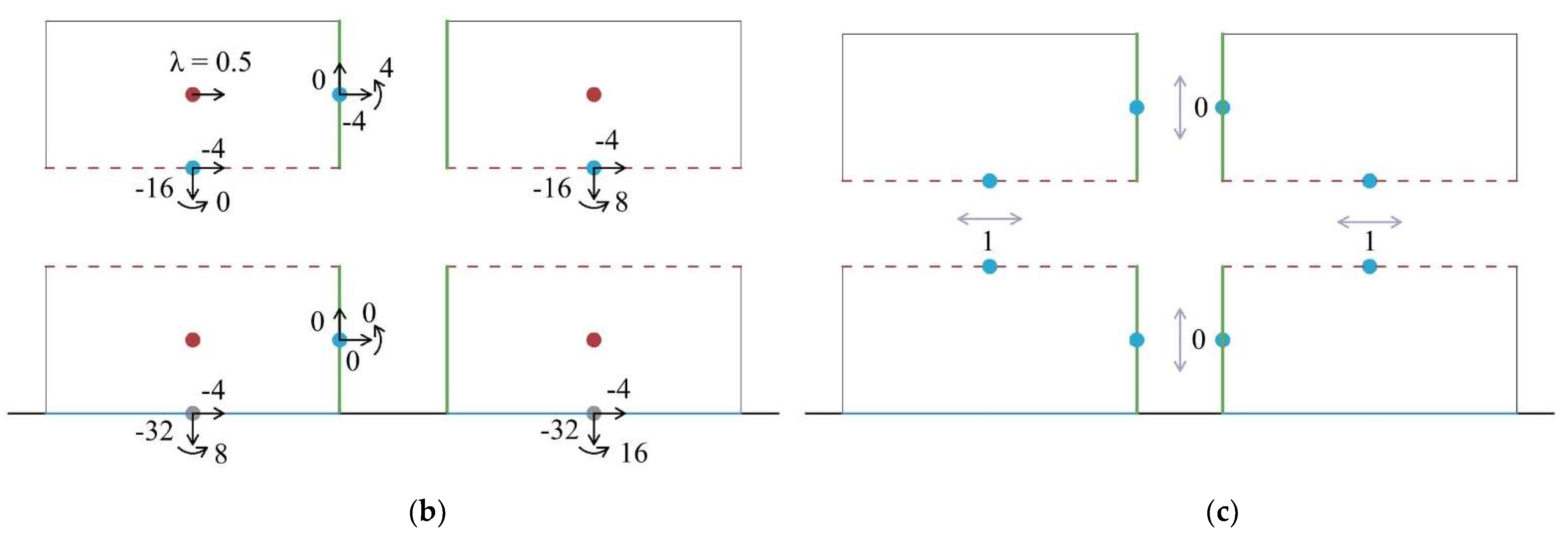
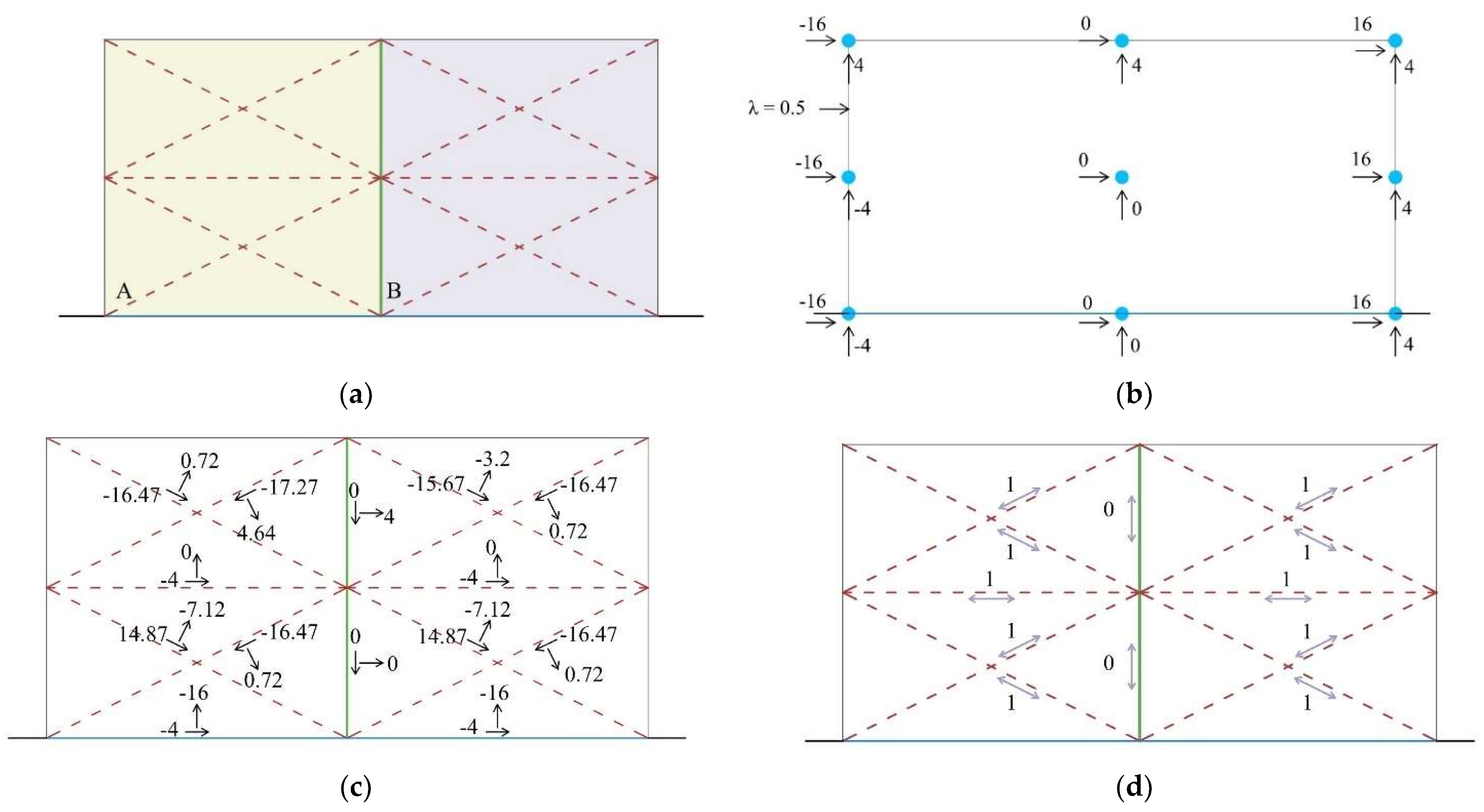
2.2. Joint Layout Optimization Based on Classic Limit Analysis
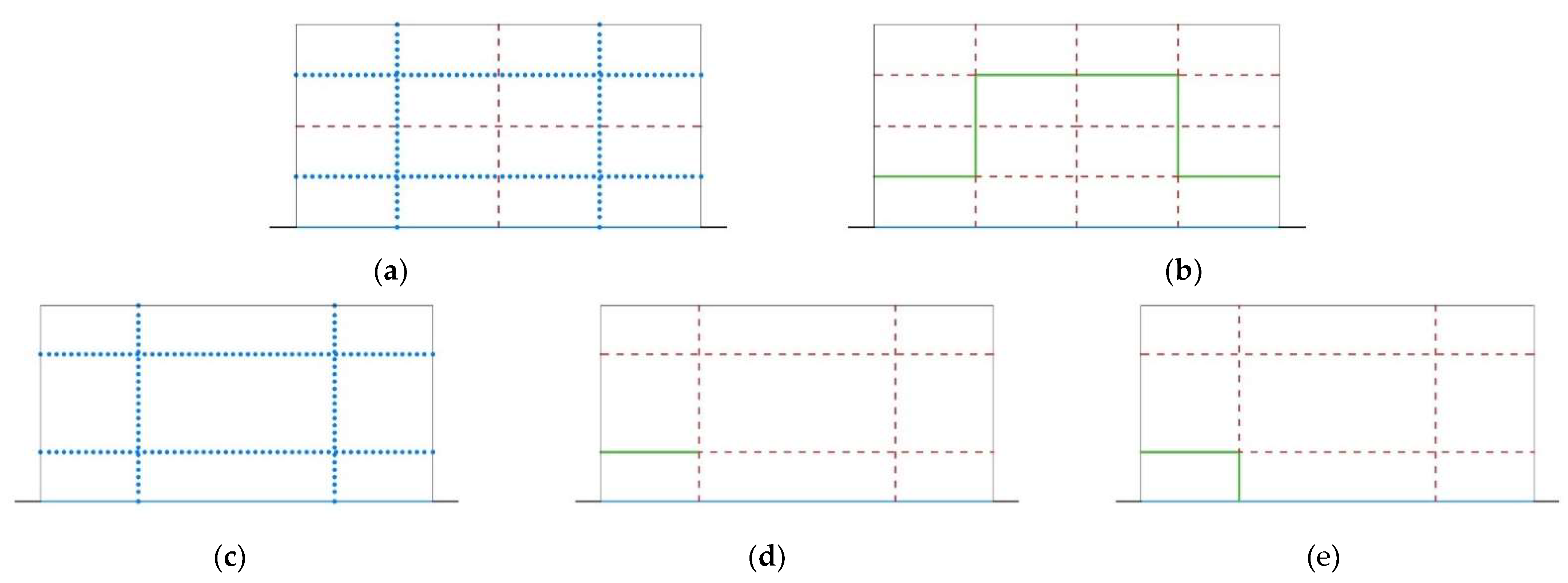
2.3. Joint Layout Optimization Based on DLO
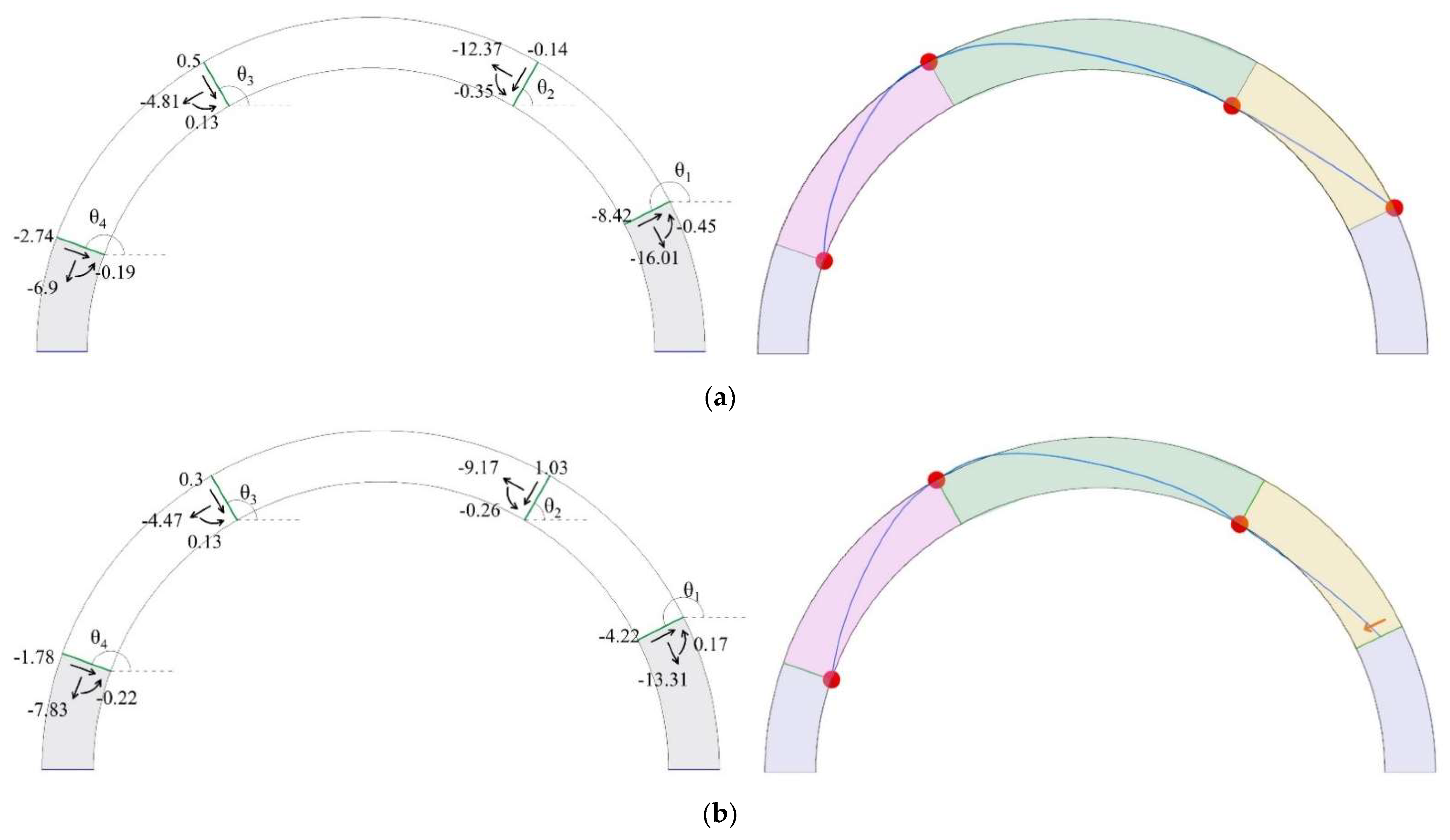
3. Implementation and Validation
4. Conclusions and Future Work
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Block, P.; Van Mele, T.; Rippmann, M.; Ranaudo, F.; Calvo Barentin, C.J.; Paulson, N. Redefining structural art: Strategies, necessities and opportunities. Struct. Eng. 2020, 98, 66–72. [Google Scholar]
- Yıldız, A.R.; Saitou, K.; Yildiz, A.R. Topology Synthesis of Multi-Component Structural Assemblies in Continuum Domains. J. Mech. Des. 2011, 133, 1235–1245. [Google Scholar] [CrossRef]
- Luo, L.; Baran, I.; Rusinkiewicz, S.; Matusik, W. Chopper: Partitioning models into 3D-printable parts. ACM Trans. Graph. (TOG) 2012, 31, 129. [Google Scholar] [CrossRef] [Green Version]
- Chen, X.; Zhang, H.; Lin, J.; Hu, R.; Lu, L.; Huang, Q.; Benes, B.; Cohen-Or, D.; Chen, B. Dapper: Decompose-and-pack for 3D printing. ACM Trans. Graph. (TOG) 2015, 34, 213. [Google Scholar] [CrossRef]
- Araújo, C.; Cabiddu, D.; Attene, M.; Livesu, M.; Vining, N.; Sheffer, A. Surface2Volume: Surface segmentation conforming assemblable volumetric partition. ACM Trans. Graph. (TOG) 2019, 38, 80. [Google Scholar] [CrossRef]
- Song, P.; Fu, C.W.; Cohen-Or, D. Recursive interlocking puzzles. ACM Trans. Graph. (TOG) 2012, 31, 128. [Google Scholar] [CrossRef] [Green Version]
- Giresini, L.; De Paola, D.; Puppio, M.L.; Buratti, G. Graphical methods, kinematic and finite element analysis of the Premilcuore masonry bridge. In ECCOMAS Proceedia, Proceedings of the 8th International Conference on Computational Methods in Structural Dynamics and Earthquake Engineering (COMPDYN 2021), Athens, Greece, 28–30 June 2021; Papadrakakis, M., Fragiadakis, M., Eds.; National Technical University of Athens (NTUA): Athens, Greece, 2021; Volume 1, pp. 682–701. [Google Scholar]
- Jiang, H.; Chen, L.; Ma, Z.J.; Feng, W. Shear behavior of dry joints with castellated keys in precast concrete segmental bridges. J. Bridge Eng. 2015, 20, 04014062. [Google Scholar] [CrossRef]
- Puppio, M.L.; Giresini, L. Estimation of tensile mechanical parameters of existing masonry through the analysis of the collapse of Volterra’s urban walls. Frat. Ed. Integr. Strutt. 2019, 13, 725–738. [Google Scholar] [CrossRef]
- Chen, S.; Bagi, K. Crosswise tensile resistance of masonry patterns due to contact friction. Proc. R. Soc. A Math. Phys. Eng. Sci. 2020, 476, 20200439. [Google Scholar] [CrossRef]
- Lourenco, P.; Oliveira, D.; Roca, P.; Orduña, A. Dry Joint Stone Masonry Walls Subjected to In-Plane Combined Loading. J. Struct. Eng. 2005, 131, 1665–1673. [Google Scholar] [CrossRef] [Green Version]
- Ceroni, F.; Cuzzilla, R.; Pecce, M. Assessment of performance of steel and GFRP bars as injected anchors in masonry walls. Constr. Build. Mater. 2016, 123, 78–98. [Google Scholar] [CrossRef]
- Giresini, L.; Puppio, M.L.; Taddei, F. Experimental pull-out tests and design indications for strength anchors installed in ma-sonry walls. Mater. Struct. 2020, 53, 103. [Google Scholar] [CrossRef]
- Solarino, F.; Giresini, L. Fragility curves and seismic demand hazard analysis of rocking walls restrained with elastoplastic ties. Earthq. Eng. Struct. Dyn. 2021, 50, 3602–3622. [Google Scholar] [CrossRef]
- Mousavian, E.; Casapulla, C. The role of different sliding resistances in limit analysis of hemispherical masonry domes. Frat. Ed. Integr. Strutt. 2019, 14, 336–355. [Google Scholar] [CrossRef]
- D’Ayala, D.; Casapulla, C. Limit state analysis of hemispherical domes with finite friction. In Proceedings of the III Interna-tional Seminar on Structural Analysis of Historical Constructions (SAHC01), Guimarães, Portugal, 7–9 November 2001; pp. 617–626. [Google Scholar]
- Boni, C.; Ferretti, D.; Lenticchia, E. Effects of Brick Pattern on the Static Behavior of Masonry Vaults. Int. J. Arch. Herit. 2021, volume, 1–21. [Google Scholar] [CrossRef]
- Cecchi, A.; Milani, G. A kinematic FE limit analysis model for thick English bond masonry walls. Int. J. Solids Struct. 2008, 45, 1302–1331. [Google Scholar] [CrossRef] [Green Version]
- Silva, L.C.; Lourenço, P.B.; Milani, G. Numerical homogenization-based seismic assessment of an English-bond masonry pro-totype: Structural level application. Earthq. Eng. Struct. Dyn. 2020, 49, 841–862. [Google Scholar] [CrossRef]
- Milani, G.; Cecchi, A. Compatible model for herringbone bond masonry: Linear elastic homogenization, failure surfaces and structural implementation. Int. J. Solids Struct. 2013, 50, 3274–3296. [Google Scholar] [CrossRef] [Green Version]
- Alforno, M.; Calderini, C.; Monaco, A.; Venuti, F. Numerical modelling of masonry vaults with different brick pattern. In Proceedings of the IASS Annual Symposium 2019 on Form and Force, Barcelona, Spain, 7–10 October 2019; Lázaro, C., Bletzinger, K.U., Oñate, E., Eds.; International Association for Shell and Spatial Structures (IASS): Madrid, Spain, 2019; pp. 1626–1633. [Google Scholar]
- Gáspár, O.; Sajtos, I.; Sipos, A.A. Friction as a geometric constraint on stereotomy in the minimum thickness analysis of circular and elliptical masonry arches. Int. J. Solids Struct. 2021, 225, 111056. [Google Scholar] [CrossRef]
- Gáspár, O.; Sipos, A.A.; Sajtos, I. Effect of stereotomy on the lower bound value of minimum thickness of semi-circular ma-sonry arches. Int. J. Archit. Herit. 2018, 12, 899–921. [Google Scholar] [CrossRef]
- Makris, N.; Alexakis, H. The effect of stereotomy on the shape of the thrust-line and the minimum thickness of semicircular masonry arches. Arch. Appl. Mech. 2013, 83, 1511–1533. [Google Scholar] [CrossRef]
- Nikolich, D. Thrust line analysis of triangular arches. Arch. Appl. Mech. 2020, 90, 1861–1874. [Google Scholar] [CrossRef]
- Forgács, T.; Sarhosis, V.; Bagi, K. Influence of construction method on the load bearing capacity of skew masonry arches. Eng. Struct. 2018, 168, 612–627. [Google Scholar] [CrossRef]
- Sassu, M.; Giresini, L.; Bonannini, E.; Puppio, M.L. On the Use of Vibro-Compressed Units with Bio-Natural Aggregate. Buildings 2016, 6, 40. [Google Scholar] [CrossRef] [Green Version]
- Giresini, L.; Stochino, F.; Sassu, M. Economic vs environmental isocost and isoperformance curves for the seismic and energy improvement of buildings considering Life Cycle Assessment. Eng. Struct. 2021, 233, 111923. [Google Scholar] [CrossRef]
- Mirkhalaf, M.; Zhou, T.; Barthelat, F. Simultaneous improvements of strength and toughness in topologically interlocked ceramics. Proc. Natl. Acad. Sci. USA 2018, 115, 9128–9133. [Google Scholar] [CrossRef] [Green Version]
- Javan, A.R.; Seifi, H.; Lin, X.; Xie, Y.M. Mechanical behaviour of composite structures made of topologically interlocking concrete bricks with soft interfaces. Mater. Des. 2019, 186, 108347. [Google Scholar] [CrossRef]
- Liu, H.; Liu, P.; Lin, K.; Zhao, S. Cyclic Behavior of Mortarless Brick Joints with Different Interlocking Shapes. Materials 2016, 9, 166. [Google Scholar] [CrossRef] [PubMed]
- Hossain, M.A.; Totoev, Y.; Masia, M.J. Friction on mortar-less joints in semi interlocking masonry. In Proceedings of the 16th International Brick and Block Masonry Conference (IBMAC 2016), Padova, Italy, 26–30 June 2016; Modena, C., da Porto, F., Valluzzi, M.R., Eds.; pp. 1635–1643. [Google Scholar]
- Ali, M.; Gultom, R.J.; Chouw, N. Capacity of innovative interlocking blocks under monotonic loading. Constr. Build. Mater. 2012, 37, 812–821. [Google Scholar] [CrossRef]
- Xu, W.; Lin, X.; Xie, Y.M. A novel non-planar interlocking element for tubular structures. Tunn. Undergr. Space Technol. 2020, 103, 103503. [Google Scholar] [CrossRef]
- Kaneko, Y.; Connor, J.J.; Triantafillou, T.; Leung, C.K.Y. Fracture Mechanics Approach for Failure of Concrete Shear Key. I: Theory. J. Eng. Mech. 1993, 119, 681–700. [Google Scholar] [CrossRef]
- Quinonez, A.; Zessin, J.; Nutzel, A.; Ochsendorf, J. Small-Scale Models for Testing Masonry Structures. In Advanced Materials Research; Gu, X., Song, X., Eds.; Trans Tech Publications Ltd.: Freienbach, Switzerland, 2010; Volume 133, pp. 497–502. [Google Scholar]
- Geboers, E. Rebuilding the Notre Dame: A Phoenix Rising from the Ashes. Available online: https://medium.com/@eric_geboers/rebuiling-notre-dame-a-phoenix-rising-from-the-ashes-f087bf89f5ed (accessed on 1 January 2022).
- Menna, C.; Mata-Falcón, J.; Bos, F.P.; Vantyghem, G.; Ferrara, L.; Asprone, D.; Salet, T.; Kaufmann, W. Opportunities and challenges for structural engineering of digitally fabricated concrete. Cem. Concr. Res. 2020, 133, 106079. [Google Scholar] [CrossRef]
- Vantyghem, G.; De Corte, W.; Shakour, E.; Amir, O. 3D printing of a post-tensioned concrete girder designed by topology op-timization. Autom. Constr. 2020, 112, 103084. [Google Scholar] [CrossRef]
- Block Research Group. Striatus-3D Concrete Printed Masonry Bridge, Venice, Italy. 2021. Available online: https://www.block.arch.ethz.ch/brg/project/striatus-3d-concrete-printed-masonry-bridge-venice-italy-2021 (accessed on 1 January 2022).
- Rippmann, M.; Curry, J.; Escobedo, D.; Block, P. Optimising stone-cutting strategies for freeform masonry vaults. In Proceedings of the IASS Annual Symposium 2013, Wroclaw, Poland, 23–27 September 2013; Obrębski, J.B., Tarczewski, R., Eds.; International Association for Shell and Spatial Structures (IASS): Madrid, Spain; pp. 1–7.
- Weizmann, M.; Amir, O.; Grobman, Y.J. The effect of block geometry on structural behavior of topological interlocking as-semblies. Autom. Constr. 2021, 128, 103717. [Google Scholar] [CrossRef]
- Bast, K.M. Feasibility study of Leonardo da Vinci’s Bridge Proposal over the Golden Horn in Istanbul. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 2019. [Google Scholar]
- Heisel, F.; Schlesier, K.; Lee, J.; Rippmann, M.; Saeidi, N.; Javadian, A.; Hebel, D.E.; Block, P. Design of a load-bearing mycelium structure through informed structural engineering. In Proceedings of the World Congress on Sustainable Technologies (WCST-2017), Cambridge, MA, USA, 11–14 December 2017; Institute for Design and Construction Technology (IEB): Karlsruhe, Germany, 2017; pp. 45–49. [Google Scholar]
- Mele, T.V.; Mehrotra, A.; Echenagucia, M.T.; Frick, U.; Ochsendorf, J.; Dejong, M.; Block, P. Form finding and structural analysis of a freeform stone vault. In Proceedings of the IASS Annual Symposia 2016, Tokyo, Japan, 26–30 September 2016; International Association for Shell and Spatial Structures (IASS): Madrid, Spain, 2016; pp. 1–10. [Google Scholar]
- Rippmann, M. Funicular Shell Design: Geometric Approaches to Form Finding and Fabrication of Discrete Funicular Structures. Ph.D. Thesis, ETH Zurich, Zürich, Switzerland, 2016. [Google Scholar]
- Mousavian, E.; Casapulla, C. SiDMACIB Project. Marie Skłodowska-Curie Individual Fellowship, Grant Agreement No. 791235, (2018–2020). Available online: https://cordis.europa.eu/project/id/791235 (accessed on 1 January 2022).
- Mousavian, E.; Casapulla, C. Structurally informed design of interlocking block assemblages using limit analysis. J. Comput. Des. Eng. 2020, 7, 448–468. [Google Scholar] [CrossRef]
- Mousavian, E.; Casapulla, C. Quantifiable feasibility check of masonry assemblages composed of interlocking blocks. Adv. Eng. Softw. 2020, 149, 102898. [Google Scholar] [CrossRef]
- Mousavian, E.; Casapulla, C. Automated Shape Adjustment of Interlocking Joints for Structurally Informed Design of Masonry Block Assemblages. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1044, 012006. [Google Scholar] [CrossRef]
- Ferris, M.C.; Tin-Loi, F. Limit analysis of frictional block assemblies as a mathematical program with complementarity con-straints. Int. J. Mech. Sci. 2001, 43, 209–224. [Google Scholar] [CrossRef] [Green Version]
- Smith, C.; Gilbert, M. Application of discontinuity layout optimization to plane plasticity problems. Proc. R. Soc. A Math. Phys. Eng. Sci. 2007, 463, 2461–2484. [Google Scholar] [CrossRef] [Green Version]
- Baraldi, D.; Reccia, E.; Cecchi, A. In plane loaded masonry walls: DEM and FEM/DEM models. A critical review. Meccanica 2017, 53, 1613–1628. [Google Scholar] [CrossRef] [Green Version]
- Lourenço, P.B. Masonry modeling. In Encyclopedia of Earthquake Engineering; Beer, M., Kougioumtzoglou, I., Patelli, E., Au, I.K., Eds.; Springer: Berlin/Heidelberg, Germany, 2014; pp. 1–13. [Google Scholar]
- Sarhosis, V.; Bagi, K.; Lemos, J.V.; Milani, G. (Eds.) Computational Modeling of Masonry Structures Using the Discrete Element Method; IGI Global: Harrisburg, PA, USA, 2016. [Google Scholar]
- Casapulla, C.; Argiento, L.U.; Maione, A.; Speranza, E. Upgraded formulations for the onset of local mechanisms in mul-ti-storey masonry buildings using limit analysis. Structures 2021, 31, 380–394. [Google Scholar] [CrossRef]
- Casapulla, C. Lower and upper bounds in closed form for out-of-plane strength of masonry structures with frictional resistances. Proceedings of Structural Analysis of Historic Constructions (SAHC08), Bath, UK, 2–4 July 2008; D’Ayala, D., Fodde, E., Eds.; Balkema: London, UK, 2008; Volume 2, pp. 1191–1198. [Google Scholar]
- Grillanda, N.; Chiozzi, A.; Milani, G.; Tralli, A. Efficient meta-heuristic mesh adaptation strategies for NURBS upper–bound limit analysis of curved three-dimensional masonry structures. Comput. Struct. 2020, 236, 106271. [Google Scholar] [CrossRef]
- Livesley, R.K. Limit analysis of structures formed from rigid blocks. Int. J. Numer. Methods Eng. 1978, 12, 1853–1871. [Google Scholar] [CrossRef]
- Livesley, R.K. A computational model for the limit analysis of three-dimensional masonry structures. Meccanica 1992, 27, 161–172. [Google Scholar] [CrossRef]
- Portioli, F.; Cascini, L.; Casapulla, C.; D’Aniello, M. Limit analysis of masonry walls by rigid block modelling with cracking units and cohesive joints using linear programming. Eng. Struct. 2013, 57, 232–247. [Google Scholar] [CrossRef]
- Dowling, N.E. Mechanical Behavior of Materials, 2nd ed.; Prentice-Hall: Englewood Cliffs, NJ, USA, 1998. [Google Scholar]
- Gilbert, M.; Smith, C.; Pritchard, T.J. Masonry arch analysis using discontinuity layout optimisation. Proc. Inst. Civ. Eng.-Eng. Comput. Mech. 2010, 163, 155–166. [Google Scholar] [CrossRef]
- Stockdale, G.L.; Sarhosis, V.; Milani, G. Seismic capacity and multi-mechanism analysis for dry-stack masonry arches subjected to hinge control. Bull. Earthq. Eng. 2020, 18, 673–724. [Google Scholar] [CrossRef]
- Iannuzzo, A.; Dell’Endice, A.; Van Mele, T.; Block, P. Numerical limit analysis-based modelling of masonry structures sub-jected to large displacements. Comput. Struct. 2021, 242, 106372. [Google Scholar] [CrossRef]
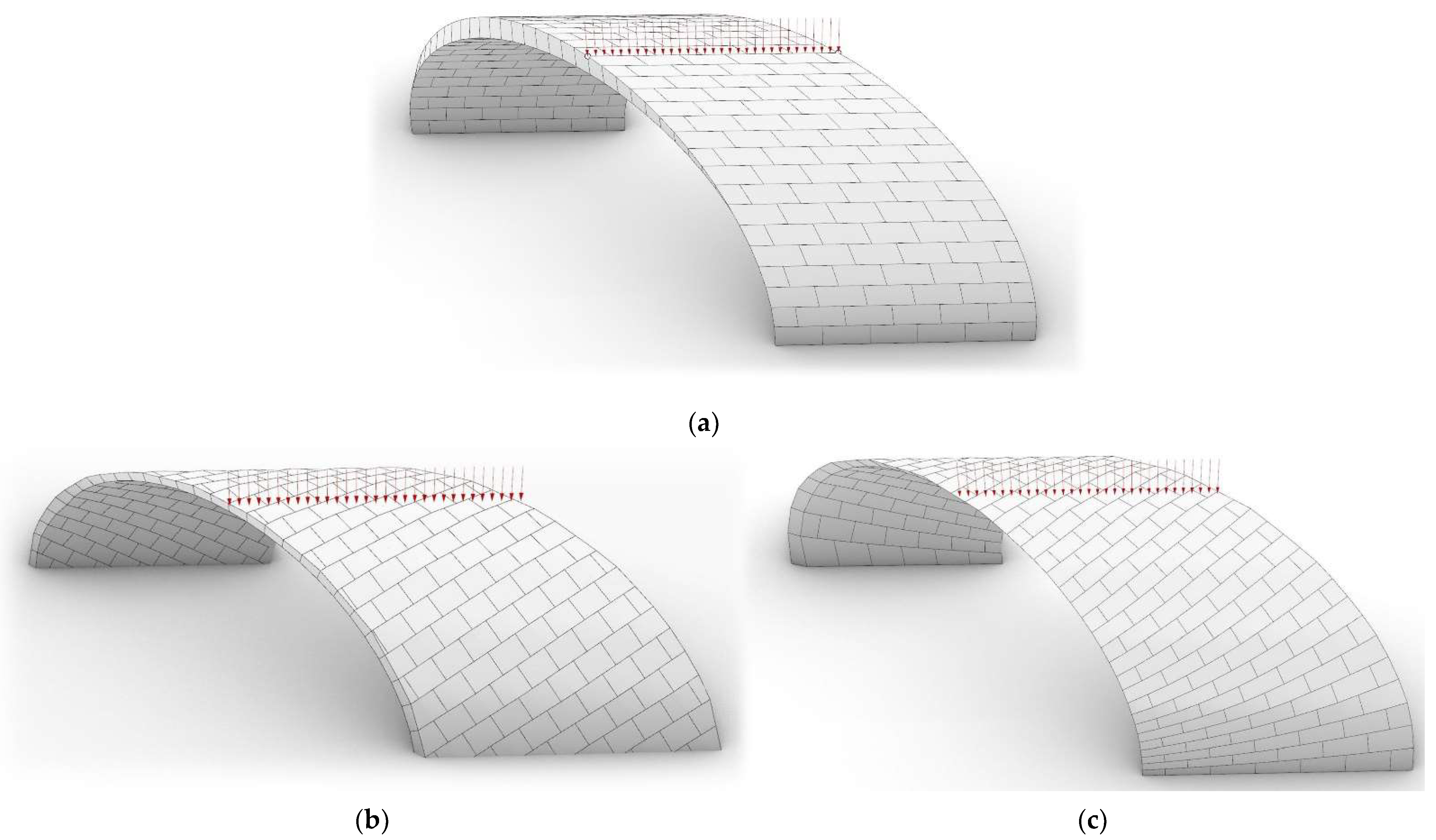
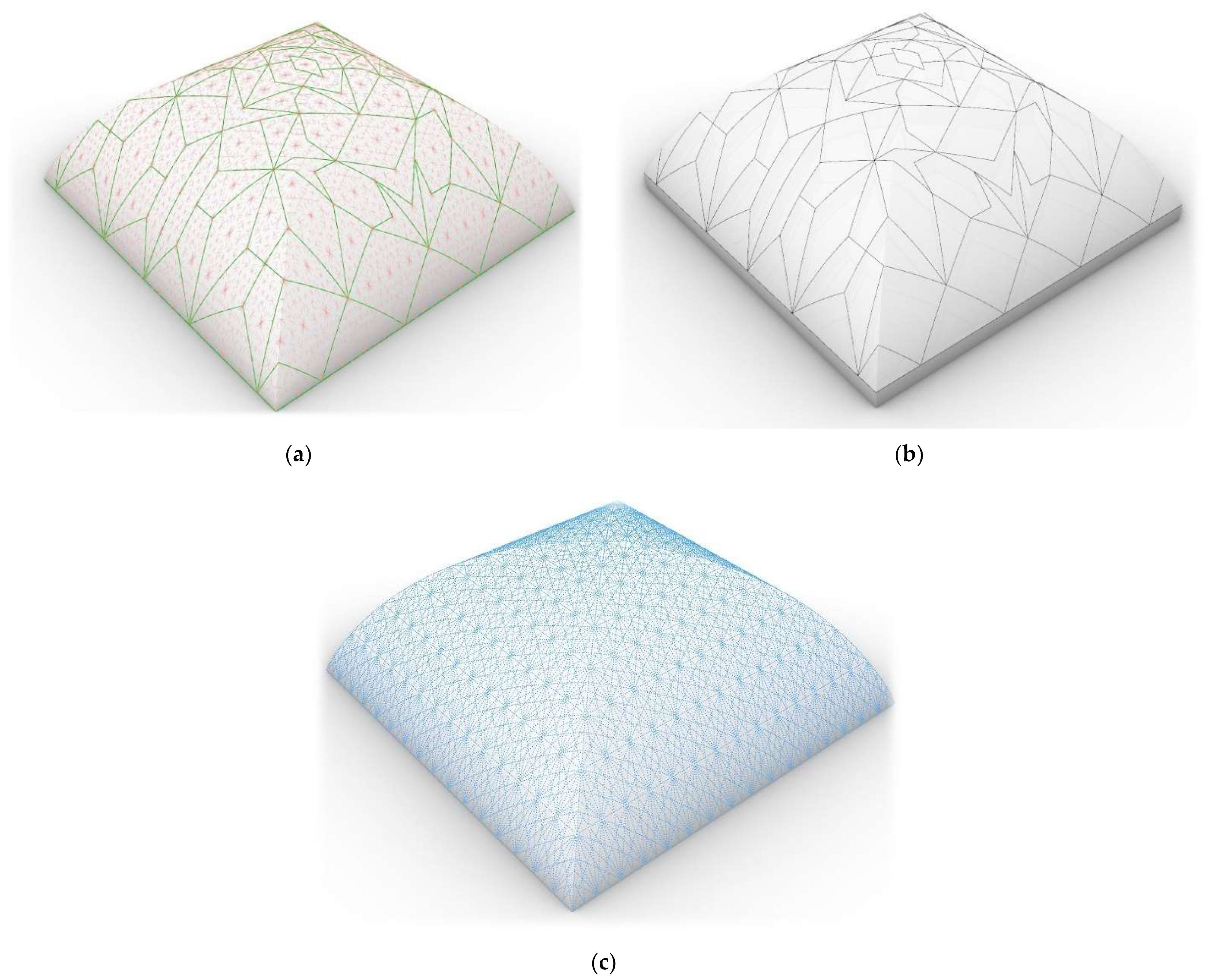




| Options | Block Combination | Interface Layout | λ | Options | Block Combination | Interface Layout | λ |
|---|---|---|---|---|---|---|---|
| Ep1 |  |  | 0.5 | Ep7 |  |  | 0.25 |
| Ep2 | 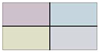 | 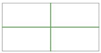 | 0 | Ep8 |  |  | 0.25 |
| Ep3 |  |  | 0 | Ep9 |  |  | 0.25 |
| Ep4 |  |  | 0.5 | Ep10 |  | 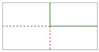 | 0.25 |
| Ep5 |  | 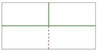 | 0 | Ep11 |  |  | 0.25 |
| Ep6 |  |  | 0 | Ep12 |  |  | 0.25 |
| φ = 35° | φ = 17.6° | |
|---|---|---|
| λ obtained by Equation (8) | 0.8279 | 0.483 |
| λ obtained by Stockdale et al. [64] | 0.8118 | 0.4878 |
| φ = 17.6° |  | λ = 0.7969 |  | λ = 0.483 | 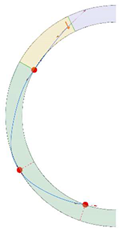 | λ = 0.483 |  | λ = 0.7969 |
| φ = 27.28° | 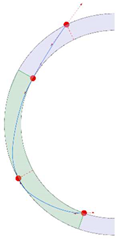 | λ = 0.8279 | 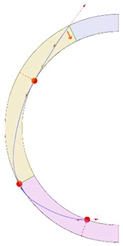 | λ = 0.8084 | 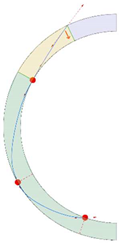 | λ = 0.8084 |  | λ = 0.8279 |
| Ec5 | Ec6 | Ec7 | Ec8 | |||||
| φ = 17.6° | 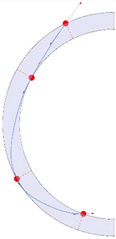 | λ = 0.8279 |  | λ = 0.483 | 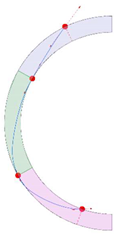 | λ = 0.8279 |  | λ = 0.483 |
| φ = 27.28° | 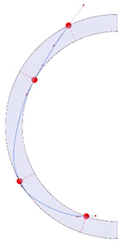 | λ = 0.8279 | 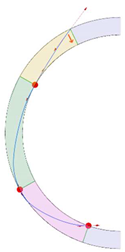 | λ = 0.8084 | 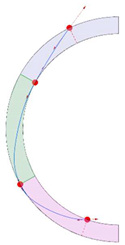 | λ = 0.8279 | 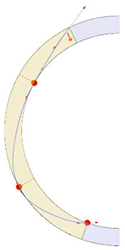 | λ = 0.8084 |
| Ec1 | Ec2 | Ec3 | Ec4 | |||||
| φ = 17.6° | 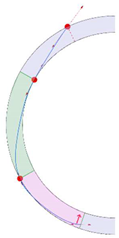 | λ = 0.7969 | 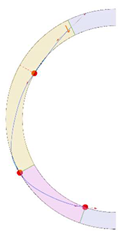 | λ = 0.483 | 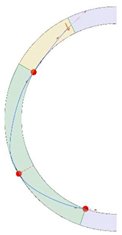 | λ = 0.483 | 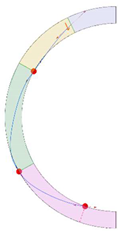 | λ = 0.483 |
| φ = 27.28° | 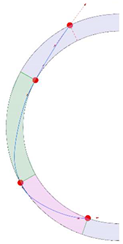 | λ = 0.8279 | 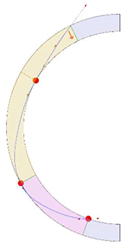 | λ = 0.8084 |  | λ = 0.8084 |  | λ = 0.8084 |
| Ec13 | Ec14 | Ec15 | Ec16 | |||||
| φ = 17.6° | 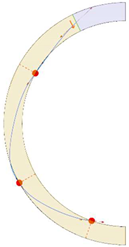 | λ = 0.483 | 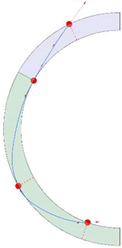 | λ = 0.8279 | 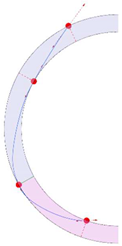 | λ = 0.8279 |  | λ = 0.7969 |
| φ = 27.28° | 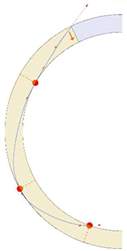 | λ = 0.8084 | 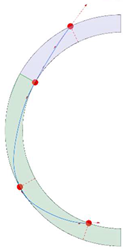 | λ = 0.8279 |  | λ = 0.8279 | 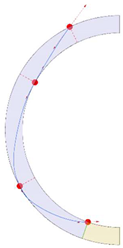 | λ = 0.8279 |
| Ec9 | Ec10 | Ec11 | Ec12 | |||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mousavian, E.; Casapulla, C. Joint Layout Design: Finding the Strongest Connections within Segmental Masonry Arched Forms. Infrastructures 2022, 7, 9. https://doi.org/10.3390/infrastructures7010009
Mousavian E, Casapulla C. Joint Layout Design: Finding the Strongest Connections within Segmental Masonry Arched Forms. Infrastructures. 2022; 7(1):9. https://doi.org/10.3390/infrastructures7010009
Chicago/Turabian StyleMousavian, Elham, and Claudia Casapulla. 2022. "Joint Layout Design: Finding the Strongest Connections within Segmental Masonry Arched Forms" Infrastructures 7, no. 1: 9. https://doi.org/10.3390/infrastructures7010009
APA StyleMousavian, E., & Casapulla, C. (2022). Joint Layout Design: Finding the Strongest Connections within Segmental Masonry Arched Forms. Infrastructures, 7(1), 9. https://doi.org/10.3390/infrastructures7010009







