Static and Impact Response of a Single-Span Stone Masonry Arch
Abstract
:1. Introduction
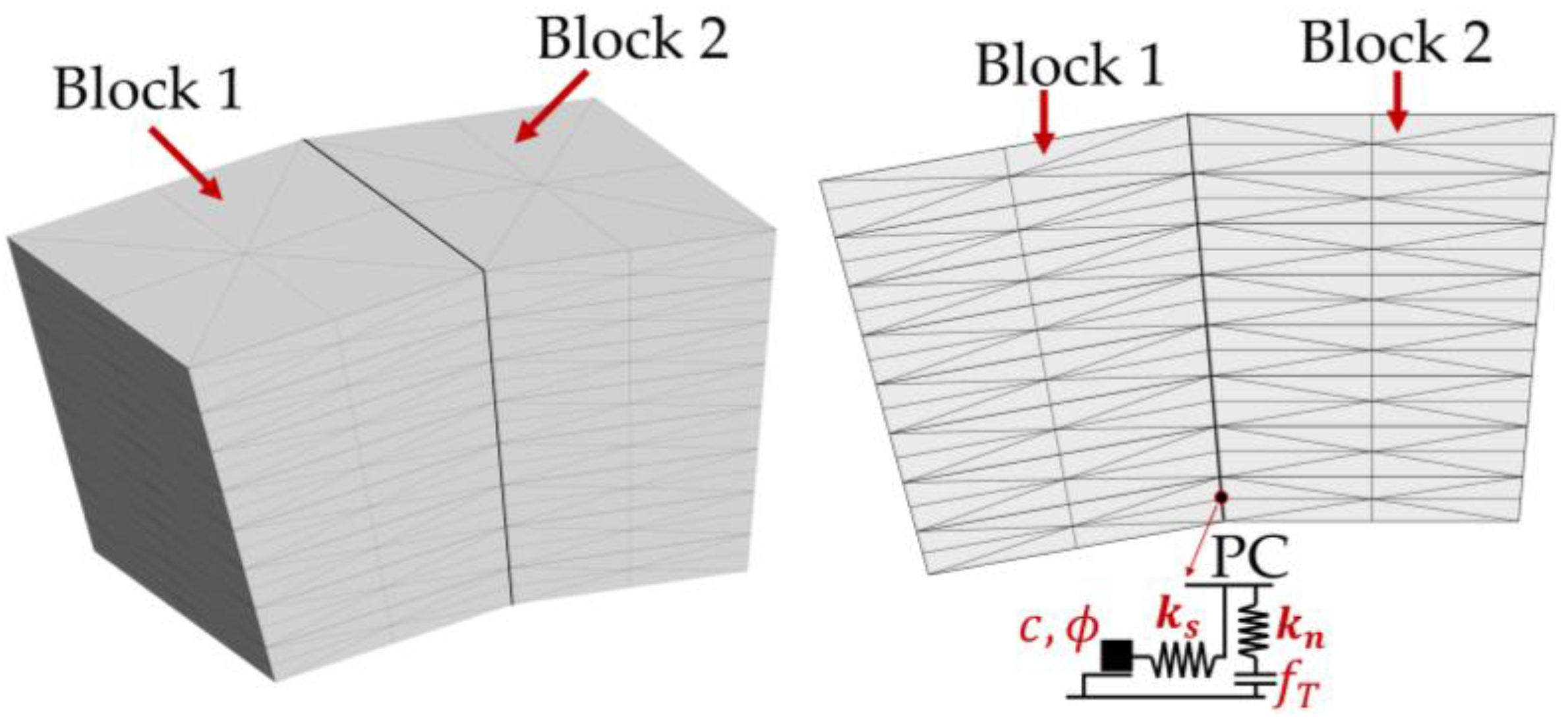
2. Background: Discrete Element Modeling
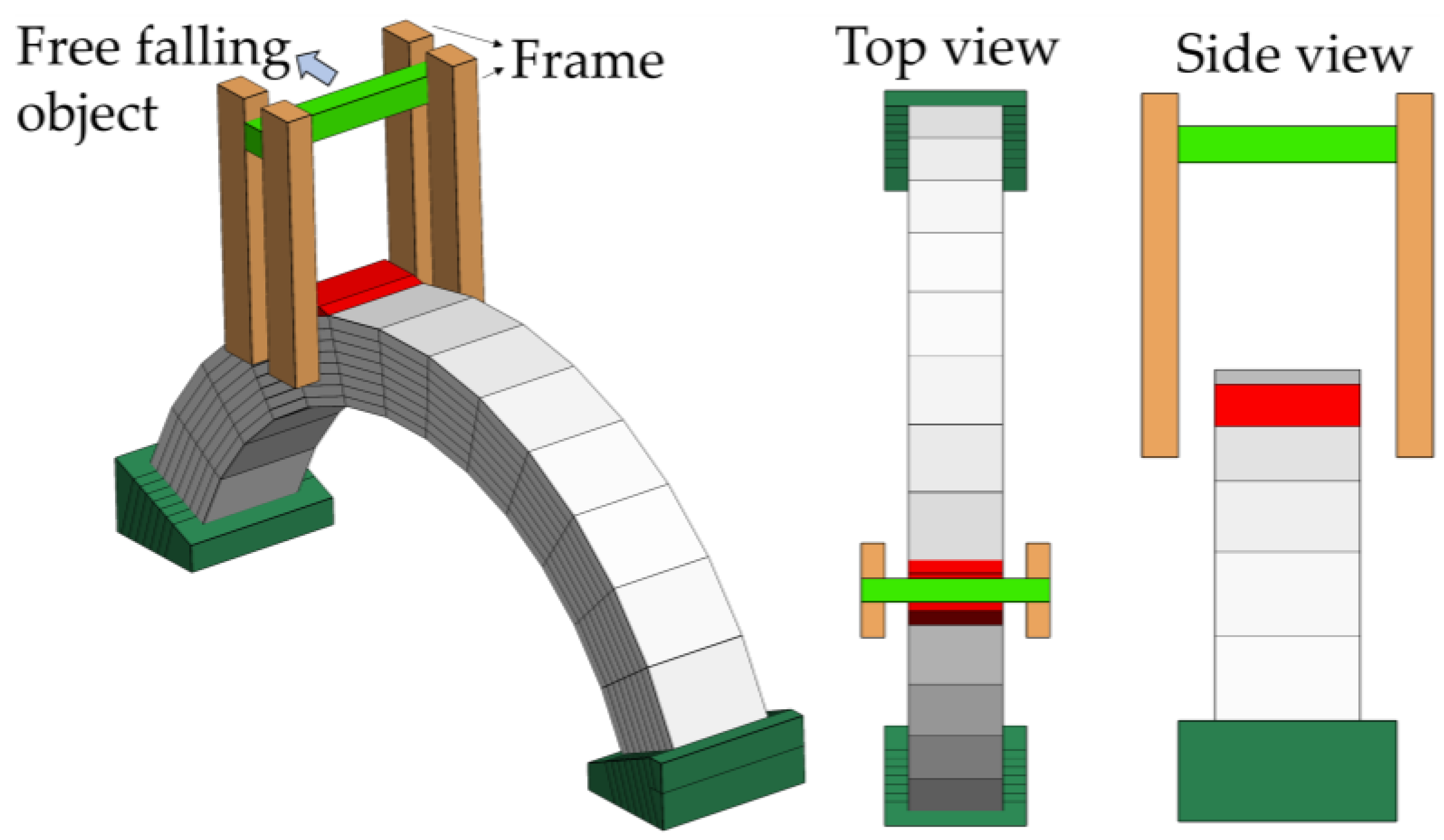
3. Benchmark Experiment: Static and Impact Loading on the Single-Span Stone Masonry Arch
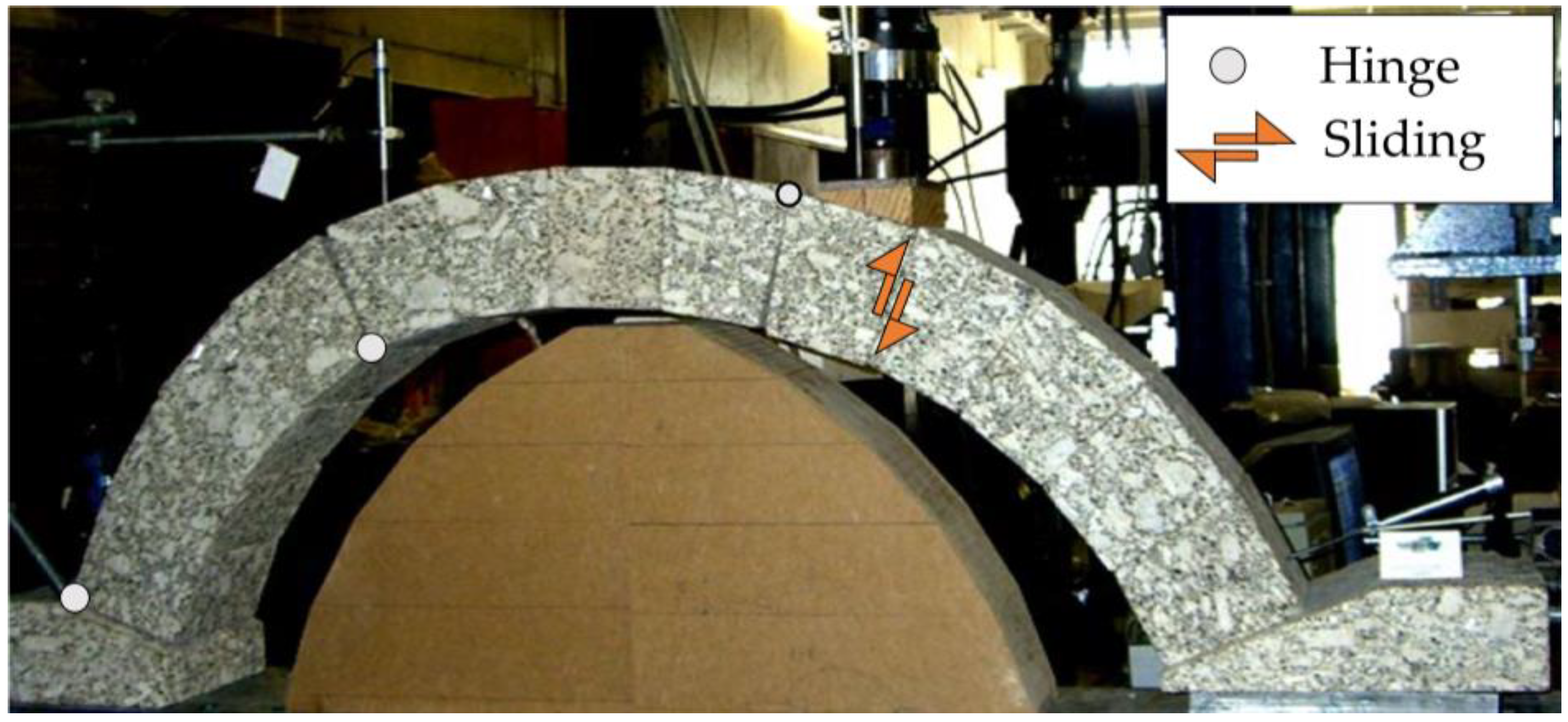
3.1. Quasistatic Loading
3.2. Impact Loading
4. DEM-Based Analysis of the Masonry Arch under Static and High-Rate Loadings
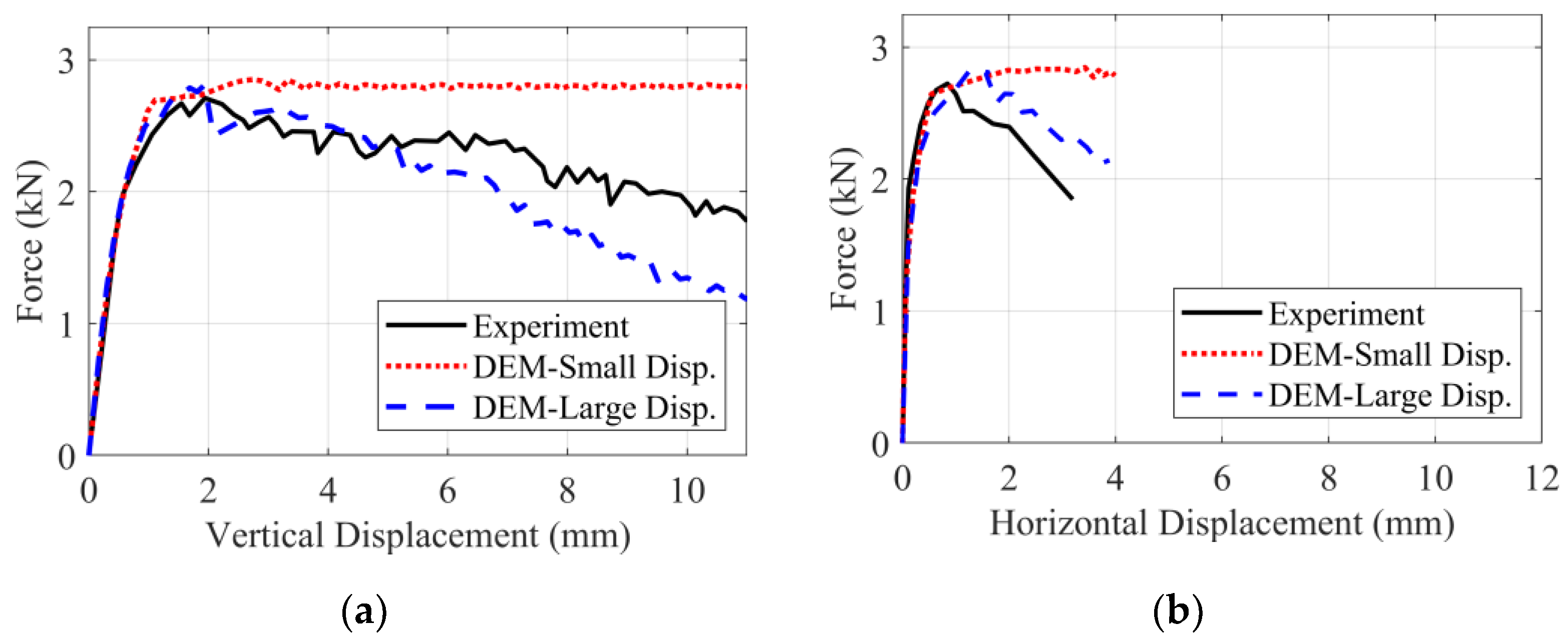
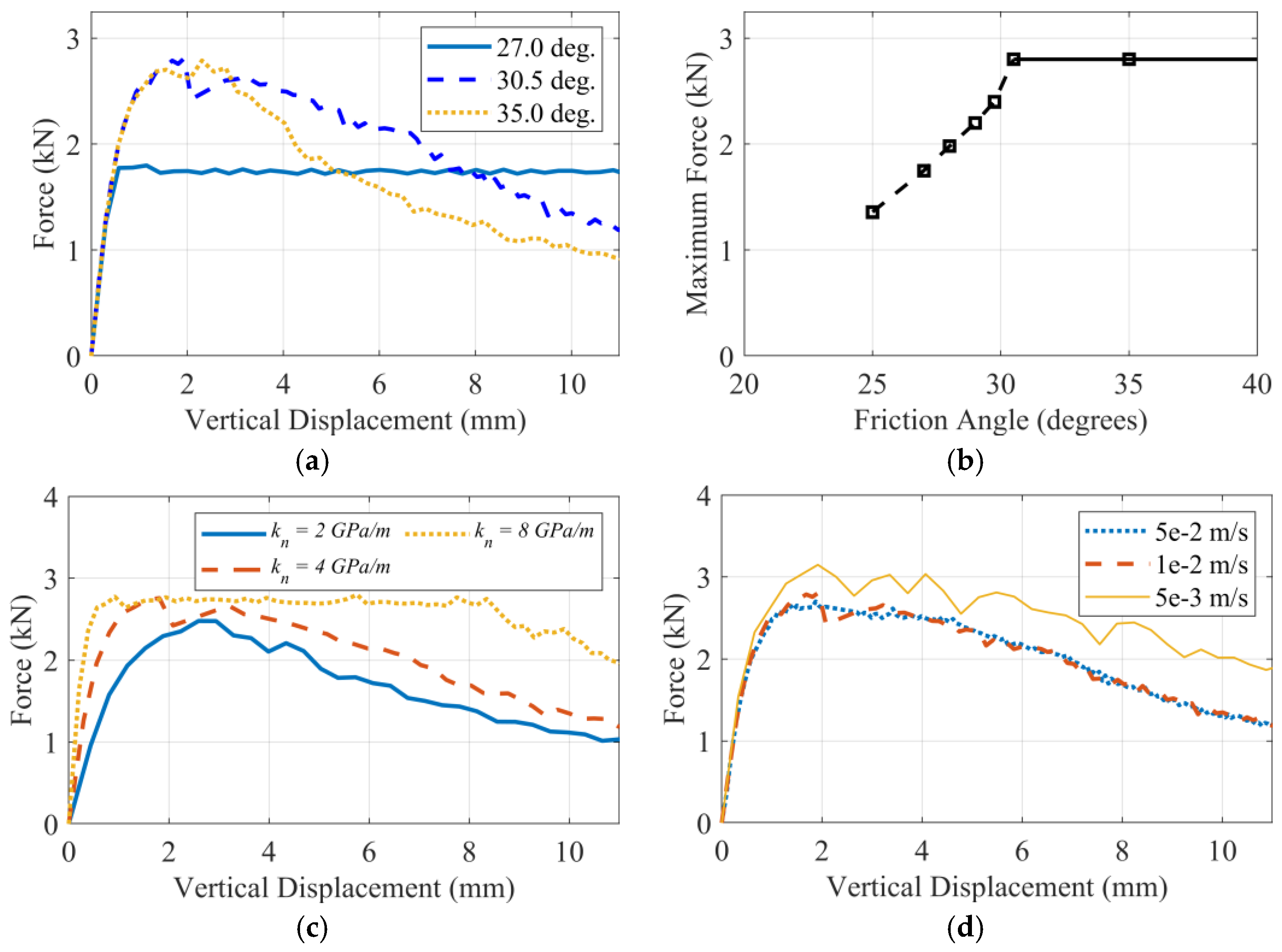
4.1. Modeling Quasistatic Loading Using DEM
4.2. Modeling Impact Loading Using DEM
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gilbert, M.; Hobbs, B.; Molyneaux, T. The performance of unreinforced masonry walls subjected to low-velocity impacts: Experiments. Int. J. Impact Eng. 2002, 27, 231–251. [Google Scholar] [CrossRef]
- Mayrhofer, C. Reinforced masonry walls under blast loading. Int. J. Mech. Sci. 2002, 44, 1067–1080. [Google Scholar] [CrossRef]
- Kernicky, T.P.; Whelan, M.; Weggel, D.C.; Rice, C.D. Structural Identification and Damage Characterization of a Masonry Infill Wall in a Full-Scale Building Subjected to Internal Blast Load. J. Struct. Eng. 2015, 141, D4014013. [Google Scholar] [CrossRef]
- Pourfalah, S.; Cotsovos, D.; Suryanto, B.; Moatamedi, M. Out-of-plane behaviour of masonry specimens strengthened with ECC under impact loading. Eng. Struct. 2018, 173, 1002–1018. [Google Scholar] [CrossRef]
- Parisi, F.; Balestrieri, C.; Asprone, D. Blast resistance of tuff stone masonry walls. Eng. Struct. 2016, 113, 233–244. [Google Scholar] [CrossRef]
- Tse, D.; Pereira, J.M.; Lourenço, P.B. Numerical Analysis of an Earthen Masonry Structure Subjected to Blast Loading. CivilEng 2021, 2, 969–985. [Google Scholar] [CrossRef]
- Rafsanjani, S.H.; Lourenço, P.; Peixinho, N. Dynamic interface model for masonry walls subjected to high strain rate out-of-plane loads. Int. J. Impact Eng. 2015, 76, 28–37. [Google Scholar] [CrossRef] [Green Version]
- Burnett, S.; Gilbert, M.; Molyneaux, T.; Beattie, G.; Hobbs, B. The performance of unreinforced masonry walls subjected to low-velocity impacts: Finite element analysis. Int. J. Impact Eng. 2007, 34, 1433–1450. [Google Scholar] [CrossRef]
- Pulatsu, B.; Erdogmus, E.; Lourenço, P.B.; Lemos, J.; Tuncay, K. Simulation of the in-plane structural behavior of unreinforced masonry walls and buildings using DEM. Structures 2020, 27, 2274–2287. [Google Scholar] [CrossRef]
- Pulatsu, B.; Bretas, E.M.; Lourenco, P. Discrete element modeling of masonry structures: Validation and application. Earthq. Struct. 2016, 11, 563–582. [Google Scholar] [CrossRef]
- Milani, G.; Lourenço, P.B.; Tralli, A. Homogenized rigid-plastic model for masonry walls subjected to impact. Int. J. Solids Struct. 2009, 46, 4133–4149. [Google Scholar] [CrossRef] [Green Version]
- Silva, L.C.; Lourenco, P.; Milani, G. Rigid block and spring homogenized model (HRBSM) for masonry subjected to impact and blast loading. Int. J. Impact Eng. 2017, 109, 14–28. [Google Scholar] [CrossRef]
- Forgács, T.; Sarhosis, V.; Ádány, S. Shakedown and dynamic behaviour of masonry arch railway bridges. Eng. Struct. 2021, 228, 111474. [Google Scholar] [CrossRef]
- Asad, M.; Dhanasekar, M.; Zahra, T.; Thambiratnam, D. Failure analysis of masonry walls subjected to low velocity impacts. Eng. Fail. Anal. 2020, 116, 104706. [Google Scholar] [CrossRef]
- Asad, M.; Zahra, T.; Thambiratnam, D.P.; Chan, T.H.; Zhuge, Y. Assessing vibration induced damage in unreinforced masonry walls subject to vehicular impact—A numerical study. Eng. Struct. 2021, 245, 112843. [Google Scholar] [CrossRef]
- Masi, F.; Stefanou, I.; Maffi-Berthier, V.; Vannucci, P. A Discrete Element Method based-approach for arched masonry structures under blast loads. Eng. Struct. 2020, 216, 110721. [Google Scholar] [CrossRef]
- Saloustros, S.; Pelà, L.; Cervera, M.; Roca, P. An Enhanced Finite Element Macro-Model for the Realistic Simulation of Localized Cracks in Masonry Structures: A Large-Scale Application. Int. J. Arch. Herit. 2017, 12, 432–447. [Google Scholar] [CrossRef]
- Lourenço, P.B. Computational Strategies for Masonry Structures; Delft University of Technology: Delft, The Netherlands, 1996. [Google Scholar]
- Gönen, S.; Soyöz, S. Seismic analysis of a masonry arch bridge using multiple methodologies. Eng. Struct. 2020, 226, 111354. [Google Scholar] [CrossRef]
- Zucchini, A.; Lourenço, P. A micro-mechanical homogenisation model for masonry: Application to shear walls. Int. J. Solids Struct. 2009, 46, 871–886. [Google Scholar] [CrossRef] [Green Version]
- Cervera, M.; Pelà, L.; Clemente, R.; Roca, P. A crack-tracking technique for localized damage in quasi-brittle materials. Eng. Fract. Mech. 2010, 77, 2431–2450. [Google Scholar] [CrossRef]
- Saloustros, S.; Cervera, M.; Pelà, L. Tracking multi-directional intersecting cracks in numerical modelling of masonry shear walls under cyclic loading. Meccanica 2017, 53, 1757–1776. [Google Scholar] [CrossRef] [Green Version]
- De Felice, G. Out-of-Plane Seismic Capacity of Masonry Depending on Wall Section Morphology. Int. J. Arch. Herit. 2011, 5, 466–482. [Google Scholar] [CrossRef]
- Gobbin, F.; de Felice, G.; Lemos, J.V. Numerical procedures for the analysis of collapse mechanisms of masonry structures using discrete element modelling. Eng. Struct. 2021, 246, 113047. [Google Scholar] [CrossRef]
- Pulatsu, B.; Gencer, F.; Erdogmus, E. Study of the effect of construction techniques on the seismic capacity of ancient dry-joint masonry towers through DEM. Eur. J. Environ. Civ. Eng. 2020, 1–18. [Google Scholar] [CrossRef]
- Erdogmus, E.; Pulatsu, B.; Gaggioli, A.; Hoff, M. Reverse Engineering a Fully Collapsed Ancient Roman Temple through Geoarchaeology and DEM. Int. J. Arch. Herit. 2020, 15, 1795–1815. [Google Scholar] [CrossRef]
- Cundall, P.A. A computer model for simulating progressive, large-scale movements in blocky rock systems. In Proceedings of the International Symposium on Rock Mechanics, Nancy, France, 4–6 October 1971; Volume 2, pp. 47–65. [Google Scholar]
- Lemos, J.V. The Basis for Masonry Analysis with UDEC and 3DEC. In Computational Modeling of Masonry Structures Using the Discrete Element Method; IGI GLOBAL: Hershey, PA, USA, 2016; pp. 61–89. ISBN 9781522502319. [Google Scholar]
- Lemos, J.V. Block modelling of rock masses. Concepts and application to dam foundations. Rev. Eur. Génie Civ. 2008, 12, 915–949. [Google Scholar] [CrossRef]
- Gonen, S.; Pulatsu, B.; Erdogmus, E.; Karaesmen, E.; Karaesmen, E. Quasi-Static Nonlinear Seismic Assessment of a Fourth Century A.D. Roman Aqueduct in Istanbul, Turkey. Heritage 2021, 4, 401–421. [Google Scholar] [CrossRef]
- Hart, R.; Cundall, P.; Lemos, J. Formulation of a three-dimensional distinct element model—Part II. Mechanical calculations for motion and interaction of a system composed of many polyhedral blocks. Int. J. Rock Mech. Min. Sci. Géoméch. Abstr. 1988, 25, 117–125. [Google Scholar] [CrossRef]
- Lemos, J.V. Discrete Element Modeling of the Seismic Behavior of Masonry Construction. Buildings 2019, 9, 43. [Google Scholar] [CrossRef] [Green Version]
- Cundall, P. Formulation of a three-dimensional distinct element model—Part I. A scheme to detect and represent contacts in a system composed of many polyhedral blocks. Int. J. Rock Mech. Min. Sci. Géoméch. Abstr. 1988, 25, 107–116. [Google Scholar] [CrossRef]
- Itasca Consulting Group Inc. 3DEC Three Dimensional Distinct Element Code; Itasca Consulting Group Inc.: Minneapolis, MN, USA, 2013. [Google Scholar]
- Birhane, T.H. Blast Analysis of Railway Masonry Bridges. Master’s Thesis, University of Minho, Braga, Portugal, 2009. [Google Scholar]
- Vasconcelos, G. Experimental Investigations on the Mechanics of Stone Masonry: Characterization of Granites and Behavior of Ancient Masonry Shear Walls. 2005. Available online: http://hdl.handle.net/1822/17383 (accessed on 15 December 2021).
- Godio, M.; Stefanou, I.; Sab, K. Effects of the dilatancy of joints and of the size of the building blocks on the mechanical behavior of masonry structures. Meccanica 2017, 53, 1629–1643. [Google Scholar] [CrossRef]
- Pulatsu, B.; Gonen, S.; Erdogmus, E.; Lourenço, P.B.; Lemos, J.V.; Prakash, R. In-plane structural performance of dry-joint stone masonry Walls: A spatial and non-spatial stochastic discontinuum analysis. Eng. Struct. 2021, 242, 112620. [Google Scholar] [CrossRef]
- Lourenco, P.; Oliveira, D.; Roca, P.; Orduña, A. Dry Joint Stone Masonry Walls Subjected to In-Plane Combined Loading. J. Struct. Eng. 2005, 131, 1665–1673. [Google Scholar] [CrossRef] [Green Version]
- Lourenco, P.; Ramos, L. Characterization of Cyclic Behavior of Dry Masonry Joints. J. Struct. Eng. 2004, 130, 779–786. [Google Scholar] [CrossRef]
- Lourenço, P.B. Analysis of historical constructions: From thrust-lines to advanced simulations. Hist. Constr. 2001, 91–116. [Google Scholar]
- Pulatsu, B.; Kim, S.; Erdogmus, E.; Lourenço, P.B. Advanced analysis of masonry retaining walls using mixed discrete–continuum approach. Proc. Inst. Civ. Eng.—Geotech. Eng. 2021, 174, 302–314. [Google Scholar] [CrossRef]








| Fresh Granite | Weathered Granite | ||
|---|---|---|---|
| Compressive Strength | 148 | 90 | |
| Elasticity Modulus | 52,250 | 35,090 | |
| Tensile Strength | 6.01 | 3.52 | |
| Elasticity Modulus | 12,550 | 3180 | |
| Fracture Energy | 0.148 | 0.200 |
| Test Setup | Drop Weight (kg) | Drop Height (cm) | Target Kinetic Energy (J) | Response after Impact | |
|---|---|---|---|---|---|
| Dry-joint Masonry | M80H45 | 81.00 | 45.0 | 357.6 | Full collapse |
| M75H45 | 74.35 | 45.5 | 331.9 | Full collapse | |
| M75H20 | 74.35 | 20.5 | 149.5 | Full collapse | |
| M60H20 | 60.93 | 20.5 | 122.5 | Hinge (3R+1S) | |
| M60H25 | 60.93 | 25.5 | 152.5 | Hinge (3R+1S) | |
| M60H30 | 60.93 | 30.5 | 182.3 | Hinge (3R+1S) |
| Test Setup | Displacement (m) | Velocity (m/s) | Acceleration (m/s2) | |
|---|---|---|---|---|
| Dry-joint Masonry | M80H45 | * | 3.19 | 5115.4 |
| M75H45 | * | 2.99 | 2138.6 | |
| M75H20 | * | 1.91 | 1416.3 | |
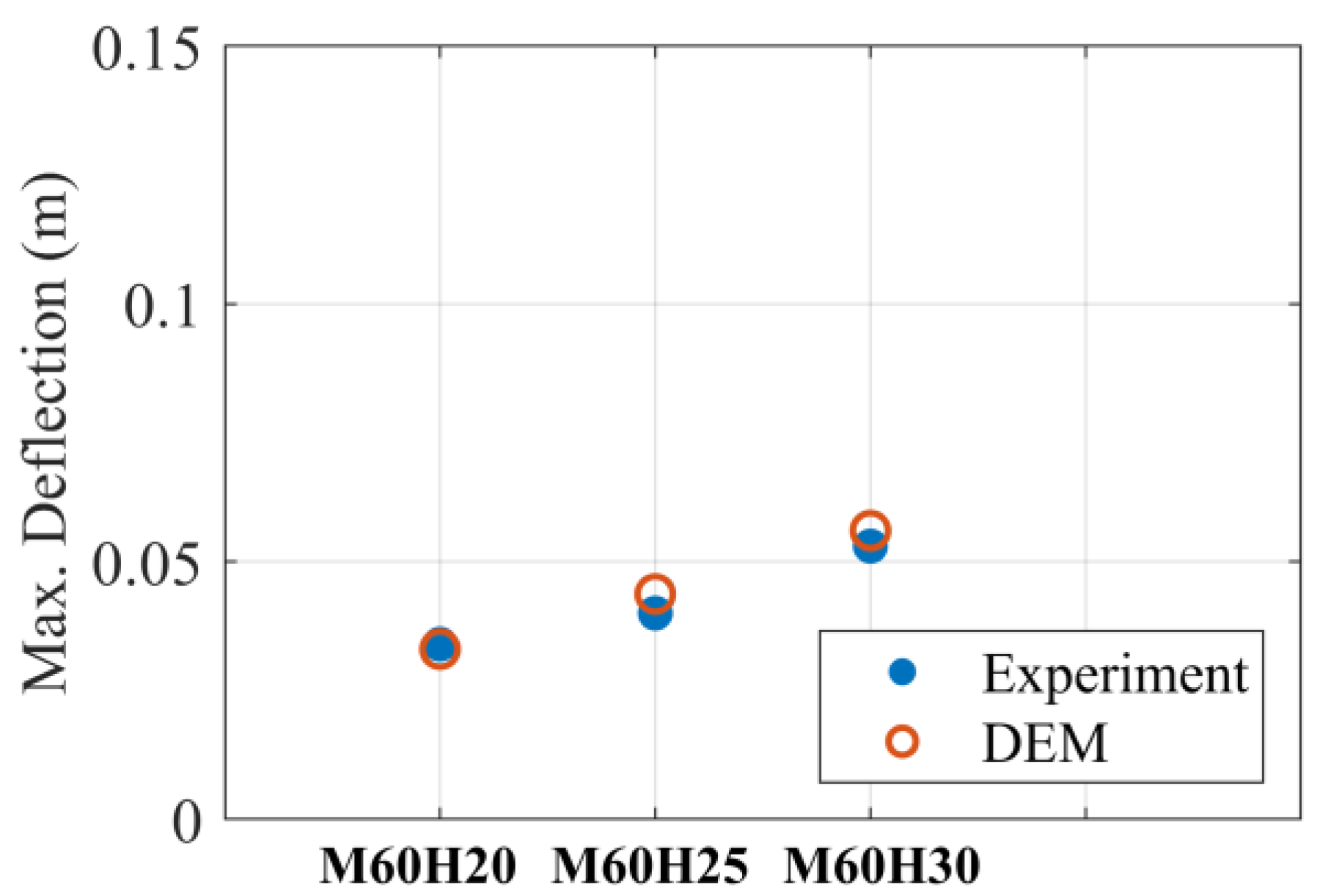
| M60H20 | 0.034 | 1.99 | 1417.1 | |
| M60H25 | 0.040 | 2.13 | 1588.6 | |
| M60H30 | 0.053 | 2.08 | 1440.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pulatsu, B.; Gonen, S.; Lourenço, P.B. Static and Impact Response of a Single-Span Stone Masonry Arch. Infrastructures 2021, 6, 178. https://doi.org/10.3390/infrastructures6120178
Pulatsu B, Gonen S, Lourenço PB. Static and Impact Response of a Single-Span Stone Masonry Arch. Infrastructures. 2021; 6(12):178. https://doi.org/10.3390/infrastructures6120178
Chicago/Turabian StylePulatsu, Bora, Semih Gonen, and Paulo B. Lourenço. 2021. "Static and Impact Response of a Single-Span Stone Masonry Arch" Infrastructures 6, no. 12: 178. https://doi.org/10.3390/infrastructures6120178
APA StylePulatsu, B., Gonen, S., & Lourenço, P. B. (2021). Static and Impact Response of a Single-Span Stone Masonry Arch. Infrastructures, 6(12), 178. https://doi.org/10.3390/infrastructures6120178








