1. Introduction
Most gravity dams have been in operation for 30 to 50 years, and were built with the knowledge and technologies of the time. Certainly, concrete gravity dams are designed to remain operational under normal conditions, to sustain minimal damage under infrequent operative conditions and to prevent total loss of the reservoir under extreme events such as seismic actions. The importance of the behaviour of the dam under seismic loading then arises, given the human fatalities and the economic losses that can occur as a result. In recent decades, the knowledge of seismic engineering has evolved considerably, making it necessary to reassess the stability of dams under seismic loading.
Methods for analysing the structural response of a dam–reservoir–foundation system rely on deterministic or probabilistic approaches. Deterministic methods are often considered too safe, or even unsafe in some cases, because they neglect the different sources of uncertainty and because of the use of extreme load cases with very low probabilities of occurrence [
1,
2,
3]. Procedures that provide a more rational way of assessing the safety of concrete gravity dams are required to establish priorities. Moreover, a probabilistic approach is required to manage the various sources of uncertainty that may impact the dam performance and decisions related thereto [
1]. Within this probabilistic framework, a fragility analysis is a promising alternative, particularly suited to study the seismic vulnerability of structures and to estimate the level of damage likely to be caused by seismic events.
The implementation of fragility curves in the domain of dams is a relatively new practice. To the best of the authors’ knowledge, the first study in the field was carried out by Ellingwood and Tekie [
4]. Four limit states were considered in the seismic fragility analyses, and non-linear temporal dynamic analysis were performed including the dam–reservoir–foundation (DRF) interaction effects. Samples of the model were obtained using the method of Latin hypercube sampling (LHS) and were coupled with twelve historical accelerograms. These samples were analysed for six levels of seismic intensity, by calibrating the acceleration time compared to the spectral acceleration measured at the pitch period, to obtain the fragility curves. This study reveals that in all cases the log-normal model of fragility fit the results of the simulation very well. In the same manner, Mirzahosseinkashani and Ghaemian [
5] and Ghanaat et al. [
6,
7] applied the same methodology proposed by Ellingwood and Tekie [
4] and performed non-linear analysis to assess the vulnerability of a case study dam considering finite element models with different degrees of complexity. Similarly, the work performed by Lupoi and Callari [
8] presents a probabilistic seismic assessment method able to manage the physical complexity of the DRF system and the uncertainties regarding structural data and external actions. The fragility curves were obtained via a standard Monte Carlo simulation procedure, and no probability was assumed to represent the fragility curves. Further studies on the subject were performed by Zhong and Lin [
9], where considering the ground motion and material uncertainties, 180 non-linear seismic analyses were performed, based on which typical seismic damage modes were obtained. This research used samples obtained by thirty Monte Carlo simulations and six levels of seismic intensity.
The most recent studies were from Bernier et al. [
10,
11], Hariri-Ardebili and Saouma [
12], Lallemant et al. [
13] and Segura et al. [
14]. In the first two studies [
10,
11], the finite element method is used to model a single block of a case study dam considering DRF interaction. Uncertainties in the ground motions and modelling parameters are included, and an LHS technique is used to propagate these sources of uncertainty. The fragility curves are developed using non-linear time-history analysis to evaluate two limit states: sliding at the dam base and at lift-joints. In the fist paper of Bernier et al. [
10], the uncertainty related to the spatial variation of angle of friction is included in the fragility analysis through the incorporation of random fields modelling. In their second paper [
11] the same methodology is used together with the conditional spectrum method to select ground motion times series. Hariri-Ardebili and Saouma [
12] explored the development of seismic fragility curves for gravity dams with and without vertical ground motion. The derived fragility curves using scaled records were compared with those from probabilistic seismic demand analysis, showing acceptable consistency between the two methods. Similarly, the study performed by Lallemant et al. [
13] provides a synthesis of the most commonly used methods for fitting fragility curves and highlights some of their significant limitations. Finally, in the study by Segura et al. [
14], a similar model of the case study dam in Bernier et al. [
10,
11] is used to develop up-to-date fragility curves for the sliding limit states using a record selection method based on the generalised conditional intensity measure approach. These fragility functions are then combined with the recently developed regional hazard data to evaluate the annual risk, which is measured in terms of the unconditional probability of limit state exceedance. Further and more exhaustive information can be found in the state-of-the-art review of seismic fragility applied to concrete dams by Hariri-Ardebili and Saouma [
15].
These studies show that the most reliable methods should be implemented where dam safety is concerned. Undoubtedly, one of the best methods for analysing gravity dams is non-linear dynamic time history analysis [
16]. However, sampling techniques must be used in order to minimise the cost of the non-linear finite element analyses required to develop the fragility functions. While these previous studies have mainly focused on the methodology to develop fragility curves, they provide limited guidelines regarding the modelling and characterisation of concrete gravity dams for such analysis. Moreover, the simplifications regarding the modelling assumptions can lead to the improper evaluation of the seismic response of the dam. Among these simplifications, of note are the use of a very general or wide range of parameters due to the lack of rigorous material properties characterisation, the consideration of linear or unverified numerical models, the lack of a soil–fluid–structure interaction, the assumption of a massless foundation and the inadequate use of border conditions to describe the physical behaviour of the structure. In light of the abovementioned limitations, the main objective of this paper is to present a case study dam to illustrate a systematic methodology for modelling the DRF system and characterisation of the uncertainties associated with this type of structure to perform a seismic fragility analysis. Accordingly, the major contributions of this paper, in order of presentation, can be listed as follows: (i) the development, calibration and validation of a nonlinear finite element model (FEM) of a dam-type structure; (ii) the presentation of a procedure for applying static and dynamic loading in the DRF system, including the detailed deconvolution of the ground motion records; (iii) the design of experiments to generate samples of the system to perform a probabilistic analysis; (iv) the provision of insight into the parameters relevant for the uncertainty modelling and determination of their distributions; (v) illustration of the procedure for selection and scaling of the seismic input and (vi) the presentation of a fragility framework to generate fragility curves and assess the expected seismic performance under extreme events. The proposed methodology was applied to a case study structure in Eastern Canada.
4. Uncertainty Modelling
Each parameter of the analysis was defined either as a fixed value or as a random variable associated with a probability density function (PDF). The preliminary analyses carried out in the finite element model showed that some parameters (e.g., moduli of elasticity) had to be defined as random variables in order to account for the uncertainty about the estimated value. The lack of knowledge relative to the parameters quantifying the resistance of the structure, loading conditions and seismic input were considered in this study. All the parameters considered as random variables and their associated PDFs are listed in
Table 5, while all the material properties considered constant throughout the analysis are summarised in
Table 6. The 11 uncertain parameters listed in
Table 5 were sampled using Latin hypercube sampling (LHS) to generate
model samples to propagate sources of uncertainty in the fragility analysis. When using an LHS experimental design, the minimum number of design runs required for optimally spaced design points is typically at least twice the number of parameters considered [
29,
30]. This sampling method was chosen because of its ability to efficiently generate representative samples by dividing the range of possible values for each variable into
N equiprobable intervals.
4.1. Material Properties
To test the accuracy of the finite element model, it was necessary to specify the densities, moduli of elasticity, damping and Poisson coefficients of the concrete and foundation material (
Table 2). However, these values were re-evaluated here for estimation of the impact of their uncertainty on the dynamic behaviour of the structure.
4.1.1. Modulus of Elasticity
As already pointed out, the seismic response of the system changes according to the value of the concrete and foundation modulus of elasticity. It is therefore appropriate to define these parameters as a random variables, especially given the lack of empirical or experimental data to calibrate them accurately. From a series of tests carried out at the dam site, it was determined that the concrete static modulus of elasticity could be associated with extreme values between 14.8 and 21.5 GPa. As recommended by [
26,
31], the concrete modulus of elasticity is amplified by 25% in the presence of dynamic loadings. Therefore, the extreme values of the modulus of elasticity were 18.5–26.9 GPa. Regarding the foundation, because no test was carried out to characterise the modulus of elasticity, the values adopted in models M0 and M1 (40–50 GPa) were considered herein as the extreme values of the uniform distribution associated with this variable.
4.1.2. Damping
The concrete damping is difficult to estimate, especially since the possible cracking of the structure under the effect of the seismic load will cause additional energy dissipation. Given that the concrete is associated with an elastic linear behaviour in the numerical model, the increase in damping with cracking is not automatically taken into account. The average damping should therefore be increased with respect to the 1.5% value obtained from an experimental test for relatively small vibrations [
24] and used during model verification to anticipate cracking of the structure and the nonlinear mechanisms that can develop during an earthquake. As proposed by Ghanaat et al. [
6], for the concrete damping, a log-normal distribution with a median of 5% and a standard deviation of 0.35 was adopted. Taking these values into account, the system damping was estimated with the free-decay method, and it was verified that the total system damping remained under 7% [
32]. With respect to the foundation damping, given that the knowledge on this parameter is limited, the viscous damping of the foundation was assumed to be 5% for all samples [
19].
4.1.3. Shear and Tensile Strength
In the absence of sufficient data, a uniform PDF was also used to describe the parameters characterising the shear and tensile strength. For determining the shear and tensile strength parameters at the rock–concrete contact and based on the construction plan of the dam, the foundation rock at the dam site was determined to be composed of diorite, gneiss and granite. The maximum and minimum values for the cohesion and the angle of friction were estimated from literature test results for the same type of rock foundation [
33]. As a result, a range of values of 0.1–1.5 MPa and 30–45° were assigned to the uniform PDF for the cohesion and the angle of friction, respectively. Similarly, for the tensile strength, the adopted extreme values varied between 0.05 and 0.8 MPa.
4.2. Load Parameters
4.2.1. Drain Efficiency
The efficiency of the drainage system is defined by the proportion of the flow that can be captured by this system to reduce the hydraulic load. To account for the uncertainties in the uplift pressures, the drain efficiency was defined as a random variable with a uniform PDF. From previous studies of the dam owners at the base of block-6 and using the extreme values of a water column height to calculate the uplift load, it was concluded that the drains allow for a decrease in the uplift pressures between 15% and 75%, while the USBR [
19] recommends limiting the effectiveness of the drains to 67%. Consequently, the efficiency of the drain system was sampled by considering values between 0% and 70% to also include the undrained case.
4.2.2. Directionality Factor
The directionality factor
is defined as the ratio of the vertical to the horizontal peak ground acceleration (PGA), as expressed by Equation (
7):
where
and
represent the peak accelerations in the vertical and horizontal directions, respectively. Traditionally, this factor is assumed to be equal to 2/3, but its value can vary greatly, having a significant influence on the response of the structure. In fact, from the available recorded ground motions, it can be seen that this ratio generally varies between 0.3 and 1.0 depending on the magnitude, the epicentral distance, the tectonic environment and the spectral period [
34,
35,
36]. Due to the lack of seismic data at the dam site, the range of values was slightly reduced to 0.5–0.8.
4.3. Ground Motions
To perform the fragility analysis for assessing the seismic vulnerability of the case-study structure, a representative set of ground motion time series (GMTS) that efficiently depicts the aleatory uncertainty was needed. Each of the 22 samples generated with the LHS procedure was randomly coupled with a seismic event. Thus, 22 ground motion time series in the vertical and horizontal direction were selected.
4.3.1. Levels of Seismic Intensity
The response of each sample was analysed for several levels of seismic intensity through an incremental dynamic analysis (IDA). Most of the studies found in the literature concerning seismic fragility assessment for gravity-dam-type structures use the peak ground acceleration (PGA) or the spectral acceleration at fundamental period of the structure as the seismic intensity measure [
10,
14,
15]. Nevertheless, given that the fundamental period depends on the concrete and foundation modulus of elasticity, defined herein as random variables, the spectral acceleration at the fundamental period varies according to the sample considered for the analysis. Therefore, in this study, the PGA in the horizontal direction was chosen to characterise the seismic solicitation at seven target intensity levels:
to efficiently cover return periods corresponding to 200–20,000 years. Thus, the 22 selected horizontal accelerograms were scaled according to these seven intensity levels, while the vertical accelerograms were scaled taking into account the directionality factor associated with the sample in question.
Due to the limited availability of strong ground motion records for the considered region, synthetic accelerograms developed by Atkinson [
37] for Eastern Canada were used. The accelerograms were simulated for the following cases: magnitude 6 and epicentral distances between 10 and 15 km and between 20 and 30 km, and magnitude 7 and epicentral distances of 15–25 km and 50–70 km. For each of these four sets, three random components of the earthquake were simulated at 15 random locations around the epicentre. Seismic solicitations were then selected considering accelerograms from the four sets.
4.3.2. Selection and Scaling of Accelerograms
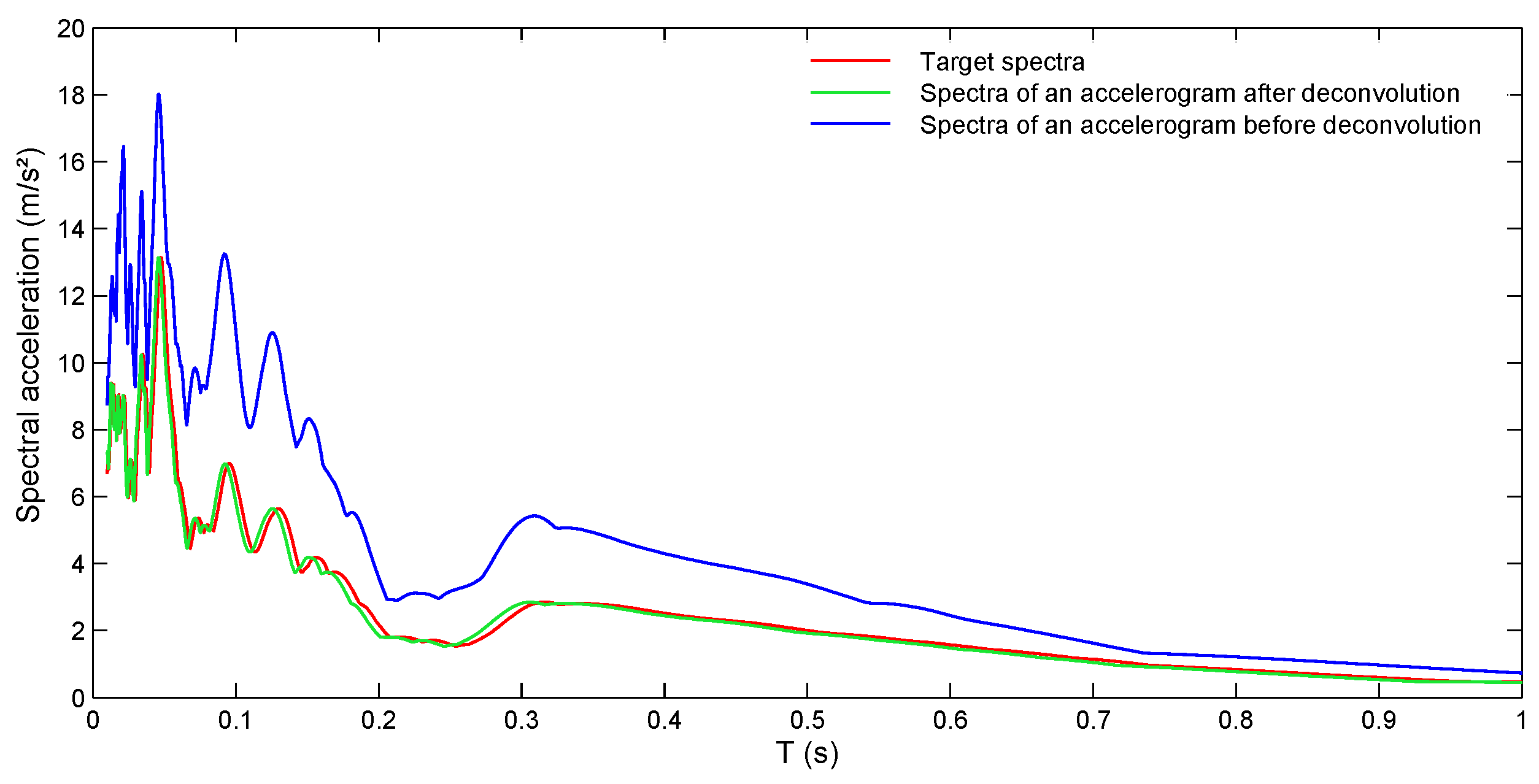
Current seismic guidelines for dimensioning recommend the use of seismic events compatible with the uniform hazard spectrum (UHS) for the studied site. The UHS provided by the Geological Survey of Canada (GSC) at the dam site for soil type A (
m/s), referred to as the target spectrum, was used for this study, and it is shown in
Figure 6a. Taking into consideration the different simulated sets of accelerograms in terms of magnitude and epicentral distance, GMTS were selected for soil type A, keeping those whose response spectrum closely matched the target spectrum. For that purpose, the method proposed by Atkinson [
37] was used to compare the shape of the response spectra of the target and the selected ground motion over the range of periods of interest,
. Finally, the 22 accelerograms presenting the smallest values of the standard deviation within the considered range of periods were selected. Each simulated record, with initial
, was then multiplied by the factor
to match the peak ground acceleration at each intensity level.
Figure 6b shows the selected horizontal accelerograms for the seismic intensity level
, corresponding to the UHS PGA. It is possible to note in this figure that the spectra are relatively well dispersed, which will allows for the representation of the random nature of the seismic solicitation.
Each horizontal accelerogram is associated with a vertical accelerogram from the two remaining simulated components, and the one with the closest response spectrum to the UHS was kept. Following the same procedure as the horizontal component, in addition to the directionality factor associated with the sample, the vertical acceleration values were multiplied by the factor .
6. Expected Seismic Performance
For structures of special importance, such as dams and nuclear power plants, it is typical to consider the high confidence low probability of failure (HCLPF) criteria. This notion represents the level for which the probability of exceedance of a limit state is sufficiently low to consider that it will not be reached throughout the life of the structure [
39] and usually corresponds to a probability of less than 5%. Accordingly, to evaluate the expected seismic performance of the case-study structure for the HCLPF level, the spectral acceleration for each limit state was extracted from the fragility curves for a probability of exceedance of 5%. Moreover, given that the PGA at the dam site for seismic events with a return period of 2475 could be computed, the expected seismic performance of the structure for events with these characteristics, usually used in the safety guidelines for dimensioning and verification, could also be estimated. Considering a
from the UHS, the probability of exceedance was extracted from the fragility curves for each limit state.
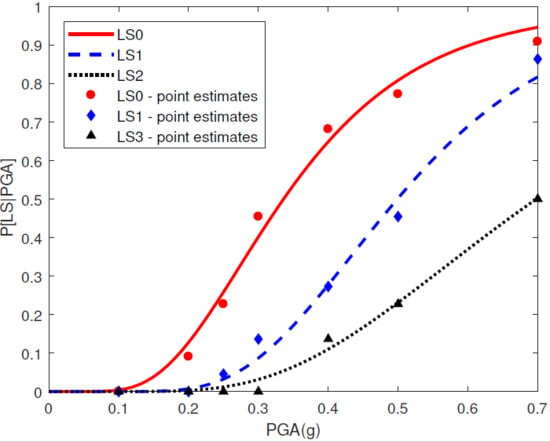
The results presented in
Table 10 show that the sliding at the concrete–rock interface of block-6 could be initiated for a PGA greater than 0.16
g. Additionally, the sliding remained low (<25 mm) for PGA values below 0.27
g, while it is unlikely to reach such a level of damage during an earthquake with a return period of 2475 years. Finally, the probability of reaching a severe damage limit state that could lead to the loss of control of the reservoir becomes significant when the PGA reaches values close to 0.33
g.
7. Conclusions
The main objective of this study was to assess the seismic vulnerability of a dam-type structure through a fragility framework and to present all the required analyses and verifications of the numerical model prior to obtaining the fragility curves. The methodology was applied to the bottom outlet spillway structure of the case study dam and more particularly to block-6 of the latter. For that purpose, a finite element model of the block–reservoir–foundation system was developed to describe the real behaviour of the structure as closely as possible. Following the results of the preliminary analyses, the study of the fragility of the system was restricted to a single damage state related to the sliding at the base of the block. Fragility curves were generated, taking into account the results of nonlinear dynamic analyses that were calculated with the structure samples obtained with LHS and coupled to GMTS, describing the seismic scenario at the dam site.
The methodology proposed herein makes it possible to account for the uncertainties associated with the modelling parameters as well as the randomness in the seismic solicitation. The results obtained from the fragility curves indicate that the probability of observing an incipient sliding at the base of the block, when solicited with PGA values used by the current guidelines for dimensioning and verification, is 20%. In contrast, it is unlikely that more severe damage to the structure for this intensity level will be observed.