A Predesign Methodology for Power Electronics Based on Optimization and Continuous Models: Application to an Interleaved Buck Converter
Abstract
:1. Introduction
2. Proposed Approach for Preliminary Design
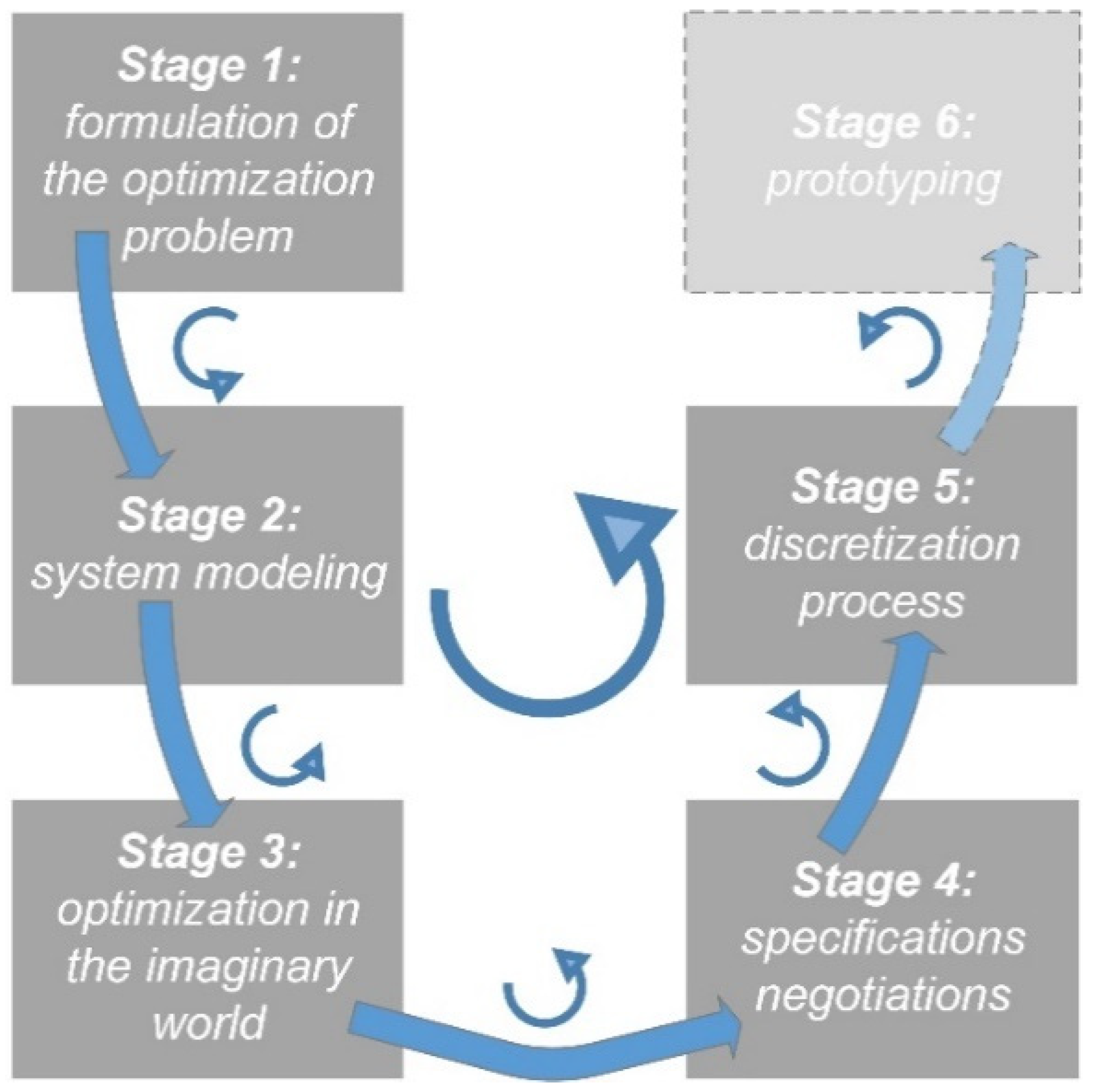
2.1. Presentation of the Design Method
- The discretized variables (D): variables that are physically continuous but industrially discretized, such as a set of capacitors references.
- The natural number variables (N): variables of the system that are constrained to natural numbers for practical reasons (number of levels of a multi-level converter, turn number of an inductor, etc.)
- The technology or topology choice variables (T): variables that only have distinct values (such as topology choice between buck or boost, or technology choice: SiC vs. Si, magnetic material).
- Statement: if there are no solutions in the imaginary (continuous) world, then there are no solutions in the real (discrete) world.
- Assumption: the performances of the imaginary solutions are very similar to the performances of the locally discretized solutions.
2.2. The Different Stages of the Proposed Method
3. Study Case
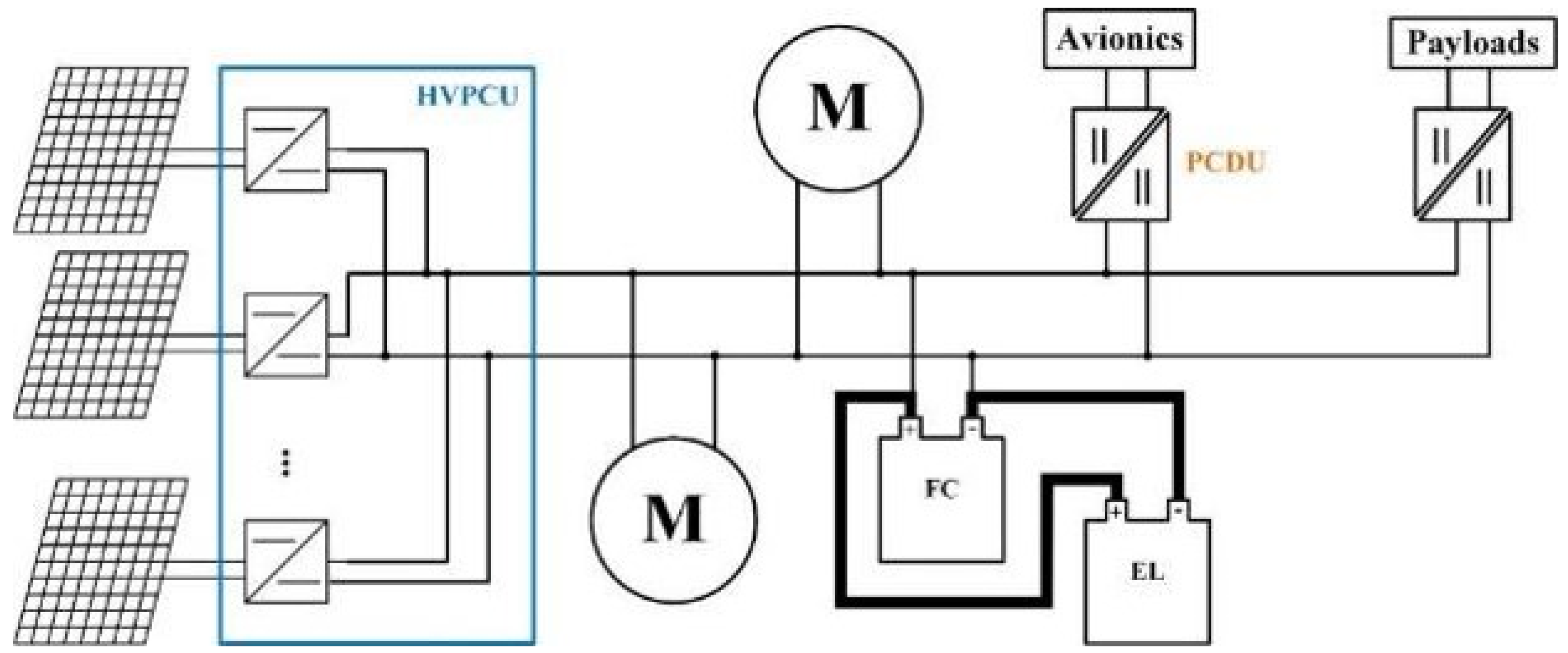
3.1. Context
3.2. DC-DC Converter Study
| Criteria | Minimum Value | Maximum Value |
|---|---|---|
| Power density | 7 kW/kg is a must | 10 kW/kg nice to have |
| Input power | 0 W | 4.5 kW/5 kW for peak value |
| Input voltage | 450 V | 800 V |
| Output voltage | 200 V | 430 V |
| Efficiency | 96% | NA |
| Input/Output current THD | NA | 5% |
| Cooling temperature | −40 °C | 65 °C |
| Ambient temperature | TBD | 85 °C |
| Ambient pressure | 0.07 bar | 1 bar |
4. Formulation of the Optimization Problem for Pre-Sizing in the Imaginary World
- Defining the problem objectives, constraints and design parameters (in other words optimization input, output and objective variables);
- Selecting an optimization method and algorithm;
- Setting the model of the system to be optimized (define the right organization of the sub-models).
4.1. Definition of the Study Case Problem Objectives, Constraints and Design Parameters
4.2. Selection of the Method and the Algorithm
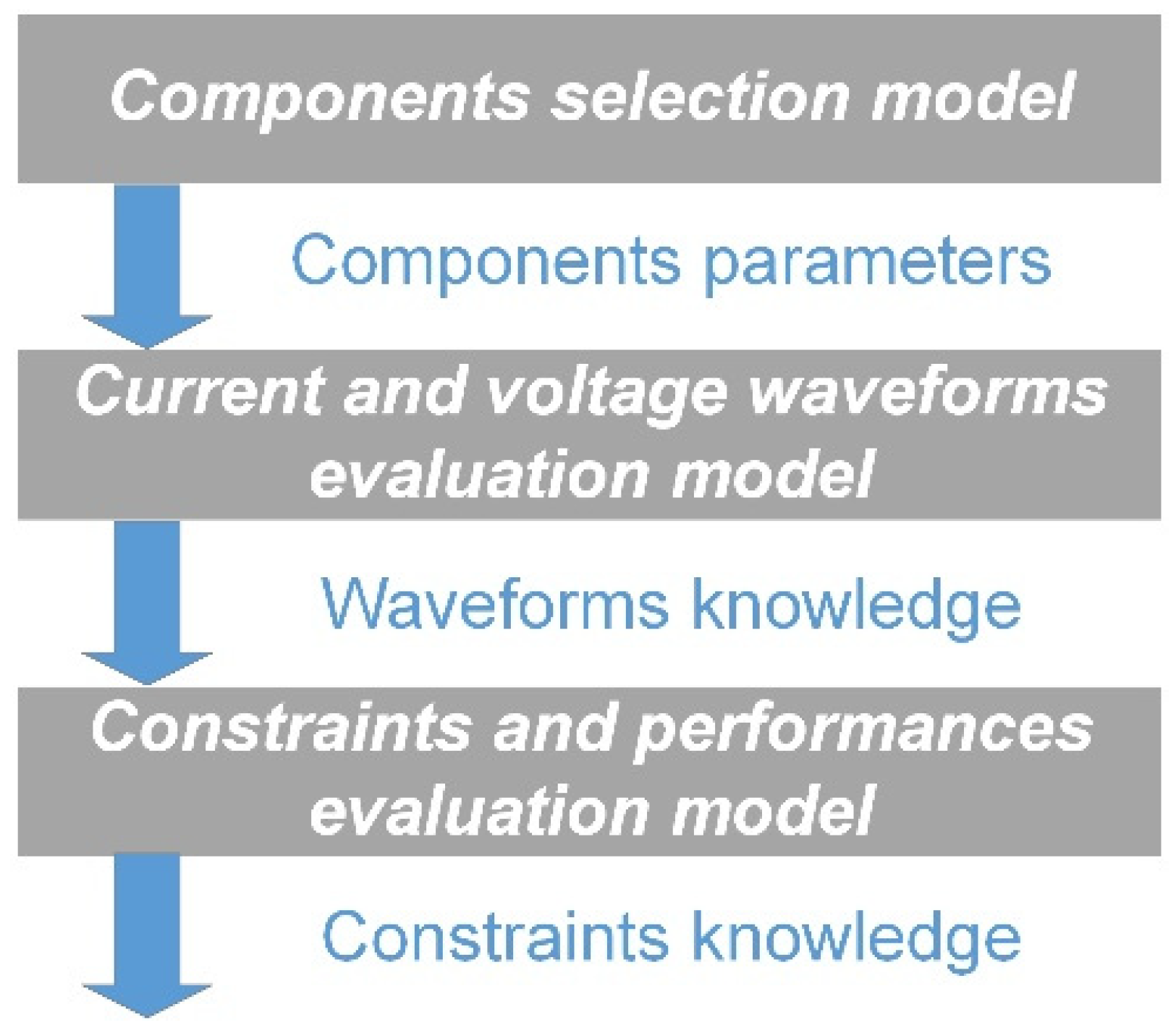
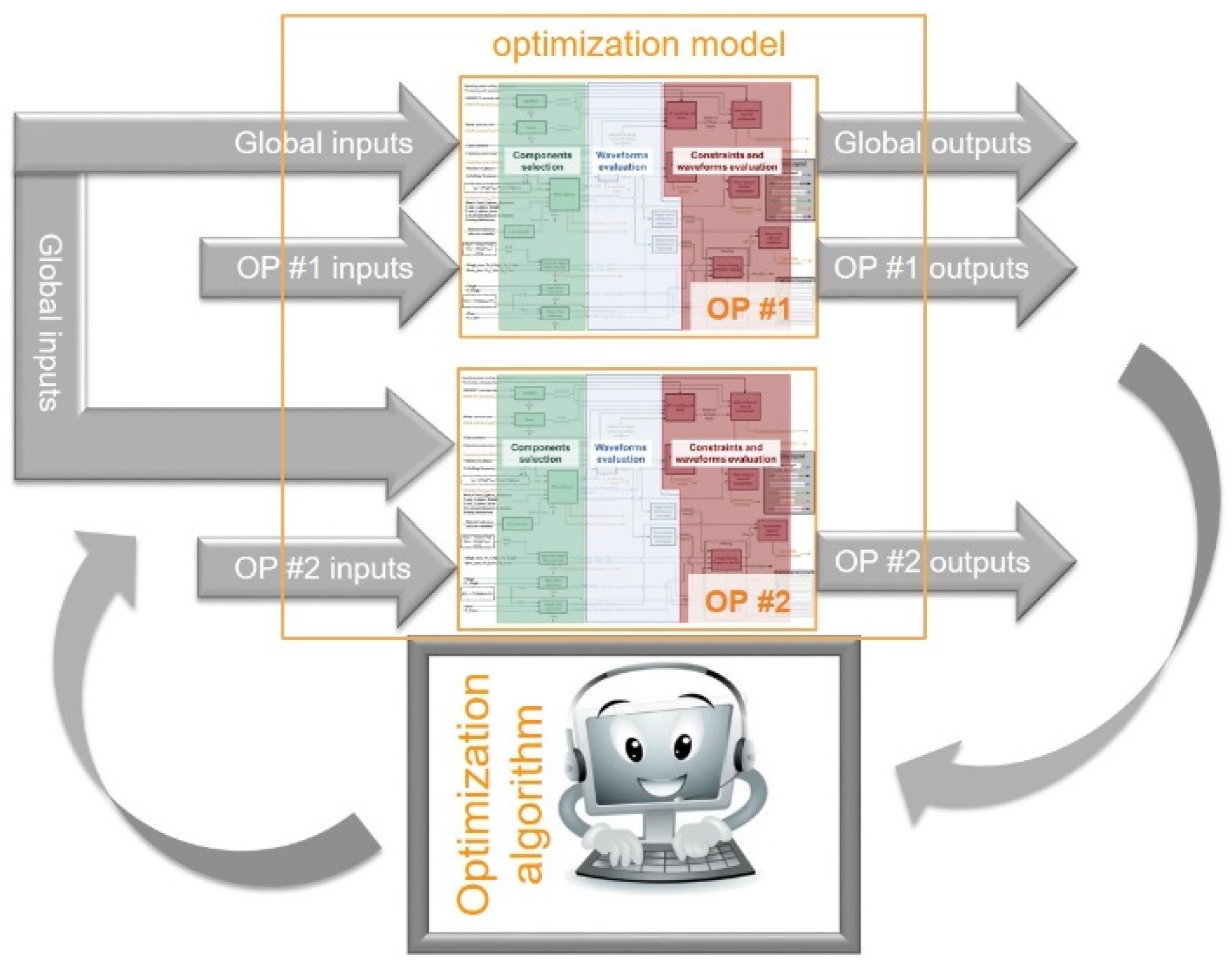
4.3. Definition of the Model for the Proposed Approach with the Study Case
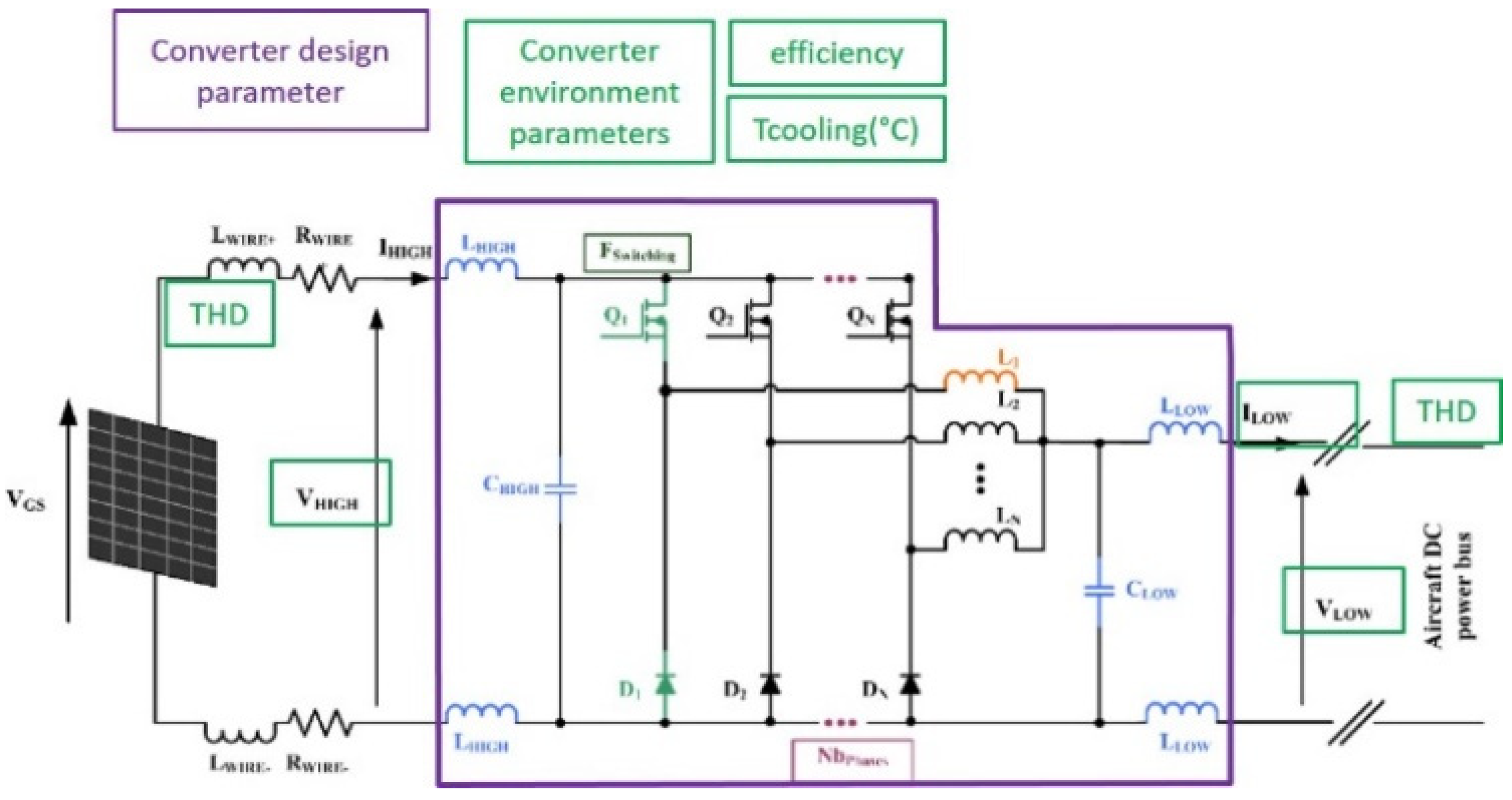
5. Modeling Power Electronics in the Imaginary World
5.1. Modeling the Filtering Function
5.1.1. Filtering Components Selection Models
5.1.2. Converter Input and Output Current Switched Waveforms Evaluation Models
5.1.3. Converter Input and Output Current THD Evaluation Models
5.2. Phase Inductor Modeling
5.2.1. Phase Inductor Sizing Direct Model
5.2.2. Phase Inductor Constraints Evaluation
5.3. Semiconductor Selection Model
5.3.1. Semiconductors Losses and Thermal Model
5.3.2. Management of the Junction Temperature Implicit Equation
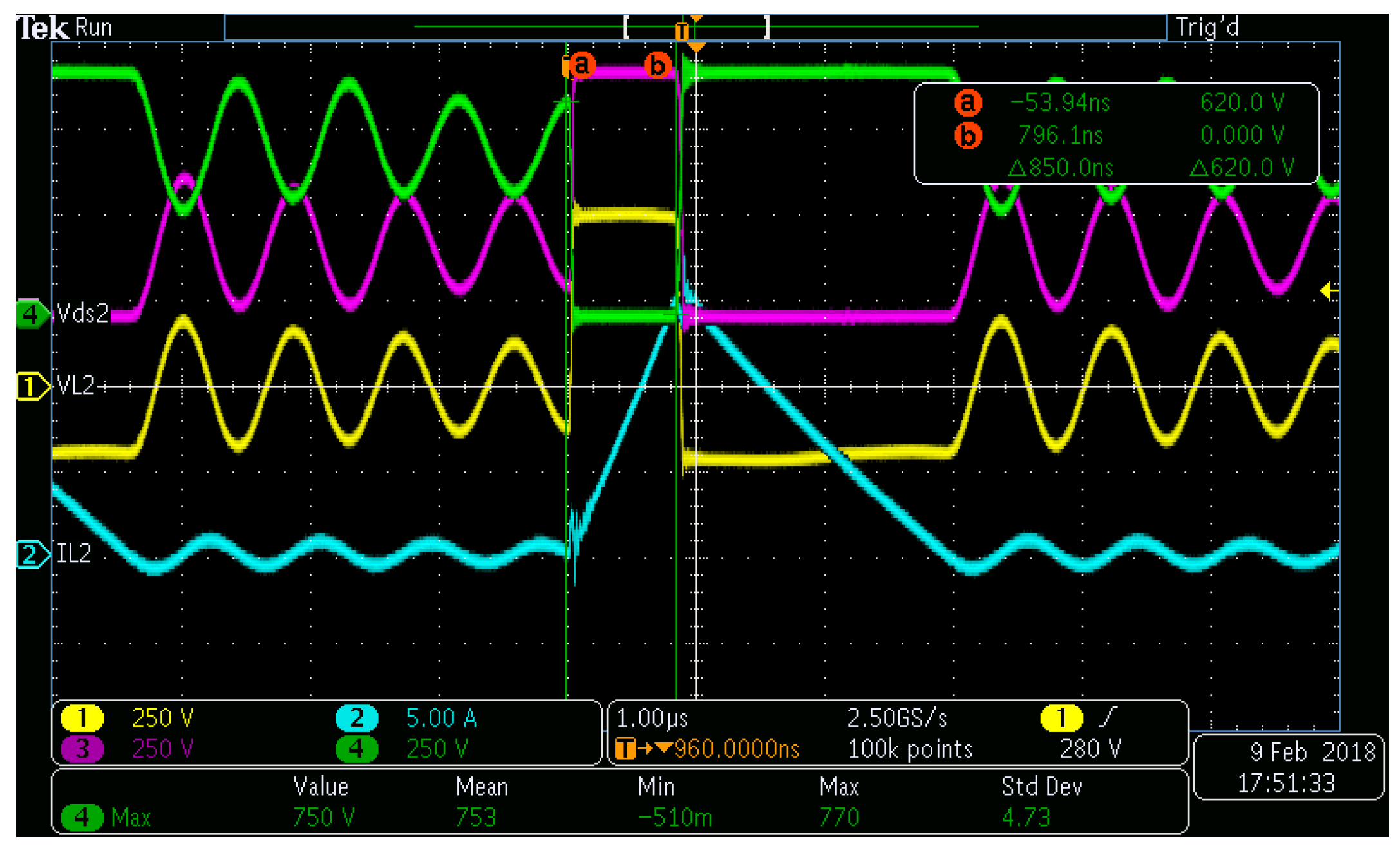
5.4. Test of the Models’ Validity Domain
6. Optimizations in the Imaginary World
6.1. Optimization Problem Set Up according to Initial Set of Specifications
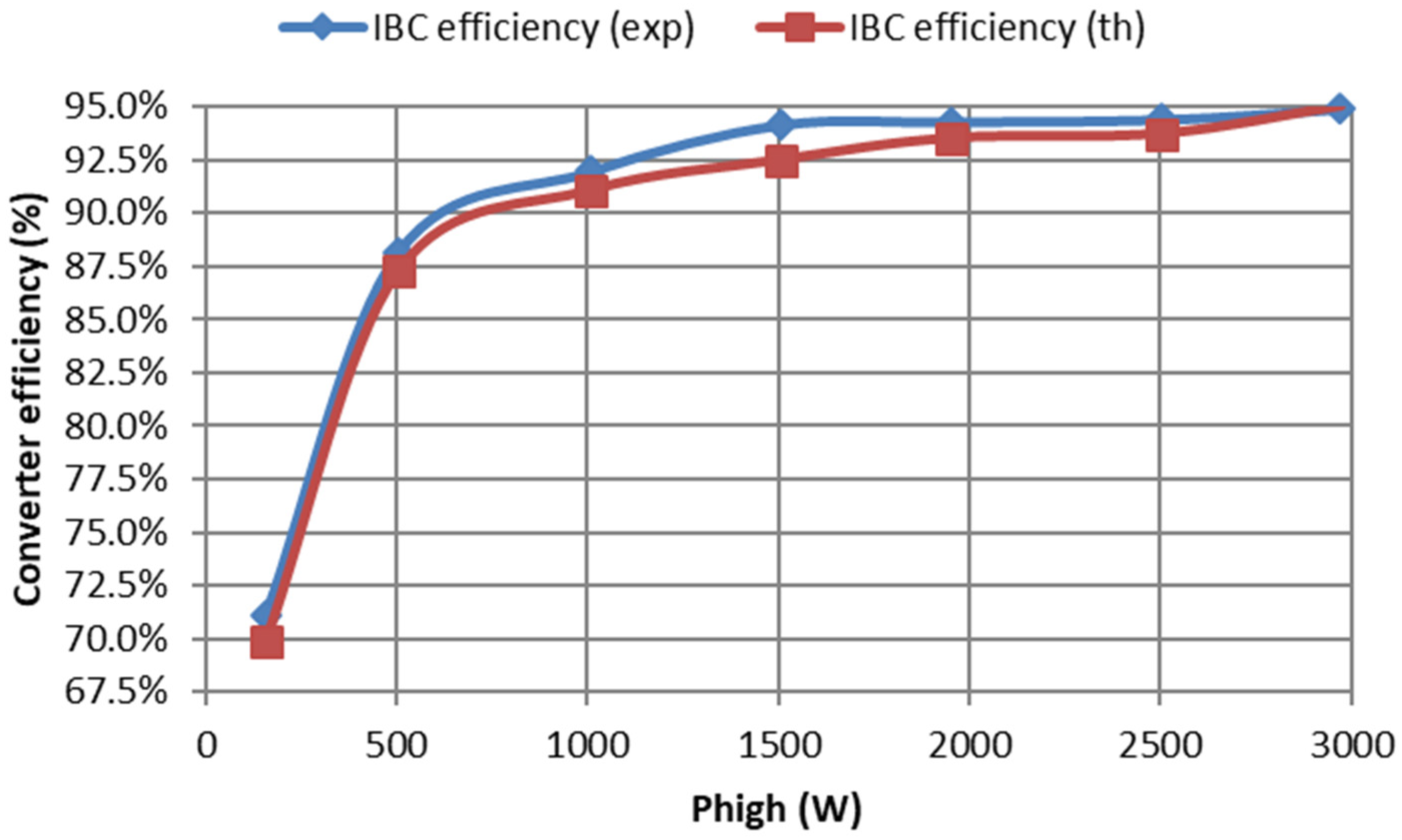
6.2. Optimization in the Imaginary World Results
7. Negotiation of the Set of Specifications
7.1. Specific Procedure for the Purpose
7.2. Examples of Specifications Negotiation in the Imaginary World
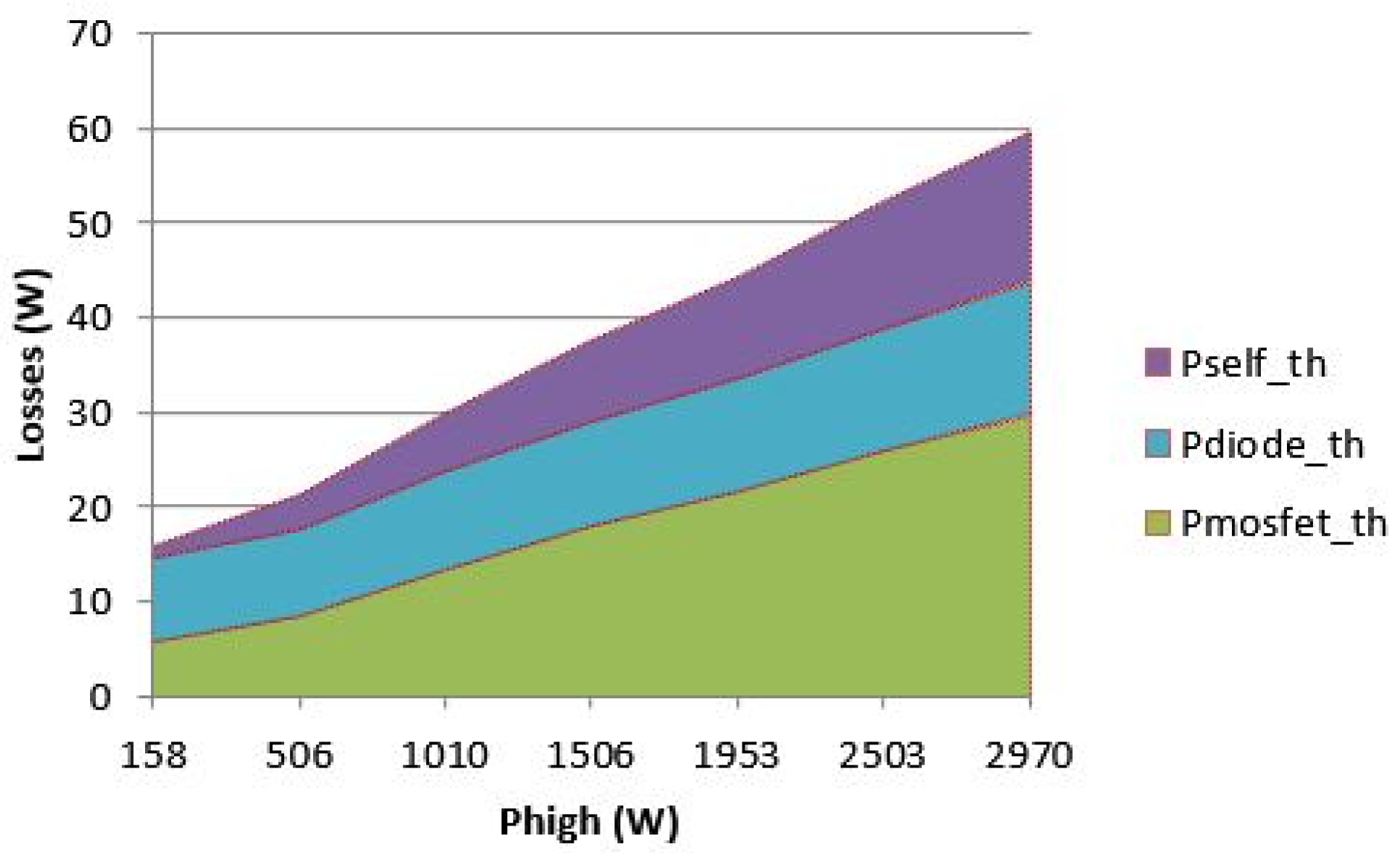
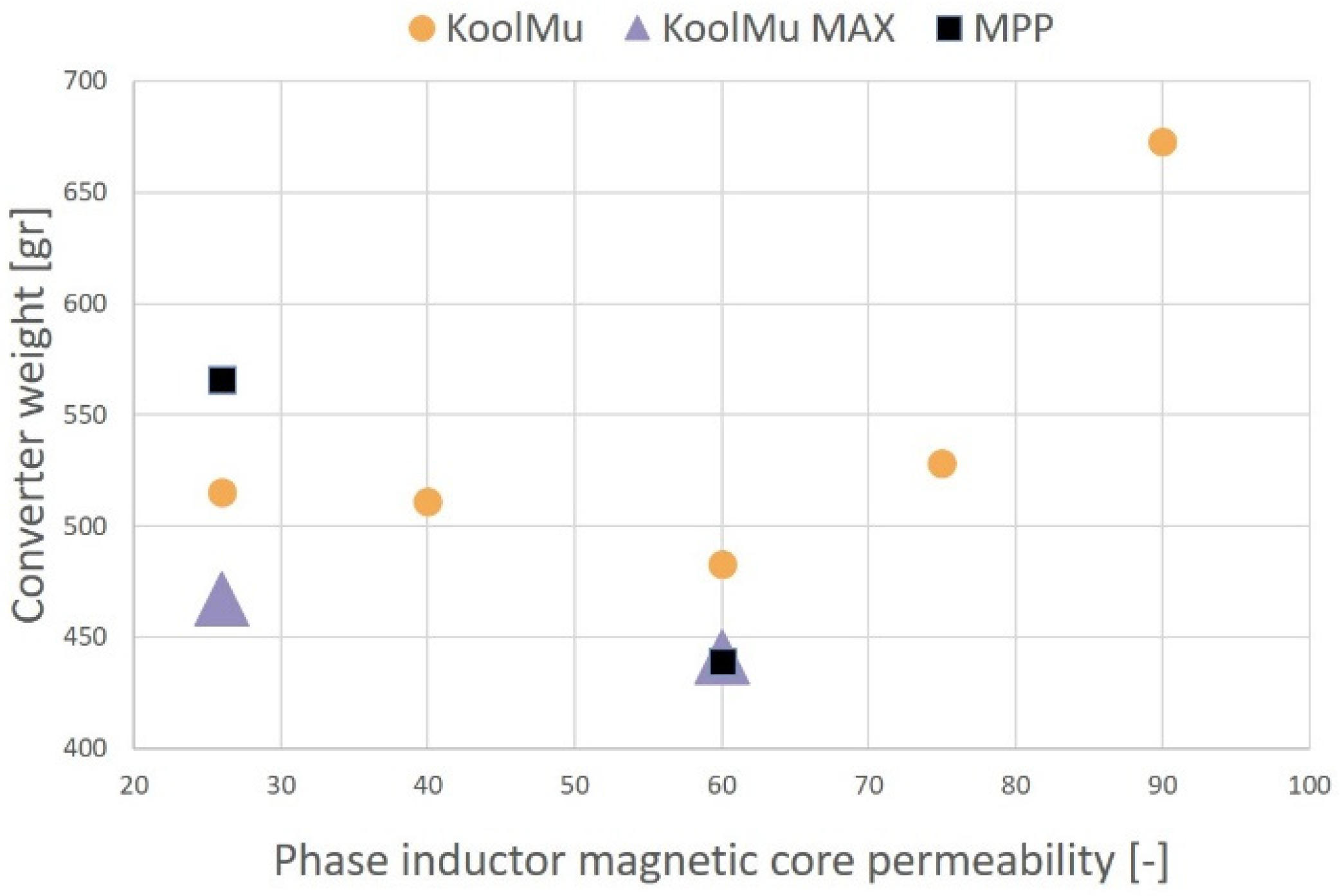
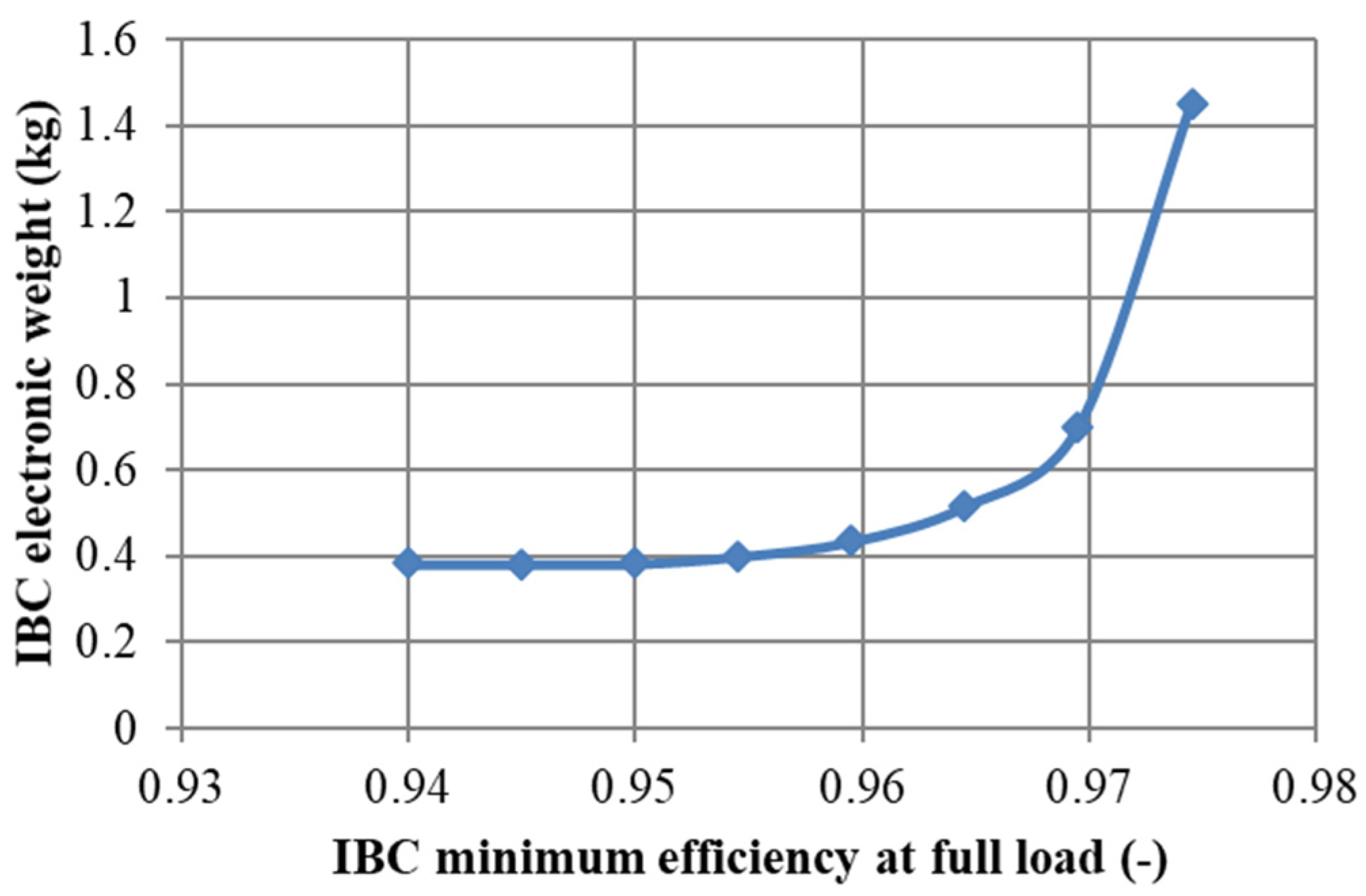
7.2.1. IBC Efficiency vs. Weight
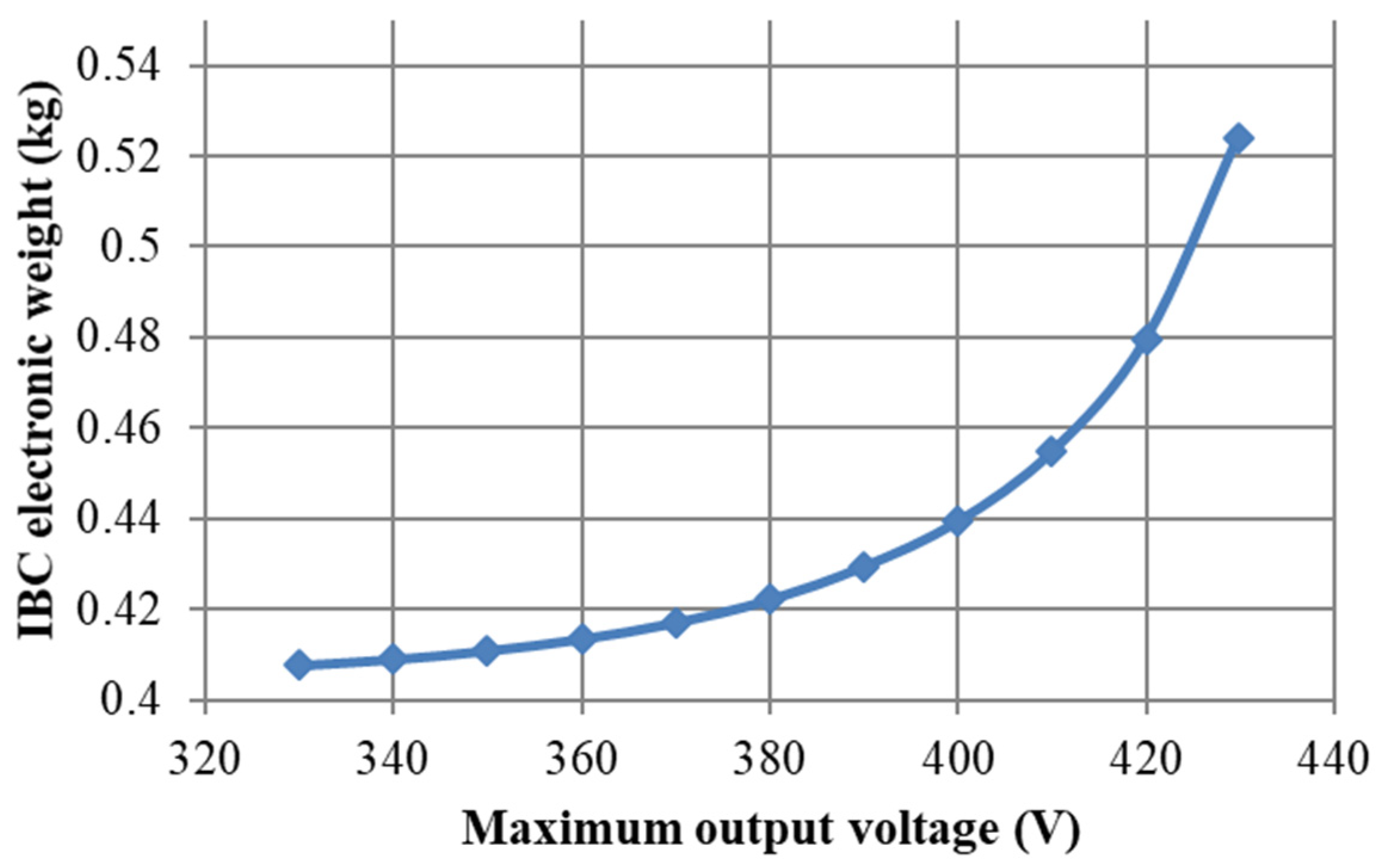
7.2.2. Maximum Output Voltage vs. Weight
7.2.3. Conclusions
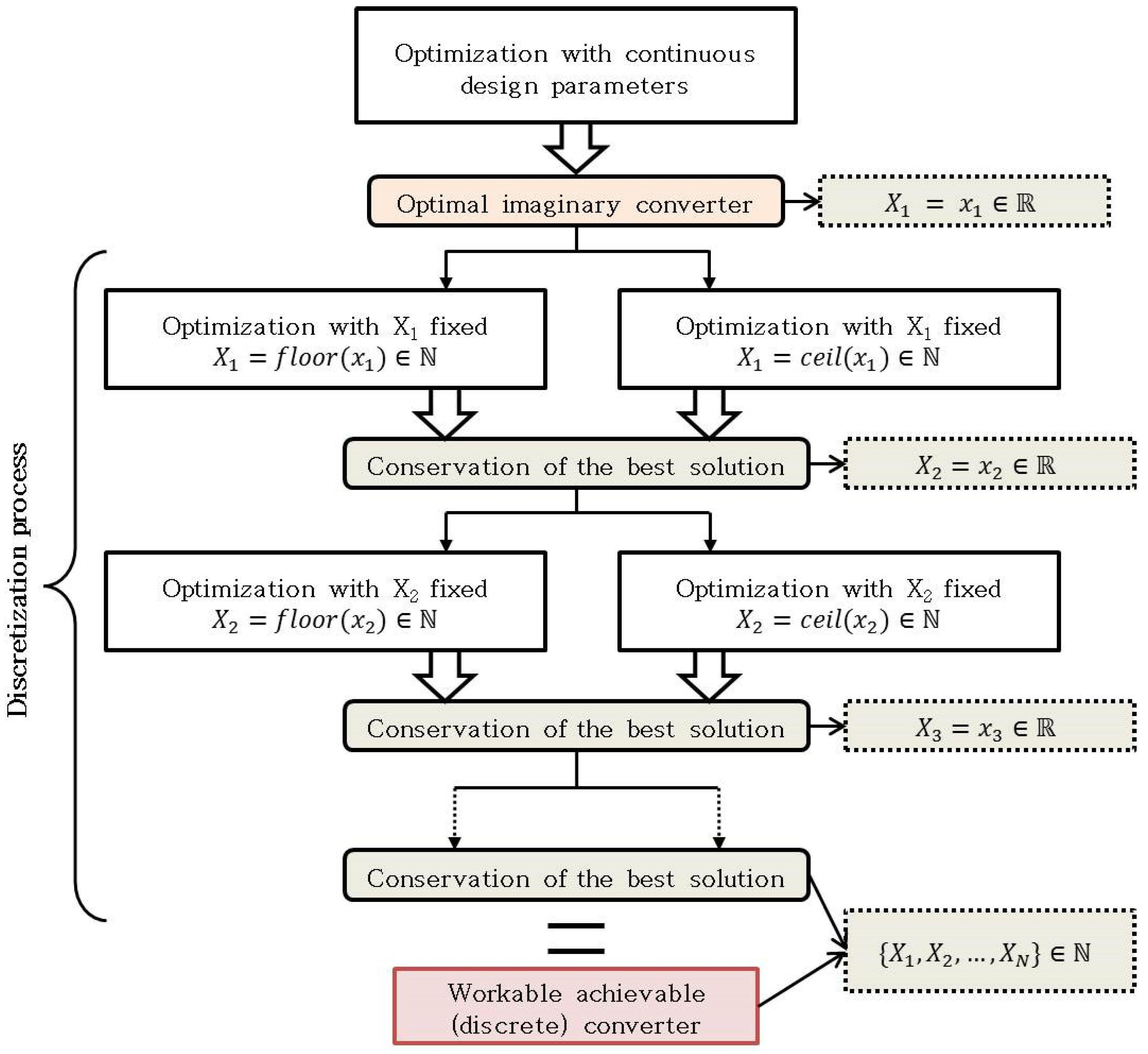
8. Discretization Process
9. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kolar, J.W.; Biela, J.; Waffler, S.; Friedli, T.; Badstuebner, U. Performance trends and limitations of power electronic systems. In Proceedings of the 2010 6th International Conference on Integrated Power Electronics Systems, Nuremberg, Germany, 16–18 March 2010; pp. 1–20. [Google Scholar]
- Berliner, C.; Brimson, J.A. Cost Management for Today’s Advanced Manufacturing: The CAM-I Conceptual Design; Harvard Business School Press: Boston, MA, USA, 1988. [Google Scholar]
- Kline, W.A.; Schindel, W.D. Engineering design, a shift from a process to a model-based view. In Proceedings of the 2017 IEEE Frontiers in Education Conference (FIE), Indianapolis, IN, USA, 18–21 October 2017; pp. 1–3. [Google Scholar]
- Delhommais, M. Review of optimization methods for the design of power electronics systems. In Proceedings of the European Conference on Power Electronics and Application, Lyon, France, 7–11 September 2020. [Google Scholar]
- Marchand, C.; Coquery, G.; Larouci, C.; Bendali, M.; Azib, T. Design methodology of an interleaved buck converter for onboard automotive application, multi-objective optimisation under multi-physic constraints. IET Electr. Syst. Transp. 2015, 5, 53–60. [Google Scholar]
- Li, X.; Zhang, X.; Lin, F.; Blaabjerg, F. Artificial-Intelligence-Based Design for Circuit Parameters of Power Converters. IEEE Trans. Ind. Electron. 2021, 69, 11144–11155. [Google Scholar] [CrossRef]
- Busquets-Monge, S.; Crebier, J.-C.; Ragon, S.; Hertz, E.; Boroyevich, D.; Gurdal, Z.; Arpilliere, M.; Lindner, D. Design of a Boost Power Factor Correction Converter Using Optimization Techniques. IEEE Trans. Power Electron. 2004, 19, 1388–1396. [Google Scholar] [CrossRef]
- Stupar, A.; McRae, T.; Vukadinovic, N.; Prodic, A.; Taylor, J.A. Multi-Objective Optimization of Multi-Level DC–DC Converters Using Geometric Programming. IEEE Trans. Power Electron. 2019, 34, 11912–11939. [Google Scholar] [CrossRef]
- Baurreau, F.; Staraj, R.; Ferrero, F.; Lizzi, L.; Ribero, J.M.; Chessel, J.P. Stratospheric platform for telecommunication missions. In Proceedings of the IEEE Antennas and Propagation Society AP-S International Symposium, Vancouver, BC, Canada, 19–24 July 2015; pp. 914–915. [Google Scholar]
- Thales, “Space Q & A: All about Stratobus”. 2017. Available online: https://www.thalesgroup.com/en/worldwide/space/news/space-qa-all-about-stratobus (accessed on 13 June 2017).
- Mühlethaler, J.; Biela, J.; Kolar, J.W.; Ecklebe, A. Improved core loss calculation for magnetic components employed in power electronic system. In Proceedings of the Twenty-Sixth Annual IEEE Applied Power Electronics Conference and Exposition (APEC), Fort Worth, TX, USA, 6–11 March 2011; pp. 1729–1736. [Google Scholar]
- Voldoire, A.; Schanen, J.L.; Ferrieux, J.P.; Sarrazin, B.; Gautier, C. Predesign Methodology of Voltage Inverters using a Gradient-Based Optimization Algorithm. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 9, 5895–5905. [Google Scholar] [CrossRef]
- Delhommais, M.; Schanen, J.-L.; Wurtz, F.; Rigaud, C.; Chardon, S. Design by Optimization Methodology: Application to a Wide Input and Output Voltage Ranges Interleaved Buck Converter. In Proceedings of the Energy Conversion Congress & Exposition, Cincinnati, OH, USA, 1–5 October 2017; pp. 1–8. [Google Scholar]
- Enciu, P.; Gerbaud, L.; Wurtz, F. Automatic differentiation for sensitivity calculation in electromagnetism: Application for optimization of a linear actuator. IEEE Trans. Magn. 2011, 47, 1238–1241. [Google Scholar] [CrossRef]
- Gerbaud, L.; Baraston, A.; Schanen, J.; Delhommais, M. Selectivity in Frequency Modelling of Electrical Circuit for the Sizing by Optimization of Emc Filter for Power Electronics. In Proceedings of the Electrimacs, Toulouse, France, 4–6 July 2017; pp. 2–7. [Google Scholar]
- Boggs, P.T.; Tolle, J.W. Sequential Quadratic Programming. Acta Numer. 1996, 4, 1–52. [Google Scholar] [CrossRef] [Green Version]
- Zhang, S.; Yu, X. The Output Current Analysis and Its Applications in the Interleaved Boost Converter; Institute of Electrical and Electronics Engineers: Scottsdale, AZ, USA, 2012; pp. 1–5. [Google Scholar] [CrossRef]
- Choe, G.-Y.; Kim, J.-S.; Kang, H.-S.; Lee, B.-K. An Optimal Design Methodology of an Interleaved Boost Converter for Fuel Cell Applications. J. Electr. Eng. Technol. 2010, 5, 319–328. [Google Scholar] [CrossRef] [Green Version]
- Zhang, S.; Yu, X. A Unified Analytical Modeling of the Interleaved Pulse Width Modulation (PWM) DC-DC Converter and Its Applications. IEEE Trans. Power Electron. 2013, 28, 5147–5158. [Google Scholar] [CrossRef]
- Venkatachalam, K.; Sullivan, C.R.; Abdallah, T.; Tacca, H. Accurate prediction of ferrite core loss with non sinusoidal waveforms using only steinmetz parameters. In Proceedings of the IEEE Workshop on Computers in Power Electronics, COMPEL, Portland, OR, USA, 14 August 2002; pp. 36–41. [Google Scholar]
- New England Wire Technologies, “Litz Wire Theory”. 2017. Available online: https://www.newenglandwire.com/products/litz-wire-and-formed-cables/theory (accessed on 13 June 2017).
- Voldoire, A.; Schanen, J.; Ferrieux, J.-P.; Rizet, C.; Gautier, C.; Saber, C. Validation of Inductor Analytical Loss Models under Saturation Conditions for PWM Inverter. In Proceedings of the 21th European Power Electronics and Applications, in Acceptance Process, Genova, Italy, 3–5 September 2019; Volume 2, pp. 2–7. [Google Scholar]
- Delhommais, M.; Schanen, J.-L.; Wurtz, F.; Rigaud, C.; Chardon, S.; Vighetti, S. Thermal model of Litz wire toroidal inductor based on experimental measurements. In Proceedings of the IEEE Applied Power Electronics Conference and Exposition (APEC), San Antonio, TX, USA, 4–8 March 2018. [Google Scholar]
- Kyaw, P.A.; Delhommais, M.; Qiu, J.; Sullivan, C.R.; Schanen, J.; Rigaud, C. Thermal Modeling of Inductor and Transformer Windings Including Litz Wire. J. Submitt. IEEE Trans. Power Electron. 2019, 35, 1–38. [Google Scholar] [CrossRef]
- Delhommais, M.; Schanen, J.; Wurtz, F.; Rigaud, C.; Chardon, S. First Order Design by Optimization Method: Application to an Interleaved Buck Converter and Validation. In Proceedings of the IEEE Applied Power Electronics Conference and Exposition (APEC), San Antonio, TX, USA, 4–8 March 2018; pp. 944–951. [Google Scholar]
- Peng, K.; Santi, E. Performance Projection and Scalable Loss Model of SiC MOSFETs and SiC Schottky Diodes. In Proceedings of the IEEE Electric Ship Technologies Symposium, Old Town Alexandria, VA, USA, 21–24 June 2015; pp. 281–286. [Google Scholar]
- Peng, K.; Eskandari, S.; Santi, E. Analytical loss model for power converters with SiC MOSFET and SiC schottky diode pair. In Proceedings of the 2015 IEEE Energy Conversion Congress and Exposition (ECCE), Montreal, QC, Canada, 20–24 September 2015; pp. 6153–6160. [Google Scholar]
- Coutel, C.; Wurtz, F.; Bigeon, J. A comparative study of two methods for constrained optimisation with analytical models dealing with implicit parameters. IEEE Trans. Magn. 1999, 35, 1738–1741. [Google Scholar] [CrossRef]
- Vesta System, “CADES 4” . 2019. Available online: http://www.cades-solutions.com/?page_id=390 (accessed on 1 September 2019).
- Voldoire, A.; Schanen, J.-L.; Ferrieux, J.-P.; Derbey, A.; Gautier, C. Three-Phase PWM Voltage-Source-Inverter Weight Optimization for Aircraft Application Using Deterministic Algorithm. Electronics 2020, 9, 1393. [Google Scholar] [CrossRef]


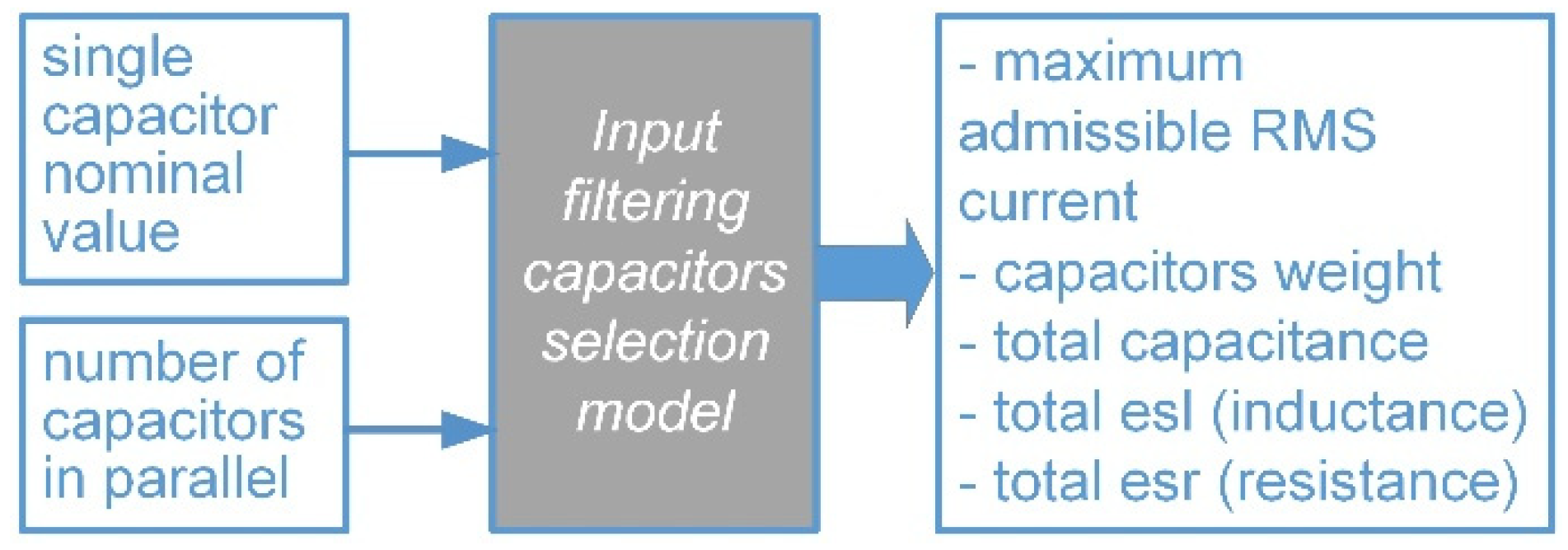
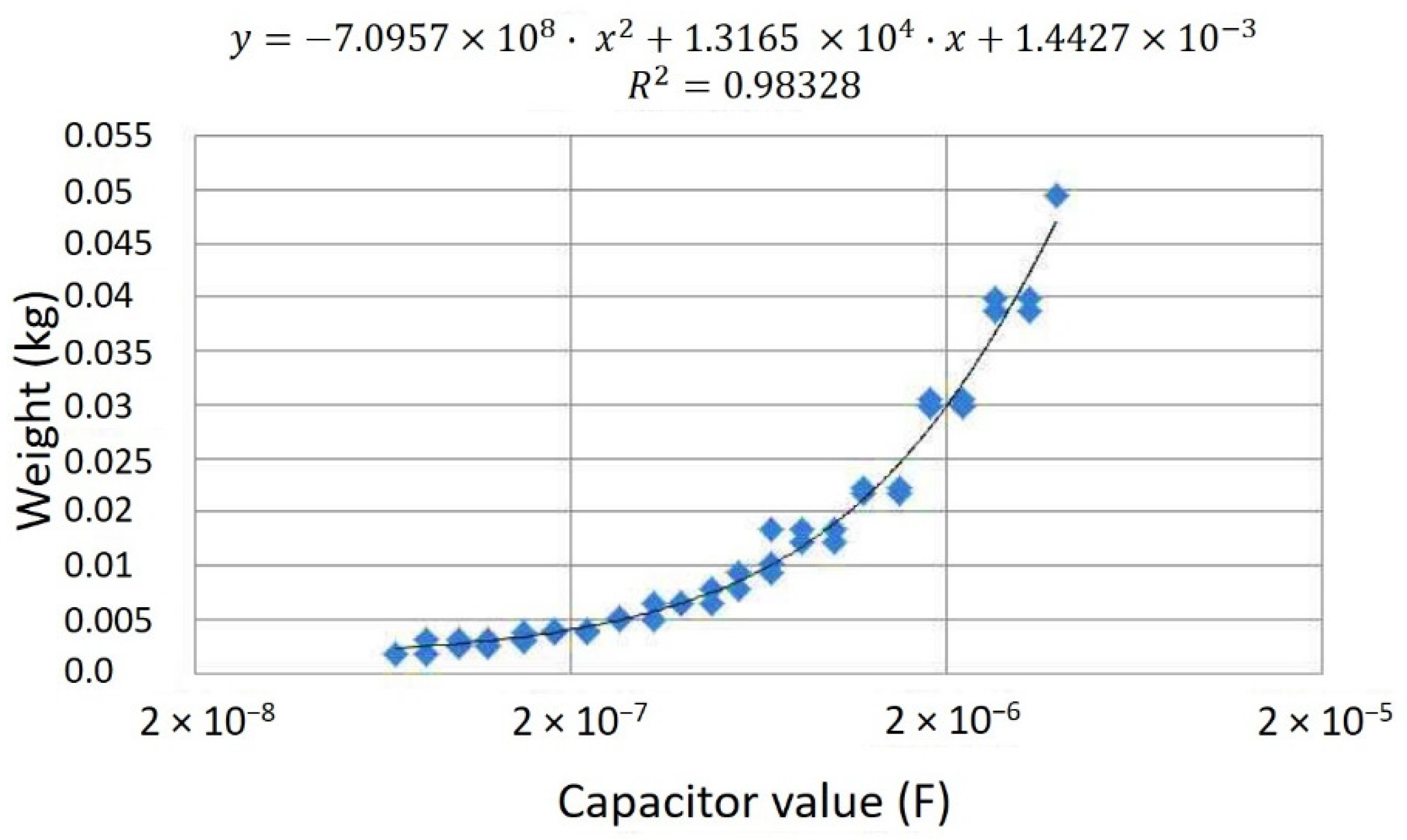
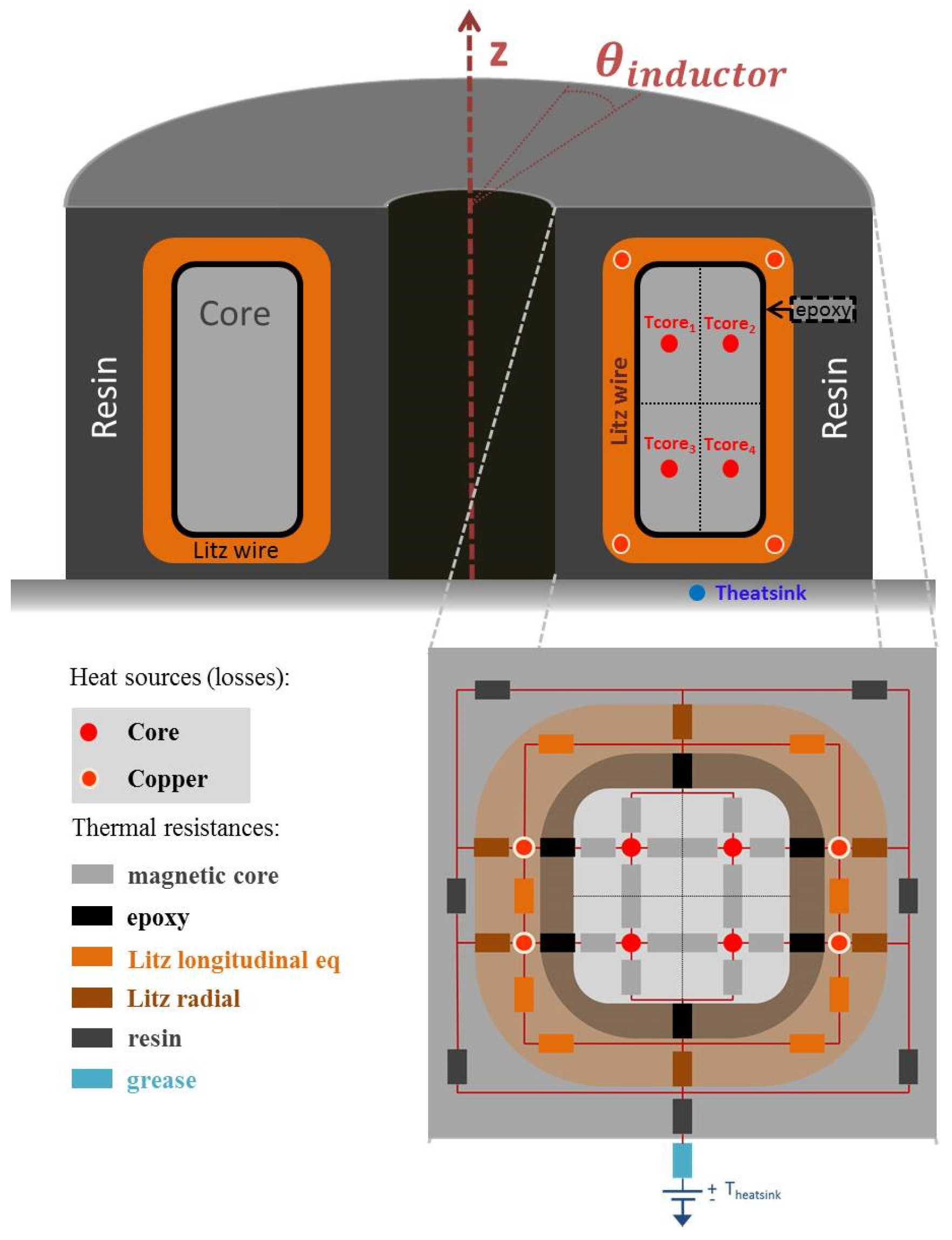
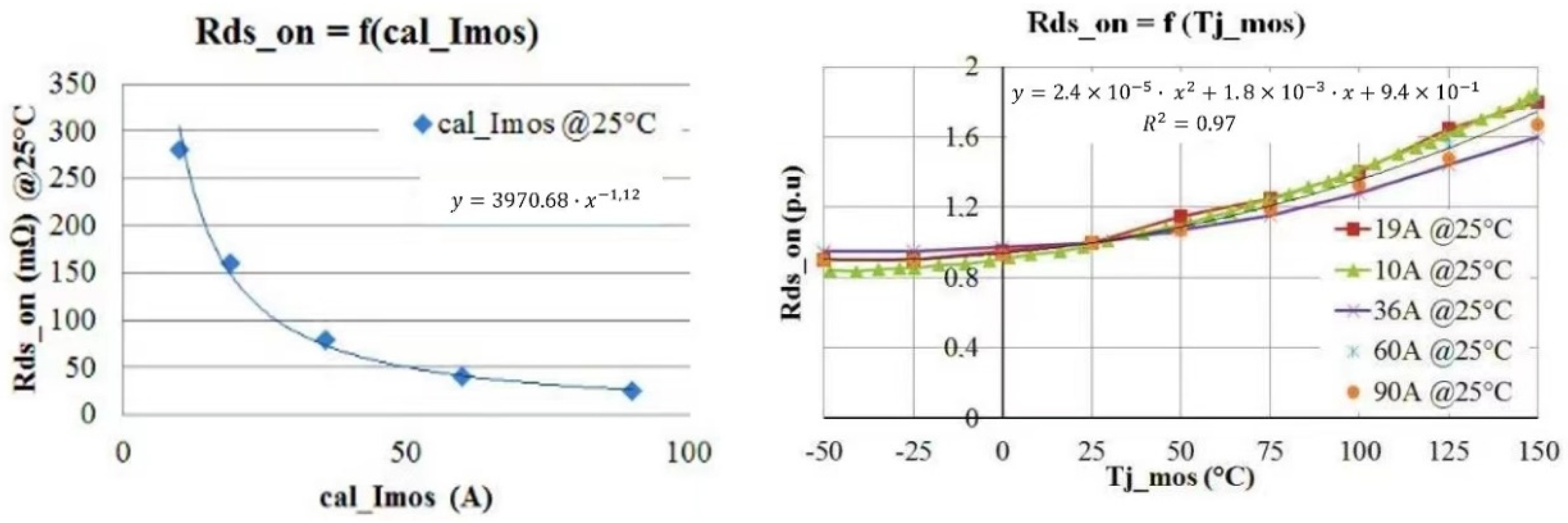

| Design Parameter | Abbreviation | Nb of Discrete Choices |
|---|---|---|
| Switching frequency | Fs | - |
| Number of IBC Phase | NBphaseN | 6 |
| Conduction mode | CDT | 2 |
| Inductor core material | MaterialT | 3 |
| Inductor core main permeabilities (14, 26, 40, 60, 125) | MaterialD | 5 |
| Toroidal inductor core size (External/Internal diameter/Height) | DoutD, DinD, HD | ~36 |
| Litz strand diameter (AWG 33, 36, 38, 40, 42) | DlitzLphaseD | 5 |
| Litz number of strands | nstrandLphaseN | 15 |
| Phase inductor number of turns | NturnsLphaseN | ~100 |
| SiC 1200 V MOSFET current rating | Cal_ImosD | 5 |
| SiC 1200 V Schottky diode current rating | Cal_IdiodeD | 10 |
| Input single capacitor value | ChighD | 17 |
| Output single capacitor value | ClowD | 46 |
| Number of input capacitors in parallel | N_ChighN | 15 |
| Number of output capacitors in parallel | N_ClowN | 15 |
| Input single filtering inductor value @ 0A | Lhigh_maxD | 17 |
| Output single filtering inductor value @ 0A | Llow_maxD | 17 |
| Number of input inductors in series | Ns_LhighN | 5 |
| Number of output inductors in series | Ns_LlowN | 5 |
| Number of input inductors in parallel | Np_LhighN | 5 |
| Material | MPP 60 |
|---|---|
| Optimization iterations (#) | 10 |
| Optimization time (s) | 75 |
| Converter weight (gr) | 439 |
| Switching frequency (kHz) | 164 |
| number of phases | 3.2 |
| Inductor value (µH) | 33.6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Delhommais, M.; Delaforge, T.; Schanen, J.-L.; Wurtz, F.; Rigaud, C. A Predesign Methodology for Power Electronics Based on Optimization and Continuous Models: Application to an Interleaved Buck Converter. Designs 2022, 6, 68. https://doi.org/10.3390/designs6040068
Delhommais M, Delaforge T, Schanen J-L, Wurtz F, Rigaud C. A Predesign Methodology for Power Electronics Based on Optimization and Continuous Models: Application to an Interleaved Buck Converter. Designs. 2022; 6(4):68. https://doi.org/10.3390/designs6040068
Chicago/Turabian StyleDelhommais, Mylène, Timothé Delaforge, Jean-Luc Schanen, Frédéric Wurtz, and Cécile Rigaud. 2022. "A Predesign Methodology for Power Electronics Based on Optimization and Continuous Models: Application to an Interleaved Buck Converter" Designs 6, no. 4: 68. https://doi.org/10.3390/designs6040068
APA StyleDelhommais, M., Delaforge, T., Schanen, J.-L., Wurtz, F., & Rigaud, C. (2022). A Predesign Methodology for Power Electronics Based on Optimization and Continuous Models: Application to an Interleaved Buck Converter. Designs, 6(4), 68. https://doi.org/10.3390/designs6040068







