Appendix A. Specified Loads
Appendix A.1. Snow Load NBCC 4.1.6
The governing snow load [
56] is composed of multiple factors shown in Equation (A1),
The Importance Factor, IS, is taken as 0.8 because the failure of a PV system has little to no risk of the loss of life.
The Ground Snow Load Factor,
SS, is dependent on the location of the structure. It is the 1-in-50-year snow load found in Table C-2 in NBCC 4.1.6 For London, Ontario, a value of 1.90 must be taken [
56].
The Basic Roof Snow Load Factor, Cb, is 0.80 for small structures.
The Wind Exposure Factor, Cw, is taken as 0.75 if the structure is exposed to wind in all directions.
The Slope Factor,
Cs, is dependent on the tilt angle of the system. It can be calculated using the following equation from NBCC 4.1.6.
The Accumulation Factor, Ca, is taken as 1.00 for small monoslope structures.
The Rain Factor,
Sr, depends on the location of the structure. For London, Ontario, a value of 0.40 must be taken according to Table C-2 in NBCC 4.1.6 [
56].
The factors and calculated net snow load are summarized in
Table A1.
Table A1.
Design Snow Load. Note that the minimum specified snow load is to be taken as 1.00 kPa.
Table A1.
Design Snow Load. Note that the minimum specified snow load is to be taken as 1.00 kPa.
| Coefficient | Value |
|---|
| Is | 0.80 |
| Ss | 1.90 1 |
| Cb | 0.80 |
| CW | 0.75 |
| CS | 0.58 2 |
| Ca | 1.00 |
| Sr | 0.40 1 |
| S | 0.85 3 |
Appendix A.2. Wind Load
The specified wind load is adapted from the National Building Code of Canada 2015, Division B, 4.1.7, and is composed of both an external wind pressure, and internal wind pressure,
where the external wind pressure is composed of the following factors,
The internal wind pressure is composed of the following factors,
The Wind Importance Factor, IW, is taken as 0.80 because the failure of a PV system has little to no risk of the loss of life.
The Hourly Wind Pressure Factor, q, is dependent on the location of the structure. It is the 1-in-50-year wind load found in Table C-2 in NBCC 4.1.6 For London, Ontario, a value of 0.47 must be taken.
The Exposure Factor, Ce, shall be taken as 0.90 for structures less than 10 m in height.
The topographic factor, Ct, is to be taken as 1.00.
The Pressure and Gust Factors,
Cp and
Cg, are combined and found using the table from Figure 4.1.7.6-A from NBCC [
56]. From the figure, the governing building for 34-degree systems is 2, which corresponds to a
CpCg value of −1.30.
The Internal Exposure Factor, Cei, is the same as the External Exposure Factor, Ce since the structure has a “dominant opening”, meaning that wind can attack the inside of the system just as easily as it can attack the outside.
The Internal Gust Factor, Cgi, is taken as 2.00 since is not a large unpartitioned volume, such as an arena.
The Internal Pressure Factor, Cpi, will be taken as −0.70 because the wind has easy access to push against the back of the system.
The factors and calculated net snow load are summarized in
Table A2.
Table A2.
Design Wind Load as per NBCC 4.1.7.3.
Table A2.
Design Wind Load as per NBCC 4.1.7.3.
| Coefficient | Value |
|---|
| IW | 0.80 |
| q | 0.47 |
| Ce | 0.90 |
| Ct | 1.00 |
| CpCg | −1.30 |
| Cei | 0.90 |
| Cgi | 2.00 |
| CPi | −0.70 |
| p | −0.44 |
| pi | −0.47 |
| W | −0.91 kPa |
Appendix A.3. Specified Dead Load
The dead load, or the self-weight of the structure, will consist of the weight of the PV modules, and the weights of the wooden members. The weight of brackets and fasteners is much smaller than the design load and can be considered negligible. According to Natural Resources Canada’s CanmetENERGY research centre [
78], a dead load of PV systems, also known as the superimposed dead load, shall be taken as 0.24 kPa. The self-weight of the lumber depends on what dimensions of lumber has sufficient capacity to carry the load. The self-weight of lumber is variable due to changing moisture content and amount of knots. For analysis purposes, it is best practice to use the lumber weights provided by the supplier, and translate the given weight into a uniform distributed load in kN/m.
Appendix A.4. Load Combinations
Since many simple assumptions are made in the analysis process, safety factors must be applied to the specified loads to reduce the probability of failure. These factored loads are then combined as principle loads and companion loads via the load combinations found in NBCC 4.1.3.2 [
56]. The combination of principal loads and companion loads that creates the largest net load shall be used in the analysis. Principle loads are the mandatory loads that must be checked, and companion loads are only added if they act in the same direction as the principal loads. Since the design wind load acts in the negative direction and the governing snow load is in the positive direction, they shall not be combined as doing so will reduce the net load. The combinations that produced the largest positive and negative are found in
Table A3.
Table A3.
Load Combinations as per NBCC 4.1.3.2. Based on Design Dead, Snow, and Wind Loads.
Table A3.
Load Combinations as per NBCC 4.1.3.2. Based on Design Dead, Snow, and Wind Loads.
| Combination | Net Load [kPa] |
|---|
| 0.9D 1 + 1.4W | −1.06 |
| 1.25D + 1.5S | 1.80 |
It should be noted that the load path for the positive case is the same as the negative case, the only difference being the direction of the load. Since all connections can resist loads in both directions, and all members have the same material properties in both directions, the analysis for the negative case is the same as the positive case. Thus, the negative case can be ignored, and the positive case will be used for the analysis.
The same process will be applied to a system located in Lomé, Togo. For this system, it is justified to assume that the snow load is 0 kPa. All wind coefficients can be the same as the London, Ontario system with the exception of q and
CpCg because they depend on the wind speed and tilt angle, respectively. The hourly wind pressure q for Lomé, Togo, is not readily tabulated, but can be calculated using the wind pressure Equation (A6) shown below,
where
ρ is the density of air, and
V is the 1-in-50 year wind speed. NBCC suggests taking a
ρ value of 1.29 kg/m
3 [
56]. According to the European Conference on Severe Storms 2019,
V for Lomé, Togo will be taken as 20 m/s [
79]. Plugging these values into the equation,
q becomes 0.26 kPa.
CpCg for 10 degrees turns out to be −1.30 as well, thus producing a net wind load of −0.42 KPa. The governing load combination for this system is 0.9D + 1.4W, which produces a specified design load for this system to be −0.81 KPa.
Appendix B. Lumber Structural Capacity
The material properties of lumber are extremely variable due to the multiple different species of wood, the existence of knots that alter the load path in the member, and the fluctuating moisture content carried inside the wood. The National Design Speciation for Wood Construction 2018 [
80] provides engineers with trustworthy design values for the vast majority of wood species. Almost all pressure-treated lumber in Canada is made of Spruce Pine Fir grades number 1 and 2 [
81], which possess the structural capacities summarized in
Table A4.
Table A4.
Unfactored capacities for No. 1/2 Spruce Pine Fir Lumber.
Table A4.
Unfactored capacities for No. 1/2 Spruce Pine Fir Lumber.
| Capacity | Value [MPa] |
|---|
| fb | 6.03 |
| fv | 0.93 |
| ft | 3.10 |
| fc | 7.93 |
| E | 9652.60 |
| Emin | 3516.30 |
Although these capacities have proven to be reliable, resistance factors must be applied to the capacities to account for unexpected weaknesses and to ensure a safe and serviceable design.
The load duration factor, Cd, is selected as 1.15 since the snow load is the governing load on the structure.
The temperature factor, Ct, is 1.00 since the structure will not be exposed to temperatures over 100 degrees Fahrenheit.
The beam stability factor,
CL, is calculated as 0.98 as per the guidelines outlined in
Appendix C of the NDS.
The wet service factor, CM, is calculated as 1.00 because the product of fb and Cf is less than 7.9.
The flat use factor, Cfu, is 1.00 because all members are to be loaded upon their strong axis in which h > b.
The incising factor,
Ci, is selected as 0.8 as per Table 4.3.8 in the NDS [
80].
The repetitive member factor, Cr, is 1.00 since the structure cannot be considered a floor or roof system with multiple of the same member.
Finally, the size factor, Cf, is selected as 1.00 because lumber greater than 2 × 12 will not be used for this design.
These factors have been summarized in
Table A5,
Table A5.
Resistance factors as per the National Design Specification for Wood Construction.
Table A5.
Resistance factors as per the National Design Specification for Wood Construction.
| Coefficient | Value |
|---|
| Cd | 1.15 |
| Ct | 1.00 |
| CL | 0.98 |
| CM | 1.00 |
| Cfu | 1.00 |
| Ci | 0.80 |
| Cr | 1.00 |
| Cf | 1.00 |
As per the National Design Specification for Wood Construction, the factored bending capacity is calculated as,
The factored shear stress is calculated as,
The factored tensile stress is calculated as,
The factored compressive stress is calculated as,
Finally, the factored elastic modulus is calculated as,
These factored capacities have been summarized in
Table A6.
Table A6.
Factored capacities for No. 1/2 Spruce Pine Fir Lumber.
Table A6.
Factored capacities for No. 1/2 Spruce Pine Fir Lumber.
| Factored Capacity | Value [MPa] |
|---|
| fb* | 5.44 |
| fv* | 0.86 |
| ft* | 2.85 |
| fc* | 7.29 |
| E* | 9169.97 |
Using the factored capacities and dimensional properties, resistance values can be computed using the following equations,
The resisting bending moment,
Mr, can be calculated as,
The resisting shear force,
Vr, can be calculated as,
The resisting tensile force,
Tr, can be calculated as,
The resisting compressive force,
Cr, can be as,
Resisting values for each dimensional lumber have been summarized in
Table A7.
Table A7.
Resisting values for No. 1/2 Spruce Pine Fir Lumber.
Table A7.
Resisting values for No. 1/2 Spruce Pine Fir Lumber.
| Lumber | Mr [kN·m] | Vr [kN] | Tr [kN] | Cr [kN] 1 |
|---|
| 2 × 4 | 0.27 | 1.93 | 9.65 | 24.66 |
| 2 × 6 | 0.67 | 3.03 | 15.18 | 38.79 |
| 2 × 8 | 1.17 | 3.99 | 19.95 | 50.98 |
| 2 × 10 | 1.90 | 5.09 | 25.48 | 65.12 |
| 2 × 12 | 2.82 | 6.20 | 31.01 | 79.25 |
| 4 × 4 | 0.64 | 4.52 | 22.60 | 57.76 |
| 6 × 6 | 2.49 | 11.18 | 55.92 | 142.92 |
To ensure that the structure does not fail, the following conditions must be met for each member:
The resisting bending moment must be greater than or equal to the maximum applied bending moment,
The resisting shear force must be greater than or equal to the maximum applied shear force,
The resisting tensile force must be greater than or equal to the maximum applied tensile force,
The resisting compressive force must be greater than or equal to the maximum applied compressive force,
Finally, the maximum deflection cannot exceed the member length divided by 360 as per NBCC 9.4.3 [NRC],
Now that a design load and material properties are given, structural analysis can be conducted to determine the optimal dimensions of lumber needed to build a serviceable system.
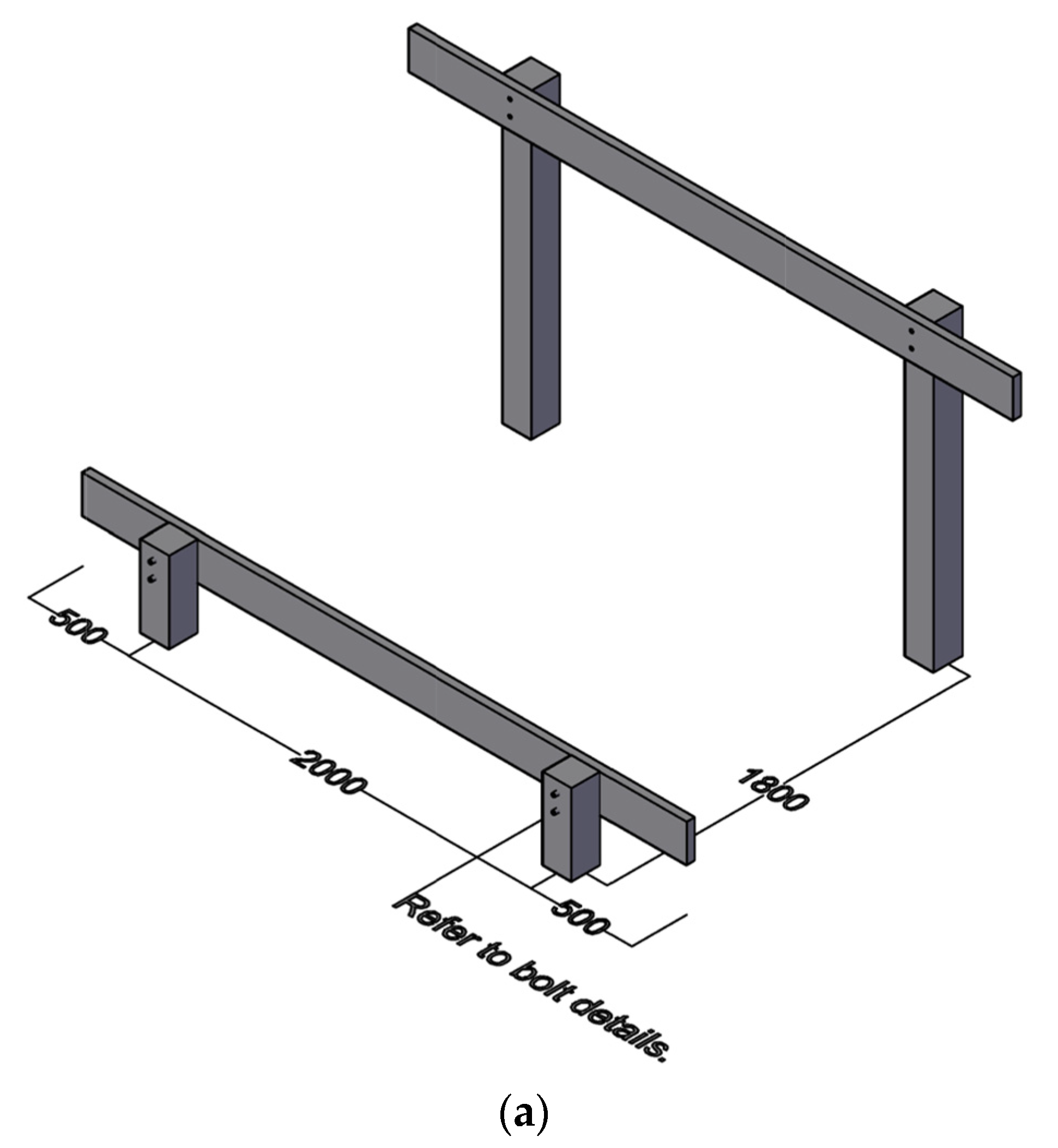
Appendix C. 34 Degree Fixed System Structural Analysis
The net load is distributed evenly throughout the surface of the modules. As per the supplier of the modules [volts.ca], it is assumed that the panels have sufficient capacity to carry these loads. The load is then transferred from the panels to the joists. Each joist carries its own weight as a uniform distributed load,
w, and 4-point loads that represent the block connections.
w is calculated using Equation (A21),
OW represents the own weight of the member, which needs to be multiplied by a factor of 1.25 because it is a dead load [
82]. Since the required dimensions of lumber to carry the load is unknown, an assumption needs to be made (for example, assume 2 × 8) to carry out the analysis. If the assumption results in the maximum applied value being greater than the resistance values, then a larger member needs to be used.
The point loads can be calculated by dividing each joist’s tributary loading into four points because it is assumed that the load is distributed evenly throughout the modules. The tributary area represents how much width of the panels each joist is responsible for carrying. For example, in this three-module system, which is 3 m wide, the middle joists have a tributary width of 1 m (0.5 m on each side), and the end joists have a tributary width of 0.5 m (only one side). The value for each point load on the joists is calculated as,
Once
w and the point loads are calculated, the free body diagram for each joist can be made (
Figure A1),
Figure A1.
Free body diagram of joists. Note that the two outside joists will have half the tributary area of the inside joists, and thus will carry approximately half of the load.
Figure A1.
Free body diagram of joists. Note that the two outside joists will have half the tributary area of the inside joists, and thus will carry approximately half of the load.
Each joist is supported by a beam on each end. The reaction, and thus the load that each joist transfers to each beam is the following,
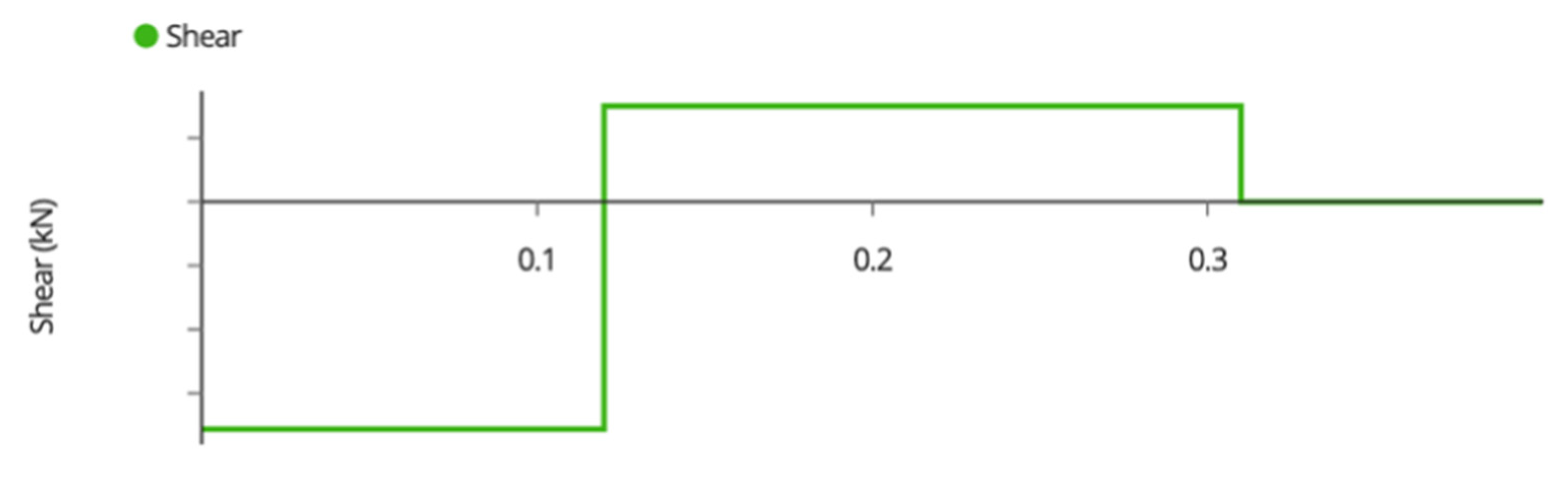
The shear force diagram throughout each joist is the seen as
Figure A2,
Figure A2.
Joist Shear Force Diagram.
Figure A2.
Joist Shear Force Diagram.
The maximum shear force occurs at the supports, and thus the maximum shear is calculated as the reaction shown above.
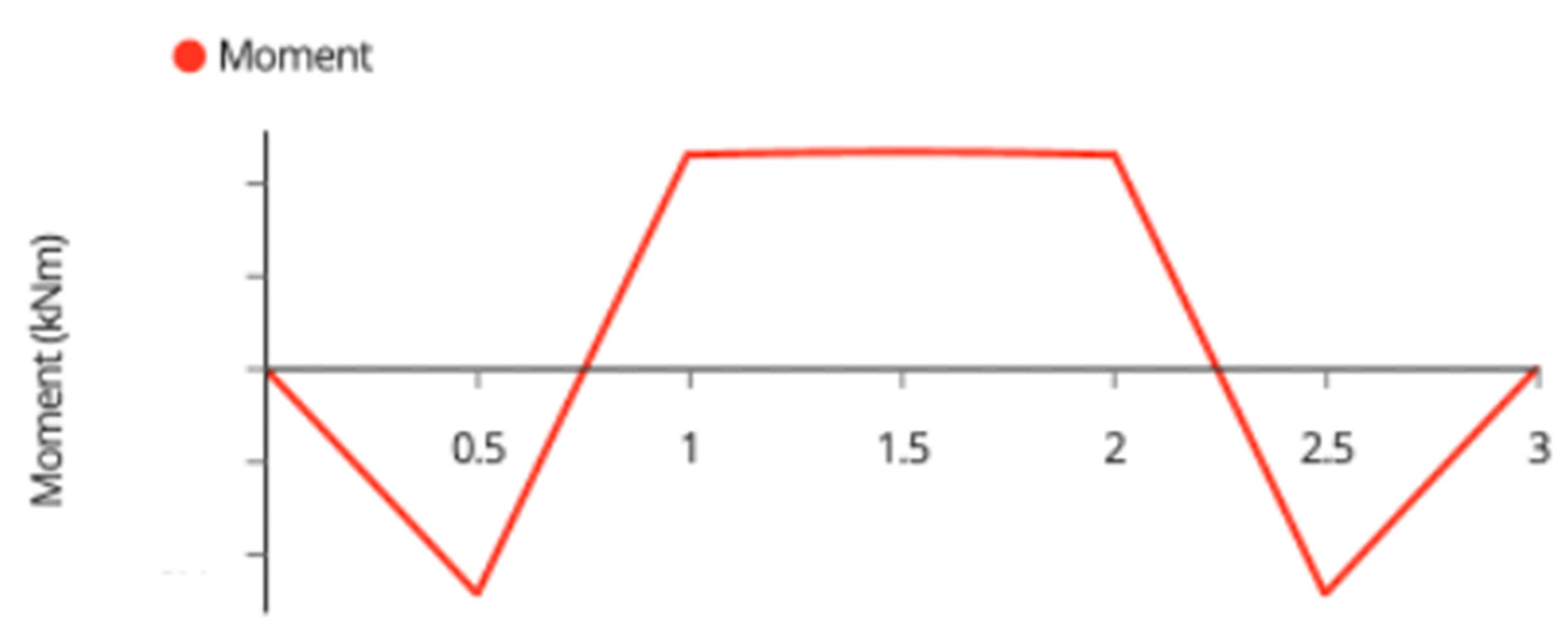
The bending moment diagram in each joist is seen as
Figure A3,
Figure A3.
Joist Bending Moment Diagram.
Figure A3.
Joist Bending Moment Diagram.
The maximum bending moment of the joists occurs at the midspan. The maximum bending moment can easily be calculated by
The deflection diagram throughout each joist is seen as
Figure A4,
Figure A4.
Joist Deflection Diagram.
Figure A4.
Joist Deflection Diagram.
The maximum deflection of the joists occurs at the midspan. For simplicity of analysis, assume the 4-point loads serve as a uniform distributed load and calculate the maximum deflection using the following equation,
The beams then carry the point loads of each joist and the factored own weight of the member. The free-body diagram is described in
Figure A5,
Figure A5.
Beam Free-Body Diagram.
Figure A5.
Beam Free-Body Diagram.
Due to the symmetric loading of the beams, the post loads, or the support reactions are described as,
The shear force diagram for the beams is described in
Figure A6,
Figure A6.
Beam Shear Force Diagram.
Figure A6.
Beam Shear Force Diagram.
The maximum shear forces occur on the inside of the reactions with a value of,
The bending moment diagram for the beam is shown in
Figure A7,
Figure A7.
Beam Bending Moment Diagram.
Figure A7.
Beam Bending Moment Diagram.
The maximum moment occurs at the supports and can be found by integrating the shear force throughout the first sixth of the beam as described in Equation (A28), or by simply finding the area under the shear force diagram.
The deflection diagram is shown in
Figure A8,
Figure A8.
Beam Deflection Diagram.
Figure A8.
Beam Deflection Diagram.
The maximum deflection occurs at midspan and can be solved using the differential equation and initial conditions below, or by using the moment area theorem or virtual work method described in many structural engineering textbooks.
The load is then transferred to the posts. It should be noted that the posts are not loaded purely in compression; an eccentricity described in the free body diagram in
Figure A9 induces a bending moment. The post can be idealized as a cantilever with a support reaction from a 2 × 4 brace.
Figure A9.
Post Free Body Diagram.
Figure A9.
Post Free Body Diagram.
The compressive load of the column is equal to the beam reaction solved above. Along with this compressive load comes a shear loading that is induced by wind and snow loads. This loading can act in either the left or right direction, but the load should be analyzed in the direction that induces bending in the same direction as the beam reaction for assessment of the critical case. The magnitude of this shear load in each post is described in Equation (A30),
where
θ is the tilt angle of the system. It should be noted that this cantilever with bracing support is an indeterminate structure, meaning that it has too many supports to be solved with static analysis, and thus can not be expressed with generalized equations. The structure can be solved by using finite element analysis, or by an analytical method such as the moment distribution or slope-deflection method.
The shear force diagram of the post is seen in
Figure A10,
Figure A10.
Post Shear Force Diagram.
Figure A10.
Post Shear Force Diagram.
The bending moment diagram of the post is seen in
Figure A11,
Figure A11.
Post-Bending Moment Diagram.
Figure A11.
Post-Bending Moment Diagram.
The deflection diagram of the post is seen in
Figure A12,
Figure A12.
Post Deflection Diagram.
Figure A12.
Post Deflection Diagram.
The 2 × 4 brace support is a slender compression member. Not only should the applied force be less than the compressive resistance, but should be less than the buckling resistance governed by the following Euler Buckling equation,
Once all components of the post have been analyzed, the load will finally transfer itself to the ground. Table 9.4.4.1 of the NBCC provides maximum allowable bearing pressures for different types of soil and rock [
56]. In the worst case, soft clays support a maximum bearing pressure of 75 kPa. To ensure, that the ground is not overloaded and settles, the bearing pressure can be calculated with the following equation,
If the applied pressure is more than the allowable, 150 mm of compacted clear stone gravel can be added to the bottom of the footing, or the footing diameter can be increased.
Throughout the system, each connection transfers the load from one member to another via a shear force within the fasteners that compose that connection. For bolts complying with ASTM A307A, the shear resistance of a ½” carriage bolt holding the beams is about 23.8 kN, and the shear resistance of a ¼” carriage bolt holding the modules is 5.21 kN [
83], both of which are beyond the demand of these systems, and thus will not be critical to the design.