Design of Distributed Multi-Actuator Systems with Incomplete State Information for Vibration Control of Large Structures †
Abstract
1. Introduction
2. Building Model
3. Controllers Design
3.1. Controllers with Full-State Information
3.2. Controllers with Partial State Information
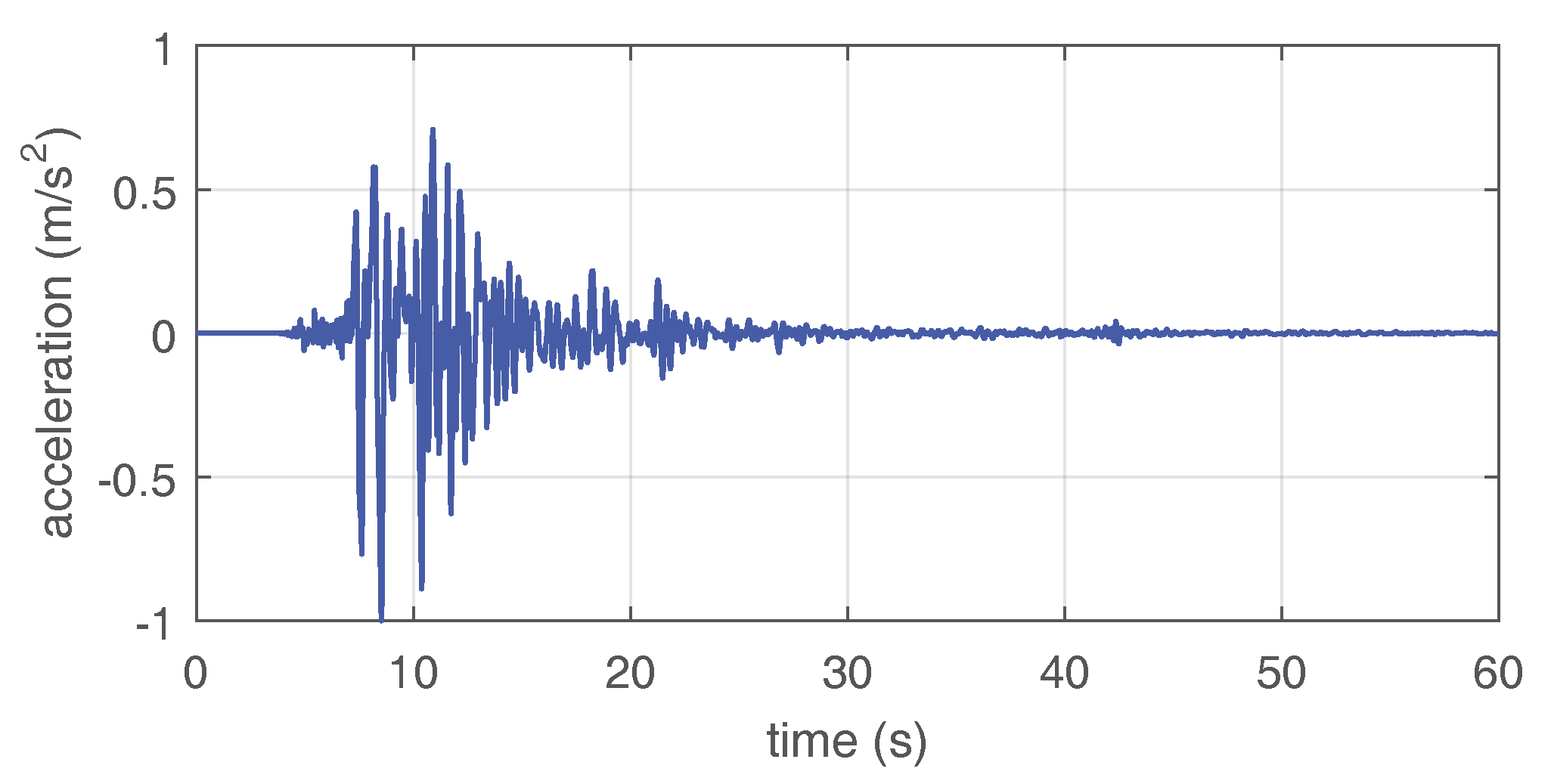
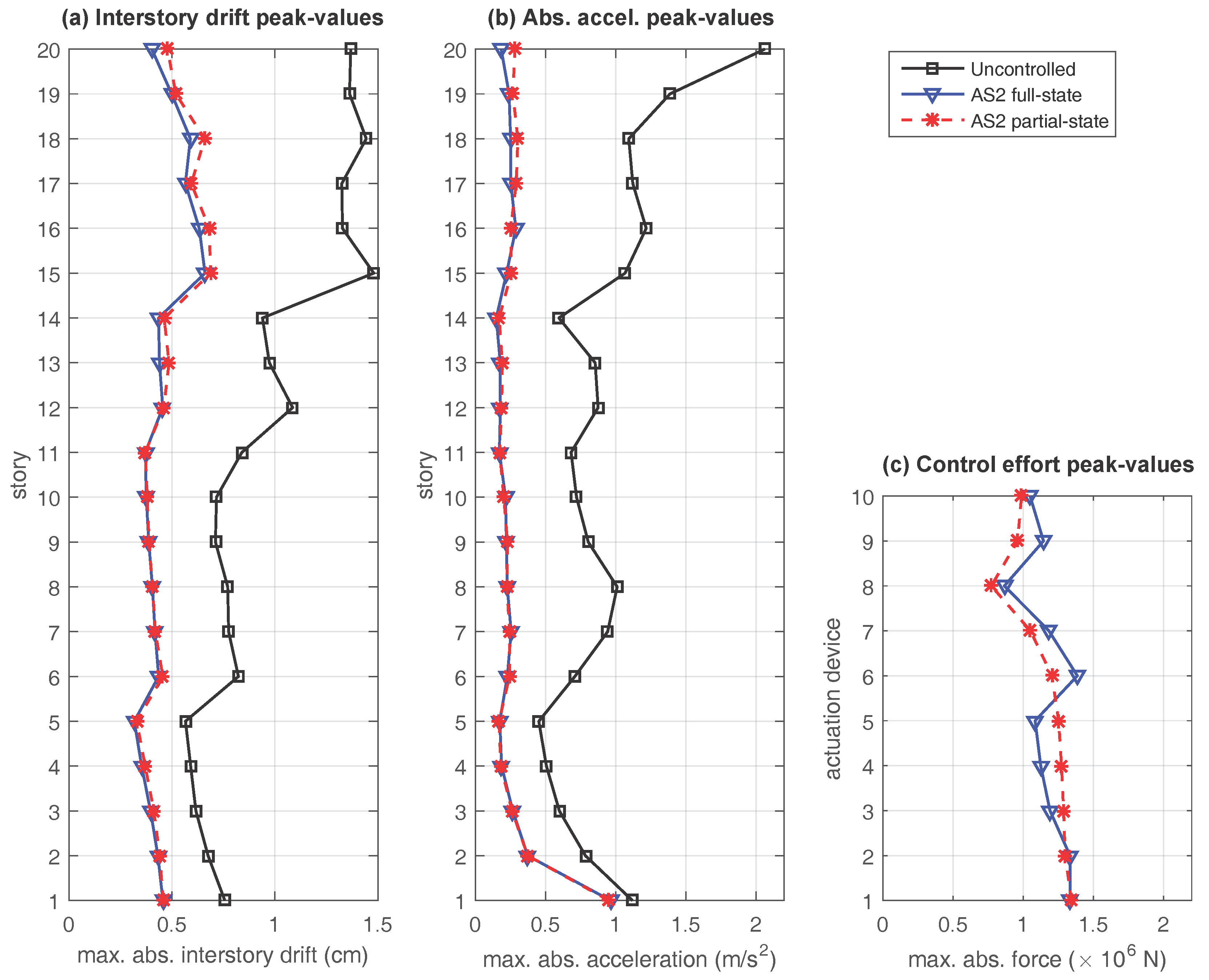
4. Numerical Results
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Spencer, B.; Nagarajaiah, S. State of the art of structural control. J. Struct. Eng. 2003, 129, 845–856. [Google Scholar] [CrossRef]
- Chu, S.; Soong, T.; Reinhorn, A. Active, Hybrid and Semi-Active Structural Control; Wiley: Hoboken, NJ, USA, 2005. [Google Scholar]
- Li, H.; Huo, L. Advances in structural control in civil engineering in China. Math. Probl. Eng. 2010, 2010, 936081. [Google Scholar]
- Thenozhi, S.; Yu, W. Advances in modeling and vibration control of building structures. Annu. Rev. Control 2013, 37, 346–364. [Google Scholar] [CrossRef]
- Basu, B.; Bursi, O.S.; Casciati, F.; Casciati, S.; Del Grosso, A.E.; Domaneschi, M.; Faravelli, L.; Holnicki-Szulc, J.; Irschik, H.; Krommer, M.; et al. A European Association for the Control of Structures joint perspective. Recent studies in civil structural control across Europe. Struct. Control Health Monit. 2014, 21, 1414–1436. [Google Scholar] [CrossRef]
- Zhan, W.; Cui, Y.; Feng, Z.; Cheung, K.C.; Lam, J.; Gao, H. Joint optimization approach to building vibration control via multiple active tuned mass dampers. Mechatronics 2013, 23, 355–368. [Google Scholar] [CrossRef]
- Yang, F.; Sedaghati, R.; Esmailzadeh, E. Optimal design of distributed tuned mass dampers for passive vibration control of structures. Struct. Control Health Monit. 2015, 22, 221–236. [Google Scholar] [CrossRef]
- Elias, S.; Matsagar, V.; Datta, T.K. Distributed multiple tuned mass dampers for wind response control of chimney with flexible foundation. Procedia Eng. 2017, 199, 1641–1646. [Google Scholar] [CrossRef]
- Xiang, P.; Nishitani, A. Seismic vibration control of building structures with multiple tuned mass damper floors integrated. Earthq. Eng. Struct. Dyn. 2014, 43, 909–925. [Google Scholar] [CrossRef]
- Sakr, T. Vibration control of buildings by using partial floor loads as multiple tuned mass dampers. HBRC J. 2017, 13, 133–144. [Google Scholar] [CrossRef]
- Wang, L.; Zhao, X.; Zheng, Y.M. A combined tuned damper and an optimal design method for wind-induced vibration control for super tall buildings. Struct. Des. Tall Spec. Build. 2016, 25, 468–502. [Google Scholar] [CrossRef]
- Nazarimofrad, E.; Zahrai, S.M. Seismic control of irregular multistory buildings using active tendons considering soil–structure interaction effect. Soil Dyn. Earthq. Eng. 2016, 89, 100–115. [Google Scholar] [CrossRef]
- He, H.; Xie, X.; Wang, W. Vibration control of tower structure with multiple cardan gyroscopes. Shock Vib. 2017, 2017, 3548360. [Google Scholar] [CrossRef]
- Wang, Y.; Lynch, J.; Law, K. Decentralized H∞ controller design for large-scale civil structures. Earthq. Eng. Struct. Dyn. 2009, 38, 377–401. [Google Scholar] [CrossRef]
- Gluck, N.; Reinhorn, A.; Gluck, J.; Levy, R. Design of supplemental dampers for control of structures. J. Struct. Eng. 1996, 122, 1394–1399. [Google Scholar] [CrossRef]
- Palacios-Quiñonero, F.; Rubió-Massegú, J.; Rossell, J.M.; Karimi, H.R. Optimal passive-damping design using a decentralized velocity-feedback H∞ approach. Model. Identif. Control 2012, 33, 87–97. [Google Scholar] [CrossRef]
- Wang, Y. Time-delayed dynamic output feedback H∞ controller design for civil structures: A decentralized approach through homotopic transformation. Struct. Control Health Monit. 2011, 18, 121–139. [Google Scholar] [CrossRef]
- Palacios-Quiñonero, F.; Rossell, J.M.; Karimi, H.R. Semi-decentralized strategies in structural vibration control. Model. Identif. Control 2011, 32, 57–77. [Google Scholar] [CrossRef]
- Lei, Y.; Wu, D.; Liu, L. A decentralized structural control algorithm with application to the benchmark control problem for seismically excited buildings. Struct. Control Health Monit. 2013, 20, 1211–1225. [Google Scholar] [CrossRef]
- Bakule, L.; Rehák, B.; Papík, M. Decentralized networked control of building structures. Comput.-Aided Civ. Infrastruct. Eng. 2016, 31, 871–886. [Google Scholar] [CrossRef]
- Rubió-Massegú, J.; Palacios-Quiñonero, F.; Rossell, J.M. Decentralized static output-feedback H∞ controller design for buildings under seismic excitation. Earthq. Eng. Struct. Dyn. 2012, 41, 1199–1205. [Google Scholar] [CrossRef]
- Chen, Y.; Zhang, W.; Gao, H. Finite frequency H∞ control for building under earthquake excitation. Mechatronics 2010, 20, 128–142. [Google Scholar] [CrossRef]
- Zhang, H.; Wang, R.; Wang, J.; Shi, Y. Robust finite frequency H∞ static-output-feedback control with application to vibration active control of structural systems. Mechatronics 2014, 24, 354–366. [Google Scholar] [CrossRef]
- Bitaraf, M.; Hurlebaus, S. Semi-active adaptive control of seismically excited 20-story nonlinear building. Eng. Struct. 2013, 56, 2107–2118. [Google Scholar] [CrossRef]
- Kazemy, A.; Zhang, X.M.; Han, Q.L. Dynamic output feedback control for seismic-excited buildings. J. Sound Vib. 2017, 411, 88–107. [Google Scholar] [CrossRef]
- Sakthivel, R.; Aravindh, D.; Selvaraj, P.; Kumar, S.; Anthoni, S. Vibration control of structural systems via robust non-fragile sampled-data control scheme. J. Frankl. Inst. 2017, 354, 1265–1284. [Google Scholar] [CrossRef]
- Rubió-Massegú, J.; Rossell, J.M.; Karimi, H.R.; Palacios-Quiñonero, F. Static output-feedback control under information structure constraints. Automatica 2013, 49, 313–316. [Google Scholar] [CrossRef]
- Palacios-Quiñonero, F.; Rubió-Massegú, J.; Rossell, J.M.; Karimi, H.R. Feasibility issues in static output-feedback controller design with application to structural vibration control. J. Frankl. Inst. 2014, 351, 139–155. [Google Scholar] [CrossRef]
- Palacios-Quiñonero, F.; Rubió-Massegú, J.; Rossell, J.M.; Karimi, H.R. Vibration control strategy for large-scale structures with incomplete multi-actuator system and neighbouring state information. IET Control Theory Appl. 2016, 10, 407–416. [Google Scholar] [CrossRef]
- Chopra, A. Dynamics of Structures. Theory and Applications to Earthquake Engineering, 3rd ed.; Prentice Hall: Upper Saddle River, NJ, USA, 2007. [Google Scholar]
- Boyd, S.; Ghaoui, L.E.; Feron, E.; Balakrishnan, V. Linear Matrix Inequalities in System and Control Theory; SIAM Studies in Applied Mathematics: Philadelphia, PA, USA, 1994. [Google Scholar]
- Cha, Y.J.; Raich, A.; Barroso, L.; Agrawal, A. Optimal placement of active control devices and sensors in frame structures using multi-objective genetic algorithms. Struct. Control Health Monit. 2013, 20, 16–44. [Google Scholar] [CrossRef]
- Sonmez, M.; Aydin, E.; Karabork, T. Using an artificial bee colony algorithm for the optimal placement of viscous dampers in planar building frames. Struct. Multidiscip. Optim. 2013, 48, 395–409. [Google Scholar] [CrossRef]
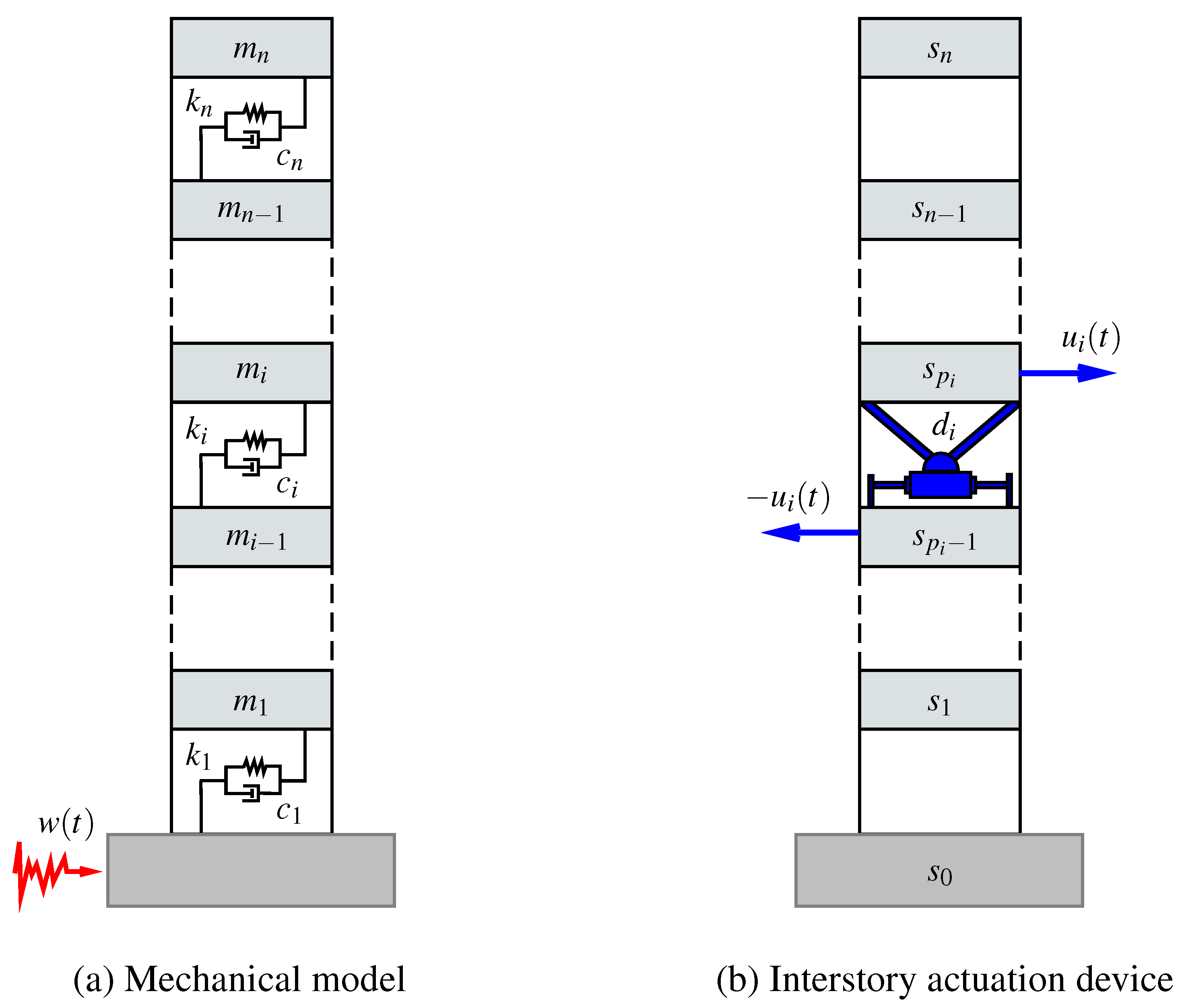
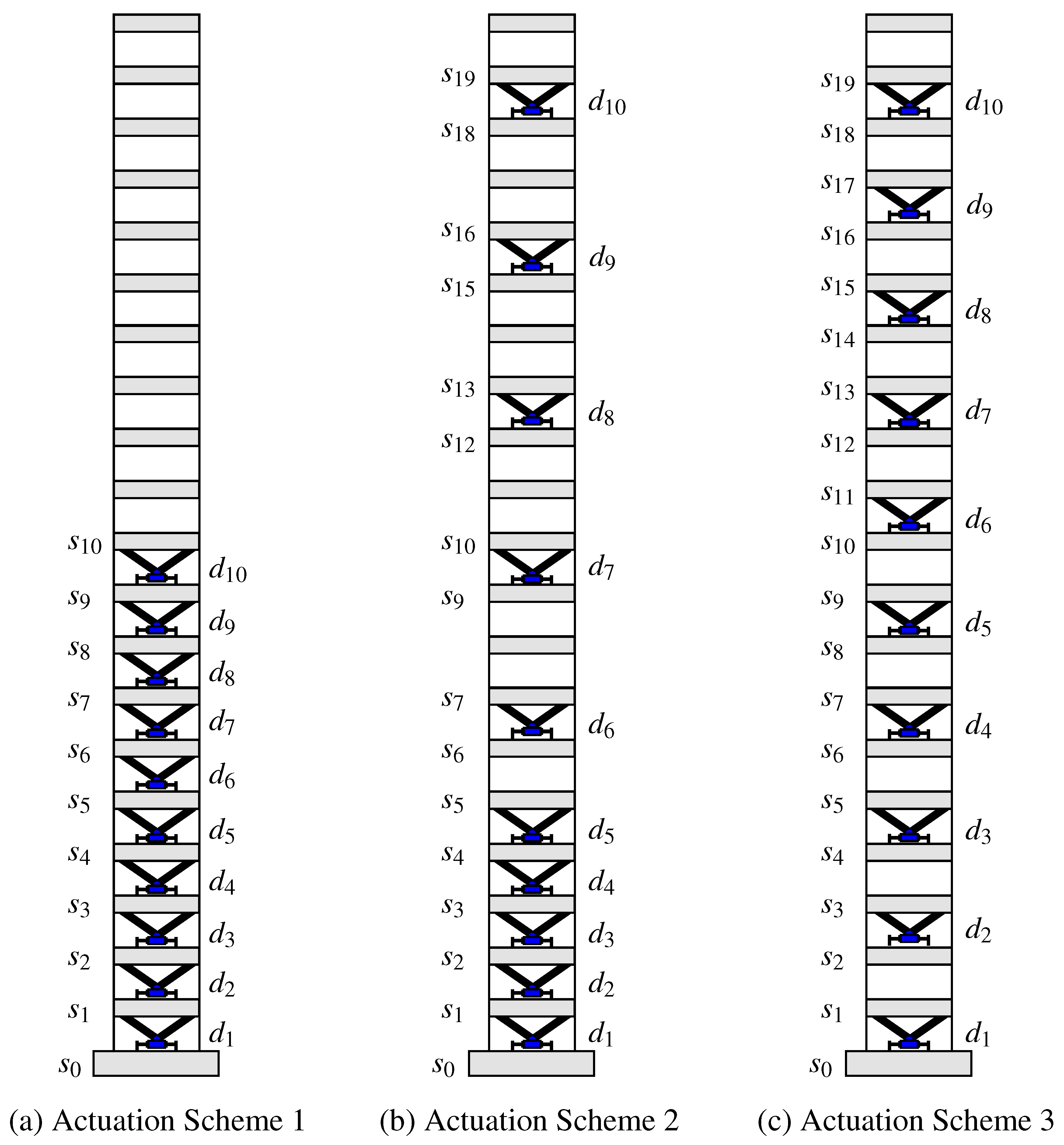

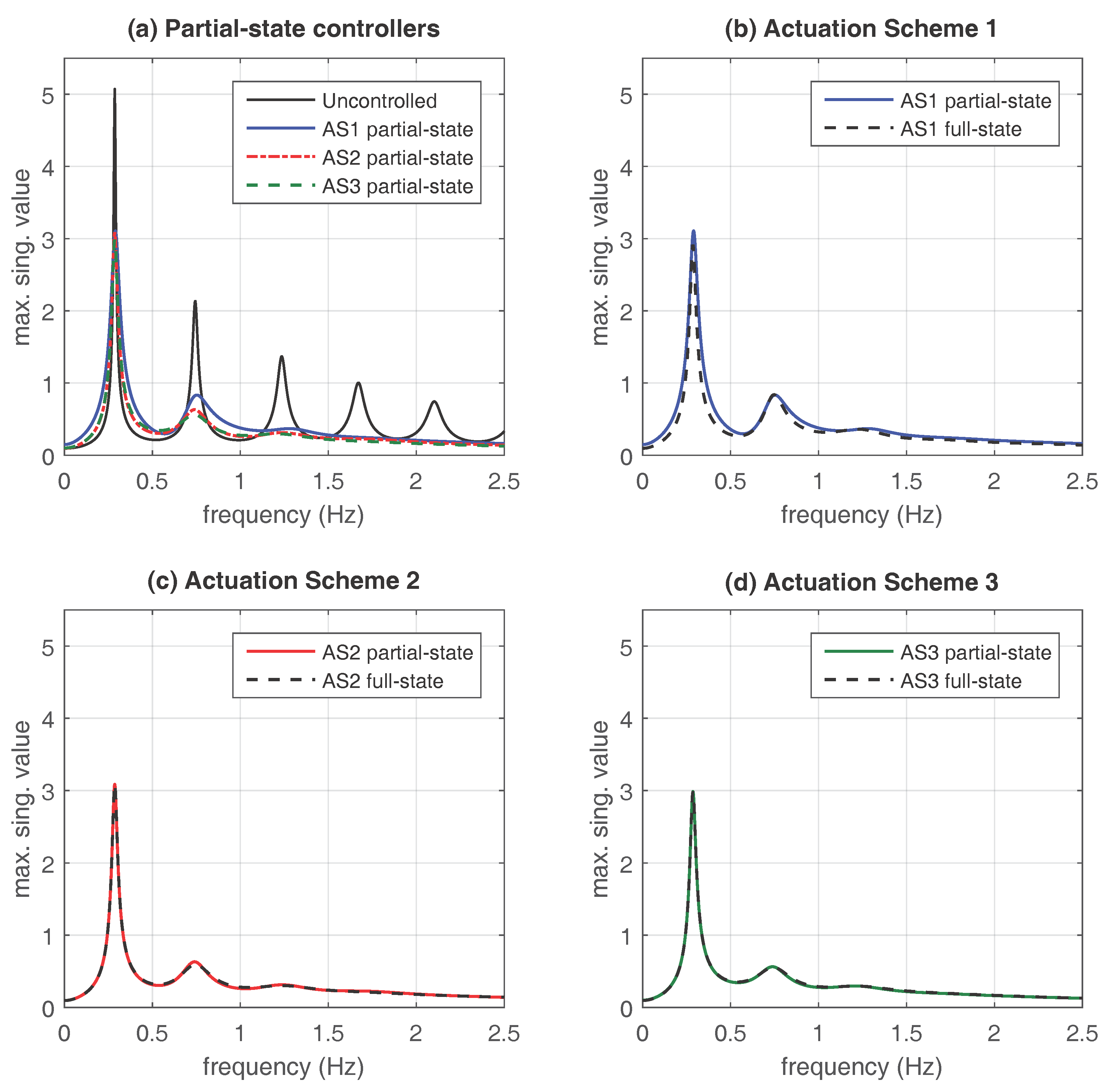


| Story | 1–5 | 6–11 | 12–14 | 15–17 | 18–19 | 20 |
|---|---|---|---|---|---|---|
| mass ( Kg) | 1.10 | 1.10 | 1.10 | 1.10 | 1.10 | 1.10 |
| stiffness (× N/m) | 8.62 | 5.54 | 4.54 | 2.91 | 2.56 | 1.72 |
| relative damping | 2% |
| Actuation Scheme | AS1 | AS2 | AS3 |
|---|---|---|---|
| Full-state controller | 42.52 | 39.18 | 41.14 |
| Partial-state controller | 38.74 | 39.18 | 41.14 |
| Story Level | 1 | 6 | 12 | 15 | 18 | 20 |
|---|---|---|---|---|---|---|
| full-state controller | 32.41 | 41.37 | 55.94 | 49.31 | 52.97 | 65.24 |
| full-state controller | 39.76 | 47.12 | 58.11 | 55.38 | 59.19 | 70.63 |
| full-state controller | 40.89 | 43.57 | 60.03 | 56.20 | 59.83 | 71.94 |
| partial-state controller | 37.77 | 47.43 | 58.88 | 51.15 | 54.89 | 64.76 |
| partial-state controller | 39.60 | 44.67 | 57.66 | 53.55 | 54.37 | 65.06 |
| partial-state controller | 40.07 | 42.15 | 58.96 | 54.89 | 58.08 | 69.78 |
| Controller | ||||||
|---|---|---|---|---|---|---|
| maximum control-effort peak-value ( N) | 1.656 | 1.385 | 1.342 | 2.132 | 1.343 | 1.273 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Palacios-Quiñonero, F.; Rubió-Massegú, J.; Rossell, J.M.; Rodellar, J. Design of Distributed Multi-Actuator Systems with Incomplete State Information for Vibration Control of Large Structures. Designs 2018, 2, 6. https://doi.org/10.3390/designs2010006
Palacios-Quiñonero F, Rubió-Massegú J, Rossell JM, Rodellar J. Design of Distributed Multi-Actuator Systems with Incomplete State Information for Vibration Control of Large Structures. Designs. 2018; 2(1):6. https://doi.org/10.3390/designs2010006
Chicago/Turabian StylePalacios-Quiñonero, Francisco, Josep Rubió-Massegú, Josep Maria Rossell, and José Rodellar. 2018. "Design of Distributed Multi-Actuator Systems with Incomplete State Information for Vibration Control of Large Structures" Designs 2, no. 1: 6. https://doi.org/10.3390/designs2010006
APA StylePalacios-Quiñonero, F., Rubió-Massegú, J., Rossell, J. M., & Rodellar, J. (2018). Design of Distributed Multi-Actuator Systems with Incomplete State Information for Vibration Control of Large Structures. Designs, 2(1), 6. https://doi.org/10.3390/designs2010006








