Adaptive Sliding Mode Control for High-Frequency Sampled-Data Systems with Actuator Faults

Abstract

This paper investigates the sliding mode control for high-frequency sampled-data systems with actuator faults. Besides matched nonlinearity, this paper also considers unmeasurable states and unknown actuator degradation ratio as important factors of the overall system. The estimates of system state vector are obtained by an adaptive sliding mode observer method firstly. Then, a novel integral-type sliding surface, corresponding to the unified closed-loop delta operator system, is provided based on aforementioned estimation values, and the fault closed-loop system is proven to be stable by the proposed sliding mode control law. Finally, the fault-tolerant control theory is verified to be valid via a practical simulation example.

1. Introduction

In practical engineering, unexpected faults of components including sensors and actuator and/or the system’s structure always occur inevitably in practice due to components burn-in, damage, physical constraint, etc. The impact of a fault or failure can lead to performance deterioration or instability of the systems, and could even cause unexpected catastrophic accidents. For example, an actuator of the vehicle system is stuck and failed to deflect the certain control state, which may result in a serious problem. Hence, developing effective fault-tolerance design techniques to accommodate sensor/actuator failures, and ensure high degree safe operation performances of the overall control systems has been an essential and significant issue in recent years [1,2,3], and some interesting results have been achieved [4,5,6,7]. In particular, adaptive control and sliding mode control methods have been applied to different systems to cope with sensor/actuator faults and unknown external disturbances (for instance, [8,9,10,11,12], and the references therein).

A great deal of attention has been paid to networked control systems (NCSs) in recent years [13,14,15,16,17], because they are able to be combined with different kinds of practical systems widely [18,19,20]. It should be noticed that, in modern industrial systems, the high frequency sampling situation always exists. Conventional discretization means derived from the model built for traditional systems failed to get the original system dynamic if the sampling time becomes more and more close to zero. However, the appearance of delta operator systems has worked out this restriction and the feature of high frequency sampling systems is able to be described accurately, the control result of using the delta operator approach is much better than applying shift operator method. For this reason, the delta operator systems have attracted abundant concern and many relevant theories such as $H_{\infty }$ control, adaptive control and sliding mode control methods have been applied on this issue.

The coexistence of sudden system structure change, high frequency data sampling [21], unknown model nonlinearity [22,23] and actuator faults in practical system makes it important to deal with the fault-tolerant control problems of the systems mentioned above, which motivates our work. In this paper, we simultaneously consider model nonlinearity and obtain the expected adaptive fault-tolerant control method for the delta operator system. First, the estimates of the state vector are derived from the proposed adaptive sliding mode observer and a special switching term is introduced to dispose the actuator faults. In addition, stability of the fault closed-loop system is guaranteed by the novel integral-type sliding mode controller we designed and the simulation result is presented in the end to prove the effectiveness of the method.

The structure of this paper is as follows. In Section 2, the existing problem is presented in detail. Section 3 and Section 4 introduce the stability criterion and develop the adaptive controller, respectively. The system trajectory is analyzed in Section 5 to illustrate its reachability and property. In Section 6, a practical problem is provided and the validity of the proposed method is proven by simulation results. Finally, the paper is concluded in Section 7.

2. Problem Statement

The delta operator owns the following form:

$$\delta x\left(k\right)=\left\{\begin{array}{cc}\frac{dx\left(k\right)}{dt},\hfill & T=0,\\ \frac{x\left(k+T\right)-x\left(k\right)}{T},\hfill & T\ne 0.\end{array}\right.$$

(1)

where T is the sampling period. In this paper, description of the following uncertain linear delta operator system is

$$\begin{array}{cc}\hfill \delta x\left(k\right)=& Ax\left(k\right)+B\left[u_{hl}^F\left(k\right)+f\left(x\left(k\right),k\right)\right]\hfill \\ \hfill y\left(k\right)=& Cx\left(k\right)\hfill \\ \hfill u_{hl}^F\left(k\right)=& {\rho }_h^l{u}_h\left(k\right)+{\eta }_h^l{\omega }_{sh}\left(k\right),\hfill \end{array}$$

(2)

where $x\left(k\right)\in R^n$ means the immeasurable system state, the signal from the hth faulted actuator in the lth faulty mode is presented by $u_{hl}^F\left(k\right)$, $h\in \left\{1,2,\dots ,m\right\}$, $l\in \left\{1,2,\dots ,L\right\}$, the total number of faulty modes is L. $y\left(k\right)\in R^p$ is the measurable output, $f\left(x\left(k\right),k\right)\in R^m$ stands for the unknown sensor output. $A\in {\mathbb{R}}^{n\times n}$, $B\in {\mathbb{R}}^{n\times m}$ and $C\in {\mathbb{R}}^{n\times p}$ are system matrices with appropriate dimensions. ${\rho }_h^l$ means the unknown actuator efficiency factor which consist the diagonal matrix ${\rho }^l=diag\left\{{\rho }_1^l,{\rho }_2^l,\dots ,{\rho }_m^l\right\}$, definition of the unknown constant ${\eta }_h^l$ is

$${\eta }_h^l=\left\{\begin{array}{cc}0,\hfill & {\rho }_h^l>0,\hfill \\ 0\mathrm{or}1,\hfill & {\rho }_h^l=0.\hfill \end{array}\right.$$

(3)

Define ${\eta }^l=diag\left\{{\eta }_1^l,{\eta }_2^l,\dots {\eta }_m^l\right\}$; if ${\rho }_h^l=0$ and ${\eta }_h^l=0$, it means that the hth actuator is outage in the lth fault mode, ${\rho }_h^l=0$ and ${\eta }_h^l=1$ stand for the fault-stuck problem on the hth actuator in the lth fault mode, $0<{\rho }_h^l<1$ means that effectiveness of the hth actuator is damaged in the lth fault mode, ${\rho }_h^l=1$ is the no fault state symbol of the hth actuator in the lth fault mode. ${\omega }_{sh}\left(k\right)$ is the unknown time-varying bounded fault-stuck in the hth actuator. For simplicity, in the following, the model is exploited by:

$$u^F\left(k\right)=\rho u\left(k\right)+\eta {\omega }_s\left(k\right),$$

(4)

where $\rho =diag\left\{{\rho }_1,\dots ,{\rho }_m\right\}\in \left\{{\rho }^1,\dots ,{\rho }^L\right\}$.

The following assumptions are made in this paper.

(A1)

$∥f\left(x\left(k\right),k\right)∥\le \alpha ∥y\left(k\right)∥+\beta$, where $\alpha >0$, $\beta >0$ are known constants.

(A2)

The actuator fault redundancy condition is that $rank\left(B\right)=rank\left(\rho B\right)$.

(A3)

The unknown time-varying bounded fault-deviation vector ${\omega }_s\left(k\right)\stackrel{\Delta }{=}{\left[{\omega }_{s1}\left(k\right)\dots {\omega }_{sm}\left(k\right)\right]}^T$ satisfies:

$${\omega }_{bs}\left(k\right)\le {\omega }_s\left(k\right)\le {\omega }_{ts}\left(k\right),h=1,\dots ,m,$$

(5)

where ${\omega }_{ts}\left(k\right)$ and ${\omega }_{bs}\left(k\right)$ stand for the unknown upper and lower bounds of ${\omega }_s$, respectively, and we denote

$${\omega }_{bs}\left(k\right)\stackrel{\Delta }{=}{\left[{\omega }_{bs1}\left(k\right)\dots {\omega }_{bsm}\left(k\right)\right]}^T,{\omega }_{ts}\left(k\right)\stackrel{\Delta }{=}{\left[{\omega }_{ts1}\left(k\right)\dots {\omega }_{tsm}\left(k\right)\right]}^T.$$

(6)

Lemma 1.

[24] Considering known matrices $G\in R^{n\times n}$ and $U\in R^{n\times m}$, assume that U has full column rank $m<n$ and $G=G^T$. Then, $\alpha U^{T}U-G>0$ holds for a scalar α if and only if ${\tilde{U}}^{T}G\tilde{U}<0$ where $\tilde{U}$ is any matrix whose columns are able to build the null space basis of $U^T$.

The design of observer for the system in Equation (2) is as follows:

$$\begin{array}{cc}\hfill \delta \widehat{x}\left(k\right)=& A\widehat{x}\left(k\right)+B\widehat{\rho }u\left(k\right)+B{u}_s\left(k\right)+L\left(y\left(k\right)\right.\hfill \\ & -\left.C\widehat{x}\left(k\right)\right)+B\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{d}}_{ts}\left(k\right)+\tau {\widehat{d}}_{bs}\left(k\right)\right]\hfill \\ \hfill \widehat{y}\left(k\right)=& C\widehat{x}\left(k\right),\hfill \end{array}$$

(7)

where $\widehat{x}\left(k\right)$ means the estimation of $x\left(k\right)$, L is the observer gain to be designed, and $u_s\in R^m$ stands for the discontinuous term. $\widehat{\rho }=diag\left\{{\widehat{\rho }}_1,{\widehat{\rho }}_2,\dots ,{\widehat{\rho }}_m\right\}$ is the estimation of the actuator efficiency factor. ${\widehat{d}}_{ts}$ and ${\widehat{d}}_{bs}$ are estimations of ${\omega }_{ts}$ and ${\omega }_{bs}$, $\tau =diag\left\{{\tau }_1,\dots ,{\tau }_m\right\}$,

$${\tau }_h=\left\{\begin{array}{c}0,x^T\left(k\right)P{b}_h\ge 0,\\ 1,x^T\left(k\right)P{b}_h<0,\end{array}\right.$$

(8)

$b_h$ means the hth column of B, $P\in R^{n\times n}$ is the Lyapunov matrix to be designed. The definition of sliding surface $s_e\left(k\right)\in R^m$ is as follows:

$$s_e\left(k\right)=B^{T}Pe\left(k\right),$$

(9)

Define the error $e\left(k\right)=\widehat{x}\left(k\right)-x\left(k\right)$; the error dynamic can be obtained as follows:

$$\begin{array}{ccc}\hfill \delta e\left(k\right)& \hfill =& Ae\left(k\right)+B\left[u_s\left(k\right)+\left(\widehat{\rho }-\rho \right)u\left(k\right)-f\left(x\left(k\right),k\right)\right]-LCe\left(k\right)-B\eta {\omega }_s\left(k\right)\hfill \\ & & +B\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{d}}_{ts}\left(k\right)+\tau {\widehat{d}}_{bs}\left(k\right)\right]\hfill \\ & \hfill =& \left(A-LC\right)e_k+B\left(u_s\left(k\right)+\left(\widehat{\rho }-\rho \right)u\left(k\right)-f\left(x_k,k\right)\right)-B\eta {\omega }_s\left(k\right)\hfill \\ & & +B\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{d}}_{ts}\left(k\right)+\tau {\widehat{d}}_{bs}\left(k\right)\right].\hfill \end{array}$$

(10)

Let $B^{T}P=NC$, then rewrite the sliding surface in Equation (9) as:

$$s_e\left(k\right)=NCe\left(k\right).$$

(11)

Using the following coordinate transformation

$$z\left(k\right)=\left[\begin{array}{c}{z}_1\left(k\right)\\ z_2\left(k\right)\end{array}\right]=We\left(k\right), W=\left[\begin{array}{c}{\left({\tilde{B}}^T{P}^{-1}\tilde{B}\right)}^{-1}{\tilde{B}}^T\\ {\left(B^{T}PB\right)}^{-1}{B}^{T}P\end{array}\right]$$

(12)

with $z_1\left(k\right)\in R^{n-m}$ and $z_2\left(k\right)\in R^m$, $\tilde{B}$ is any basis of the null space of $B^T$, $\tilde{B}\in \left\{J:B^T=0\right\}$. Then, it can be obtained that

$$s_e\left(k\right)=B^{T}PB{z}_2\left(k\right), W^{-1}=\left[\begin{array}{cc}{P}^{-1}\tilde{B}& B\end{array}\right].$$

(13)

In this situation, we can obtain the reduced-order sliding mode dynamics for the sliding surface $s_e\left(z\left(k\right)\right)=0$

$$\delta z_1\left(k\right)={\left({\tilde{B}}^T{P}^{-1}\tilde{B}\right)}^{-1}{\tilde{B}}^T\left(A-LC\right)P^{-1}\tilde{B}{z}_1\left(k\right).$$

(14)

Then, we analyze the stochastic stability of the sliding mode Equation (14).

3. Stability Analysis of the Sliding Motion Equation

In this section, the reduced-order sliding mode dynamics will be analyzed to guarantee the stochastic stability of the overall system.

Theorem 1.

The reduced-order sliding mode dynamics in Equation (14) is stochastic stable in delta domain, if there exists a matrix $P>0$ with appropriate dimension, and scalars ${\alpha }_1>0$ such that the following LMI holds

$$\left[\begin{array}{cccc}\psi & A^{T}P-C^T{Y}^T& PB& 0\\ PA-YC& \left(T-2\right)P& 0& PB\\ B^{T}P& 0& -{\alpha }_1^{-1}I& 0\\ 0& B^{T}P& 0& -{\alpha }_1^{-1}I\end{array}\right]<0,$$

(15)

with

$$\psi =A^{T}P+PA-C^T{Y}^T-YC.$$

(16)

Proof. 

In delta domain, define a Lyapunov functional as follows:

$$V_1\left(k\right)=z_1^T\left(k\right)\left({\tilde{B}}^T{P}^{-1}\tilde{B}\right)z_1\left(k\right).$$

(17)

Based on Lemma 1, and taking the delta operator manipulations of $V_1\left(k\right)$ along the trajectory of delta operator system, we can obtain:

$$\begin{array}{ccc}\hfill \delta V_1\left(k\right)& \hfill =& {\delta }^T\left(z_1\left(k\right)\right)\left({\tilde{B}}^T{P}^{-1}\tilde{B}\right)z_1\left(k\right)+z_1^T\left(k\right)\left({\tilde{B}}^T{P}^{-1}\tilde{B}\right)\delta \left(z_1\left(k\right)\right)\hfill \\ & & +T{\delta }^T\left(z_1\left(k\right)\right)\left({\tilde{B}}^T{P}^{-1}\tilde{B}\right)\delta \left(z_1\left(k\right)\right)\hfill \\ & \hfill =& z_1^T\left(k\right){\tilde{B}}^T{P}^{-1}{\left(A-LC\right)}^T\tilde{B}{z}_1\left(k\right)+z_1^T\left(k\right){\tilde{B}}^T\left(A-LC\right)P^{-1}\tilde{B}{z}_1\left(k\right)\hfill \\ & & +T{\delta }^T\left(z_1\left(k\right)\right)\left({\tilde{B}}^T{P}^{-1}\tilde{B}\right)\delta \left(z_1\left(k\right)\right).\hfill \end{array}$$

(18)

Thinking about the certain zero term

$$\begin{array}{cc}\hfill 0=& -2{\delta }^T\left(z_1\left(k\right)\right)\left({\tilde{B}}^T{P}^{-1}\tilde{B}\right)\delta \left(z_1\left(k\right)\right)+{\delta }^T\left(z_1\left(k\right)\right){\tilde{B}}^T\left(A-LC\right)P^{-1}\tilde{B}{z}_1\left(k\right)\hfill \\ & +z_1^T\left(k\right){\tilde{B}}^T{P}^{-1}{\left(A-LC\right)}^T\tilde{B}\delta \left(z_1\left(k\right)\right).\hfill \end{array}$$

(19)

Then, it can be obtained that

$$\delta V_1\left(k\right)\le \left[\begin{array}{cc}{z}_1^T\left(k\right)& {\delta }^T\left(z_1\left(k\right)\right)\end{array}\right]\Gamma {\left[\begin{array}{cc}{z}_1^T\left(k\right)& {\delta }^T\left(z_1\left(k\right)\right)\end{array}\right]}^T$$

(20)

holds if

$$\Gamma =\left[\begin{array}{cc}{\psi }_1& {\tilde{B}}^T{P}^{-1}{\left(A-LC\right)}^T\tilde{B}\\ {\tilde{B}}^T\left(A-LC\right)P^{-1}\tilde{B}& \left(T-2\right){\tilde{B}}^T{P}^{-1}\tilde{B}\end{array}\right]<0$$

(21)

with

$${\psi }_1={\tilde{B}}^T{P}^{-1}{\left(A-LC\right)}^T\tilde{B}+{\tilde{B}}^T\left(A-LC\right)P^{-1}\tilde{B}.$$

(22)

which is equivalent to

$$\left[\begin{array}{cc}{\tilde{B}}^T& 0\\ 0& {\tilde{B}}^T\end{array}\right]\left[\begin{array}{cc}{\psi }_2& P^{-1}{\left(A-LC\right)}^T\\ \left(A-LC\right)P^{-1}& \left(T-2\right)P^{-1}\end{array}\right]\left[\begin{array}{cc}\tilde{B}& 0\\ 0& \tilde{B}\end{array}\right]<0,$$

(23)

$${\psi }_2=P^{-1}{\left(A-LC\right)}^T+\left(A-LC\right)P^{-1}.$$

(24)

It is not difficult to derive from Lemma 2 that $\Gamma <0$ is solvable for $P>0$ and ${\alpha }_1>0$ if and only if the following holds

$$\left[\begin{array}{cc}{\psi }_2-{\alpha }_{1}B{B}^T& P^{-1}{\left(A-LC\right)}^T\\ \left(A-LC\right)P^{-1}& \left(T-2\right)P^{-1}-{\alpha }_{1}B{B}^T\end{array}\right]<0,$$

(25)

the formulation is equivalent to

$$\left[\begin{array}{cc}{\psi }_2-{\alpha }_{1}B{B}^T& P^{-1}\left(A^T-C^T{L}^T\right)\\ A{P}^{-1}-LC{P}^{-1}& \left(T-2\right)P^{-1}-{\alpha }_{1}B{B}^T\end{array}\right]<0.$$

(26)

Pre- and post-multiplying (26) by $\left[\begin{array}{cc}P& 0\\ 0& P\end{array}\right]$ and its transpose, we have

$$\left[\begin{array}{cc}{\psi }_3-P{\alpha }_{1}B{B}^{T}P& \left(A^T-C^T{L}^T\right)P\\ P\left(A-LC\right)& \left(T-2\right)P-{\alpha }_{1}PB{B}^{T}P\end{array}\right]<0,$$

(27)

$${\psi }_3=\left(A^T-C^T{L}^T\right)P+P\left(A-LC\right),$$

(28)

where $Y=PL$, $Y^T=L^{T}P$, then Equation (28) can be rewritten as:

$$\left[\begin{array}{cc}{\psi }_4-P{\alpha }_{1}B{B}^{T}P& A^{T}P-C^T{Y}^T\\ PA-YC& \left(T-2\right)P-{\alpha }_{1}PB{B}^{T}P\end{array}\right]<0,$$

(29)

$${\psi }_4=A^{T}P+PA-C^T{Y}^T-YC.$$

(30)

Equation (30) can be rewritten as

$$\left[\begin{array}{cc}{\psi }_4& A^{T}P-C^T{Y}^T\\ PA-YC& \left(T-2\right)P\end{array}\right]-\left[\begin{array}{cc}P{\alpha }_{1}B{B}^{T}P& 0\\ 0& {\alpha }_{1}PB{B}^{T}P\end{array}\right]<0,$$

(31)

where the second term is equal to $\left[\begin{array}{cc}PB& 0\\ 0& PB\end{array}\right]\left[\begin{array}{cc}{\alpha }_{1}I& 0\\ 0& {\alpha }_{1}I\end{array}\right]\left[\begin{array}{cc}{B}^{T}P& 0\\ 0& B^{T}P\end{array}\right]$. If the following LMI holds, LMI (Equation (31)) can be solved

$$\left[\begin{array}{cc}{\psi }_4& A^{T}P-C^T{Y}^T\\ PA-YC& \left(T-2\right)P\end{array}\right]+\phi <0,$$

(32)

$$\phi =\left[\begin{array}{cc}PB& 0\\ 0& PB\end{array}\right]\left[\begin{array}{cc}{\alpha }_{1}I& 0\\ 0& {\alpha }_{1}I\end{array}\right]\left[\begin{array}{cc}{B}^{T}P& 0\\ 0& B^{T}P\end{array}\right].$$

(33)

According to the Schur complement, it is easy to obtain that Equation (33) is equivalent to

$$\left[\begin{array}{cccc}{\psi }_4& A^{T}P-C^T{Y}^T& PB& 0\\ PA-YC& \left(T-2\right)P& 0& PB\\ B^{T}P& 0& -{\alpha }_1^{-1}I& 0\\ 0& B^{T}P& 0& -{\alpha }_1^{-1}I\end{array}\right]<0,$$

(34)

$${\psi }_4=A^{T}P+PA-C^T{Y}^T-YC.$$

(35)

It can be seen that the reduced-order sliding mode dynamics in Equation (14) is stochastic stable in delta domain if Equation (15) holds. Thus, the proof is completed. ☐

4. Stability Analysis of the Error Dynamic

This section will focus on designing the discontinuous term $u\left(k\right)$ to guarantee the stochastic stability of the error system in Equation (10). $u_s\left(k\right)$ can be designed as

$$u_s\left(k\right)=-\left(\alpha ∥y\left(k\right)∥+\beta +\gamma \right)\mathrm{sgn}\left(s_e\left(k\right)\right)$$

(36)

with the sliding surface in Equation (9). Moreover, the adaption laws are given as follows:

$$\begin{array}{cc}\hfill \delta {\widehat{\rho }}_h\left(k\right)& =-c_h{s}_{eh}\left(k\right)u_h\left(k\right),\hfill \\ \hfill \delta {\widehat{\eta }}_h\left(k\right)& =g_{h1}{x}^T\left(k\right)P{b}_h\left[\left(I-{\tau }_h\right){\widehat{\omega }}_{ts}\left(k\right)+{\tau }_h{\widehat{\omega }}_{bs}\left(k\right)\right],\hfill \\ \hfill \delta {\widehat{\omega }}_{ts}\left(k\right)& =\delta {\widehat{\omega }}_{bs}\left(k\right)=-g_{h2}{x}^T\left(k\right)P{b}_h.\hfill \end{array}$$

(37)

Theorem 2.

With the sliding mode controller $u_s\left(k\right)$, the error system in Equation (10) is stochastic stable if the following matrix constraint is satisfied:

$$P\left(A-LC\right)+{\left(A-LC\right)}^{T}P<0.$$

(38)

Proof. 

Define Lyapunov function $V\left(k\right)=V_2\left(k\right)+V_3\left(k\right)$ and the error variable

$$\tilde{\rho }=\widehat{\rho }-\rho ,\tilde{\eta }=\widehat{\eta }-\eta ,{\tilde{d}}_{ts}={\widehat{\omega }}_{ts}-{\omega }_{ts},{\tilde{d}}_{bs}={\widehat{\omega }}_{bs}-{\omega }_{bs},$$

$$V_2\left(k\right)=e^T\left(k\right)Pe\left(k\right),$$

$$V_3\left(k\right)=\sum _{h=1}^m\frac{{\tilde{\rho }}_h^2}{c_h}+\sum _{h=1}^m\frac{{\tilde{\eta }}_h^2}{g_{h1}}+\sum _{h=1}^m\frac{{\eta }_h\left(1-{\tau }_h\right){\tilde{\omega }}_{ts}^2}{g_{h2}}+\sum _{h=1}^m\frac{{\eta }_h{\tau }_h{\tilde{\omega }}_{bs}^2}{g_{h2}}.$$

Taking the stochastic delta operator manipulations of along the trajectory of system, we obtain:

$$\begin{array}{ccc}\hfill \delta V_2\left(k\right)& \hfill =& \frac{E\left\{V\left(k+1\right)\right\}-V\left(k\right)}{T}\hfill \\ & \hfill =& T^2{\delta }^T\left(e\left(k\right)\right)P\delta \left(e\left(k\right)\right)+T{\delta }^T\left(e\left(k\right)\right)Pe\left(k\right)+T{e}^T\left(k\right)P\delta \left(e\left(k\right)\right)\hfill \\ & & +T{\delta }^T\left(e\left(k\right)\right)P\delta \left(e\left(k\right)\right)+{\delta }^T\left(e\left(k\right)\right)Pe\left(k\right)+e^T\left(k\right)P\delta \left(e\left(k\right)\right)\hfill \\ & \hfill =& 2e^T\left(k\right)P\delta e\left(k\right)+2T{e}^T\left(k\right)P\delta e\left(k\right)+T{\delta }^T\left(e\left(k\right)\right)P\delta \left(e\left(k\right)\right)\hfill \\ & & +T^2{\delta }^T\left(e\left(k\right)\right)P\delta \left(e\left(k\right)\right)\hfill \\ & \hfill =& 2e^T\left(k\right)P\left[\left(A-LC\right)e\left(k\right)+B\left(u_s\left(k\right)+\tilde{\rho }u\left(k\right)-f\left(x\left(k\right),k\right)\right)\right]-2e^T\left(k\right)PB\eta {\omega }_s\left(k\right)\hfill \\ & & +T^2{\delta }^T\left(e\left(k\right)\right)P\delta \left(e\left(k\right)\right)+T{\delta }^T\left(e\left(k\right)\right)P\delta \left(e\left(k\right)\right)+2T{e}^T\left(k\right)P\delta e\left(k\right)\hfill \\ & & +2e^T\left(k\right)PB\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{\omega }}_{ts}\left(k\right)+\tau {\widehat{\omega }}_{bs}\left(k\right)\right]\hfill \\ & \hfill =& 2e^T\left(k\right)P\left(A-LC\right)e\left(k\right)+2e^T\left(k\right)PB{u}_s\left(k\right)+2e^T\left(k\right)PB\left[\tilde{\rho }u\left(k\right)-f\left(x\left(k\right),k\right)\right]\hfill \\ & & +2e^T\left(k\right)PB\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{\omega }}_{ts}\left(k\right)+\tau {\widehat{\omega }}_{bs}\left(k\right)\right]-2e^T\left(k\right)PB\eta {\omega }_s\left(k\right)\hfill \\ & & +2T{e}^T\left(k\right)P\delta e\left(k\right)+T^2{\delta }^T\left(e\left(k\right)\right)P\delta \left(e\left(k\right)\right)+T{\delta }^T\left(e\left(k\right)\right)P\delta \left(e\left(k\right)\right).\hfill \end{array}$$

(39)

Recalling $B^{T}P=NC$, Equation (39) becomes

$$\begin{array}{cc}\hfill \delta V_2\left(k\right)=& 2e^T\left(k\right)P\left(A-LC\right)e\left(k\right)+2e^T\left(k\right)C^T{N}^T{u}_s\left(k\right)+2e^T\left(k\right)PB\left[\tilde{\rho }u\left(k\right)-f\left(x\left(k\right),k\right)\right]\hfill \\ & +2e^T\left(k\right)PB\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{\omega }}_{ts}\left(k\right)+\tau {\widehat{\omega }}_{bs}\left(k\right)\right]-2e^T\left(k\right)PB\eta {\omega }_s\left(k\right)\hfill \\ & +2T{e}^T\left(k\right)P\delta e\left(k\right)+T^2{\delta }^T\left(e\left(k\right)\right)P\delta \left(e\left(k\right)\right)+T{\delta }^T\left(e\left(k\right)\right)P\delta \left(e\left(k\right)\right).\hfill \end{array}$$

(40)

In Equation (40) the term $2e^T\left(k\right)PB\left[\tilde{\rho }u\left(k\right)-f\left(x\left(k\right),k\right)\right]$ can be amplified as:

$$\begin{array}{cc}\hfill 2e^T\left(k\right)PB\left[\tilde{\rho }u\left(k\right)-f\left(x\left(k\right),k\right)\right]& =2e^T\left(k\right)PB\tilde{\rho }u\left(k\right)-2e^T\left(k\right)PBf\left(x\left(k\right),k\right)\hfill \\ & \le 2e^T\left(k\right)PB\tilde{\rho }u\left(k\right)+2∥e^T\left(k\right)PB∥∥f\left(x\left(k\right),k\right)∥\hfill \\ & \le 2s_e^T\left(k\right)\tilde{\rho }u\left(k\right)+2∥s_e\left(k\right)∥\left(\alpha ∥y\left(k\right)∥+\beta \right).\hfill \end{array}$$

(41)

Then, the expression of $\delta V_2\left(k\right)$ will become:

$$\begin{array}{cc}\hfill \delta V_2\left(k\right)\le & 2e^T\left(k\right)P\left(A-LC\right)e\left(k\right)+2s_e^T\left(k\right)u_s\left(k\right)+2e^T\left(k\right)PB\tilde{\rho }u\left(k\right)+2∥s_e\left(k\right)∥\left(\alpha ∥y_k∥\right.\hfill \\ & \left.+\beta \right)+2T{e}^T\left(k\right)P\delta e\left(k\right)+T^2{\delta }^T\left(e\left(k\right)\right)P\delta \left(e\left(k\right)\right)+T{\delta }^T\left(e\left(k\right)\right)P\delta \left(e\left(k\right)\right)\hfill \\ & +2e^T\left(k\right)PB\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{\omega }}_{ts}\left(k\right)+\tau {\widehat{\omega }}_{bs}\left(k\right)\right]-2e^T\left(k\right)PB\eta {\omega }_s\left(k\right).\hfill \end{array}$$

(42)

Substituting sliding mode controller into Equation (42), it can be obtained that:

$$\begin{array}{cc}\hfill 2s_e^T\left(k\right)u_s\left(k\right)& =-2s_e^T\left(k\right)\left(\alpha ∥y\left(k\right)∥+\beta +\gamma \right)\mathrm{sgn}\left(s_e\left(k\right)\right)\hfill \\ & =-2s_e^T\left(k\right)\left(\alpha ∥y\left(k\right)∥+\beta +\gamma \right)s_e^T\left(k\right)\mathrm{sgn}\left(s_e\left(k\right)\right)\hfill \\ & =-2\left(\alpha ∥y\left(k\right)∥+\beta +\gamma \right){\left|s_e\left(k\right)\right|}_1\hfill \\ & \le -2\left(\alpha ∥y\left(k\right)∥+\beta +\gamma \right)∥s_e\left(k\right)∥.\hfill \end{array}$$

(43)

Hence, we can see that

$$\begin{array}{cc}\hfill \delta V_2\left(k\right)\le & 2e^T\left(k\right)P\left(A-LC\right)e\left(k\right)+2s_e^T\left(k\right){\tilde{\rho }}_{k}u\left(k\right)+2e^T\left(k\right)PB\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{\omega }}_{ts}\left(k\right)+\tau {\widehat{\omega }}_{bs}\left(k\right)\right]\hfill \\ & -2e^T\left(k\right)PB\eta {\omega }_s\left(k\right)+T^2{\delta }^T\left(e\left(k\right)\right)P\delta \left(e\left(k\right)\right)+T{\delta }^T\left(e\left(k\right)\right)P\delta \left(e\left(k\right)\right)\hfill \\ & +2T{e}^T\left(k\right)P\delta e\left(k\right)+e^T\left(k\right)Pe\left(k\right)-\gamma {∥s_e\left(k\right)∥}_2.\hfill \end{array}$$

(44)

For the Lyapunov function $V_3\left(k\right)$, it can be derived that the $\delta V_3\left(k\right)$ can described as:

$$\begin{array}{cc}\hfill \delta V_3\left(k\right)=& \sum _{h=1}^m\frac{2}{c_h}{\tilde{\rho }}_h\delta {\widehat{\rho }}_h+T\sum _{h=1}^m\frac{1}{c_h}{\delta }^2{\widehat{\rho }}_h+\sum _{h=1}^m\frac{2}{g_{h1}}{\tilde{\eta }}_h\delta {\tilde{\eta }}_h+T\sum _{h=1}^m\frac{1}{g_{h1}}{\delta }^2{\tilde{\eta }}_h+\sum _{h=1}^m\frac{2}{g_{h2}}{\eta }_h\left(1-{\tau }_h\right){\tilde{d}}_{ts}\delta {\tilde{d}}_{ts}\hfill \\ & +T\sum _{h=1}^m\frac{1}{g_{h2}}{\eta }_h\left(1-{\tau }_h\right){\delta }^2{\tilde{d}}_{ts}+\sum _{h=1}^m\frac{2}{g_{h2}}{\eta }_h{\tau }_h{\tilde{d}}_{bs}\delta {\tilde{d}}_{bs}+T\sum _{h=1}^m\frac{1}{g_{h2}}{\eta }_h{\tau }_h{\delta }^2{\tilde{d}}_{bs},\hfill \end{array}$$

(45)

then, we can rewrite the expression of $\delta \left(V\left(k\right)\right)$ as:

$$\begin{array}{cc}\hfill \delta \left(V\left(k\right)\right)\le & 2e^T\left(k\right)P\left(A-LC\right)e\left(k\right)+2s_e^T\left(k\right){\tilde{\rho }}_{k}u\left(k\right)+2e^T\left(k\right)PB\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{\omega }}_{ts}\left(k\right)+\tau {\widehat{\omega }}_{bs}\left(k\right)\right]\hfill \\ & -2e^T\left(k\right)PB\eta {\omega }_s\left(k\right)+\sum _{h=1}^m\frac{2}{c_h}{\tilde{\rho }}_h\delta {\widehat{\rho }}_h+T\sum _{h=1}^m\frac{1}{c_h}{\delta }^2{\widehat{\rho }}_h+\sum _{h=1}^m\frac{2}{g_{h1}}{\tilde{\eta }}_h\delta {\tilde{\eta }}_h+T\sum _{h=1}^m\frac{1}{g_{h1}}{\delta }^2{\tilde{\eta }}_h\hfill \\ & +\sum _{h=1}^m\frac{2}{g_{h2}}{\eta }_h\left(1-{\tau }_h\right){\tilde{d}}_{ts}\delta {\tilde{d}}_{ts}+T\sum _{h=1}^m\frac{1}{g_{h2}}{\eta }_h\left(1-{\tau }_h\right){\delta }^2{\tilde{d}}_{ts}+\sum _{h=1}^m\frac{2}{g_{h2}}{\eta }_h{\tau }_h{\tilde{d}}_{bs}\delta {\tilde{d}}_{bs}\hfill \\ & +T\sum _{h=1}^m\frac{1}{g_{h2}}{\eta }_h{\tau }_h{\delta }^2{\tilde{d}}_{bs}+2T{e}^T\left(k\right)P\delta e\left(k\right)+e^T\left(k\right)Pe\left(k\right)\hfill \\ & +T^2{\delta }^T\left(e\left(k\right)\right)P\delta \left(e\left(k\right)\right)+T{\delta }^T\left(e\left(k\right)\right)P\delta \left(e\left(k\right)\right)-\gamma {∥s_e\left(k\right)∥}_2.\hfill \end{array}$$

(46)

It should be noticed that, if we select a small enough sampling interval T, the terms containing T are able to be suppressed, and the expression becomes:

$$\begin{array}{cc}\hfill \delta \left(V\left(k\right)\right)\le & e^T\left(k\right)\left[P\left(A-LC\right)+{\left(A-LC\right)}^{T}P\right]e\left(k\right)-2e^T\left(k\right)PB\eta {\omega }_s\left(k\right)\hfill \\ & +2e^T\left(k\right)PB\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{\omega }}_{ts}\left(k\right)+\tau {\widehat{\omega }}_{bs}\left(k\right)\right]+2\sum _{h=1}^m{s}_{eh}^T\left(k\right){\tilde{\rho }}_h\left(k\right)u_h\left(k\right)+\sum _{h=1}^m\frac{2}{g_{h1}}{\tilde{\eta }}_h\delta {\tilde{\eta }}_h\hfill \\ & +\sum _{h=1}^m\frac{2}{c_h}{\tilde{\rho }}_h\left(k\right)\delta {\widehat{\rho }}_h\left(k\right)+\sum _{h=1}^m\frac{2}{g_{h2}}{\eta }_h\left(1-{\tau }_h\right){\tilde{d}}_{ts}\delta {\tilde{d}}_{ts}+\sum _{h=1}^m\frac{2}{g_{h2}}{\eta }_h{\tau }_h{\tilde{d}}_{bs}\delta {\tilde{d}}_{bs}-\gamma {∥s_e\left(k\right)∥}_2.\hfill \end{array}$$

(47)

Let $\delta {\widehat{\rho }}_h\left(k\right)=-c_h{s}_{eh}\left(k\right)u_h\left(k\right)$, then, it can be obtained that

$$2\sum _{h=1}^m{s}_{eh}^T\left(k\right){\rho }_h\left(k\right)u_h\left(k\right)+\sum _{h=1}^m\frac{2}{c_h}{\tilde{\rho }}_h\left(k\right)\delta {\widehat{\rho }}_h\left(k\right)=0.$$

Note that

$$x^T\left(k\right)PB\sum _{h=1}^m{\eta }_h{\omega }_{sh}\le x^T\left(k\right)PB\sum _{h=1}^m{\eta }_h\left[\left(I-{\tau }_h\right){\omega }_{tsh}+{\tau }_l{\omega }_{bsh}\right],$$

define

$$x^T\left(k\right)PB\sum _{h=1}^m{\eta }_h{\omega }_{sh}=x^T\left(k\right)PB\sum _{h=1}^m{\eta }_h\left[\left(I-{\tau }_h\right){\omega }_{tsh}+{\tau }_h{\omega }_{bsh}\right]-\phi {∥s_e\left(k\right)∥}_2.$$

The estimation of ${\eta }_h$, which is ${\widehat{\eta }}_h$ and can be adjusted according to the adaptive laws:

$$\delta {\widehat{\eta }}_h=g_{h1}{x}^T\left(k\right)P{b}_h\left[\left(I-{\tau }_h\right){\widehat{\omega }}_{ts}+{\tau }_h{\widehat{\omega }}_{bs}\right].$$

(48)

In addition, ${\widehat{\omega }}_{ts}$ and ${\widehat{\omega }}_{bs}$ are updated by the adaptive laws:

$$\delta {\widehat{\omega }}_{ts}=\delta {\widehat{\omega }}_{bs}=-g_{h2}{x}^T\left(k\right)P{b}_h,$$

(49)

then, the formulation of $\delta \left(V\left(k\right)\right)$ will be rewritten as:

$$\delta \left(V\left(k\right)\right)\le e^T\left(k\right)\left[P\left(A-LC\right)+{\left(A-LC\right)}^{T}P\right]e\left(k\right)-\gamma {∥s_e\left(k\right)∥}_2+\phi {∥s_e\left(k\right)∥}_2.$$

(50)

Choose appropriate constants $\gamma$ and $\phi$ satisfying $\gamma >\phi$. Then, the stability condition will be obtained:

$$\delta \left(V\left(k\right)\right)<e^T\left(k\right)\left(P\left(A-LC\right)+{\left(A-LC\right)}^{T}P\right)e\left(k\right)<0.$$

(51)

It is obvious that $\delta \left(V\left(k\right)\right)<0$ is correct if the matrix inequality in Equation (38) holds, which completes the proof. ☐

5. Section Stabilization of the Overall Closed-Loop Systems

The overall closed-loop system is described as follows:

$$\{\begin{array}{cc}\delta \widehat{x}\left(k\right)=\hfill & A\widehat{x}\left(k\right)+B{\widehat{\rho }}_{k}u\left(k\right)+B{u}_s\left(k\right)-LCe\left(k\right)+B\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{\omega }}_{ts}\left(k\right)+\tau {\widehat{\omega }}_{bs}\left(k\right)\right]\hfill \\ \delta e\left(k\right)=\hfill & \left(A-LC\right)e\left(k\right)+B\left(u_s\left(k\right)+\tilde{\rho }{u}_k-f\left(x_k,k\right)\right)-B\eta {\omega }_s\left(k\right)\hfill \\ & +B\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{\omega }}_{ts}\left(k\right)+\tau {\widehat{d}}_{bs}\left(k\right)\right],\hfill \end{array}$$

(52)

then, the sliding surface $\widehat{s}\left(k\right)$ will be written as:

$$\widehat{s}\left(k\right)=F\widehat{x}\left(k\right)-\sum _{q=0}^{kT-T}TF\left(A+BK\widehat{\rho }\left(q\right)\right)\widehat{x}\left(q\right)+\left(A\left(0\right)+B\left(0\right)K\left(0\right)\widehat{\rho }\left(0\right)\right)\widehat{x}\left(0\right),$$

(53)

where F is designed such that $FB$ is non-singular, and $K\in R^{m\times n}$ is designed to meet that $A+BK\widehat{\rho }\left(q\right)$ is Hurwitz. It can be seen that:

$$\delta \left(\widehat{s}\left(k\right)\right)=F\delta \widehat{x}\left(k\right)-\sum _{q=T}^{kT-T}TF\delta \left(\left(A+BK\widehat{\rho }\left(q\right)\right)\widehat{x}\left(q\right)\right).$$

(54)

For $q=kT-T$, it can be obtained that

$$\begin{array}{c}\hfill \frac{T\left(A\left(r_{kT-T+T}\right)+B\left(r_{kT-T+T}\right)K\left(r_{kT-T+T}\right)\widehat{\rho }\left(r_{kT-T+T}\right)\right)\widehat{x}\left(kT-T+T\right)}{T}\\ \hfill -\frac{T\left(A\left(r_{kT-T}\right)+B\left(r_{kT-T}\right)K\left(r_{kT-T}\right)\widehat{\rho }\left(r_{kT-T}\right)\right)\widehat{x}\left(kT-T\right)}{T}.\end{array}$$

(55)

For $q=kT-T-T=\left(k-2\right)T$, it is calculated that

$$\begin{array}{c}\hfill \frac{T\left(A\left(r_{kT-T}\right)+B\left(r_{kT-T}\right)K\left(r_{kT-T}\right)\widehat{\rho }\left(r_{kT-T}\right)\right)\widehat{x}\left(kT-T\right)}{T}\\ \hfill -\frac{T\left(A\left(r_{kT-2T}\right)+B\left(r_{kT-2T}\right)K\left(r_{kT-2T}\right)\widehat{\rho }\left(r_{kT-2T}\right)\right)\widehat{x}\left(kT-2T\right)}{T}.\end{array}$$

(56)

Thus, it is easy to derive that:

$$\delta \widehat{s}\left(k\right)=F\delta \widehat{x}\left(k\right)-F\left(A+BK\widehat{\rho }\left(k\right)\right)\widehat{x}\left(k\right).$$

(57)

According to the definition of $\delta \widehat{s}\left(k\right)$, it can be derived that:

$$\begin{array}{ccc}\hfill \delta \widehat{s}\left(k\right)& \hfill =& F\left(A\widehat{x}\left(k\right)+B\widehat{\rho }\left(k\right)u\left(k\right)+B{u}_s\left(k\right)+L\left(y\left(k\right)-C\widehat{x}\left(k\right)\right)\right)-F\left(A+BK\widehat{\rho }\left(k\right)\right)\widehat{x}\left(k\right)\hfill \\ & & +FB\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{\omega }}_{ts}\left(k\right)+\tau {\widehat{d}}_{bs}\left(k\right)\right]\hfill \\ & \hfill =& FA\widehat{x}\left(k\right)+FB\widehat{\rho }\left(k\right)u\left(k\right)+FB{u}_s\left(k\right)+FL\left(y\left(k\right)-C\widehat{x}\left(k\right)\right)-FA\widehat{x}\left(k\right)-FBK\widehat{\rho }\left(k\right)\widehat{x}\left(k\right)\hfill \\ & & +FB\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{\omega }}_{ts}\left(k\right)+\tau {\widehat{d}}_{bs}\left(k\right)\right]\hfill \\ & \hfill =& FB\widehat{\rho }\left(k\right)u\left(k\right)+FB{u}_s\left(k\right)+FL\left(y\left(k\right)-C\widehat{x}\left(k\right)\right)-FBK\widehat{\rho }\left(k\right)\widehat{x}\left(k\right)\hfill \\ & & +FB\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{\omega }}_{ts}\left(k\right)+\tau {\widehat{\omega }}_{bs}\left(k\right)\right].\hfill \end{array}$$

(58)

If $\delta \widehat{s}\left(k\right)=0$, it can be obtained that:

$$FB\widehat{\rho }\left(k\right)u\left(k\right)=-FB{u}_s\left(k\right)+FLCe\left(k\right)+FBK\widehat{\rho }\left(k\right)\widehat{x}\left(k\right)-FB\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{\omega }}_{ts}\left(k\right)+\tau {\widehat{d}}_{bs}\left(k\right)\right].$$

(59)

Therefore, the equivalent control law $u_{eq}\left(k\right)$ can be written as:

$$\begin{array}{ccc}\hfill u_{eq}\left(k\right)& \hfill =& \widehat{\rho }{\left(k\right)}^{-1}{\left(FB\right)}^{-1}\left(-FB{u}_s\left(k\right)+FLCe\left(k\right)+FBK\widehat{\rho }\left(k\right)\widehat{x}\left(k\right)-FB\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{\omega }}_{ts}\left(k\right)\right.\right.\hfill \\ & & \left.\left.+\tau {\widehat{\omega }}_{bs}\left(k\right)\right]\right)\hfill \\ & \hfill =& \widehat{\rho }{\left(k\right)}^{-1}\left(-u_s\left(k\right)+{\left(FB\right)}^{-1}F{L}_{\mathrm{i}}Ce\left(k\right)+K\widehat{\rho }\left(k\right)\widehat{x}\left(k\right)-\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{\omega }}_{ts}\left(k\right)+\tau {\widehat{\omega }}_{bs}\left(k\right)\right]\right).\hfill \end{array}$$

(60)

Substituting Equation (60) into Equation (58), we can obtain:

$$\delta \widehat{x}\left(k\right)=\left(A+BK\widehat{\rho }\left(k\right)\right)\widehat{x}\left(k\right).$$

(61)

Based on the observer equation, the present objective is to design $u\left(k\right)$ to ensure that the closed-loop system is able to be driven onto the sliding surface $\widehat{s}\left(k\right)=0$ with probability 1 in finite time.

The $u\left(k\right)$ can be defined as

$$u\left(k\right)=-\widehat{\rho }{\left(k\right)}^{-1}{\left(FB\right)}^{-1}\zeta \widehat{s}\left(k\right)+u_{adv}\left(k\right)$$

(62)

with

$$\begin{array}{cc}\hfill u_{adv}\left(k\right)=& \left\{\begin{array}{cc}-\widehat{\rho }{\left(k\right)}^{-1}{\left(FB\right)}^{-1}\vartheta \left(k\right)\frac{\widehat{s}\left(k\right)}{∥\widehat{s}\left(k\right)∥},\hfill & \widehat{s}\left(k\right)>\epsilon ,\hfill \\ -\widehat{\rho }{\left(k\right)}^{-1}{\left(FB\right)}^{-1}\vartheta \left(k\right)\frac{\widehat{s}\left(k\right)}{\epsilon },\hfill & \widehat{s}\left(k\right)\le \epsilon ,\hfill \end{array}\right.\hfill \\ \hfill \vartheta \left(k\right)=& ∥F∥∥B∥\left(\alpha ∥y\left(k\right)∥+\beta +\gamma \right)\sqrt{m}+∥F∥∥BK∥∥\widehat{\rho }\left(k\right)∥∥\widehat{x}\left(k\right)∥\hfill \\ & +∥F∥∥B∥∥\widehat{\eta }∥\left(∥I-\tau ∥∥{\widehat{\omega }}_{ts}\left(k\right)∥+∥\tau ∥∥{\widehat{\omega }}_{bs}\left(k\right)∥\right)+∥F∥∥L∥∥Ce\left(k\right)∥.\hfill \end{array}$$

(63)

Theorem 3.

Supposing Inequalities (15) and (38) have solutions, the sliding surface is given by Equation (53). Then, the trajectory of delta operator system in Equation (52) can be driven onto the sliding surface in finite time with the following control law in Equation (62), and evolve in a neighborhood around the sliding surface, converging to a residual set at the origin in the end.

Proof. 

Considering $\widehat{s}\left(k\right)$, define a Lyapunov function $V_s\left(k\right)=\frac{1}{2}{\widehat{s}}^T\left(k\right)\widehat{s}\left(k\right)$,

$$\begin{array}{ccc}\hfill \delta V_s\left(k\right)& \hfill =& {\widehat{s}}^T\left(k\right)\delta \widehat{s}\left(k\right)+\frac{T}{2}\left[\delta {\widehat{s}}^T\left(k\right)\delta \widehat{s}\left(k\right)\right]\hfill \\ & \hfill =& {\widehat{s}}^T\left(k\right)\left(F\delta \widehat{x}\left(k\right)-F\left(A+BK\widehat{\rho }\left(k\right)\right)\widehat{x}\left(k\right)\right)+\frac{T}{2}\left[\delta {\widehat{s}}^T\left(k\right)\delta \widehat{s}\left(k\right)\right]\hfill \\ & \hfill =& {\widehat{s}}^T\left(k\right)F\delta \widehat{x}\left(k\right)-{\widehat{s}}^T\left(k\right)F\left(A+BK\widehat{\rho }\left(k\right)\right)\widehat{x}\left(k\right)+\frac{T}{2}\left[\delta {\widehat{s}}^T\left(k\right)\delta \widehat{s}\left(k\right)\right]\hfill \\ & \hfill =& {\widehat{s}}^T\left(k\right)F\left(A\widehat{x}\left(k\right)+B\widehat{\rho }\left(k\right)u\left(k\right)+B{u}_s\left(k\right)-LCe\left(k\right)+B\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{\omega }}_{ts}\left(k\right)+\tau {\widehat{\omega }}_{bs}\left(k\right)\right]\right)\hfill \\ & & -{\widehat{s}}^T\left(k\right)F\left(A+BK\widehat{\rho }\left(k\right)\right)\widehat{x}\left(k\right)+\frac{T}{2}\left[\delta {\widehat{s}}^T\left(k\right)\delta \widehat{s}\left(k\right)\right]\hfill \\ & \hfill =& {\widehat{s}}^T\left(k\right)FB\widehat{\rho }\left(k\right)u\left(k\right)+\frac{T}{2}\left[\delta {\widehat{s}}^T\left(k\right)\delta \widehat{s}\left(k\right)\right]+{\widehat{s}}^T\left(k\right)F\left(A\widehat{x}\left(k\right)+B{u}_s\left(k\right)-LCe\left(k\right)\right.\hfill \\ & & -\left.\left(A+BK\widehat{\rho }\left(k\right)\right)\widehat{x}\left(k\right)+B\widehat{\eta }\left(k\right)\left[\left(I-\tau \right){\widehat{\omega }}_{ts}\left(k\right)+\tau {\widehat{\omega }}_{bs}\left(k\right)\right]\right)\hfill \\ & \hfill \le & {\widehat{s}}^T\left(k\right)FB\widehat{\rho }\left(k\right)u\left(k\right)+∥\widehat{s}\left(k\right)∥∥F∥\left(∥B∥\left(\alpha ∥y\left(k\right)∥+\beta +\gamma \right)\sqrt{m}+∥L∥∥Ce\left(k\right)∥\right)\hfill \\ & & +∥\widehat{s}\left(k\right)∥∥F∥\left(∥BK∥∥\widehat{\rho }\left(k\right)∥∥\widehat{x}\left(k\right)∥+∥B∥∥\widehat{\eta }∥\left(∥I-\tau ∥∥{\widehat{\omega }}_{ts}\left(k\right)∥+∥\tau ∥∥{\widehat{\omega }}_{bs}\left(k\right)∥\right)\right)\hfill \\ & & +\frac{T}{2}\left[\delta {\widehat{s}}^T\left(k\right)\delta \widehat{s}\left(k\right)\right].\hfill \end{array}$$

(64)

It should be noticed that the following fact holds:

$$\begin{array}{cc}\hfill ∥\mathrm{sgn}\left(\widehat{s}\left(k\right)\right)∥& =∥{\left(\mathrm{sgn}{\left({\widehat{s}}_1\left(k\right)\right)}^T,\mathrm{sgn}{\left({\widehat{s}}_2\left(k\right)\right)}^T,\dots \mathrm{sgn}{\left({\widehat{s}}_m\left(k\right)\right)}^T\right)}^T∥\hfill \\ & =∥{\left({\left(\frac{{\widehat{s}}_1\left(k\right)}{\left|{\widehat{s}}_1\left(k\right)\right|}\right)}^T,{\left(\frac{{\widehat{s}}_2\left(k\right)}{\left|{\widehat{s}}_2\left(k\right)\right|}\right)}^T,\dots {\left(\frac{{\widehat{s}}_3\left(k\right)}{\left|{\widehat{s}}_3\left(k\right)\right|}\right)}^T\right)}^T∥\hfill \\ & =\sqrt{m}.\hfill \end{array}$$

(65)

We substitute Equations (62) and (63) into Equation (64), then $\delta V_s\left(k\right)$ will become:

$$\begin{array}{cc}\hfill \delta V_s\left(k\right)& \le -{\widehat{s}}^T\left(k\right)\zeta \widehat{s}\left(k\right)-{\widehat{s}}^T\left(k\right)FB{\widehat{\rho }}_k{\rho }_k^{-1}{\left(FB\right)}^{-1}\vartheta \left(k\right)\frac{\widehat{s}\left(k\right)}{∥\widehat{s}\left(k\right)∥}+∥\widehat{s}\left(k\right)∥\vartheta \left(k\right)+\frac{T}{2}\left[\delta {\widehat{s}}^T\left(k\right)\delta \widehat{s}\left(k\right)\right]\hfill \\ & \le -{\widehat{s}}^T\left(k\right)\gamma \widehat{s}\left(k\right)+{\widehat{s}}^T\left(k\right)\left(\gamma -\zeta \right)\widehat{s}\left(k\right)+\frac{T}{2}\left[\delta {\widehat{s}}^T\left(k\right)\delta \widehat{s}\left(k\right)\right].\hfill \end{array}$$

(66)

In addition, considering $\widehat{s}\left(k\right)\le \epsilon$ and the control law, there will exist

$$\begin{array}{c}\hfill \delta V_s\left(k\right)\le -{\widehat{s}}^T\left(k\right)\gamma \widehat{s}\left(k\right)+{\widehat{s}}^T\left(k\right)\left(\gamma -\zeta \right)\widehat{s}\left(k\right)+\frac{T}{2}\left[\delta {\widehat{s}}^T\left(k\right)\delta \widehat{s}\left(k\right)\right]+\frac{\epsilon }{4}.\end{array}$$

(67)

The term $\frac{T}{2}\left[\delta {\widehat{s}}^T\left(k\right)\delta \widehat{s}\left(k\right)\right]$ in the above inequality contains the parameter uncertainties and a properly selected $\zeta$ can suppress the uncertainty. If the parameter uncertainties are large, the sampling interval q should be selected small to guarantee that the term $\frac{T}{2}\left[\delta {\widehat{s}}^T\left(k\right)\delta \widehat{s}\left(k\right)\right]$ becomes small enough. Appropriately selecting parameter $\zeta$, it can be obtained that

$${\xi }_{max}\left({\left(F-F\left(A+BK\widehat{\rho }\left(k\right)\right)\right)}^T\left(\gamma -2\omega \right)\left(F-F\left(A+BK\widehat{\rho }\left(k\right)\right)\right)\right)<-\eta$$

where $\gamma$ and $\eta$ are proper positive scalars. We can derive that

$$\delta V_s\left(k\right)<-\gamma {\widehat{s}}^T\left(k\right)\widehat{s}\left(k\right)+\eta \left(\epsilon \right),$$

(68)

$$\eta \left(\epsilon \right)=\left\{\begin{array}{c}0,\widehat{s}\left(k\right)>\epsilon \hfill \\ \frac{\epsilon }{4},\widehat{s}\left(k\right)\le \epsilon .\end{array}\right.$$

(69)

The proof is completed. ☐

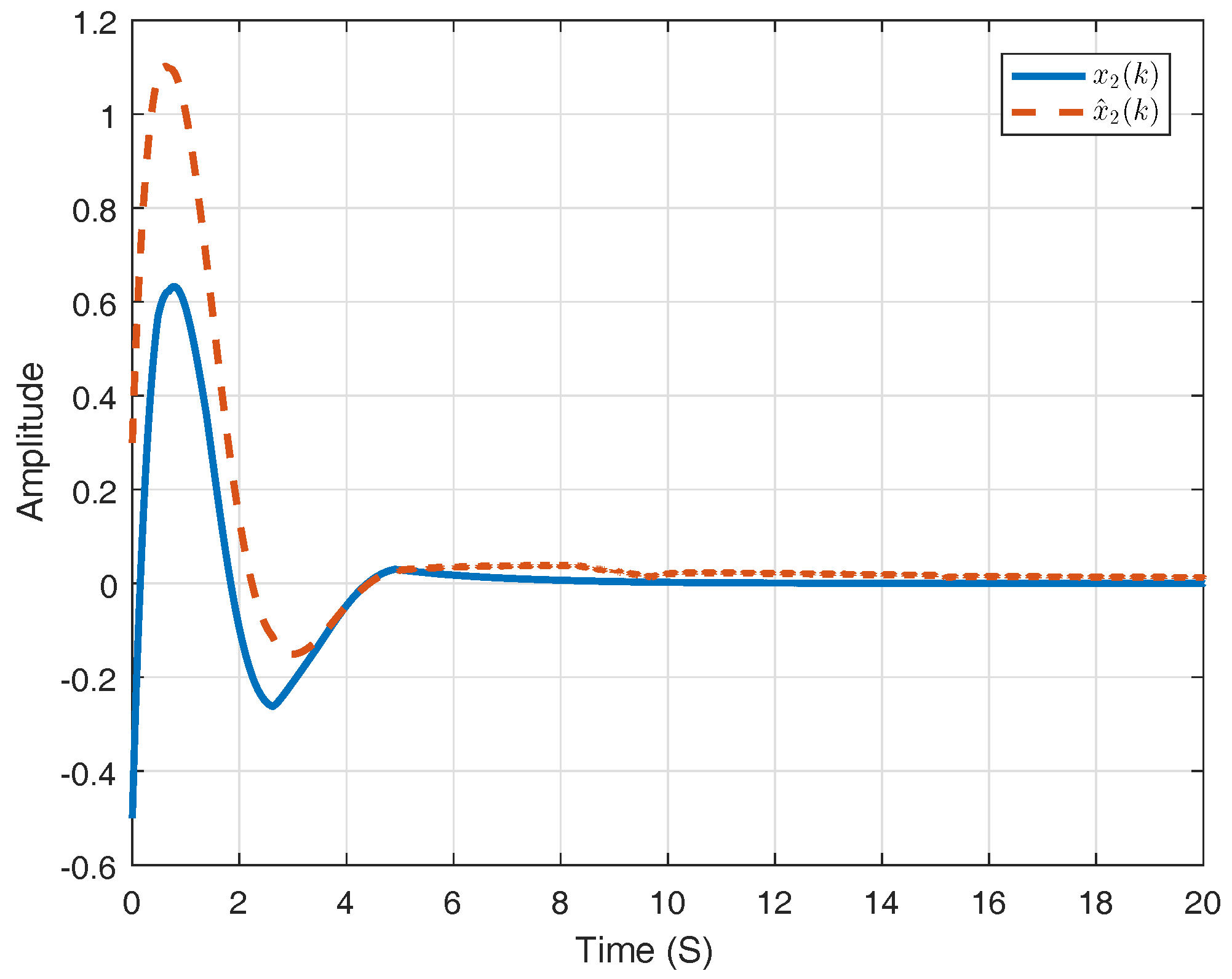
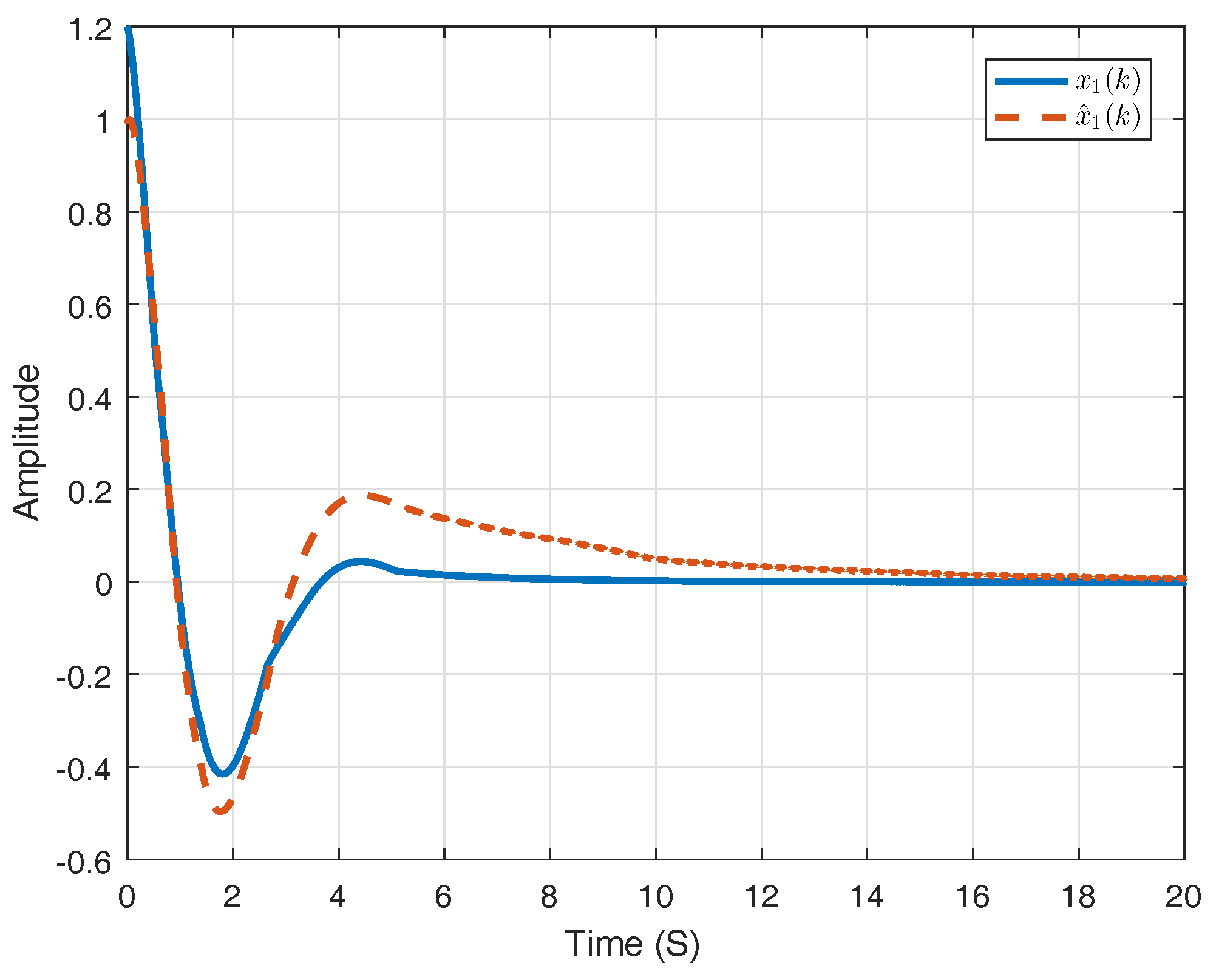
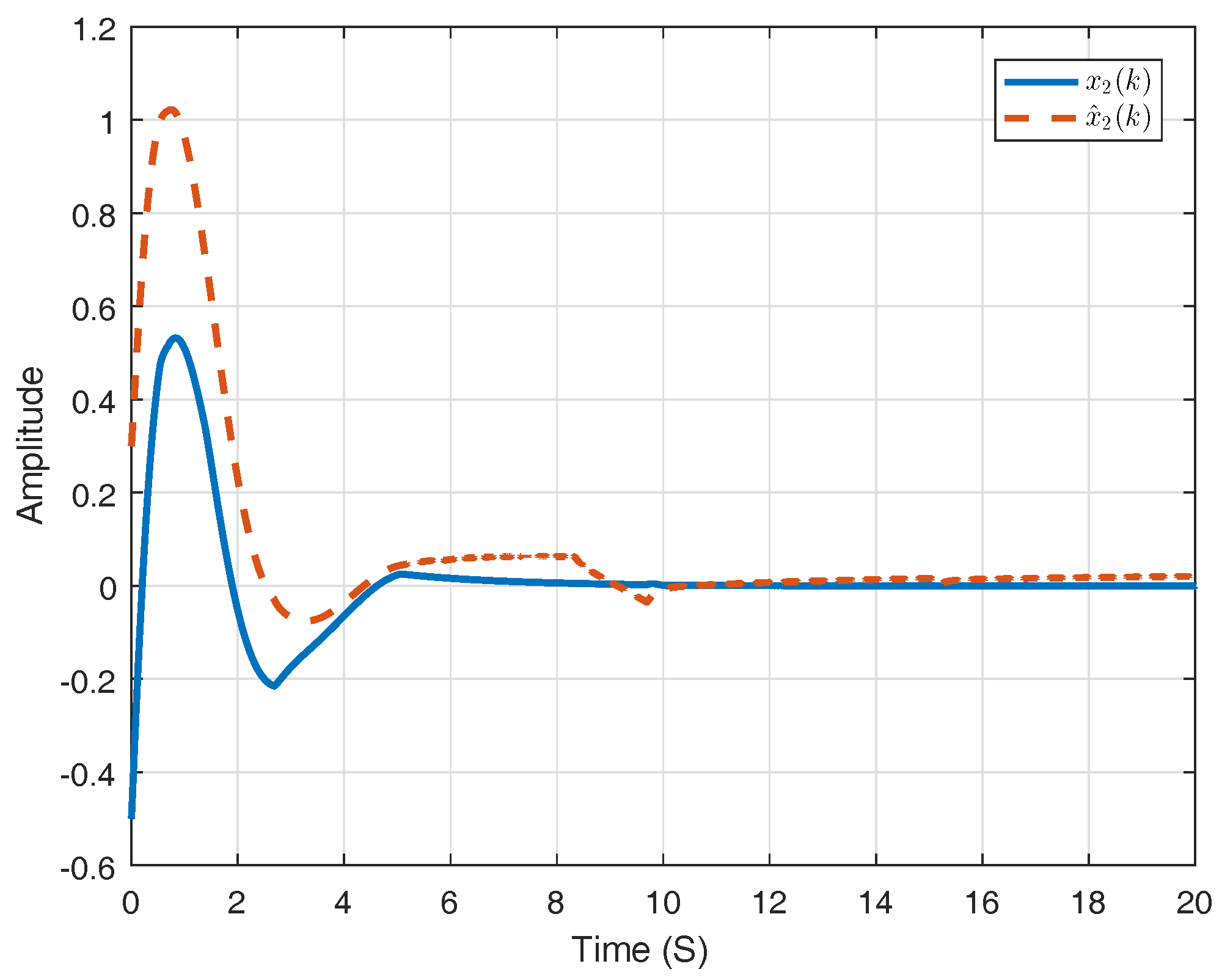
6. Numerical Example

We provide an example to prove the validity of the results mentioned above in this section. The proposed method will be applied to design a robust sliding mode controller for the simplified truck-trailer system, which was proposed as in [25], and the system associated with delta operator is described as

$$\delta x\left(k\right)=Ax\left(k\right)+B\left[u_{hl}^F\left(k\right)+f\left(x\left(k\right),k\right)\right]$$

with

$$A=\left[\begin{array}{cc}-0.7734& -0.6691\\ 1.3382& -1.4425\end{array}\right], B=\left[\begin{array}{cc}-0.1828& -0.2360\\ 1.0629& -0.9260\end{array}\right].$$

Define the sampling period as $T=0.001$ and the actuator efficiency value as $\rho =0.75$. The original state $x\left(0\right)$ of system can be chosen as ${\left[\begin{array}{cc}2& -0.5\end{array}\right]}^T$, $\zeta =0.6$.

After solving matrix constraint in Equation (15), the following solution can be obtained that:

$$P=\left[\begin{array}{cc}0.4132& 0.2139\\ 0.2139& 0.6069\end{array}\right], L=\left[\begin{array}{c}2.4745\\ 0.6889\end{array}\right], {\alpha }_1=0.25.$$

Figure 1 shows the sliding surface applied in the $\delta$-domain, which can be denoted by $\widehat{s}\left(k\right)$. Considering state variable $x\left(k\right)$, Figure 2 and Figure 3 compare the corresponding state trajectories of system and its observer. It is easy to conclude that the system is stochastically stable. Figure 4 and Figure 5 compare the current results with the consequences gotten by the methods in previous work.

7. Conclusions

In this paper, we have investigated delta operator method to research the adaptive sliding mode control for high-frequency sampled-data systems with actuator faults. A novel observer-based sliding mode control method is proposed to deal with the problem. In future work, we will pay attention to the situation in which is influenced by network-induced communication delay and data packet losses are taken into account, simultaneously. In the future, we will focus on the combination of delta operator with semi-Markov systems, switched positive system, etc. and consider the influence of dead-zone or saturation to the overall system.